A Method for the Ambient Equivalent Dose Estimation in a Wide Range of Altitudes during SEP and GLE Events
Abstract
1. Introduction
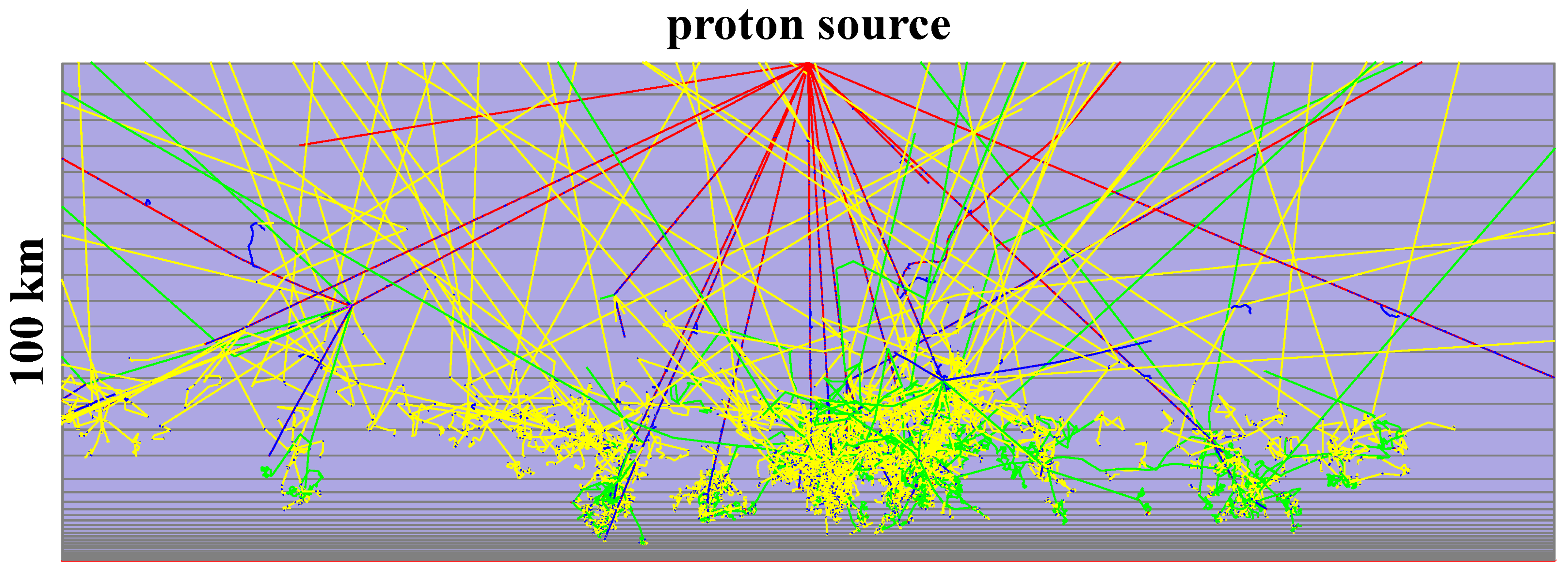
2. Data and Methods
3. Results and Discussions
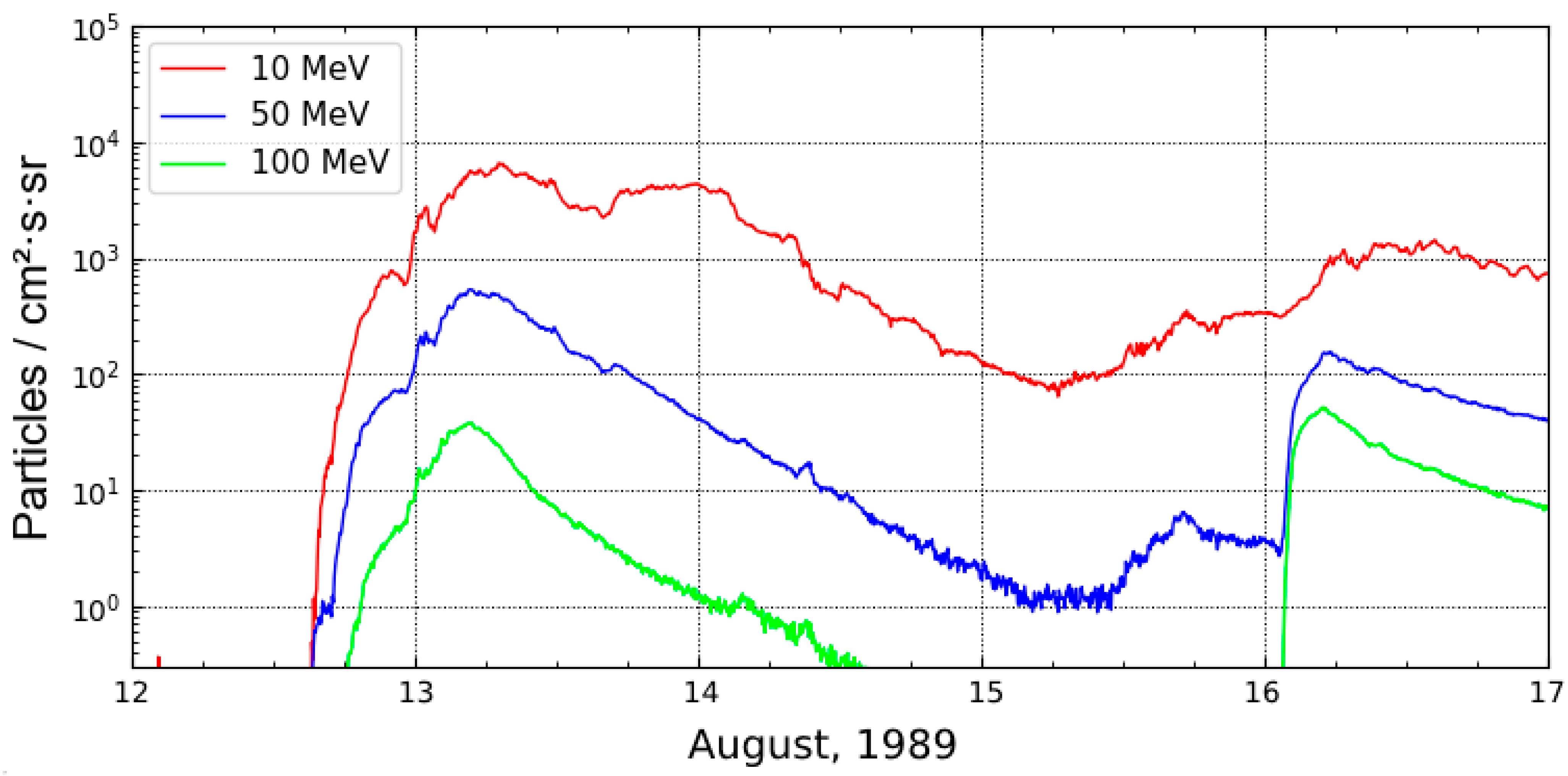
3.1. SEP on 12 August 1989
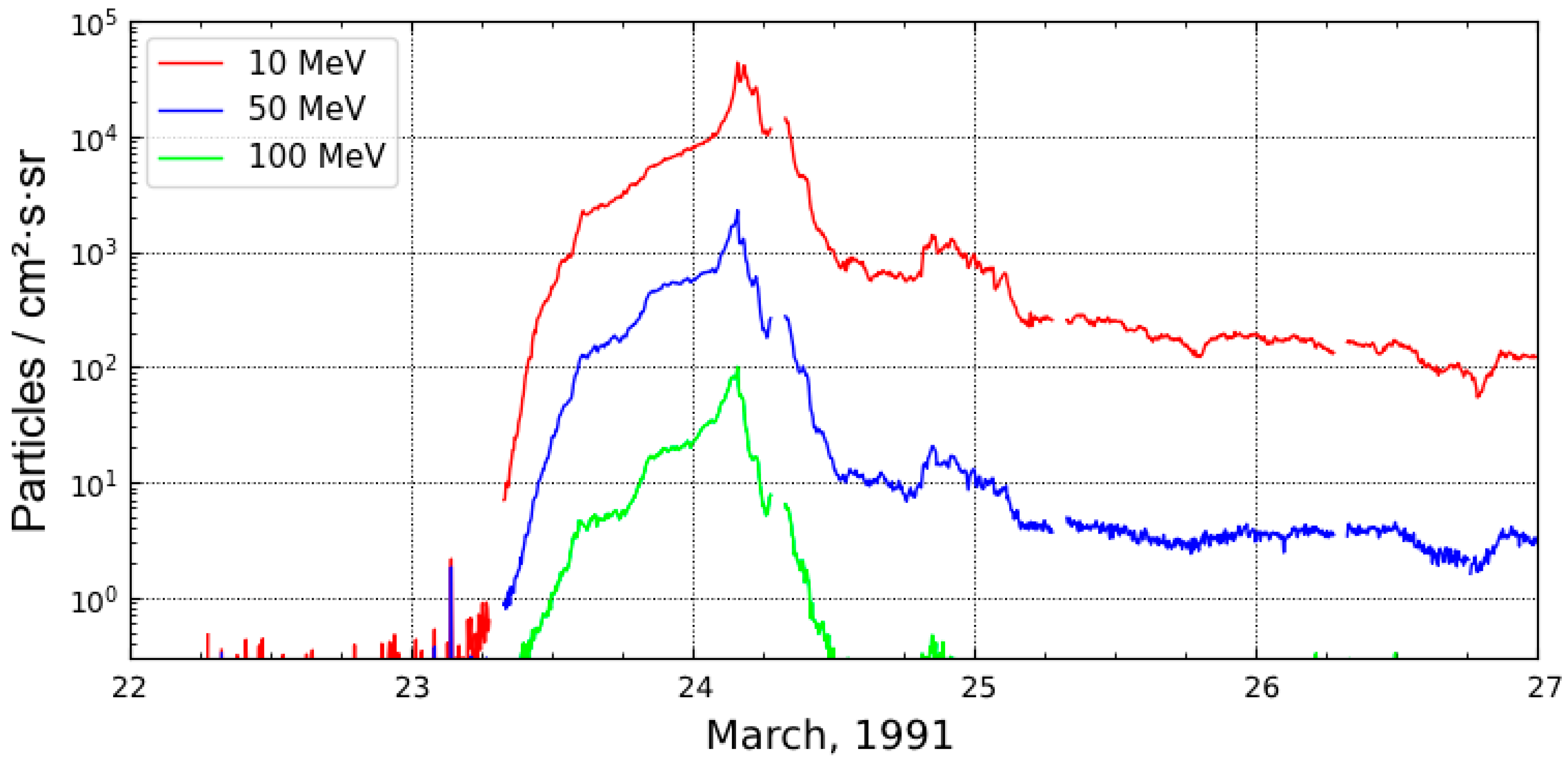
3.2. SEP on 23 March 1991
3.3. SEP on 9 November 2000
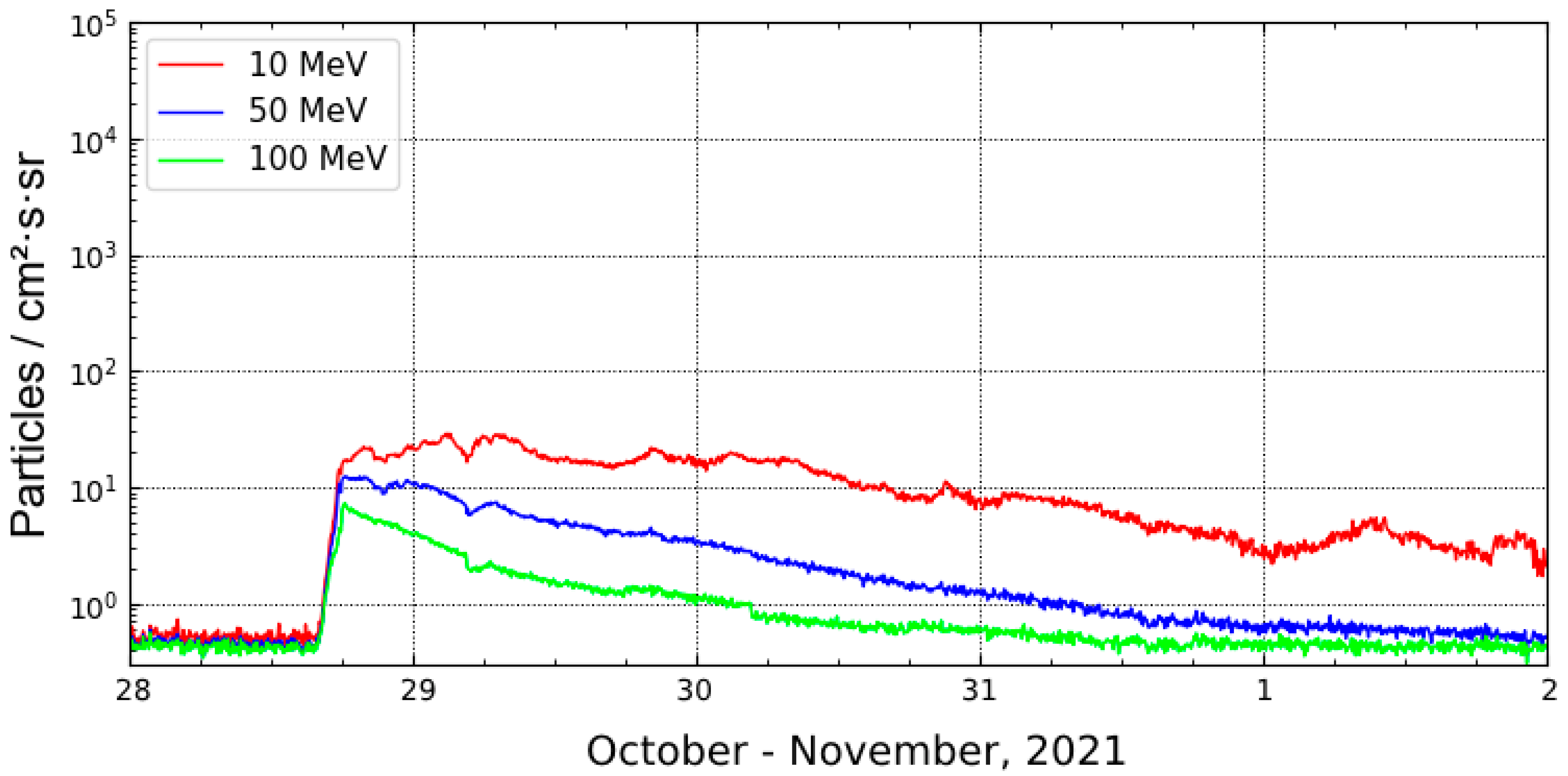
3.4. GLE 73 on 28 October 2021
3.5. Primary Protons Differential Energy Spectra
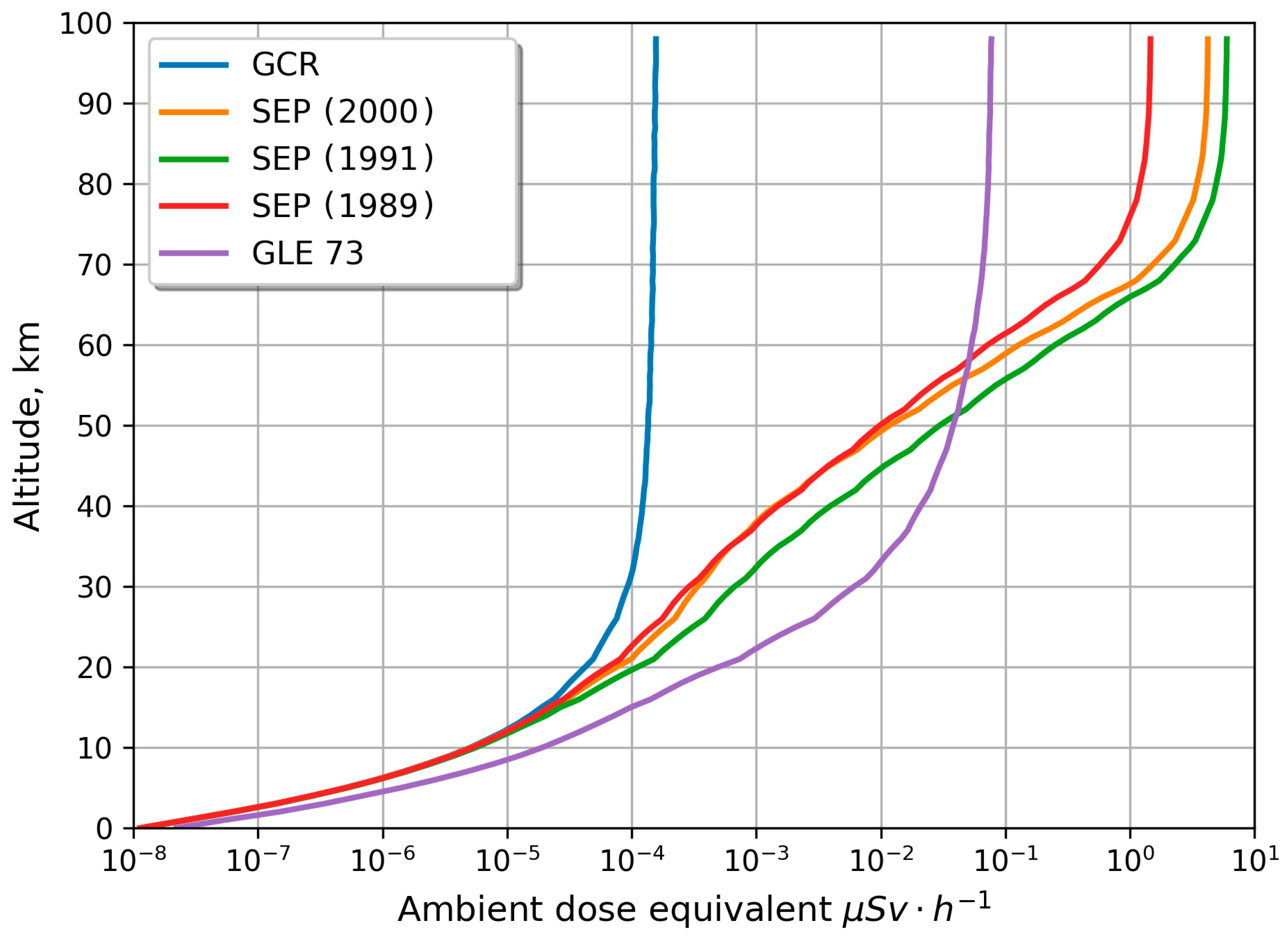
3.6. Secondary Particles Energy Spectra and the Ambient Dose Equivalent
4. Discussion
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Dorman, L. Cosmic Ray Interactions, Propagation, and Acceleration in Space Plasmas; Springer: Dordrecht, The Netherlands, 2006; 847p. [Google Scholar]
- Adriani, O.; Barbarino, G.C.; Bazilevskaya, G.A. PAMELA Measurements of Cosmic–Ray Proton and Helium Spectra. Science 2011, 332, 69–72. [Google Scholar] [CrossRef] [PubMed]
- Adriani, O.; Barbarino, G.C.; Bazilevskaya, G.A. Time dependence of the proton flux measured by PAMELA during 2006 July–2009 December solar minimum. Astrophys. J. 2013, 765, 91. [Google Scholar] [CrossRef]
- Aguilar, M.; Cavasonza, L.A.; Alpat, B.; Ambrosi, G.; Arruda, L.; Attig, N.; Aupetit, S.; Azzarello, P.; Bachlechner, A.; Barao, F.; et al. Observation of Fine Time Structures in the Cosmic Proton and Helium Fluxes with the Alpha Magnetic Spectrometer on the International Space Station. Phys. Rev. Lett. 2018, 121, 051101. [Google Scholar] [CrossRef]
- Martucci, M.; Ammendola, R.; Badoni, D.; Bartocci, S.; Battiston, R.; Beolè, S.; Burger, W.J.; Campana, D.; Castellini, G.; Cipollone, P.; et al. Time Dependence of 50–250 MeV Galactic Cosmic-Ray Protons between Solar Cycles 24 and 25, Measured by the High-energy Particle Detector on board the CSES-01 Satellite. Astrophys. J. Lett. 2023, 945, L39. [Google Scholar] [CrossRef]
- Mishev, A.; Usoskin, I.G. Numerical model for computation of effective and ambient dose equivalent at flight altitudes: Application for dose assessment during GLEs. J. Space Weather Space Clim. 2015, 5, A10. [Google Scholar] [CrossRef]
- Mishev, A.L.; Adibpour, F.; Usoskin, I.G.; Felsberger, E. Computation of dose rate at flight altitudes during ground level enhancements no. 69, 70 and 71. Adv. Space Res. 2015, 55, 354–362. [Google Scholar] [CrossRef]
- Mishev, A.L.; Kocharov, L.G.; Koldobskiy, S.A. High-Resolution Spectral and Anisotropy Characteristics of Solar Protons during the GLE N◦73 on 28 October 2021 Derived with Neutron-Monitor Data Analysis. Sol. Phys. 2022, 297, 88. [Google Scholar] [CrossRef]
- Kocharov, L.; Mishev, A.; Riihonen, E.; Vainio, R.; Usoskin, I. A Comparative Study of Ground-level Enhancement Events of Solar Energetic Particles. Astrophys. J. 2023, 958, 122. [Google Scholar] [CrossRef]
- Belov, A.V. Flares, ejections, proton events. Geomagn. Aeron. 2017, 57, 727–737. [Google Scholar] [CrossRef]
- Matzka, J.; Stolle, C.; Yamazaki, Y.; Bronkalla, O.; Morschhauser, A. The geomagnetic Kp index and derived indices of geomagnetic activity. Space Weather 2021, 19, e2020SW002641. [Google Scholar] [CrossRef]
- Agostinelli, S.; Allison, J.; Amako, K.; Apostolakis, J.; Araujo, H.; Arce, P.; Asai, M.; Axen, D.; Banerjee, S.; Barrand, G.; et al. GEANT4-a simulation toolkit. Nucl. Instrum. Methods Phys. Res. Sect. A 2003, 506, 250–303. [Google Scholar] [CrossRef]
- Allison, J.; Amak, K.; Apostolakis, J.; Arce, P.; Asai, M.; Aso, T.; Bagli, E.; Bagulya, A.; Banerjee, S.; Barrand, G.; et al. Recent developments in Geant4. Nucl. Instrum. Methods Phys. Res. Sect. A 2016, 835, 186–225. [Google Scholar] [CrossRef]
- Maurchev, E.A.; Balabin, Y.V. RUSCOSMICS—The new software toolbox for detailed analysis of cosmic ray interactions with matter. Sol. Terr. Phys. 2016, 2, 3–10. [Google Scholar]
- Picone, J.M.; Hedin, A.E.; Drob, D.P.; Aikin, A.C. NRLMSISE-00 empirical model of the atmosphere: Statistical comparison and scientific issues. J. Geophys. Res. 2002, 107, 1468. [Google Scholar] [CrossRef]
- Truscott, P.; Heynderickx, D.; Nartallo, R.; Lei, F.; Sicard-Piet, A. Application of PLANETOCOSMICS to Simulate the Radiation Environment at the Galilean Moons. EPSC Abstr. 2010, 5, EPSC2010-808. [Google Scholar]
- Maurchev, E.A.; Balabin, Y.V.; Germanenko, A.V. Compact Geiger Counters as Additional Tools for Verifying Models of Cosmic Ray Transport through the Earth’s Atmosphere. Bull. Russ. Acad. Sci. Phys. 2021, 85, 1294–1296. [Google Scholar] [CrossRef]
- Heikkinen, A.; Stepanov, N.; Wellish, J.P. Bertini intra–nuclear cascade implementation in Geant4. In Proceedings of the 13th International Conference on Computing in High-Enery and Nuclear Physics (CHEP 2003), La Jolla, CA, USA, 24–28 March 2003. [Google Scholar]
- Amelin, N.S.; Armesto, N. Monte Carlo model for nuclear collisions from SPS to LHC energies. Eur. Phys. J. C Part. Fields 2001, 22, 149–163. [Google Scholar] [CrossRef]
- Amelin, N.S.; Gudima, K.K.; Toneev, V.D. Quark–Gluon String Model and Ultrarelativistic Heavy Ion Interactions. Sov. J. Nucl. Phys. 1990, 51, 327–333. [Google Scholar]
- Garny, S.; Leuthold, G.; Mares, V.; Paretzke, H.G.; Ruhm, W. GEANT4 Transport Calculations for Neutrons and Photons below 15 MeV. IEEE Trans. Nucl. Sci. 2009, 56, 2392–2396. [Google Scholar] [CrossRef]
- Vashenyuk, E.V.; Balabin, Y.V.; Gvozdevsky, B.B. Features of relativistic solar proton spectra derived from ground level enhancement events (GLE) modeling. Astrophys. Space Sci. Trans. 2011, 7, 459–463. [Google Scholar] [CrossRef]
- Balabin, Y.V.; Gvozdevsky, B.B.; Germanenko, A.V.; Maurchev, E.A.; Michalko, E.A. GLE73 Event (October 28, 2021) in Solar Cosmic Rays. Bull. Russ. Acad. Sci. Phys. 2022, 86, 1542–1548. [Google Scholar] [CrossRef]
- Kellerer, A.M. Weighting factors for radiation quality: How to unite the two current concepts. Radiat. Prot. Dosim. 2004, 110, 781–787. [Google Scholar] [CrossRef] [PubMed]
- Belov, A.; Garcia, H.; Kurt, V.; Mavromichalaki, H.; Gerontidou, M. Proton Enhancements and Their Relation to the X-ray Flares During the Three Last Solar Cycles. Sol. Phys. 2005, 229, 135–159. [Google Scholar] [CrossRef]
- Cliver, E.W.; Ling, A.G.; Belov, A.; Yashiro, S. Size distributions of solar flares and solar energetic particle events. Astrophys. J. Lett. 2012, 756, L29. [Google Scholar] [CrossRef]
- Dierckxsens, M.; Tziotziou, K.; Dalla, S.; Patsou, I.; Marsh, M.S.; Crosby, N.B.; Malandraki, O.; Tsiropoula, G. Relationship between Solar Energetic Particles and Properties of Flares and CMEs: Statistical Analysis of Solar Cycle 23 Events. Sol. Phys. 2015, 290, 841–874. [Google Scholar] [CrossRef]
- Bazilevskaya, G.A.; Sladkova, A.I.; Svirzhevskaya, A.K. Features of the solar X-ray bursts related to solar energetic particle events. Adv. Space Res. 2006, 37, 1421–1425. [Google Scholar] [CrossRef]
- Kahler, S.W. The correlation between solar energetic particle peak intensities and speeds of coronal mass ejections: Effects of ambient particle intensities and energy spectra. J. Geophys. Res. Space Phys. 2001, 106, 20947–20956. [Google Scholar] [CrossRef]
- Gopalswamy, N.; Makela, P.; Akiyama, S.; Yashiro, S.; Xie, H.; Thakur, N.; Kahler, S.W. Large Solar Energetic Particle Events Associated with Filament Eruptions Outside of Active Regions. Astrophys. J. Lett. 2015, 806, 8. [Google Scholar] [CrossRef]
- Miteva, R.; Klein, K.L.; Malandraki, O.; Dorrian, G. Solar Energetic Particle Events in the 23rd Solar Cycle: Interplanetary Magnetic Field Configuration and Statistical Relationship with Flares and CMEs. Sol. Phys. 2013, 282, 579–613. [Google Scholar] [CrossRef][Green Version]
- Richardson, I.G.; Cane, H.V.; Von Rosenvinge, T.T. Prompt Arrival of Solar Energetic Particles from Far Eastern Events: The Role of Large-Scale Interplanetary Magnetic Field Structure. J. Geophys. Res. Space Phys. 1991, 96, 7853–7860. [Google Scholar] [CrossRef]
- Lario, D.; Aran, A.; Gómez-Herrero, R.; Dresing, N.; Heber, B.; Ho, G.C.; Decker, R.B.; Roelof, E.C. Longitudinal and Radial Dependence of Solar Energetic Particle Peak Intensities: STEREO, ACE, SOHO, GOES, and MESSENGER Observations. Astrophys. J. 2013, 767, 41. [Google Scholar] [CrossRef]
- Cohen, C.M.S.; Mason, G.M.; Mewaldt, R.A. Characteristics of Solar Energetic Ions as a Function of Longitude. Astrophys. J. 2017, 843, 132. [Google Scholar] [CrossRef]
- Zhang, J.; Temmer, M.; Gopalswamy, N.; Malandraki, O.; Nitta, N.V.; Patsourakos, S.; Shen, F.; Vršnak, B.; Wang, Y.; Webb, D.; et al. Earth-affecting solar transients: A review of progresses in solar cycle 24. Prog. Earth Planet. Sci. 2021, 8, 56. [Google Scholar] [CrossRef] [PubMed]
- El-Borie, M.A. Major solar-energetic particle fluxes: I. Comparison with the associated ground level enhancements of cosmic rays. Astropart. Phys. 2003, 19, 549–558. [Google Scholar] [CrossRef]
- Sladkova, A.I.; Bazilevskaya, G.A.; Ishkov, V.N.; Nazarova, M.N.; Pereyaslova, N.K.; Stupishin, A.G.; Ulyev, V.A.; Chertok, I.M. Catalog of Solar Proton Events 1987–1997; Logachev, Y.I., Ed.; Moscow University Press: Moscow, Russia, 1998; 246p, Available online: http://www.wdcb.ru/stp/data/SPE/SPE_1987-1996.pdf (accessed on 8 December 2023).
- Logachev, Y.I.; Bazilevskaya, G.A.; Vashenyuk, E.V.; Daibog, E.I.; Ishkov, V.N.; Lazutin, L.L.; Miroshnichenko, L.I.; Nazarova, M.N.; Petrenko, I.E.; Stupishin, A.G.; et al. Catalog of Solar Proton Events in the 23rd Cycle of Solar Activity (1996–2008). 2016. Available online: http://www.wdcb.ru/stp/data/SPE/SPE_1997-2009/Catalog_SPE_23_cycle_SA.pdf (accessed on 8 December 2023).
- Papaioannou, A.; Kouloumvakos, A.; Mishev, A.; Vainio, R.; Usoskin, I.; Herbst, K.; Rouillard, A.P.; Anastasiadis, A.; Gieseler, J.; Wimmer-Schweingruber, R.; et al. The first ground-level enhancement of solar cycle 25 on 28 October 2021. Astron. Astrophys. 2022, 660, L5. [Google Scholar] [CrossRef]
- Mavromichalaki, H.; Paschalis, P.; Gerontidou, M.; Papailiou, M.-C.; Paouris, E.; Tezari, A.; Lingri, D.; Livada, M.; Stassinakis, A.N.; Crosby, N.; et al. The Updated Version of the A.Ne.Mo.S. GLE Alert System: The Case of the Ground-Level Enhancement GLE73 on 28 October 2021. Universe 2022, 8, 378. [Google Scholar] [CrossRef]
- Kirillov, A.S.; Belakhovsky, V.B.; Maurchev, E.A.; Balabin, Y.V.; Germanenko, A.V.; Gvozdevsky, B.B. Vibrational Kinetics of NO and N2 in the Earth’s Middle Atmosphere during GLE69 on January 20, 2005. JGR Atmos. 2003, 128, e2023JD038600. [Google Scholar] [CrossRef]
- Copeland, K.; Matthiä, D.; Meier, M.M. Solar cosmic ray dose rateassessments during GLE 72 using MIRA and PANDOCA. Space Weather 2018, 16, 969–976. [Google Scholar] [CrossRef]
- Matthiä, D.; Heber, B.; Reitz, G.; Sihver, L.; Berger, T.; Meier, M. The ground level event 70 on December 13th, 2006 and related effective doses at aviation altitudes. Radiat. Prot. Dosim. 2009, 136, 304–310. [Google Scholar] [CrossRef]

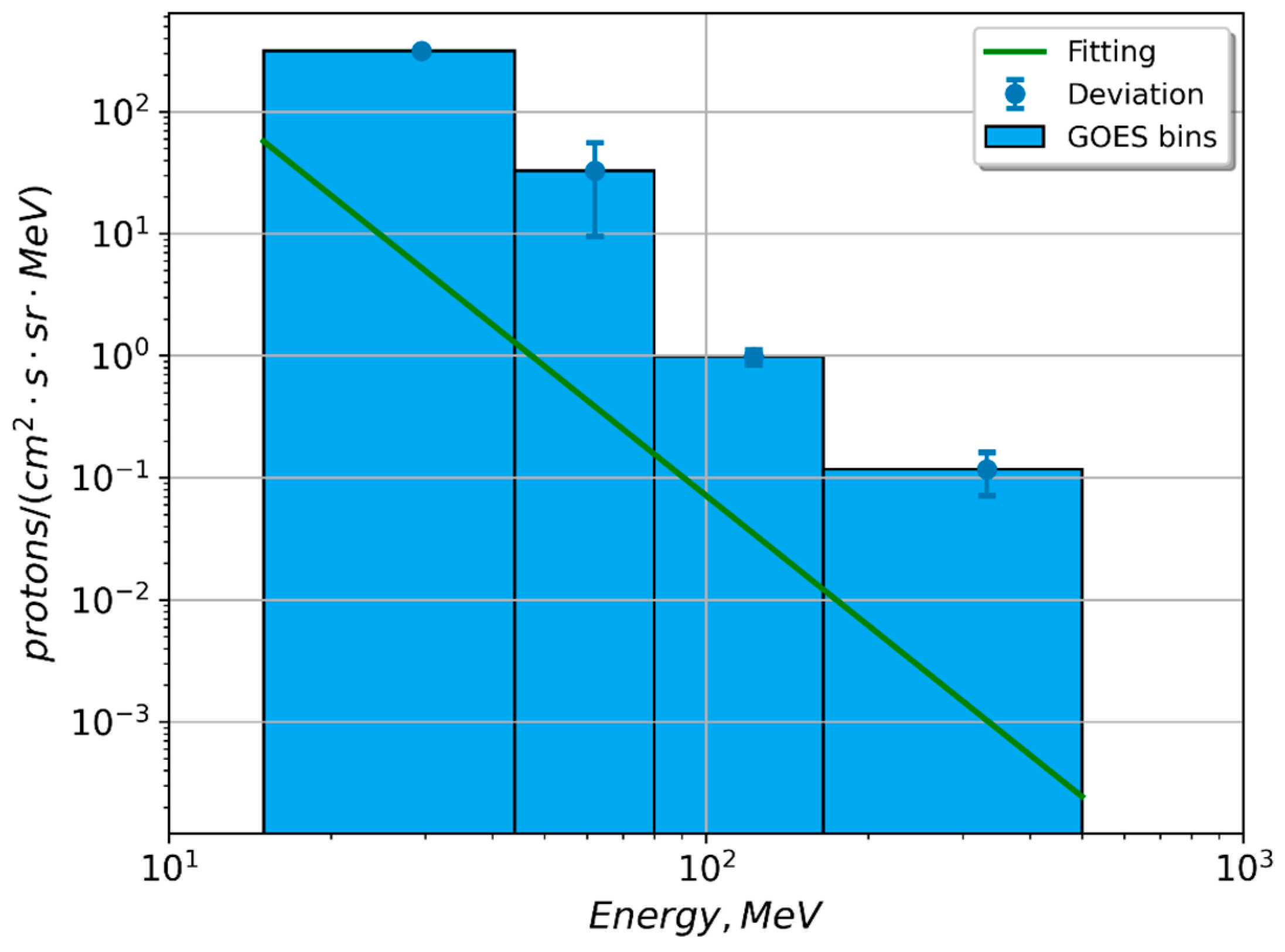
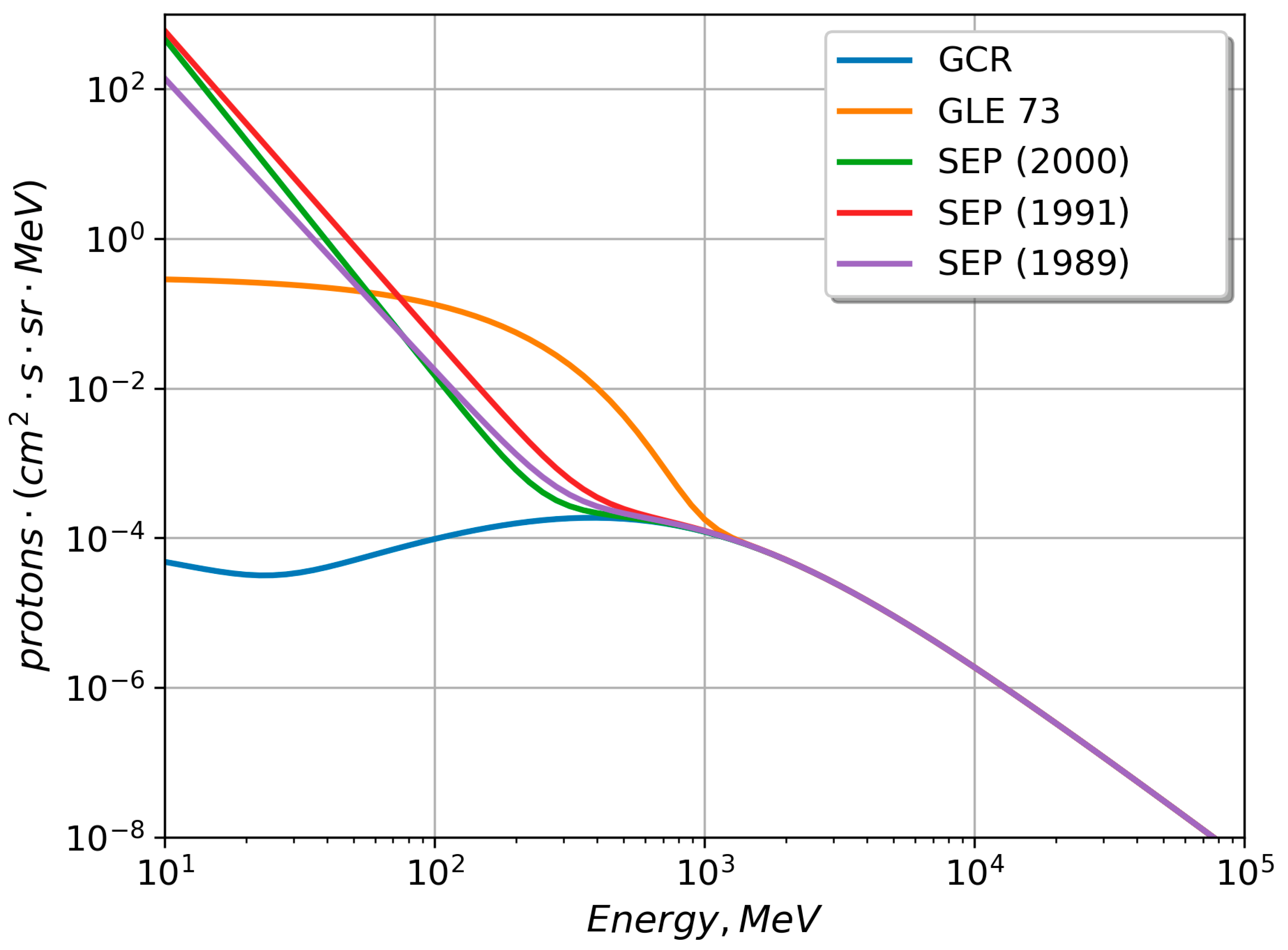

| Solar Flare Date and Time | Coord | X-Flare | Max P10, pfu | Max P50, pfu | Max P100, pfu | GLE, % | CME Speed, km/s |
|---|---|---|---|---|---|---|---|
| 12 August 1989 13:57 | S16W37 | X2.6 | 6000 | 875 | 23.7 | - | - |
| 22 March 1991 22:43 | S26E28 | X9.4 | 43,000 | 866 | 100 | - | - |
| 8 November 2000 22:42 | N10W77 | M7.4 | 14,800 | 1580 | 451 | - | 1738 |
| 28 October 2021 15:17 | S26W05 | X1.0 | 29 | 11 | 7 | 1 | 1519 |
| Altitude | GCR, µSv∙h−1 | SEP (1989), µSv∙h−1 | SEP (1991), µSv∙h−1 | SEP (2000), µSv∙h−1 | GLE 73, µSv∙h−1 |
|---|---|---|---|---|---|
| 0 | 0.01 | 0.01 | 0.01 | 0.01 | 0.02 |
| 1 | 0.03 | 0.03 | 0.03 | 0.03 | 0.05 |
| 2 | 0.06 | 0.06 | 0.06 | 0.06 | 0.15 |
| 3 | 0.13 | 0.14 | 0.14 | 0.14 | 0.34 |
| 4 | 0.27 | 0.27 | 0.28 | 0.27 | 0.69 |
| 5 | 0.5 | 0.51 | 0.52 | 0.5 | 1.41 |
| 6 | 0.88 | 0.91 | 0.92 | 0.89 | 2.65 |
| 7 | 1.45 | 1.51 | 1.55 | 1.48 | 4.73 |
| 8 | 2.29 | 2.37 | 2.47 | 2.34 | 7.94 |
| 9 | 3.48 | 3.62 | 3.79 | 3.55 | 12.68 |
| 10 | 5.07 | 5.26 | 5.6 | 5.18 | 19.16 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Maurchev, E.; Shlyk, N.; Abunina, M.; Abunin, A.; Belov, A.; Didenko, K. A Method for the Ambient Equivalent Dose Estimation in a Wide Range of Altitudes during SEP and GLE Events. Atmosphere 2024, 15, 92. https://doi.org/10.3390/atmos15010092
Maurchev E, Shlyk N, Abunina M, Abunin A, Belov A, Didenko K. A Method for the Ambient Equivalent Dose Estimation in a Wide Range of Altitudes during SEP and GLE Events. Atmosphere. 2024; 15(1):92. https://doi.org/10.3390/atmos15010092
Chicago/Turabian StyleMaurchev, Eugene, Nataly Shlyk, Maria Abunina, Artem Abunin, Anatoly Belov, and Kseniia Didenko. 2024. "A Method for the Ambient Equivalent Dose Estimation in a Wide Range of Altitudes during SEP and GLE Events" Atmosphere 15, no. 1: 92. https://doi.org/10.3390/atmos15010092
APA StyleMaurchev, E., Shlyk, N., Abunina, M., Abunin, A., Belov, A., & Didenko, K. (2024). A Method for the Ambient Equivalent Dose Estimation in a Wide Range of Altitudes during SEP and GLE Events. Atmosphere, 15(1), 92. https://doi.org/10.3390/atmos15010092






