Realizable Eddy Damped Markovian Anisotropic Closure for Turbulence and Rossby Wave Interactions
Abstract
1. Introduction
2. Herring’s Statistical Dynamical Theories and Their Impacts and Extensions
2.1. Equilibrium Statistical Mechanics
2.1.1. Complete Statistical Mechanics Theories
2.1.2. Empirical Statistical Mechanics Theories
2.2. Eulerian Statistical Dynamical Closure Theories
2.3. Quasi-Lagrangian Statistical Dynamical Closure Theories
2.4. Homogeneous Closures for Turbulent Flows over Topography
2.5. Inhomogeneous Closures for Turbulent Flows over Topography
2.6. Markovian Statistical Closure Theories without Waves
2.7. Markovian Statistical Closure Theories with Waves
2.8. Classical and Quantum Statistical Field Theory Formalisms
3. Two-Dimensional Barotropic Flows on a β-Plane
4. Dynamical Equations in Fourier Space
5. Eulerian Non-Markovian Statistical Dynamical Closures
5.1. The DIA Closure for Homogeneous Turbulence
5.2. The SCFT and LET Closures for Homogeneous Turbulence
5.3. Regularized non-Markovian Closures for Homogeneous Turbulence
6. Statistical Dynamical Equations for Markovian Anisotropic Closures
7. Realizable Eddy-Damped Markovian Anisotropic Closure
7.1. Analytical Triad Relaxation Function for EDQNM
7.2. Analytical Relaxation Function for EDMAC
7.3. EDMAC for Three-Dimensional Turbulent Flows
8. Reflections and Perspectives
8.1. Jorgen’s Personal Reflections
8.2. Perspectives on Strong Interaction Theories
9. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A. Positive Semi-Definite Triad Relaxation Time with Rossby Waves
Appendix B. Langevin Equation for EDMAC Model

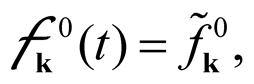
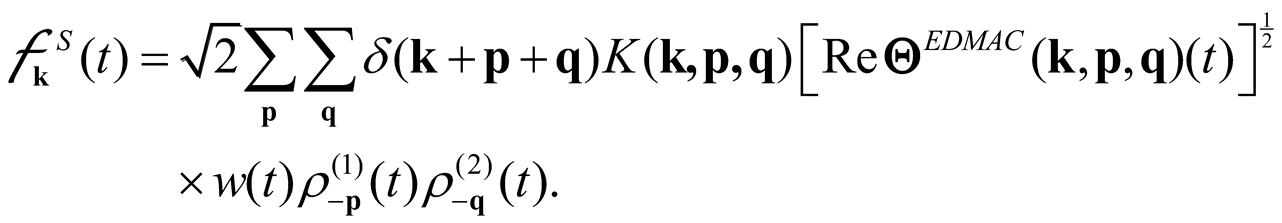
References
- Herring, J.R. Theory of two-dimensional anisotropic turbulence. J. Atmos. Sci. 1975, 32, 2252–2271. [Google Scholar] [CrossRef]
- Orszag, S.A. Analytical theories of turbulence. J. Fluid Mech. 1970, 41, 363–386. [Google Scholar] [CrossRef]
- Leith, C.E. Atmospheric predictability and two-dimensional turbulence. J. Atmos. Sci. 1971, 28, 145–161. [Google Scholar] [CrossRef]
- Herring, J.R. On the statistical theory of two-dimensional topographic turbulence. J. Atmos. Sci. 1977, 34, 1731–1750. [Google Scholar] [CrossRef]
- Onsager, L. Statistical hydrodynamics. Nouvo Cimento 1949, 6, 279–287. [Google Scholar] [CrossRef]
- Kraichnan, R.H. Inertial ranges in two-dimensional turbulence. Phys. Fluids 1967, 10, 1417–1423. [Google Scholar] [CrossRef]
- Kraichnan, R. Statistical dynamics of two-dimensional flow. J. Fluid Mech. 1975, 67, 155–175. [Google Scholar] [CrossRef]
- Salmon, R.; Holloway, G.; Hendershott, M. The equilibrium statistical mechanics of simple quasigeostrophic models. J. Fluid Mech. 1976, 75, 691–703. [Google Scholar] [CrossRef]
- Frederiksen, J.S.; Sawford, B.L. Statistical dynamics of two-dimensional inviscid flow on a sphere. J. Atmos Sci. 1980, 37, 717–732. [Google Scholar] [CrossRef]
- Frederiksen, J.S.; Sawford, B.L. Topographic waves in nonlinear and linear spherical barotropic models. J. Atmos. Sci. 1981, 38, 69–86. [Google Scholar] [CrossRef]
- Salmon, R.; Pizzo, N. Two-dimensional flow on the sphere. Atmosphere 2023, 14, 747. [Google Scholar] [CrossRef]
- Bretherton, F.; Haidvogel, D. Two-dimensional turbulence above topography. J. Fluid Mech. 1976, 78, 129–154. [Google Scholar] [CrossRef]
- Arnold, V. On conditions for nonlinear stability of plane stationary curvilinear flows of an ideal fluid. Sov. Math. Dokl. 1965, 162, 773–777. [Google Scholar]
- Frederiksen, J.S.; Carnevale, G.F. Stability properties of exact nonzonal solutions for flow over topography. Geophys. Astrophys. Fluid Dyn. 1986, 35, 173–207. [Google Scholar] [CrossRef]
- Frederiksen, J.S. Nonlinear stability of baroclinic flows over topography. Geophys. Astrophys. Fluid Mech. 1991, 57, 85–97. [Google Scholar] [CrossRef]
- Frederiksen, J.S. Nonlinear studies on the effect of topography on baroclinic zonal flows. Geophys. Astrophys. Fluid Dyn. 1991, 59, 57–82. [Google Scholar] [CrossRef]
- Carnevale, G.F.; Frederiksen, J.S. Nonlinear stability and statistical mechanics of flow over topography. J. Fluid Mech. 1987, 175, 157–181. [Google Scholar] [CrossRef]
- Miller, J. Statistical mechanics of Euler equations in two dimensions. Phys. Rev. Lett. 1990, 65, 2137–2140. [Google Scholar] [CrossRef]
- Miller, J.; Weichman, P.; Cross, M. Statistical mechanical mechanics, Euler equation, and Jupiter’s red spot. Phys. Rev. A 1992, 45, 2328–2359. [Google Scholar] [CrossRef]
- Robert, R. A maximum entropy principle for two-dimensional Euler equations. J. Stat. Phys. 1991, 65, 531–553. [Google Scholar] [CrossRef]
- Sommeria, J.; Nore, C.; Dumont, T.; Robert, R. Theorie statistique de la tache rouge de Jupiter. C. R. Acad. Sci. Paris 1991, 312, 999–1005. [Google Scholar]
- Chorin, A.J. Partition functions and equilibrium measures in two-dimensional and quasi-three-dimensional turbulence. Phys. Fluids 1996, 8, 2656–2660. [Google Scholar] [CrossRef]
- Turkington, B. Statistical equilibrium measures and coherent states in two-dimensional turbulence. Commun. Pure Appl. Math. 1999, 52, 781–809. [Google Scholar] [CrossRef]
- Majda, A.J.; Wang, X. Nonlinear Dynamics and Statistical Theories for Basic Geophysical Flows; Cambridge University Press: Cambridge, UK, 2006; ISBN -9780511616778. [Google Scholar] [CrossRef]
- Frederiksen, J.S.; O’Kane, T.J. Entropy, closures and subgrid modeling. Entropy 2008, 10, 635–683. [Google Scholar] [CrossRef]
- Bouchet, F.; Venaille, A. Statistical mechanics of two-dimensional and geophysical flows. Phys. Rep. 2012, 515, 227–295. [Google Scholar] [CrossRef]
- Zhou, Y. Turbulence theories and statistical closure approaches. Phys. Rep. 2021, 935, 1–117. [Google Scholar] [CrossRef]
- Kraichnan, R.H. The structure of isotropic turbulence at very high Reynolds numbers. J. Fluid Mech. 1959, 5, 497–543. [Google Scholar] [CrossRef]
- Kraichnan, R.H. Decay of isotropic turbulence in the direct-interaction approximation. Phys. Fluids 1964, 7, 1030–1048. [Google Scholar] [CrossRef]
- Wyld, H.W., Jr. Formulation of the theory of turbulence in an incompressible fluid. Ann. Phys. 1961, 14, 143–165. [Google Scholar] [CrossRef]
- Lee, L.L. A formulation of the theory of isotropic hydromagnetic turbulence in an incompressible fluid. Ann. Phys. 1965, 32, 292–321. [Google Scholar] [CrossRef]
- Frederiksen, J.S. Quasi-diagonal inhomogeneous closure for classical and quantum statistical dynamics. J. Math. Phys. 2017, 58, 103303. [Google Scholar] [CrossRef]
- Edwards, S.F. The statistical dynamics of homogeneous turbulence. J. Fluid Mech. 1964, 18, 239–273. [Google Scholar] [CrossRef]
- Herring, J.R. Self-consistent-field approach to turbulence theory. Phys. Fluids 1965, 8, 2219–2225. [Google Scholar] [CrossRef]
- Herring, J.R. Self-consistent-field approach to nonstationary turbulence. Phys. Fluids 1966, 9, 2106–2110. [Google Scholar] [CrossRef]
- Carnevale, G.F.; Frederiksen, J.S. Viscosity renormalization based on direct-interaction closure. J. Fluid Mech. 1983, 131, 289–304. [Google Scholar] [CrossRef]
- McComb, W.D. Jackson R. Herring and the Statistical Closure Problem of Turbulence: A Review of Renormalized Perturbation Theories. Atmosphere 2023, 14, 827. [Google Scholar] [CrossRef]
- McComb, W.D. A local energy-transfer theory of isotropic turbulence. J. Phys. A 1974, 7, 632–649. [Google Scholar] [CrossRef]
- McComb, W.D. The Physics of Fluid Turbulence; Oxford University Press: Oxford, UK, 1990; ISBN 9780198562566. [Google Scholar]
- McComb, W.D. Homogeneous, Isotropic Turbulence: Phenomenology, Renormalization and Statistical Closures; Oxford University Press: Oxford, UK, 2014; ISBN 9780199689385. [Google Scholar] [CrossRef]
- Frederiksen, J.S.; Davies, A.G.; Bell, R.C. Closure theories with non-Gaussian restarts for truncated two-dimensional turbulence. Phys. Fluids 1994, 6, 3153–3163. [Google Scholar] [CrossRef]
- Herring, J.R.; Orszag, S.A.; Kraichnan, R.H.; Fox, D.G. Decay of two-dimensional homogeneous turbulence. J. Fluid Mech. 1974, 66, 417–444. [Google Scholar] [CrossRef]
- Frederiksen, J.S.; Davies, A.G. Dynamics and spectra of cumulant update closures for two-dimensional turbulence. Geophys. Astrophys. Fluid Dyn. 2000, 92, 197–231. [Google Scholar] [CrossRef]
- Frederiksen, J.S.; O’Kane, T.J. Statistical Dynamics of Mean Flows Interacting with Rossby Waves, Turbulence, and Topography. Fluids 2022, 7, 200. [Google Scholar] [CrossRef]
- Lesieur, M. Turbulence in Fluids; Springer: Dordrecht, The Netherlands, 2008. [Google Scholar]
- Cambon, C.; Mons, V.; Gréa, B.-J.; Rubinstein, R. Anisotropic triadic closures for shear-driven and buoyancy-driven turbulent flows. Computers Fluids 2017, 151, 73–84. [Google Scholar] [CrossRef]
- Sagaut, P.; Cambon, C. Homogeneous Turbulence Dynamics; Springer Nature: Cham, Switzerland, 2018. [Google Scholar]
- Kraichnan, R.H. Lagrangian-history approximation for turbulence. Phys. Fluids 1965, 8, 575–598. [Google Scholar] [CrossRef]
- Kraichnan, R.H. Eulerian and Lagrangian renormalization in turbulence theory. J. Fluid Mech. 1977, 83, 349–374. [Google Scholar] [CrossRef]
- Herring, J.R.; Kraichnan, R.H. A numerical comparison of velocity-based and strain-based Lagrangian-history turbulence approximations. J. Fluid Mech. 1979, 91, 581–597. [Google Scholar] [CrossRef]
- Kaneda, Y. Renormalised expansions in the theory of turbulence with the use of the Lagrangian position function. J. Fluid Mech. 1981, 107, 131–145. [Google Scholar] [CrossRef]
- Gotoh, T.; Kaneda, Y.; Bekki, N. Numerical integration of the Lagrangian renormalized approximation. J. Phys. Soc. JPN. 1988, 57, 866–880. [Google Scholar] [CrossRef]
- Martin, P.C.; Siggia, E.D.; Rose, H.A. Statistical dynamics of classical systems. Phys. Rev. A 1973, 8, 423–437. [Google Scholar] [CrossRef]
- Kraichnan, R.H. Kolmogorov’s hypothesis and Eulerian turbulence theory. Phys. Fluids 1964, 7, 1723–1733. [Google Scholar] [CrossRef]
- Sudan, R.N.; Pfirsch, D. On the relation between ‘‘mixing length’’ and ‘‘direct interaction approximation” theories of turbulence. Phys. Fluids 1985, 28, 1702–1718. [Google Scholar] [CrossRef]
- Frederiksen, J.S.; Davies, A.G. The regularized DIA closure for two-dimensional turbulence. Geophys. Astrophys. Fluid Dyn. 2004, 98, 203–223. [Google Scholar] [CrossRef]
- O’Kane, T.J.; Frederiksen, J.S. The QDIA and regularized QDIA closures for inhomogeneous turbulence over topography. J. Fluid Mech. 2004, 65, 133–165. [Google Scholar] [CrossRef]
- Kraichnan, R.H. An almost-Markovian Galilean-invariant turbulence model. J. Fluid Mech. 1971, 47, 513–524. [Google Scholar] [CrossRef]
- Frederiksen, J.S. Subgrid-scale parameterizations of eddy-topographic force, eddy viscosity and stochastic backscatter for flow over topography. J. Atmos. Sci. 1999, 56, 1481–1494. [Google Scholar] [CrossRef]
- Frederiksen, J.S. Statistical dynamical closures and subgrid modeling for QG and 3D inhomogeneous turbulence. Entropy 2012, 14, 32–57. [Google Scholar] [CrossRef]
- Frederiksen, J.S. Self-Energy Closure for Inhomogeneous Turbulent Flows and Subgrid Modeling. Entropy 2012, 14, 769–799. [Google Scholar] [CrossRef]
- Frederiksen, J.S.; O’Kane, T.J. Inhomogeneous closure and statistical mechanics for Rossby wave turbulence over topography. J. Fluid Mech. 2005, 539, 137–165. [Google Scholar] [CrossRef]
- Frederiksen, J.S.; Kitsios, V.; O’Kane, T.J.; Zidikheri, M.J. Stochastic Subgrid Modelling for Geophysical and Three-Dimensional Turbulence. In Nonlinear and Stochastic Climate Dynamics; Franzke, C.J.E., O’Kane, T.J., Eds.; Cambridge University Press: Cambridge, UK, 2017; pp. 241–275. [Google Scholar] [CrossRef]
- Kitsios, V.; Frederiksen, J.S.; O’Kane, T.J. Subgrid parameterization of eddy, meanfield and topographic interactions in simulations of an idealized Antarctic Circumpolar Current. J. Adv. Model. Earth Syst. 2023, 15, e2022MS003412. [Google Scholar] [CrossRef]
- Rose, H.A. An efficient non-Markovian theory of non-equilibrium dynamics. Physica D 1985, 14, 216–226. [Google Scholar] [CrossRef]
- Herring, J.R.; Kraichnan, R.H. Statistical Models and Turbulence. In Lecture Notes in Physics: Proceedings of a Symposium Held at the University of California; Ehlers, J.K., Hepp, K.H.A., Weidenmuller, H.A., Eds.; Springer: Berlin/Heidelberg, Germany, 1972; pp. 148–194. [Google Scholar]
- Bowman, J.C.; Krommes, J.A.; Ottaviani, M. The realizable Markovian closure. I. General theory, with application to three-wave dynamics. Phys. Fluids 1993, 5, 3558–3589. [Google Scholar] [CrossRef]
- Carnevale, G.F.; Martin, P.C. Field theoretic techniques in statistical fluid dynamics: With application to nonlinear wave dynamics. Geophys. Astrophys. Fluid Dyn. 1982, 20, 131–164. [Google Scholar] [CrossRef]
- Carnevale, G.F.; Frederiksen, J.S. A statistical dynamical theory of strongly nonlinear internal gravity waves. Geophys. Astrophys. Fluid Dyn. 1983, 23, 175–207. [Google Scholar] [CrossRef]
- Zhou, Y.; Matthaeus, W.H.; Dmitruk, P. Magnetohydrodynamic turbulence and time scales in astrophysical and space plasmas. Rev. Mod. Phys. 2004, 76, 1015–1034. [Google Scholar] [CrossRef]
- Sukoriansky, S.; Galperin, B. QNSE theory of turbulence anisotropization and onset of the inverse energy cascade by solid body rotation. J. Fluid Mech. 2016, 805, 384–421. [Google Scholar] [CrossRef]
- Galperin, B.; Sukoriansky, S.; Qiu, B. Seasonal oceanic variability on meso- and sub-mesoscales: A turbulence perspective. Ocean Dynam. 2021, 71, 475–489. [Google Scholar] [CrossRef]
- Hu, G.; Krommes, J.A.; Bowman, J.C. Statistical theory of resistive drift-wave turbulence and transport. Phys. Plasmas 1997, 4, 2116–2133. [Google Scholar] [CrossRef]
- Bowman, J.C.; Krommes, J.A. The realizable Markovian closure and realizable test-field model. II. Application to anisotropic drift-wave dynamics. Phys. Plasmas 1997, 4, 3895–3909. [Google Scholar] [CrossRef]
- Frederiksen, J.S.; O’Kane, T.J. Markovian inhomogeneous closures for Rossby waves and turbulence over topography. J. Fluid Mech. 2019, 858, 45–70. [Google Scholar] [CrossRef]
- Rose, H.A. Aspects of Statistical Dynamics of Classical Systems. Ph.D. Thesis, Harvard University, Cambridge, MA, USA, 1974. [Google Scholar]
- Feynman, R.P.; Hibbs, A.R. Quantum Mechanics and Path Integrals; Dover Publications: Mineola, NY, USA, 1965. [Google Scholar]
- Phythian, R. The functional formalism of classical statistical dynamics. J. Phys. A Math. Gen. 1977, 10, 777–789. [Google Scholar] [CrossRef]
- Jensen, R.V. Functional integral approach to classical statistical dynamics. J. Stat. Phys. 1981, 25, 183–201. [Google Scholar] [CrossRef]
- Berera, A.; Salewski, M.; McComb, W.D. Eulerian field-theoretic closure formalisms for fluid turbulence. Phys. Rev. E 2013, 87, 013007. [Google Scholar] [CrossRef] [PubMed]
- Schwinger, J. Brownian motion of a quantum oscillator. J. Math. Phys. 1961, 2, 407–432. [Google Scholar] [CrossRef]
- Keldysh, L.V. Diagram technique for nonequilibrium processes. Sov. Phys. JETP 1965, 20, 1018–1026. [Google Scholar]
- Cooper, F.; Khare, A.; Rose, H. Classical limit of time-dependent quantum field theory—A Schwinger-Dyson approach. Phys. Lett. B 2001, 515, 463–469. [Google Scholar] [CrossRef]
- Blagoev, K.B.; Cooper, F.; Dawson, J.F.; Mihaila, B. Schwinger-Dyson approach to nonequilibrium classical field theory. Phys. Rev. D 2001, 64, 125033. [Google Scholar] [CrossRef]
- Frederiksen, J.S. Renormalized closure theory and subgrid-scale parameterizations for two-dimensional turbulence. In Nonlinear Dynamics: From Lasers to Butterflies, World Scientific Lecture Notes in Complex Systems; Ball, R., Akhmediev, N., Eds.; World Scientific: Singapore, 2003; Volume 1, pp. 225–256. ISBN 978-981-4486-36-1. [Google Scholar] [CrossRef]
- Pouquet, A.; Lesieur, M.; Andre, J.C.; Basdevant, C. Evolution of high Reynolds number two-dimensional turbulence. J. Fluid Mech. 1975, 72, 305–319. [Google Scholar] [CrossRef]
- Mandelstam, S. Determination of the pion-pion scattering amplitude from dispersion relations and unitarity. General theory. Phys. Rev. 1958, 112, 1344–1360. [Google Scholar] [CrossRef]
- Frederiksen, J.S. Sommerfeld-Watson representation for double spectral functions. II. Crossing symmetric pion-pion scattering amplitude without Regge poles. Commun. Math. Phys. 1975, 43, 1–16. [Google Scholar] [CrossRef]
- Atkinson, D.; Kaekebeke, M.; Frederiksen, J.S.; Johnson, P.W. Sommerfeld-Watson representation for double-spectral functions. III. Crossing symmetric pion-pion scattering amplitude with Regge poles. Commun. Math. Phys. 1976, 51, 67–84. [Google Scholar] [CrossRef]
- Tourkine, P.; Zhiboedov, A. Scattering amplitudes from dispersive iterations of unitarity. arXiv 2023, arXiv:2303.088339v1. [Google Scholar]
- Gasenzer, T. Ultracold gases far from equilibrium. Eur. Phys. J. Spec. Top. 2009, 168, 89–148. [Google Scholar] [CrossRef]
- Berges, J.; Sexty, D. Bose-Einstein condensation in relativistic field theories far from equilibrium. Phys. Rev. Lett. 2012, 108, 161601. [Google Scholar] [CrossRef] [PubMed]
- Kofman, L.; Linde, A.; Starbinsky, A.A. Towards the theory of reheating after inflation. Phys. Rev. D 1997, 56, 3258–3295. [Google Scholar] [CrossRef]
- Micha, R.; Tkachev, I.I. Relativistic turbulence: A long way from preheating to equilibrium. Phys. Rev. Lett. 2003, 90, 121301. [Google Scholar] [CrossRef]
- Kofman, L. Preheating after Inflation. In Inflationary Cosmology; Lemoine, M., Martin, J., Peter, P., Eds.; Lecture Notes Phys; Springer: Berlin/Heidelberg, Germany, 2007; Volume 738, pp. 55–79. ISBN 978-3-540-74352-1. [Google Scholar]
- Arnold, P. Quark-gluon plasmas and thermalization. Int. J. Mod. Phys. E 2007, 16, 2555–2594. [Google Scholar] [CrossRef]
- Kraichnan, R.H. Test-field model for inhomogeneous turbulence. J. Fluid Mech. 1972, 56, 287–304. [Google Scholar] [CrossRef]
- Aarts, G.; Berges, J. Classical aspects of quantum fields far from equilibrium. Phys. Rev. Lett. 2002, 88, 041603. [Google Scholar] [CrossRef]
- Cooper, F.; Dawson, J.F.; Mihaila, B. Quantum dynamics of phase transitions in broken symmetry λφ4 field theory. Phys. Rev. D 2003, 67, 056003. [Google Scholar] [CrossRef]
- Boyanovsky, D.; Destri, C.; Vega, H.J. The approach to thermalization in the classical φ4 theory in 1+1 dimensions: Energy cascades and universal scaling. Phys. Rev. D 2004, 69, 045003. [Google Scholar] [CrossRef]
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Frederiksen, J.S.; O’Kane, T.J. Realizable Eddy Damped Markovian Anisotropic Closure for Turbulence and Rossby Wave Interactions. Atmosphere 2023, 14, 1098. https://doi.org/10.3390/atmos14071098
Frederiksen JS, O’Kane TJ. Realizable Eddy Damped Markovian Anisotropic Closure for Turbulence and Rossby Wave Interactions. Atmosphere. 2023; 14(7):1098. https://doi.org/10.3390/atmos14071098
Chicago/Turabian StyleFrederiksen, Jorgen S., and Terence J. O’Kane. 2023. "Realizable Eddy Damped Markovian Anisotropic Closure for Turbulence and Rossby Wave Interactions" Atmosphere 14, no. 7: 1098. https://doi.org/10.3390/atmos14071098
APA StyleFrederiksen, J. S., & O’Kane, T. J. (2023). Realizable Eddy Damped Markovian Anisotropic Closure for Turbulence and Rossby Wave Interactions. Atmosphere, 14(7), 1098. https://doi.org/10.3390/atmos14071098







