Kinetic Studies on the Steam Gasification of Chars Derived from Coals by Different Isoconversional Methods
Abstract
1. Introduction
2. Experiments and Theory
2.1. Coal Samples and Char Preparation
2.2. Steam Gasification
2.3. Kinetics Analysis
2.3.1. Activation Energy Calculation
2.3.2. Kinetic Model Analysis Methodology
2.3.3. Pre-Exponential Factor Calculation
3. Results and Discussion
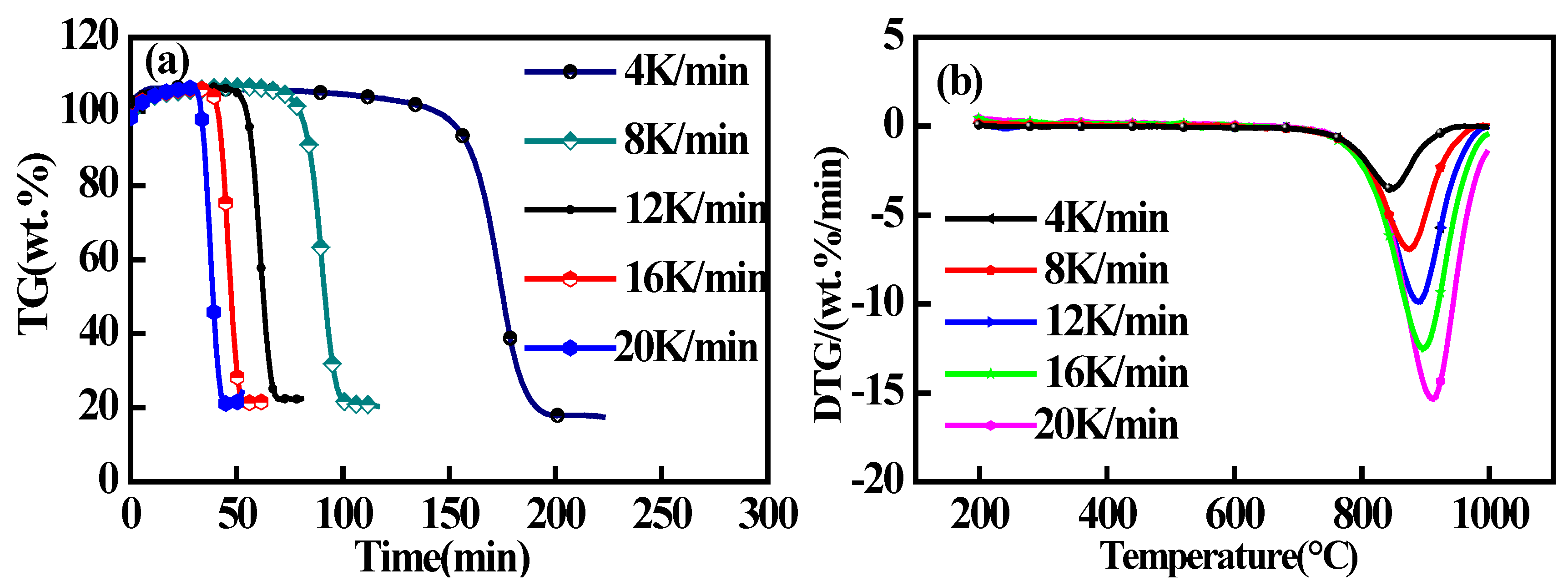
3.1. Effect of the Heating Rate on the Char Conversion
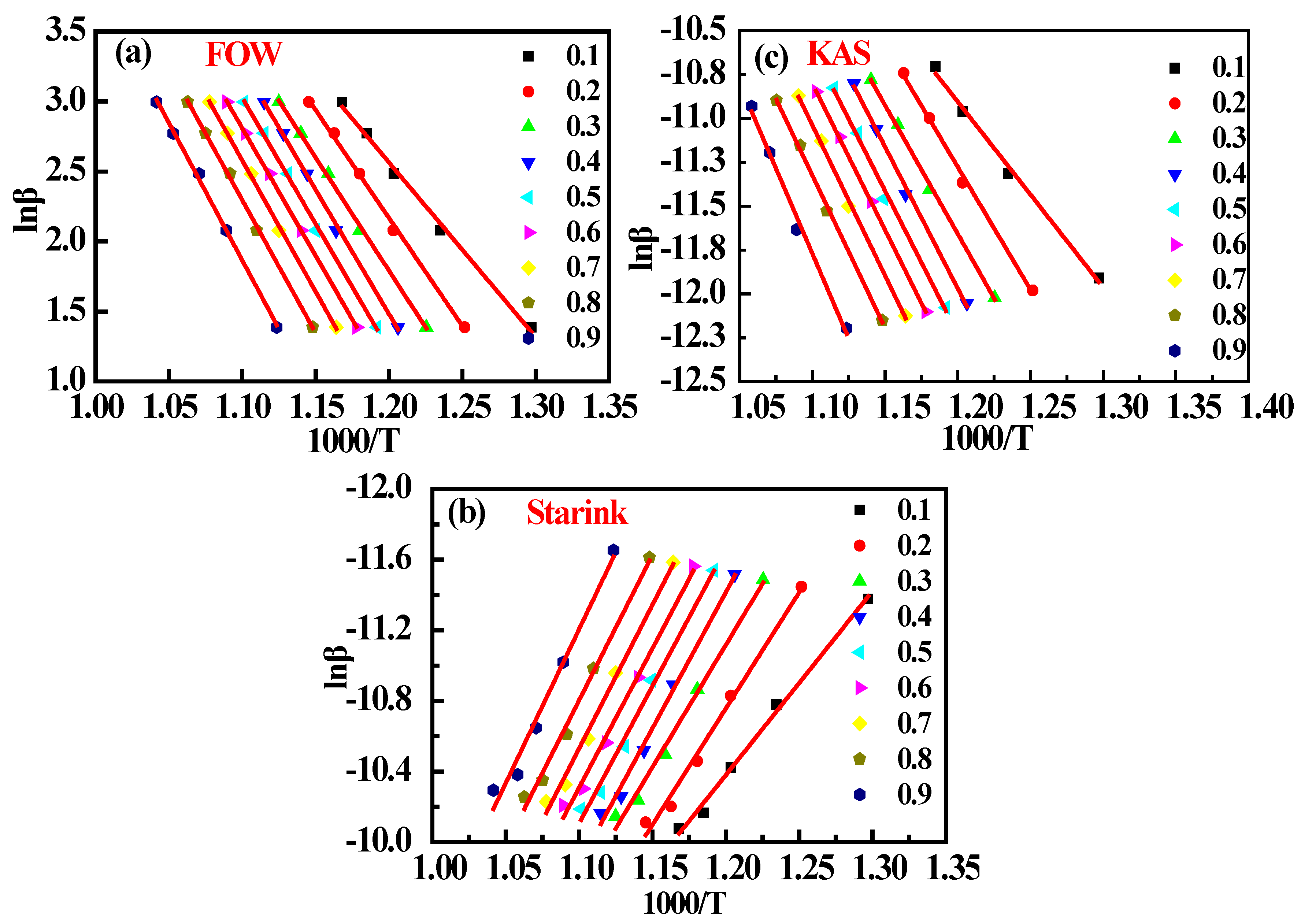
3.2. Activation Energy Values Obtained Using Isoconversional Methods
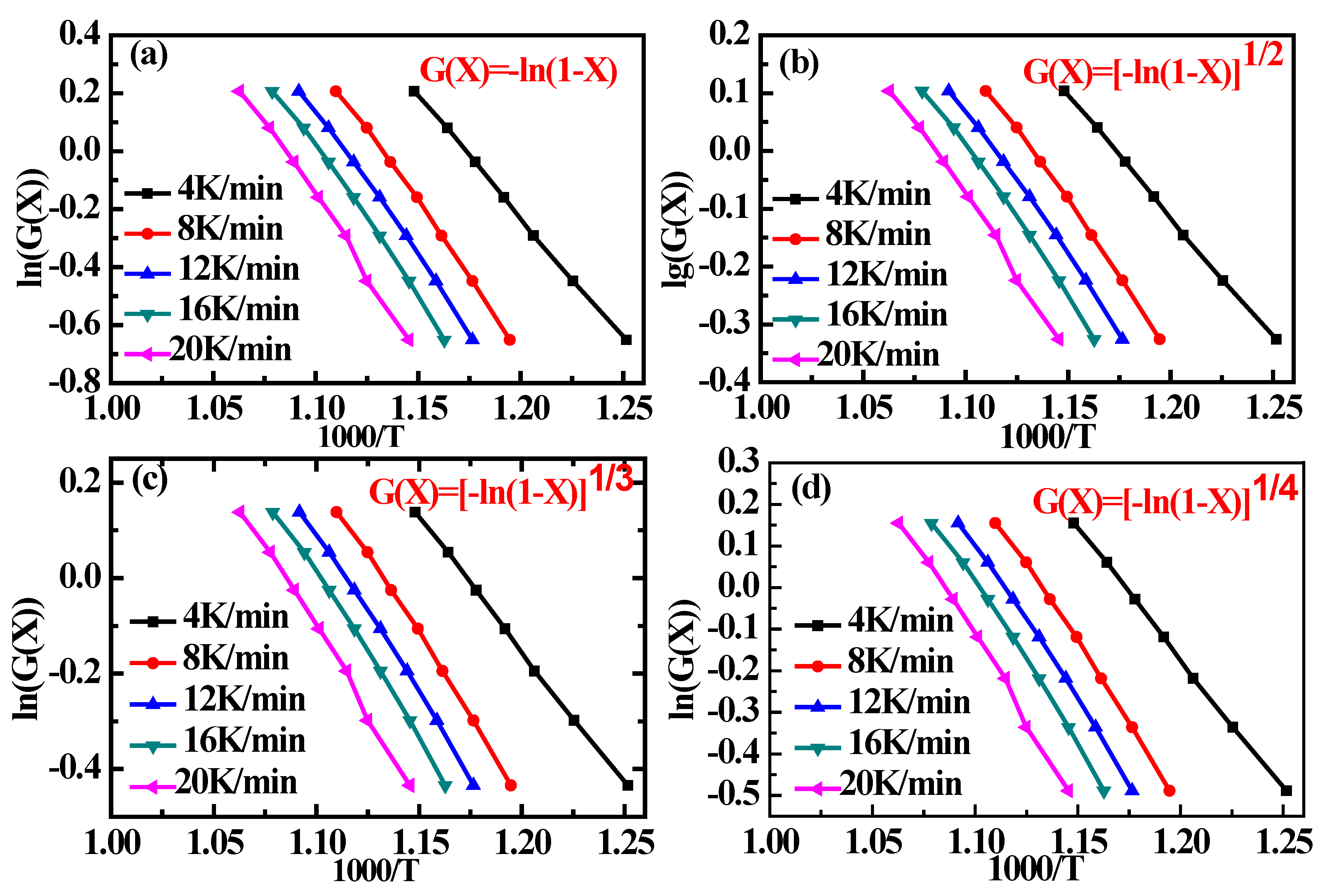
3.3. Analysis of Kinetic Models
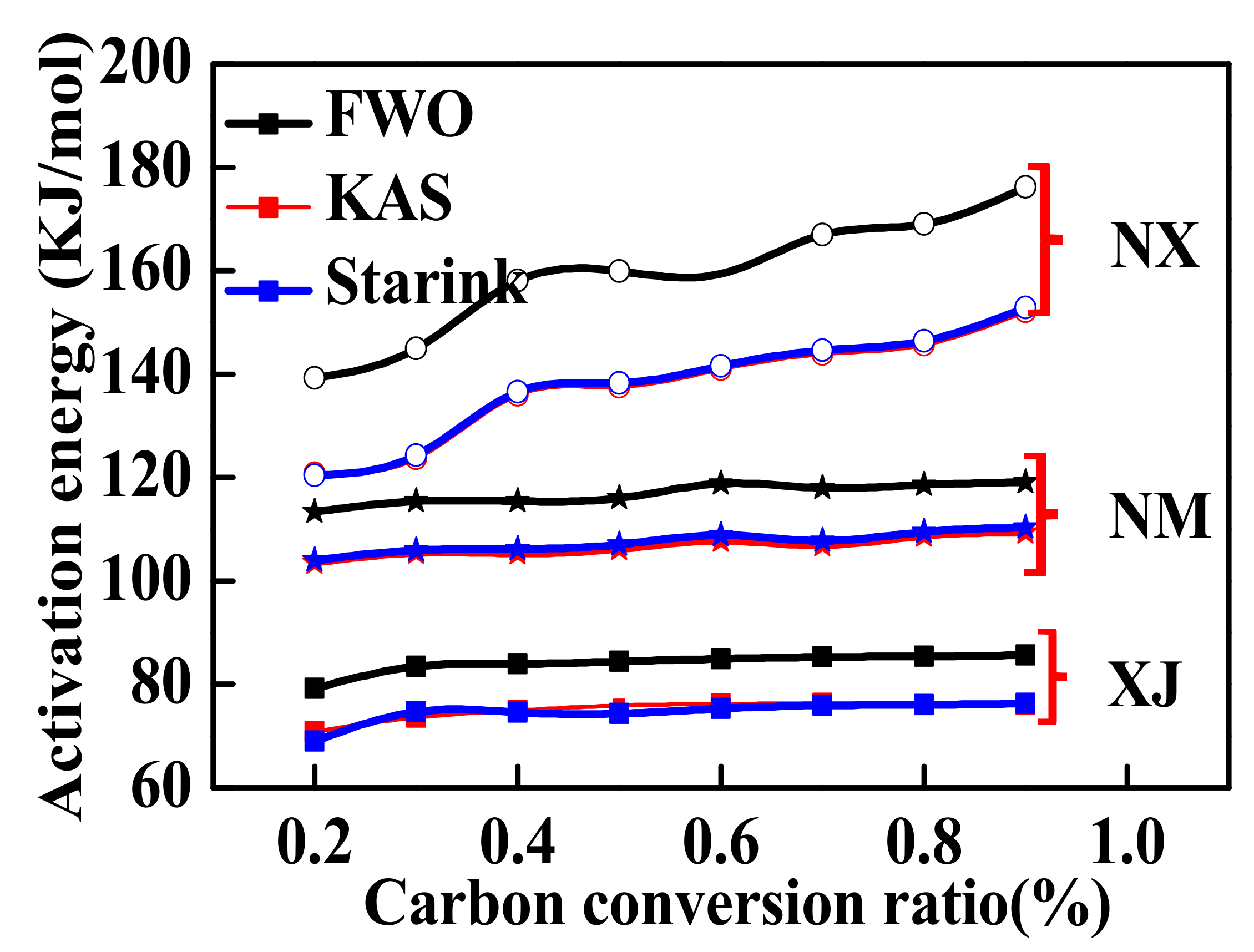
3.4. Application and Analysis
4. Conclusions
- The weight loss of the char samples in the steam atmosphere was relatively similar at different heating rates, while the value of X increased with the increasing heating rate.
- The FWO method provided somewhat greater values of Ea than the KAS and Starink methods, while the latter two methods provided consistent Ea values for all the values of X. The average value of Ea obtained by the Starink method (EStarink) was deemed optimal.
- The 3D Avrami–Erofeev model provided the best fit with the data, while the 3D Avrami–Erofeev model yielded a value of Eave closest to EStarink. The two values differed by only 0.70 KJ.mol−1.
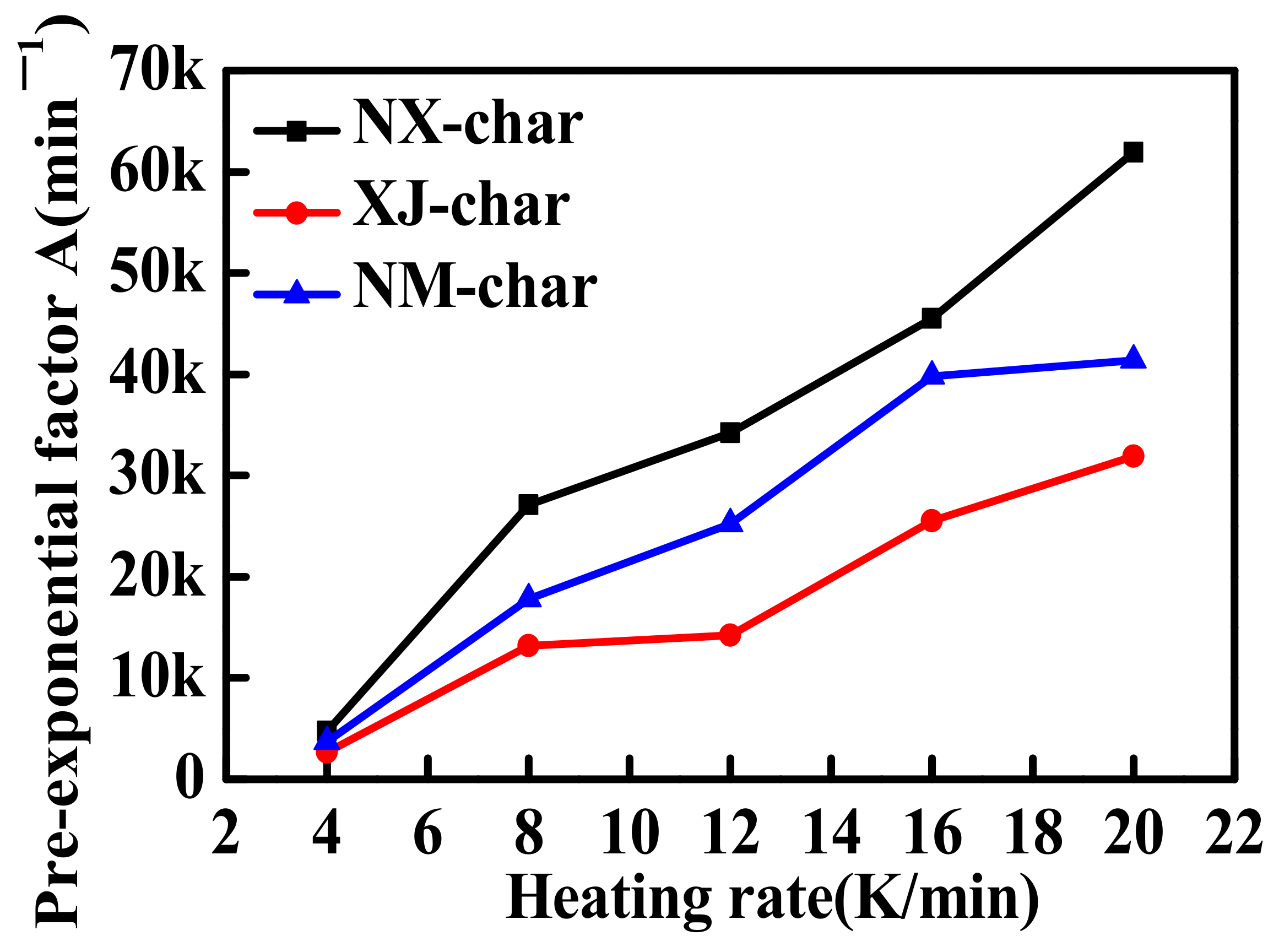
- The values of Ea obtained from the fitted 3D Avrami–Erofeev model for chars derived from the three coal sources decreased in the order of NX char (115–153 KJ.mol−1) > NM char (103–110 KJ.mol−1) > XJ char (70–78 KJ.mol−1). The pre-exponential factor A followed an order of decreasing values consistent with that obtained for Ea.
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Brown, M.E.; Maciejewski, M.; Vyazovkin, S.; Nomen, R.; Sempere, J.; Burnham, A.; Opfermann, J.; Strey, R.; Anderson, H.L.; Kemmler, A. Computational aspects of kinetic analysis.: Part A: The ICTAC kinetics project-data, methods and results. Thermochim. Acta 2000, 355, 125–143. [Google Scholar] [CrossRef]
- Kök, M.V. Recent developments in the application of thermal analysis techniques in fossil fuels. J. Therm. Anal. Calorim. 2008, 91, 763–773. [Google Scholar] [CrossRef]
- Vyazovkin, S. Computational aspects of kinetic analysis.: Part C. The ICTAC Kinetics Project—The light at the end of the tunnel. Thermochim. Acta 2000, 355, 155–163. [Google Scholar] [CrossRef]
- Wang, F.; Zeng, X.; Wang, Y.; Su, H.; Yu, J.; Xu, G. Non-isothermal coal char gasification with CO2 in a micro fluidized bed reaction analyzer and a thermogravimetric analyzer. Fuel 2016, 164, 403–409. [Google Scholar] [CrossRef]
- Ashraf, A.; Sattar, H.; Munir, S. A comparative applicability study of model-fitting and model-free kinetic analysis approaches to non-isothermal pyrolysis of coal and agricultural residues. Fuel 2019, 240, 326–333. [Google Scholar] [CrossRef]
- Liu, Y.; Fu, P.; Bie, K.; Xu, T.; Ahsan, M. Non-isothermal kinetics of coal char oxyfuel combustion by isothermal model-fitting method. Energy Rep. 2022, 8, 2062–2071. [Google Scholar] [CrossRef]
- Yang, S.; Ding, J.; Zhang, X.; Ye, S.; Guo, Z.; Chen, W. Fusion method of model-free and model-fitting for complex reactions in accelerating rate calorimetry. Thermochim. Acta 2022, 712, 179212. [Google Scholar] [CrossRef]
- Mahmood, H.; Shakeel, A.; Abdullah, A.; Khan, M.; Moniruzzaman, M. A Comparative Study on Suitability of Model-Free and Model-Fitting Kinetic Methods to Non-Isothermal Degradation of Lignocellulosic Materials. Polymers 2021, 13, 2504. [Google Scholar] [CrossRef]
- Gomez, A.; Mahinpey, N. A new method to calculate kinetic parameters independent of the kinetic model: Insights on CO2 and steam gasification. Chem. Eng. Res. Des. 2015, 95, 346–357. [Google Scholar] [CrossRef]
- Mahinpey, N.; Gomez, A. Review of gasification fundamentals and new findings: Reactors, feedstock, and kinetic studies. Chem. Eng. Sci. 2016, 148, 14–31. [Google Scholar] [CrossRef]
- Jain, A.A.; Mehra, A.; Ranade, V.V. Processing of TGA data: Analysis of isoconversional and model fitting methods. Fuel 2016, 165, 490–498. [Google Scholar] [CrossRef]
- Czerski, G.; Zubek, K.; Grzywacz, P.; Porada, S. Effect of Char Preparation Conditions on Gasification in a Carbon Dioxide Atmosphere. Energy Fuels 2016, 31, 815–823. [Google Scholar] [CrossRef]
- Sebakhy, K.O.; Vitale, G.; Hassan, A.; Pereira-Almao, P. New Insights into the Kinetics of Structural Transformation and Hydrogenation Activity of Nano-crystalline Molybdenum Carbide. Catal. Lett. 2018, 148, 904–923. [Google Scholar] [CrossRef]
- Byambajav, E.; Hachiyama, Y.; Kudo, S.; Norinaga, K.; Hayashi, J. Kinetics and Mechanism of CO2 Gasification of Chars from 11 Mongolian Lignites. Energy Fuels 2015, 30, 1636–1646. [Google Scholar] [CrossRef]
- Korotkikh, A.G.; Slyusarskiy, K.V. Kinetic study of coals gasification into carbon dioxide atmosphere. MATEC Web Conf. 2015, 23, 1020. [Google Scholar] [CrossRef]
- Gomez, A.; Mahinpey, N. Kinetic study of coal steam and CO2 gasification: A new method to reduce interparticle diffusion. Fuel 2015, 148, 160–167. [Google Scholar] [CrossRef]
- Gomez, A.; Silbermann, R.; Mahinpey, N. A comprehensive experimental procedure for CO2 coal gasification: Is there really a maximum reaction rate? Appl. Energy 2014, 124, 73–81. [Google Scholar] [CrossRef]
- Yang, Z.; Gao, M.; Wang, Y.; Bai, Y.; Li, F. Identification for the behavior of maximum reaction rate during the initial stage of coal char gasification. J. Therm. Anal. Calorim. 2017, 128, 1183–1194. [Google Scholar] [CrossRef]
- Vyazovkin, S.; Burnham, A.K.; Criado, J.M.; Pérez-Maqueda, L.A.; Popescu, C.; Sbirrazzuoli, N. ICTAC Kinetics Committee recommendations for performing kinetic computations on thermal analysis data. Thermochim. Acta 2011, 520, 1–19. [Google Scholar] [CrossRef]
- Doyle, C.D. Estimating isothermal life from thermogravimetric data. J. Appl. Polym. Sci. 1962, 6, 639–642. [Google Scholar] [CrossRef]
- Liu, H.; Zhu, H.; Kaneko, M.; Kato, S.; Kojima, T. High-Temperature Gasification Reactivity with Steam of Coal Chars Derived under Various Pyrolysis Conditions in a Fluidized Bed. Energy Fuels 2010, 24, 68–75. [Google Scholar] [CrossRef]
- Zhang, Y.; Hara, S.; Kajitani, S.; Ashizawa, M. Modeling of catalytic gasification kinetics of coal char and carbon. Fuel 2010, 89, 152–157. [Google Scholar] [CrossRef]
- Wang, Y.L.; Zhu, S.H.; Gao, M.Q.; Yang, Z.R.; Yan, L.J.; Bai, Y.H.; Li, F. A study of char gasification in H2O and CO2 mixtures: Role of inherent minerals in the coal. Fuel Process. Technol. 2015, 141, 9–15. [Google Scholar] [CrossRef]
- Dai, P.; Dennis, J.S.; Scott, S.A. Using an experimentally-determined model of the evolution of pore structure for the gasification of chars by CO2. Fuel 2016, 171, 29–43. [Google Scholar] [CrossRef][Green Version]
- Jayaraman, K.; Gokalp, I.; Bonifaci, E.; Merlo, N. Kinetics of steam and CO2 gasification of high ash coal–char produced under various heating rates. Fuel 2015, 154, 370–379. [Google Scholar] [CrossRef]
- Fan, D.; Zhu, Z.; Na, Y.; Lu, Q. Thermogravimetric analysis of gasification reactivity of coal chars with steam and CO2 at moderate temperatures. J. Therm. Anal. Calorim. 2013, 113, 599–607. [Google Scholar] [CrossRef]
- Everson, R.C.; Okolo, G.N.; Neomagus, H.W.J.P.; Dos Santos, J. X-ray diffraction parameters and reaction rate modeling for gasification and combustion of chars derived from inertinite-rich coals. Fuel 2013, 109, 148–156. [Google Scholar] [CrossRef]
- Everson, R.C.; Neomagus, H.W.J.P.; Kaitano, R.; Falcon, R.; du Cann, V.M. Properties of high ash coal-char particles derived from inertinite-rich coal: II. Gasification kinetics with carbon dioxide. Fuel 2008, 87, 3403–3408. [Google Scholar] [CrossRef]
- Fermoso, J.; Gil, M.V.; Garciía, S.; Pevida, C.; Pis, J.J.; Rubiera, F. Kinetic Parameters and Reactivity for the Steam Gasification of Coal Chars Obtained under Different Pyrolysis Temperatures and Pressures. Energy Fuels 2011, 25, 3574–3580. [Google Scholar] [CrossRef]
- Liu, Z.; Wang, Q.; Zou, Z.; Tan, G. Arrhenius parameters determination in non-isothermal conditions for the uncatalyzed gasification of carbon by carbon dioxide. Thermochim. Acta 2011, 512, 1–4. [Google Scholar] [CrossRef]
- Ding, H.; Jiang, Y.; Chen, Y.F.; Li, W.H. Experimental study on gasification kinetics of Lu’an coal char with steam by non-isothermal thermogravimetry. J. China Coal Soc. 2010, 35, 666–669. [Google Scholar]
- Edreis, E.M.A.; Luo, G.; Yao, H. Investigations of the structure and thermal kinetic analysis of sugarcane bagasse char during non-isothermal CO2 gasification. J. Anal. Appl. Pyrol. 2014, 107, 107–115. [Google Scholar] [CrossRef]
| Proximate Analysis (w%) | Ultimate Analysis (w%) | ||||||||
|---|---|---|---|---|---|---|---|---|---|
| Moisture | Ash | Volatile | Fixed C | C | H | N | S | O | |
| NX | 10.14 | 5.40 | 27.03 | 67.57 | 79.18 | 4.40 | 1.50 | 0.60 | 9.00 |
| SX | 15.40 | 3.88 | 32.37 | 65.00 | 76.06 | 3.50 | 0.29 | 0.44 | 15.83 |
| XJ | 6.88 | 5.07 | 29.26 | 67.15 | 65.33 | 4.989 | 0.75 | 0.14 | 24.02 |
| β (K·min−1) | Tmax (°C) | Mr (wt%) | Rmax (wt%·min−1) |
|---|---|---|---|
| 20 | 908 | 23.05 | 15.69 |
| 16 | 900 | 22.81 | 12.71 |
| 12 | 889 | 22.71 | 9.95 |
| 8 | 872 | 22.47 | 7.00 |
| 4 | 850 | 22.46 | 3.48 |
| Degree of Conversion (X) | FOW Method | KAS Method | Starink Method | |||
|---|---|---|---|---|---|---|
| Ea | R2 | Ea | R2 | Ea | R2 | |
| 0.1 | 109.54 | 0.9948 | 90.66 | 0.9918 | 91.27 | 0.9933 |
| 0.2 | 135.28 | 0.9974 | 114.74 | 0.9959 | 115.39 | 0.9861 |
| 0.3 | 144.96 | 0.9982 | 123.66 | 0.9978 | 124.33 | 0.9881 |
| 0.4 | 158.07 | 0.9981 | 135.94 | 0.9961 | 136.62 | 0.9899 |
| 0.5 | 159.94 | 0.9974 | 137.54 | 0.9933 | 138.23 | 0.9880 |
| 0.6 | 159.44 | 0.9998 | 140.89 | 0.9999 | 141.59 | 0.9901 |
| 0.7 | 166.98 | 0.9986 | 143.90 | 0.9970 | 144.61 | 0.9896 |
| 0.8 | 169.07 | 0.9984 | 145.69 | 0.9972 | 146.40 | 0.9887 |
| 0.9 | 176.19 | 0.9978 | 152.13 | 0.9888 | 152.87 | 0.9743 |
| Eave | 156.25 ± 20 | 134.62 ± 20 | 135.31 ± 20 | |||
| Reaction Model | G(X) | β = 4 | β = 8 | β = 12 | β = 16 | β = 20 | |
|---|---|---|---|---|---|---|---|
| 1 | Power law | X1/4 | 0.992 | 0.973 | 0.972 | 0.961 | 0.964 |
| 2 | Power law | X1/3 | 0.995 | 0.981 | 0.980 | 0.971 | 0.973 |
| 3 | Power law | X1/2 | 0.990 | 0.969 | 0.968 | 0.957 | 0.960 |
| 4 | Power law | X3/2 | 0.968 | 0.931 | 0.929 | 0.912 | 0.918 |
| 5 | Mampel (first order) | −ln(1 − X) | 0.998 | 0.993 | 0.992 | 0.986 | 0.987 |
| 6 | Avrami–Erofeev | [−ln(1 − X)]1/2 | 0.998 | 0.993 | 0.992 | 0.986 | 0.987 |
| 7 | Avrami–Erofeev | [−ln(1 − X)]1/3 | 0.998 | 0.993 | 0.992 | 0.986 | 0.987 |
| 8 | Avrami–Erofeev | [−ln(1 − X)]1/4 | 0.998 | 0.993 | 0.992 | 0.986 | 0.987 |
| 9 | Contracting sphere | 1 − (1 − X)1/3 | 0.995 | 0.981 | 0.980 | 0.971 | 0.973 |
| 10 | Contracting cylinder | 1 − (1 − X)1/2 | 0.992 | 0.973 | 0.972 | 0.961 | 0.964 |
| 11 | One-dimensional diffusion | X2 | 0.995 | 0.981 | 0.980 | 0.971 | 0.973 |
| 12 | Two-dimensional diffusion | (1 − X)ln(1 − X) + X | 0.992 | 0.973 | 0.972 | 0.961 | 0.964 |
| 13 | Three-dimensional diffusion | 1 − (1 − X)1/3 | 0.899 | 0.868 | 0.865 | 0.843 | 0.851 |
| 14 | Three-dimensional diffusion | 1 − 2/3X − (1 − X)3/2 | 0.864 | 0.794 | 0.791 | 0.764 | 0.774 |
| Kinetic Model | Ea (KJ.mol−1) | Eave | EStarink | Eave − EStarink | ||||
|---|---|---|---|---|---|---|---|---|
| β = 4 | β = 8 | β = 12 | β = 16 | β = 20 | ||||
| 1D Avrami–Erofeev | 72.39 | 92.91 | 92.28 | 95.30 | 96.46 | 89.87 | 135.31 | 45.44 |
| 2D Avrami–Erofeev | 96.52 | 123.88 | 123.05 | 127.06 | 128.61 | 119.82 | 15.49 | |
| 3D Avrami–Erofeev | 134.65 | 133.92 | 134.79 | 137.49 | 139.22 | 136.01 | 0.70 | |
| 4D Avrami–Erofeev | 181.19 | 185.82 | 184.57 | 190.60 | 192.91 | 187.02 | 51.71 | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
An, M.; Guo, Q. Kinetic Studies on the Steam Gasification of Chars Derived from Coals by Different Isoconversional Methods. Atmosphere 2022, 13, 1480. https://doi.org/10.3390/atmos13091480
An M, Guo Q. Kinetic Studies on the Steam Gasification of Chars Derived from Coals by Different Isoconversional Methods. Atmosphere. 2022; 13(9):1480. https://doi.org/10.3390/atmos13091480
Chicago/Turabian StyleAn, Mei, and Qingjie Guo. 2022. "Kinetic Studies on the Steam Gasification of Chars Derived from Coals by Different Isoconversional Methods" Atmosphere 13, no. 9: 1480. https://doi.org/10.3390/atmos13091480
APA StyleAn, M., & Guo, Q. (2022). Kinetic Studies on the Steam Gasification of Chars Derived from Coals by Different Isoconversional Methods. Atmosphere, 13(9), 1480. https://doi.org/10.3390/atmos13091480







