Design Hyetograph for Short-Duration Rainstorm in Jiangsu
Abstract
:1. Introduction
2. Materials and Methods
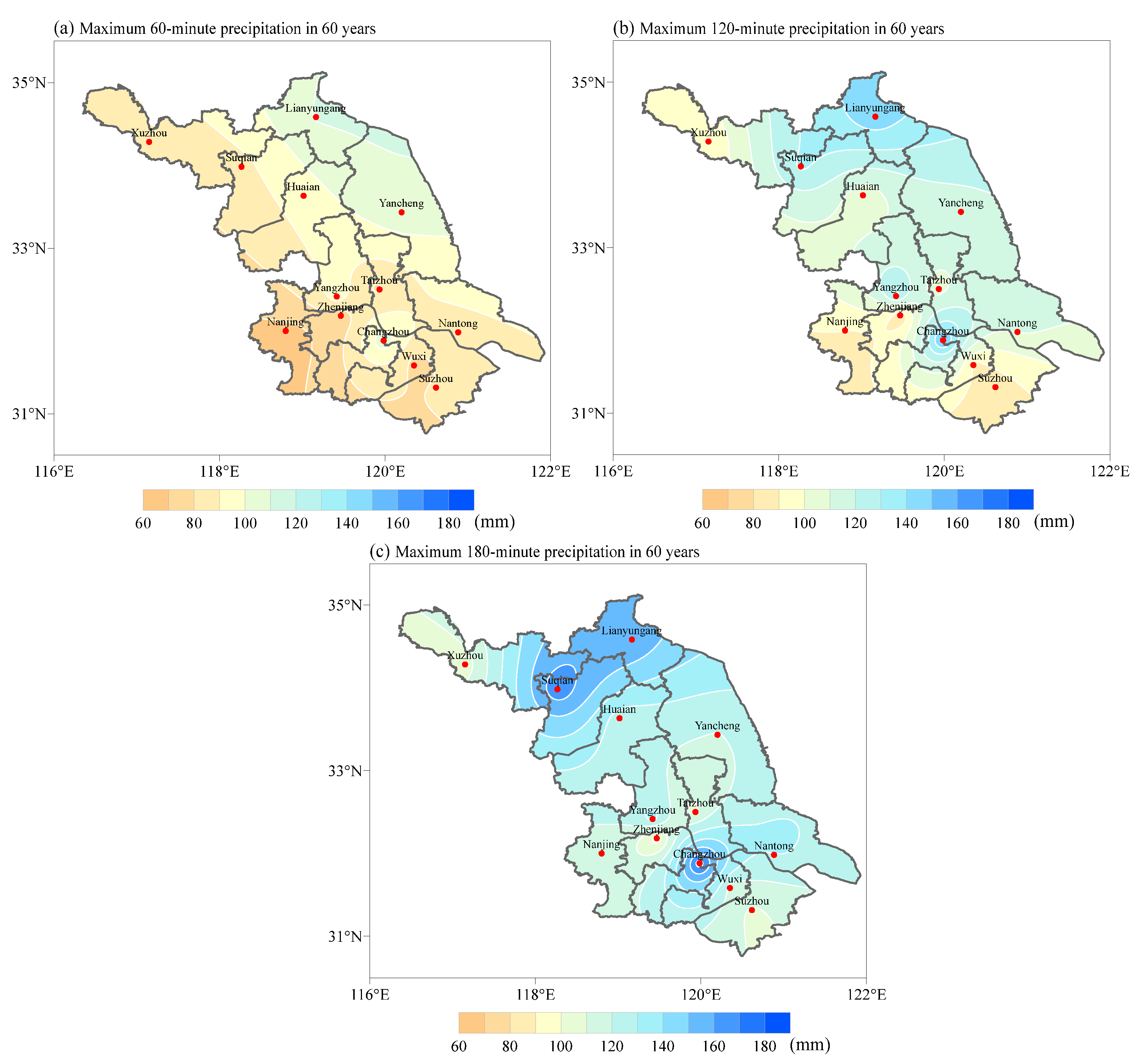
2.1. Data
2.2. Rainstorm Intensity–Duration–Frequency Formula
2.3. Chicago Hyetograph Method
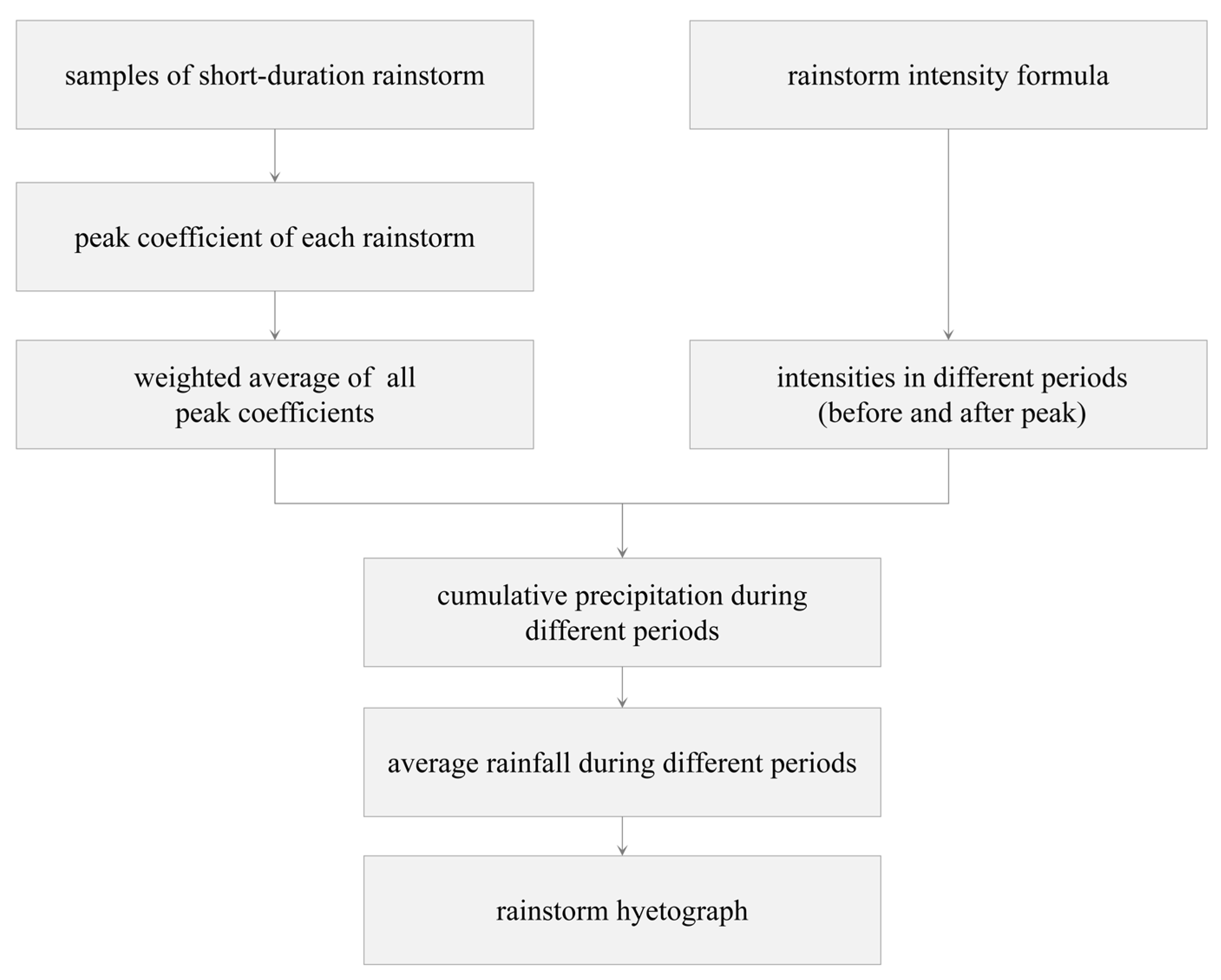
2.4. Pilgrim and Cordery Method
- A sample with a prolonged rainstorm is selected. Multiple rainfall events with the greatest amount of rainfall are selected; the more statistics, the clearer the statistical significance;
- The rain duration is divided into multiple time periods, the length of which are determined by the distribution time step required, which is often as minimal as possible. For instance, 120 min rainfall data were split every 5 min to infer a rainstorm pattern. The rainfall chosen in step 1 is split into 24 segments;
- The serial number of each time period is determined based on the rainfall from each time interval for each selected rainfall. High rainfall values correlate to low numbers, and the serial number of each time period is averaged. The values are ordered from low to high in accordance with the sequence of rainfall intensity;
- After sorting, the percentage of rainfall and total rainfall for each time period is computed, and the percentages for each time period are averaged;
- The time periods are ordered in accordance with the maximum possible order determined in the third stage and the distribution ratio determined in the fourth step to create a rainfall process line.
3. Results
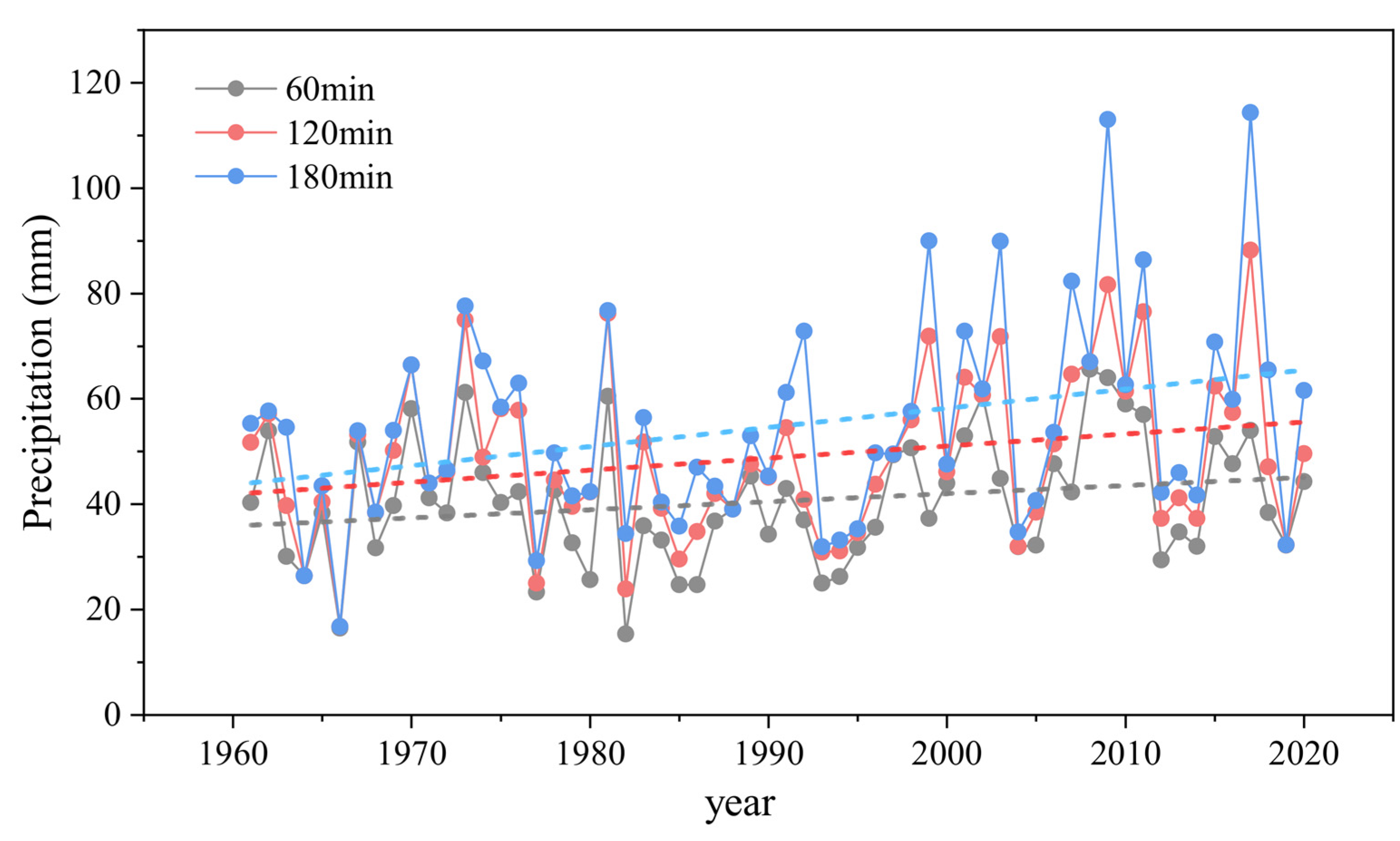
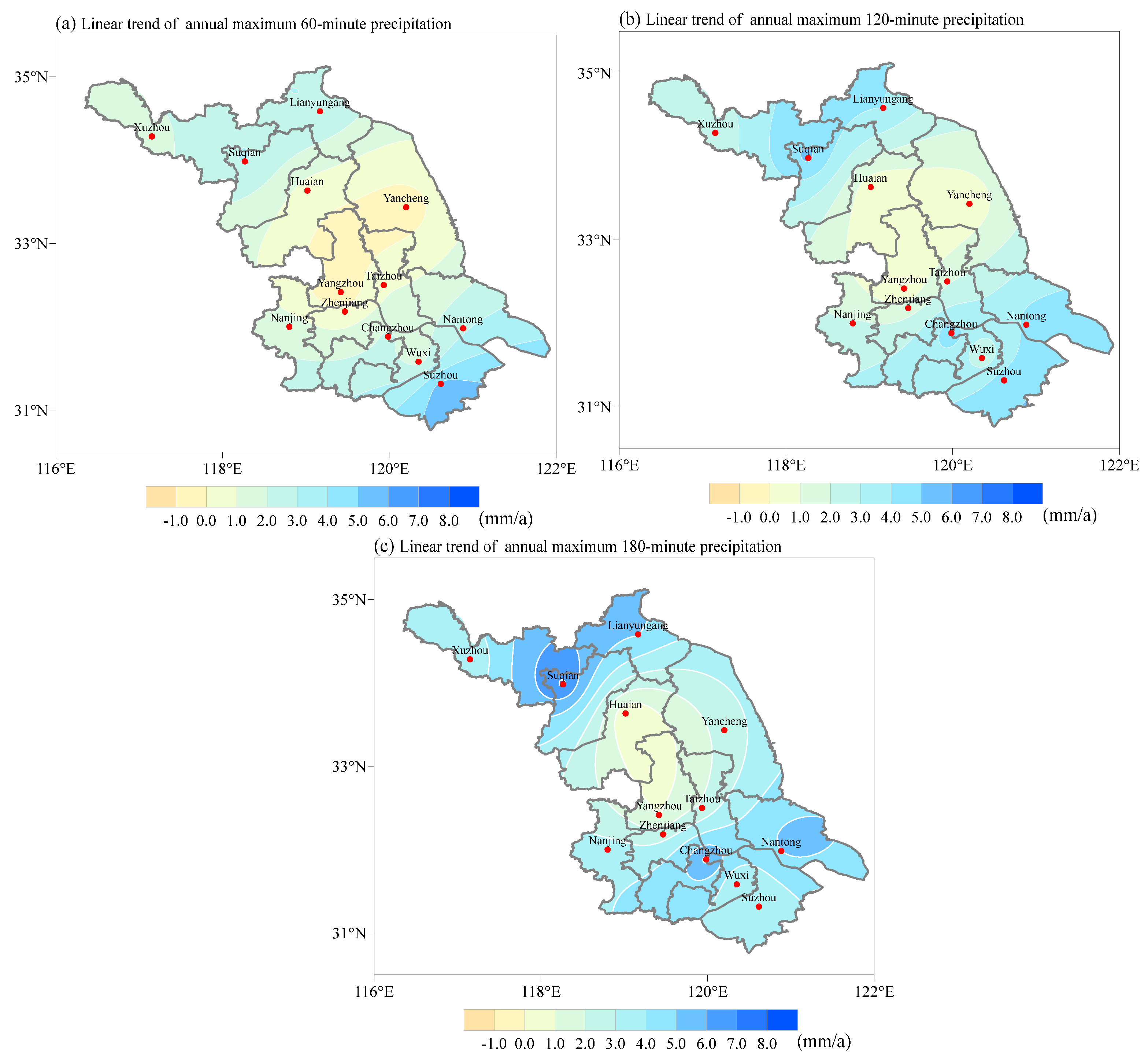
3.1. Interdecadal Variation in Rainfall Intensity
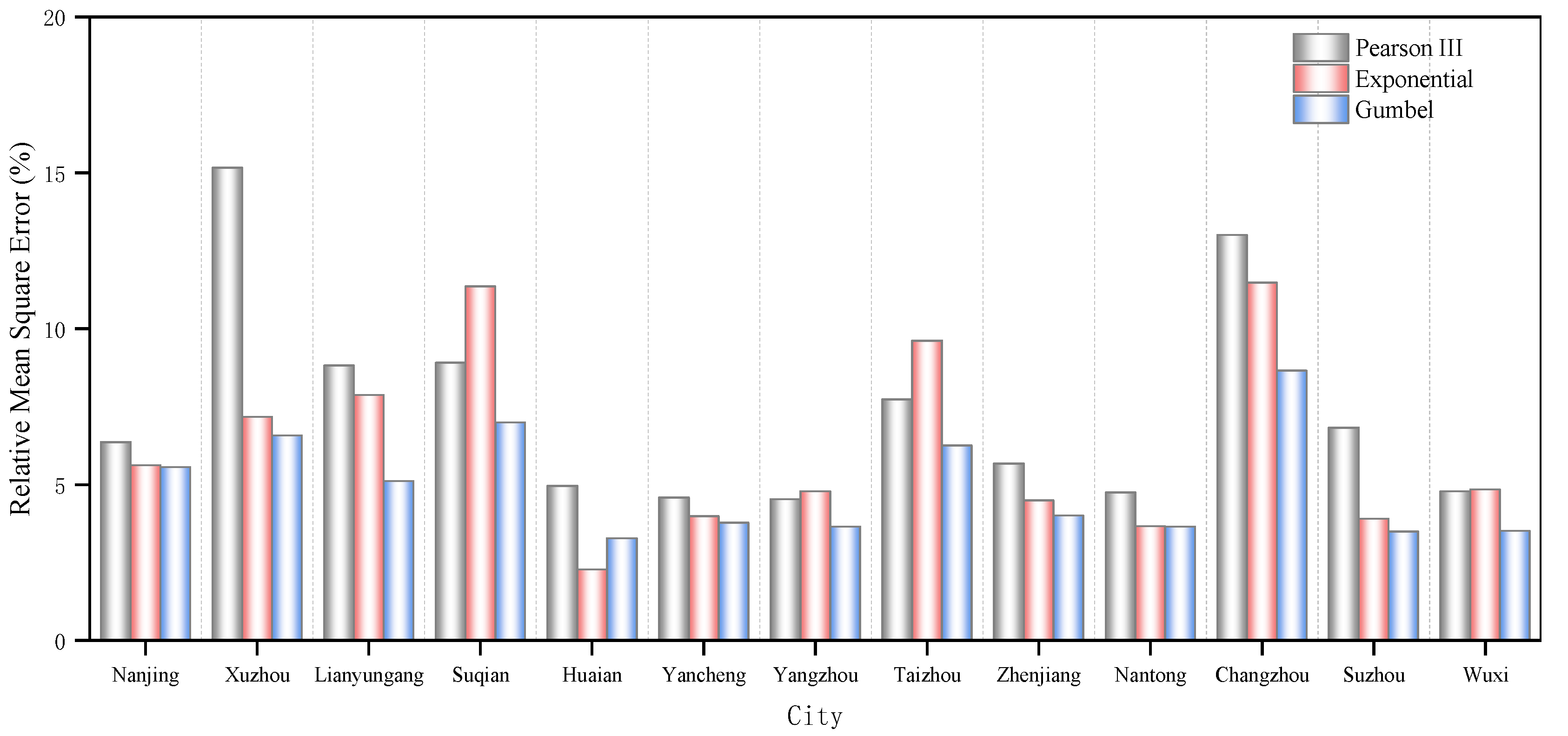
3.2. Revision of the Rainstorm Intensity Formula
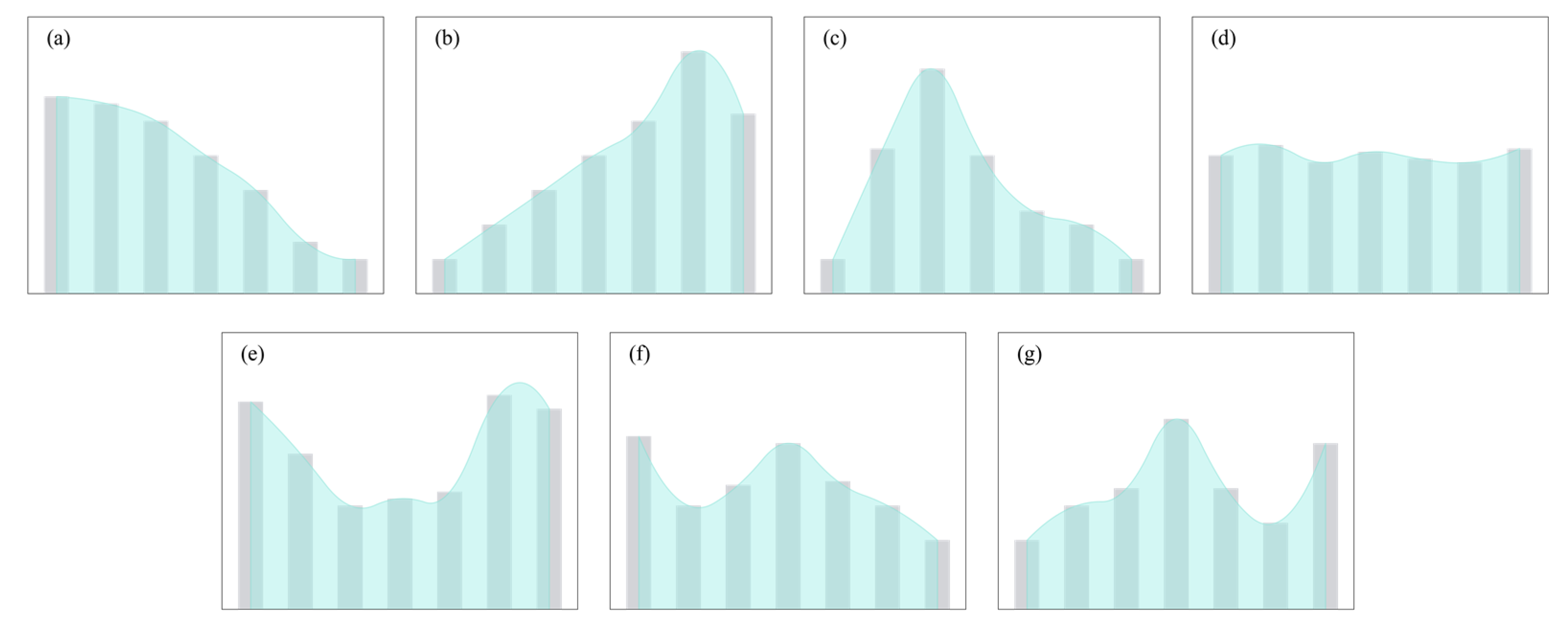
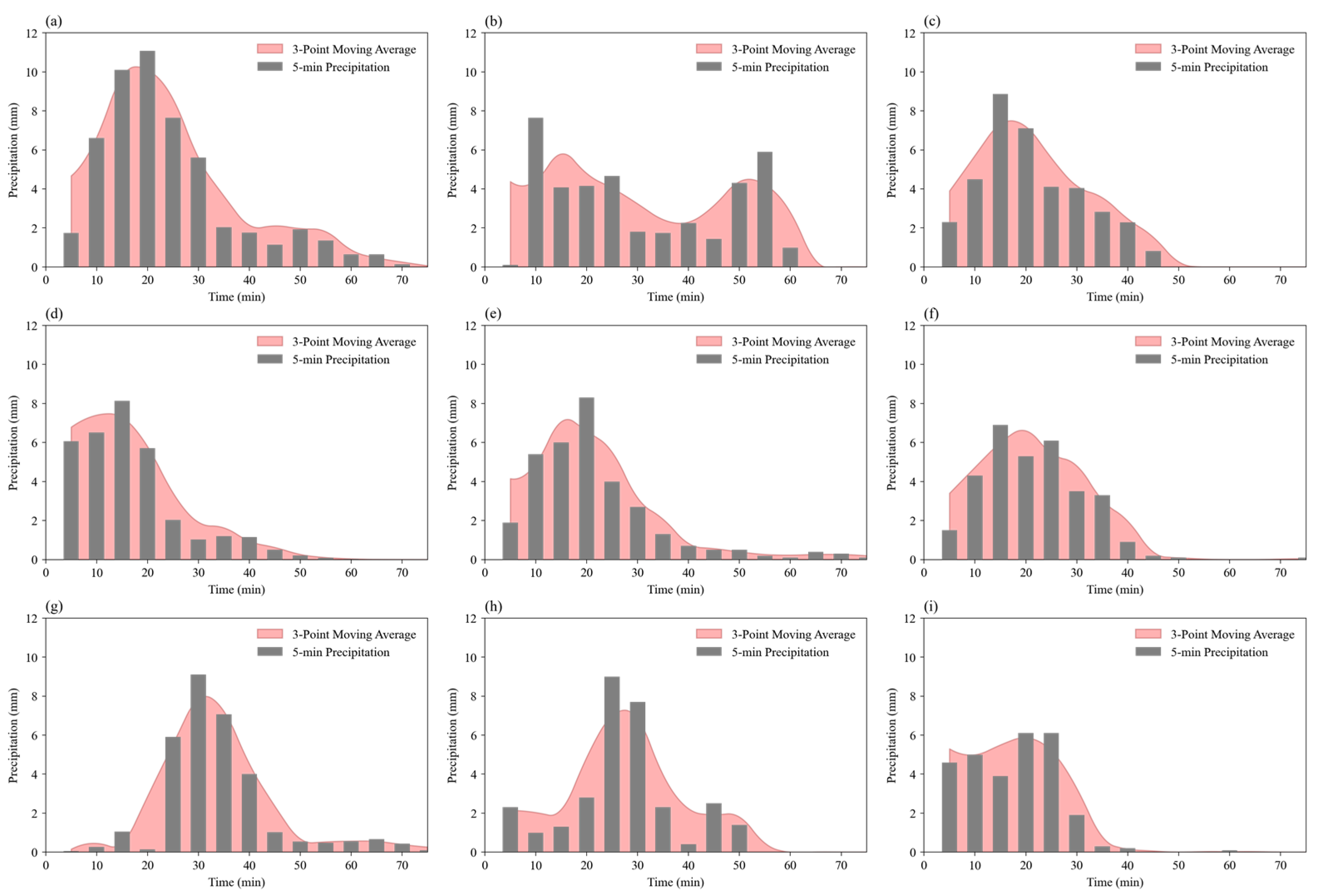
3.3. Rainfall Peak Coefficient of Short-Duration Rainstorm
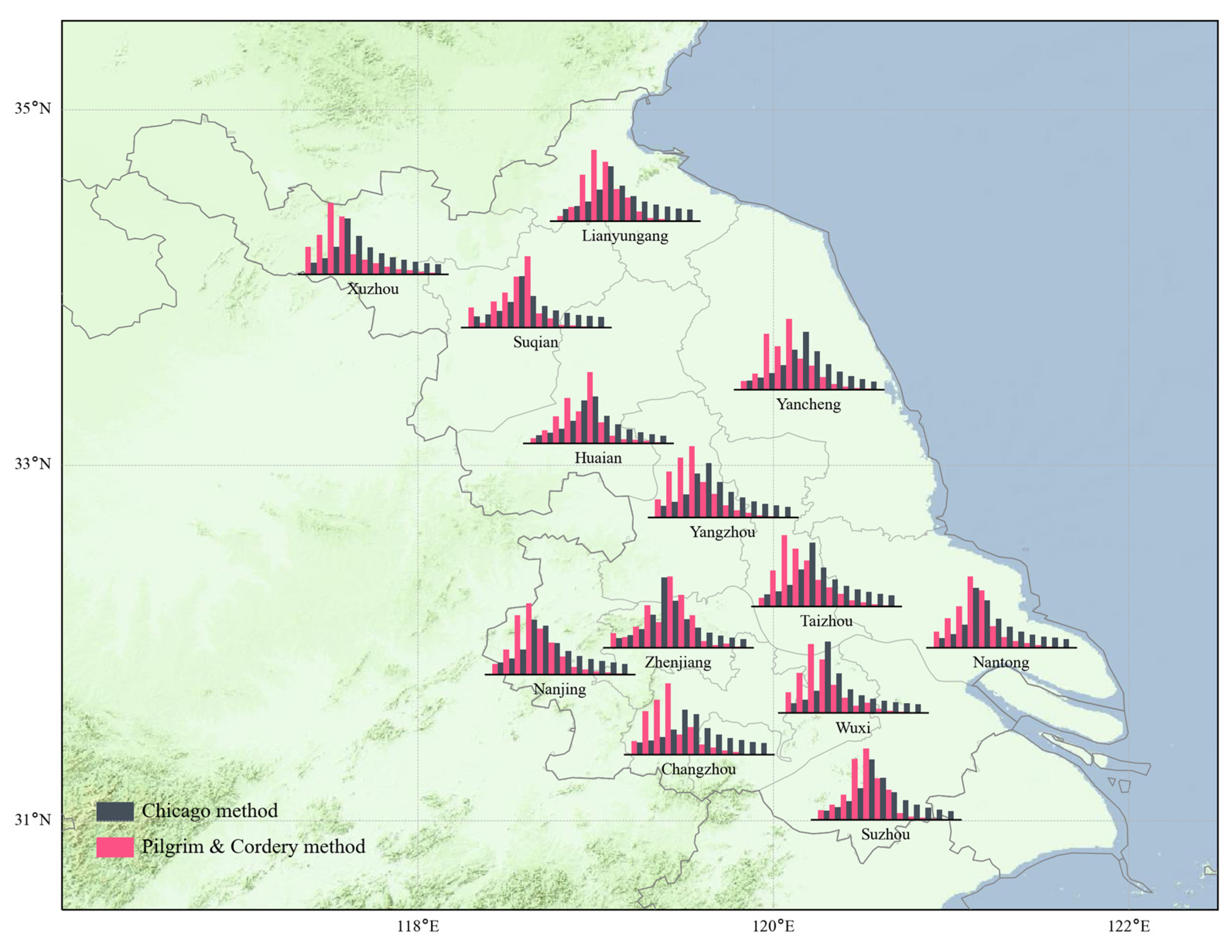
3.4. Designing Hyetographs of Short-Duration Rainstorms
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Guo, X.M.; Ren, G.Y.; Guo, Y.X.; Zhao, C.P. Influential Factors of City Water Logging and Meteorological Service. J. Catastrophology 2003, 23, 46–49. [Google Scholar]
- Li, W.T.; Sui, J.; Liu, C.L.; Niu, Y.; Zhou, J.H.; Tan, J.X. Analysis of Influence of Design Rainfall Peak Cofficient on Design Flow of Drainage Pipeline Network. Water Purif. Technol. 2015, 34, 100–103. [Google Scholar]
- Ma, J.J.; Song, L.L.; Zhang, X.J. The comparison of two different sampling schemes on design storm pattern by the Pilgrim & Cordery. Torrential Rain Disasters 2016, 35, 220–226. [Google Scholar]
- Ding, Y.H.; Ren, G.Y.; Shi, G.Y.; Gong, P. National Assessment Report of Climate Change (I): Climate change in China and its future trend. Adv. Clim. Chang. Res. 2006, 2, 3–8. [Google Scholar]
- Arnbjerg-Nielsen, K.; Willems, P.; Olsson, J.; Beecham, S.; Pathirana, A.; Bülow Gregersen, I.; Madsen, H.; Nguyen, V.T.V. Impacts of climate change on rainfall extremes and urban drainage systems: A review. Water Sci. Technol. 2013, 68, 16–28. [Google Scholar] [CrossRef]
- Zhai, P.M.; Li, L. Observed Atmosphere and Surface Climate Changes in the IPCC Fifth Assessment Report. Adv. Clim. Chang. Res. 2014, 10, 20–24. [Google Scholar]
- Qiu, Z.F.; Zhou, Q.; Zhang, Z.; Hao, Y.Q. Study on the derivation method of storm intensity formula. Urban Roads Bridges Flood Control 2004, 1, 47–49. [Google Scholar]
- Ren, M.; Hao, Y.; Chen, Y. Statistic and Analysis of Rainstorm Areas. J. Meteorol. Sci. 2007, 2, 214–219. [Google Scholar]
- Zhi, S.Q.; Song, L.L.; Luo, J.L.; Wu, K.J. Calculating System of Heavy Rain Intensity and Its Application. Meteorol. Mon. 2000, 26, 30–32. [Google Scholar]
- Xu, L.J.; Li, J.Q.; Li, T.; Liu, X.H. Research of the short duration storm intensity formula of Shanghai. China Munic. Eng. 2007, 4, 46–48. [Google Scholar]
- Wang, Z.H.; Hu, L.W.; Zhu, L.Y. Research and Application of the Derivation of Urban Storm Intensity Formula. Sci. Technol. Eng. 2012, 12, 4987–4991. [Google Scholar]
- Jin, J.M. Urban rainstorm intensity formula formulation and application methods. China Munic. Eng. 2010, 1, 38–41. [Google Scholar]
- Shao, Y.M.; He, M.J. Discussion on Some Problems about Urban Rainstorm Intensity Formula in Current Code. China Water Wastewater 2008, 24, 99–102. [Google Scholar]
- Gu, J.Q.; Chen, H.Y.; Xu, J.Y. Research on parameter estimate of probability distribution formula of storm intensity in Rui’an. J. Appl. Meteorol. Sci. 2000, 11, 355–363. [Google Scholar]
- Gu, J.Q.; Xu, J.Y.; Chen, H.Y.; Huang, J.P. Parameter estimation in torrential rain intensity formula with its application. J. Nanjing Inst. Meteorol. 2000, 23, 63–67. [Google Scholar]
- Chen, Z.; Wang, H.; Zhang, X. An Optimized Method for Estimating Parameters of the Rainstorm Intensity Formula. J. Appl. Meteorol. Sci. 2007, 18, 237–241. [Google Scholar]
- Cen, G.P.; Shen, J.; Fan, R.S. Research on Rainfall Pattern of Urban Design Storm. Adv. Water Sci. 1998, 9, 41–46. [Google Scholar]
- Mo, L.K. Stormwater Channels and Combined Waterways; Architectural Engineering Press: Beijing, China, 1956; pp. 17–19. [Google Scholar]
- Keifer, C.J.; Chu, H.H. Synthetic storm pattern for drainage design. J. Hydraul. Div. 1957, 83, 1–25. [Google Scholar] [CrossRef]
- Kibler, D.F. Urban Storm Water Hydrology; American Geophysical Union: Washington, DC, USA, 1982; Volume 7, pp. 48–60. [Google Scholar]
- Huff, F.A. Time distribution of rainfall in heavy storms. Water Resour. Res. 1967, 3, 1007–1019. [Google Scholar] [CrossRef]
- Pilgrim, D.H.; Cordery, I. Rainfall temporal patterns for design floods. J. Hydraul. Div. 1975, 101, 81–95. [Google Scholar] [CrossRef]
- Arnell, V.; Harremoës, P.; Jensen, M.; Johansen, N.B.; Niemczynowicz, J. Review of rainfall data application for design and analysis. Water Sci. Technol. 1984, 16, 1–45. [Google Scholar] [CrossRef]
- Deng, P.D. Establishment and Application of Mathematical Model for Storm Sampling And Frequency Distribution. Water Wastewater Eng. 1996, 22, 2–9. [Google Scholar]
- Wang, M.; Tan, X.C. A Study on Storm and Rainfall Pattern in Beijing City. J. China Hydrol. 1994, 3, 1–6. [Google Scholar]
- Cen, G.P. A Comparison of Design Storm Patterns for Calculating the Volume of Detention Ponding. Water Resour. Water Eng. 1993, 2, 30–35. [Google Scholar]
- Su, X.L.; Dong, X.; Lei, L.; Liang, C.R.; Liu, Z.R.; Li, Y.; Lan, Q.; Ren, L. Study on Design Rainstorm Profile in Liuzhou City Based on Pilgrim & Cordery Method. J. Geosci. Environ. Prot. 2019, 7, 136–144. [Google Scholar]
- Zhang, C.; Zhi, X.H.; Shi, H.; Zhu, G.H.; Chen, Y.; Chen, Y.; Chen, Y.L.; Xiao, J.; Shao, R.M.; Zhou, Y.W. Outdoors Drainage Criterion (GB50014-2006, 2011); China Planning Press: Beijing, China, 2011. [Google Scholar]
- Wu, X.J.; Fei, J.F.; Cheng, X.P.; Huang, X.G.; Lang, F.W. Diagnostic analysis on precipitation intensity of the rainstorm with “double peak”. J. Meteorol. Sci. 2014, 34, 252–260. [Google Scholar]
- Zhuang, Z.F.; Wang, K.Q.; Yang, J.; Chen, B.; Zhu, H.T. Research on new generation rainstorm intensity formula and design of rainfall hyetograph in Zhenjiang. J. Meteorol. Sci. 2015, 35, 506–513. [Google Scholar]
- Zhang, L.; Li, J.; Pei, H.Y.; He, G.X. Rainfall Pattern Analysis of Short Duration Rainstorm in Nanjing. Adv. Meteorol. Sci. Technol. 2019, 3, 15–20. [Google Scholar]
- Xie, D.; Su, X.L.; Liu, L.; Liang, C.R.; Liu, Z.R.; Li, Y.; Lan, Q.; Ren, L. Study on rainstorm type design of Liuzhou based on Chicago Method. J. Meteorol. Res. Appl. 2018, 39, 72–75. [Google Scholar]
- Yan, Z.X.; Xia, J.; Song, J.X.; Zhao, L.L.; Pang, G.W. Research progress on design hyetographs in small and medium-scale basins. Prog. Geogr. 2020, 39, 1224–1235. [Google Scholar] [CrossRef]
- Zhang, X.J. The Features of Summer Precipitation and the Influence of Design storm in Beijing Area; Nanjing University of Information Science & Technology: Nanjing, China, 2015. [Google Scholar]






| City | A1 | C | b | n |
|---|---|---|---|---|
| Xuzhou | 12.37 | 0.889 | 13.241 | 0.683 |
| Lianyungang | 5.93 | 1.687 | 10.205 | 0.594 |
| Suqian | 5.28 | 1.057 | 5.681 | 0.529 |
| Huai’an | 14.78 | 2.283 | 18.662 | 0.836 |
| Yancheng | 57.09 | 1.276 | 32.762 | 1.033 |
| Nanjing | 10.31 | 0.854 | 12.989 | 0.691 |
| Yangzhou | 15.87 | 0.847 | 18.979 | 0.767 |
| Taizhou | 11.38 | 0.784 | 12.805 | 0.686 |
| Zhenjiang | 18.69 | 0.913 | 14.257 | 0.815 |
| Nantong | 14.13 | 0.811 | 11 | 0.735 |
| Changzhou | 8.36 | 0.875 | 11.655 | 0.586 |
| Suzhou | 20.57 | 1.652 | 19.384 | 0.856 |
| Wuxi | 8.87 | 0.675 | 4.649 | 0.648 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yang, J.; Xiang, Y.; Xu, X.; Sun, J. Design Hyetograph for Short-Duration Rainstorm in Jiangsu. Atmosphere 2022, 13, 899. https://doi.org/10.3390/atmos13060899
Yang J, Xiang Y, Xu X, Sun J. Design Hyetograph for Short-Duration Rainstorm in Jiangsu. Atmosphere. 2022; 13(6):899. https://doi.org/10.3390/atmos13060899
Chicago/Turabian StyleYang, Jie, Ying Xiang, Xiazhen Xu, and Jiali Sun. 2022. "Design Hyetograph for Short-Duration Rainstorm in Jiangsu" Atmosphere 13, no. 6: 899. https://doi.org/10.3390/atmos13060899
APA StyleYang, J., Xiang, Y., Xu, X., & Sun, J. (2022). Design Hyetograph for Short-Duration Rainstorm in Jiangsu. Atmosphere, 13(6), 899. https://doi.org/10.3390/atmos13060899






