Depolarization Ratio for Randomly Oriented Ice Crystals of Cirrus Clouds
Abstract
1. Introduction
2. Scattering Matrix and Depolarization Ratio
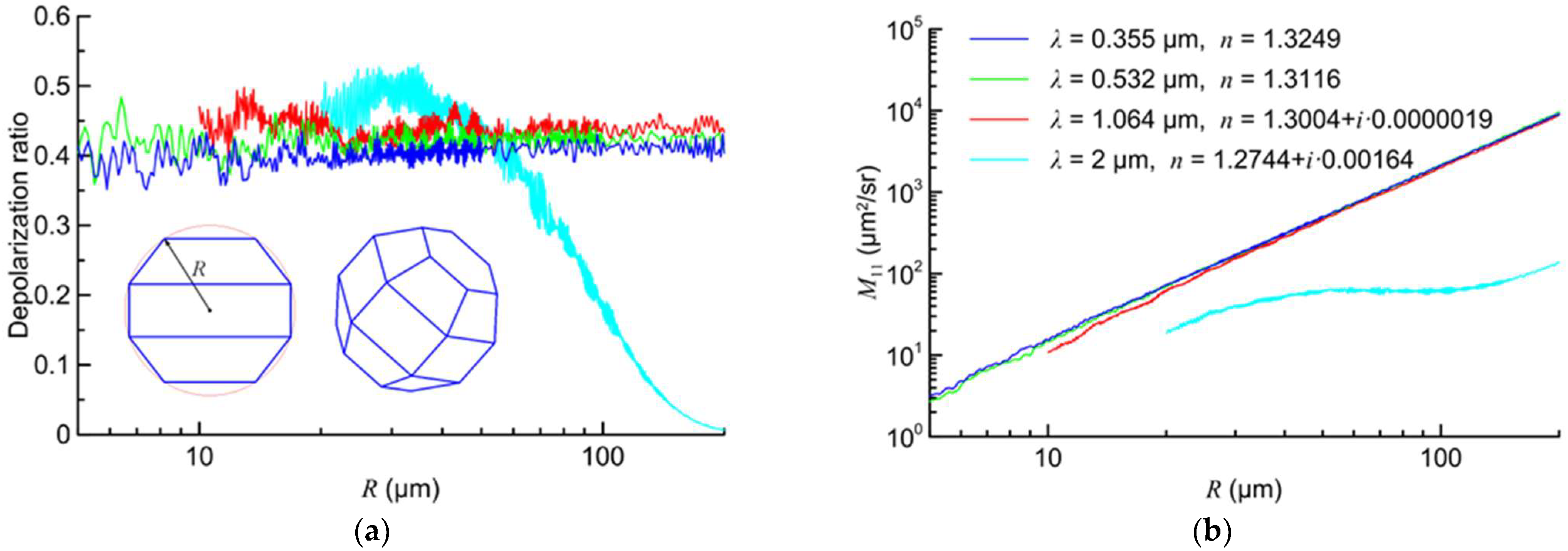
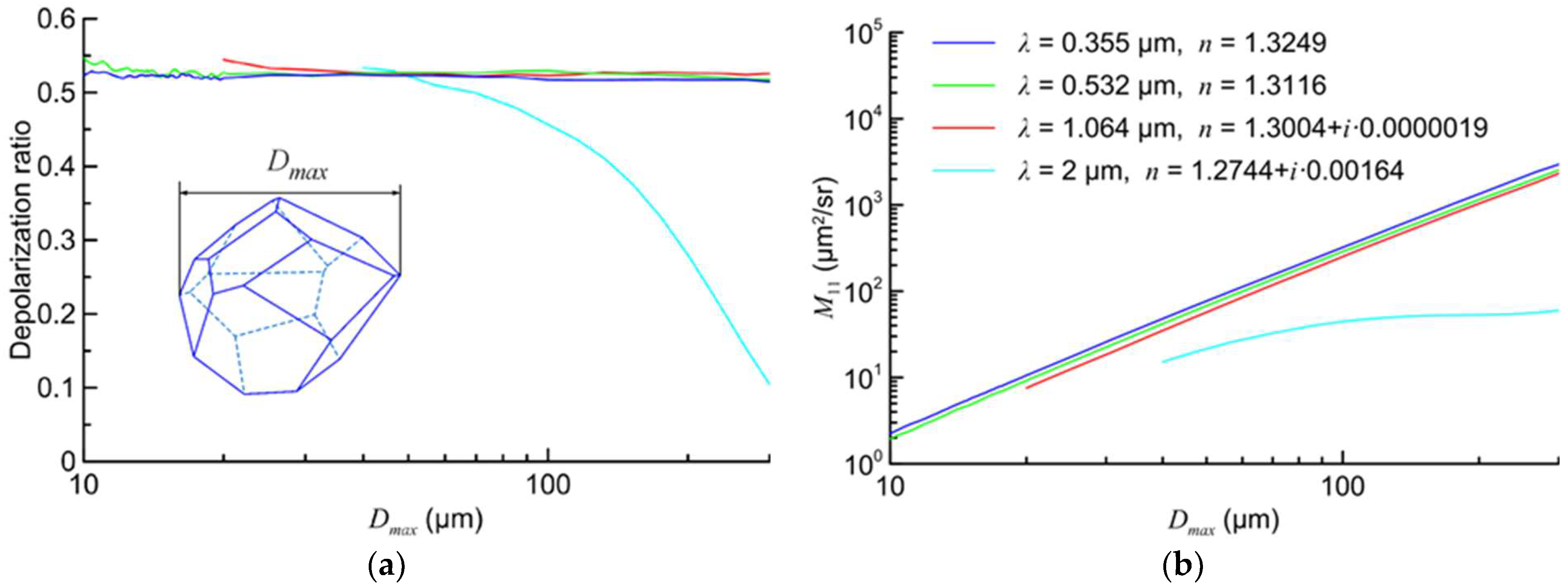
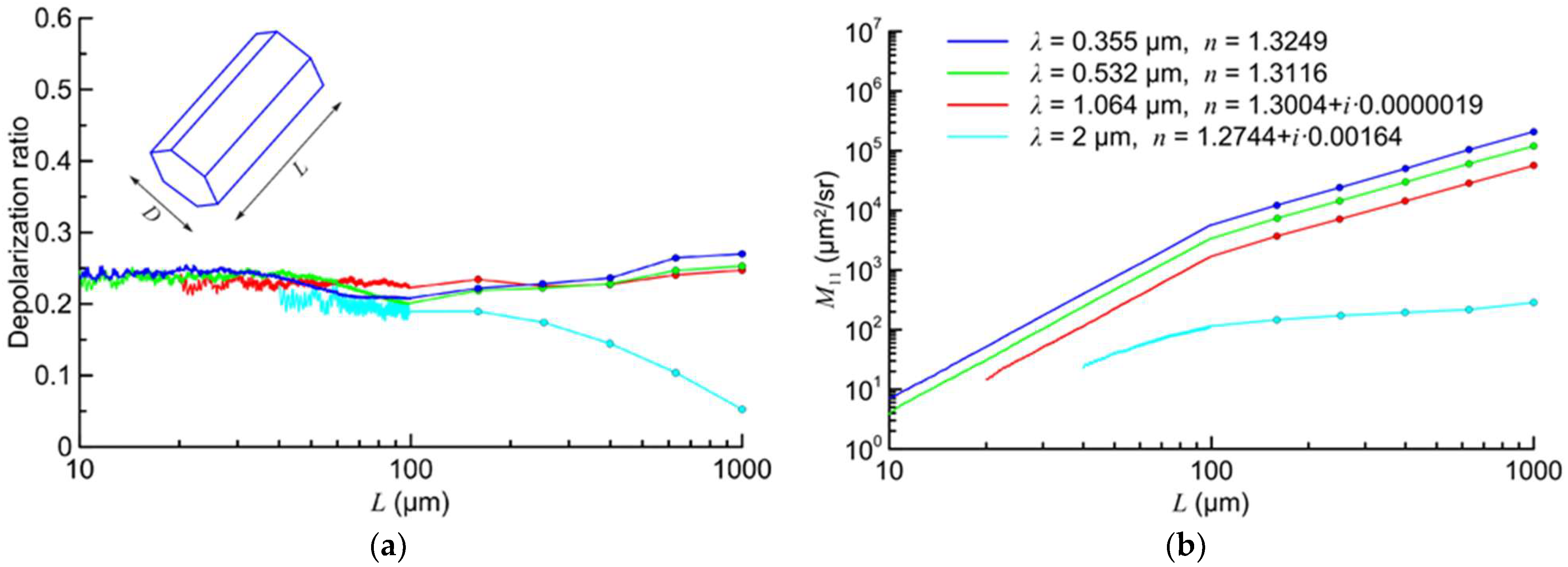
3. Depolarization Ratio vs. Crystal Size
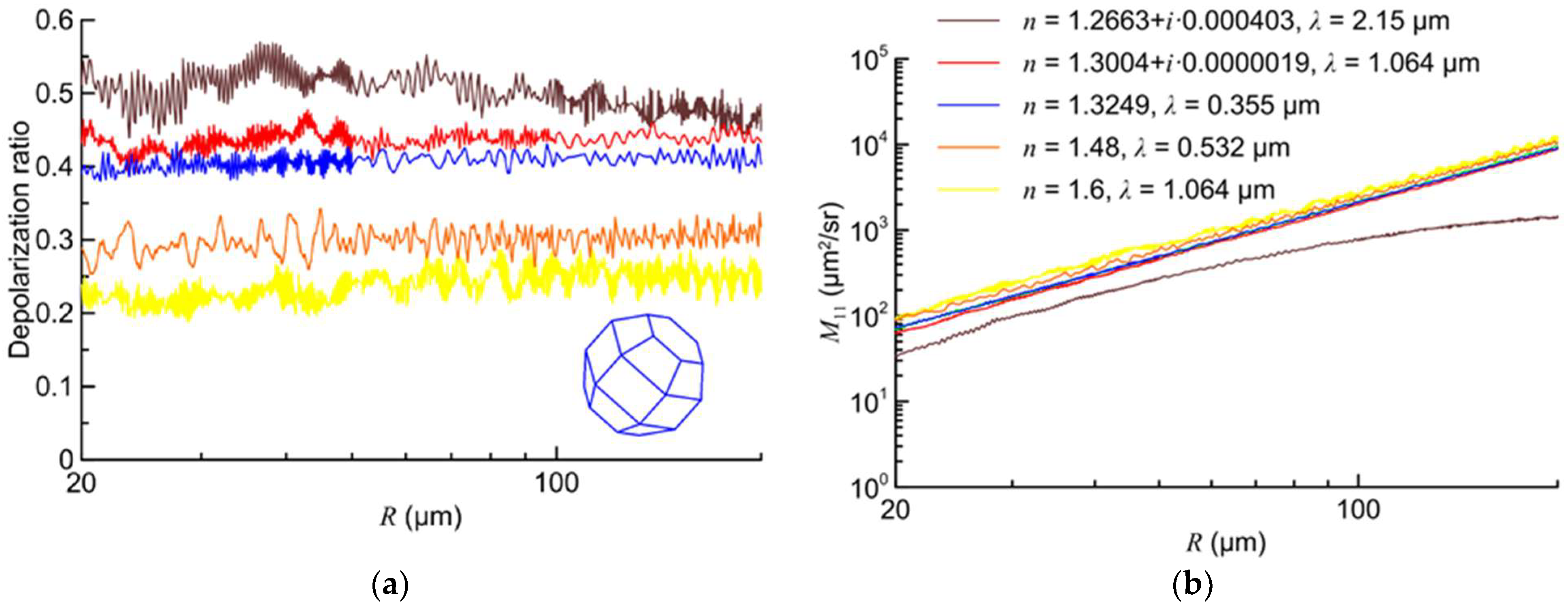
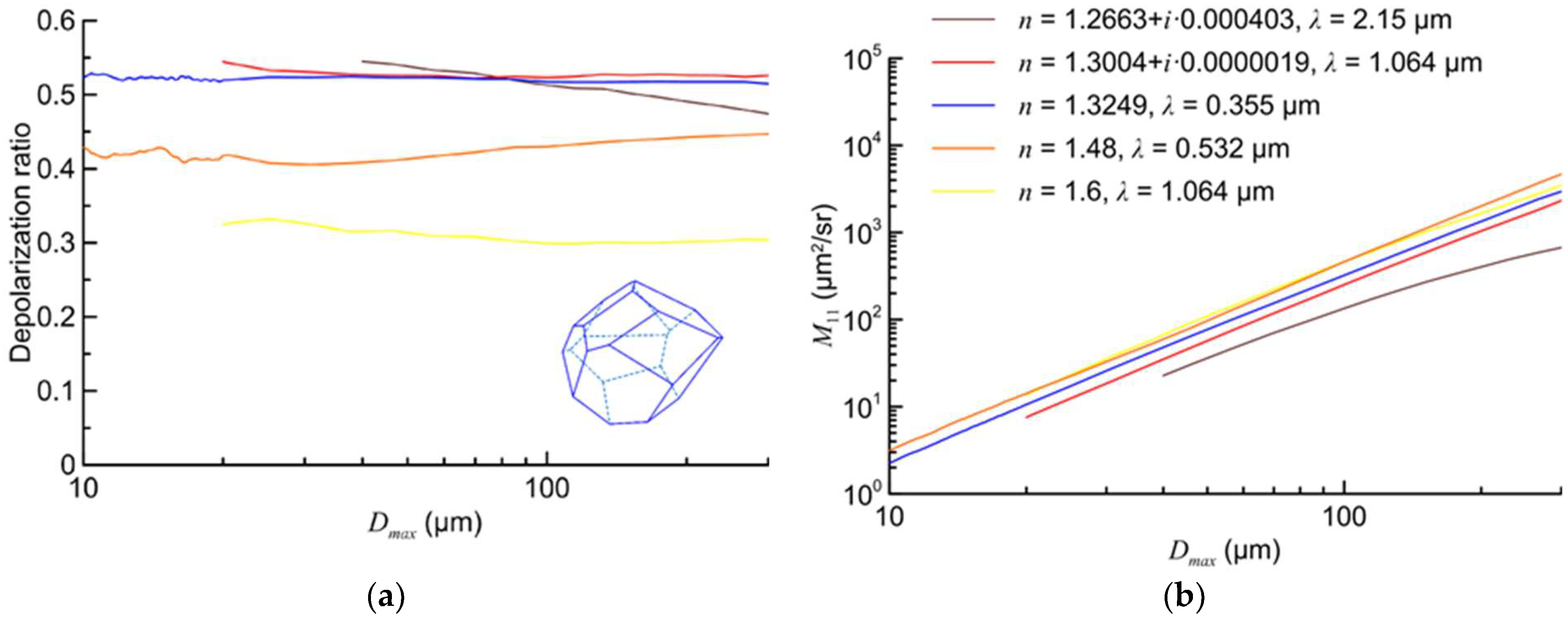
4. Depolarization Ratio for Hexagonal Columns and Plates
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Liou, K.-N.; Yang, P. Light Scattering by Ice Crystals. Fundamentals and Applications; Cambridge University Press: Cambridge, UK, 2016; p. 460. [Google Scholar]
- Tsekeri, A.; Amiridis, V.; Louridas, A.; Georgoussis, G.; Freudenthaler, V.; Metallinos, S.; Doxastakis, G.; Gasteiger, J.; Siomos, N.; Paschou, P.; et al. Polarization lidar for detecting dust orientation: System design and calibration. Atmos. Meas. Tech. 2021, 14, 7453–7474. [Google Scholar] [CrossRef]
- Sassen, K. The polarization lidar technique for cloud research: A review and current assessment. Bull. Am. Meteorol. Soc. 1991, 72, 1848–1866. [Google Scholar] [CrossRef]
- Sassen, K.; Cambell, J.R. A mid-latitude cirrus cloud climatology from the facility for atmospheric remote sensing: Part I. Mi-crophysical and synoptic properties. J. Atmos. Sci. 2001, 58, 481–496. [Google Scholar] [CrossRef]
- Sassen, K.; Comstock, J.M. A Midlatitude Cirrus Cloud Climatology from the Facility for Atmospheric Remote Sensing. Part III: Radiative Properties. J. Atmos. Sci. 2001, 58, 2113–2127. [Google Scholar] [CrossRef]
- Del Guasta, M. Simulation of LIDAR returns from pristine and deformed hexagonal ice prisms in cold cirrus by means of “face tracing”. J. Geophys. Res. Earth Surf. 2001, 106, 12589–12602. [Google Scholar] [CrossRef]
- Noel, V.; Chepfer, H.; Ledanois, G.; Delaval, A.; Flamant, P.H. Classification of particle effective shape ratios in cirrus clouds based on the lidar depolarization ratio. Appl. Opt. 2002, 41, 4245–4257. [Google Scholar] [CrossRef] [PubMed]
- Hu, Y. Depolarization ratio–effective lidar ratio relation: Theoretical basis for space lidar cloud phase discrimination. Geophys. Res. Lett. 2007, 34, 29584. [Google Scholar] [CrossRef]
- Wang, Z.; Sassen, K. Cirrus Cloud Microphysical Property Retrieval Using Lidar and Radar Measurements. Part II: Midlatitude Cirrus Microphysical and Radiative Properties. J. Atmos. Sci. 2002, 59, 2291–2302. [Google Scholar] [CrossRef]
- Seifert, P.; Ansmann, A.; Müller, D.; Wandinger, U.; Althausen, D.; Heymsfield, A.J.; Massie, S.T.; Schmitt, C. Cirrus optical properties observed with lidar, radio-sonde, and satellite over the tropical Indian Ocean during the aerosol-polluted north east and clean maritime south west monsoon. J. Geophys. Res. 2007, 112, D17205. [Google Scholar] [CrossRef]
- Hu, Y.; Vaughan, M.; McClain, C.; Behrenfeld, M.; Maring, H.; Anderson, D.; Sun-Mack, S.; Flittner, D.; Huang, J.; Wielicki, B.; et al. Global statistics of liquid water content and effective number concentration of water clouds over ocean derived from combined CALIPSO and MODIS measurements. Atmos. Chem. Phys. 2007, 7, 3353–3359. [Google Scholar] [CrossRef]
- Garnier, A.; Pelon, J.; Vaughan, M.A.; Winker, D.M.; Trepte, C.R.; Dubuisson, P. Lidar multiple scattering factors inferred from CALIPSO lidar and IIR retrivals of semitransparent cirrus cloud optical depths over oceans. Atmos. Meas. Tech. 2015, 8, 2759–2774. [Google Scholar] [CrossRef]
- Garnier, A.; Pelon, J.; Dubuisson, P.; Faivre, M.; Chomette, O.; Pascal, N.; Kratz, D.P. Retrieval of Cloud Properties Using CALIPSO Imaging Infrared Radiometer. Part I: Effective Emissivity and Optical Depth. J. Appl. Meteorol. Clim. 2012, 51, 1407–1425. [Google Scholar] [CrossRef]
- Zubko, E.; Shmirko, K.; Pavlov, A.; Sun, W.; Schuster, G.L.; Hu, Y.; Stamnes, S.; Omar, A.; Baize, R.R.; McCormick, M.P.; et al. Active remote sensing of atmospheric dust using relationships between their depolarization ratios and reflectivity. Opt. Lett. 2021, 46, 2352–2355. [Google Scholar] [CrossRef] [PubMed]
- Chen, W.-N.; Chiang, C.-W.; Nee, J.-B. Lidar ratio and depolarization ratio for cirrus clouds. Appl. Opt. 2002, 41, 6470–6476. [Google Scholar] [CrossRef] [PubMed]
- Sato, K.; Okamoto, H.; Ishimoto, H. Modeling the depolarization of space-borne lidar signals. Opt. Express 2019, 27, A117–A132. [Google Scholar] [CrossRef]
- Mishchenko, M.I.; Sassen, K. Depolarization of lidar returns by small ice crystals: An application to contrails. Geophys. Res. Lett. 1998, 25, 309–312. [Google Scholar] [CrossRef]
- Hayman, M.; Spuler, S.; Morley, B. Polarization lidar observations of backscatter phase matrices from oriented ice crystals and rain. Opt. Express 2014, 22, 16976–16990. [Google Scholar] [CrossRef]
- Hayman, M.; Spuler, S.; Morley, B.; VanAndel, J. Polarization lidar operation for measuring backscatter phase matrices of oriented scatterers. Opt. Express 2012, 20, 29553–29567. [Google Scholar] [CrossRef] [PubMed]
- Hayman, M.; Thayer, J.P. General description of polarization in lidar using Stokes vectors and polar decomposition of Mueller matrices. J. Opt. Soc. Am. A 2012, 29, 400–409. [Google Scholar] [CrossRef]
- Wang, W.; Yi, F.; Liu, F.; Zhang, Y.; Yu, C.; Yin, Z. Characteristics and seasonal variations of cirrus clouds from polarization lidar observations at a 30 degrees N plain site. Remote Sens. 2020, 12, 3998. [Google Scholar] [CrossRef]
- He, Y.; Liu, F.; Yin, Z.; Zhang, Y.; Zhan, Y.; Yi, F. Horizontally oriented ice crystals observed by the synergy of zenith- and slant-pointed polarization lidar over Wuhan (30.5 °N, 114.4 °E), China. J. Quant. Spectr. Radiat. Transf. 2021, 268, 107626. [Google Scholar] [CrossRef]
- Hu, Y.; Vaughan, M.; Liu, Z.; Lin, B.; Yang, P.; Flittner, D.; Hunt, B.; Kuehn, R.; Huang, J.; Wu, D.; et al. The depolarization–attenuated backscatter relation: CALIPSO lidar measurements vs. theory. Opt. Express 2007, 15, 5327–5332. [Google Scholar] [CrossRef] [PubMed]
- O’Connor, E.J.; Illingworth, A.J.; Hogan, R. A Technique for Autocalibration of Cloud Lidar. J. Atmos. Ocean. Technol. 2004, 21, 777–786. [Google Scholar] [CrossRef]
- Sassen, K.; Kayetha, V.K.; Zhu, J. Ice cloud depolarization for nadir and off-nadir CALIPSO measurements. Geophys. Res. Lett. 2012, 39, 53116. [Google Scholar] [CrossRef]
- Mishchenko, M.I.; Hovenier, J.W.; Travis, L.D. Light Scattering by Nonspherical Particles; Academic Press: Cambridge, MA, USA, 2000; p. 720. [Google Scholar]
- Masuda, K.; Ishimoto, H. Backscatter ratios for nonspherical ice crystals in cirrus clouds calculated by geomet-rical-optics-integral-equation method. J. Quant. Spectr. Radiat. Transf. 2017, 190, 60–68. [Google Scholar] [CrossRef]
- Bi, L.; Yang, P.; Kattawar, G.W.; Hu, Y.; Baum, B.A. Scattering and absorption of light by ice particles: Solution by a new physical-geometric optics hybrid method. J. Quant. Spectrosc. Radiat. Transf. 2011, 112, 1492–1508. [Google Scholar] [CrossRef]
- Konoshonkin, A.; Borovoi, A.; Kustova, N.; Okamoto, H.; Ishimoto, H.; Grynko, Y.; Förstner, J. Light scattering by ice crystals of cirrus clouds: From exact numerical methods to physical-optics approximation. J. Quant. Spectrosc. Radiat. Transf. 2017, 195, 132–140. [Google Scholar] [CrossRef]
- Konoshonkin, A.; Borovoi, A.; Kustova, N.; Reichardt, J. Power laws for backscattering by ice crystals of cirrus clouds. Opt. Express 2017, 25, 22341–22346. [Google Scholar] [CrossRef] [PubMed]
- Okamoto, H.; Sato, K.; Borovoi, A.; Ishimoto, H.; Masuda, K.; Konoshonkin, A.; Kustova, N. Interpretation of lidar ratio and depolarization ratio of ice clouds using spaceborne high-spectral-resolution polarization lidar. Opt. Express 2019, 27, 36587–36600. [Google Scholar] [CrossRef]
- Zhang, Z.; Yang, P.; Kattawar, G.W.; Tsay, S.-C.; Baum, B.A.; Huang, H.-L.; Hu, Y.X.; Heymsfield, A.J.; Reichardt, J. Geo-metrical-optics solution to light scattering by droxtal ice crystals. Appl. Opt. 2004, 43, 2490–2499. [Google Scholar] [CrossRef]
- Warren, S.G.; Brandt, R.E. Optical constants of ice from the ultraviolet to the microwave: A revised compilation. J. Geophys. Res. Earth Surf. 2008, 113, 9744. [Google Scholar] [CrossRef]
- Mitchell, D.L.; Arnott, W.P. A Model Predicting the Evolution of Ice Particle Size Spectra and Radiative Properties of Cirrus Clouds. Part II: Dependence of Absorption and Extinction on Ice Crystal Morphology. J. Atmos. Sci. 1994, 51, 817–832. [Google Scholar] [CrossRef]
- Borovoi, A.; Konoshonkin, A.; Kustova, N. Backscattering by hexagonal ice crystals of cirrus clouds. Opt. Lett. 2013, 38, 2881–2884. [Google Scholar] [CrossRef] [PubMed]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kustova, N.; Konoshonkin, A.; Shishko, V.; Timofeev, D.; Tkachev, I.; Wang, Z.; Borovoi, A. Depolarization Ratio for Randomly Oriented Ice Crystals of Cirrus Clouds. Atmosphere 2022, 13, 1551. https://doi.org/10.3390/atmos13101551
Kustova N, Konoshonkin A, Shishko V, Timofeev D, Tkachev I, Wang Z, Borovoi A. Depolarization Ratio for Randomly Oriented Ice Crystals of Cirrus Clouds. Atmosphere. 2022; 13(10):1551. https://doi.org/10.3390/atmos13101551
Chicago/Turabian StyleKustova, Natalia, Alexander Konoshonkin, Victor Shishko, Dmitry Timofeev, Ilya Tkachev, Zhenzhu Wang, and Anatoli Borovoi. 2022. "Depolarization Ratio for Randomly Oriented Ice Crystals of Cirrus Clouds" Atmosphere 13, no. 10: 1551. https://doi.org/10.3390/atmos13101551
APA StyleKustova, N., Konoshonkin, A., Shishko, V., Timofeev, D., Tkachev, I., Wang, Z., & Borovoi, A. (2022). Depolarization Ratio for Randomly Oriented Ice Crystals of Cirrus Clouds. Atmosphere, 13(10), 1551. https://doi.org/10.3390/atmos13101551





