Spatial and Temporal Characteristics of Rainfall Anomalies in 1961–2010 in the Yangtze River Basin, China
Abstract
:1. Introduction
2. Materials and Methods
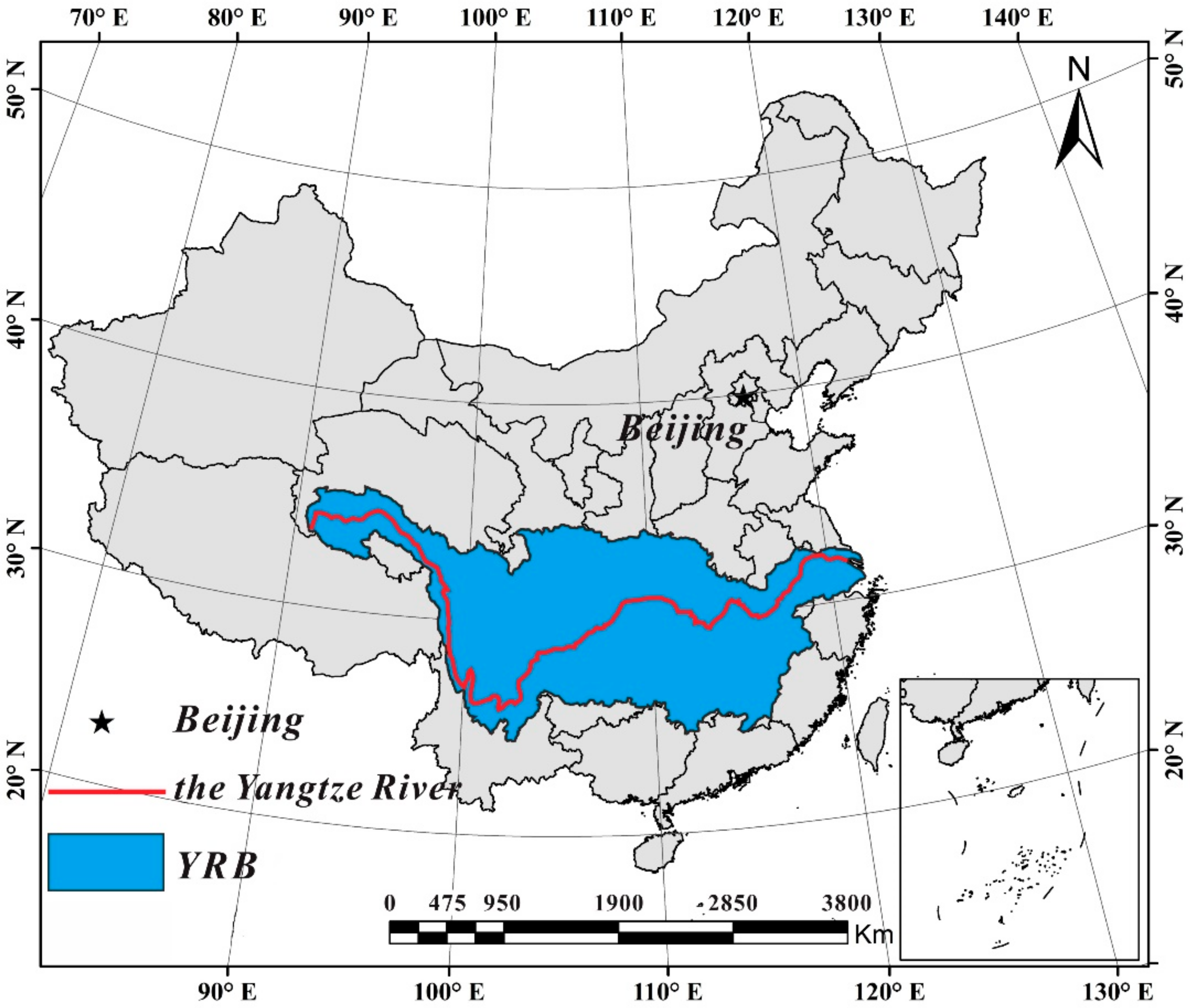
2.1. Study Area
2.2. Data Sources
- (1).
- Rainfall data
- (2).
- Elevation data
2.3. Data Pretreatments
2.4. Calculation Method
2.4.1. Areal Watershed Rainfall Calculation
2.4.2. Analysis of Abrupt Changes
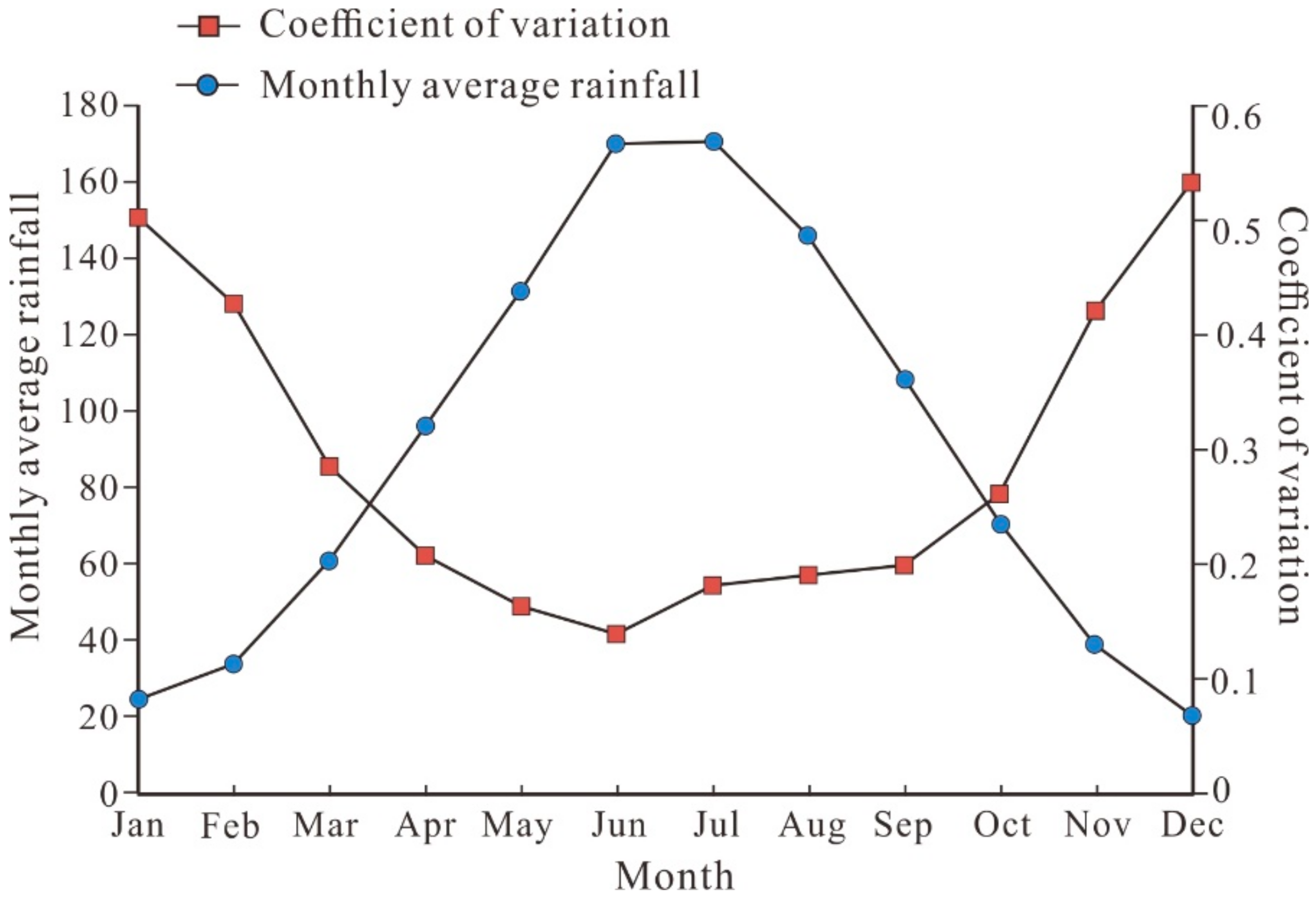
2.4.3. Coefficient of Variation for Monthly Rainfall
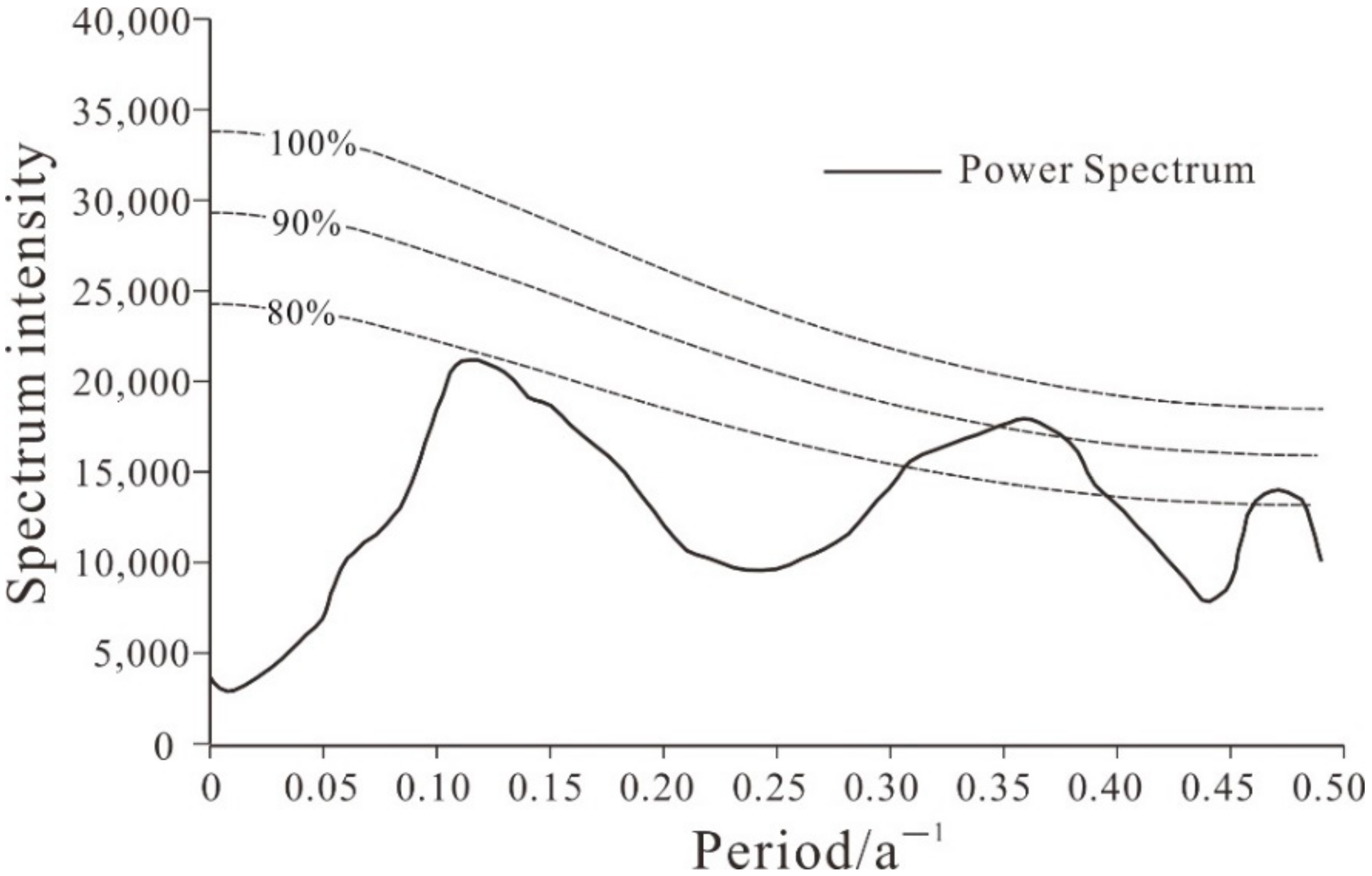
2.4.4. Analysis of the Annual Rainfall Variation Cycle
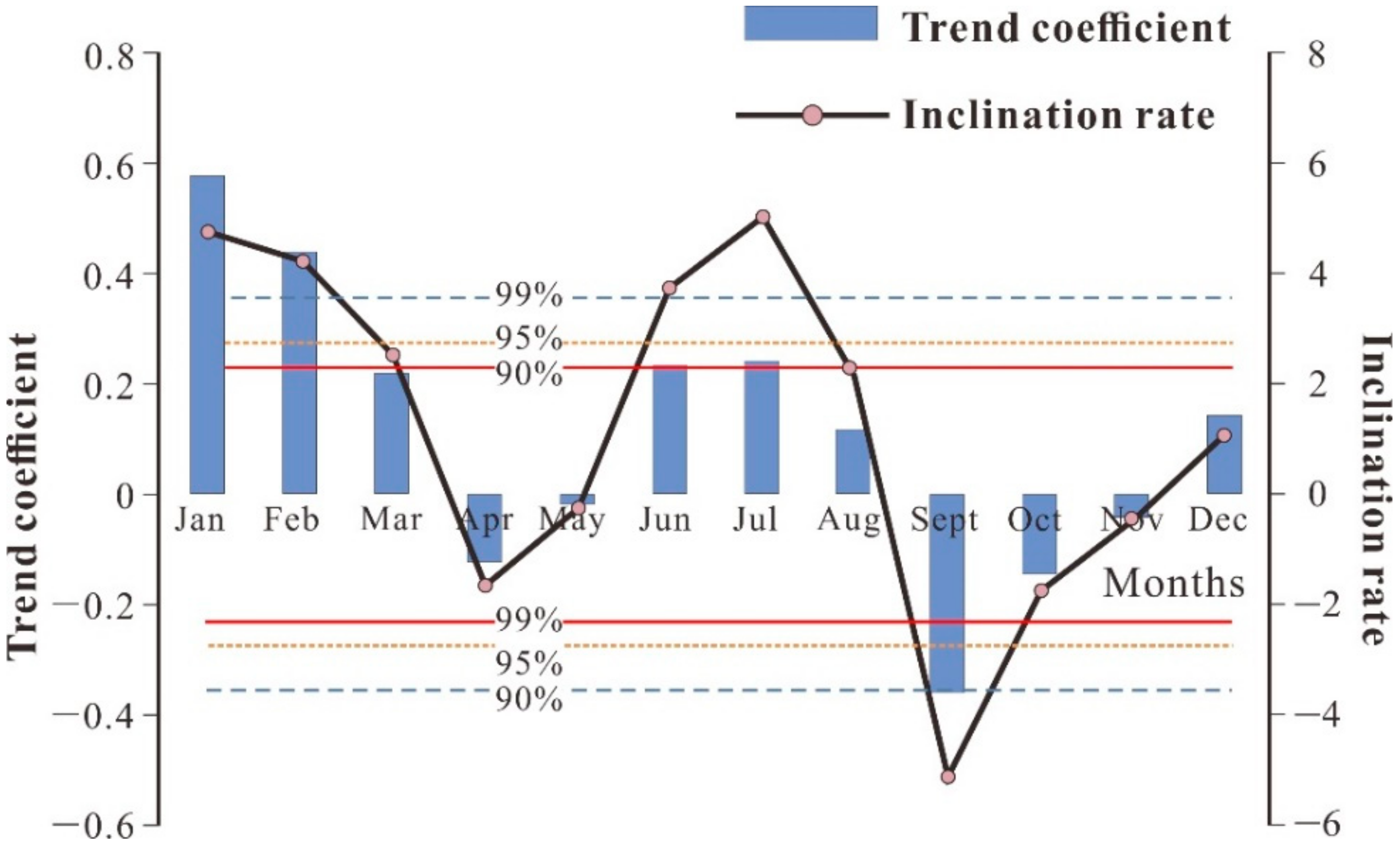
2.4.5. Mann–Kendall Trend Test for Annual Rainfall
3. Results and Discussion
3.1. Temporal Patterns of Rainfall in the YRB from 1961 to 2010
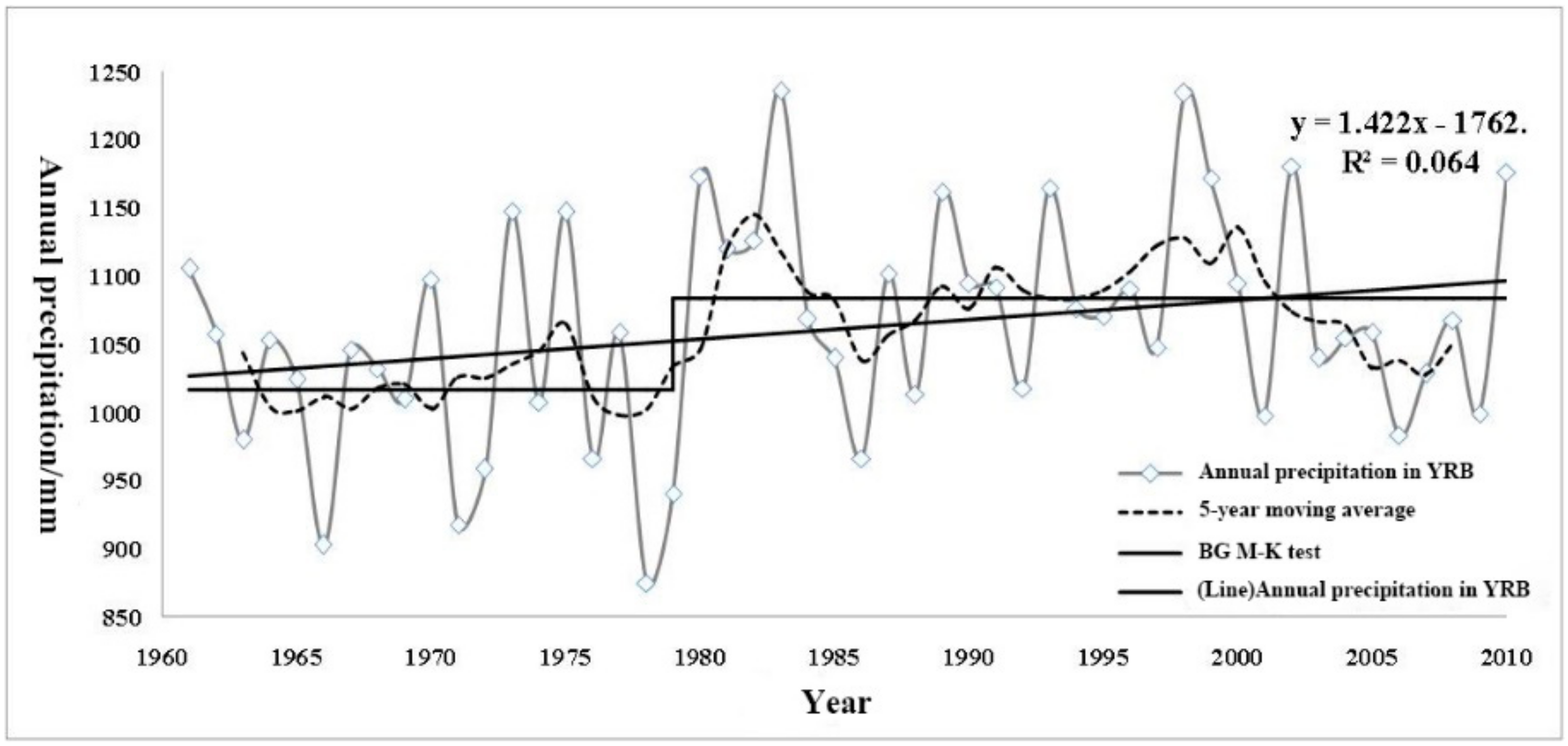
3.1.1. Annual Rainfall Variation
3.1.2. Seasonal Rainfall Variations
3.1.3. Monthly Rainfall Variations
3.2. Spatial Distribution Patterns of Rainfall in the YRB from 1961 to 2010
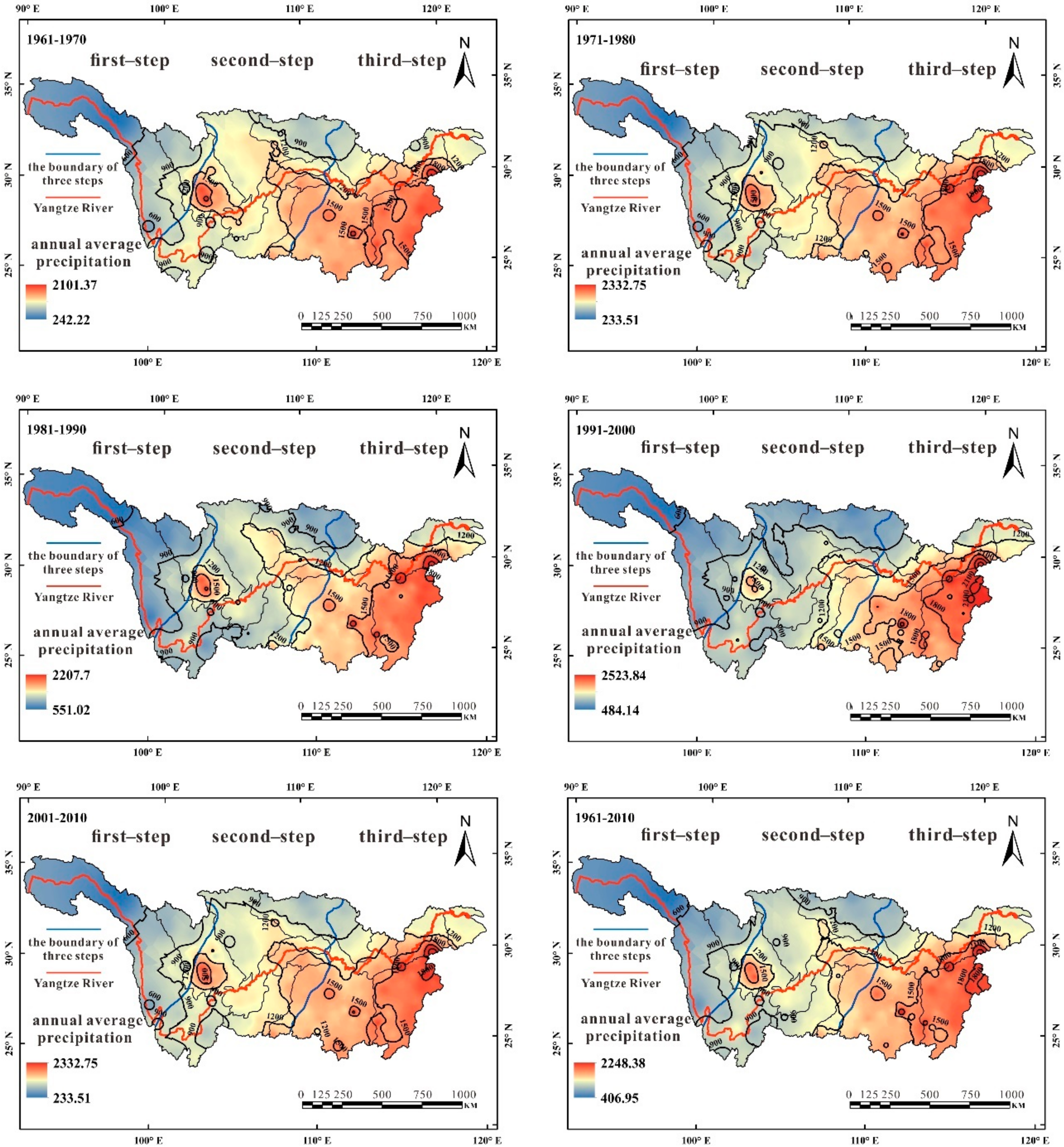
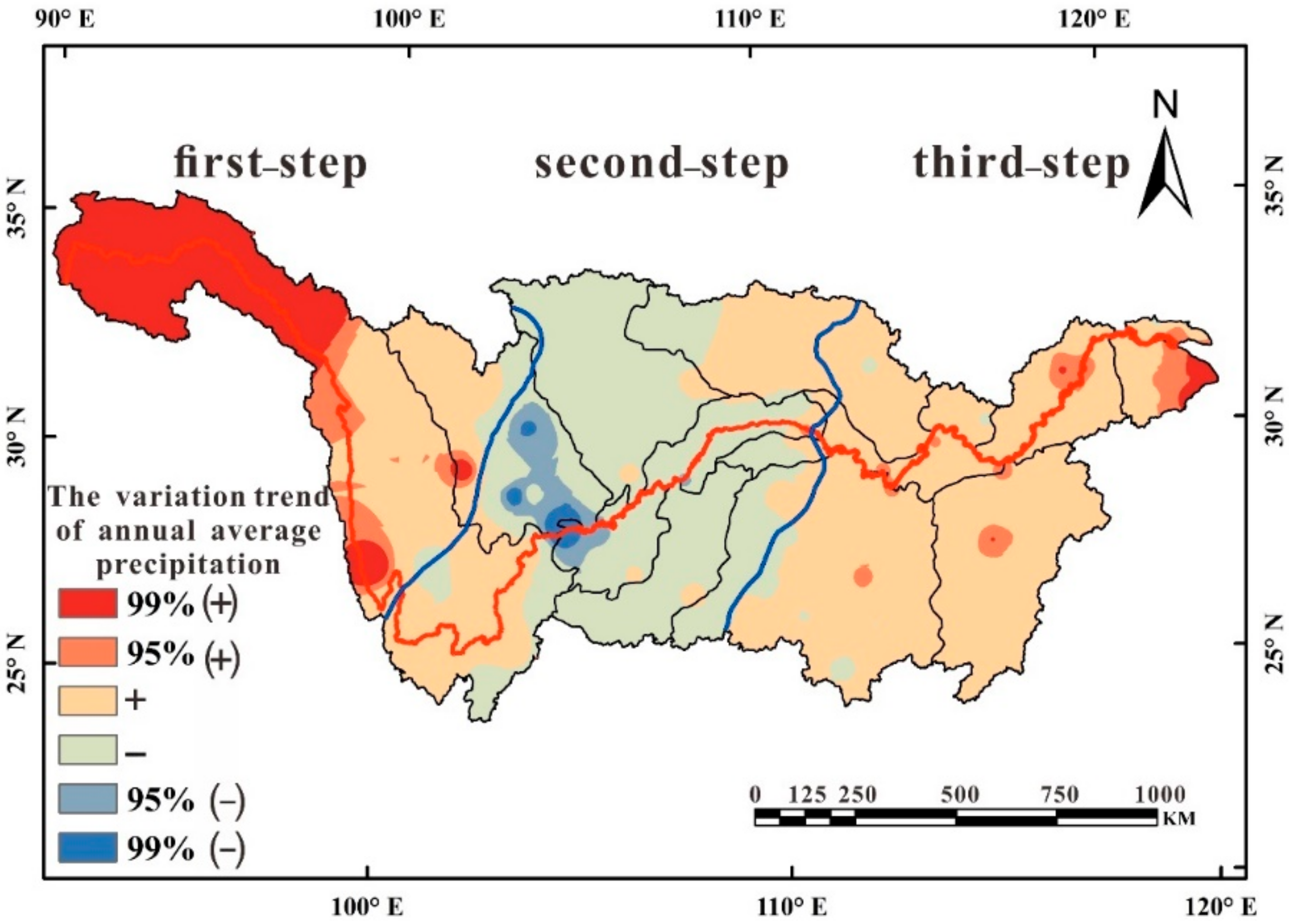
3.2.1. Spatial Distribution Patterns of Annual Rainfall
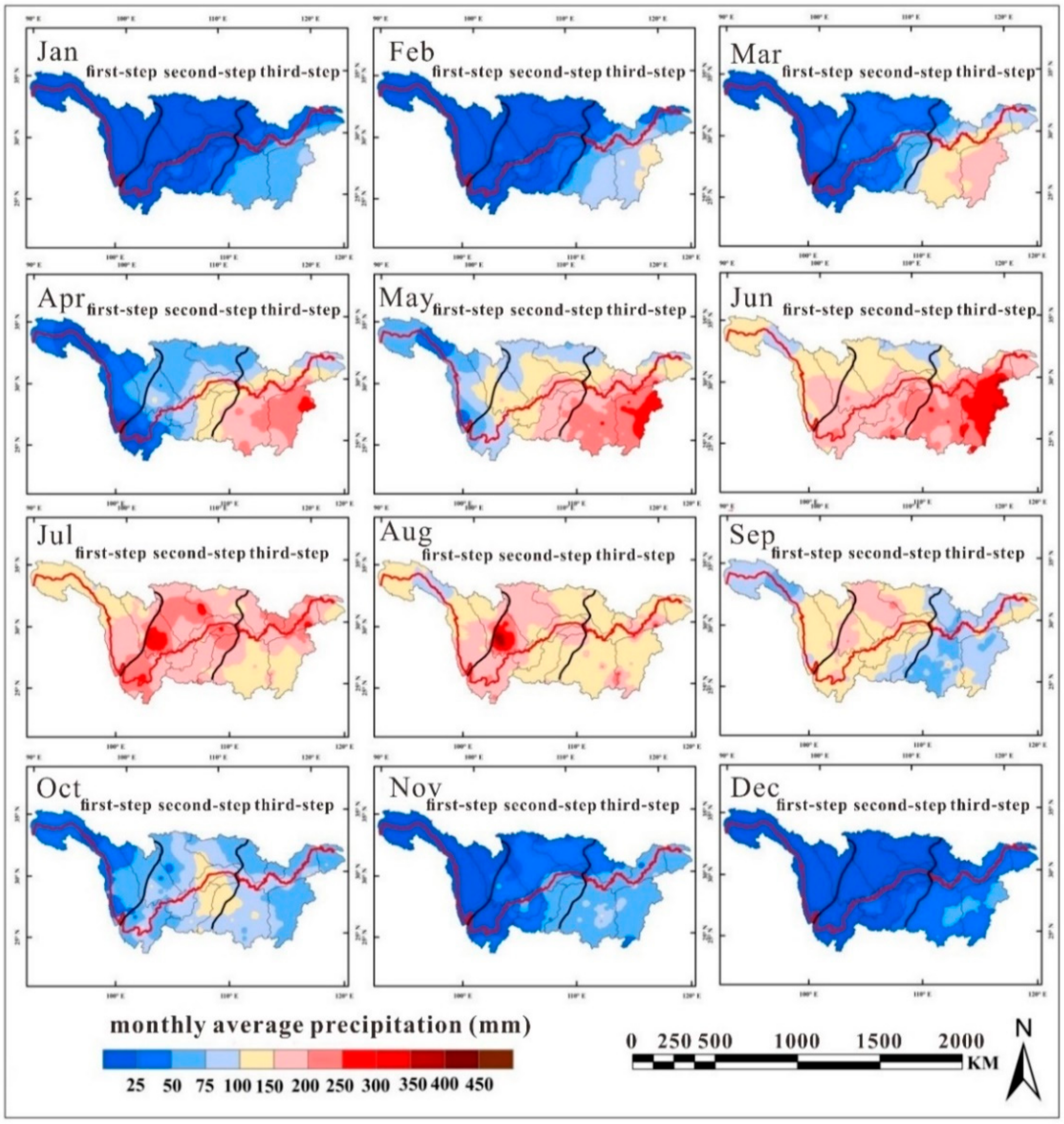
3.2.2. Spatial Distribution Characteristics of the Monthly Rainfall
3.3. Annual Rainfall Characteristics with Respect to Floods in the YRB
3.3.1. Annual and Seasonal Rainfall Anomalies in the YRB from 1961 to 2010
3.3.2. The Rainfall Anomalies in the Flood Years of the YRB
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Woldemeskel, F.; Sharma, A. Should flood regimes change in a warming climate? The role of antecedent moisture conditions. Geophys. Res. Lett. 2016, 43, 7556–7563. [Google Scholar] [CrossRef]
- Mayes, W.; Walsh, C.; Bathurst, J.; Kilsby, C.; Quinn, P.; Wilkinson, M.; Daugherty, A.; O’Connell, P. Monitoring a flood event in a densely instrumented catchment, the Upper Eden, Cumbria, UK. Water Environ. J. 2006, 20, 217–226. [Google Scholar] [CrossRef]
- Daksiya, V.; Su, H.T.; Chang, Y.H.; Lo, E. Incorporating socio-economic effects and uncertain rainfall in flood mitigation decision using MCDA. Nat. Hazards 2017, 87, 515–531. [Google Scholar] [CrossRef]
- Shen, H.; Yu, Z.; Yu, G.; Shi, X. Yu Grain Size and Pollen of Sediments in Wanghu Lake (Central China) Linked to Hydro-Environmental Changes. Water 2019, 12, 45. [Google Scholar] [CrossRef] [Green Version]
- Tarhule, A. Damaging Rainfall and Flooding: The Other Sahel Hazards. Clim. Chang. 2005, 72, 355–377. [Google Scholar] [CrossRef]
- Zeng, H.; Sun, X.; Lall, U.; Feng, P. Nonstationary extreme flood/rainfall frequency analysis informed by large-scale oceanic fields for Xidayang Reservoir in North China. Int. J. Clim. 2016, 37, 3810–3820. [Google Scholar] [CrossRef]
- Zhou, X.; Bai, Z.; Yang, Y. Linking trends in urban extreme rainfall to urban flooding in China. Int. J. Clim. 2017, 37, 4586–4593. [Google Scholar] [CrossRef]
- Wei, L.; Hu, K.-H.; Hu, X.-D. Rainfall occurrence and its relation to flood damage in China from 2000 to 2015. J. Mt. Sci. 2018, 15, 2492–2504. [Google Scholar] [CrossRef]
- Wang, W.; Shao, Q.; Peng, S.; Zhang, Z.; Xing, W.; An, G.; Yong, B. Spatial and temporal characteristics of changes in precipitation during 1957–2007 in the Haihe River basin, China. Stoch. Environ. Res. Risk Assess. 2011, 25, 881–895. [Google Scholar] [CrossRef]
- Chen, M.; Dong, Z.; Jia, W.; Ni, X.; Yao, H. Multi-Objective Joint Optimal Operation of Reservoir System and Analysis of Objectives Competition Mech-anism: A Case Study in the Upper Reach of the Yangtze River. Water 2019, 11, 2542. [Google Scholar] [CrossRef] [Green Version]
- Zhang, Z.; Gao, J.; Fan, X.; Lan, Y.; Zhao, M. Response of ecosystem services to socioeconomic development in the Yangtze River Basin, China. Ecol. Indic. 2017, 72, 481–493. [Google Scholar] [CrossRef]
- Zhang, Q.; Liu, C.; Xu, C.-Y.; Xu, Y.; Jiang, T. Observed trends of annual maximum water level and streamflow during past 130 years in the Yangtze River basin, China. J. Hydrol. 2006, 324, 255–265. [Google Scholar] [CrossRef]
- Buda, S.; Tong, J.; Guoyu, R.; Zhenghong, C. Observed trends of precipitation extremes in the Yangtze River basin during 1960 to 2004. Adv. Clim. Chang. Res. 2006, 2, 9–14. [Google Scholar]
- 1Zhang, Q.; Zhao, J. Causes of Floods in Past Fifty Years in Weihe River Basin and Their Control Measures. J. Desert Res. 2006, 26, 117–121. [Google Scholar]
- Zhang, L.-J.; Qian, Y.-F. A Study on the Feature of Precipitation Concentration and Its Relation to Flood-Producing in the Yangtze River Valley of China. Chin. J. Geophys. 2004, 47, 709–718. [Google Scholar] [CrossRef]
- Bai, A.; Liu, X. Characteristics of rainfall variation over east china during the last 50 years and their relationships with droughts and floods. J. Trop. Meteorol. 2010, 26, 194–200. [Google Scholar]
- Dore, M.H. Climate change and changes in global precipitation patterns: What do we know? Environ. Int. 2005, 31, 1167–1181. [Google Scholar] [CrossRef] [PubMed]
- Lin, Z.; Levy, J.K.; Xu, X.; Zhao, S.; Hartmann, J. Weather and seasonal climate prediction for flood planning in the Yangtze River Basin. Stoch. Environ. Res. Risk Assess. 2005, 19, 428–437. [Google Scholar] [CrossRef]
- Chen, W.; He, B.; Ma, J.; Wang, C. A WebGIS-based flood control management system for small reservoirs: A case study in the lower basin of the Yangtze River. J. Hydroinform. 2017, 19, 299–314. [Google Scholar] [CrossRef]
- Galván, P.; Ángel, B.; Ivanov, P.C.; Amaral, L.; Stanley, H.E. Scale Invariance in the Nonstationarity of Human Heart Rate. Phys. Rev. Lett. 2001, 87, 168105. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Robinson, P. Estimation of a time series model from unequally spaced data. Stoch. Process. Appl. 1977, 6, 9–24. [Google Scholar] [CrossRef] [Green Version]
- Mudelsee, M. TAUEST: A computer program for estimating persistence in unevenly spaced weather/climate time series. Comput. Geosci. 2002, 28, 69–72. [Google Scholar] [CrossRef] [Green Version]
- Lomb, N.R. Least-squares frequency analysis of unequally spaced data. Astrophys. Space Sci. 1976, 39, 447–462. [Google Scholar] [CrossRef]
- Xiao, S.; Xia, J.; Zou, L. Evaluation of Multi-Satellite Precipitation Products and Their Ability in Capturing the Character-istics of Extreme Climate Events over the Yangtze River Basin, China. Water 2020, 12, 1179. [Google Scholar] [CrossRef] [Green Version]
- Corbari, C.; Huber, C.; Yesou, H.; Huang, Y.; Su, Z.; Mancini, M. Multi-Satellite Data of Land Surface Temperature, Lakes Area, and Water Level for Hydrological Model Calibration and Validation in the Yangtze River Basin. Water 2019, 11, 2621. [Google Scholar] [CrossRef] [Green Version]
- Mason, S.; Goddard, L. Probabilistic Precipitation Anomalies Associated with ENSO. Bull. Am. Meteorol. Soc. 2001, 82, 619–638. [Google Scholar] [CrossRef] [Green Version]
- Huang, W.; Feng, Z. Comparative analysis of 2000 flood and 1996 flood of the Yangtze river. Yangtze River 2003, 34, 39–40. [Google Scholar]
| Name | Spring | Summer | Autumn | Winter |
|---|---|---|---|---|
| YRB | −0.63 | +10.00 * | −7.59 ** | +10.05 *** |
| Dongting Lake Basin | −11.13 | +14.98 | −14.28 * | +19.65 *** |
| Hanjiang River Basin | −6.42 | +15.62 * | −13.51 * | +9.64 *** |
| Jialing River Basin | −6.85 | −4.25 | −18.64 ** | +3.41 *** |
| Jinsha River Basin | +14.41 *** | +10.25 ** | +7.68 *** | +3.19 *** |
| Mintuojiang River Basin | +4.24 | −11.52 * | −9.35 ** | +0.25 |
| Poyang Lake Basin | −7.96 | +19.30 | −5.14 | +22.28 *** |
| Upper basin of the main stream | −4.38 | +5.10 | −16.12 *** | +2.80 * |
| Taihu Lake Basin | −7.93 | +38.05 ** | −19.55 ** | +27.67 *** |
| Wujiang River Basin | −7.05 | +6.99 | −15.10 *** | +4.70 ** |
| Lower basin of the main stream | −7.58 | +25.33 | −13.00 * | +25.29 *** |
| Middle basin of the main stream | −2.98 | +13.85 | −12.53 | +19.41 *** |
| Anomalously Abundant Years | Anomalously Scarce Years | |
|---|---|---|
| Spring | 1973(+), 1975(+), 1977(+), 1992(+), 2002(+) | 1979(−), 1986(−) |
| Summer | 1980(+), 1993(+), 1996(+), 1998(+), 1999(+) | 1972(−), 1978(−), 2006(−) |
| Autumn | 1975(+), 1982(+), 1983(+) | 1992(−), 1998(−), 2007(−), 2009(−), |
| Winter | 1989(+), 1992(+), 1994(+), 1997(+), 2002(+) | 1962(−), 1967(−), 1976(−) |
| Annual | 1983(+), 1998(+), 2002(+) | 1966(−), 1971(−), 1978(−), |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bai, S.; Gao, J.; Xue, Y.; Mansour, R. Spatial and Temporal Characteristics of Rainfall Anomalies in 1961–2010 in the Yangtze River Basin, China. Atmosphere 2021, 12, 960. https://doi.org/10.3390/atmos12080960
Bai S, Gao J, Xue Y, Mansour R. Spatial and Temporal Characteristics of Rainfall Anomalies in 1961–2010 in the Yangtze River Basin, China. Atmosphere. 2021; 12(8):960. https://doi.org/10.3390/atmos12080960
Chicago/Turabian StyleBai, Shuying, Jixi Gao, Yu Xue, and Romany Mansour. 2021. "Spatial and Temporal Characteristics of Rainfall Anomalies in 1961–2010 in the Yangtze River Basin, China" Atmosphere 12, no. 8: 960. https://doi.org/10.3390/atmos12080960
APA StyleBai, S., Gao, J., Xue, Y., & Mansour, R. (2021). Spatial and Temporal Characteristics of Rainfall Anomalies in 1961–2010 in the Yangtze River Basin, China. Atmosphere, 12(8), 960. https://doi.org/10.3390/atmos12080960







