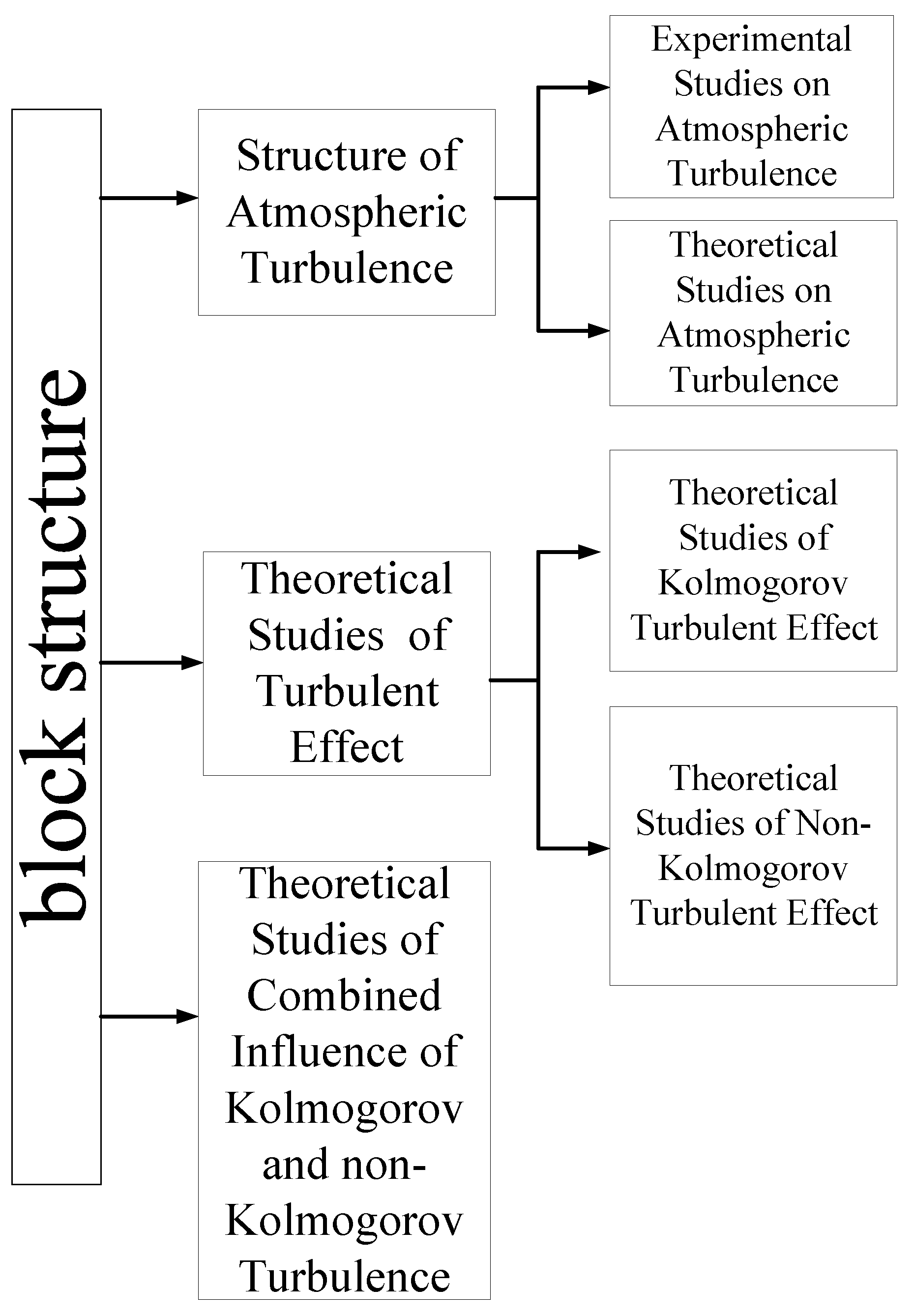
A Survey of Structure of Atmospheric Turbulence in Atmosphere and Related Turbulent Effects

Abstract

The Earth’s atmosphere is the living environment in which we live and cannot escape. Atmospheric turbulence is a typical random inhomogeneous medium, which causes random fluctuations of both the amplitude and phase of optical wave propagating through it. Currently, it is widely accepted that there exists two kinds of turbulence in the aerosphere: one is Kolmogorov turbulence, and the other is non-Kolmogorov turbulence, which have been confirmed by both increasing experimental evidence and theoretical investigations. The results of atmospheric measurements have shown that the structure of atmospheric turbulence in the Earth’s atmosphere is composed of Kolmogorov turbulence at lower levels and non-Kolmogorov turbulence at higher levels. Since the time of Newton, people began to study optical wave propagation in atmospheric turbulence. In the early stage, optical wave propagation in Kolmogorov atmospheric turbulence was mainly studied and then optical wave propagation in non-Kolmogorov atmospheric turbulence was also studied. After more than half a century of efforts, the study of optical wave propagation in atmospheric turbulence has made great progress, and the theoretical results are also used to guide practical applications. On this basis, we summarize the development status and latest progress of propagation theory in atmospheric turbulence, mainly including propagation theory in conventional Kolmogorov turbulence and one in non-Kolmogorov atmospheric turbulence. In addition, the combined influence of Kolmogorov and non-Kolmogorov turbulence in Earth’s atmosphere on optical wave propagation is also summarized. This timely summary is very necessary and is of great significance for various applications and development in the aerospace field, where the Earth’s atmosphere is one part of many links.

1. Introduction

It is well known that the Earth’s atmosphere is a typical random inhomogeneous medium that contains two kinds of medium: one is a discrete turbid atmospheric medium composed of particles, and the other is a “continuous” turbulent atmospheric medium composed of thermally moving molecules. The first medium causes the energy attenuation of optical waves, namely the attenuation effect. The second medium causes atmospheric turbulence effects such as intensity scintillation, angle-of-arrival fluctuations, beam wander, beam spread, spot distortion, and so on [1]. The study of optical wave propagation in atmospheric turbulence began in Newton’s time, however, systematic research began in the mid-20th century. The lower part of the Earth’s atmosphere shows Kolmogorov statistic characteristics, as even in 1960 there were studies that showed that spectra deformated significantly in the lower atmosphere (in different stratifications). The free atmosphere often demonstrates “non Kolmogorov fluctuations” but at least turbulence spectrum contains −3, −5/3, and other ranges. The early theoretical research on optical wave propagation in atmospheric turbulence was mainly about Kolmogorov turbulence. However, with further measurements of the Earth’s atmosphere and further development of theoretical study on the statistical characteristics of atmospheric turbulence, it was confirmed that Kolmogorov turbulence is only a part of the statistical characteristics of the atmosphere, and non-Kolmogorov statistical features are exhibited in some parts of the Earth’s atmosphere. This has also prompted experts and scholars at home and abroad to carry out the study of optical wave propagation in non-Kolmogorov turbulence. Currently, it is necessary and significant to conclude the structure of turbulence in the Earth’s atmosphere and review the research progress of optical wave propagation theory in atmospheric turbulence.

In this paper, we summarize the experimental measurements and related theoretical results on statistical characteristics of atmospheric turbulence and further conclude the turbulent structure in Earth’s atmosphere. On this basis, we will summarize the development status and latest progress of propagation theory in the atmospheric turbulence, mainly including the propagation theory in conventional Kolmogorov, turbulence, and one in non-Kolmogorov atmospheric turbulence. Finally, the research progress on the combined effects of non-Kolmogorov and Kolmogorov atmospheric turbulence in the Earth’s atmosphere is also summarized. The structural block diagram is shown in Figure 1. This study will help researchers in transforming their ideas into shape and in obtaining clear direction for future research.

2. Structure of Atmospheric Turbulence in Earth’s Atmosphere

2.1. Theoretical Studies on Atmospheric Turbulence

Viscous fluid has two flow states: laminar flow and turbulent flow. When the flow velocity of the fluid is low, the streamline is smooth, and the fluid is in a laminar flow state. When the velocity is very high, with a continuous increase in flow velocity of a fluid, the streamline is no longer smooth, and the entire fluid moves randomly and irregularly, forming a velocity random field is formed, and the fluid is in a turbulent flow state. Here, it should be emphasized that the turbulence mentioned above refers to velocity turbulence. In fact, any physical quantity with uneven random change can constitute turbulence, such as temperature turbulence, humidity turbulence, and refractive-index turbulence, namely atmospheric turbulence [1].

Since turbulent motion is a random motion and turbulent field is a random function of time and space variables, statistics are required to describe it. In order to fully understand and describe the statistical properties of turbulent field, it is necessary to know all the structural functions of velocity. However, because it is difficult to obtain accurately high-order moments both theoretically and experimentally, turbulence a statistical theory of turbulence usually focuses on low-order moments, especially second-order statistical properties. In 1941, Kolmogorov, a former Soviet mathematician, established the statistical theory of turbulent velocity field based on the Richardson cascade model and by using a dimensional analysis method. Currently, this turbulence is also called Kolmogorov turbulence [2]. Kolmogorov believed that these turbulent vortices of different scales coexisted under large Reynolds number, and the small scale turbulent vortices eventually reached statistical equilibrium in the cascade process, which formed local isotropic turbulence. At the same time, he believed that there was a uniform rate of energy dissipation in turbulent fields. According to the above assumptions, Kolmogorov used a dimensional analysis method and finally provided the structural function of turbulent velocity field, which was a 2/3 power function of the space variable. In addition, spectral analysis is an important mathematical method for studying second-order statistical properties. The power spectral density of turbulence has become an important mathematical tool to describe the statistical properties of turbulent field in the statistical theory of turbulence. The power spectral density of the velocity-turbulent field is proportional to the spatial frequency to the −11/3 power law [1].

As a viscous fluid, the atmosphere also has two flow states: laminar flow and turbulent flow. Normally, the atmosphere is in a state of turbulent flow. In an atmospheric turbulent field, the random change of velocity inevitably results in a random change of other physical quantities in space and time, such as temperature and refractive index. Some of these quantities are passive conservative quantities, which forms passive and conservative quantity random fields. It is proved that the structure function of passive and conservative turbulent fields should also satisfy the 2/3 law for Kolmogorov turbulence. Therefore, as one of of the passive conservative quantities, the refractive-index turbulent field should also satisfy the 2/3 law of the following form:

$$D_n\left(r\right)=C_n^2{r}^{11/3},$$

(1)

where $C_n^2$ is a refractive-index structure constant. Moreover, the refractive-index fluctuation power spectrum has the following form:

$${\Phi }_n\left(\kappa \right)=0.033C_n^2{\kappa }^{-11/3},$$

(2)

where $\kappa$ is the magnitude of the spatial frequency vector in units of rad/m, which is known as the Kolmogorov power spectrum [1].

Batchelor discussed small-scale variations of convected quantities such as temperature in turbulent fluid, which showed characteristics that are different from the conventional Kolmogorov spectrum at higher wave-numbers [3]. Golbraikh and Kopeika thought that the deviations from Kolmogorov theory were not random. On the other hand, it is currently known that in addition to the classical Kolmogorov spectrum, other types of spectra also appear at different scales depending on external conditions. They constructed a unified physical method to develop a generalized model of electromagnetic radiation propagation in a random atmosphere by taking into account the behavior characteristics of PSF under different conditions and at different scales [4]. Moiseev and Chkhetiani investigated helical scaling in turbulence. When helicity was considered, an $E{\kappa }^{-7/3}$ energy spectrum was obtained in the asymptotic limit [5]. Elperin et al. studied the isotropic and anisotropic spectra of passive scalar fluctuations in turbulent fluid flow, and it was shown that the anisotropic ${\kappa }^{-3}$ spectrum of passive scalar fluctuations was universal and the derived general spectra recovered the known spectra of passive scalar fluctuations ${\kappa }^{-5/3}$ and ${\kappa }^{-17/3}$ in the particular case of the Kolmogorov spectrum of turbulent velocity field [6]. Brissaud et al. investigated helicity cascades in fully developed isotropic turbulence, and it was concluded that the existence of simultaneous energy and helicity cascades was envisaged based on total helicity conservation in viscid incompressible flows, and the helicity spectrum was developed for the above case [7]. Gibson et al. explored mechanisms of turbulent mixing by numerical simulations of one-dimensional and two-dimensional mixing with Prandtl-number $Pr<1$ [8]. Smith et al. presented evidence for a saturated spectrum of atmospheric gravity waves [9]. To summarize, the further development of the theory of passive conservative flux transport has shown that although Kolmogolov spectrum is important, it is only part of the more general behavior of turbulent passive conservative flux transport. When the atmosphere is in a relatively stable state, the behavior is inconsistent with Kolmogorov turbulence statistics. Beland defined this type of turbulence as non-Kolmogorov turbulence [10]. The existence of non-Kolmogorov turbulence was proved theoretically and above all, other theoretical studies have further confirmed this conclusion.

2.2. Experimental Studies on Atmospheric Turbulence

Recent stellar scintillation and temperature measurements from tether balloons in the sky and ground-based radar indicate deviations from Kolmogolov’s turbulent model in parts of Earth’s atmosphere. Balin et al. studied the fluctuation behavior of aerosols in the lower troposphere using lidar and then studied the statistical law of passive conservative turbulent field at different heights in the troposphere. The results showed that the statistical law of turbulent field in this region was inconsistent with the Kolmogorov turbulence model, and there was a −5 power spectrum [11]. Since then, a series of experimental studies of the stratospheric atmosphere has been carried out. Dalaudier et al. measured the power spectrum of one-dimensional temperature fluctuations along the slant path of Earth’s atmosphere by using balloons and radar. The results showed that the upper troposphere and the entire stratospheric turbulent atmosphere did not follow Kolmogorov statistical law and the three-dimensional power spectrum of −5 power rate was also confirmed [12]. Aleksandrov et al. used spacecraft to record the time power spectrum of starlight flickering and obtained the stratospheric temperature fluctuation power spectrum, which was shown to contradict predictions based on the Kolmogorov turbulence model [13]. Dewan conducted a numerical study on the power spectrum of internal gravity waves, and the results confirmed the existence of a turbulent power spectrum with a power ratio of −5 [14]. Salathe et al. studied the temperature microstructure of the stratosphere by using airplanes and obtained the same conclusion as that of Dalaudier et al [15]. Kyrazis et al. measured the refractive index turbulence field in the top layer and stratosphere of convection, and the results showed that Kolmogorov statistical law was not followed in most cases of turbulence in this region [16]. Gurvich et al. used radar to study the inhomogeneity of anisotropic turbulence, and the analysis of radar return signals showed that the inhomogeneity of temperature in the stratosphere was flattened and did not have isotropic characteristics [17]. Hostetler et al. studied experimentally the horizontal and vertical wave number spectra of gravity waves in the stratosphere and mesosphere over the Central Pacific, and the results obtained confirmed that the power spectrum of this region was inconsistent with the Kolmogorov turbulence model [18]. Gurvich’s research showed further that the reason why the stratospheric temperature fluctuation power spectrum deviated from the traditional locally uniform Kolmogorov model is due to the strong and stable stratification structure of the Earth’s atmosphere at higher altitudes [19]. Belen’kii et al. conducted an experimental study on the effect of stratospheric turbulence on star image jitters with telescopes on the ground, and the results showed that the turbulence in this layer did not conform to Kolmogorov’s statistical law [20]. Belen’kii also observed experimental evidence for wave-front tilts that do not follow Kolmogorov turbulence [21]. Thus far, the statistical law of stratospheric atmospheric turbulence does not meet the statistical behavior of Kolmogorov turbulence, which has a −5 power spectral density. This also prompted the measurement of statistical behavior in different regions of the atmosphere. Zilberman et al. used lidar to measure the intensity of backscattered light to study the fluctuation of passive conservative turbulent field in the top troposphere and stratosphere. The results showed that the passive conservative turbulent field in these regions did not follow Kolmogorov statistical law. That is, the turbulence in Earth’s atmosphere is composed of the Kolmogorov turbulence in the boundary layer, the −10/3 non-Kolmogorov turbulence in the free atmosphere, and the −5 non-Kolmogorov turbulence in the stratosphere [22,23,24,25]. The research results by Nosov et al. showed that non-Kolmogorov turbulence can be considered as coherent structures, and Kolmogorov turbulence could be considered as a mixture of different coherent structures. Coherent non-Kolmogorov turbulence differed from incoherent Kolmogorov turbulence in a more rapid decrease in the one-dimensional spectrum of temperature fluctuations (with exponential value −8/3 instead of −5/3) in the inertial interval.

The results of atmospheric measurements show that the structure of atmospheric turbulence is composed of Kolmogorov turbulence at lower levels and non-Kolmogorov turbulence at higher levels. Thus far, there are two models for the structure of atmospheric turbulence in Earth’s atmosphere: (a) A two-layer model, namely, the turbulence in the Earth’s atmosphere is composed of Kolmogorov turbulence in the troposphere and −5 non-Kolmogorov turbulence in the stratosphere, constructed by Gurvich and Belen ’Kii based on observations of early Earth’s atmosphere [26]. Later, Fu et al. revised the two-layer model based on the consistency between the refractive index structure function and the power spectral density [27]; (b) a three-layer model, namely, that the turbulence in the Earth’s atmosphere is composed of Kolmogorov turbulence in the boundary layer, −10/3 non-Kolmogorov turbulence in the free atmosphere, and −5 non-Kolmogorov turbulence in the stratosphere, which all have the following form:

$${\Phi }_{nB}(\kappa ,z)=0.033C_n^2\left(z\right){\kappa }^{-11/3},$$

(3)

$${\Phi }_{nF}(\kappa ,z)=0.015{\tilde{C}}_{nF}^2\left(z\right){\kappa }^{-10/3},$$

(4)

$${\Phi }_{nS}(\kappa ,z)=0.0024{\tilde{C}}_{nS}^2\left(z\right){\kappa }^{-5},$$

(5)

where z is a propagation distance that varies between $z=0$ and $z=L$, and $C_n^2\left(z\right)$ is the conventional Kolmogorov turbulent index-of-refraction structure parameter in the boundary layer that is dependent on the altitude and has units of $m^{-2/3}$, ${\tilde{C}}_{nF}^2\left(z\right)$ is the non-Kolmogorov index-of-refraction structure parameter in the free troposphere that depends on the altitude and has units of $m^{-1/3}$, and ${\tilde{C}}_{nS}^2\left(z\right)$ is a non-Kolmogorov index-of-refraction structure parameter in the stratosphere that is dependent on the altitude and has units of $m^{-2}$.

Here, it is noteworthy that the structure of atmospheric turbulence in the Earth’s atmosphere varies with different regions and atmospheric conditions.

3. Theoretical Studies of Turbulent Effect

It is well known that both the amplitude and phase of optical wave experience random fluctuations caused by small random changes in the index of refraction as an optical wave propagates through atmospheric turbulence. The effect of atmospheric turbulence on optical wave propagation is fundamentally the destruction of its coherence, which includes manifested by various atmospheric turbulence effects, such as irradiance scintillation, angle-of-arrival fluctuations, beam wander, beam spread, spot distortion, and so on. In order to describe these random amplitude and phase fluctuations, one needs to solve the wave equation for the electric field of optical wave or for the various moments of the field. In the process of solving the above equations, the specific characterization of atmospheric turbulence was not considered. Therefore, the theoretical method of Kolmogorov atmospheric turbulence effect is also applicable to non-Kolmogorov atmospheric turbulence. Here, we first review the representative and classical theory for optical wave propagation in Kolmogorov turbulence and then elaborate the research results of non-Kolmogorov atmospheric turbulence effects thus far.

3.1. Theoretical Studies of Kolmogorov Turbulent Effect

The classical theory for propagation through random media derives from the solution of stochastic Helmholtz equations, which is a governing partial differential equation for the scalar field. The Born and Rytov perturbation methods for solving the stochastic Helmholtz equations are introduced first. Both approximation methods are limited to weak fluctuations condition. The parabolic equation method is one of the most general methods that is theoretically applicable under all atmospheric conditions [1]. The extended Huygens–Fresnel principle was developed by Lutomirski and Yura [28] in the United States and Feizulin and Kravtsov in the former Soviet Union [29], and the corresponding optical field has the following form:

$$U(\overrightarrow{r},L)=-\frac{ik}{2\pi L}exp\left(ikL\right)\int {\int }_{-\infty }^{\infty }{d}^{2}s{U}_0(\overrightarrow{s},0)exp\left[\frac{ik|\overrightarrow{s}-\overrightarrow{r}{|}^2}{2L}+\mathsf{\Psi }(\overrightarrow{r},\overrightarrow{s})\right],$$

(6)

where $\overrightarrow{r}$ and $\overrightarrow{s}$ are the distance vectors transverse to the propagation axis, k represents the optical wave number, $U_0(\overrightarrow{s},0)$ denotes the unperturbed field in the absence of turbulence at the point $(\overrightarrow{s},0)$, and $\mathsf{\Psi }(\overrightarrow{r},\overrightarrow{s})$ is the random part of the complex phase of a spherical wave propagating in the turbulent medium from point $(\overrightarrow{s},0)$ to point $(\overrightarrow{r},L)$. The extended Huygens–Fresnel principle is applicable for first-order and second-order field moments under weak or strong fluctuation conditions of atmospheric turbulence. Another approximation method was introduced based on a modification of the Rytov theory that permits extending many weak-fluctuation results into the moderate-to-strong fluctuations regimes. Moreover, the corresponding optical field has the following form:

$$U(\overrightarrow{r},L)=U_0(\overrightarrow{r},L)exp\left[{\mathsf{\Psi }}_X(\overrightarrow{r},L)\right]exp\left[{\mathsf{\Psi }}_Y(\overrightarrow{r},L)\right],$$

(7)

where ${\mathsf{\Psi }}_X(\overrightarrow{r},L)$ and ${\mathsf{\Psi }}_Y(\overrightarrow{r},L)$ are statistcally independent complex phase perturbations due only to the large-scale and small-scale effects of the effective refractive index, respectively, on propagating wave. By using the Rytov method, the first and second moments of the optical field were examined from which several important statistical quantities were deduced, mainly mutual coherence function, spatial coherence radius for plane and spherical wave and a Gaussian-beam wave, angel-of-arrival fluctuations and image jitter for a plane wave, beam wander of a Gaussian-beam wave, and angular and temporal frequency spectra for a plane wave [1]. Based on the parabolic equation method and the extended Huygens–Fresnel principle, second-order statistics were studied in strong fluctuation conditions. Using the parabolic equation method, the mean irradiance and mutual coherence function for a Gaussian-beam wave were deduced in the strong fluctuation regime. Based on the extended Huygens–Fresnel principle, the second-order moments of the complex phase perturbation were investigated, and mean irradiance, beam spread, and mutual coherence function for a Gaussian-beam wave were deduced in the strong fluctuation regime. In addition, applying the method of effective beam parameters, the spatial coherence radius and beam wander for a Gaussian-beam wave were deduced in the strong fluctuations condition [1]. The various fourth-order statistical quantities such as the scintillation index and the irradiance covariance function were also examined. By using the Rytov method, the scintillation index, covariance function of irradiance, temporal spectrum of irradiance for a plane wave, a spherical wave, and a Gaussian-beam wave were deduced, and beam wander for a Gaussian-beam wave were developed in weak irradiance fluctuation regimes. Furthermore, phase variance, phase structure functions, covariance function, and temporal power spectrum for a plane wave and a Gaussian-beam wave were also developed in weak fluctuations regimes [1]. By using the extended Rytov theory, the examination of various fourth-order statistical quantities, such as the scintillation index and the irradiance covariance function, were extended to the strong fluctuations regimes. The scintillations for plane wave, spherical wave, and Gaussian-beam wave were developed. Moreover, the covariance functions of irradiance models for plane wave and spherical wave were also deduced. The temporal spectrum of irradiance for plane wave was examined in the strong fluctuations condition [1]. Baskov and Chumak developed a new method. They derived the Boltzmann–Langevin kinetic equation from the first principles of quantum optics and the scintillation index was obtained for the practically important range of weak and moderate atmospheric turbulence [30]. After more than half a century of efforts, the theory of optical wave propagation in atmospheric turbulence has made great progress. In recent years, major research efforts have been devoted to the theoretical study of propagation of special beams in atmospheric turbulence, including vortex laser beam, vortex cosine hyperbolic Gaussian beam, airy prime beam, lowest order Bessel Gaussian beams, high power partially coherent laser beams, low order Laguerre–Gaussian beams, Bessel–Gaussian–Schell model beam, vortex Bessel-like beams, optical bottle beam, radial phased-locked rotating elliptical Gaussian beam array, airy Gaussian vortex beam array, flat-topped Gaussian vortex beam, generalized Bessel–Laguerre–Gaussian beams, and so on. Furthermore, the atmospheric turbulent effects involve mean intensity, scintillation, coherence, beam spreading, wander, and other propagation properties [31,32,33,34,35,36,37,38,39,40,41,42,43,44,45,46,47].

To sum up, the theoretical research on optical wave propagation in the turbulent atmosphere starts from plane waves, spherical waves, and Gaussian beams, which are conventional optical waves. Currently, people are expanding to special beams to explore the statistical law of the impact of atmospheric turbulence on special beams in order to obtain a better solution to overcome the influence of atmospheric turbulence on optical wave propagation.

3.2. Theoretical Studies of Non-Kolmogorov Turbulent Effect

Contrary to many experimental facts and some relevant theoretical results of Kolmogorov turbulence, the study of optical wave propagation in non-Kolmogorov atmospheric turbulence has attracted considerable attention, and experts and scholars at home and abroad have begun to study the topics related to optical wave propagation in non-Kolmogorov atmospheric turbulence. To our knowledge, a more influential study is summarized below. Beland developed a refractive index fluctuation spatial power spectrum with a general power ratio. It has the following form:

$${\Phi }_n(\kappa ,\alpha )=A\left(\alpha \right)\widetilde{C_n^2}{\kappa }^{-\alpha },\kappa >0,3<\alpha <4,$$

(8)

where $\alpha$ denotes the spectral power-law exponent that can assume all the values between the range of 3 to 4, $\widetilde{C_n^2}$ is a generalized refractive-index structure parameter with units $m^{3-\alpha }$, and $A\left(\alpha \right)$ is defined by the following:

$$A\left(\alpha \right)=\frac{1}{4{\pi }^2}\mathsf{\Gamma }(\alpha -1)cos\left(\frac{\alpha \pi }{2}\right),$$

(9)

where the symbol $\mathsf{\Gamma }\left(x\right)$ represents the gamma function. Then, the Rytov smoothing perturbation approximation method was used to study theoretically the representative amplitude and phase effects of optical wave propagation in non-Kolmogorov turbulence, and the logarithmic amplitude fluctuation variances and coherence length of the plane wave and spherical wave under the condition of weak fluctuation were derived. The analytic expressions were obtained [10]. Stribling et al. studied optical wave propagation by using a non-classical power spectrum, which described media based on the report of statistical law of non-Kolmogorov atmospheric turbulence, and developed a spatial power spectrum of refractive index fluctuation with a generalized power law. Based on the Mellin transform, the structural functions of plane and spherical waves under the condition of weak fluctuations were derived, and then the Strehl ratio was calculated by using the structural functions, and the relative contributions of logarithmic amplitude structural functions and phase structural functions to the wave structural functions were studied, which were often used to describe the statistical properties of an optical field [48]. Boreman et al. obtained an analytical expression for the forward difference of non-Kolmogorov turbulent waves by using Zernik polynomials [49]. Rao et al. developed a theoretical method and then used this method to analyze the temporal and spatial characteristics of the phase fluctuation of optical wave propagation in non-Kolmogolov turbulence and deduced the spatial structure function, time structure function, and time frequency spectrum of the phase fluctuation. The cross-correlation functions of long exposure and short exposure and the Strehl ratio of imaging were calculated [50]. Rao et al. also studied adaptive compensation of distributed beacons in non-Kolmogorov turbulence [51]. Zunino introduced a fractional Brownian motion representation of the turbulent wavefront phase and applied the method to the wavefront construction of non-Kolmogorov turbulence [52]. Wang et al. developed a new stochastic phase screen time series simulation algorithm for dynamic atmospheric turbulence wavefront generator, which was applicable not only to Kolmogorov turbulence but also to non-Kolmogorov turbulence, and studied the spatial and temporal statistical laws of atmospheric turbulence by using this method [53]. Toselli et al. developed a spatial power spectrum with a general power index, then studied the angle-of-arrival fluctuation of optical wave propagation in non-Kolmogorov turbulence by using the geometric optical approximation method and deduced the analytical expressions of the variance of the angle-of-arrival fluctuation of plane wave and spherical wave under the condition of weak fluctuation. It should be noted here that the spectrum and evolution spectrum based on it are widely used in the field of non-Kolmogorov large turbulence effects [54]. Cui et al. investigated the general atmospheric turbulence modulation transfer function for optical wave propagation in non-Kolmogorov turbulence [55]. Wu et al. investigated beam propagation and the directionality of Gaussian–Schell beams propagating in non-Kolmogorov turbulence [56]. Zhou et al. studied the average expansion of a Gaussian beam array propagating in non-Kolmogorov turbulence [57]. Shchepakina and Korotkova studied the second-order statistical law of the random electromagnetic beam propagating in non-Kolmogorov turbulence [58]. Tang et al. investigated the mean beam propagation of a radial Gaussian beam array in non-Kolmogorov atmospheric turbulence [59]. Cui et al. studied intensity scintillation, optical wave propagation function, optical intensity temporal power spectrum, and angle-of-arrival fluctuation of Gaussian beams progagated in weak non-Kolmogorov turbulence [60]. Xu et al. studied the propagation of Laguerre–Gaussian beams in non-Kolmogorov turbulence [61]. He and Lu studied the propagation of partially coherent flat-topped vortex beams in non-Kolmogorov atmospheric turbulence [62]. Cang and Liu studied the scintillation index and performance of optical wireless communication links in non-Kolmogorov weak turbulence based on the general atmospheric turbulence spectrum model [63]. Baykal studied the intensity fluctuation characteristics of higher order laser beams in non-Kolmogorov atmospheric turbulence [64]. Gudimetla et al. simulated laser propagation in non-Kolmogorov atmospheric turbulence using a random phase screen method [65]. Based on the modified Rytov method, Liu et al. developed the expression of the spiral spectrum of the beam in slant atmospheric turbulence using the non-Kolmogorov power spectrum with the atmospheric turbulent inner scale and the outer scale, which changed with altitude [66]. Zhang and Fu derived the analytical expressions for elements of the cross-spectral density matrix of a Square Multi-Gaussian–Schell Model (SMGSM) beam propagating in non-Kolmogorov turbulence, based on the extended Huygens–Fresnel integral [67]. Based on the generalized Huygens–Fresnel principle, Tang et al. derived the analytical expressions of partially coherent Lommel beams propagating in a turbulent atmosphere. The propagation properties of vortex mode intensity (including mode probability density and crosstalk probability density) of a partially coherent Lommel beam in non-Kolmogorov turbulence were investigated [68]. Xu and Zhang investigated the effects of the moderate to strong fluctuation on the propagation of orbital angular momentum (OAM) modes carried by Bessel–Gaussian beams in turbulent atmosphere. The analytic expression of the received probability of the signal and crosstalk OAM mode was derived based on the theories of extended Rytov and non-Kolmogorov turbulent power spectrum [69]. Zeng et al. established a theoretical model for calculating the spiral spectrum, also called the orbital angular momentum (OAM) spectrum, of a Laguerre-Gaussian (LG) beam after propagation through anisotropic non-Kolmogorov turbulence along a horizontal link [70]. In the study of optical wave propagation in non-Kolmogorov atmospheric turbulence, our research group has also performed some studies. The scintillation index of Gaussian beam, the temporal power spectrum of plane wave and spherical wave intensity fluctuations, the variance and temporal power spectrum of the angle-of-arrival fluctuation for plane wave and spherical waves, beam wander, and the two-frequency mutual correlation function of Gaussian beam were studied, respectively, by using Rytov perturbation approximation and a spectral analysis method [71,72,73,74,75,76,77].

With the further development of research, the characteristics of optical wave propagation in non-Kolmogorov atmospheric turbulence under strong fluctuation conditions have become the focus of research, and some research achievements have been obtained. Yi et al. studied the coherence radius, phase structure function, and scintillation index of plane wave and spherical wave in non-Kolmogorov atmospheric turbulence under strong fluctuations by using universal von-Karman power spectrum [78]. Based on the universal von-Karman power spectrum, Cui et al. studied the angle-of-arrival fluctuation variances of plane and spherical waves in non-Kolmogorov turbulence under strong fluctuation conditions by using the modified Rytov theory [79]. Cui et al. also studied the influence of non-Kolmogorov atmospheric turbulence on angle-of-arrival fluctuations for plane wave and spherical wave under moderate to strong fluctuations, and a new analytical expression of variance was obtained [80]. Cui et al. investigated the effects of moderate-to-strong non-Kolmogorov turbulence on the angle-of-arrival fluctuations for plane and spherical waves in detail both analytically and numerically. New analytical expressions for the variance of angle-of-arrival fluctuations were derived for moderate-to-strong non-Kolmogorov turbulence [81]. Deng et al. studied the scintillation index of Gaussian beams in non-Kolmogorov atmospheric turbulence under strong fluctuation regimes by using a modified Rytov theory based on a non-Kolmogorov atmospheric turbulence power spectrum density [82]. Cui et al. studied the temporal characteristics of angle-of-arrival fluctuations of plane and spherical waves in non-Kolmogorov atmospheric turbulence under strong fluctuation regime and obtained the temporal power spectrum expressions of angle-of-arrival fluctuation [83]. Cui et al. studied the influence of non-Kolmogorov atmospheric turbulence on the plane wave and spherical wave structure functions under strong fluctuation regimes and then studied the influence of the modulation transfer function [84]. Cui et al. theoretically derived a new power spectrum model of atmospheric turbulence and then used this spectral model to re-derive the expressions of plane wave and spherical wave scintillation index in non-Kolmogorov atmospheric turbulence under strong fluctuation regime [85]. Ma et al. derived and further analyzed the scintillation index of Gaussian beams in a new non-Kolmogorov atmospheric turbulence with strong fluctuations by using a universal effective atmospheric turbulence power spectrum [86].

As previously mentioned, the theoretical research performed on propagation of optical waves in a Kolmogorov turbulent atmosphere is relatively early and mature. The theoretical research methods developed have been widely used in the study of optical wave propagation in non-Kolmogorov atmospheric turbulence. The statistical properties of optical wave are explored when the power law of refractive index fluctuation power spectrum deviates from -11/3. The atmospheric turbulent effects of plane wave, spherical wave, and Gaussian-beam wave have been studied more than that of special beams. Therefore, we believe that it will be one of the future trends to study the statistical characteristics of special beam propagation in non-Kolmogorov atmospheric turbulence.

4. Theoretical Studies of Combined Influence of Kolmogorov and Non-Kolmogorov Turbulence

To conclude, the existence of non-Kolmogorov turbulence is an indisputable fact both theoretically and experimentally. As the measurement of atmospheric turbulence deepens and the understanding of atmospheric turbulence structure is further refined, various models describing atmospheric turbulence structure of Earth’s atmosphere have been developed. Gurvich and Belen’kii established a two-layer turbulence model based on the early measured results of atmospheric turbulence; that is, the turbulence in Earth’s atmosphere is composed of Kolmogorov turbulence in the troposphere and −5 non-Kolmogorov turbulence in the stratosphere. They further studied the combined effects of these two kinds of turbulence on infrared imaging [26]. Later, Belen’kii studied the combined effects of Kolmogorov turbulence in the troposphere and −5 non-Kolmogorov turbulence in the stratosphere on star image jitters [87]. Fu et al. modified the two-layer model from Gurvich and Belen’kii based on the consistency between the refractive index fluctuation power spectrum and the structure function and the measurement results of Zilberman et al. The influence of Kolmogorov turbulence in troposphere and −5 non-Kolmogorov turbulence in the stratosphere on the fluctuation of starlight angle-of-arrival was studied [27]. Zilberman et al. developed a three-layer atmospheric turbulence model of Earth’s atmosphere based on recent measurements of atmospheric turbulence; that is, the turbulence in Earth’s atmosphere is composed of the Kolmogorov turbulence in the boundary layer, the −10/3 non-Kolmogorov turbulence in the free atmosphere, and the −5 non-Kolmogorov turbulence in the stratosphere. Then, the logarithmic amplitude variance of the electromagnetic wave propagating in Earth’s atmosphere was studied by using this model [88]. Zilberman et al. studied the combined influence of Kolmogorov turbulence and non-Kolmogorov turbulence on space optical communication under the condition of weak fluctuations based on the three-layer model of atmospheric turbulence in satellite–Earth links [89]. Based on the study of Zilberman et al., Yi et al. also used a three-layer model to study the bit error rate of satellite-ground laser communication uplink under weak fluctuation conditions [90]. Our research group has also performed some studies on the basis of establishing a theoretical model of long-term beam spreading and scintillation index of Gaussian beam in the stratosphere non-Kolmogorov turbulence under the condition of weak fluctuations. The combined influence of Kolmogorov turbulence in troposphere and −5 non-Kolmogorov turbulence in the stratosphere on satellite–Earth laser communication was studied based on a two-layer model of atmospheric turbulence with respect to the satellite–Earth link. The combined effects of tropospheric Kolmogorov atmospheric turbulence and stratosphere −5 non-Kolmogorov atmospheric turbulence on long-term beam spreading [91] and the scintillation index of Gaussian beams were analyzed. On this basis, the effects of combined atmospheric turbulence on the performance of space-ground laser links were studied, and the bit error rate under the OOK system was analyzed [27,92,93]. Based on the three-layer combined atmospheric turbulence model, the intensity fluctuation and beam propagation of uplinks and downlinks under strong fluctuation regimes were analyzed, and the relationship between bit error rate and SNR of fading channels in satellite-based laser communication links was then analyzed. The influence of strong combined atmospheric turbulence on the angle-of-arrival fluctuation of uplinks and downlinks was also studied. Furthermore, the variance and time-frequency spectrum models of angle-of-arrival fluctuation for uplinks and downlinks under a strong fluctuation regime were established and analyzed theoretically [94,95,96].

To summarize, it is an indisputable fact that the atmospheric turbulence in Earth’s atmosphere consists of Kolmogorov turbulence and non-Kolmogorov turbulence. It is a scientific problem used to study the effect of combined atmospheric turbulence on optical wave propagation according to the actual situation of atmospheric turbulence. It is an important area for the future investigations of science.

5. Conclusions

In this paper, we review the experimental and theoretical results of atmospheric turbulence and then present the structure of atmospheric turbulence in Earth’s atmosphere. It is composed of the Kolmogorov turbulence in the boundary layer, the −10/3 non-Kolmogorov turbulence in the free atmosphere, and the −5 non-Kolmogorov turbulence in the stratosphere. The progress of propagation theory for Kolmogorov atmospheric turbulence was briefly introduced firstly, and the research results of non-Kolmogorov atmospheric turbulence effects was elaborated. This mainly includes various atmospheric turbulence effects such as intensity scintillation, angle-of-arrival fluctuations, beam wander, beam spread, temporal power spectrum, and so on. Finally, we summarize the research progress on the combined effects of non-Kolmogorov and Kolmogorov atmospheric turbulence on optical wave propagation. In addition, future research directions and trends of optical wave propagation in atmospheric turbulence were provided from the perspective of our understanding.