Disturbances of the Thermosphere and the Ionosphere during a Meteorological Storm
Abstract
1. Introduction
2. Lidar and Satellite Measurements
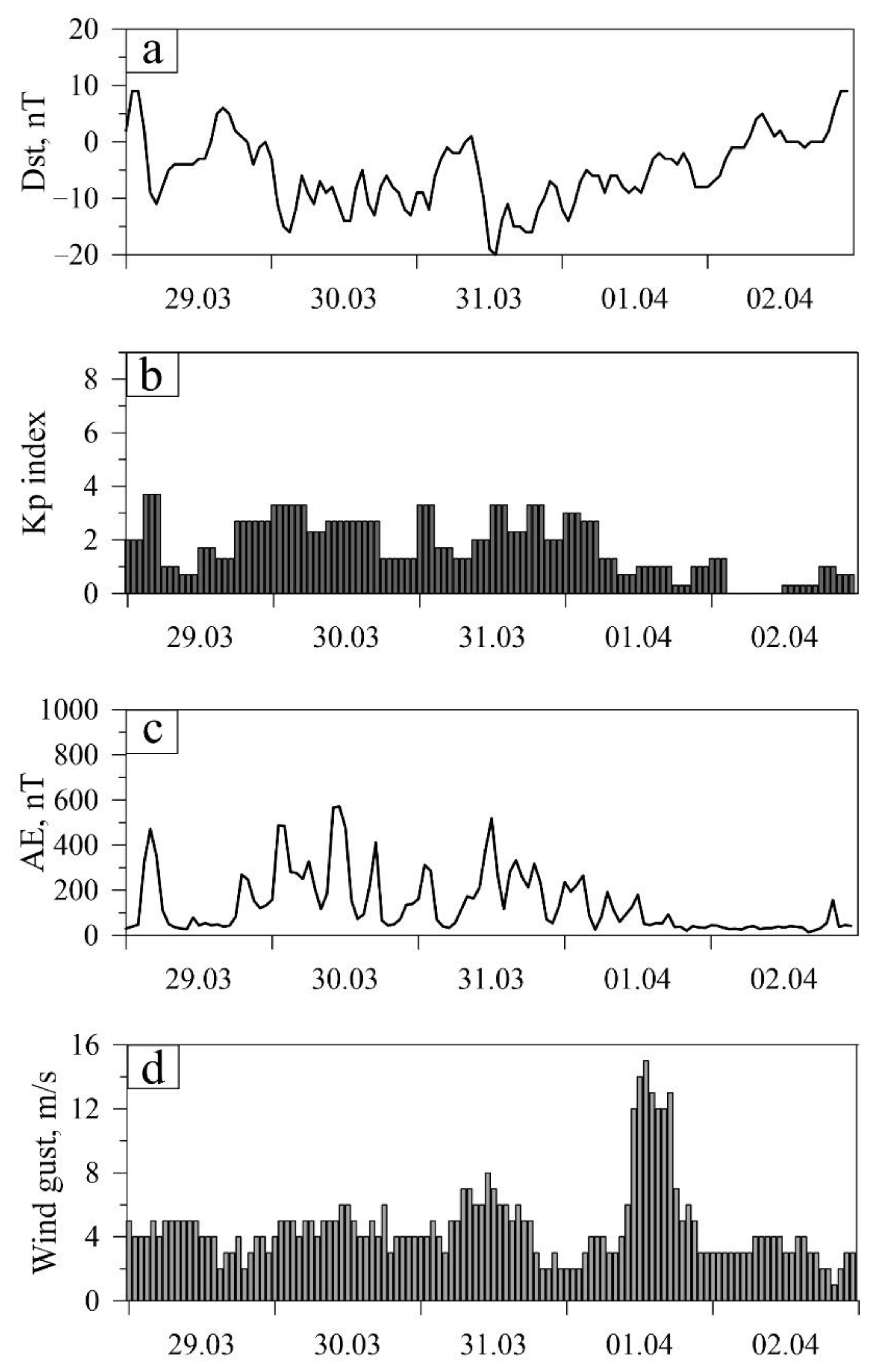
2.1. Geomagnetic Conditions during the Experiment
2.2. Lidar Measurement Technique
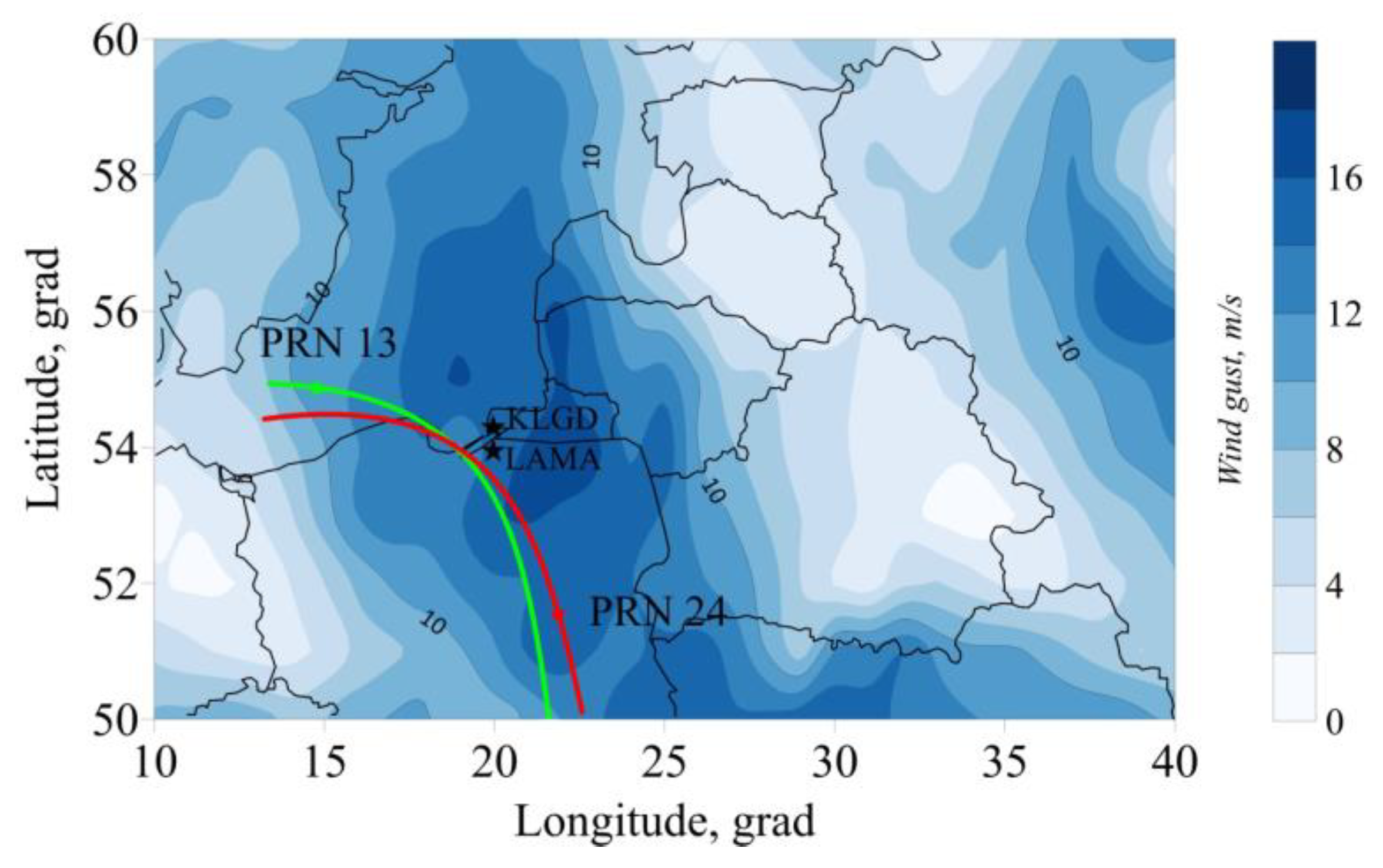
2.3. Technique of Satellite GPS Measurements
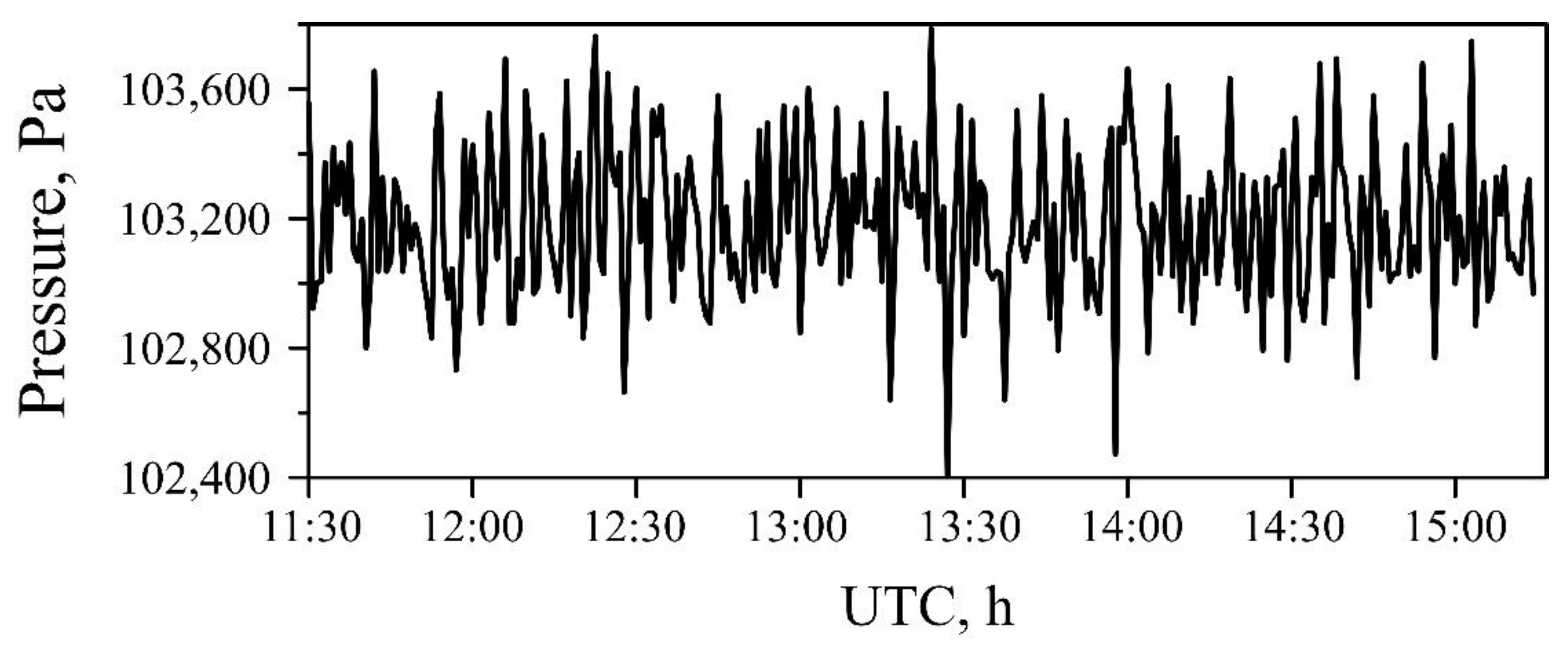
2.4. Determination of the Parameters of a Meteorological Storm
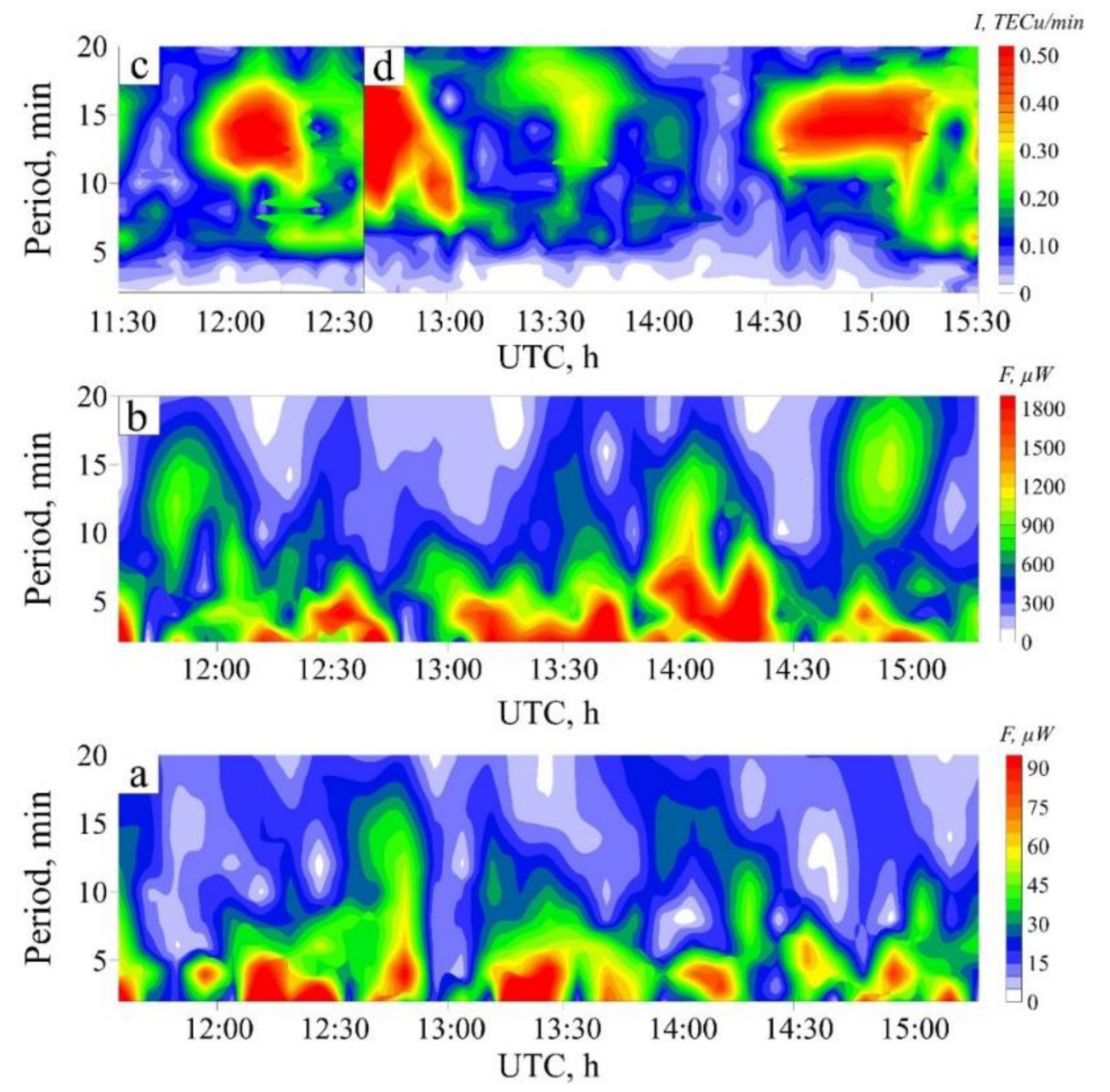
2.5. Results of Experiments
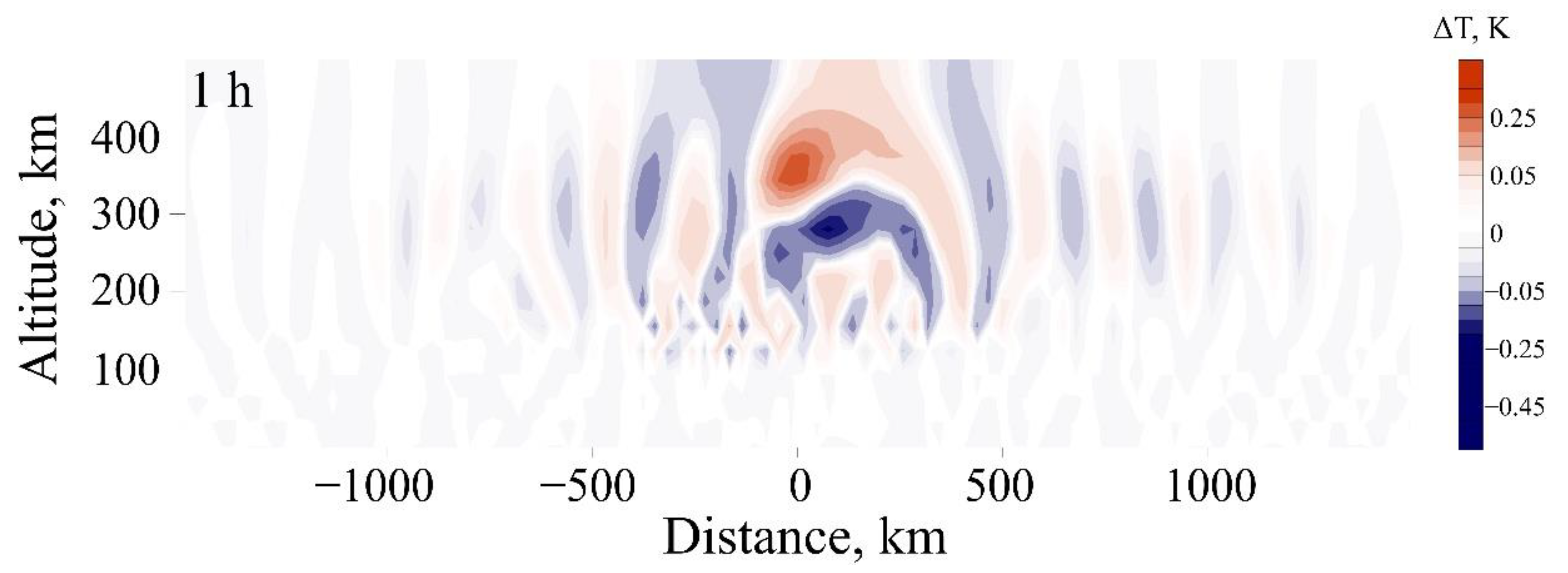
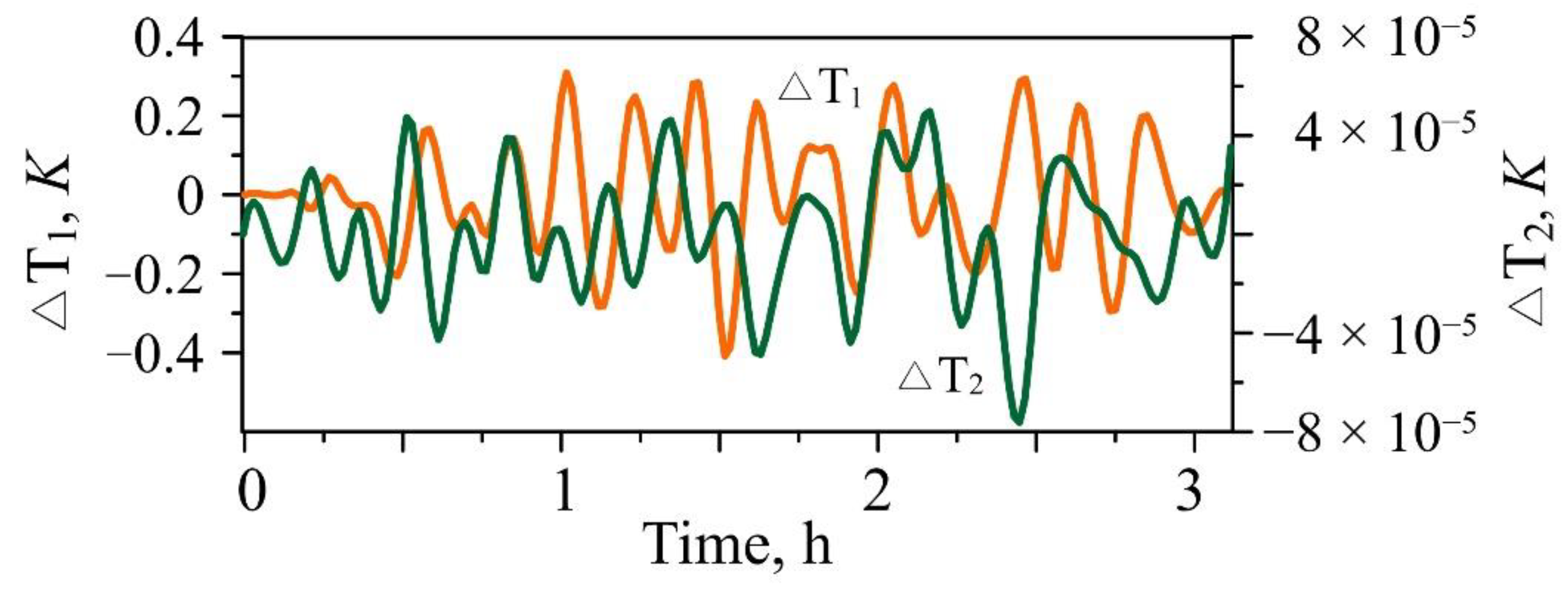
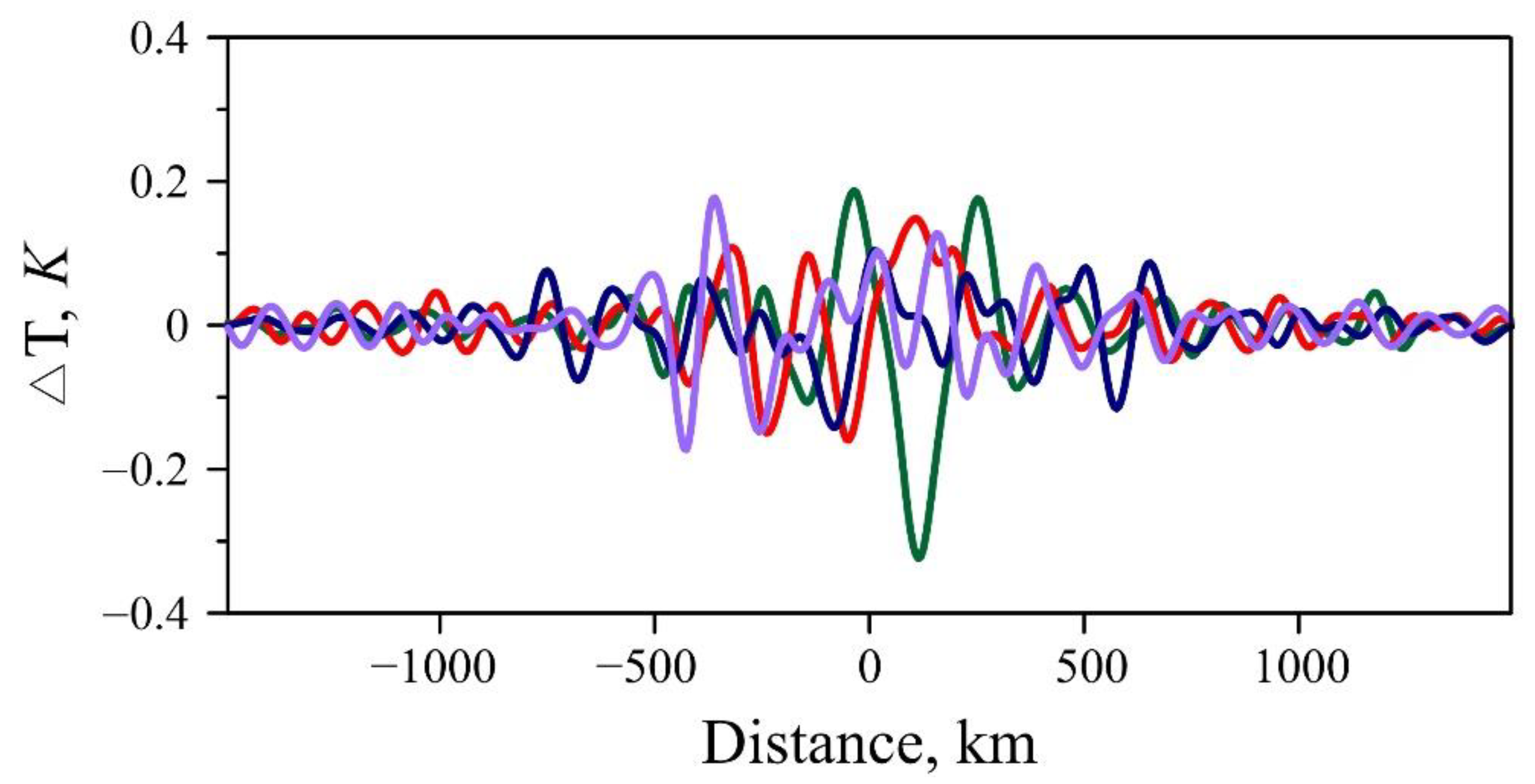
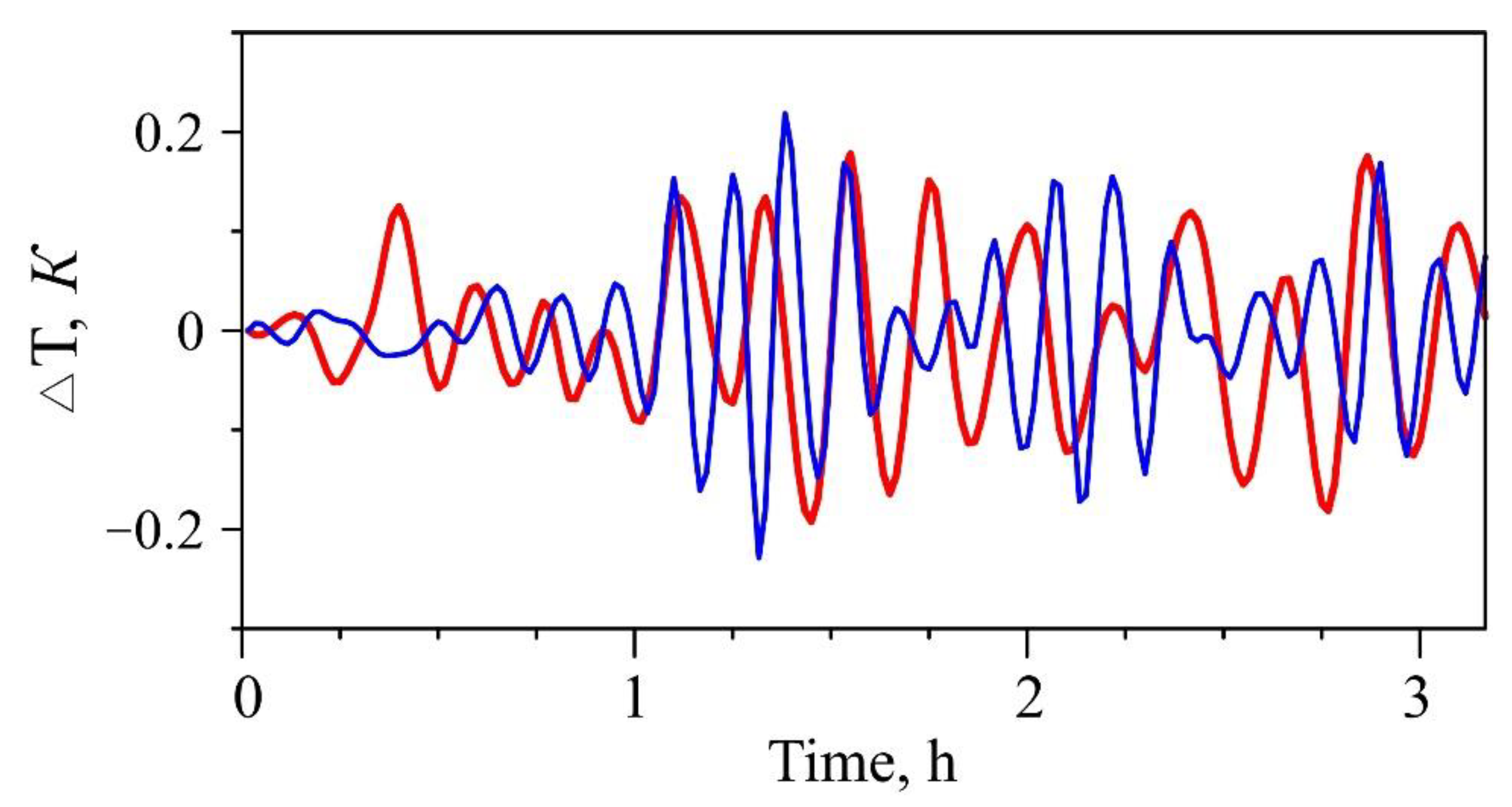
3. Numerical Simulation
4. Analysis of Wave Variations in the Atmosphere and Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Laštovička, J. Forcing of the ionosphere by waves from below. J. Atmos. Sol. Terr. Phys. 2006, 68, 479–497. [Google Scholar] [CrossRef]
- Forbes, J.M.; Maute, A.; Zhang, X.; Hagan, M.E. Oscillation of the ionosphere at planetary-wave periods. J. Geophys. Res. Space Phys. 2018, 123, 7634–7649. [Google Scholar] [CrossRef]
- Rishbeth, H.; Mendillo, M. Patterns of F2-Layer Variability. J. Atmos. Sol. Terr. Phys. 2001, 63, 1661–1680. [Google Scholar] [CrossRef]
- Yiǧit, E.; Koucká Knížová, P.; Georgieva, K.; Wardm, W. A review of vertical coupling in the Atmosphere-Ionosphere system: Effects of waves, sudden stratospheric warmings, space weather, and of solar activity. J. Atmos. Sol. Terr. Phys. 2016, 141, 1–12. [Google Scholar] [CrossRef]
- Fritts, D.C.; Alexander, M.J. Gravity wave dynamics and effects in the middle atmosphere. Rev. Geophys. 2003, 41. 1003:1–1003:64. [Google Scholar] [CrossRef]
- Pertsev, N.N.; Shalimov, S.L. Generation of atmosphere gravitation waves in the seismically active region and their effect on the ionosphere. Geomagn. Aeron. 1996, 36, 111–118. [Google Scholar]
- Klimenko, M.; Klimenko, V.V.; Karpov, I.V.; Zakharenkova, I.E. Simulation of seismo-ionospheric effects initiated by internal gravity wave. Russ. J. Phys. Chem. B 2011, 5, 393–401. [Google Scholar] [CrossRef]
- Karpov, I.V.; Kshevetskii, S.P. Formation of large-scale disturbances in the upper atmosphere caused by acoustic gravity wave sources on the Earth’s surface. Geomagn. Aeron. 2014, 54, 513–522. [Google Scholar] [CrossRef]
- Hickey, M.P.; Schubert, G.; Walterscheid, R.L. Acoustic wave heating of the thermosphere. J. Geophys. Res. Space Phys. 2001, 106, 21543–21548. [Google Scholar] [CrossRef]
- Drobzheva, Y.; Krasnov, V. Acoustic energy transfer to the upper atmosphere from surface chemical and underground nuclear explosions. J. Atmos. Sol. Terr. Phys. 2006, 68, 578–585. [Google Scholar] [CrossRef]
- Fritts, D.; Vadas, S.L.; Wan, K.; Werne, J.A. Mean and variable forcing of the middle atmosphere by gravity waves. J. Atmos. Sol. Terr. Phys. 2006, 68, 247–265. [Google Scholar] [CrossRef]
- Gavrilov, N.; Koval, A. Parameterization of mesoscale stationary orographic wave forcing for use in numerical models of atmospheric dynamics. Izv. Atmos. Ocean. Phys. 2013, 49, 244–251. [Google Scholar] [CrossRef]
- Gavrilov, N.; Koval, A.; Pogoreltsev, A.I.; Savenkova, E.N. Numerical modeling of inhomogeneous orographic wave influence on planetary waves in the middle atmosphere. Adv. Space Res. 2013, 51, 2145–2154. [Google Scholar] [CrossRef]
- Astafyeva, E. Ionospheric detection of natural hazards air plasma parameters in normal and seismic conditions. Rev. Geophys. 2019, 57, 1265–1288. [Google Scholar] [CrossRef]
- Shalimov, S.; Rozhnoi, A.; Solov’eva, M.; Olshanskaya, E. Impact of earthquakes and tsunamis on the ionosphere. Izv. Phys. Sol. Earth 2019, 55, 168–181. [Google Scholar] [CrossRef]
- Jakowski, N.; Stankov, S.; Wilken, V.; Borries, C.; Altadill, D.; Chum, J.; Buresova, D.; Boska, J.; Sauli, P.; Hruska, F.; et al. Ionospheric behavior over Europe during the solar eclipse of October 3, 2005. J. Atmos. Sol. Terr. Phys. 2008, 70, 836–853. [Google Scholar] [CrossRef]
- Altadill, D.; Sole, J.G.; Apostolov, E.M. Vertical structure of a gravity wave like oscillation in the ionosphere generated by the solar eclipse. J. Geophys. Res. 2001, 10, 21419–21428. [Google Scholar] [CrossRef]
- Jones, T.B.; Wright, D.M.; Milner, J.; Yeoman, T.K.; Reid, T.; Chapman, P.J.; Senior, A. The detection of atmospheric waves produced by the total solar eclipse of August 11, 1999. J. Atmos. Sol. Terr. Phys. 2004, 66, 363–374. [Google Scholar] [CrossRef]
- Kumar, K.V.; Ajeet, K.M.; Kumar, S.; Singh, R. July 22, 2009, Total Solar Eclipse induced gravity waves in the ionosphere as inferred from GPS observations over EIA. Adv. Space Res. 2016, 58, 1755–1762. [Google Scholar] [CrossRef]
- Rybnov, Y.; Soloviev, S. Synchronous variations in the atmospheric pressure and electric field during the passage of the solar terminator. Geomagn. Aeron. 2019, 59, 234–241. [Google Scholar] [CrossRef]
- Zakharov, V.I.; Kunitsyn, V.E. Regional features of atmospheric manifestations of tropical cyclones according to ground-based GPS network data. Geomagn. Aeron. 2012, 52, 533–545. [Google Scholar] [CrossRef]
- Kazimirovsky, E.S. Coupling from below as a source of ionospheric variability: A review. Ann. Geophys. 2002, 45, 1–29. [Google Scholar] [CrossRef]
- Koucká Knížová, P.; Mošna, Z.; Kouba, D.; Potužníková, K.; Boška, J. Influence of meteorological systems on the ionosphere over Europe. J. Atmos. Sol. Terr. Phys. 2015, 136, 244–250. [Google Scholar] [CrossRef]
- Chernigovskaya, M.A.; Shpynev, B.G.; Ratovsky, K.G. Meteorological effects of ionospheric disturbances from vertical radio sounding data. J. Atmos. Sol. Terr. Phys. 2015, 136, 235–243. [Google Scholar] [CrossRef]
- Borchevkina, O.; Karpov, I.; Karpov, M. Meteorological storm influence on the ionosphere parameters. Atmosphere 2020, 11, 1017. [Google Scholar] [CrossRef]
- Plougonven, R.; Zhang, F. Internal gravity waves from atmospheric jets and fronts. Rev. Geophys. 2014, 52, 33–76. [Google Scholar] [CrossRef]
- Karpov, I.V.; Borchevkina, O.P.; Karpov, M.I. Local and regional ionospheric disturbances during meteorological disturbances. Geomagn. Aeron. 2019, 59, 458–466. [Google Scholar] [CrossRef]
- Karpov, I.V.; Karpov, M.I.; Borchevkina, O.P.; Yakimova, G.A.; Korenkova, N.A. Spatial and temporal variations of the ionosphere during meteorological disturbances in December 2010. Russ. J. Phys. Chem. B 2019, 13, 714–719. [Google Scholar] [CrossRef]
- Koucká Knížová, P.; Podolská, K.; Potužníková, K.; Boška, J.; Kozubek, M. Evidence of vertical coupling: Meteorological storm Fabienne on 23 September 2018 and its related effects observed up to the ionosphere. Ann. Geophys. 2020, 38, 73–93. [Google Scholar] [CrossRef]
- Martinis, C.R.; Manzano, J.R. The influence of active meteorological systems on the ionosphere F region. Ann. Geofis. 1999, 42, 1–7. [Google Scholar] [CrossRef]
- Isaev, N.; Kostin, V.; Belyaev, G.; Ovcharenko, O.; Trushkina, E. Disturbances of the topside ionosphere caused by typhoons. Geomagn. Aeron. 2010, 50, 243–255. [Google Scholar] [CrossRef]
- Isaev, N.; Sorokin, V.; Chmyrev, V.; Serebryakova, O.N.; Yashchenko, A.K. Disturbance of the electric field in the ionosphere by sea storms and typhoons. Cosmic Res. 2002, 40, 547–553. [Google Scholar] [CrossRef]
- Polyakova, A.S.; Perevalova, N.P. Comparative analysis of TEC disturbances over tropical cyclone zones in the North-West Pacific Ocean. Adv. Space Res. 2013, 52, 1416–1426. [Google Scholar] [CrossRef]
- Chou, M.Y.; Lin, C.C.H.; Yue, J.; Tsai, H.F.; Sun, Y.Y.; Liu, J.Y.; Chen, C.H. Concentric traveling ionosphere disturbances triggered by Super Typhoon Meranti (2016). Geophys. Res. Lett. 2017, 44, 1219–1226. [Google Scholar] [CrossRef]
- Li, W.; Yue, J.; Yang, Y.; Li, Z.; Guo, J.; Pan, Y.; Zhang, K. Analysis of ionospheric disturbances associated with powerful cyclones in East Asia and North America. J. Atmos. Sol. Terr. Phys. 2017, 161, 43–54. [Google Scholar] [CrossRef]
- Hocke, K.; Schlegel, K. A review of atmospheric gravity waves and travelling ionospheric disturbances: 1982–1995. Ann. Geophys. 1996, 14, 917–940. [Google Scholar] [CrossRef]
- Golubkov, G.V.; Bychkov, V.L.; Gotovtsev, V.O.; Adamson, S.O.; Dyakov, Y.A.; Rodionov, I.D.; Golubkov, M.G. Glow of Heavy Dust Particles in Earth’s Atmosphere during an Earthquake. Russ. J. Phys. Chem. B 2020, 14, 351–354. [Google Scholar] [CrossRef]
- Karpov, M.I.; Karpov, I.V.; Borchevkina, O.P.; Yakimova, G.A.; Korenkova, N.A. Ionospheric disturbances during meteorological storms. Geomagn. Aeron. 2020, 60, 611–618. [Google Scholar] [CrossRef]
- Borchevkina, O.P.; Korenkova, N.A.; Leshchenko, V.S.; Klimenko, M.K.; Karpov, I.V.; Radievskii, A.V.; Bessarab, F.S.; Vlasov, V.I.; Kotova, D.S.; Nosikov, I.A.; et al. Complex of radiophysical, geomagnetic, and meteorological observations (IZMIRAN), Kaliningrad Branch. Russ. J. Phys. Chem. B 2020, 14, 883–891. [Google Scholar] [CrossRef]
- Grigoriev, G.I. Acoustic-gravity waves in the Earth’s atmosphere (Review). Radiophys. Quant. Electron. 1999, 42, 1–21. [Google Scholar] [CrossRef]
- Nekrasov, A.; Shalimov, S.; Shukla, P.K.; Stenflo, L. Nonlinear disturbances in the ionosphere due to acoustic gravity waves. J. Atmos. Terr. Phys. 1995, 57, 737–741. [Google Scholar] [CrossRef]
- Yigit, E.; Medvedev, A.S. Internal wave coupling processes in Earth’s atmosphere. Adv. Space Res. 2015, 55, 983–1003. [Google Scholar] [CrossRef]
- Khaykin, S.M.; Hauchecorne, A.; Mze, N.; Keckhut, P. Seasonal variation of gravity wave activity at midlatitudes from 7 years of COSMIC GPS and Rayleigh lidar temperature observations. Geophys. Res. Lett. 2015, 42, 1251–1258. [Google Scholar] [CrossRef]
- Mze, N.; Hauchecorne, A.; Keckhut, P.; Thetis, M. Vertical distribution of gravity wave potential energy from long-term Rayleigh lidar data at a northern middle-latitude site. J. Geophys. Res. Atmos. 2014, 119, 12069–12083. [Google Scholar] [CrossRef]
- Gong, S.; Yang, G.; Xu, J.; Liu, X.; Li, Q. Gravity wave propagation from the stratosphere into the mesosphere studied with lidar, meteor radar, and TIMED/SABER. Atmosphere 2019, 10, 81. [Google Scholar] [CrossRef]
- Matvienko, G.G.; Babushkin, P.A.; Bobrovnikov, S.M.; Borovoi, A.G.; Bochkovskii, D.A.; Galileiskii, V.P.; Grishin, A.I.; Dolgii, S.I.; Elizarov, A.I.; Kokarev, D.V.; et al. Laser and optical sounding of the atmosphere. Atmos. Ocean. Opt. 2020, 33, 51–68. [Google Scholar] [CrossRef]
- Yuan, T.; Heale, C.J.; Snively, B.; Cai, X.; Pautet, P.D.; Fish, C.; Zhao, Y.; Tailor, M.J.; Pendleton, W.R., Jr.; Wickwar, V.; et al. Evidence of dispersion and refraction of a spectrally broad gravity wave packet in the mesopause region observed by the Na lidar and mesospheric temperature mapper above Logan, Utah. J. Geophys. Res. Atmos. 2015, 121, 579–594. [Google Scholar] [CrossRef]
- Huang, K.M.; Liu, H.; Liu, A.Z.; Zhang, S.D.; Huang, C.M.; Gong, Y.; Ning, W.H. Investigation on spectral characteristics of gravity waves in the MLT using lidar observations at Andes. J. Geophys. Res. Space Phys. 2021, 126. e2020JA028918:1–e2020JA028918:18. [Google Scholar] [CrossRef]
- Weitkamp, C. Lidar: Range-Resolved Optical Remote Sensing of the Atmosphere; Springer: New York, NY, USA, 2005; p. 456. ISBN 978-0-387-40075-4. [Google Scholar] [CrossRef]
- Azeem, I.; Vadas, S.L.; Crowley, G.; Makela, J.J. Traveling ionospheric disturbances over the United States induced by gravity waves from the 2011 Tohoku tsunami and comparison with gravity wave dissipative theory. J. Geophys. Res. Space Phys. 2017, 122, 3430–3447. [Google Scholar] [CrossRef]
- Shalimov, S.L.; Olshanskaya, E.V. On Ionospheric Variations Recorded by GPS during Meteotsunami. Izv. Atmos. Ocean. Phys. 2020, 56, 576–584. [Google Scholar] [CrossRef]
- Chen, J.; Zhang, X.; Ren, X.; Zhang, J.; Freeshah, M.; Zhao, Z. Ionospheric disturbances detected during a typhoon based on GNSS phase observations: A case study for typhoon Mangkhut over Hong Kong. Adv. Space Res. 2020, 66, 1743–1753. [Google Scholar] [CrossRef]
- Yasyukevich, Y.V.; Kiselev, A.V.; Zhivetiev, I.V.; Edemskiy, I.K.; Syrovatskii, S.V.; Maletckii, B.M.; Vesnin, A.M. SIMuRG: System for Ionosphere Monitoring and Research from GNSS. GPS Solut. 2020, 24, 69. [Google Scholar] [CrossRef]
- Golubkov, G.V.; Manzhelii, M.I.; Lushnikov, A.A. Radiochemical physics of the upper Earth’s atmosphere. Russ. J. Phys. Chem. B 2014, 8, 604–611. [Google Scholar] [CrossRef]
- Golubkov, G.V.; Manzhelii, M.I.; Berlin, A.A.; Lushnikov, A.A. Fundamentals of radiochemical physics of the Earth’s atmosphere. Russ. J. Phys. Chem. B 2016, 10, 77–90. [Google Scholar] [CrossRef]
- Kuverova, V.V.; Adamson, S.O.; Berlin, A.A.; Bychkov, V.L.; Dmitriev, A.V.; Dyakov, Y.A.; Eppelbaum, L.V.; Golubkov, G.V.; Lushnikov, A.A.; Manzhelii, M.I.; et al. Chemical physics of D and E layers of the ionosphere. Adv. Space Res. 2019, 64, 1876–1886. [Google Scholar] [CrossRef]
- Golubkov, G.V.; Manzhelii, M.I.; Berlin, A.A.; Bezuglov, N.N.; Klyucharev, A.N.; Borchevkina, O.P.; Adamson, S.O.; Dyakov, Y.A.; Karpov, I.V.; Morozov, I.I.; et al. Remote sensing of the Earth’s surface using GPS signals. Russ. J. Phys. Chem. B 2021, 15, 362–365. [Google Scholar] [CrossRef]
- Kshevetskii, S.P. Analytical and numerical investigation of nonlinear internal gravity waves. Nonlin. Proc. Geophys. 2001, 8, 37–53. [Google Scholar] [CrossRef][Green Version]
- Akhmedov, R.R.; Kunitsyn, V.E. Simulation of the ionospheric disturbances caused by earthquakes and explosions. Geomagn. Aeron. 2004, 44, 95–101. [Google Scholar]
- Yigit, E.; Medvedev, A.S.; Aylward, A.D.; Hartogh, P.; Harris, M.J. Modeling the effects of gravity wave momentum deposition on the general circulation above the turbopause. J. Geophys. Res. Atmos. 2009, 114. D07101:1–D07101:14. [Google Scholar] [CrossRef]
- Gavrilov, N.M.; Kshevetskii, S.P.; Koval, A.V. Verifications of the high-resolution numerical model and polarization relations of atmospheric acoustic-gravity waves. Geosci. Mod. Dev. 2015, 8, 1831–1838. [Google Scholar] [CrossRef]
- Dyakov, Y.A.; Kurdyaeva, Y.A.; Borchevkina, O.P.; Karpov, I.V.; Adamson, S.O.; Golubkov, G.V.; Olkhov, O.A.; Peskov, V.D.; Rodionov, A.I.; Rodionova, I.P.; et al. Vertical propagation of acoustic gravity waves from the lower atmosphere during a solar eclipse. Russ. J. Phys. Chem. B 2020, 14, 355–361. [Google Scholar] [CrossRef]
- Kurdyaeva, Y.; Borchevkina, O.; Karpov, I.; Kshevetskii, S. Thermospheric disturbances caused by the propagation of acoustic-gravity waves from the lower atmosphere during a solar eclipse. Adv. Space Res. 2021, 68, 1390–1400. [Google Scholar] [CrossRef]
- Kurdyaeva, Y.; Kulichkov, S.; Kshevetskii, S.; Borchevkina, O.; Golikova, E. Propagation to the upper atmosphere of acoustic-gravity waves from atmospheric fronts in the Moscow region. Ann. Geophys. 2019, 37, 447–454. [Google Scholar] [CrossRef]
- Kshevetskii, S.; Kurdyaeva, Y.; Kulichkov, S.; Borchevkina, O.; Gavrilov, N. Simulation of propagation of acoustic-gravity waves generated by tropospheric front instabilities into the upper atmosphere. Pure Appl. Geophys. 2020, 177, 5567–5584. [Google Scholar] [CrossRef]
- Cai, X.; Burns, A.G.; Wang, W.; Qian, L.; Solomon, S.C.; Easters, R.W.; Pedatella, N.; Daniell, R.E.; McClintock, W.E. The two-dimensional evolution of thermospheric ΣO/N2 response to weak geomagnetic activity during solar-minimum observed by GOLD. Geophys. Res. Lett. 2020, 47. e2020GL088838:1–e2020GL088838:9. [Google Scholar] [CrossRef]
- Korshunov, V.A. Automated algorithm of data processing of two-wavelength lidar sensing at slant paths. Ecol. Syst. Dev. 2009, 12, 3–10. [Google Scholar]
- Korshunov, V.A. Retrieval of integral parameters of tropospheric aerosol from two-wavelength lidar sensing. Izv. Atmos. Ocean. Phys. 2007, 43, 618–633. [Google Scholar] [CrossRef]
- Astafyeva, N.M. Wavelet analysis: Basic theory and some applications. Phys. Usp. 1996, 39, 1085–1108. [Google Scholar] [CrossRef]
- Shagimuratov, I.I.; Chernyak, Y.V.; Zakharenkova, I.E.; Yakimova, G.A. Use of total electron content maps for analysis of spatial-temporal structures of the ionosphere. Russ. J. Phys. Chem. B 2013, 7, 656–662. [Google Scholar] [CrossRef]
- Shagimuratov, I.I.; Cherniak, Y.V.; Zakharenkova, I.E.; Yakimova, G.A.; Tepenitsyna, N.Y.; Efishov, I.I. Internet service for generating GPS/GLONASS maps of ionospheric total electron content over the European region. Sovr. Probl. Dist. Zond. Zeml. Kosm. 2016, 13, 197–209. [Google Scholar] [CrossRef]
- Hersbach, H.; Bell, B.; Berrisford, P.; Hirahara, S.; Horányi, A.; Muñoz-Sabater, J.; Nicolas, J.; Peubey, C.; Radu, R.; Schepers, D.; et al. The ERA5 global reanalysis. Q. J. R. Met. Soc. 2020, 146, 1999–2049. [Google Scholar] [CrossRef]
- Kshevetskii, S.P. Modeling of propagation of internal gravity waves in gases. Comput. Math. Math. Phys. 2001, 41, 273–288. [Google Scholar]
- Kshevetskii, S.P. Numerical simulation of nonlinear internal gravity waves. Comput. Math. Math. Phys. 2001, 12, 1777–1791. [Google Scholar]
- Kurdyaeva, Y.A.; Kshevetskii, S.P.; Gavrilov, N.M.; Kulichkov, S.N. Correct Boundary Conditions for the High-Resolution Model of Nonlinear Acoustic-Gravity Waves Forced by Atmospheric Pressure Variations. Pure Appl. Geophys. 2018, 175, 3639–3652. [Google Scholar] [CrossRef]
- Gavrilov, N.M.; Kshevetskii, S.P. Three-dimensional numerical simulation of nonlinear acoustic-gravity wave propagation from the troposphere to the thermosphere. Earth Planets Space 2014, 66. 88:1–88:8. [Google Scholar] [CrossRef]
- Karpov, I.; Kshevetskii, S. Numerical study of heating the upper atmosphere by acoustic-gravity waves from a local source on the Earth’s surface and influence of this heating on the wave propagation conditions. J. Atmos. Sol. Terr. Phys. 2017, 164, 89–96. [Google Scholar] [CrossRef]
- Kshevetskii, S.P.; Kulichkov, S.N. Effects of internal gravity waves from convective clouds on atmospheric pressure and spatial temperature-disturbance distribution. Izv. Atmos. Ocean. Phys. 2015, 51, 42–48. [Google Scholar] [CrossRef]
- Gavrilov, N.M.; Kshevetskii, S.P. Numerical modeling of propagation of breaking nonlinear acoustic-gravity waves from the lower to the upper atmosphere. Adv. Space Res. 2013, 51, 1168–1174. [Google Scholar] [CrossRef]
- Kshevetskii, S.; Gavrilov, N. Vertical propagation of nonlinear gravity waves and their breaking in the atmosphere. Geomagn. Aeron. 2003, 43, 69–76. [Google Scholar]
- Vasil’ev, P.A.; Karpov, I.V.; Kshevetskii, S.P. Simulation of Internal Gravity Wave Propagation Due to Sudden Stratospheric Warming. Russ. J. Phys. Chem. B 2017, 11, 1028–1032. [Google Scholar] [CrossRef]
- Blanc, E.; Farges, T.; Le Pichon, A.; Heinrich, P. Ten-year observations of gravity waves from thunderstorms in western Africa. J. Geophys. Res. Atmos. 2014, 119, 6409–6418. [Google Scholar] [CrossRef]
- Azeem, I.; Barlage, M. Atmosphere-ionosphere coupling from convectively generated gravity waves. Adv. Space Res. 2018, 61, 1931–1941. [Google Scholar] [CrossRef]
- Schubert, G.; Hickey, M.P.; Walterscheid, R.L. Physical processes in acoustic wave heating of the thermosphere. J. Geophys. Res. 2005, 110. D07106:1–D07106:5. [Google Scholar] [CrossRef]
- Hickey, M.P.; Walterscheid, R.L.; Schubert, G. Gravity wave heating and cooling of the thermosphere: Sensible heat flux and viscous flux of kinetic energy. J. Geophys. Res. 2011, 116. A12326:1–A12326:9. [Google Scholar] [CrossRef]
- Vadas, S.L.; Liu, H.-L. Generation of large-scale gravity waves and neutral winds in the thermosphere from the dissipation of convectively generated gravity waves. J. Geophys. Res. Space Phys. 2009, 114. A10310:1–A10310:25. [Google Scholar] [CrossRef]
- Rishbeth, H. How the thermospheric circulation affects the ionospheric F2-layer. J. Atmos. Sol. Terr. Phys. 1998, 60, 1385–1402. [Google Scholar] [CrossRef]


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Borchevkina, O.P.; Kurdyaeva, Y.A.; Dyakov, Y.A.; Karpov, I.V.; Golubkov, G.V.; Wang, P.K.; Golubkov, M.G. Disturbances of the Thermosphere and the Ionosphere during a Meteorological Storm. Atmosphere 2021, 12, 1384. https://doi.org/10.3390/atmos12111384
Borchevkina OP, Kurdyaeva YA, Dyakov YA, Karpov IV, Golubkov GV, Wang PK, Golubkov MG. Disturbances of the Thermosphere and the Ionosphere during a Meteorological Storm. Atmosphere. 2021; 12(11):1384. https://doi.org/10.3390/atmos12111384
Chicago/Turabian StyleBorchevkina, Olga P., Yuliya A. Kurdyaeva, Yurii A. Dyakov, Ivan V. Karpov, Gennady V. Golubkov, Pao K. Wang, and Maxim G. Golubkov. 2021. "Disturbances of the Thermosphere and the Ionosphere during a Meteorological Storm" Atmosphere 12, no. 11: 1384. https://doi.org/10.3390/atmos12111384
APA StyleBorchevkina, O. P., Kurdyaeva, Y. A., Dyakov, Y. A., Karpov, I. V., Golubkov, G. V., Wang, P. K., & Golubkov, M. G. (2021). Disturbances of the Thermosphere and the Ionosphere during a Meteorological Storm. Atmosphere, 12(11), 1384. https://doi.org/10.3390/atmos12111384







