Two Ways to Quantify Korean Drought Frequency: Partial Duration Series and Bivariate Exponential Distribution, and Application to Climate Change
Abstract
1. Introduction
2. Methods
2.1. Data
2.2. Partial Duration Series
- Obtain a moving average time series, , for each duration from the monthly SPI time series, .
- The smallest sample in time series is selected first, and the selected sample is deleted. Then, the sample series as many as the number of months of the duration before and after the deleted sample is deleted to form a time series .
- The smallest sample among time series is selected as the second rank, and the selected sample is deleted. Then, samples as many as the number of months in the duration before and after the deleted sample are deleted to form a new time series .
- Create a PDS by repeating this procedure and selecting the smallest samples.
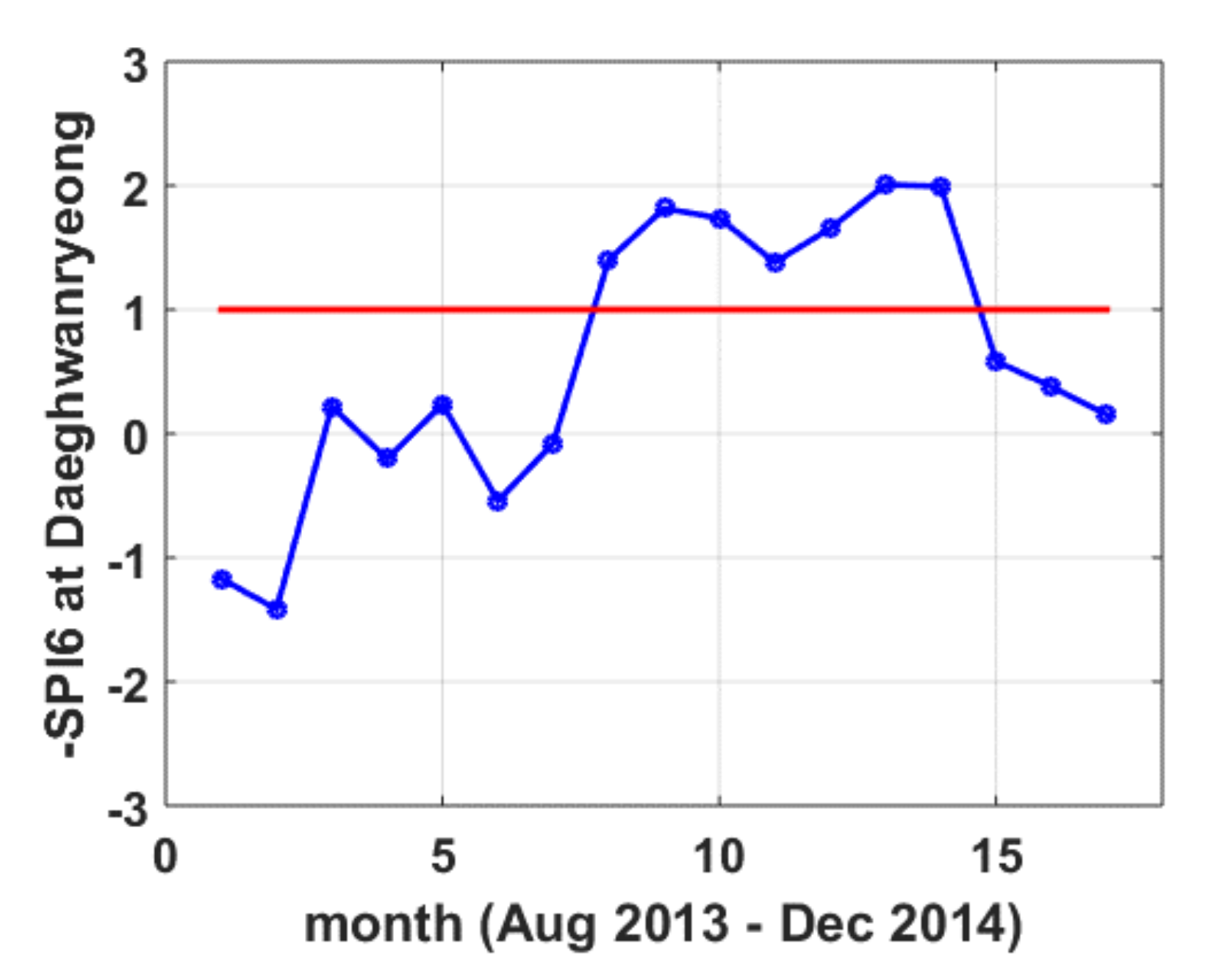
2.3. Drought Events
2.4. Bivariate Exponential Distribution
3. Results
3.1. Frequency Analysis Using Partial Duration Series
3.2. Frequency Analysis Using Bivariate Exponential Distribution
4. Discussion and Applications
4.1. Comparison of Two Kinds of SDF Curves
4.2. Applicability for Awareness of Drought Progress
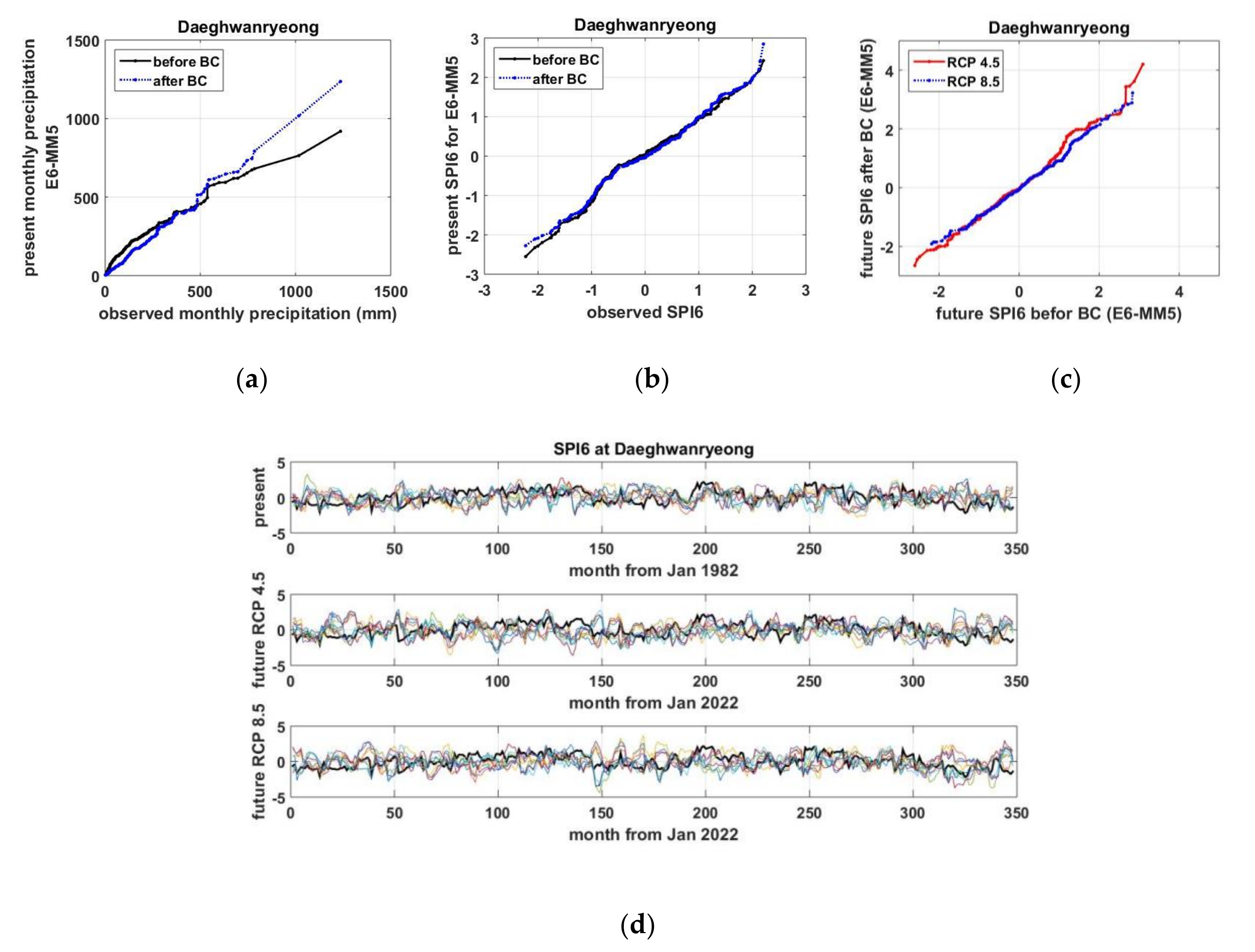
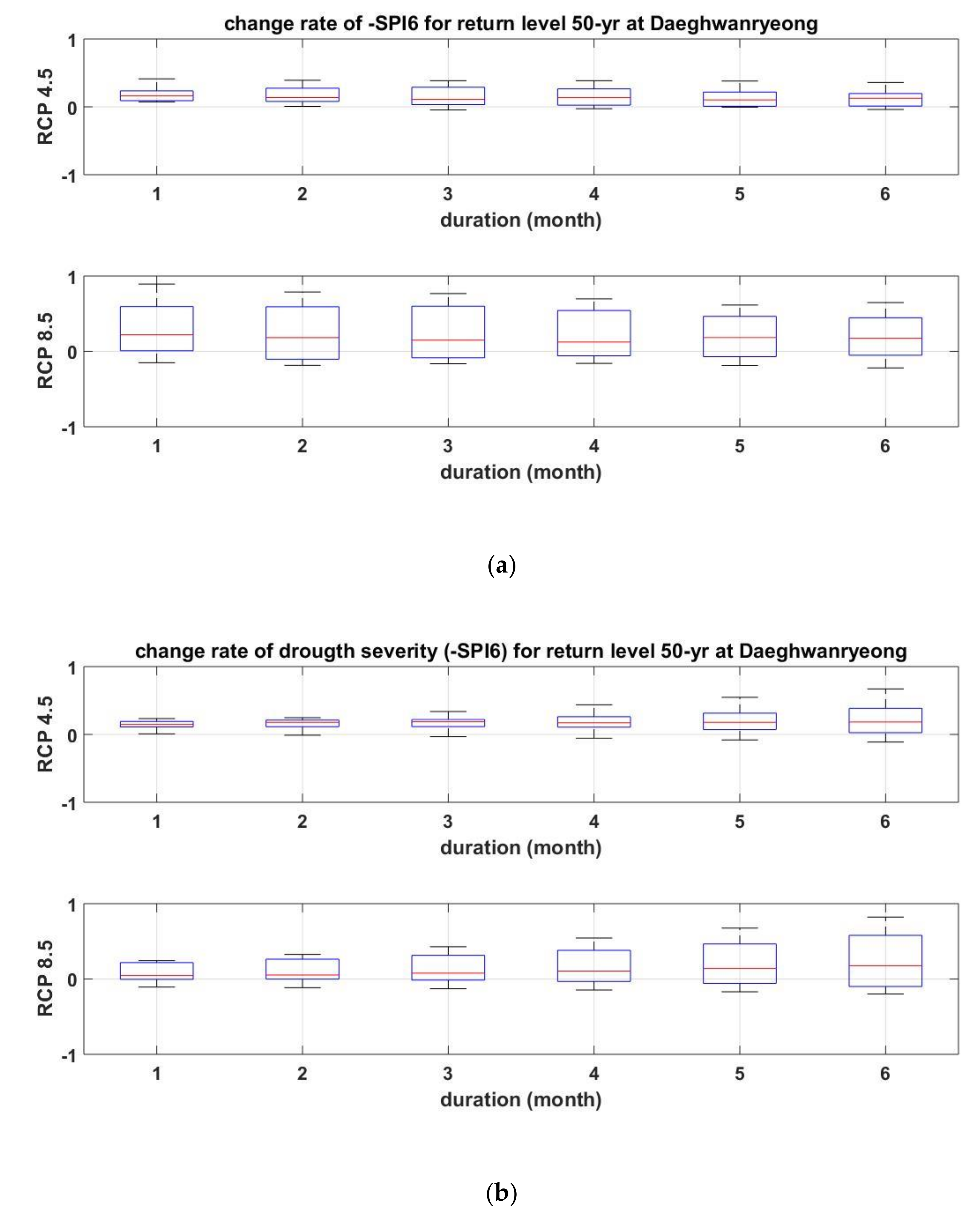
4.3. Climate Change Drought Impact Assessment
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Chang, Y.; Kim, S.; Choi, G. A study of drought spatio-temporal characteristics using SPI-EOF analysis. J. Korea Water Resour. Assoc. 2006, 39, 691–702. [Google Scholar] [CrossRef][Green Version]
- Kim, S.; Kim, B.; Ahn, T.; Kim, H. Spatio-temporal characterization of Korean drought using severity-area-duration curve analysis. Water Environ. J. 2011, 25, 22–30. [Google Scholar] [CrossRef]
- Yoo, C.; Kim, D. Evaluation of drought events using the rectangular pulses Poisson process model. J. Korea Water Resour. Assoc. 2006, 39, 373–382. [Google Scholar]
- McKee, T.; Doesken, N.; Kleist, J. Drought monitoring with multiple time scales preprints. In Proceedings of the 9th Conference on Applied Climatology, Dallas, TX, USA, 15–20 January 1995; pp. 233–236. [Google Scholar]
- Kim, D.; Yoo, C. Analysis of spatial distribution of droughts in Korea through drought severity-duration-frequency analysis. J. Korea Water Resour. Assoc. 2006, 39, 745–754. [Google Scholar]
- Ghosh, S.; Mujumdar, P. Nonparametric methods for modeling GCM and scenario uncertainty in drought assessment. Water Resour. Res. 2007, 43, W07405–W07406. [Google Scholar] [CrossRef]
- Lehner, B.; Doll, P.; Alcamo, J. Estimating the impact of global change on flood and drought risks in Europe: A continental, integrated analysis. Clim. Chang. 2008, 75, 273–299. [Google Scholar] [CrossRef]
- Mishra, A.; Singh, V. Analysis of drought severity-area frequency curves using a general circulation model and scenario uncertainty. J. Geophys. Res. Space Phys. 2009, 114, D06120. [Google Scholar] [CrossRef]
- Vidal, J.; Wade, S. A multimodel assessment of future climatological droughts in the United Kingdom. Int. J. Clim. 2007, 29, 2056–2071. [Google Scholar] [CrossRef]
- Lee, J.; Kim, C. Derivation of drought severity-duration-frequency curves using drought frequency analysis. J. Korea Water Resour. Assoc. 2011, 44, 889–902. [Google Scholar] [CrossRef]
- Kang, S.; Moon, J. Drought Analysis using SC-PDSI and Derivation of Drought Severity-Duration-Frequency Curves in North Korea. J. Korea Water Resour. Assoc. 2014, 47, 813–824. [Google Scholar] [CrossRef]
- Kim, C.; Park, M.; Lee, J. Analysis of climate change impacts on the spatial and frequency patterns of drought using a potential drought hazard mapping approach. Int. J. Clim. 2014, 34, 61–80. [Google Scholar] [CrossRef]
- Park, M.; Sim, H.; Park, Y.; Kim, S. Drought severity-duration-frequency analysis based on KMA 1-km resolution RCP Scenario. J. Korean Soc. Hazard Mitig. 2015, 15, 347–355. [Google Scholar] [CrossRef][Green Version]
- Kim, J.; Lee, J.; Park, M.; Joo, J. Effect of Climate Change Scenarios and Regional Climate Models on the Drought Severity-Duration-Frequency Analysis. J. Korean Soc. Hazard Mitig. 2016, 16, 351–361. [Google Scholar] [CrossRef][Green Version]
- Yoon, Y.; Park, M. Regional drought frequency analysis of monthly rainfall data by the method of L-moment. J. Korea Water Resour. Assoc. 1997, 30, 55–62. [Google Scholar]
- Kao, S.; Govindaraju, R. A copula-based joint deficit index for droughts. J. Hydrol. 2010, 380, 121–134. [Google Scholar] [CrossRef]
- Dalezios, N.; Loukas, A.; Vasiliades, L.; Liakopoulos, E. Severity-duration-frequency analysis of droughts and wet periods in Greece. Hydrol. Sci. J. 2000, 45, 751–769. [Google Scholar] [CrossRef]
- Palmer, W. Meteorological Drought; U.S. Department of Commerce: Washington, DC, USA, 1965.
- Shiau, J.; Shen, H. Recurrence analysis of hydrologic droughts of differing severity. J. Water Resour. Plan. Manag. 2001, 127, 30–40. [Google Scholar] [CrossRef]
- Shiau, J. Fitting drought duration and severity with two-dimensional copulas. Water Resour. Manag. 2006, 20, 795–815. [Google Scholar] [CrossRef]
- Yeon, J.; Byun, S.; Lee, J.; Kim, T. Evaluation of droughts in Seoul using two-dimensional drought frequency analysis. J. Korea Water Resour. Assoc. 2007, 40, 335–343. [Google Scholar] [CrossRef]
- Shiau, J.; Modarres, R. Copula-based drought severity–duration-frequency analysis in Iran. Meteorol. Appl. 2009, 16, 481–489. [Google Scholar] [CrossRef]
- Ganguli, P.; Reddy, M. Risk assessment of droughts in Gujarat using bivariate copulas. Water Resour. Manag. 2012, 26, 3301–3327. [Google Scholar] [CrossRef]
- Mirabbasi, R.; Fakheri-Fard, A.; Dinpashoh, Y. Bivariate drought frequency analysis using the copula method. Theor. Appl. Clim. 2012, 108, 191–206. [Google Scholar] [CrossRef]
- Chen, L.; Singh, V.; Guo, S.; Mishra, A.; Guo, J. Drought analysis using copulas. J. Hydrol. Eng. 2013, 18, 797–808. [Google Scholar] [CrossRef]
- Halwatura, D.; Lechner, A.; Arnold, S. Drought severity–duration-frequency curves: A foundation for risk assessment and planning tool for ecosystem establishment in post-mining landscape. Hydrol. Earth Syst. Sci. 2015, 19, 1069–1091. [Google Scholar] [CrossRef]
- Chun, S.; Kim, Y.; Kwon, H. Drought frequency analysis using hidden markov chain model and bivariate copula function. J. Korea Water Resour. Assoc. 2015, 48, 969–979. [Google Scholar] [CrossRef]
- Kim, J.; So, B.; Kim, T.; Kwon, H. A development of trivariate drought frequency analysis approach using copula function. J. Korea Water Resour. Assoc. 2016, 49, 823–833. [Google Scholar]
- Yu, J.; Yoo, J.; Lee, J.; Kim, T. Estimation of drought risk through the bivariate drought frequency analysis using copula functions. J. Korea Water Resour. Assoc. 2016, 49, 217–225. [Google Scholar] [CrossRef]
- Kwon, M.; Sung, J.; Kim, T.; Ahn, J. Drought assessment by bivariate frequency analysis using standardized precipitation index and precipitation deficit: Focused on Han river basin. J. Korea Water Resour. Assoc. 2018, 51, 875–886. [Google Scholar]
- Ryu, J.; Ahn, J.; Kim, S. An application of drought severity-area-duration curves using copulas-based joint drought index. J. Korea Water Resour. Assoc. 2012, 45, 1043–1050. [Google Scholar] [CrossRef]
- Stall, J. Low Flows of Illinois Streams for Impounding Reservoir Design, Bulletin 51; Illinois State Water Survey: Champaign, IL, USA, 1964. [Google Scholar]
- WMO. Experts Agree on a Universal Drought Index to Cope with Climate Risks. World Meteorological Organization Press Release No. 872. Available online: http://www.mondialisations.org/php/public/art.php?id=32782lan=EN&lan=EN (accessed on 28 July 2019).
- KMA. Hydro-Meteorologic Drought Information System. Available online: https://hydro.kma.go.kr/help/menu200.do (accessed on 3 August 2019).
- Hosking, J. L-moments: Analysis and estimation of distributions using linear combinations of order statistics. J. R. Stat. Soc. 1990, 52, 105–124. [Google Scholar] [CrossRef]
- Yevjevich, V. Objective Approach to Definitions and Investigations of Continental Hydrologic Droughts; Hydrology Paper 23; Colorado State University: Fort Collins, CO, USA, 1967. [Google Scholar]
- Nagao, M.; Kadoya, M. Two variate exponential distribution and its numerical table for engineering applications. Bull. Disaster Prev. Res. Inst. 1971, 20, 183–215. [Google Scholar]
- Yue, S. Applicability of the Nagao–Kadoya bivariate exponential distribution for modeling two correlated exponentially distributed variates. Stoch. Environ. Res. Risk Assess. 2001, 15, 244–260. [Google Scholar] [CrossRef]
- Cordova, J.; Rodriguez-Iturbe, I. On the probabilistic structure of storm surface runoff. Water Resour. Res. 1985, 21, 755–763. [Google Scholar] [CrossRef]
- Goel, N.; Kurothe, R.; Mathur, B.; Vogel, R. A derived flood frequency distribution for correlated rainfall intensity and duration. J. Hydrol. 2000, 228, 56–67. [Google Scholar] [CrossRef]
- Ashkar, F.; Jabi, N.; Issa, M. A bivariate analysis of the volume and duration of low-flow events. Stoch. Environ. Res. Risk Assess. 1998, 12, 97–116. [Google Scholar] [CrossRef]
- Park, C.; Cha, D.; Kim, G.; Lee, G.; Lee, D.; Suh, M.; Hong, S.; Ahn, J.; Min, S. Evaluation of summer precipitation over Far East Asia and South Korea simulated by multiple regional climate models. Int. J. Clim. 2019. [Google Scholar] [CrossRef]
- Teutschbein, C.; Seibert, J. Regional climate models for hydrological impact studies at the catchment scale: A review of recent modeling strategies. Geogr. Compass 2010, 4, 834–860. [Google Scholar] [CrossRef]
- Arnbjerg-Nielsen, K.; Willems, P.; Olsson, J.; Beecham, S.; Pathirana, A.; Gregersen, I.; Madsen, H.; Nguyen, V. Impacts of climate change on rainfall extremes and urban drainage systems: A review. Water Sci. Technol. 2013, 68, 16–28. [Google Scholar] [CrossRef]
- Lee, O.; Sim, I.; Kim, S. Application of the non-stationary peak-over-threshold methods for deriving rainfall extremes from temperature projections. J. Hydrol. 2019. [Google Scholar] [CrossRef]
- Li, J.; Evans, J.; Johnson, F.; Sharma, A. A comparison of methods for estimating climate change impact on design rainfall using a high-resolution RCM. J. Hydrol. 2017, 547, 413–427. [Google Scholar] [CrossRef]
- Lenderink, G.; Fowler, H. Hydroclimate: Understanding rainfall extremes. Nat. Clim. Chang. 2017, 7, 391–393. [Google Scholar] [CrossRef]
- Lee, O.; Kim, S. Estimation of Future Probable Maximum Precipitation in Korea Using Multiple Regional Climate Models. Water 2018, 10, 637. [Google Scholar] [CrossRef]
- Choi, J.; Lee, O.; Jang, J.; Jang, S.; Kim, S. Future intensity–depth–frequency curves estimation in Korea under representative concentration pathway scenarios of Fifth assessment report using scale-invariance method. Int. J. Clim. 2019, 39, 887–900. [Google Scholar] [CrossRef]
- Spinoni, J.; Barbosa, P.; Bucchignani, E.; Cassano, J.; Cavazos, T.; Christensen, J.; Christensen, O.; Coppola, E.; Evans, J.; Geyer, B.; et al. Future global meteorological drought hot spots: A study based on CORDEX Data. J. Clim. 2020, 33, 3635–3661. [Google Scholar] [CrossRef]
- Vicente-Serrano, S.; Beguería, S.; López-Moreno, J. A multiscalar drought index sensitive to global warming: The standardized precipitation evapotranspiration index. J. Clim. 2010, 23, 1696–1718. [Google Scholar] [CrossRef]
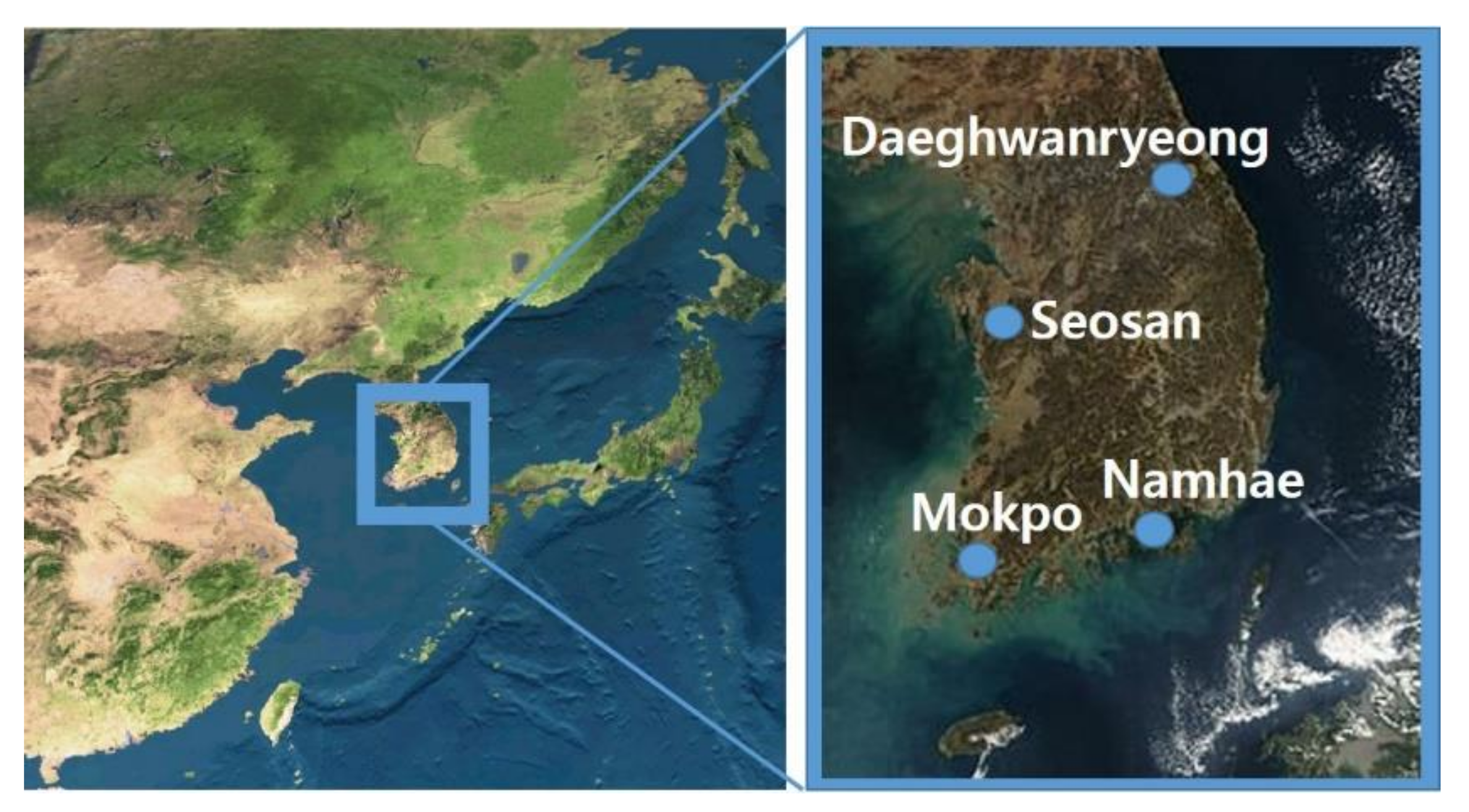
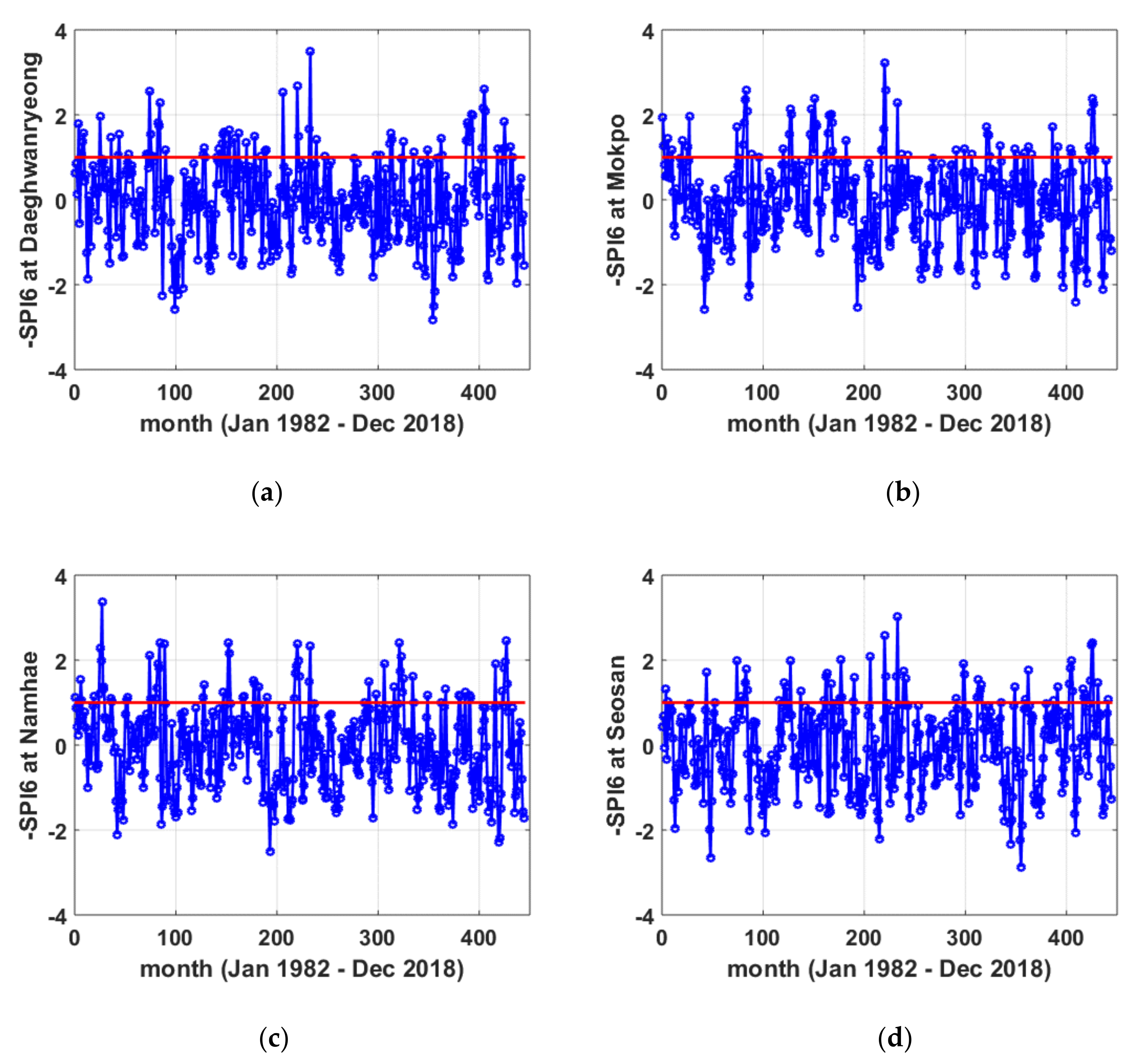





| Site | Daeghwanryeong | Mokpo | Namhae | Seosan | |
|---|---|---|---|---|---|
| Duration | |||||
| 1 month | 37 | 37 | 37 | 37 | |
| 2 months | 37 | 37 | 37 | 37 | |
| 3 months | 37 | 37 | 37 | 37 | |
| 4 months | 37 | 37 | 37 | 37 | |
| 5 months | 37 | 37 | 37 | 37 | |
| 6 months | 36 | 34 | 37 | 36 | |
| 7 months | 31 | 29 | 31 | 34 | |
| 8 months | 25 | 28 | 26 | 31 | |
| 9 months | 24 | 24 | 23 | 29 | |
| 10 months | 23 | 20 | 20 | 24 | |
| 11 months | 22 | 17 | 20 | 20 | |
| 12 months | 21 | 16 | 17 | 17 | |
| Site | Daeghwanryeong | Mokpo | Namhae | Seosan | |
|---|---|---|---|---|---|
| Duration | |||||
| 1 month | May 2001 | Apr. 2000 | Mar. 1984 | May 2001 | |
| 2 months | May 2001 | May 2000 | Mar. 1984 | Jun. 2017 | |
| 3 months | Oct. 2015 | May 2000 | Mar. 1984 | Jun. 2017 | |
| 4 months | Oct. 2015 | Dec. 1988 | Apr. 1984 | Jun. 2017 | |
| 5 months | Oct. 2015 | Dec. 1988 | May 1984 | Jul. 2017 | |
| 6 months | Sep. 2014 | Sep. 1994 | May 1984 | Aug. 2017 | |
| 7 months | Sep. 2014 | Sep. 1994 | Jun. 1984 | Sep. 2017 | |
| 8 months | Oct. 2014 | Sep. 1994 | Jul. 1984 | Nov. 2017 | |
| 9 months | Nov. 2014 | Oct. 1994 | Aug. 1984 | Nov. 2017 | |
| 10 months | Dec. 2014 | Feb. 1996 | Sep. 1984 | Nov. 1988 | |
| 11 months | Jan. 2015 | Feb. 1996 | Oct. 1984 | Dec. 1988 | |
| 12 months | Feb. 2015 | Feb. 1996 | Nov. 1984 | Dec. 1988 | |
| Site | Number of Events | Severity (-) | Duration (Month) | ||
|---|---|---|---|---|---|
| Mean | Standard Deviation | Mean | Standard Deviation | ||
| Daeghwanryeong | 38 | 0.4462 | 0.3898 | 1.7632 | 1.2398 |
| Mokpo | 33 | 0.4098 | 0.3489 | 1.9697 | 1.8789 |
| Namhae | 33 | 0.4500 | 0.3502 | 2.0303 | 1.4892 |
| Seosan | 35 | 0.4636 | 0.3541 | 1.6286 | 1.0596 |
| Site | Daeghwan-Ryeong | Mokpo | Namhae | Seosan | |
|---|---|---|---|---|---|
| Maximum severity event | Mean value of –SPI | 2.5794 | 2.2899 | 2.3726 | 2.3233 |
| Duration (month) | 2 | 1 | 1 | 2 | |
| Occurrence date | 2001/04 | 2001/05 | 1989/05 | 2001/04 | |
| Return level (year) | 33.6 | 26.1 | 23.7 | 18.4 | |
| Maximum Duration event | Duration (month) | 7 | 7 | 6 | 5 |
| Mean value of –SPI | 1.7127 | 1.7347 | 1.9222 | 1.3078 | |
| Occurrence date | 2014/03 | 1994/03 | 1983/12 | 2007/12 | |
| Return level (year) | 51.6 | 39.2 | 21.5 | 22.8 | |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Won, J.; Choi, J.; Lee, O.; Park, M.J.; Kim, S. Two Ways to Quantify Korean Drought Frequency: Partial Duration Series and Bivariate Exponential Distribution, and Application to Climate Change. Atmosphere 2020, 11, 476. https://doi.org/10.3390/atmos11050476
Won J, Choi J, Lee O, Park MJ, Kim S. Two Ways to Quantify Korean Drought Frequency: Partial Duration Series and Bivariate Exponential Distribution, and Application to Climate Change. Atmosphere. 2020; 11(5):476. https://doi.org/10.3390/atmos11050476
Chicago/Turabian StyleWon, Jeongeun, Jeonghyeon Choi, Okjeong Lee, Moo Jong Park, and Sangdan Kim. 2020. "Two Ways to Quantify Korean Drought Frequency: Partial Duration Series and Bivariate Exponential Distribution, and Application to Climate Change" Atmosphere 11, no. 5: 476. https://doi.org/10.3390/atmos11050476
APA StyleWon, J., Choi, J., Lee, O., Park, M. J., & Kim, S. (2020). Two Ways to Quantify Korean Drought Frequency: Partial Duration Series and Bivariate Exponential Distribution, and Application to Climate Change. Atmosphere, 11(5), 476. https://doi.org/10.3390/atmos11050476







