Understanding the Variability of West African Summer Monsoon Rainfall: Contrasting Tropospheric Features and Monsoon Index
Abstract
1. Introduction
2. Data and Methodology
2.1. Data
2.2. Methodology
3. Results and Discussion
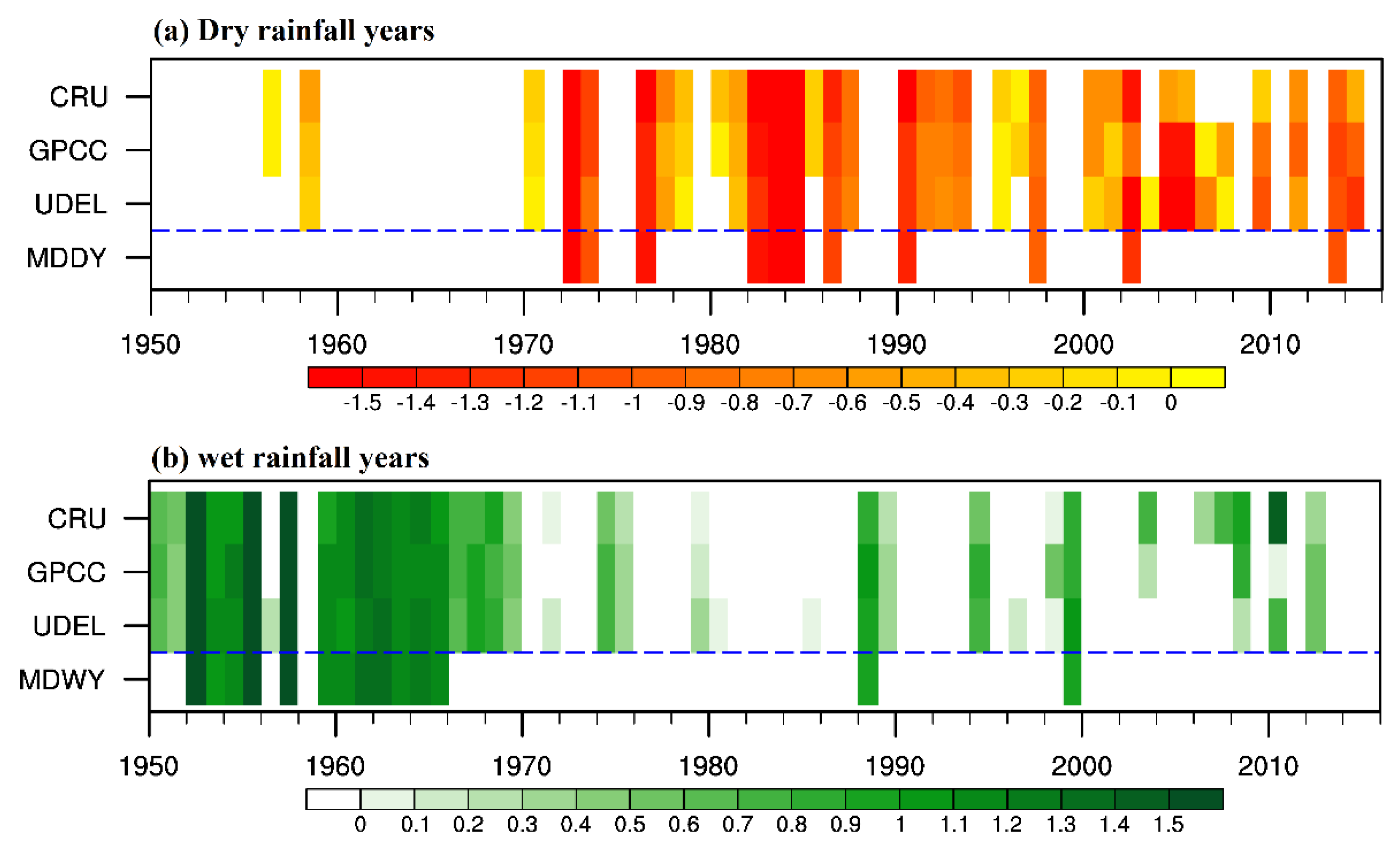
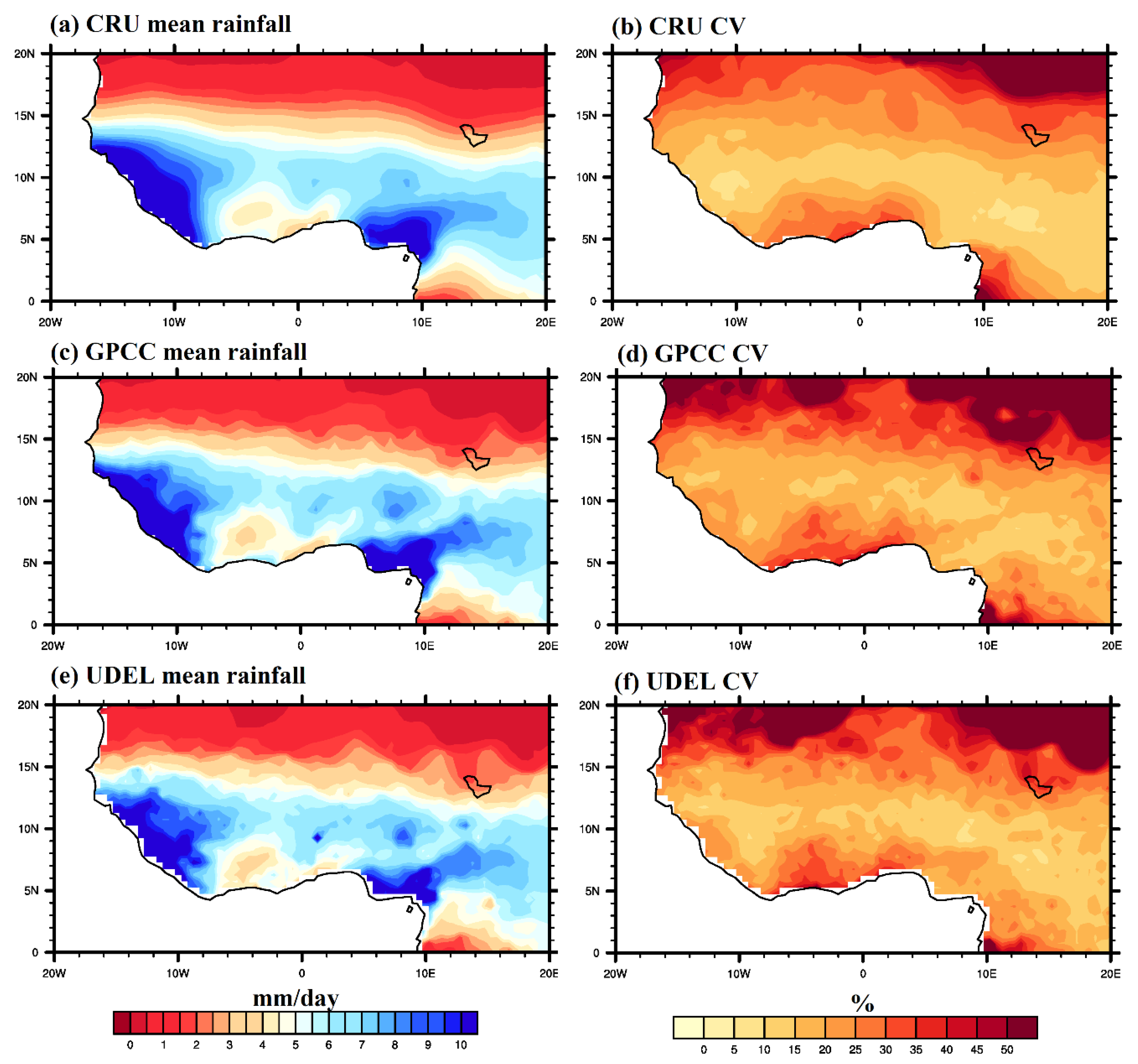
3.1. Spatial and Temporal Distributions of Summer Rainfall over West Africa
3.2. Mean Climatology of Large-Scale Tropospheric Features Associated with the WASM
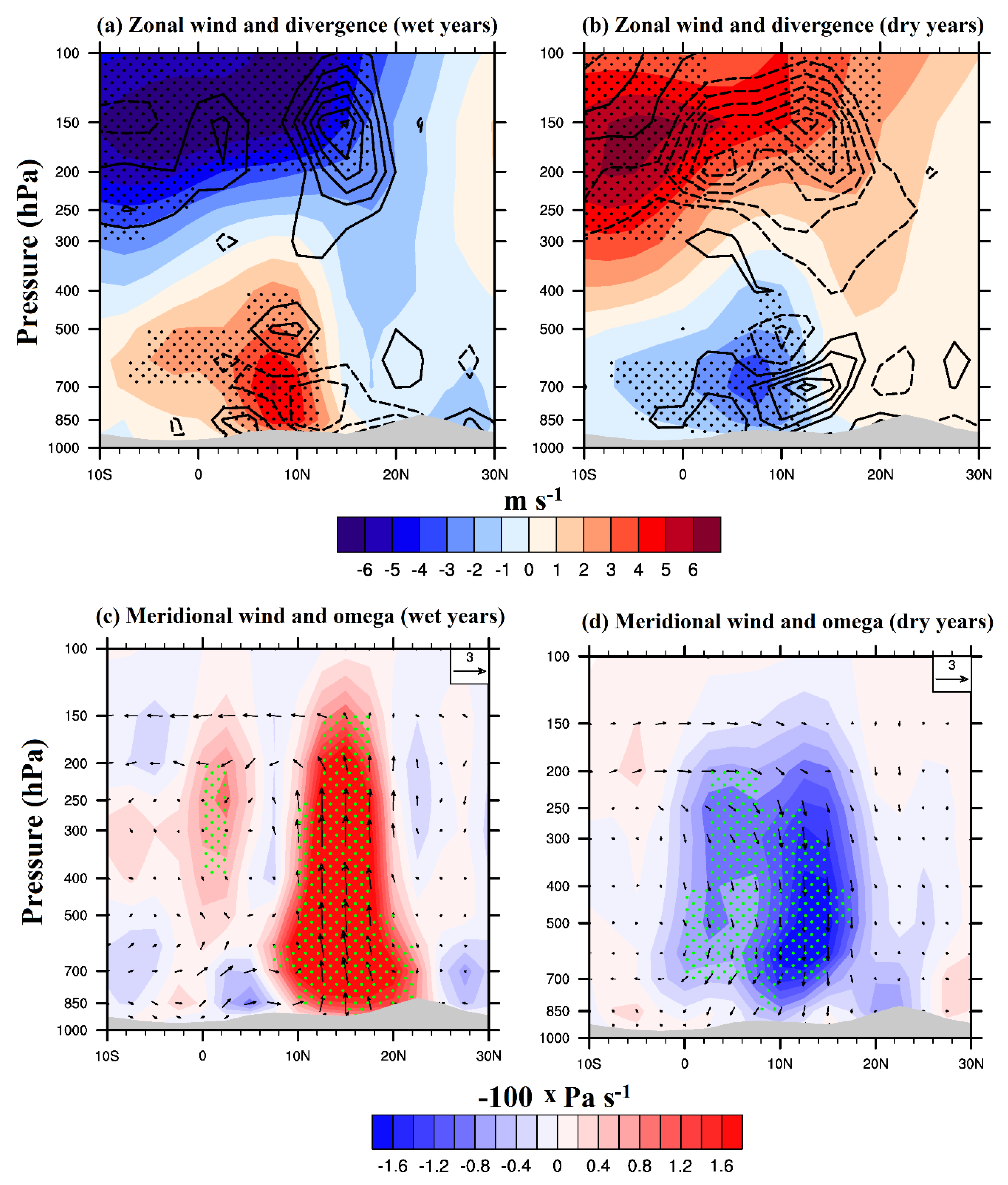
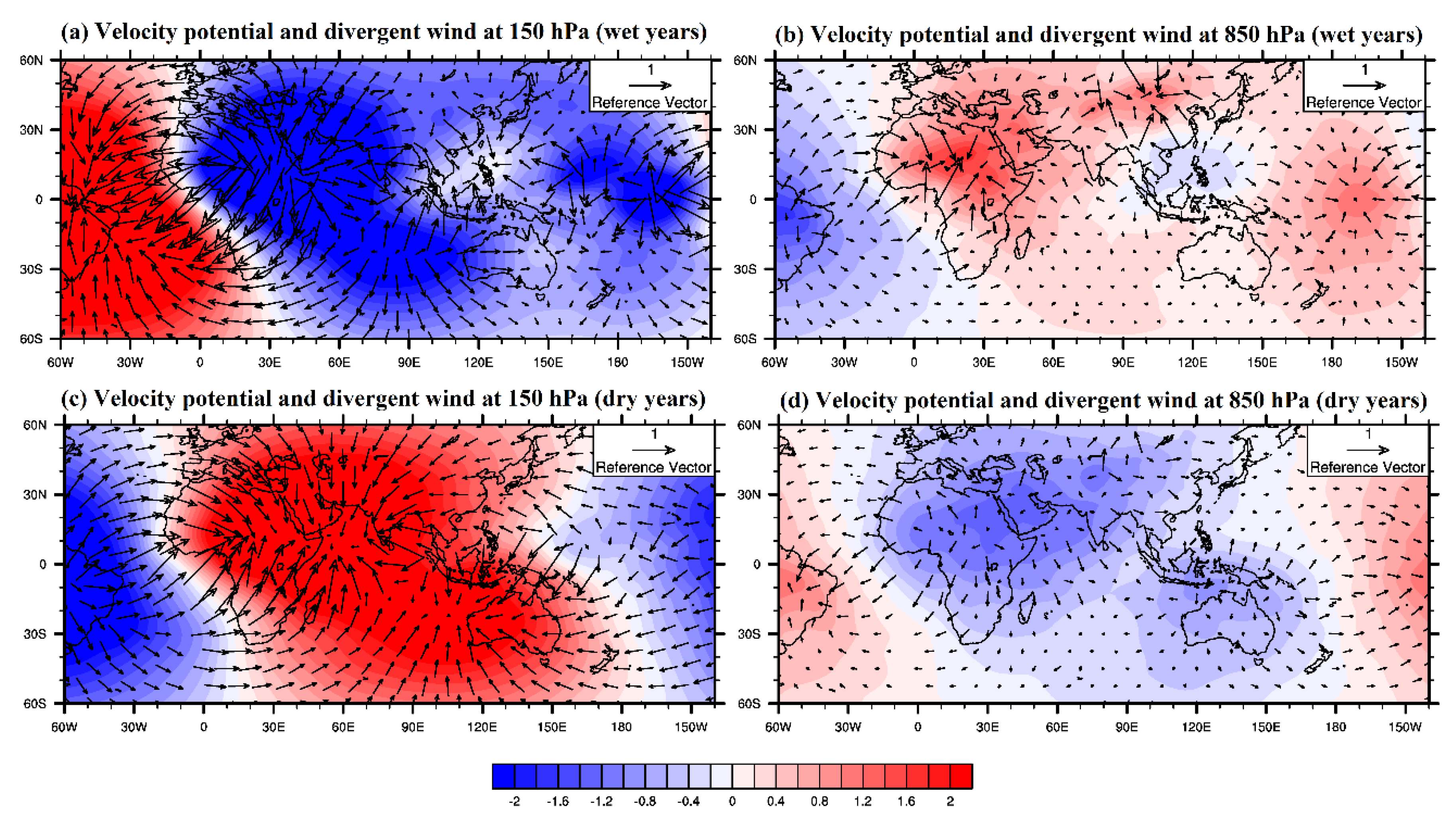
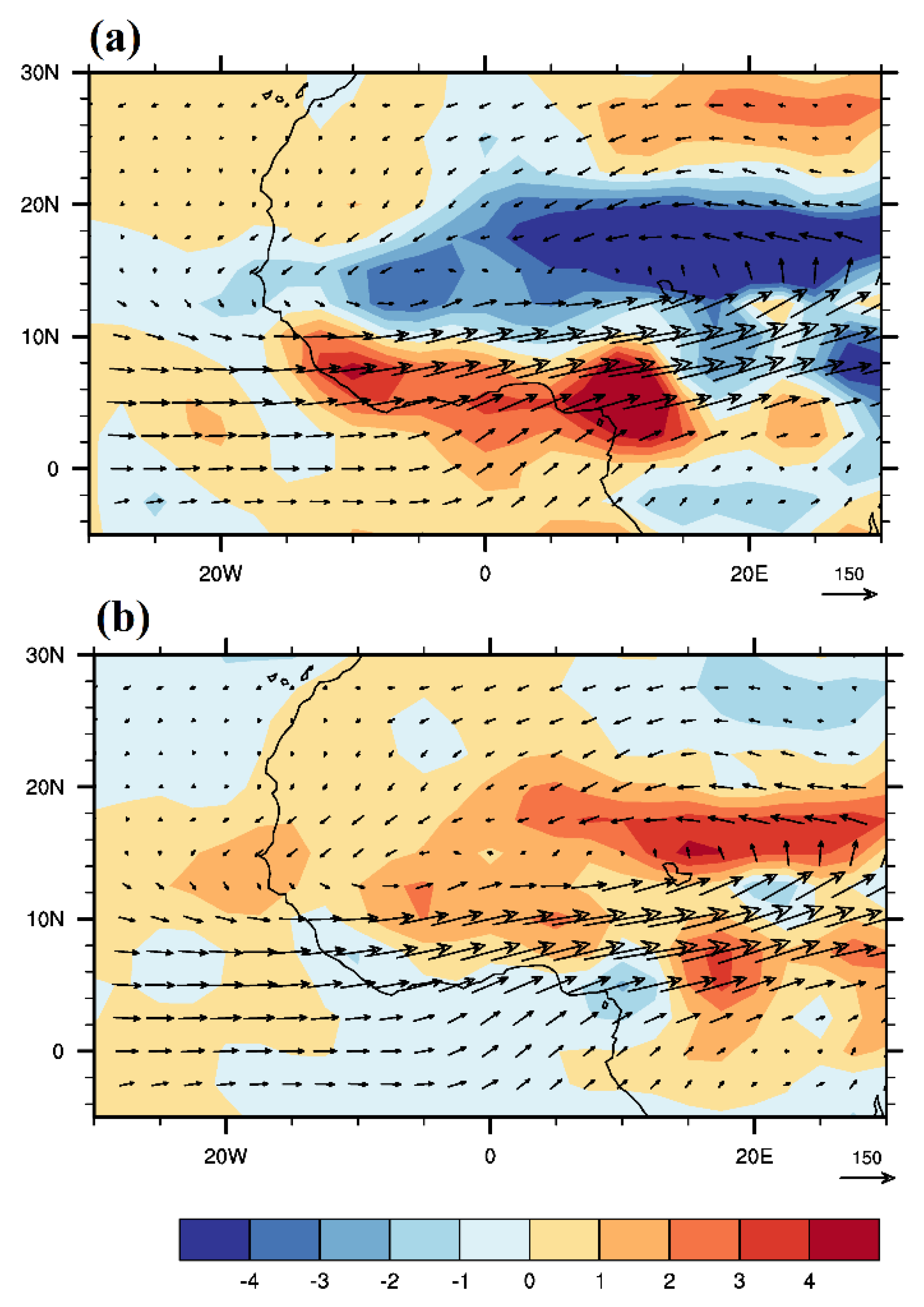
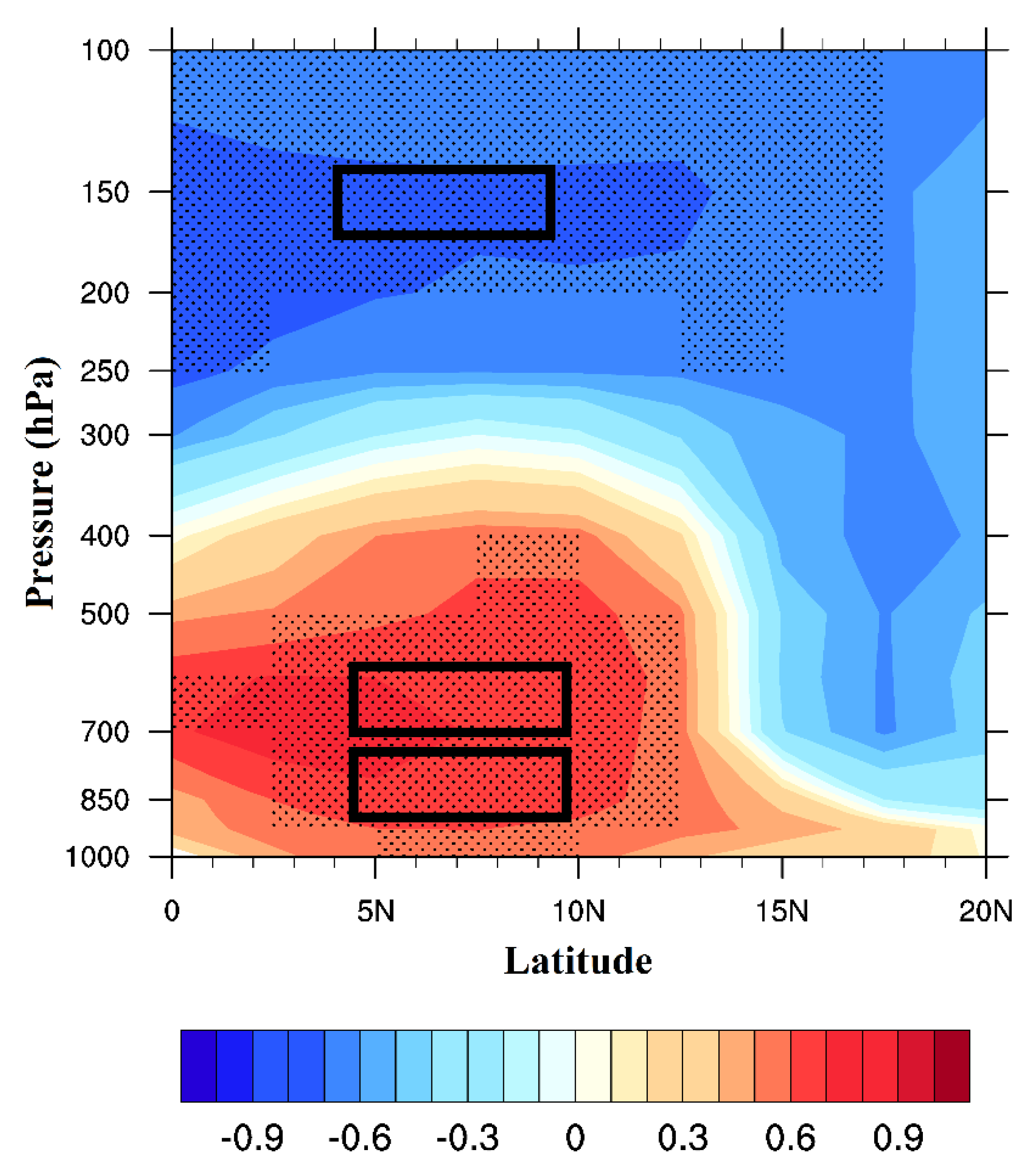
3.3. Composite Analysis of Contrasting Wet and Dry Years
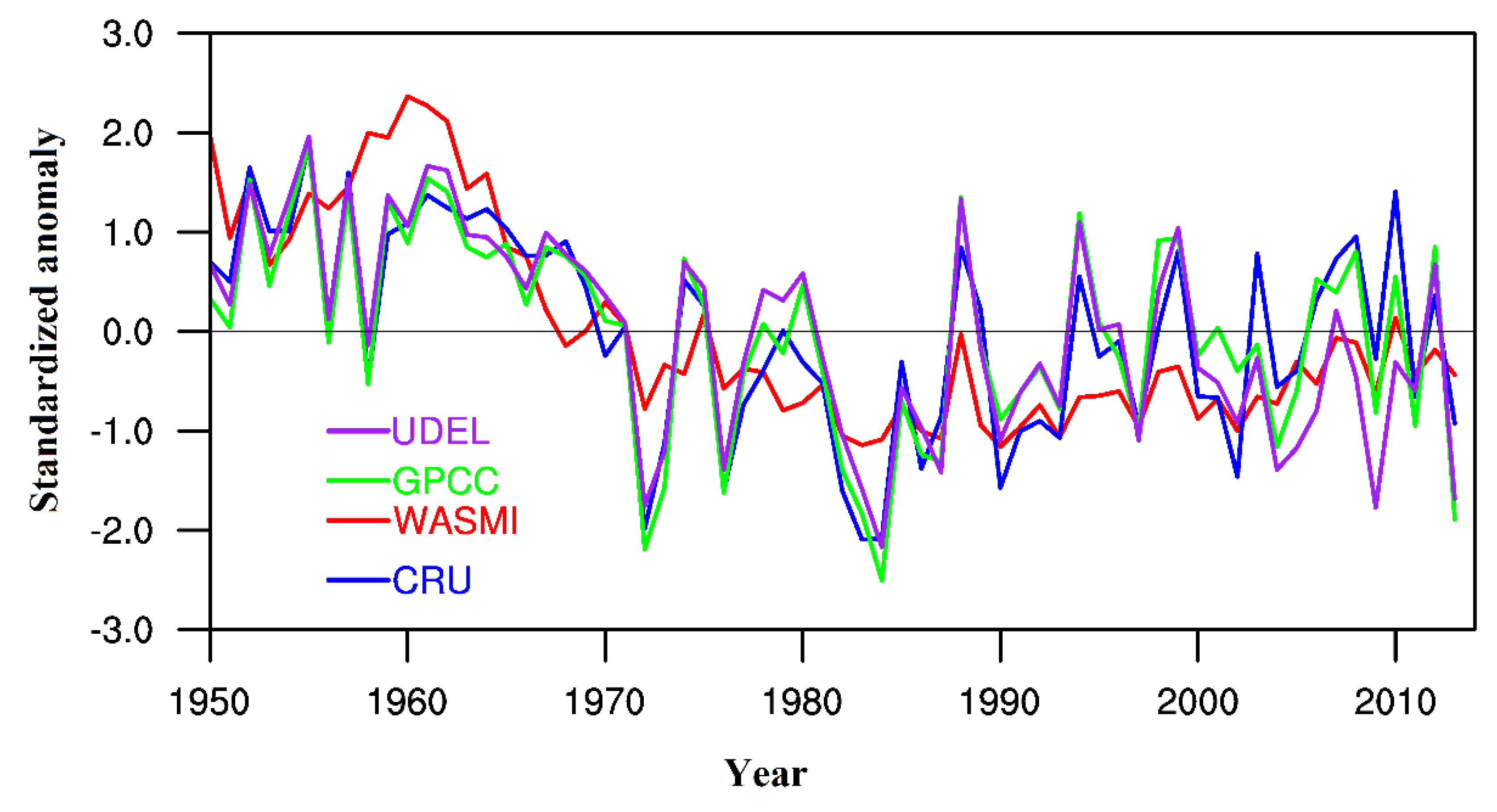
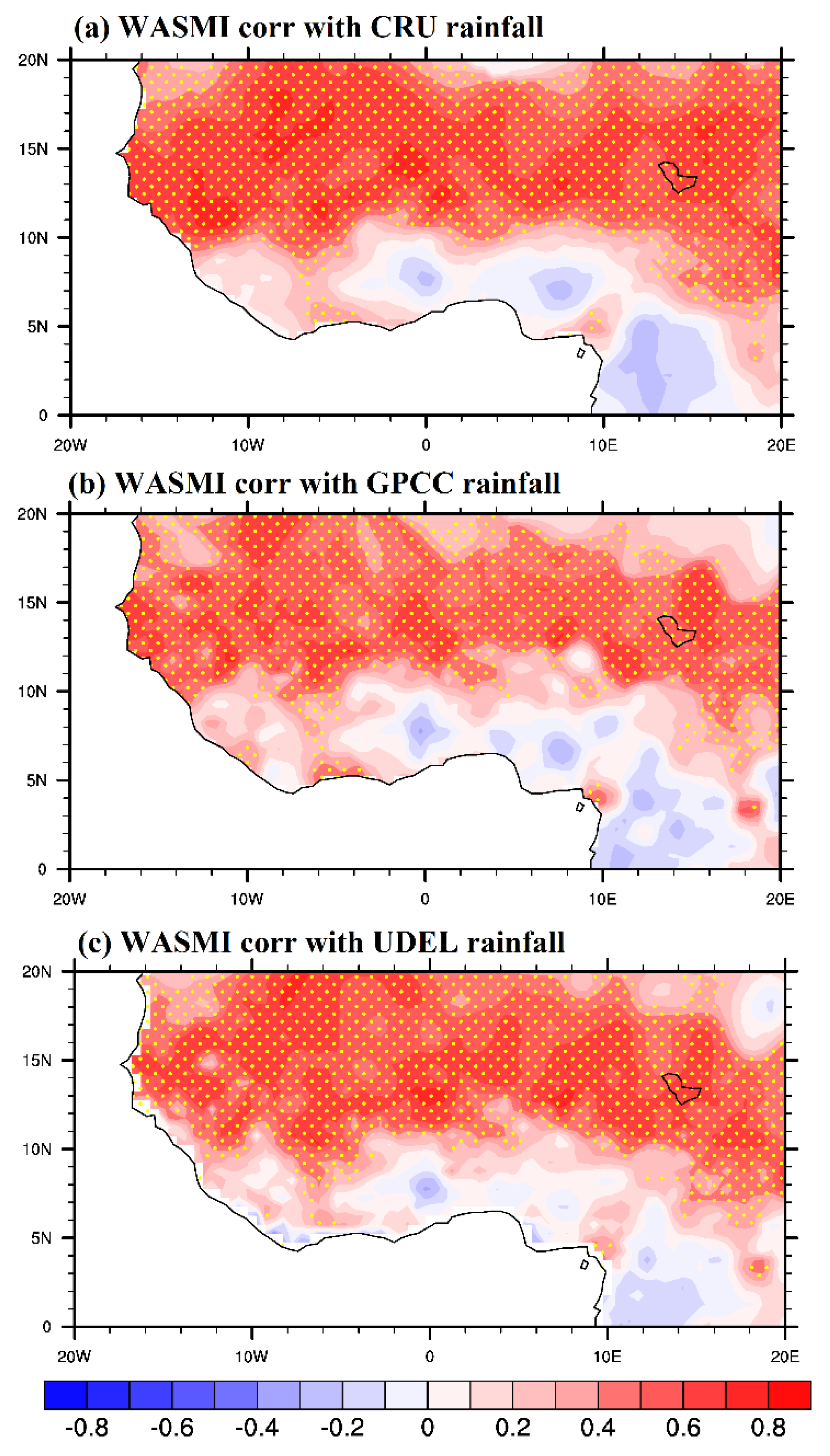
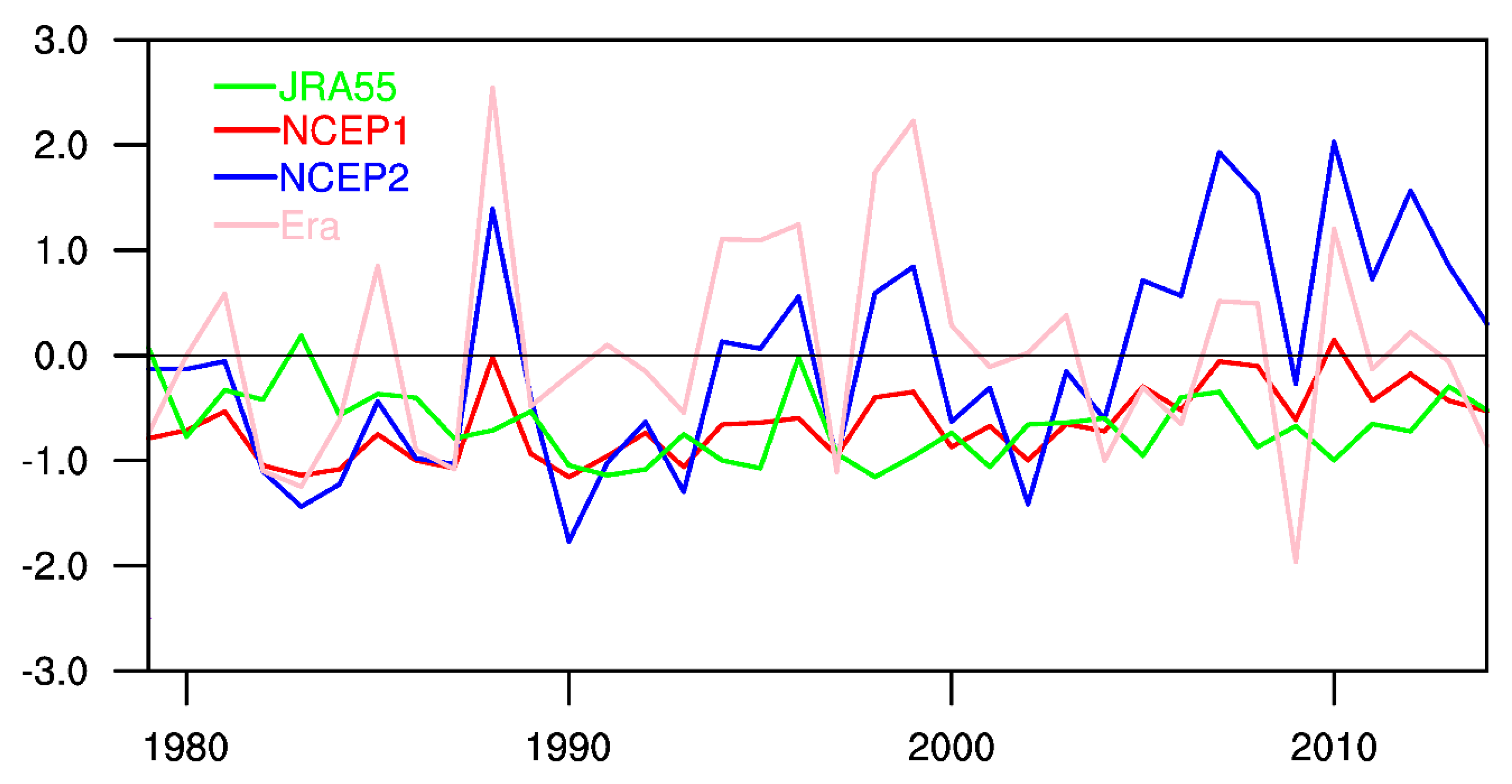
3.4. The West African Summer Monsoon Index (WASMI)
4. Summary and Conclusions
Supplementary Materials
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Akinsanola, A.A.; Zhou, W. Projection of West African summer monsoon rainfall in dynamically downscaled CMIP5 models. Clim. Dyn. 2019, 53, 81–95. [Google Scholar] [CrossRef]
- Akinsanola, A.A.; Zhou, W. Dynamic and thermodynamic factors controlling increasing summer monsoon rainfall over the West African Sahel. Clim. Dyn. 2019, 52, 4501–4514. [Google Scholar] [CrossRef]
- Sylla, M.B.; Diallo, I.; Pal, J.S. West African monsoon in state-of the-art regional climate models. In Climate Variability—Regional and Thematic Patterns; Tarhule, A., Ed.; Books on Demand: Norderstedt, Germany, 2013; ISBN 980-953-307-816-3. [Google Scholar]
- Diedhiou, A.; Janicot, S.; Viltard, A.; de Felice, P. Evidence of two regimes of easterly waves over West Africa and the tropical Africa. Geophys. Res. Lett. 1998, 25, 2805–2808. [Google Scholar] [CrossRef]
- Nicholson, S.E. A revised picture of the structure of the “monsoon” and land ITCZ over West Africa. Clim. Dyn. 2009, 32, 1155–1171. [Google Scholar] [CrossRef]
- Janicot, S. Impact of warm ENSO events on atmospheric circulation and convection over the tropical Atlantic and West Africa. Ann. Geophys. 1997, 15, 471–475. [Google Scholar] [CrossRef]
- Akinsanola, A.A.; Ogunjobi, K.O. Evaluation of present-day rainfall simulations over West Africa in CORDEX regional climate models. Environ. Earth Sci. 2017, 76, 366. [Google Scholar] [CrossRef]
- Redelsperger, J.; Diongue, A.; Diedhiou, A.; Ceron, J.; Diop, M.; Gueremy, J.; Lafore, J. Multi-scale description of a Sahelian synoptic weather system representative of the West African monsoon. Q. J. R. Meteorol. Soc. 2002, 128, 1229–1257. [Google Scholar] [CrossRef]
- Mohr, K.I.; Thorncroft, C.D. Intense convective systems in West Africa and their relationship to the African easterly jet. Q. J. R. Meteorol. Soc. 2006, 132, 163–176. [Google Scholar] [CrossRef]
- Nicholson, S.E. On the factors modulating the intensity of the tropical rainbelt over West Africa. Int. J. Climatol. 2009, 29, 673–689. [Google Scholar] [CrossRef]
- Omotosho, J.B.; Abiodun, B.J. A numerical study of moisture build-up and rainfall over West Africa. Meteorol. Appl. 2007, 14, 209–225. [Google Scholar] [CrossRef]
- Akinsanola, A.A.; Ogunjobi, K.O.; Ajayi, V.O.; Adefisan, E.A.; Omotosho, J.A.; Sanogo, S. Comparison of five gridded precipitation products at climatological scales over West Africa. Meteorol. Atmos. Phys. 2017, 129, 669–689. [Google Scholar] [CrossRef]
- Nicholson, S. Land surface processes and Sahel climate. Rev. Geophys. 2000, 38, 117–139. [Google Scholar] [CrossRef]
- Lebel, T.; Diedhiou, A.; Laurent, H. Seasonal cycle and inter-annual variability of the Sahelian rainfall at hydrological scales. J. Geophys. Res. 2003, 108. [Google Scholar] [CrossRef]
- Ward, N.M. Diagnosis and short-lead time prediction of summer rainfall in tropical North Africa at interannual and multi-decadal timescales. J. Clim. 1998, 11, 3167–3191. [Google Scholar] [CrossRef]
- Trenberth, K.E.; Smith, L.; Qian, T.; Dai, A.; Fasullo, J. Estimates of the global water budget and its annual cycle using observational and model data. J. Hydrometeorol. 2007, 8, 758–769. [Google Scholar] [CrossRef]
- Charney, J.G. Dynamics of deserts and drought in the Sahel. Q. J. R. Meteorol. Soc. 1975, 101, 193–202. [Google Scholar] [CrossRef]
- Folland, C.K.; Palmer, T.N.; Parker, D.E. Sahel rainfall and worldwide sea temperatures, 1901−85. Nature 1986, 320, 602–607. [Google Scholar] [CrossRef]
- Janicot, S.; Trzaska, S.; Poccard, I. Summer SAHEL-ENSO teleconnection and decadal time scale SST variations. Clim. Dyn. 2001, 18, 303–320. [Google Scholar] [CrossRef]
- Lu, J.; Delworth, T. Oceanic forcing of the late 20th century Sahel drought. Geophys. Res. Lett. 2005, 32. [Google Scholar] [CrossRef]
- Sahu, N.; Behera, S.K.; Ratnam, J.V.; Da Silva, R.V.; Parhi, P.; Duan, W.; Yamagata, T.; Takara, K.; Singh, R.B. El Nino Modoki connection to extremely-low streamflow of the Paranaiba River in Brazil. Clim. Dyn. 2014, 42, 1509–1516. [Google Scholar] [CrossRef]
- Xue, Y.; Shukla, J. The influence of land-surface properties on Sahel climate. Part I: Desertification. J. Clim. 1993, 6, 2232–2245. [Google Scholar] [CrossRef]
- Wang, G.; Eltahir, E.A.B. Ecosystem dynamics and the Sahel drought. Geophys. Res. Lett. 2000, 27, 795–798. [Google Scholar] [CrossRef]
- Clark, D.B.; Xue, Y.K.; Harding, R.J.; Valdes, P.J. Modeling the impact of land surface degradation on the climate of tropical North Africa. J. Clim. 2001, 14, 1809–1822. [Google Scholar] [CrossRef]
- Nicholson, S.E.; Grist, J.P. A conceptual model for understanding rainfall variability in the West African Sahel on interannual and interdecadal timescales. Int. J. Clim. 2001, 21, 1733–1757. [Google Scholar] [CrossRef]
- Jenkins, G.S.; Gaye, A.T.; Sylla, M.B. Late 20th century attribution of drying trends in the Sahel from the Regional Climate Model (RegCM3). Geophys. Res. Lett. 2005, 32. [Google Scholar] [CrossRef]
- Sanogo, S.; Fink, A.H.; Omotosho, J.A.; Ba, A.; Redl, R.; Ermert, V. Spatio-temporal characteristics of the recent rainfall recovery in West Africa. Int. J. Climatol. 2015, 35, 4589–4605. [Google Scholar] [CrossRef]
- Newell, R.E.; Kidson, J.W. African mean wind changes between Sahelian wet and dry periods. J. Climatol. 1984, 4, 27–33. [Google Scholar] [CrossRef]
- Fontaine, B.; Janicot, S.; Moron, S.V. Rainfall anomaly patterns and wind field signals over West Africa in August (1958–1989). J. Clim. 1995, 8, 1503–1510. [Google Scholar] [CrossRef]
- Grist, J.P.; Nicholson, S.E. A study of the dynamic factors influencing the rainfall variability in the West African Sahel. J. Clim. 2001, 14, 1337–1359. [Google Scholar] [CrossRef]
- Wang, B.; Fan, Z. Choice of South Asian summer monsoon indices. Bull. Am. Meteorol. Soc. 1999, 80, 629–638. [Google Scholar] [CrossRef]
- Dai, A.; Lamb, P.J.; Trenberth, K.E.; Hulme, M.; Jones, P.D.; Xie, P. The recent Sahel drought is real. Int. J. Climatol. 2004, 24, 1323–1331. [Google Scholar] [CrossRef]
- Nicholson, S.E.; Dezfuli, A.K.; Klotter, D. A two-century precipitation dataset for the continent of Africa. Bull. Am. Meteorol. Soc. 2012, 93, 1219–1231. [Google Scholar] [CrossRef]
- Li, J.P.; Zeng, Q.C. A unified monsoon index. Geophys. Res. Lett. 2002, 29. [Google Scholar] [CrossRef]
- Gallego, D.; Ordóñez, P.; Ribera, P.; Peña-Ortiz, C.; García-Herrera, R. An instrumental index of the West African Monsoon back to the nineteenth century. Q. J. R. Meteorol. Soc. 2015, 141, 3166–3176. [Google Scholar] [CrossRef]
- Harris, I.; Jones, P.D.; Osborn, T.J.; Lister, D.H. Updated high-resolution grids of monthly climatic observations—The CRU TS3.10 Dataset. Int. J. Climatol. 2014, 34, 623–642. [Google Scholar] [CrossRef]
- Schneider, U.; Ziese, M.; Meyer-Christoffer, A.; Finger, P.; Rustemeier, E.; Becker, A. The new portfolio of global precipitation data products of the Global Precipitation Climatology Centre suitable to assess and quantify the global water cycle and resources. Proc. Int. Assoc. Hydrol. Sci. 2017, 374, 29–34. [Google Scholar] [CrossRef]
- Kalnay, E.; Kanamitsu, M.; Kistler, R.; Collins, W.; Deaven, D.; Gandin, L.; Iredell, M.; Saha, S.; Zhu, Y.; Zhu, Y.; et al. The NCEP/NCAR 40-Year Reanalysis Project. Bull. Am. Meteorol. Soc. 1996, 77, 437–471. [Google Scholar] [CrossRef]
- Kanamitsu, M.; Ebisuzaki, W.; Woollen, J.; Yang, S.-K.; Hnilo, J.J.; Fiorino, M.; Potter, G.L. NCEP–DOE AMIP-II Reanalysis (R-2). Bull. Am. Meteorol. Soc. 2002, 83, 1631–1643. [Google Scholar] [CrossRef]
- Dee, D.P.; Uppala, S.M.; Simmons, A.J.; Berrisford, P.; Poli, P.; Kobayashi, S.; Bechtold, P.; Andrae, U.; Bauer, P.; Healy, S.B.; et al. The ERA-Interim reanalysis: Configuration and performance of the data assimilation system. Q. J. R. Meteorol. Soc. 2011, 137, 553–597. [Google Scholar] [CrossRef]
- Kobayashi, S.; Ota, Y.; Harada, Y.; Ebita, A.; Moriya, M.; Onoda, H.; Onogi, K.; Kamahori, H.; Endo, H.; Miyaoka, K.; et al. The JRA-55 reanalysis: General specifications and basic characteristics. J. Meteorol. Soc. Jpn. 2015, 93, 5–48. [Google Scholar] [CrossRef]
- Cook, K.H. Generation of the African Easterly Jet and its role in determining West African precipitation. J. Clim. 1999, 12, 1165–1184. [Google Scholar] [CrossRef]
- Thorncroft, C.D.; Blackburn, M. Maintenance of the African Easterly Jet. Q. J. R. Meteorol. Soc. 1999, 125, 763–786. [Google Scholar] [CrossRef]
- Sylla, M.B.; Gaye, A.T.; Jenkins, G.S.; Pal, J.S.; Giorgi, F. Consistency of projected drought over the Sahel with changes in the monsoon circulation and extremes in RegCM3 projections. J. Geophys. Res. 2010, 115. [Google Scholar] [CrossRef]
- Akinsanola, A.A.; Ogunjobi, K.O.; Gbode, I.E.; Ajayi, V.O. Assessing the capabilities of three regional climate models over CORDEX Africa in simulating West African summer monsoon precipitation. Adv. Meteorol. 2015. [Google Scholar] [CrossRef]
- Hastenrath, S. Climate Dynamics of the Tropics; Kluwer Academic Publisher: Norwell, MA, USA, 1990. [Google Scholar]
- Nicholson, S.E.; Grist, J.P. The seasonal evolution of the atmospheric circulation over West Africa and equatorial Africa. J. Clim. 2003, 16, 1013–1030. [Google Scholar] [CrossRef]
- Cornforth, R.J.; Hoskins, B.J.; Thorncroft, C.D. The impact of moist processes on the African easterly jet–African easterly wave system. Q. J. R. Meteorol. Soc. 2009, 135, 894–913. [Google Scholar] [CrossRef]

| WASMI | CRU | GPCC | UDEL | |
|---|---|---|---|---|
| West African Summer Monsoon Index, WASMI | 1.0 | 0.76 | 0.78 | 0.80 |
| (Gallego et al. 2015 [35]; African South Westerly Index, ASWI) | 0.79 | 0.59 | 0.60 | 0.55 |
| (Fontaine et al. 1995 [29]; Fontaine) | 0.97 | 0.70 | 0.71 | 0.71 |
| (Li and Zeng 2002 [34]; Li_Zeng) | 0.94 | 0.63 | 0.65 | 0.64 |
| WASMI (NCEP2) | WASMI (Era–Interim) | WASMI (JRA55) | |
|---|---|---|---|
| WASMI (NCEP 1) | 0.97 | 0.60 | 0.70 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Akinsanola, A.A.; Zhou, W. Understanding the Variability of West African Summer Monsoon Rainfall: Contrasting Tropospheric Features and Monsoon Index. Atmosphere 2020, 11, 309. https://doi.org/10.3390/atmos11030309
Akinsanola AA, Zhou W. Understanding the Variability of West African Summer Monsoon Rainfall: Contrasting Tropospheric Features and Monsoon Index. Atmosphere. 2020; 11(3):309. https://doi.org/10.3390/atmos11030309
Chicago/Turabian StyleAkinsanola, Akintomide Afolayan, and Wen Zhou. 2020. "Understanding the Variability of West African Summer Monsoon Rainfall: Contrasting Tropospheric Features and Monsoon Index" Atmosphere 11, no. 3: 309. https://doi.org/10.3390/atmos11030309
APA StyleAkinsanola, A. A., & Zhou, W. (2020). Understanding the Variability of West African Summer Monsoon Rainfall: Contrasting Tropospheric Features and Monsoon Index. Atmosphere, 11(3), 309. https://doi.org/10.3390/atmos11030309






