First Spaceborne Version of Velocity-Azimuth Display Technique for Wind Field Retrieval on Cloud and Precipitation Radar
Abstract
1. Introduction
- (1)
- The VAD method requires the radar to perform a conical scan. Compared with the stationary ground-based weather radar, the spaceborne radar has a higher flight speed, and the beam footprint of the antenna conical scanning is spiral rather than circular. This will change the first step of the Taylor series expansion of the wind field, thus affecting the determination of VAD coefficients at all levels.
- (2)
- Compared with airborne radar, the movement speed of spaceborne radar is much higher. Since the height of the satellite platform is much larger than that of the airborne platform, for the same downward viewing angle, the swath width is much larger, while it is difficult for the horizontal resolution to reach the level of the airborne radar. This poses a challenge to the application of the VAD method in the wind field retrieval of spaceborne Doppler cloud and rain radar.
2. Methodology
2.1. Observation Geometry and Coordinate System
2.2. Derivation of Spaceborne Version of VAD Technique
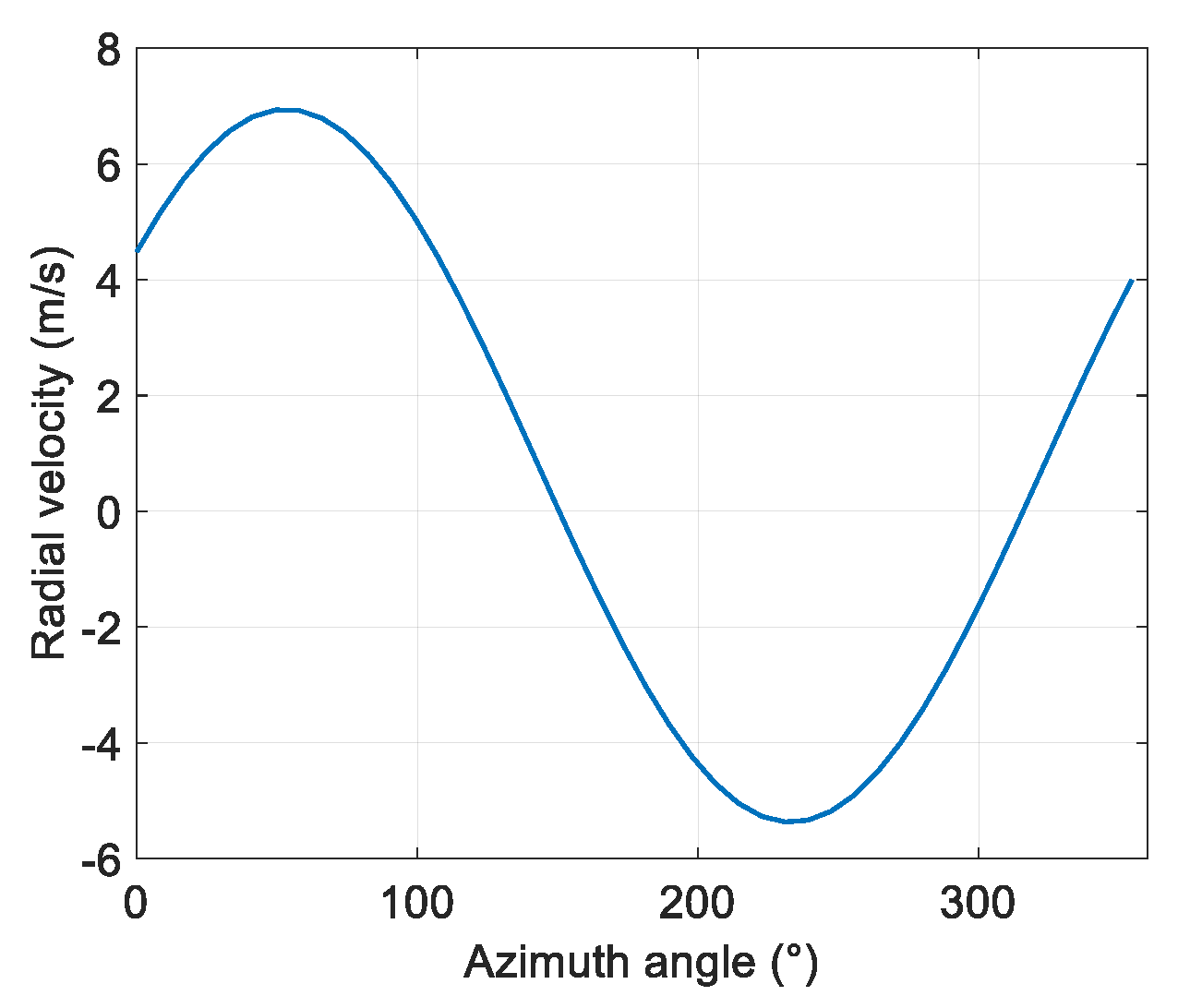
3. Numerical Simulations
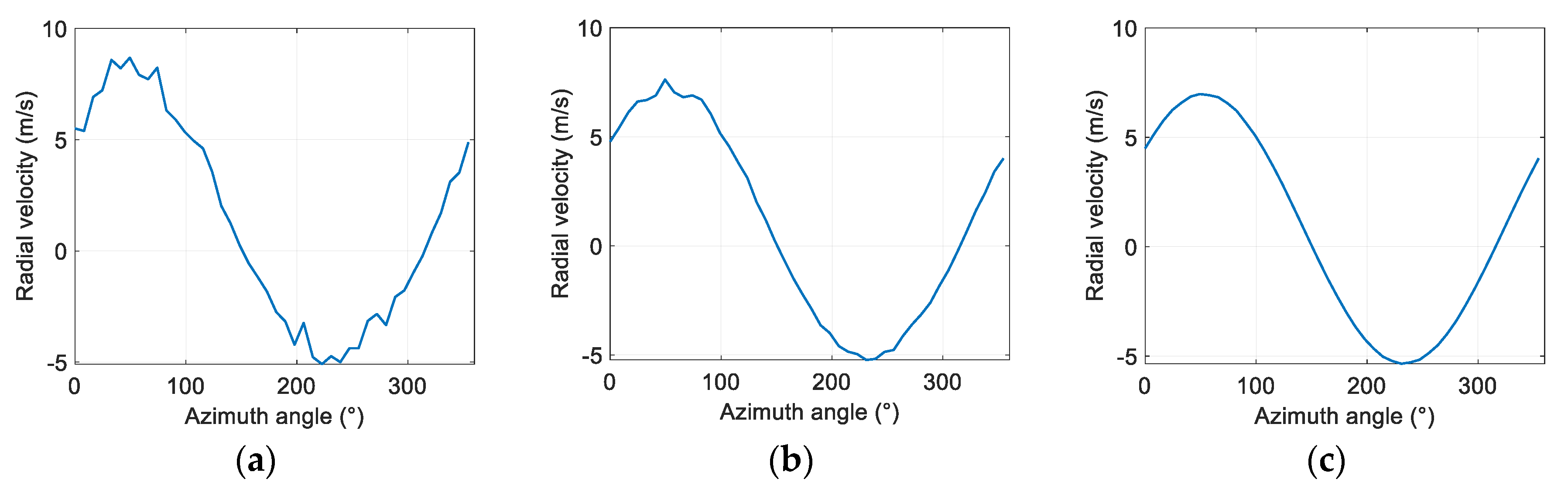
4. Validation on Airborne Radar with the Same Scanning Strategy as Spaceborne Radar
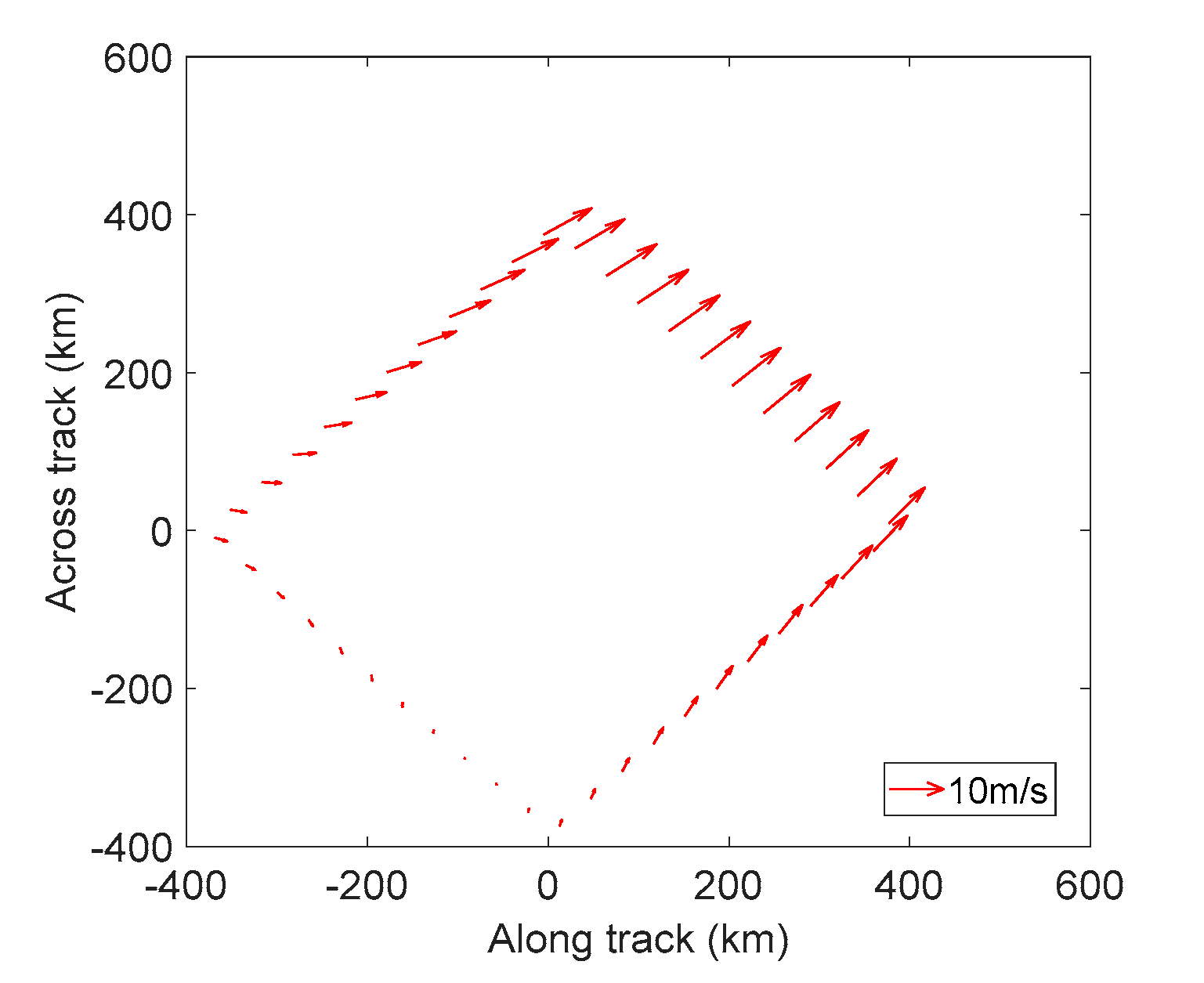
4.1. HIWRAP and Data Description
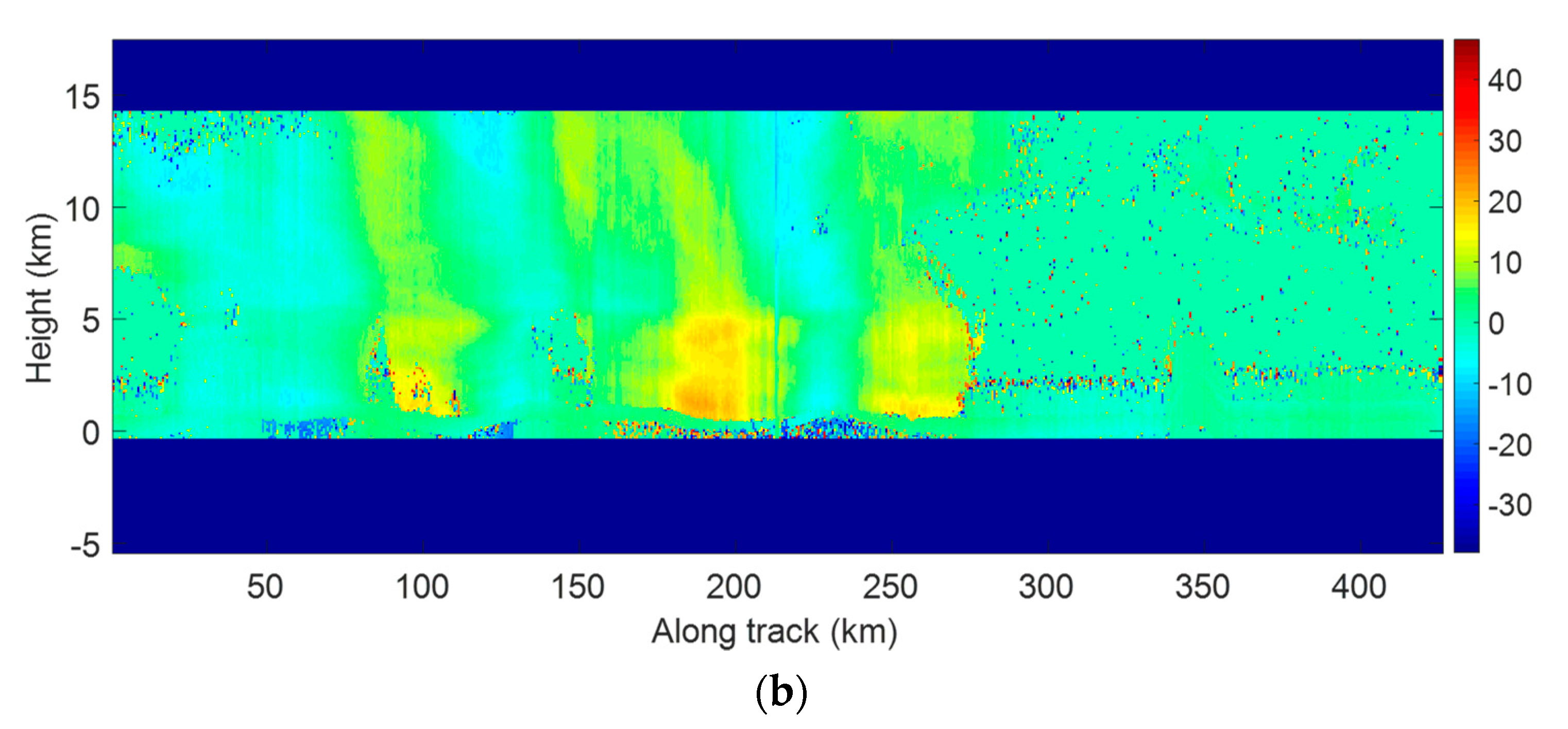
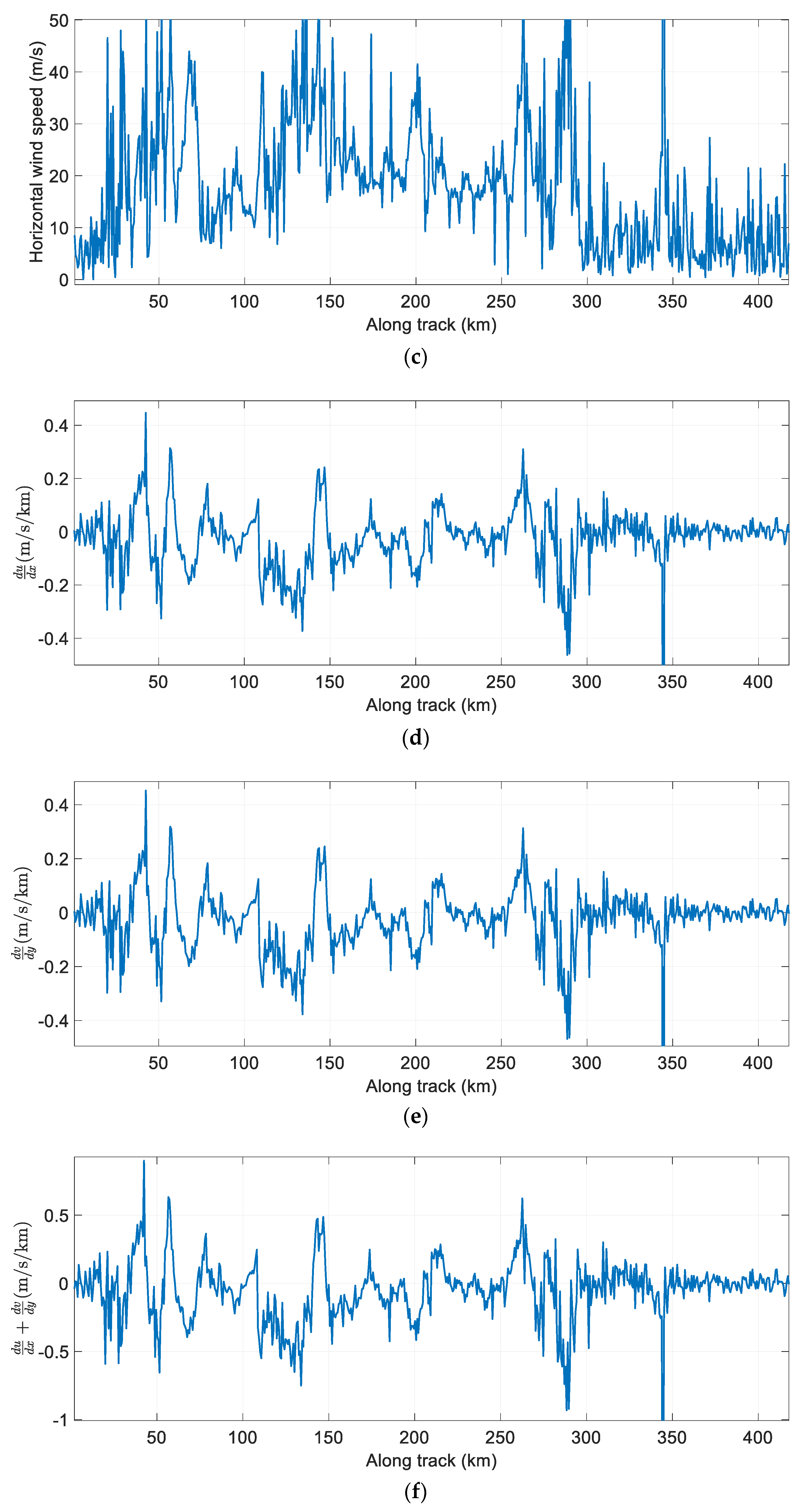
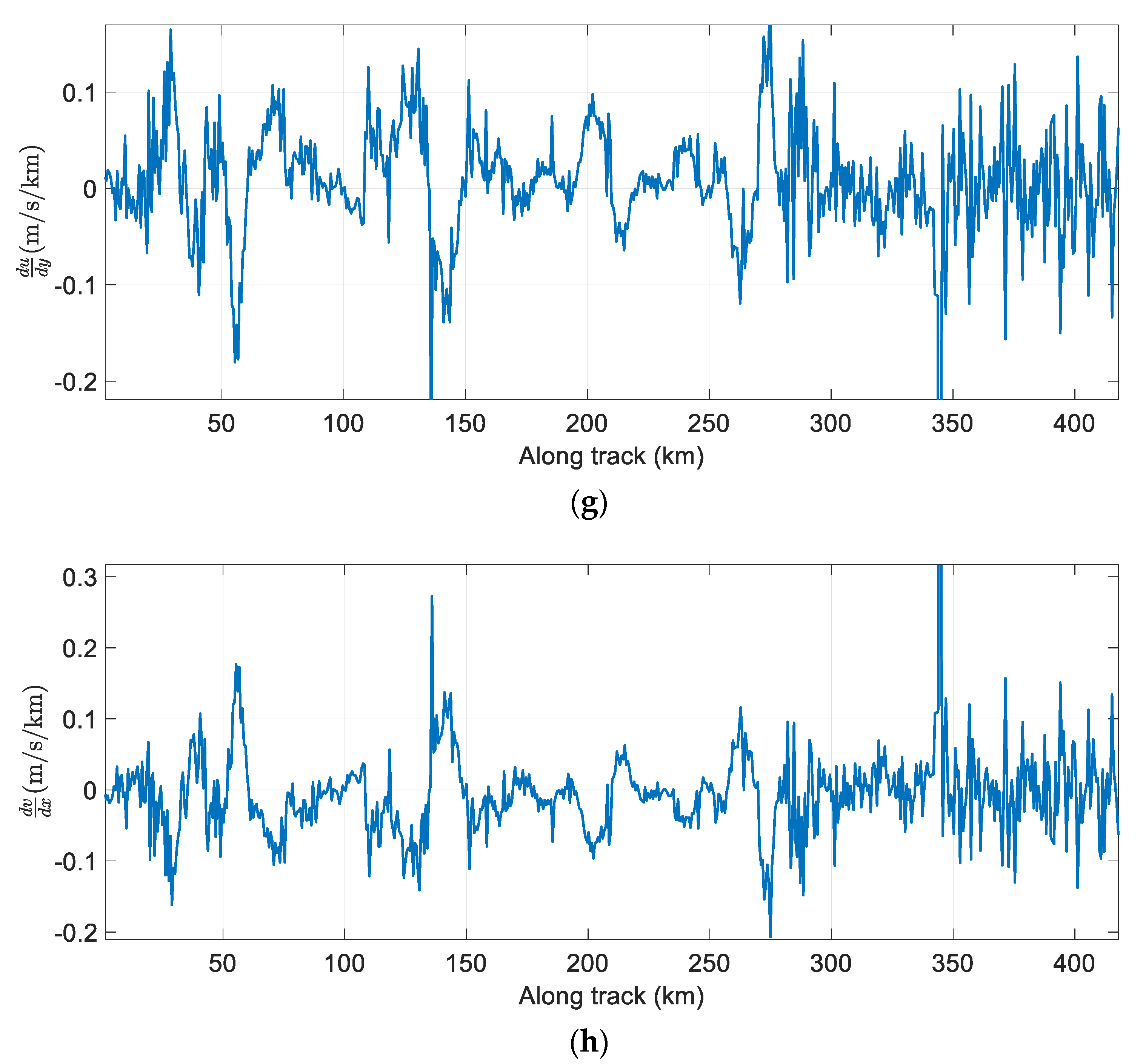
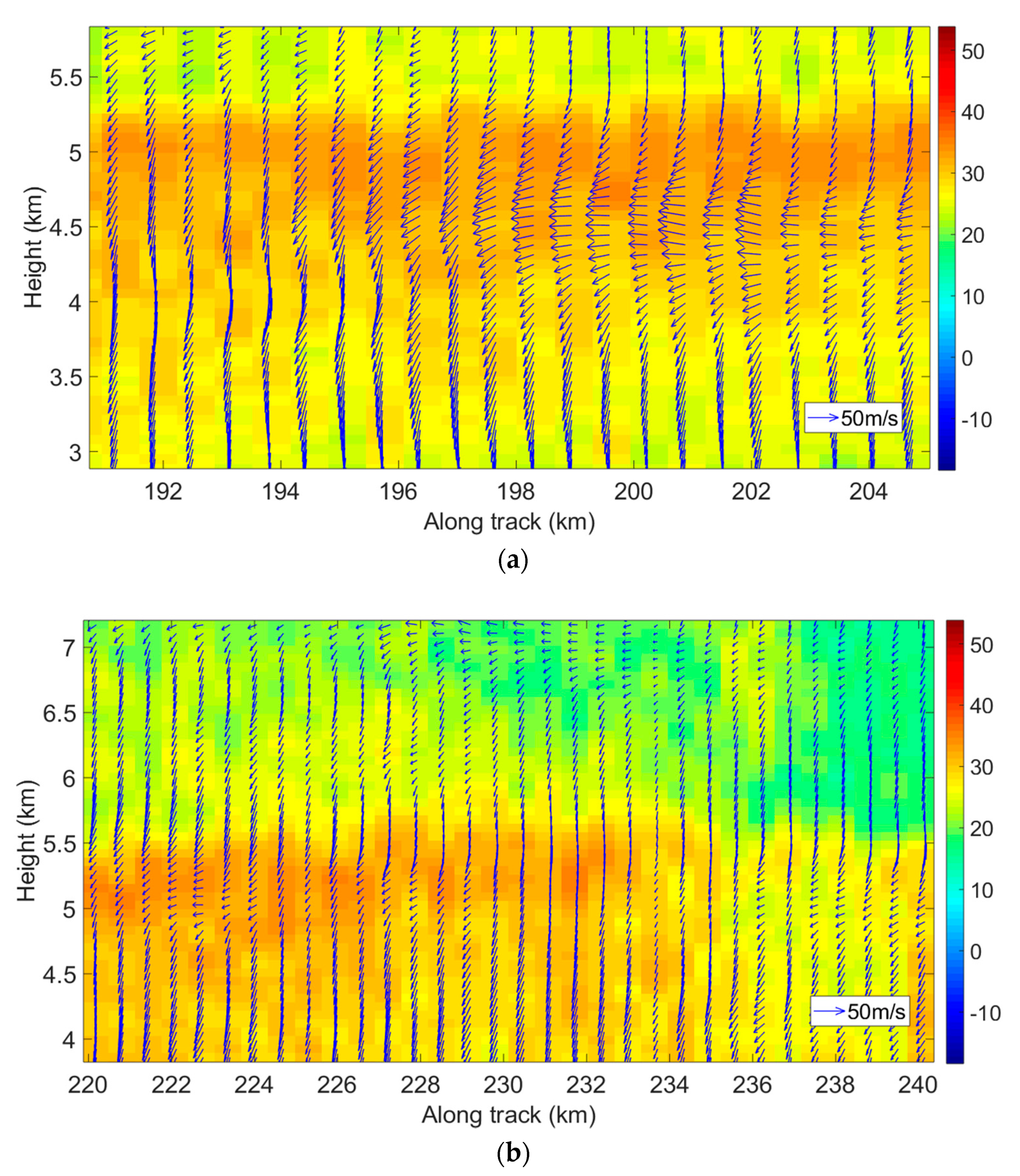
4.2. Retrieval Results and Validation
5. Discussion
6. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Probert-Jones, J.R. Meteorological use of pulsed Doppler radar. Nature 1960, 186, 271–273. [Google Scholar] [CrossRef]
- Baker, W.E.; Atlas, R.; Cardinali, C.; Clement, A.; Emmitt, G.D.; Gentry, B.M.; Hardesty, R.M.; Källén, E.; Kavaya, M.J.; Langland, R.; et al. Lidar-measured wind profiles: The missing link in the global observing system. Bull. Am. Meteorol. Soc. 2014, 95, 543–564. [Google Scholar] [CrossRef]
- Duan, B.; Zhang, W.; Yang, X.; Dai, H.; Yu, Y. Assimilation of Typhoon Wind Field Retrieved from Scatterometer and SAR Based on the Huber Norm Quality Control. Remote Sens. 2017, 9, 987. [Google Scholar] [CrossRef]
- Zhang, W. The vision of space-based component of WMO Integrated Global Observing Systems (WIGOS) in 2040—Anticipating requirements and new space technologies. In Proceedings of the 2016 EUMETSAT Meteorological Satellite Conference, Darmstadt, Germany, 26–30 September 2016. [Google Scholar]
- Hu, T.; Li, Y.; Li, Y.; Wu, Y.; Zhang, D. Retrieval of Sea Surface Wind Fields Using Multi-Source Remote Sensing Data. Remote Sens. 2020, 12, 1482. [Google Scholar] [CrossRef]
- Liu, H.; Jin, S.; Yan, Q. Evaluation of the Ocean Surface Wind Speed Change following the Super Typhoon from Space-Borne GNSS-Reflectometry. Remote Sens. 2020, 12, 2034. [Google Scholar] [CrossRef]
- WMO. GCOS, Systematic Observation Requirements for Satellite-Based Products for Climate. WMO Repoter GCOS-107. 2006. Available online: https://library.wmo.int/doc_num.php?explnum_id=3813 (accessed on 1 September 2006).
- Illingworth, A.J.; Barker, H.W.; Beljaars, A. The EarthCARE Satellite: The Next Step Forward in Global Measurements of Clouds, Aerosols, Precipitation, and Radiation. Bull. Am. Meteorol. Soc. 2014, 31, 197–210. [Google Scholar] [CrossRef]
- Lemaître, Y.; Viltard, N. 3D wind field retrieval from spaceborne Doppler radar. In Remote Sensing of the Atmosphere, Clouds, and Precipitation IV; International Society for Optics and Photonics: New York, NY, USA, 2012; Volume 8523, p. 85230Q. [Google Scholar]
- Taveneau, N.; Caubet, E.; Richard, J.; Lorenzo, J.; Albouys, V.; Souyris, J.C.; Viltard, N.; Lemaitre, Y. A spaceborne Doppler radar for 3D winds mapping inside clouds. In Proceedings of the 2011 IEEE International Geoscience and Remote Sensing Symposium, Vancouver, BC, Canada, 24–29 July 2011; pp. 2721–2724. [Google Scholar]
- Illingworth, A.J.; Battaglia, A.; Bradford, J.; Forsythe, M.; Joe, P.; Kollias, P.; Lean, K.; Lori, M.; Mahfouf, J.F.; Melo, S.; et al. WIVERN: A new satellite concept to provide global in-cloud winds, precipitation, and cloud properties. Bull. Am. Meteorol. Soc. 2018, 99, 1669–1687. [Google Scholar] [CrossRef]
- Wang, Y.X.; Wei, M.; Wang, Z.H.; Zhang, S.; Liu, L.X. Novel scanning strategy for future spaceborne Doppler weather radar with application to tropical cyclones. IEEE J. Sel. Top. Appl. Earth Obs. Remote Sens. 2017, 10, 2685–2693. [Google Scholar] [CrossRef]
- Teschke, G.; Lehmann, V. Mean wind vector estimation using the velocity–azimuth display (VAD) method: An explicit algebraic solution. Atmos. Meas. Tech. 2017, 10, 3265–3271. [Google Scholar] [CrossRef]
- Lee, W.C.; Tang, X.; Jou, B.J.D. Distance velocity–azimuth display (DVAD)—New interpretation and analysis of Doppler velocity. Mon. Weather Rev. 2014, 142, 573–589. [Google Scholar] [CrossRef][Green Version]
- Klazura, G.E.; Imy, D.A. A Description of the Initial Set of Analysis Products Available from the NEXRAD WSR-88D System. Bull. Am. Meteorol. Soc. 1993, 74, 1293–1312. [Google Scholar] [CrossRef]
- Crum, T.D.; Alberty, R.L. The WSR-88D and the WSR-88D operational support facility. Bull. Am. Meteorol. Soc. 1993, 74, 1669–1688. [Google Scholar] [CrossRef]
- Illingworth, A.J.; Cimini, D.; Gaffard, C. Exploiting Existing Ground-Based Remote Sensing Networks to Improve High-Resolution Weather Forecasts. Bull. Am. Meteorol. Soc. 2015, 96, 2107–2125. [Google Scholar] [CrossRef]
- Ma, Q.Y.; Li, Z.C.; Tao, S.W. Wind field retrieval through single Doppler weather radar and its application to NWP. J. Appl. Meteorol. Sci. 2001, 12, 488–493. [Google Scholar]
- Kelberlau, F.; Mann, J. Better turbulence spectra from velocity–azimuth display scanning wind lidar. Atmos. Meas. Tech. 2019, 12, 1871–1888. [Google Scholar] [CrossRef]
- Lhermitte, R.M.; Atlas, D. Precipitation motion by pulse Doppler radar. In Proceedings of the 9th Weather Radar Conf., Kansas, MO, USA, 23–26 October 1961; pp. 218–223. [Google Scholar]
- Browning, K.A.; Wexler, R. The determination of kinematic properties of a wind field using Doppler radar. J. Appl. Meteorol. 1968, 8, 105–113. [Google Scholar] [CrossRef]
- Srivastava, R.C.; Matejka, T.J.; Lorello, T.J. Doppler radar study of the trailing anvil region associated with a squall line. J. Atmos. Sci. 1986, 43, 356–377. [Google Scholar] [CrossRef]
- Matejka, T.; Srivastava, R.C. An Improved Version of the Extended Velocity-Azimuth Display Analysis of Single-Doppler Radar Data. J. Atmos. Ocean. Technol. 1991, 8, 453–466. [Google Scholar] [CrossRef]
- Tian, L.; Heymsfield, G.M.; Didlake, A.C. Velocity-Azimuth Display Analysis of Doppler Velocity for HIWRAP. J. Appl. Meteorol. Climatol. 2015, 54, 1792–1808. [Google Scholar] [CrossRef]
- Fernandez, D.E.; Kerr, E.M.; Castells, A.; Carswell, J.R.; Frasier, S.J.; Chang, P.S.; Marks, F.D. IWRAP: The imaging wind and rain airborne profiler for remote sensing of the ocean and the atmospheric boundary layer within tropical cyclones. IEEE Trans. Geosci. Remote Sens. 2005, 43, 1775–1787. [Google Scholar] [CrossRef]
- Guimond, S.R.; Tian, L.; Heymsfield, G.M.; Frasier, S.J. Wind retrieval algorithms for the IWRAP and HIWRAP airborne Doppler radars with applications to hurricanes. J. Atmos. Ocean. Technol. 2014, 31, 1189–1215. [Google Scholar] [CrossRef]
- Didlake, A.C.; Heymsfield, G.M.; Tian, L.; Guimond, S.R. The coplane analysis technique for three-dimensional wind retrieval using the HIWRAP airborne Doppler radar. J. Appl. Meteorol. Climatol. 2015, 54, 605–623. [Google Scholar] [CrossRef][Green Version]
- Miao, Y.; Dong, X.; Bao, Q.; Zhu, D. Perspective of a Ku-Ka Dual-Frequency Scatterometer for Simultaneous Wide-Swath Ocean Surface Wind and Current Measurement. Remote Sens. 2018, 10, 1042. [Google Scholar] [CrossRef]
- Tan, D.G.; Andersson, E.; Kloe, J.D.; Marseille, G.J.; Stoffelen, A.; Poli, P.; Flamant, P. The ADM-Aeolus wind retrieval algorithms. Tellus A Dyn. Meteorol. Oceanogr. 2008, 60, 191–205. [Google Scholar] [CrossRef]
- Li, L.; Heymsfield, G.; Carswell, J.; Schaubert, D.H.; McLinden, M.L.; Creticos, J.; Guimond, S. The NASA high-altitude imaging wind and rain airborne profiler. IEEE Trans. Geosci. Remote Sens. 2016, 54, 298–310. [Google Scholar] [CrossRef]
- Braun, S.A.; Newman, P.A.; Heymsfield, G.M. NASA’s Hurricane and Severe Storm Sentinel (HS3) Investigation. Bull. Am. Meteorol. Soc. 2016, 97, 2085–2102. [Google Scholar] [CrossRef]
- Heymsfield, G.M.; Tian, L. Hurricane and Severe Storm Sentinel (HS3) High-Altitude Imaging Wind & Rain Airborne Profiler (HIWRAP) [Indicate Subset Used]; NASA EOSDIS Global Hydrology Resource Center Distributed Active Archive Center: Huntsville, AL, USA, 2015. Available online: http://dx.doi.org/10.5067/HS3/HIWRAP/DATA101 (accessed on 27 October 2015).
- Beven, J.L.; National Hurricane Center Tropical Cyclone Report Hurricane INGRID (AL102013). National Hurricane Center. 2014. Available online: https://www.nhc.noaa.gov/data/tcr/AL102013_Ingrid.pdf (accessed on 14 April 2014).
- NOAA Satellites and Information. AL102013—Hurricane INGRID Multiplatform Satellite Surface Wind Analysis (Experimental) [EB/OL]. Available online: https://rammb-data.cira.colostate.edu/tc_realtime/archive_sub_products.asp?product=mpsatwnd&storm_identifier=al102013 (accessed on 15 September 2013).
- Atlas, R.; Hoffman, R.N.; Ardizzone, J.; Leidner, S.M.; Jusem, J.C.; Smith, D.K.; Gombos, D. A cross-calibrated, multiplatform ocean surface wind velocity product for meteorological and oceanographic applications. Bull. Am. Meteorol. Soc. 2011, 92, 157–174. [Google Scholar] [CrossRef]







| Parameters | Specifications |
|---|---|
| 8 m/s | |
| 6 m/s | |
| 1 m/s | |
| Satellite speed | 7.6 km/s |
| Orbit height | 500 km |
| Incident angle | 23°~40° |
| Azimuth rotation rate | 60 rpm |
| SNR (dB) | Retrieved Mean Wind Field Component | ||
|---|---|---|---|
| (m/s) | (m/s) | (m/s) | |
| 5 | 8.88 | 3.11 | 11.59 |
| 10 | 8.40 | 3.89 | 6.89 |
| 20 | 8.33 | 4.23 | 5.37 |
| Real value | 8.0 | 6.0 | 1.0 |
| SNR (dB) | Retrieved Mean Wind Field Component | ||||||
|---|---|---|---|---|---|---|---|
(m/s) | (m/s) | (m/s) | (m/s/km) | (m/s/km) | (m/s/km) | (m/s/km) | |
| 5 | 11.04 | 2.26 | 60.81 | 0.044 | 0.006 | 0.029 | 0.047 |
| 10 | 8.99 | 2.68 | 45.24 | 0.067 | 0.023 | 0.004 | 0.083 |
| 20 | 8.59 | 2.81 | 43.81 | 0.017 | 0.012 | 0.008 | 0.014 |
| Real value | 8.0 | 6.0 | 1.0 | 0.020 | 0.010 | 0.010 | 0.020 |
| Parameters | Specifications |
|---|---|
| Operating frequency | 35.3 GHz |
| Incident angle | 29.9° |
| Pulse repetition frequency | 3571 Hz/4464 Hz |
| Doppler accuracy | <1.5 m/s for SNR > 10 dB |
| Flying speed | 170 m/s |
| Scanning | Conical scan |
| Azimuth rotation rate | 15.86 rpm |
| SNR (dB) | RMSE of the SVAD | RMSE of the VAD | ||
|---|---|---|---|---|
| (m/s) | (m/s) | (m/s) | (m/s) | |
| 5 | 0.879 | 2.893 | 0.879 | 0.336 |
| 10 | 0.399 | 2.112 | 0.173 | 0.039 |
| 20 | 0.333 | 1.774 | 0.140 | 0.007 |
| SNR (dB) | RMSE of the SVAD | RMSE of the VAD | ||
|---|---|---|---|---|
| (m/s) | (m/s) | (m/s) | (m/s) | |
| 5 | 3.037 | 3.742 | 1.656 | 0.861 |
| 10 | 0.991 | 3.320 | 0.717 | 0.375 |
| 20 | 0.593 | 3.193 | 0.320 | 0.014 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, Y.; Wei, M.; Shi, Q. First Spaceborne Version of Velocity-Azimuth Display Technique for Wind Field Retrieval on Cloud and Precipitation Radar. Atmosphere 2020, 11, 1089. https://doi.org/10.3390/atmos11101089
Wang Y, Wei M, Shi Q. First Spaceborne Version of Velocity-Azimuth Display Technique for Wind Field Retrieval on Cloud and Precipitation Radar. Atmosphere. 2020; 11(10):1089. https://doi.org/10.3390/atmos11101089
Chicago/Turabian StyleWang, Yuexia, Ming Wei, and Quan Shi. 2020. "First Spaceborne Version of Velocity-Azimuth Display Technique for Wind Field Retrieval on Cloud and Precipitation Radar" Atmosphere 11, no. 10: 1089. https://doi.org/10.3390/atmos11101089
APA StyleWang, Y., Wei, M., & Shi, Q. (2020). First Spaceborne Version of Velocity-Azimuth Display Technique for Wind Field Retrieval on Cloud and Precipitation Radar. Atmosphere, 11(10), 1089. https://doi.org/10.3390/atmos11101089






