Wind Turbulence Statistics of the Atmospheric Inertial Sublayer under Near-Neutral Conditions
Abstract
1. Introduction
2. Method
2.1. Scaling
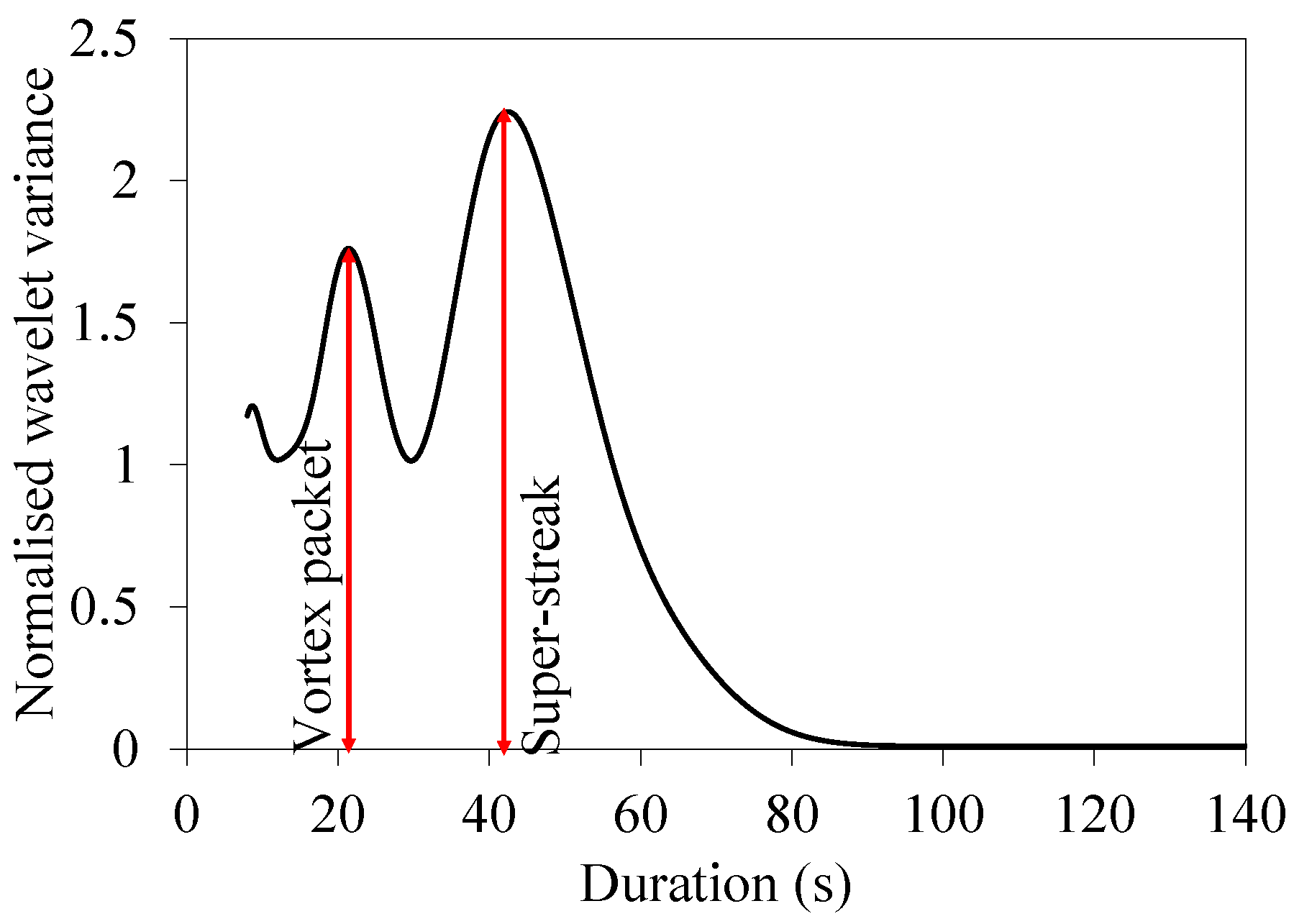
2.2. Wavelet Analysis
3. Results and Discussion
4. Conclusions
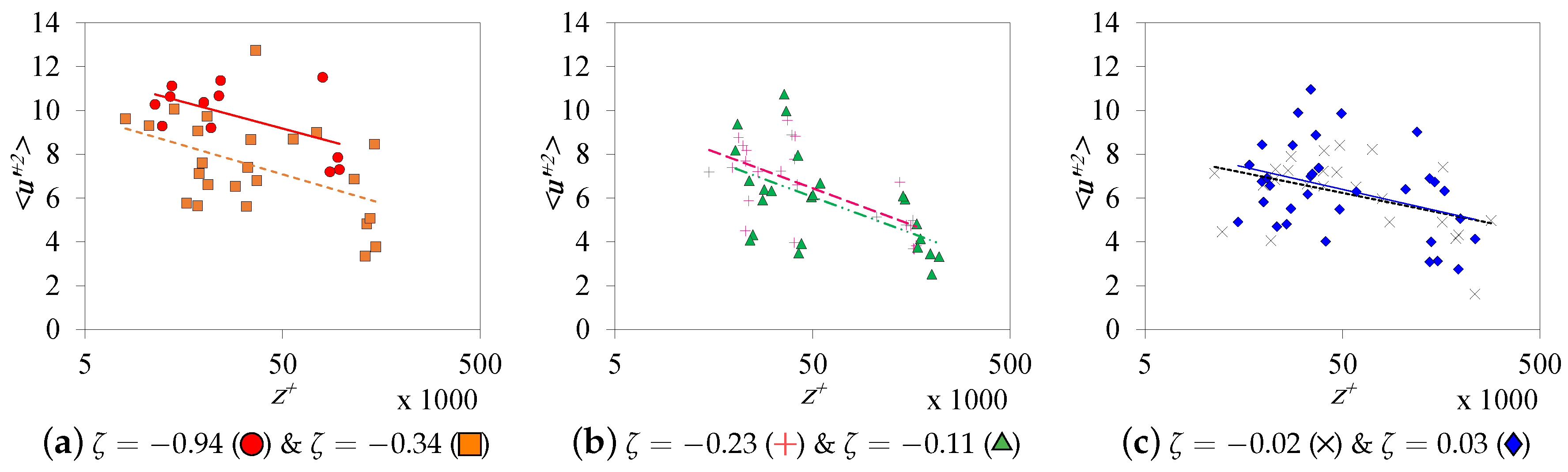
- The Townsend–Perry constant for the windward velocity variance is not a function of thermal stability in the studied range of atmospheric conditions. An average value for the current dataset is 1.302, which is consistent with those in other atmospheric studies [2].
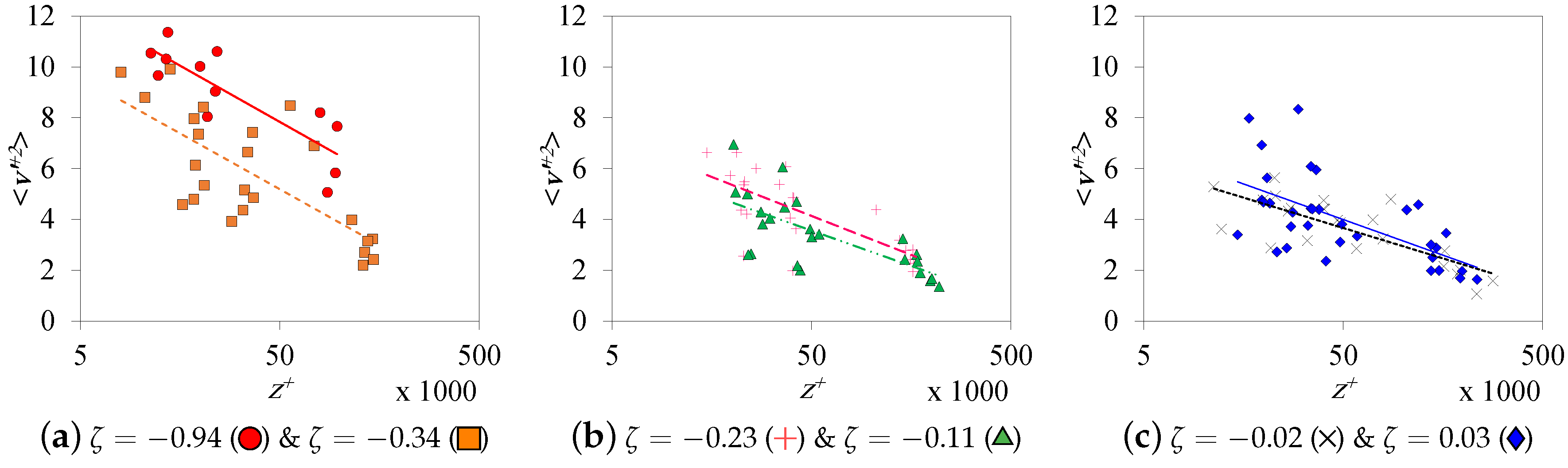
- The slope of the crosswind correlation clearly decreases in the positive stability direction. The values range between 1 and 2 for the current dataset.
- The intercept (B) of all relations increases in the convective atmosphere direction.
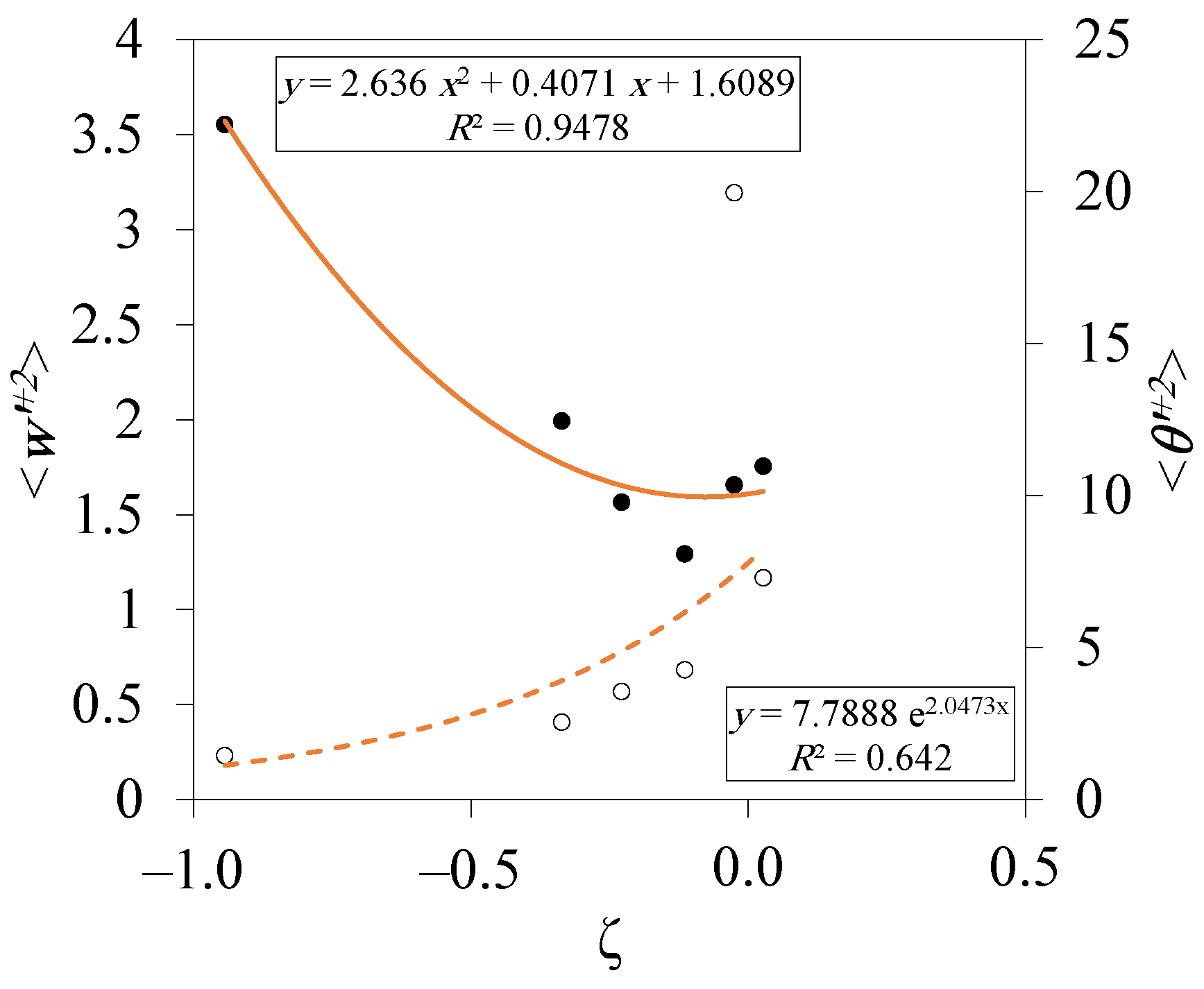
- The variances of the vertical velocity and temperature were best-fit by second-order polynomial and exponential regressions, respectively.
- The variance of the vertical velocity manifests a local peak at high altitudes and approaches asymptotic values at the ground level. The peak dampens and the asymptotic value decreases close to the neutral atmospheric condition. A ground-level (zero-) value of 1.6089 was recorded in this research.
- The temperature variance varies exponentially with , particularly at . The fluctuations strengthen with height under stable conditions and dampen with height under convective conditions. The ground-level value in neutral atmosphere is 7.7888.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Nagib, H.M.; Chauhan, K.A. Variations of von Kármán coefficient in canonical flows. Phys. Fluids 2008, 20, 101518. [Google Scholar] [CrossRef]
- Marusic, I.; Monty, J.P.; Hultmark, M.; Smits, A.J. On the logarithmic region in wall turbulence. J. Fluid Mech. 2013, 716, R3. [Google Scholar] [CrossRef]
- Miguntanna, N.S.; Moses, H.; Sivakumar, M.; Yang, S.Q.; Enever, K.J.; Riaz, M.Z.B. Re-examining log law velocity profile in smooth open channel flows. Environ. Fluid Mech. 2020, 20, 953–986. [Google Scholar] [CrossRef]
- Townsend, A. The Structure of Turbulent Shear Flow; Cambridge Monographs on Mechanics, Cambridge University Press: Cambridge, UK, 1976. [Google Scholar]
- Townsend, A. Equilibrium layers and wall turbulence. J. Fluid Mech. 1961, 11, 97–120. [Google Scholar] [CrossRef]
- Nickels, T.; Marusic, I.; Hafez, S.; Hutchins, N.; Chong, M. Some predictions of the attached eddy model for a high Reynolds number boundary layer. Philos. Trans. R. Soc. Lond. A Math. Phys. Eng. Sci. 2007, 365, 807–822. [Google Scholar] [CrossRef]
- Head, M.; Bandyopadhyay, P. New aspects of turbulent boundary-layer structure. J. Fluid Mech. 1981, 107, 297–338. [Google Scholar] [CrossRef]
- Perry, A.; Chong, M. On the mechanism of wall turbulence. J. Fluid Mech. 1982, 119, 173–217. [Google Scholar] [CrossRef]
- Perry, A.; Henbest, S.; Chong, M. A theoretical and experimental study of wall turbulence. J. Fluid Mech. 1986, 165, 163–199. [Google Scholar] [CrossRef]
- Marusic, I. On the role of large-scale structures in wall turbulence. Phys. Fluids 2001, 13, 735–743. [Google Scholar] [CrossRef]
- Adrian, R.; Meinhart, C.; Tomkins, C. Vortex organization in the outer region of the turbulent boundary layer. J. Fluid Mech. 2000, 422, 1–54. [Google Scholar] [CrossRef]
- Zhou, J.; Adrian, R.J.; Balachandar, S.; Kendall, T. Mechanisms for generating coherent packets of hairpin vortices in channel flow. J. Fluid Mech. 1999, 387, 353–396. [Google Scholar] [CrossRef]
- Dennis, D.J.; Nickels, T.B. Experimental measurement of large-scale three-dimensional structures in a turbulent boundary layer. Part 1. Vortex packets. J. Fluid Mech. 2011, 673, 180–217. [Google Scholar] [CrossRef]
- Woodcock, J.; Marusic, I. The Attached Eddy Hypothesis and von Kármán’s Constant. Dynamics 2014, 7, 8. [Google Scholar]
- Hwang, Y. Statistical structure of self-sustaining attached eddies in turbulent channel flow. J. Fluid Mech. 2015, 767, 254–289. [Google Scholar] [CrossRef]
- Hwang, Y.; Cossu, C. Self-sustained process at large scales in turbulent channel flow. Phys. Rev. Lett. 2010, 105, 044505. [Google Scholar] [CrossRef] [PubMed]
- Cossu, C.; Hwang, Y. Self-sustaining processes at all scales in wall-bounded turbulent shear flows. Philos. Trans. R. Soc. A 2017, 375, 20160088. [Google Scholar] [CrossRef] [PubMed]
- Hultmark, M. A theory for the streamwise turbulent fluctuations in high Reynolds number pipe flow. J. Fluid Mech. 2012, 707, 575–584. [Google Scholar] [CrossRef]
- Harun, Z.; Ghopa, W.A.W.; Abdullah, S.; Ghazali, M.I.; Abbas, A.A.; Rasani, M.R.; Zulkifli, R.; Wan Mahmood, W.; Mansor, M.R.A.; Abidin, Z.Z.; et al. The development of a multi-purpose wind tunnel. J. Teknol. 2016, 10, 63–70. [Google Scholar] [CrossRef][Green Version]
- Meneveau, C.; Marusic, I. Generalized logarithmic law for high-order moments in turbulent boundary layers. J. Fluid Mech. 2013, 719, R1. [Google Scholar] [CrossRef]
- Monin, A.S.; Obukhov, A.M. Osnovnye zakonomernosti turbulentnogo peremesivanija v prizemnom sloe atmosfery (Basic Laws of Turbulent Mixing in the Atmosphere Near the Ground). Tr. Geofiz. Inst. SSSR 1954, 24, 163–187. [Google Scholar]
- Dyer, A. A review of flux-profile relationships. Bound.-Layer Meteorol. 1974, 7, 363–372. [Google Scholar] [CrossRef]
- Lotfy, E.R.; Harun, Z. Effect of atmospheric boundary layer stability on the inclination angle of turbulence coherent structures. Environ. Fluid Mech. 2017, 18, 637–659. [Google Scholar] [CrossRef]
- Pahlow, M.; Parlange, M.B.; Porté-Agel, F. On Monin–Obukhov similarity in the stable atmospheric boundary layer. Bound.-Layer Meteorol. 2001, 99, 225–248. [Google Scholar] [CrossRef]
- Kader, B.; Yaglom, A. Mean fields and fluctuation moments in unstably stratified turbulent boundary layers. J. Fluid Mech. 1990, 212, 637–662. [Google Scholar] [CrossRef]
- Emeis, S. Surface-Based Remote Sensing of the Atmospheric Boundary Layer; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2010; Volume 40. [Google Scholar]
- Nieuwstadt, F. Some aspects of the turbulent stable boundary layer. In Boundary Layer Structure; Springer: Berlin/Heidelberg, Germany, 1984; pp. 31–55. [Google Scholar]
- Nieuwstadt, F.T. The turbulent structure of the stable, nocturnal boundary layer. J. Atmos. Sci. 1984, 41, 2202–2216. [Google Scholar] [CrossRef]
- Harun, Z.; Lotfy, E.R. Generation, Evolution, and Characterization of Turbulence Coherent Structures. In Turbulence and Related Phenomena; IntechOpen: London, UK, 2018. [Google Scholar]
- Adrian, R.J. Hairpin vortex organization in wall turbulence a. Phys. Fluids 2007, 19, 041301. [Google Scholar] [CrossRef]
- Dennis, D.J.; Nickels, T.B. Experimental measurement of large-scale three-dimensional structures in a turbulent boundary layer. Part 2. Long structures. J. Fluid Mech. 2011, 673, 218–244. [Google Scholar] [CrossRef]
- Lotfy, E.R.; Abbas, A.A.; Zaki, S.A.; Harun, Z. Characteristics of Turbulent Coherent Structures in Atmospheric Flow Under Different Shear–Buoyancy Conditions. Bound.-Layer Meteorol. 2019, 173, 115–141. [Google Scholar] [CrossRef]
- Chauhan, K.; Hutchins, N.; Monty, J.; Marusic, I. Structure inclination angles in the convective atmospheric surface layer. Bound.-Layer Meteorol. 2013, 147, 41–50. [Google Scholar] [CrossRef]
- Thomas, C.; Foken, T. Organised motion in a tall spruce canopy: Temporal scales, structure spacing and terrain effects. Bound.-Layer Meteorol. 2007, 122, 123–147. [Google Scholar] [CrossRef]
- Barthlott, C.; Drobinski, P.; Fesquet, C.; Dubos, T.; Pietras, C. Long-term study of coherent structures in the atmospheric surface layer. Bound.-Layer Meteorol. 2007, 125, 1–24. [Google Scholar] [CrossRef]
- Kim, K.; Adrian, R. Very large-scale motion in the outer layer. Phys. Fluids 1999, 11, 417–422. [Google Scholar] [CrossRef]
- Högström, U.; Smedman, A.S. Accuracy of sonic anemometers: Laminar wind-tunnel calibrations compared to atmospheric in situ calibrations against a reference instrument. Bound.-Layer Meteorol. 2004, 111, 33–54. [Google Scholar] [CrossRef]
- Larsén, X.; Smedman, A.S.; Högström, U. Air–sea exchange of sensible heat over the Baltic Sea. Q. J. R. Meteorol. Soc. 2004, 130, 519–539. [Google Scholar] [CrossRef]
- Hommema, S.E.; Adrian, R.J. Packet structure of surface eddies in the atmospheric boundary layer. Bound.-Layer Meteorol. 2003, 106, 147–170. [Google Scholar] [CrossRef]
- Hutchins, N.; Chauhan, K.; Marusic, I.; Monty, J.; Klewicki, J. Towards reconciling the large-scale structure of turbulent boundary layers in the atmosphere and laboratory. Bound.-Layer Meteorol. 2012, 145, 273–306. [Google Scholar] [CrossRef]
- Thomas, C.; Foken, T. Detection of long-term coherent exchange over spruce forest using wavelet analysis. Theor. Appl. Clim. 2005, 80, 91–104. [Google Scholar] [CrossRef]
- Júnior, C.D.; Sá, L.; Pachêco, V.; de Souza, C. Coherent structures detected in the unstable atmospheric surface layer above the Amazon forest. J. Wind Eng. Ind. Aerodyn. 2013, 115, 1–8. [Google Scholar] [CrossRef]
- Starkenburg, D.; Fochesatto, G.J.; Prakash, A.; Cristóbal, J.; Gens, R.; Kane, D.L. The role of coherent flow structures in the sensible heat fluxes of an Alaskan boreal forest. J. Geophys. Res. Atmos. 2013, 118, 8140–8155. [Google Scholar] [CrossRef]
- Vickers, D.; Mahrt, L. Observations of the cross-wind velocity variance in the stable boundary layer. Environ. Fluid Mech. 2007, 7, 55–71. [Google Scholar] [CrossRef]
- Mahrt, L. Characteristics of submeso winds in the stable boundary layer. Bound.-Layer Meteorol. 2009, 130, 1–14. [Google Scholar] [CrossRef]
- Lotfy, E.R.; Zaki, S.A.; Harun, Z. Modulation of the atmospheric turbulence coherent structures by mesoscale motions. J. Braz. Soc. Mech. Sci. Eng. 2018, 40, 178. [Google Scholar] [CrossRef]
- Laval, J.P.; Vassilicos, J.C.; Foucaut, J.M.; Stanislas, M. Comparison of turbulence profiles in high-Reynolds-number turbulent boundary layers and validation of a predictive model. J. Fluid Mech. 2017, 814, R2. [Google Scholar] [CrossRef]
- Carlier, J.; Stanislas, M. Experimental study of eddy structures in a turbulent boundary layer using particle image velocimetry. J. Fluid Mech. 2005, 535, 143–188. [Google Scholar] [CrossRef]
- Diwan, S.S.; Morrison, J.F. Intermediate Scaling and Logarithmic Invariance in Turbulent Pipe Flow. arXiv 2019, arXiv:1909.11951. [Google Scholar]
- Vallikivi, M.; Ganapathisubramani, B.; Smits, A.J. Spectral scaling in boundary layers and pipes at very high Reynolds numbers. J. Fluid Mech. 2015, 771, 303–326. [Google Scholar] [CrossRef]
- Sultan, T.; Ahmad, S.; Cho, J. Numerical study of the effects of surface roughness on water disinfection UV reactor. Chemosphere 2016, 148, 108–117. [Google Scholar] [CrossRef]
- Kunkel, G.J.; Marusic, I. Study of the near-wall-turbulent region of the high-Reynolds-number boundary layer using an atmospheric flow. J. Fluid Mech. 2006, 548, 375–402. [Google Scholar] [CrossRef]

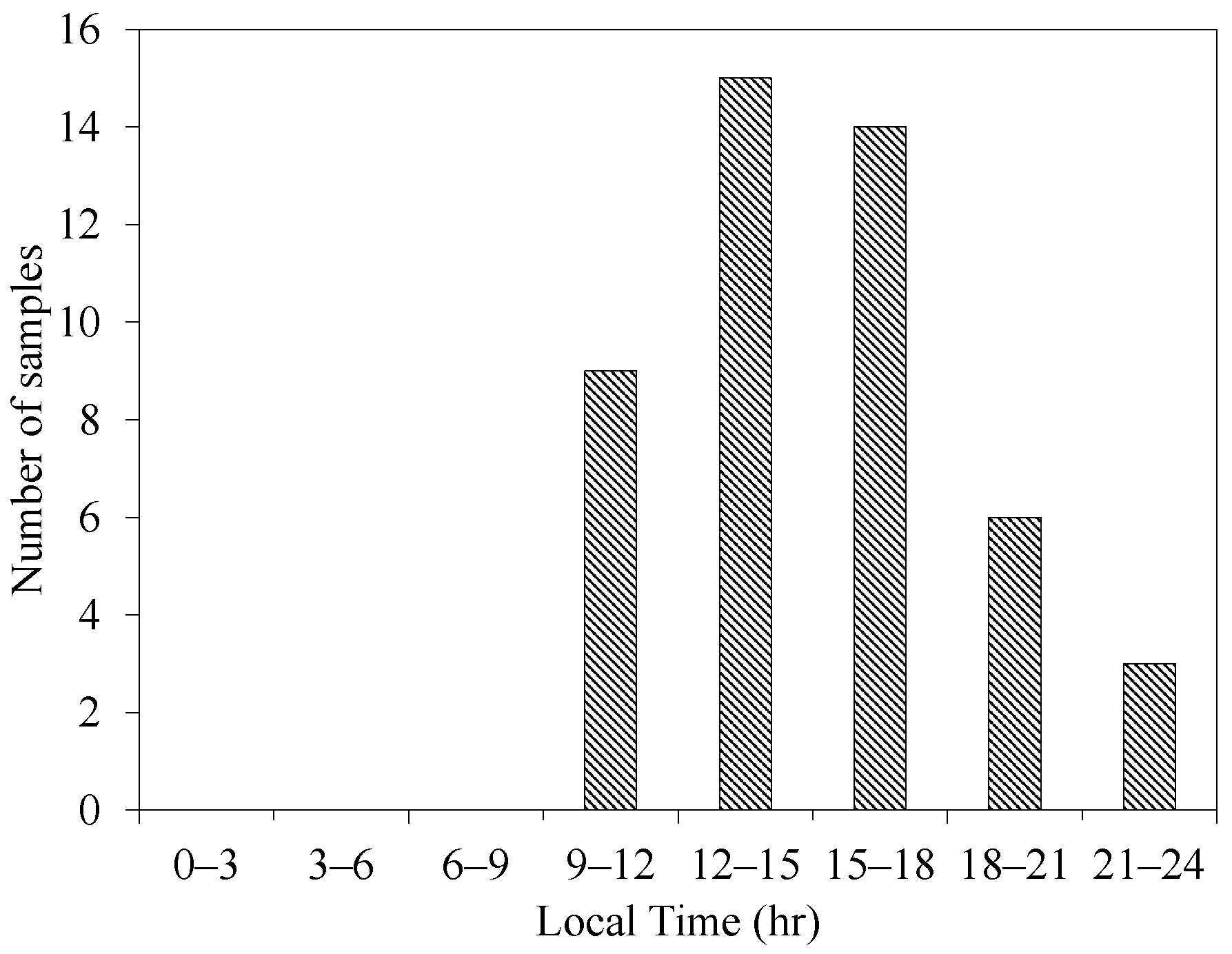







| No | Source | Nominal | Range of | Number of Samples | Data Symbol | Fitting Line |
|---|---|---|---|---|---|---|
| 1 | Current research | to | 12 |  |  | |
| 2 | Current research | to | 24 |  |  | |
| 3 | Current research | to | 24 | + |  | |
| 4 | Current research | to | 24 |  |  | |
| 5 | Current research | to | 24 | × |  | |
| 6 | Current research | to | 33 |  |  | |
| 7 | Hutchins et al. [40] | 0 | to | 4 | ○ | |
| 8 | Kunkel and Marusic [52] | 0 | ∼0 | 9 | ∗ |
| Stability Range | ||
|---|---|---|
| to | ||
| to | ||
| to | ||
| to | ||
| to | ||
| to | ||
| Relation | Source | Notes | Scale | Parameter |
|---|---|---|---|---|
| Current research | as an average for the whole dataset, Figure 8 | |||
| Marusic et al. [2] | ∼2.60 | |||
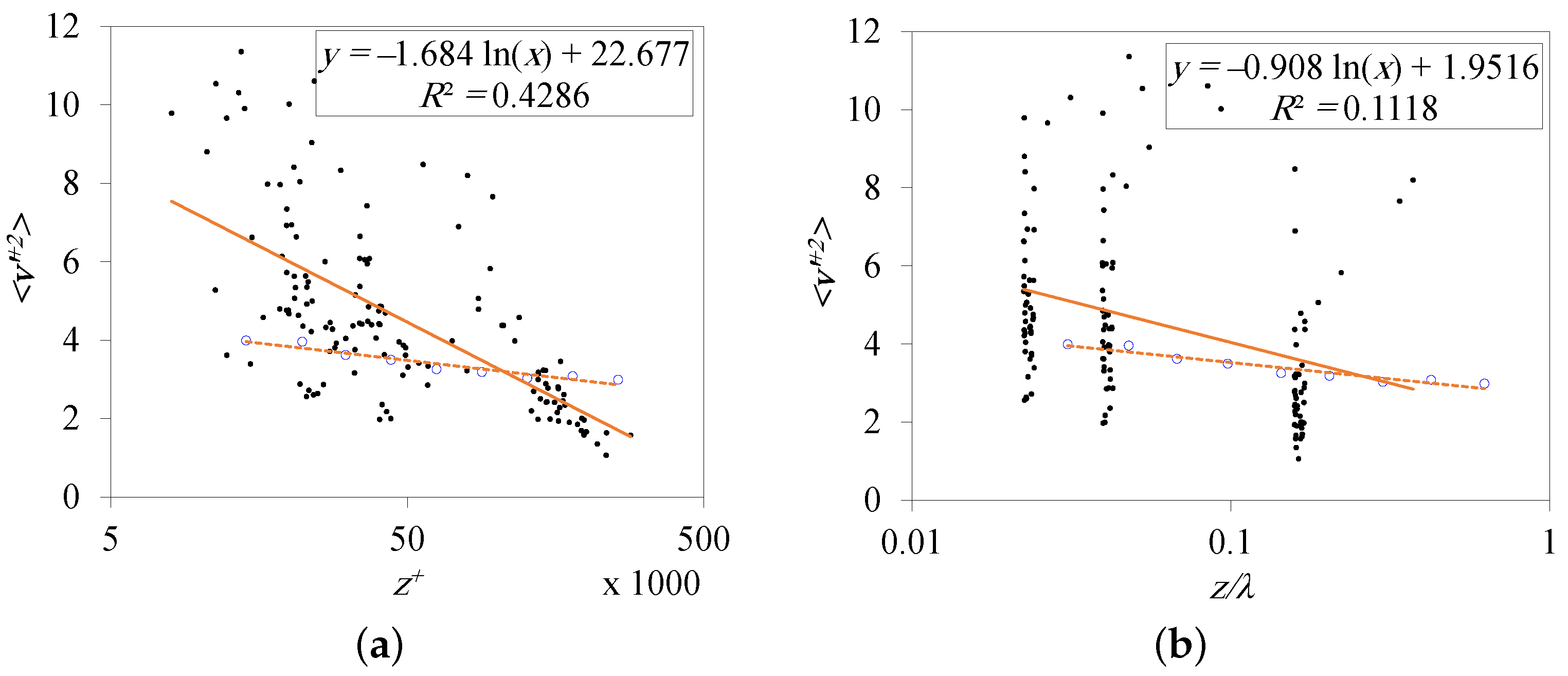
| Current research | as an average for the whole dataset, Figure 7a | |||
| Hutchins et al. [40] | ||||
| Current research | as an average for the whole dataset, Figure 7b | |||
| Marusic et al. [2] | ||||
| Current research | from second-order polynomial interpolation, Figure 13c | |||
| Hutchins et al. [40] | ||||
| Current research | from second-order polynomial interpolation, Figure 13d | |||
| Hutchins et al. [40] | ||||
| Current research | from second-order polynomial interpolation for ground-level values, Figure 14 | |||
| Kunkel and Marusic [52] | ||||
| Current research | from exponential interpolation for ground-level values, Figure 14 | |||
| Pahlow et al. [24], Nieuwstadt [28] |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lotfy, E.R.; Harun, Z. Wind Turbulence Statistics of the Atmospheric Inertial Sublayer under Near-Neutral Conditions. Atmosphere 2020, 11, 1087. https://doi.org/10.3390/atmos11101087
Lotfy ER, Harun Z. Wind Turbulence Statistics of the Atmospheric Inertial Sublayer under Near-Neutral Conditions. Atmosphere. 2020; 11(10):1087. https://doi.org/10.3390/atmos11101087
Chicago/Turabian StyleLotfy, Eslam Reda, and Zambri Harun. 2020. "Wind Turbulence Statistics of the Atmospheric Inertial Sublayer under Near-Neutral Conditions" Atmosphere 11, no. 10: 1087. https://doi.org/10.3390/atmos11101087
APA StyleLotfy, E. R., & Harun, Z. (2020). Wind Turbulence Statistics of the Atmospheric Inertial Sublayer under Near-Neutral Conditions. Atmosphere, 11(10), 1087. https://doi.org/10.3390/atmos11101087






