Hygroscopy as an Indicator of Specific Surface Area in Polymer Materials
Abstract
1. Introduction
- Derivation of the fundamental thermodynamic dependence of SSA and hygroscopic water content in dispersed materials;
- Experimental analysis of thermodynamic water retention curves and water vapor sorption isotherms in biopolymers (starch, cellulose) and synthetic composite water superabsorbents with an acrylic polymer matrix;
- Statistical comparison of the thermodynamic approach and the standard BET method for the SSA assessment, and substantiation of the methodology for assessing the SSA through hygroscopy in polymer materials.
2. Materials and Methods
2.1. Tested Biopolymers and Synthetic Polymer Materials
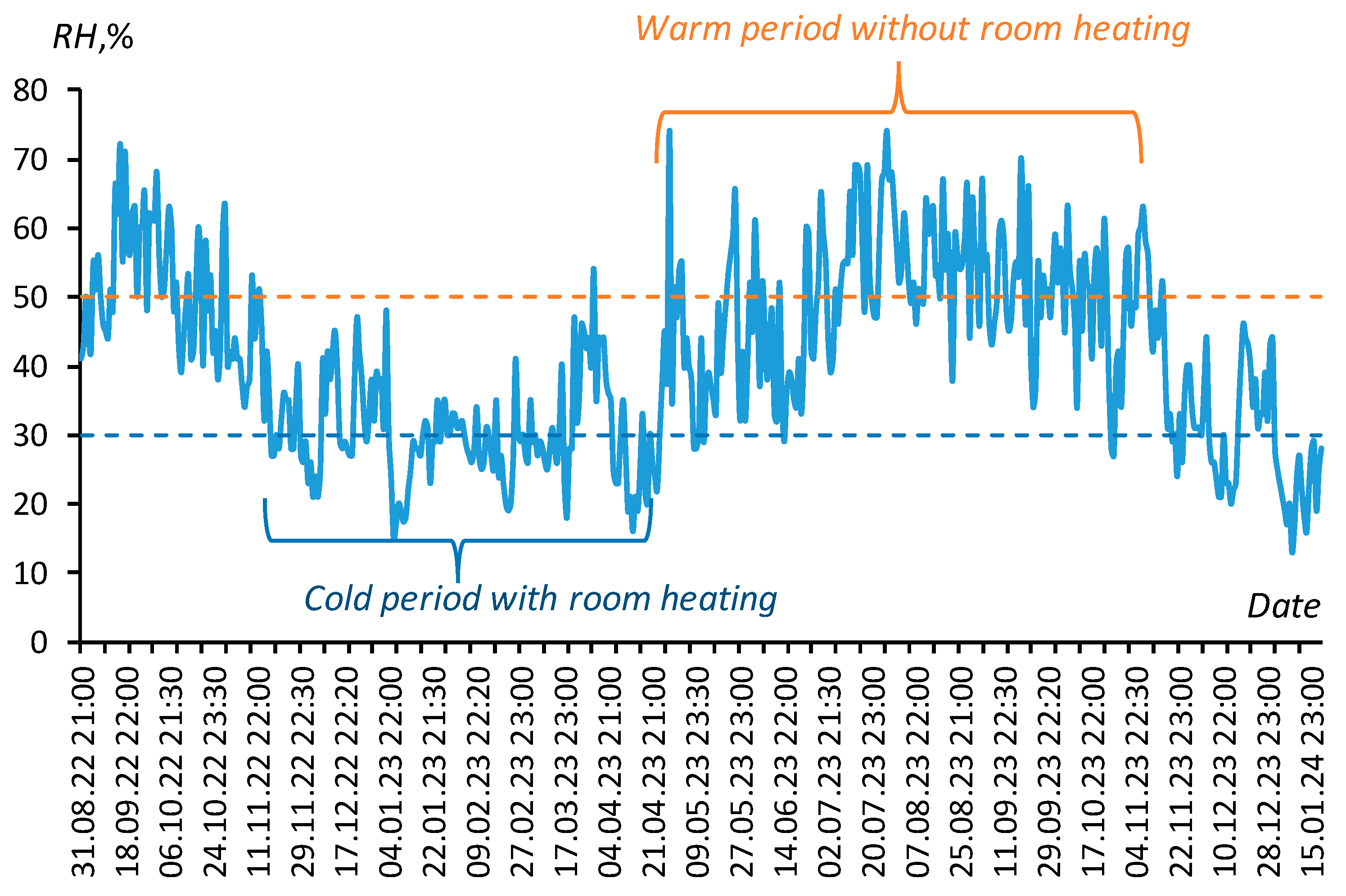
2.2. Hygroscopy, Water Retention, and Water Vapor Sorption Isotherm Analysis
2.3. Additional Methods and Data Processing
3. Results
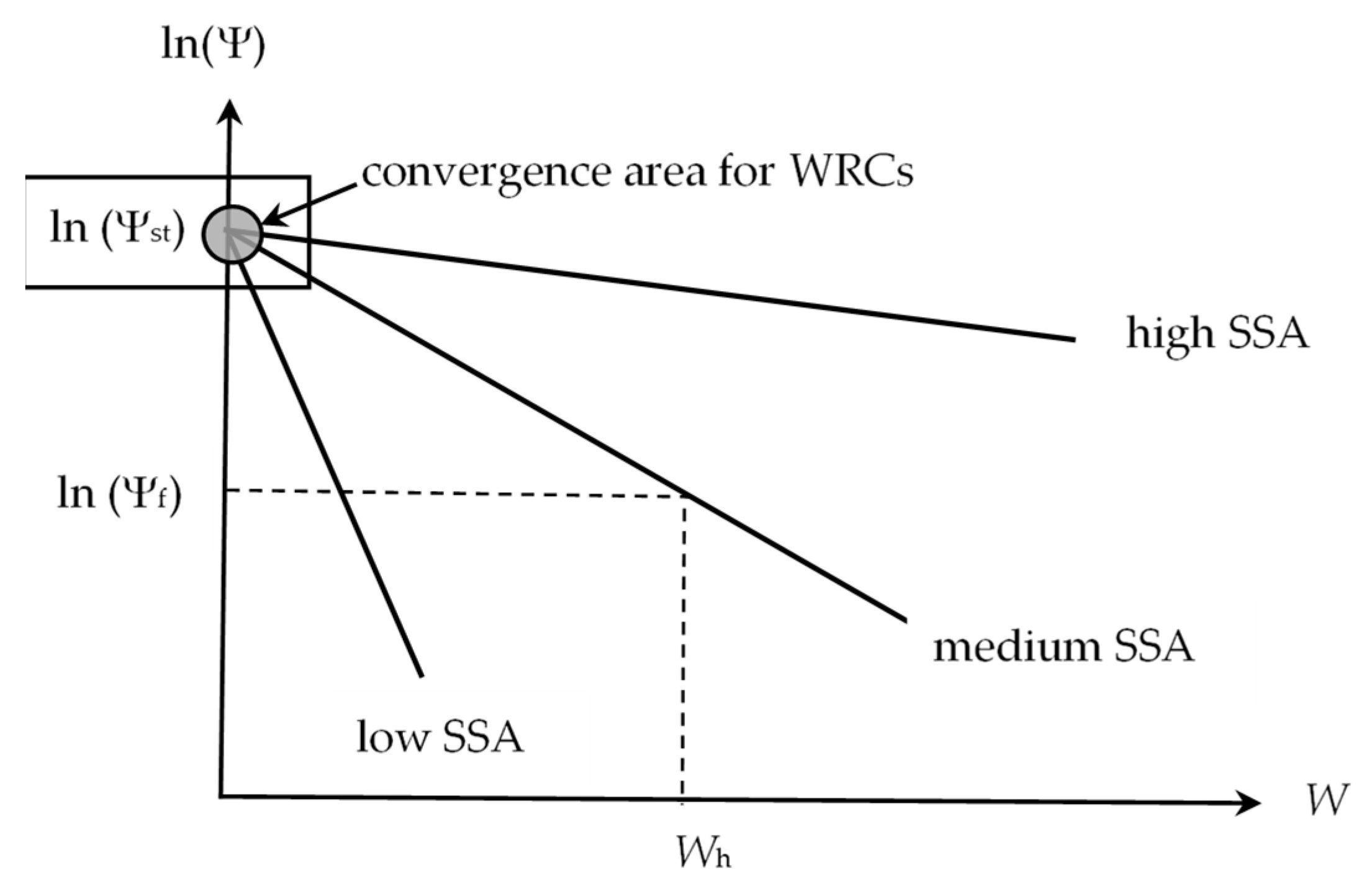
3.1. Theoretical Basis for the Thermodynamic Estimation of SSA through the Hygroscopy of Materials
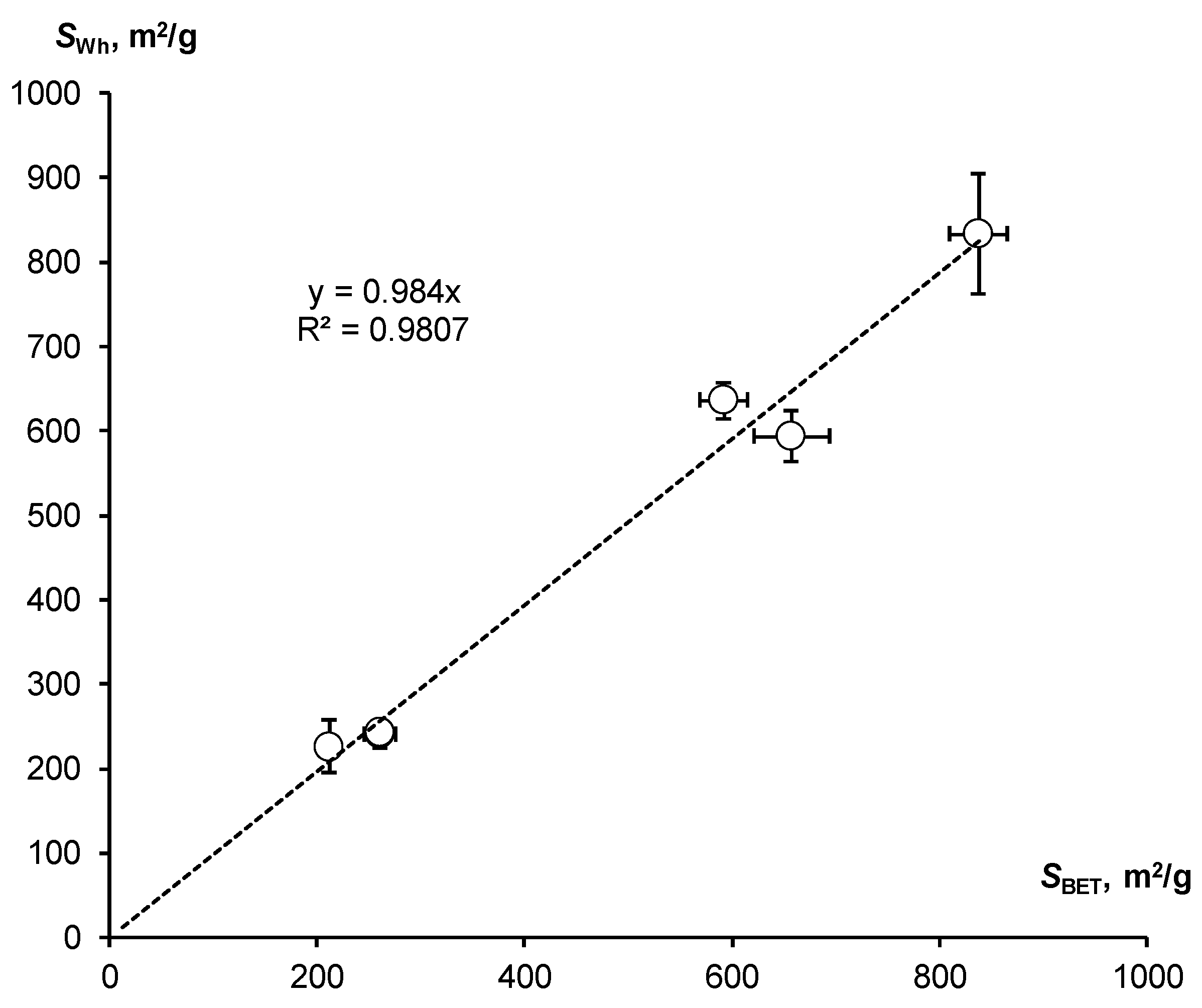
3.2. Experimental Results; Mathematical and Statistical Processing
4. Discussion
4.1. Comparison of the Obtained Results with Known Data
4.1.1. Hygroscopicity of Biopolymers and Synthetic Polymers
4.1.2. Specific Surface Area of Biopolymers and Synthetic Polymers
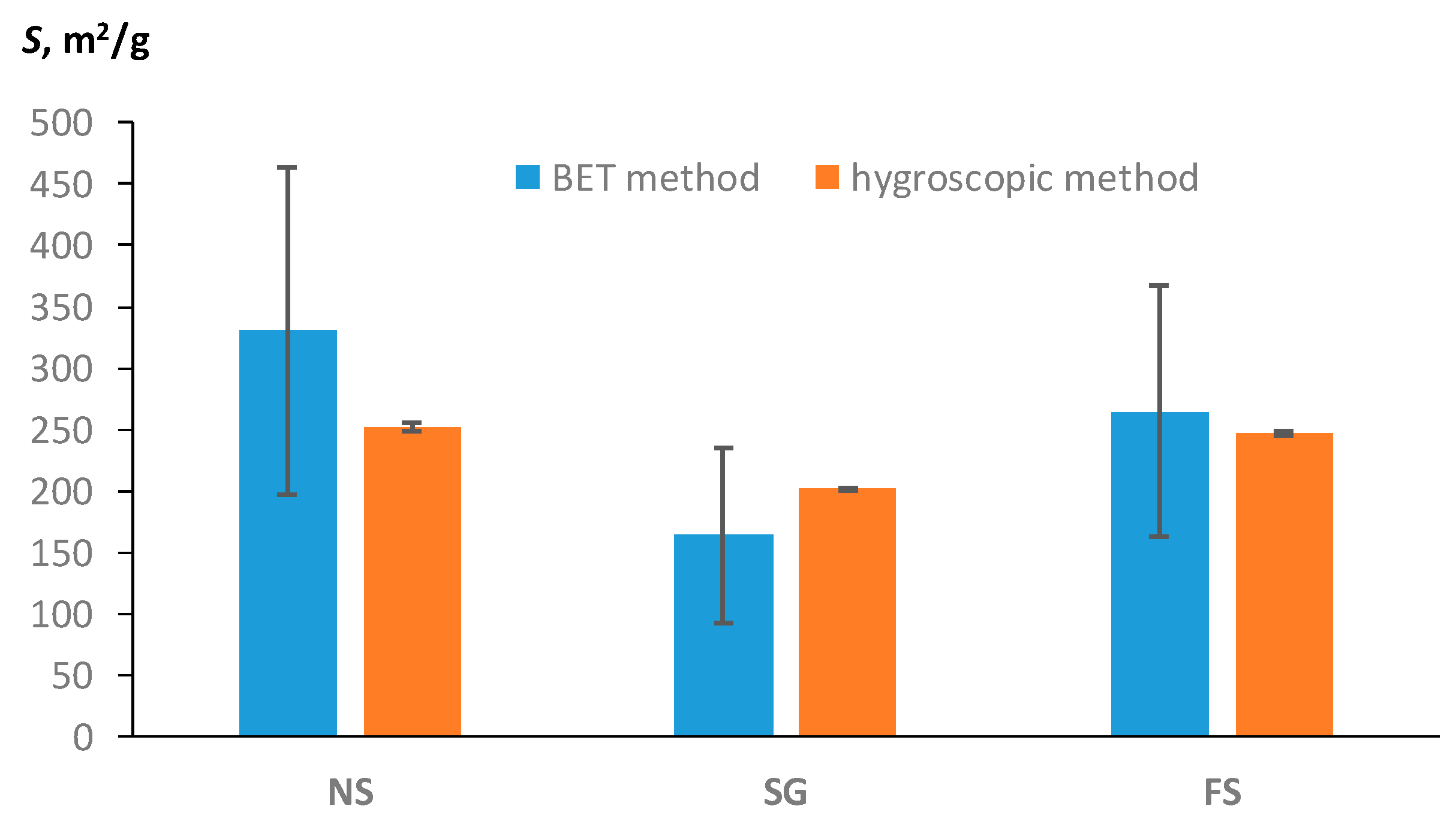
4.2. Estimation of Specific Surface Area Using Hygroscopy
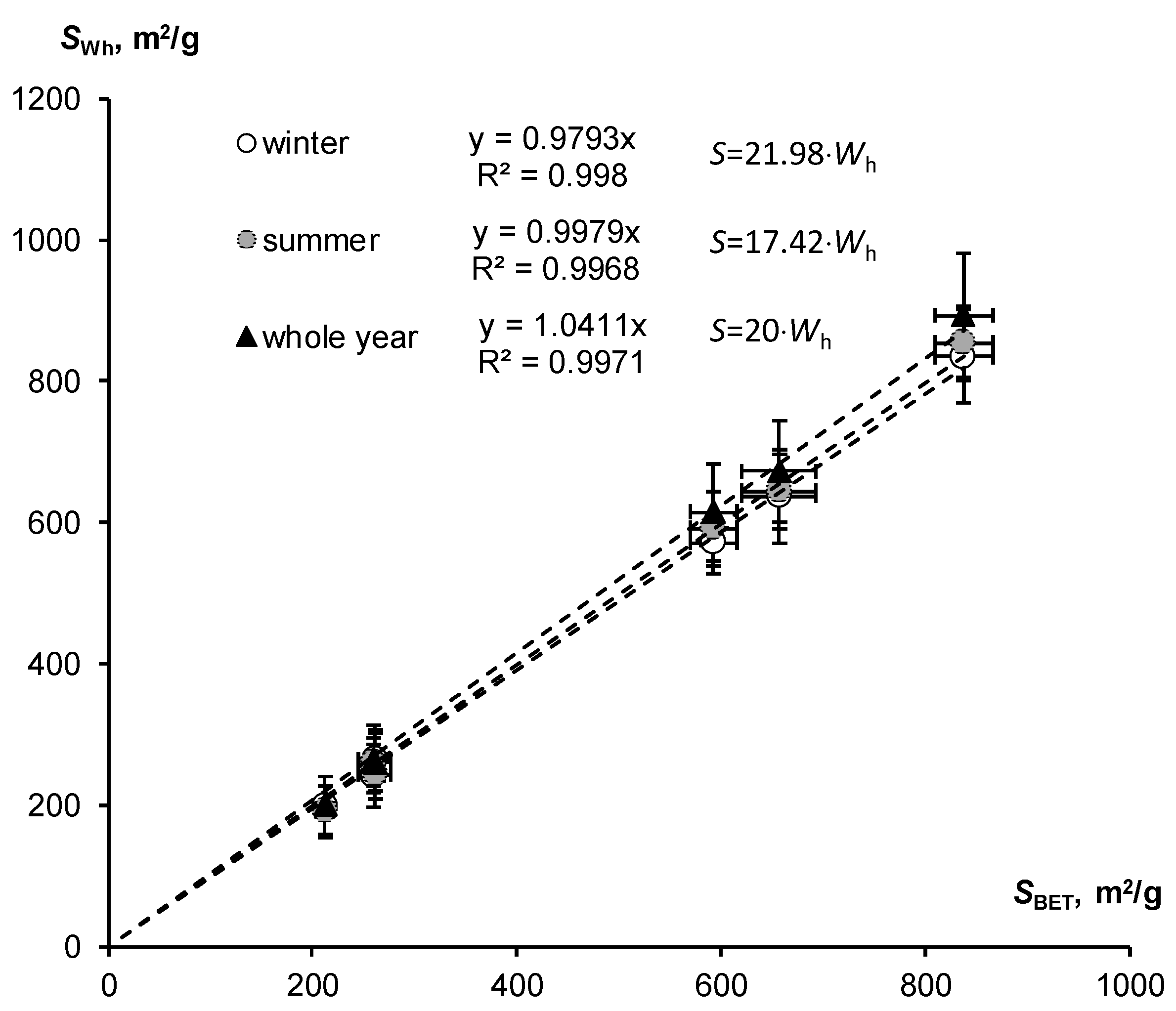
4.3. Independent Validation of a New Approach for Assessing SSA Using Hygroscopy
5. Conclusions
6. Patents
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Gregg, S.J.; Sing, K.S.W. Adsorption, Surface Area and Porosity, 2nd ed.; Academic Press Inc.: San Diego, CA, USA, 1982; p. 303. [Google Scholar]
- Lee, V.A.; Craig, R.G.; Filisko, F.E.; Zand, R. Preparation and characterization of high-surface-area polymer substrates for microcalorimetry. J. Biomed. Mater. Res. A 1996, 31, 51–62. [Google Scholar] [CrossRef]
- Kaewprasit, C.; Abidi, N.; Gourlot, J.P. Effect of Adsorbed Water on the Specific Surface Area of some Standards Cotton. In Proceedings of the Beltwide Cotton Conference, National Cotton Council, Memphis, TN, USA, 3–7 January 1999; Volume 1, pp. 710–712. [Google Scholar]
- Gu, C.; Liu, D.; Huang, W.; Liu, J.; Yang, R. Synthesis of covalent triazine-based frameworks with high CO2 adsorption and selectivity. Polym. Chem. 2015, 6, 7410–7417. [Google Scholar] [CrossRef]
- Dai, J.F.; Wang, G.J.; Ma, L.; Wu, C.K. Surface Properties of Graphene: Relationship to Graphene-Polymer Composites. Rev. Adv. Mater. Sci. 2015, 40, 60–71. [Google Scholar]
- Mansurov, Z.A. Carbon Nanomaterials in Biomedicine and the Environment; Jenny Stanford Publishing: Singapore, 2020; p. 462. [Google Scholar]
- Jiang, J.-X.; Su, F.; Trewin, A.; Wood, C.D.; Niu, H.; Jones, J.T.A.; Khimyak, Y.Z.; Cooper, A.I. Synthetic Control of the Pore Dimension and Surface Area in Conjugated Microporous Polymer and Copolymer Networks. J. Am. Chem. Soc. 2008, 130, 7710–7720. [Google Scholar] [CrossRef] [PubMed]
- Iwuozor, K.O.; Ighalo, J.O.; Emenike, E.C.; Igwegbe, C.A.; Adeniyi, A.G. Do adsorbent pore size and specific surface area affect the kinetics of methyl orange aqueous phase adsorption? J. Chem. Lett. 2021, 2, 188–198. [Google Scholar]
- Gong, L. A superhydrophobic and porous polymer adsorbent with large surface area. J. Mater. Chem. A 2021, 9, 254–258. [Google Scholar] [CrossRef]
- Rothon, R. Fillers for Polymer Applications; Springer International Publishing: London, UK, 2017; p. 489. [Google Scholar]
- Zhang, L.; Ma, J.; Lyu, B.; Zhang, Y.; Gao, D.; Liu, C.; Li, X. Mitochondrial structure-inspired high specific surface area polymer microspheres by encapsulating modified graphene oxide nanosheets. Eur. Polym. J. 2020, 130, 109682. [Google Scholar] [CrossRef]
- Xu, X.; Bizmark, N.; Christie, K.S.S.; Datta, S.S.; Ren, Z.J.; Priestley, R.D. Thermoresponsive Polymers for Water Treatment and Collection. Macromolecules 2022, 55, 1894–1909. [Google Scholar] [CrossRef]
- Guo, Y.; Guan, W.; Lei, C.; Lu, H.; Shi, W.; Yu, G. Scalable super hygroscopic polymer films for sustainable moisture harvesting in arid environments. Nat. Commun. 2022, 13, 2761. [Google Scholar] [CrossRef]
- Baldwin, P.M.; Adler, J.; Davies, M.C.; Melia, C.D. Holes in Starch Granules: Confocal, SEM and Light Microscopy Studies of Starch Granule Structure. Starch Stärke 1994, 46, 341–346. [Google Scholar] [CrossRef]
- Eichhorn, S.J.; Sampson, W.W. Relationships between specific surface area and pore size in electrospun polymer fibre networks. J. R. Soc. Interface 2010, 7, 641–649. [Google Scholar] [CrossRef] [PubMed]
- Shanti, N.O.; Chan, V.W.L.; Stock, S.R.; De Carlo, F.; Thornton, K.; Faber, K.T. X-ray micro-computed tomography and tortuosity calculations of percolating pore networks. Acta Mater. 2014, 71, 126–135. [Google Scholar] [CrossRef]
- Sing, K. The use of nitrogen adsorption for the characterization of porous materials. Colloids Surf. A Physicochem. Eng. Aspects 2001, 187–188, 3–9. [Google Scholar] [CrossRef]
- ISO 9277:2022; Determination of the Specific Surface Area of Solids by Gas Adsorption—BET Method. Available online: https://www.iso.org/standard/71014.html (accessed on 13 September 2023).
- Detsi, E. Metallic Muscles: Enhanced Strain and Electrolyte-Free Actuation. S.N. 2012. Available online: https://research.rug.nl/en/publications/metallic-muscles-enhanced-strain-and-electrolyte-free-actuation (accessed on 13 September 2023).
- Włodarczyk-Stasiak, M.; Jamroz, J. Specific surface area and porosity of starch extrudates determined from nitrogen adsorption data. J. Food Eng. 2009, 93, 379–385. [Google Scholar] [CrossRef]
- Sjostedt, A. Preparation and Characterization of Nanoporous Cellulose Fibres and their Use in New Material Concepts. Doctoral Thesis, KTH Royal Institute of Technology, Stockholm, Sweden, 2014. Available online: https://kth.diva-portal.org/smash/get/diva2:761478/FULLTEXT01.pdf (accessed on 13 September 2023).
- Ocieczek, A.; Mesinger, D.; Toczek, H. Hygroscopic Properties of Three Cassava (Manihot esculenta Crantz) Starch Products: Application of BET and GAB Models. Foods 2022, 11, 1966. [Google Scholar] [CrossRef]
- Smagin, A.V.; Sadovnikova, N.B.; Belyaeva, E.A. Hygroscopicity of gel-forming composite materials: Thermodynamic assessment and technological significance. J. Compos. Sci. 2022, 6, 269. [Google Scholar] [CrossRef]
- Andrade, R.D.; Lemus, R.; Perez, C.E. Models of sorption isotherms for food: Uses and limitations. Vitae 2011, 18, 325–334. [Google Scholar] [CrossRef]
- Asomaning, J.M.; Sacande, M.; Olympio, N.S. Water sorption isotherm characteristics of seeds of six indigenous forest tree species in Ghana. West Afr. J. Appl. Ecol. 2011, 18, 15–28. [Google Scholar]
- Ayala, A.A.; Serna-Cock, L.; Giraldo, C.J.G. Moisture adsorption isotherms in yellow pitahaya (Selenicereusmegalanthus). Dyna 2011, 78, 7–14. [Google Scholar]
- Amankwah, E.A.; Dsizi, K.A.; van Straten, G.; van Boxtel, A.J.B. Modeling the equilibrium moisture content of desorption and adsorption of yam (Dente). Agric. Eng. Int. CIGR J. 2018, 20, 184–192. [Google Scholar]
- Resurreccion, A.C.; Moldrup, P.; Tuller, M.; Ferre, T.P.A.; Kawamoto, K.; Komatsu, T.; De Jonge, L.W. Relationship between specific surface area and the dry end of the water retention curve for soils with varying clay and organic carbon contents. Water Resour. Res. 2011, 47, W06522. [Google Scholar] [CrossRef]
- Schneider, M.; Goss, K.U. Prediction of the water sorption isotherm in air dry soils. Geoderma 2012, 170, 64–69. [Google Scholar] [CrossRef]
- Yan, F.; Tuller, M.; de Jonge, L.W.; Moldrup, P.; Arthur, E. Specific surface area of soils with different clay mineralogy can be estimated from a single hygroscopic water content. Geoderma 2023, 438, 116614. [Google Scholar] [CrossRef]
- Han, S.; Hong, J.; Luo, Q.; Xu, H.; Tan, H.; Wang, Q.; Tao, J.; Zhou, Y.; Peng, L.; He, Y.; et al. Hygroscopicity of organic compounds as a function of organic functionality, water solubility, molecular weight, and oxidation level. Atmos. Chem. Phys. 2022, 22, 3985–4004. [Google Scholar] [CrossRef]
- Carslaw, K.S. Aerosols and Climate, 1st ed.; Elsevier: Amsterdam, The Netherlands, 2022; p. 854. [Google Scholar]
- Chan, M.N.; Choi, M.Y.; Ng, N.L.; Chan, C.K. Hygroscopicity of water-soluble organic compounds in atmospheric aerosols: Amino acids and biomass burning derived organic species. Environ. Sci. Technol. 2005, 39, 1555–1562. [Google Scholar] [CrossRef]
- Cheng, Y.; Su, H.; Koop, T.; Mikhailov, E.; Poschl, U. Size dependence of phase transitions in aerosol nanoparticles. Nat. Commun. 2015, 6, 1–7. [Google Scholar] [CrossRef]
- Deng, Y.; Kagami, S.; Ogawa, S.; Kawana, K.; Nakayama, T.; Kubodera, R.; Adachi, K.; Hussein, T.; Miyazaki, Y.; Mochida, M. Hygroscopicity of Organic Aerosols and Their Contributions to CCN Concentrations Over a Midlatitude Forest in Japan. J. Geophys. Res. Atmos. 2018, 123, 9703–9723. [Google Scholar] [CrossRef]
- Arigo, A.; Jawaha, N.; Nikhitha, K.; Jubie, S. Effect of Hygroscopicity on pharmaceutical ingredients, methods to determine and overcome: An Overview. J. Pharm. Sci. Res. 2019, 11, 64–70. [Google Scholar]
- Tang, M.; Chan, C.K.; Li, Y.J.; Su, H.; Ma, Q.; Wu, Z.; Zhang, G.; Wang, Z.; Ge, M.; Hu, M.; et al. A review of experimental techniques for aerosol hygroscopicity studies. Atmos. Chem. Phys. 2019, 19, 12631–12686. [Google Scholar] [CrossRef]
- Acros Organics BV—Geel, Belgium. Available online: https://www.chemeurope.com/en/companies/12696/acros-organics-bv.html (accessed on 20 January 2023).
- SNF Water Science. Available online: https://www.snf.com (accessed on 1 September 2023).
- UPL. Available online: https://www.upl-ltd.com (accessed on 1 September 2023).
- Smagin, A.V.; Budnikov, V.I.; Sadovnikova, N.B.; Kirichenko, A.V.; Belyaeva, E.A.; Krivtsova, V.N. Gel-Forming Soil Conditioners of Combined Action: Laboratory Tests for Functionality and Stability. Polymers 2022, 14, 4665. [Google Scholar] [CrossRef]
- Arthur, E.; Tuller, M.; Moldrup, P.; de Jonge, L.W. Evaluation of a Fully Automated Analyzer for Rapid Measurement of Water Vapor Sorption Isotherms for Applications in Soil Science. Soil Sci. Soc. Am. J. 2014, 78, 754–760. [Google Scholar] [CrossRef]
- Smagin, A.V. Thermogravimetric Determination of Specific Surface Area for Soil Colloids. Colloid J. 2016, 78, 391–396. [Google Scholar] [CrossRef]
- Ioelovich, M. Study of Hydrophilic Properties of Polysaccharides. Org. Polym. Mater. Res. 2021, 3, 12–23. [Google Scholar] [CrossRef]
- Li, R.; Shi, Y.; Alsaedi, M.; Wu, M.; Shi, L.; Wang, P. Hybrid hydrogel with high water vapor harvesting capacity for deployable solar-driven atmospheric water generator. Environ. Sci. Technol. 2018, 52, 11367–11377. [Google Scholar] [CrossRef] [PubMed]
- Zhao, F.; Zhou, X.; Liu, Y.; Shi, Y.; Dai, Y.; Yu, G. Super moisture-absorbent gels for all-weather atmospheric water harvesting. Adv. Mater. 2019, 31, e1806446. [Google Scholar] [CrossRef]
- Diaz-Marin, C.D.; Zhang, L.; Lu, Z.; Alshrah, M.; Grossman, J.C.; Wang, E.N. Kinetics of sorption in hygroscopic hydrogels. Nano Lett. 2022, 22, 1100–1107. [Google Scholar] [CrossRef]
- Entezari, A.; Ejeian, M.; Wang, R. Super Atmospheric Water Harvesting Hydrogel with Alginate Chains Modified with Binary Salts. ACS Mater. Lett. 2020, 2, 471–477. [Google Scholar] [CrossRef]
- Wu, M.; Li, R.; Shi, Y.; Altunkaya, M.; Aleid, S.; Zhang, C.; Wang, P. Metal and halide-free, solid-state polymeric water vapor sorbents for efficient water-sorption-driven cooling and atmospheric water harvesting. Mater. Horiz. 2021, 8, 1518–1527. [Google Scholar] [CrossRef]
- Aleid, S.; Wu, M.; Li, R.; Wang, W.; Zhang, C.; Zhang, L.; Wang, P. Salting-in effect of zwitterionic polymer hydrogel facilitates atmospheric water harvesting. ACS Mater. Lett. 2022, 4, 511–520. [Google Scholar] [CrossRef]
- Graeber, G.; Diaz-Marin, C.D.; Gaugler, L.C.; Zhong, Y.; Fil, B.E.; Liu, X.; Wang, E.N. Extreme Water Uptake of Hygroscopic Hydrogels through Maximized Swelling-Induced Salt Loading. Adv. Mater. 2023, 2211783. [Google Scholar] [CrossRef]
- Carotenuto, A.; Dell’Isola, M. An experimental verification of saturated salt solution-based humidity fixed points. Int. J. Thermophys. 1996, 1, 1423–1439. [Google Scholar] [CrossRef]
- Marestoni, L.D.; Wong, A.; Feliciano, G.T.; Marchi, M.R.R.; Tarley, C.R.T.; Sotomayor, M.D.P.T. Semi-Empirical Quantum Chemistry Method for Pre-Polymerization Rational Design of Ciprofloxacin Imprinted Polymer and Adsorption Studies. J. Braz. Chem. Soc. 2016, 27, 109–118. [Google Scholar] [CrossRef]
- Wuddivira, M.N.; Robinson, D.A.; Lebron, I.; Br´echet, L.; Atwell, M.; De Caires, S.; Oatham, M.; Jones, S.B.; Abdu, H.; Verma, A.K.; et al. Estimation of soil clay content from hygroscopic water content measurements. Soil Sci. Soc. Am. J. 2012, 76, 1529–1535. [Google Scholar] [CrossRef]
| Polymeric Materials | Composition | C% | WhS% | WhW% | pH |
|---|---|---|---|---|---|
| Biopolymers (polysaccharides): | |||||
| Starch | (C6H10O5)n 99%; ash < 0.4% | 44.1 ± 0.4 | 14 ± 2 | 12 ± 2 | 5.8 ± 0.5 |
| Cellulose microcrystalline | (C6H10O5)n 99%; ash < 0.05% | 42.0 ± 0.5 | 11 ± 2 | 9 ± 2 | 6.5 ± 0.5 |
| Synthetic composites (acrylic-based superabsorbents): | |||||
| Aquasorb | AcK, PAA *, | 39.5 ± 0.5 | 37 ± 3 | 29 ± 3 | 7.3 ± 0.1 |
| Zeba | PAA, AcK, starch | 46.6 ± 0.7 | 15 ± 2 | 11 ± 2 | 7.0 ± 0.1 |
| A11 | Ac NH4, PAA, PAA-biocatalysis waste | 45.0 ± 0.6 | 34 ± 3 | 26 ± 2 | 7.2 ± 0.1 |
| A22-Ag | AcNa, PAA, peat, silver | 47.5 ± 0.3 | 49 ± 3 | 38 ± 3 | 7.4 ± 0.1 |
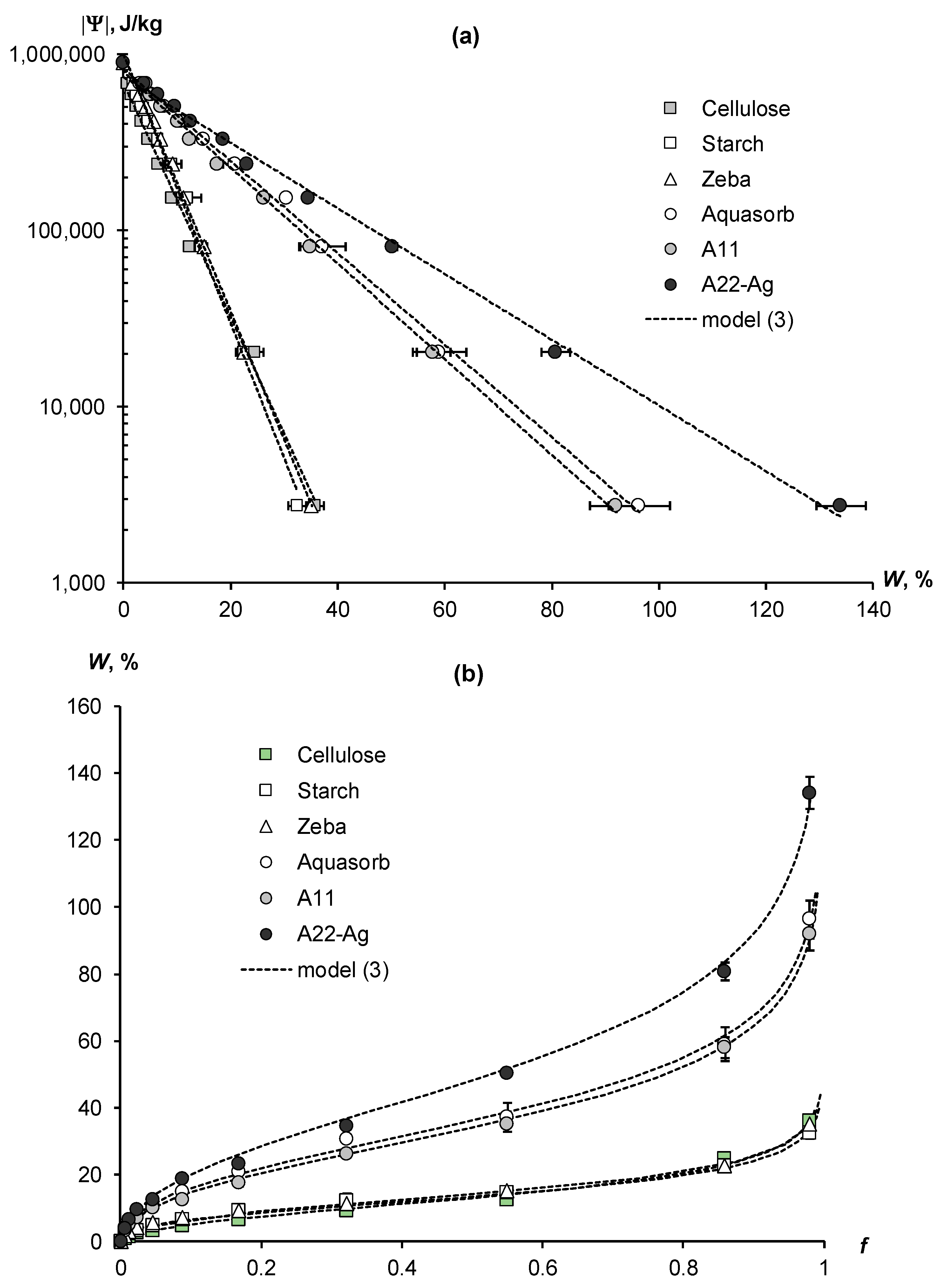
| Materials | Model (3): |Ψ| = a∙exp(–b∙W) | Model BET: W = n∙Wm∙f/{(1–f)∙(1 + (n–1)∙f} | ||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| R2 | s, [kJ/kg] | a, [kJ/kg] | b, [g/g] | R2 | s | Wm, [%] | n | S, [m2/g] | SBET, [m2/g] | |
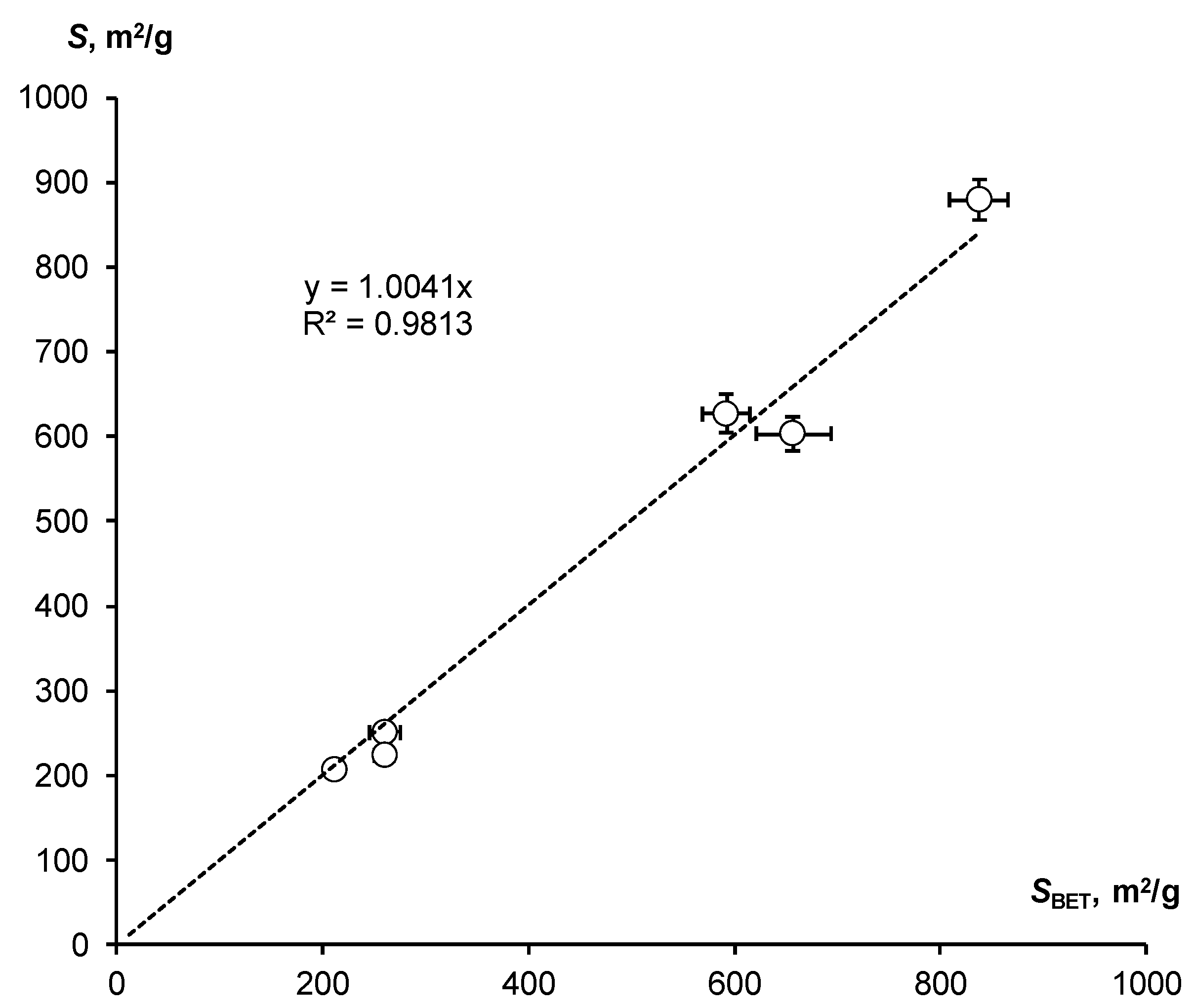
| Cellulose | 0.999 | 8.4 | 778.0 ± 8.8 | 18.2 ± 0.4 | 0.989 | 0.5 | 6.1 ± 0.2 | 26.2 ± 4.7 | 207 ± 5 | 213 ± 7 |
| Starch | 0.996 | 16.8 | 861.5 ± 22.2 | 15.0 ± 0.6 | 0.954 | 1.1 | 7.4 ± 0.4 | 39.1 ± 13.9 | 251 ± 11 | 261 ± 15 |
| Zeba | 0.998 | 15.4 | 998.5 ± 12.5 | 16.8 ± 0.4 | 0.979 | 0.7 | 7.4 ± 0.3 | 55.5 ± 13.0 | 225 ± 7 | 260 ± 10 |
| Aquasorb | 0.995 | 21.9 | 822.8 ± 16.7 | 6.0 ± 0.2 | 0.965 | 2.5 | 18.7 ± 1.0 | 30.4 ± 9.8 | 603 ± 20 | 657 ± 36 |
| A11 | 0.996 | 19.7 | 789.7 ± 16.3 | 6.3 ± 0.2 | 0.982 | 1.6 | 16.8 ± 0.7 | 30.0 ± 6.8 | 627 ± 23 | 592 ± 23 |
| A22-Ag | 0.995 | 21.8 | 742.5 ± 16.8 | 4.3 ± 0.3 | 0.995 | 1.2 | 23.8 ± 0.5 | 24.8 ± 3.0 | 879 ± 24 | 897 ± 28 |
| Pairs of Compared Methods | Tukey’s Test, p-Values | Wilcoxon Test, p-Values |
|---|---|---|
| WRC–Wh * | 0.998 | 0.790 |
| BET–Wh | 0.983 | 0.794 |
| BET–WRC | 0.993 | 0.791 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Smagin, A.V.; Sadovnikova, N.B. Hygroscopy as an Indicator of Specific Surface Area in Polymer Materials. Polymers 2024, 16, 593. https://doi.org/10.3390/polym16050593
Smagin AV, Sadovnikova NB. Hygroscopy as an Indicator of Specific Surface Area in Polymer Materials. Polymers. 2024; 16(5):593. https://doi.org/10.3390/polym16050593
Chicago/Turabian StyleSmagin, Andrey V., and Nadezhda B. Sadovnikova. 2024. "Hygroscopy as an Indicator of Specific Surface Area in Polymer Materials" Polymers 16, no. 5: 593. https://doi.org/10.3390/polym16050593
APA StyleSmagin, A. V., & Sadovnikova, N. B. (2024). Hygroscopy as an Indicator of Specific Surface Area in Polymer Materials. Polymers, 16(5), 593. https://doi.org/10.3390/polym16050593








