Numerical Investigation of Fracture Behaviour of Polyurethane Adhesives under the Influence of Moisture
Abstract
1. Introduction
2. Material Model Formulation
2.1. Finite-Strain Viscoelasticity
2.2. Phase-Field Damage
3. Governing Balance Equations
3.1. Finite Element Implementation
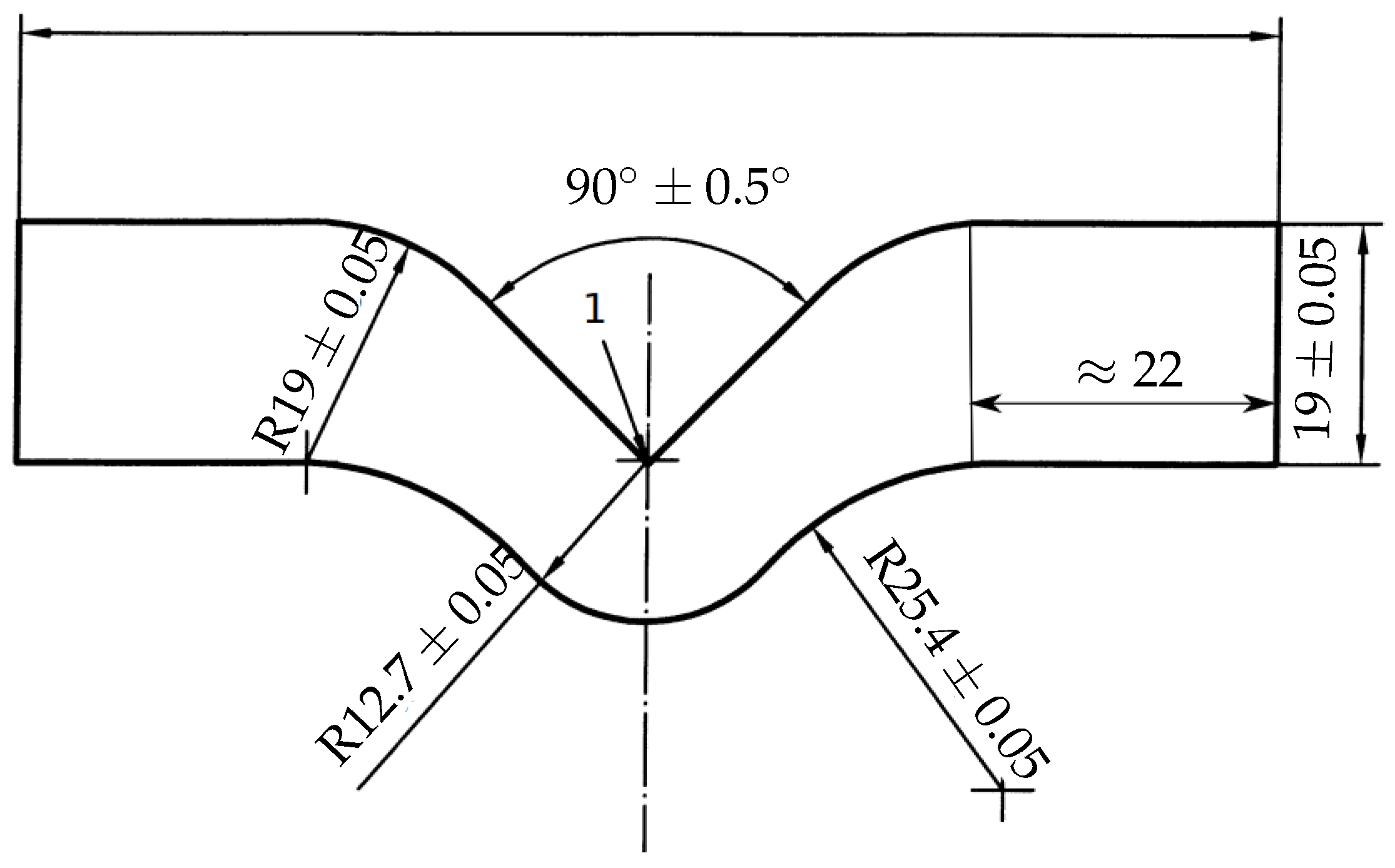
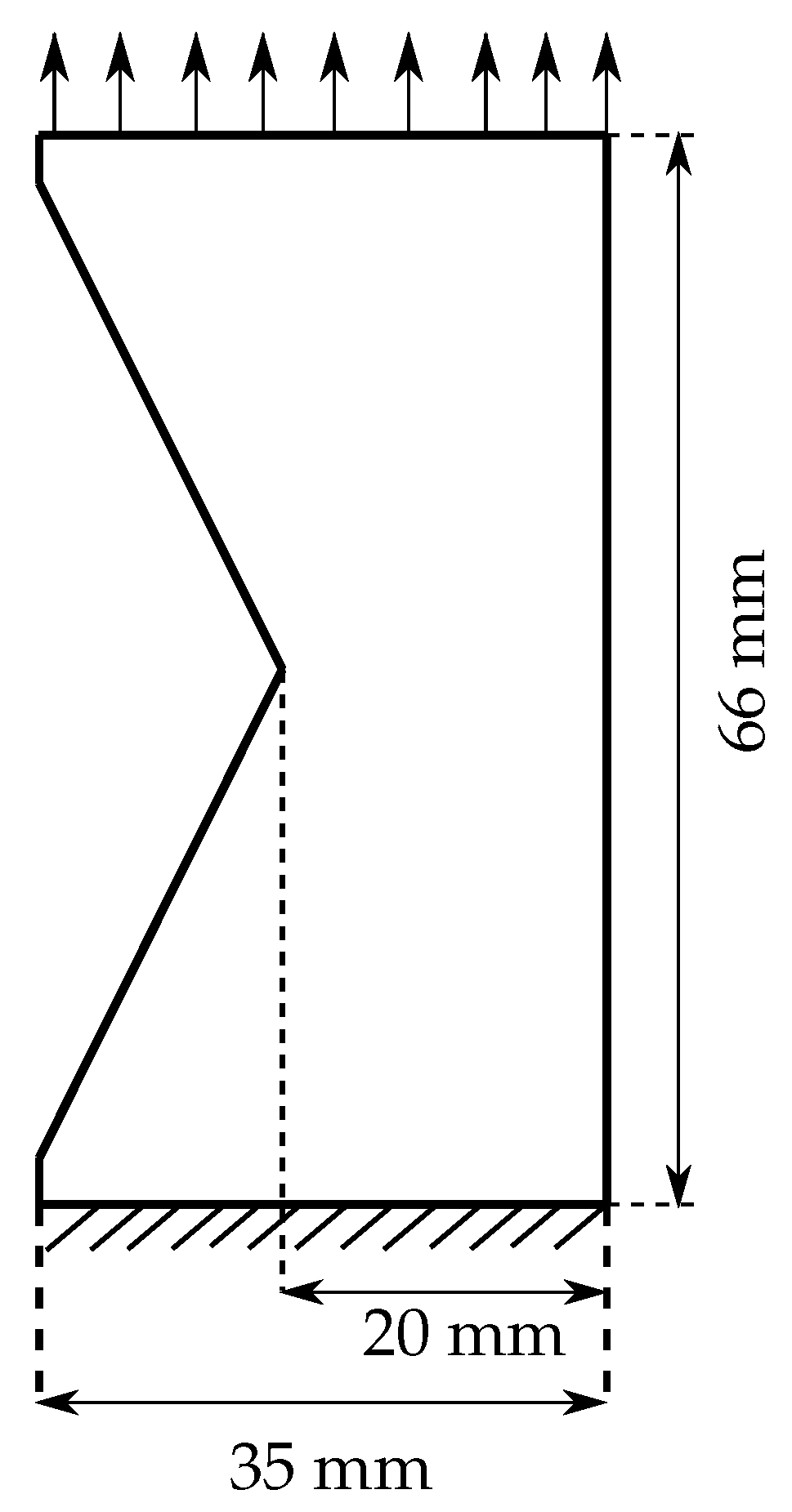
3.2. Boundary Conditions
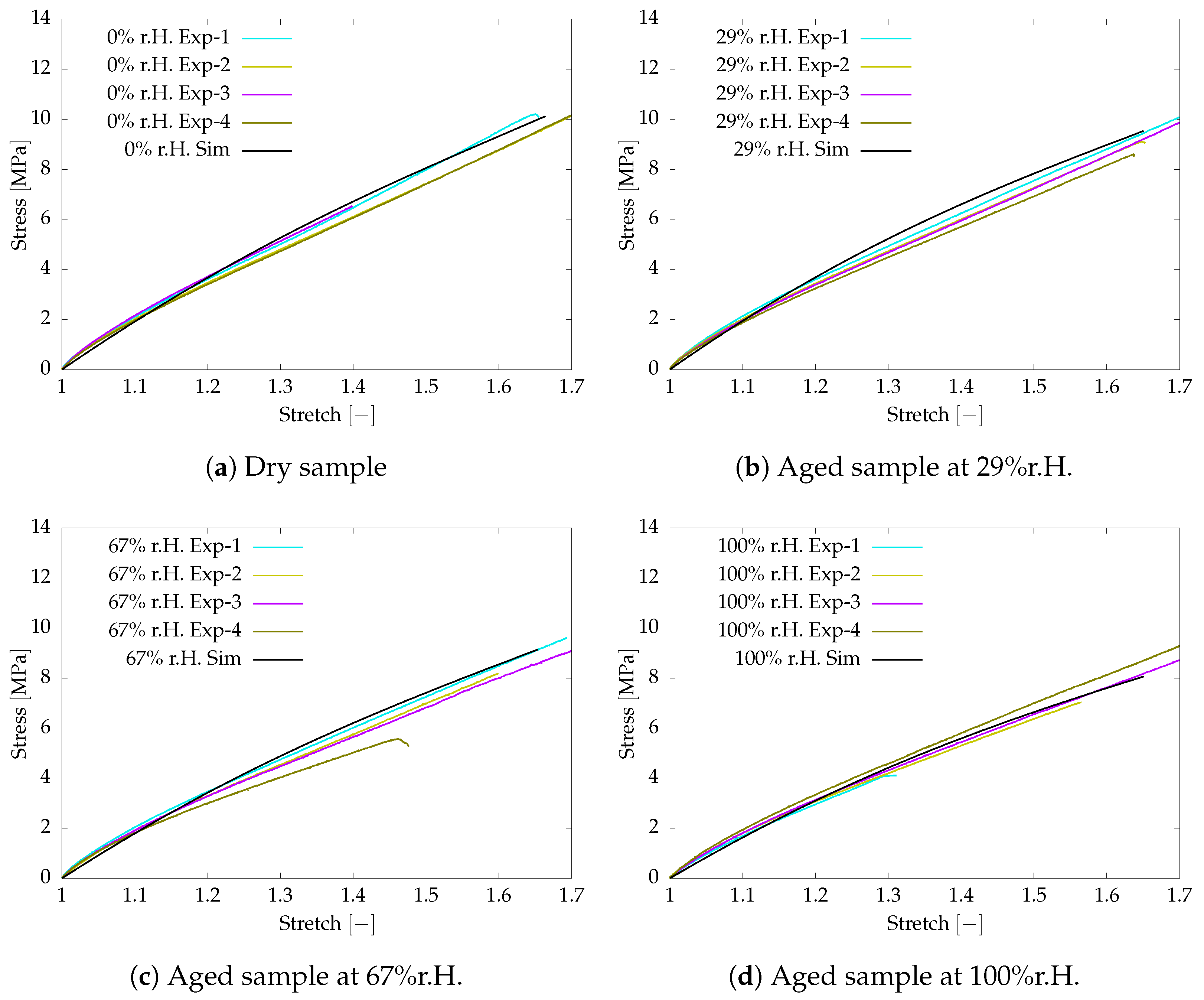
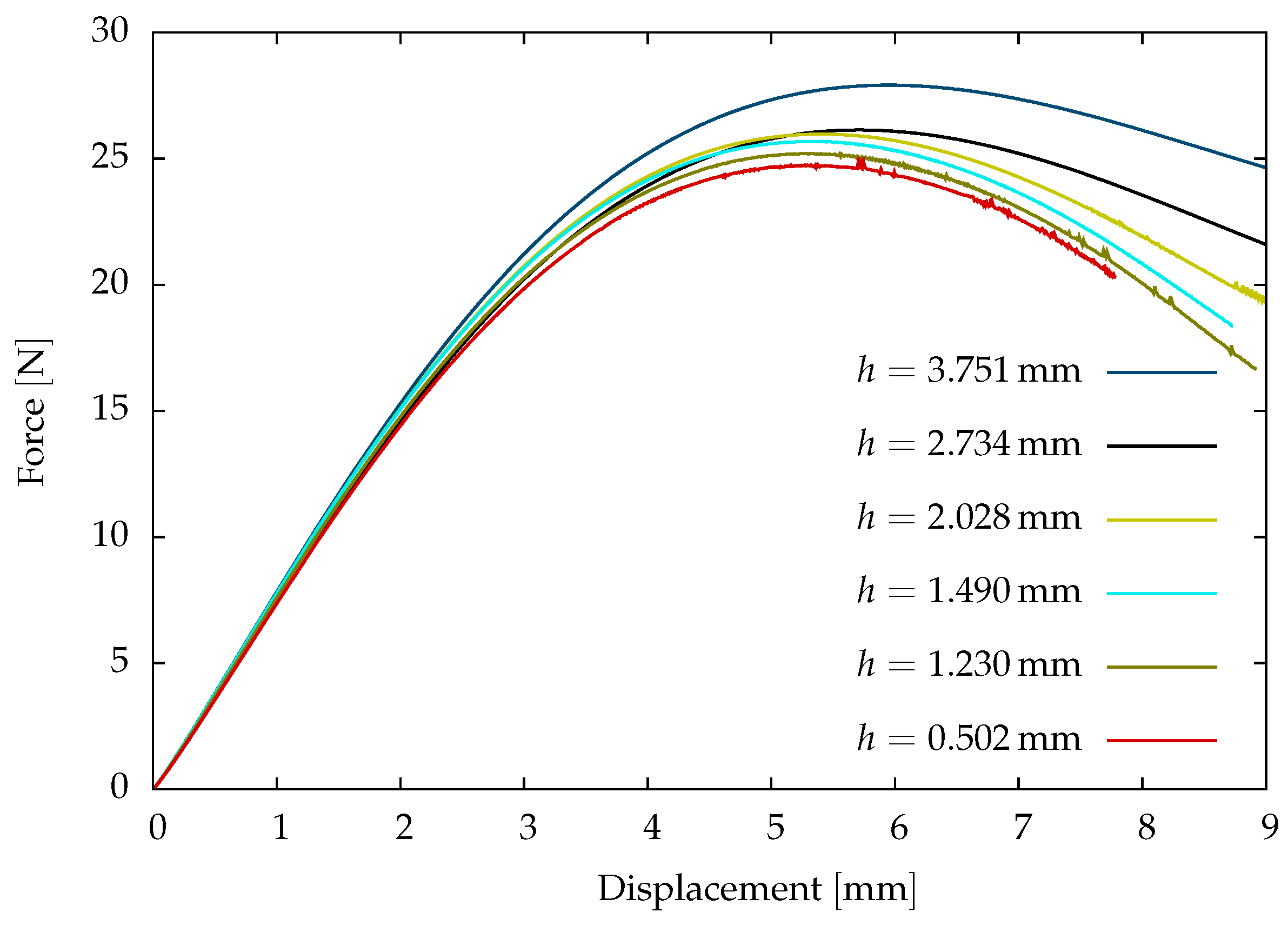
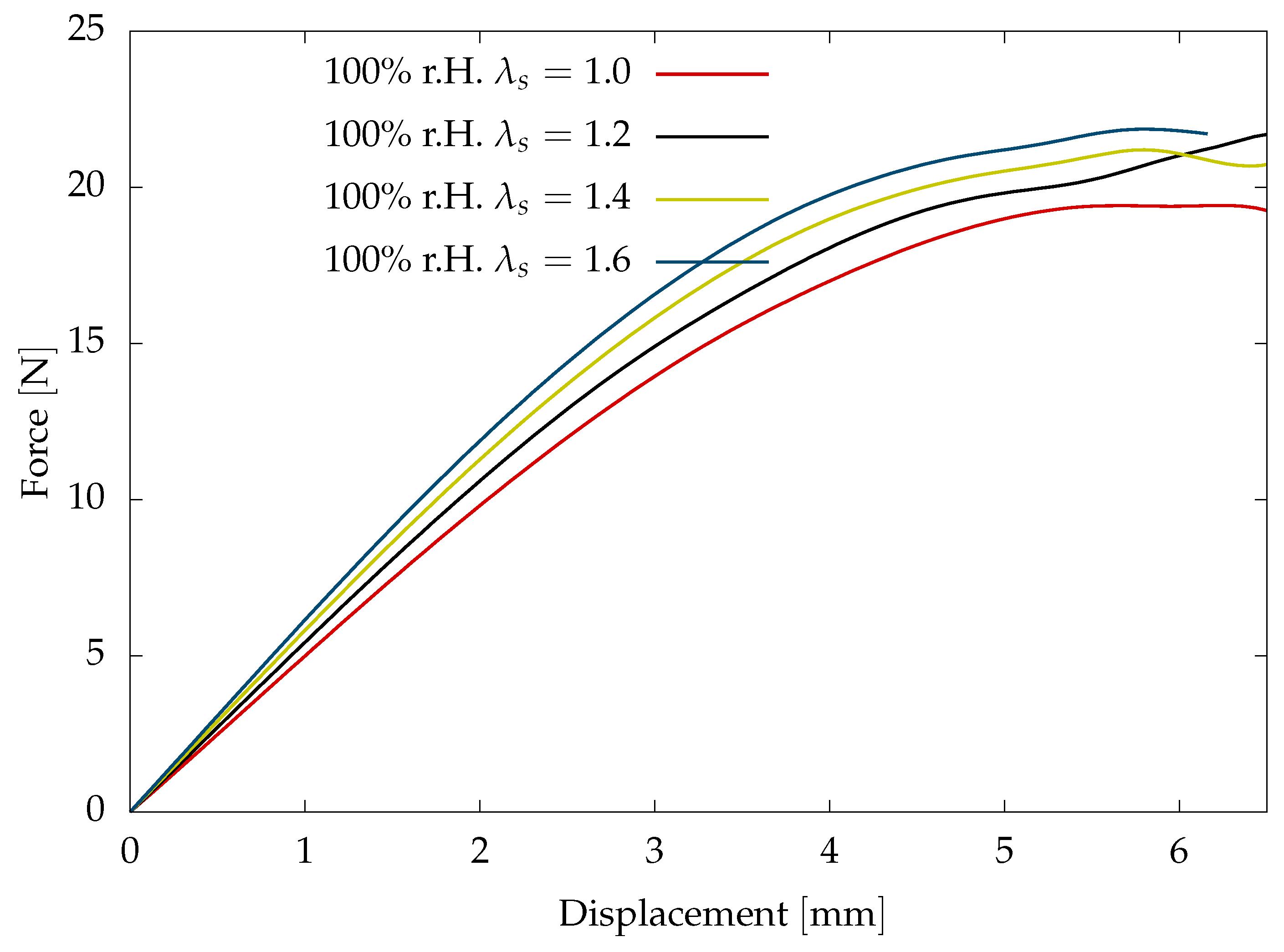
4. Results
5. Conclusions and Future Work
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A. Thermodynamic Inequality
Dissipation Inequality
References
- Das, A.; Mahanwar, P. A brief discussion on advances in polyurethane applications. Adv. Ind. Eng. Polym. Res. 2020, 3, 93–101. [Google Scholar] [CrossRef]
- Huácuja-Sanchez, J.; Müller, K.; Possart, W. Water diffusion in a crosslinked polyether-based polyurethane adhesive. Int. J. Adhes. Adhes. 2016, 66, 167–175. [Google Scholar] [CrossRef]
- Smith, T.L.; Magnusson, A.B. Diisocyanate-linked polymers. II. Mechanical and swelling properties of some polyurethane elastomers. J. Polym. Sci. 1960, 42, 391–416. [Google Scholar] [CrossRef]
- Shibayama, K.; Kodama, M. Effects of concentration of urethane linkage, crosslinking density, and swelling upon the viscoelastic properties of polyurethanes. J. Polym. Sci. Part A-1 Polym. Chem. 1966, 4, 83–108. [Google Scholar] [CrossRef]
- Ilavský, M.; Ulmer, H.; te Nijenhuis, K.; Mijs, W. Effect of charge concentration on dynamic mechanical, extraction and swelling behaviour of polyurethane networks based on triisocyanate and diethanolamine derivatives. Polym. Gels Netw. 1994, 2, 73–87. [Google Scholar] [CrossRef]
- Krauklis, A.E.; Karl, C.W.; Rocha, I.B.C.M.; Burlakovs, J.; Ozola-Davidane, R.; Gagani, A.I.; Starkova, O. Modelling of Environmental Ageing of Polymers and Polymer Composites—Modular and Multiscale Methods. Polymers 2022, 14, 216. [Google Scholar] [CrossRef]
- Hong, W.; Zhao, X.; Zhou, J.; Suo, Z. A theory of coupled diffusion and large deformation in polymeric gels. J. Mech. Phys. Solids 2008, 56, 1779–1793. [Google Scholar] [CrossRef]
- Wang, D.; Wu, M. Analytical solutions for bilayered spherical hydrogel subjected to constant dilatation. Mech. Mater. 2013, 58, 12–22. [Google Scholar] [CrossRef]
- Guo, J.; Luo, J.; Xiao, Z. On the opening profile and near tip fields of an interface crack between a polymeric hydrogel and a rigid substrate. Eng. Fract. Mech. 2016, 153, 91–102. [Google Scholar] [CrossRef]
- Dolbow, J.; Fried, E.; Ji, H. Chemically induced swelling of hydrogels. J. Mech. Phys. Solids 2004, 52, 51–84. [Google Scholar] [CrossRef]
- Liu, Y.; Zhang, H.; Zheng, Y. A multiplicative finite element algorithm for the inhomogeneous swelling of polymeric gels. Comput. Methods Appl. Mech. Eng. 2015, 283, 517–550. [Google Scholar] [CrossRef]
- Chester, S.A.; Anand, L. A coupled theory of fluid permeation and large deformations for elastomeric materials. J. Mech. Phys. Solids 2010, 58, 1879–1906. [Google Scholar] [CrossRef]
- Haupt, P.; Lion, A. On finite linear viscoelasticity of incompressible isotropic materials. Acta Mech. 2002, 159, 87–124. [Google Scholar] [CrossRef]
- Mooney, M. A Theory of Large Elastic Deformation. J. Appl. Phys. 1940, 11, 582–592. [Google Scholar] [CrossRef]
- Rivlin, R.S.; Rideal, E.K. Large elastic deformations of isotropic materials IV. further developments of the general theory. Philos. Trans. R. Soc. Lond. Ser. A Math. Phys. Sci. 1948, 241, 379–397. [Google Scholar]
- Ogden, R.W.; Hill, R. Large deformation isotropic elasticity-on the correlation of theory and experiment for incompressible rubberlike solids. Proc. R. Soc. Lond. A Math. Phys. Sci. 1972, 326, 565–584. [Google Scholar] [CrossRef]
- Treloar, L.R.G.; Hopkins, H.G.; Rivlin, R.S.; Ball, J.M.; Gee, G.; Allen, G.; Price, C. The mechanics of rubber elasticity. Proc. R. Soc. Lond. A Math. Phys. Sci. 1976, 351, 301–330. [Google Scholar] [CrossRef]
- Kuhn, W. Beziehungen zwischen Molekülgröße, statistischer Molekülgestalt und elastischen Eigenschaften hochpolymerer Stoffe. Kolloid-Zeitschrift 1936, 76, 258–271. [Google Scholar] [CrossRef]
- Kuhn, W.; Grün, F. Beziehungen zwischen elastischen Konstanten und Dehnungsdoppelbrechung hochelastischer Stoffe. Kolloid-Zeitschrift 1942, 101, 248–271. [Google Scholar] [CrossRef]
- Arruda, E.M.; Boyce, M.C. A three-dimensional constitutive model for the large stretch behavior of rubber elastic materials. J. Mech. Phys. Solids 1993, 41, 389–412. [Google Scholar] [CrossRef]
- Miehe, C.; Göktepe, S.; Lulei, F. A micro-macro approach to rubber-like materials-Part I: The non-affine micro-sphere model of rubber elasticity. J. Mech. Phys. Solids 2004, 52, 2617–2660. [Google Scholar] [CrossRef]
- Miehe, C.; Göktepe, S. A micro–macro approach to rubber-like materials. Part II: The micro-sphere model of finite rubber viscoelasticity. J. Mech. Phys. Solids 2005, 53, 2231–2258. [Google Scholar] [CrossRef]
- Lubliner, J. A model of rubber viscoelasticity. Mech. Res. Commun. 1985, 12, 93–99. [Google Scholar] [CrossRef]
- Hartmann, S. Computation in finite-strain viscoelasticity: Finite elements based on the interpretation as differential–algebraic equations. Comput. Methods Appl. Mech. Eng. 2002, 191, 1439–1470. [Google Scholar] [CrossRef]
- Grellmann, W.; Langer, B. (Eds.) Deformation and Fracture Behaviour of Polymer Materials, 1st ed.; Springer International Publishing: Cham, Switzerland, 2017; Volume 247. [Google Scholar]
- Williams, J.G. Fracture Mechanics of Polymers and Adhesives. In Fracture of Non-Metallic Materials: Proceeding of the 5th Advanced Seminar on Fracture Mechanics, Joint Research Centre, Ispra, Italy, 14–18 October 1985; Herrmann, K.P., Larsson, L.H., Eds.; Springer: Dordrecht, The Netherlands, 1987; pp. 227–255. [Google Scholar] [CrossRef]
- Krajcinovic, D.; Lemaitre, J. (Eds.) Continuum Damage Mechanics Theory and Application; Springer: Wien, Austria, 1987. [Google Scholar]
- Navidtehrani, Y.; Betegón, C.; Martínez-Pañeda, E. A Unified Abaqus Implementation of the Phase Field Fracture Method Using Only a User Material Subroutine. Materials 2021, 14, 1913. [Google Scholar] [CrossRef]
- Ambati, M.; Gerasimov, T.; De Lorenzis, L. Phase-field modeling of ductile fracture. Mech. Res. Commun. 2015, 55, 1017–1040. [Google Scholar] [CrossRef]
- Karma, A.; Kessler, D.A.; Levine, H. Phase-Field Model of Mode III Dynamic Fracture. Phys. Rev. Lett. 2001, 87, 045501. [Google Scholar] [CrossRef]
- Bourdin, B.; Francfort, G.A.; Marigo, J.J. The Variational Approach to Fracture. J. Elast. 2008, 91, 5–148. [Google Scholar] [CrossRef]
- Kuhn, C.; Müller, R. A continuum phase field model for fracture. Eng. Fract. Mech. 2010, 77, 3625–3634. [Google Scholar] [CrossRef]
- Miehe, C.; Welschinger, F.; Hofacker, M. Thermodynamically consistent phase-field models of fracture: Variational principles and multi-field FE implementations. Int. J. Numer. Methods Eng. 2010, 83, 1273–1311. [Google Scholar] [CrossRef]
- Hofacker, M.; Miehe, C. A Phase Field Model for Ductile to Brittle Failure Mode Transition. Pamm 2012, 12, 173–174. [Google Scholar] [CrossRef]
- Duda, F.P.; Ciarbonetti, A.; Sánchez, P.J.; Huespe, A.E. A phase-field/gradient damage model for brittle fracture in elastic–plastic solids. Int. J. Plast. 2015, 65, 269–296. [Google Scholar] [CrossRef]
- Ambati, M.; De Lorenzis, L. Phase-field modeling of brittle and ductile fracture in shells with isogeometric NURBS-based solid-shell elements. Comput. Methods Appl. Mech. Eng. 2016, 312, 351–373. [Google Scholar] [CrossRef]
- Miehe, C.; Hofacker, M.; Schänzel, L.M.; Aldakheel, F. Phase field modeling of fracture in multi-physics problems. Part II. Coupled brittle-to-ductile failure criteria and crack propagation in thermo-elastic–plastic solids. Comput. Methods Appl. Mech. Eng. 2015, 294, 486–522. [Google Scholar] [CrossRef]
- Miehe, C.; Schänzel, L.M.; Ulmer, H. Phase field modeling of fracture in multi-physics problems. Part I. Balance of crack surface and failure criteria for brittle crack propagation in thermo-elastic solids. Comput. Methods Appl. Mech. Eng. 2015, 294, 449–485. [Google Scholar] [CrossRef]
- Spatschek, R.; Müller-Gugenberger, C.; Brener, E.; Nestler, B. Phase field modeling of fracture and stress-induced phase transitions. Phys. Rev. E 2007, 75, 066111. [Google Scholar] [CrossRef]
- Hossain, M.; Hsueh, C.J.; Bourdin, B.; Bhattacharya, K. Effective toughness of heterogeneous media. J. Mech. Phys. Solids 2014, 71, 15–32. [Google Scholar] [CrossRef]
- Gurtin, M.E. Generalized Ginzburg-Landau and Cahn-Hilliard equations based on a microforce balance. Physica D Nonlinear Phenom. 1996, 92, 178–192. [Google Scholar] [CrossRef]
- Lee, E.H. Elastic-Plastic Deformation at Finite Strains. J. Appl. Mech. 1969, 36, 1–6. [Google Scholar] [CrossRef]
- Lee, E.; Liu, D. Finite Strain Elastic Plastic Theory with Application to Plane Wave Analysis. J. Appl. Phys. 1967, 38, 19–27. [Google Scholar] [CrossRef]
- Govindjee, S.; Reese, S. A Presentation and Comparison of Two Large Deformation Viscoelasticity Models. J. Eng. Mater. Technol. 1997, 119, 251–255. [Google Scholar] [CrossRef]
- Lion, A. A physically based method to represent the thermo-mechanical behaviour of elastomers. Acta Mech. 1997, 123, 1–25. [Google Scholar] [CrossRef]
- Reese, S.; Govindjee, S. A theory of finite viscoelasticity and numerical aspects. Int. J. Solids Struct. 1998, 35, 3455–3482. [Google Scholar] [CrossRef]
- Tallec, P.; Rahier, C.; Kaiss, A. Three-dimensional incompressible viscoelasticity in large strains: Formulation and numerical approximation. Comput. Methods Appl. Mech. Eng. 1993, 109, 233–258. [Google Scholar] [CrossRef]
- Lubarda, V.A. Constitutive theories based on the multiplicative decomposition of deformation gradient: Thermoelasticity, elastoplasticity, and biomechanics. Appl. Mech. Rev. 2004, 57, 95–108. [Google Scholar] [CrossRef]
- Haupt, P. Continuum Mechanics and Theory of Materials; Springer: Berlin/Heidelberg, Germany, 2002. [Google Scholar] [CrossRef]
- Lion, A. Thermomechanik von Elastomeren; Institut für Mechanik, Universität Kassel: Kassel, Germany, 2000. [Google Scholar]
- Seldan, K. Viskoelastisches Materialverhalten von Elastomerwerkstoffen: Experimentelle Untersuchung und Modellbildung; Universität Gesamthochschule Kassel: Kassel, Germany, 2001. [Google Scholar]
- Simo, J. On a fully three-dimensional finite-strain viscoelastic damage model: Formulation and computational aspects. Comput. Methods Appl. Mech. Eng. 1987, 60, 153–173. [Google Scholar] [CrossRef]
- Yeoh, O.H. Characterization of Elastic Properties of Carbon-Black-Filled Rubber Vulcanizates. Rubber Chem. Technol. 1990, 63, 792–805. [Google Scholar] [CrossRef]
- Yeoh, O.H. Some Forms of the Strain Energy Function for Rubber. Rubber Chem. Technol. 1993, 66, 754–771. [Google Scholar] [CrossRef]
- Holzapfel, G.A. Nonlinear Solid Mechanics: A Continuum Approach for Engineering; Wiley: Hoboken, NJ, USA, 2000. [Google Scholar]
- Brink, U.; Stein, E. On some mixed finite element methods for incompressible and nearly incompressible finite elasticity. Rubber Chem. Technol. 1996, 19, 105–119. [Google Scholar] [CrossRef]
- Oden, J. A theory of penalty methods for finite element approximations of highly nonlinear problems in continuum mechanics. Comput. Struct. 1978, 8, 445–449. [Google Scholar] [CrossRef]
- Ogden, R.W.; Sternberg, E. Nonlinear Elastic Deformations. J. Appl. Mech. 1985, 52, 740–741. [Google Scholar] [CrossRef]
- Francfort, G.; Marigo, J.J. Revisiting brittle fracture as an energy minimization problem. J. Mech. Phys. Solids 1998, 46, 1319–1342. [Google Scholar] [CrossRef]
- Griffith, A.A. The Phenomena of Rupture and Flow in Solids. Philos. Trans. R. Soc. Lond. Ser. A Contain. Pap. A Math. Phys. Character 1921, 221, 163–198. [Google Scholar]
- Bourdin, B.; Francfort, G.; Marigo, J.J. Numerical experiments in revisited brittle fracture. J. Mech. Phys. Solids 2000, 48, 797–826. [Google Scholar] [CrossRef]
- Heister, T.; Wheeler, M.F.; Wick, T. A primal-dual active set method and predictor-corrector mesh adaptivity for computing fracture propagation using a phase-field approach. Comput. Methods Appl. Mech. Eng. 2015, 290, 466–495. [Google Scholar] [CrossRef]
- Kuhn, C.; Schlüter, A.; Müller, R. On degradation functions in phase field fracture models. Comput. Mater. Sci. 2015, 108, 374–384. [Google Scholar] [CrossRef]
- Borden, M.J.; Verhoosel, C.V.; Scott, M.A.; Hughes, T.J.; Landis, C.M. A phase-field description of dynamic brittle fracture. Comput. Methods Appl. Mech. Eng. 2012, 217–220, 77–95. [Google Scholar] [CrossRef]
- Braides, A. Approximation of Free-Discontinuity Problems; Springer: Berlin/Heidelberg, Germany, 2000. [Google Scholar]
- Zimmer, B.; Nies, C.; Schmitt, C.; Paulo, C.; Possart, W. Chemistry, polymer dynamics and mechanical properties of a two-part polyurethane elastomer during and after crosslinking. Part II: Moist conditions. Polymer 2018, 149, 238–252. [Google Scholar] [CrossRef]
- Wulf, A.; Hesebeck, O.; Koschek, K.; Brede, M.; Mayer, B.; Josyula, S.; Diebels, S.; Zimmer, B.; Possart, W. Berechnung des instationären mechanischen Verhaltens von alternden Klebverbindungen unter Einfluss von Wasser auf den Klebstoff. DVS Forschungsvereinigung 2021, 469, 1–266. [Google Scholar]
- Goldschmidt, F.; Diebels, S.; Scherff, F.; Depollier, L.; Huacuja-Sanchez, J.E.; Possart, W. Viscoelasticity in Ageing Joints–Experiments and Simulation. In Adhesive Joints; John Wiley & Sons, Ltd.: Hoboken, NJ, USA, 2018; Chapter B.6; pp. 355–374. [Google Scholar]
- Johlitz, M.; Diebels, S.; Possart, W. Investigation of the thermoviscoelastic material behaviour of adhesive bonds close to the glass transition temperature. Arch. Appl. Mech. 2012, 82, 1089–1102. [Google Scholar] [CrossRef]
- Nelder, J.A.; Mead, R. A simplex method for function minimization. Comput. J. 1965, 7, 308–313. [Google Scholar] [CrossRef]
- Fried, E.; Gurtin, M.E. Continuum theory of thermally induced phase transitions based on an order parameter. Physica D Nonlinear Phenom. 1993, 68, 326–343. [Google Scholar] [CrossRef]
- Fried, E.; Gurtin, M.E. Dynamic Solid-Solid Transitions with Phase Characterized by an Order Parameter. Physica D 1994, 72, 287–308. [Google Scholar] [CrossRef]

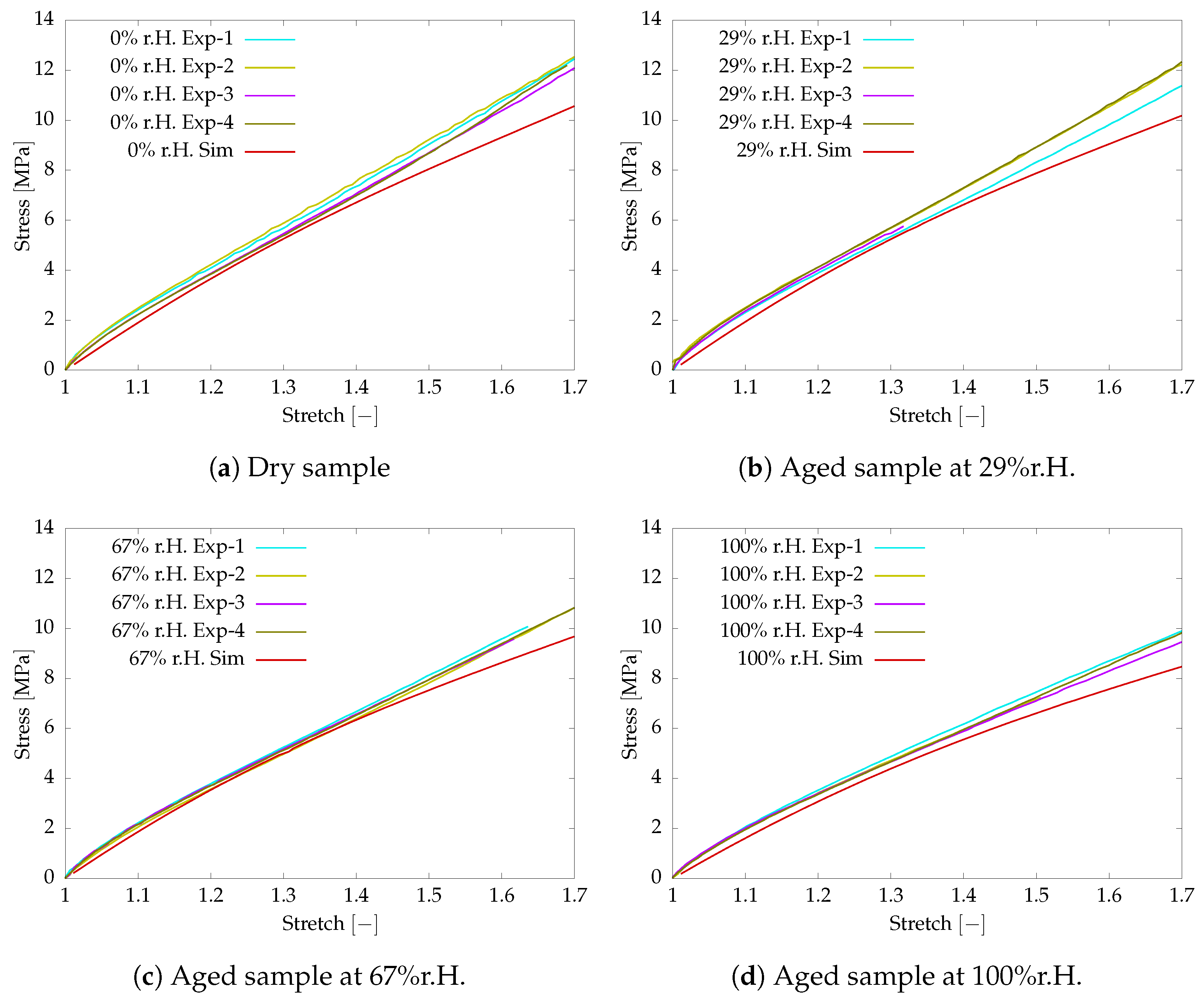



| Material Parameters of Finite-Strain Viscoelastic Model | ||||||
|---|---|---|---|---|---|---|
| Relaxation Times [s] | 0% r.H. | 29% r.H. | 67% r.H. | 100% r.H. | ||
| Equilibrium | 9.886 | 7.886 | 7.196 | 7.072 | ||
| −1.414 | −1.357 | −1.122 | −1.128 | |||
| 3.214 | 1.443 | 0.918 | 0.872 | |||
| 0.306 | 0.244 | 0.241 | 0.314 | |||
| Non-equilibrium | 0.5 | 4.886 | 2.886 | 2.296 | 2.172 | |
| 10 | 0.886 | 0.231 | 0.139 | 0.107 | ||
| 100 | 0.055 | 0.017 | 0.014 | 0.011 | ||
| 1000 | 0.005 | 0.003 | 0.002 | 0.001 | ||
| Identified Critical Energy Release Rate | ||||
|---|---|---|---|---|
| ageing condition | r.H. | r.H. | r.H. | r.H. |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Josyula, S.P.; Diebels, S. Numerical Investigation of Fracture Behaviour of Polyurethane Adhesives under the Influence of Moisture. Polymers 2024, 16, 2676. https://doi.org/10.3390/polym16182676
Josyula SP, Diebels S. Numerical Investigation of Fracture Behaviour of Polyurethane Adhesives under the Influence of Moisture. Polymers. 2024; 16(18):2676. https://doi.org/10.3390/polym16182676
Chicago/Turabian StyleJosyula, Siva Pavan, and Stefan Diebels. 2024. "Numerical Investigation of Fracture Behaviour of Polyurethane Adhesives under the Influence of Moisture" Polymers 16, no. 18: 2676. https://doi.org/10.3390/polym16182676
APA StyleJosyula, S. P., & Diebels, S. (2024). Numerical Investigation of Fracture Behaviour of Polyurethane Adhesives under the Influence of Moisture. Polymers, 16(18), 2676. https://doi.org/10.3390/polym16182676






