The High Pressure Behavior of Galenobismutite, PbBi2S4: A Synchrotron Single Crystal X-ray Diffraction Study
Abstract
1. Introduction
2. High Pressure Experiments
3. Results
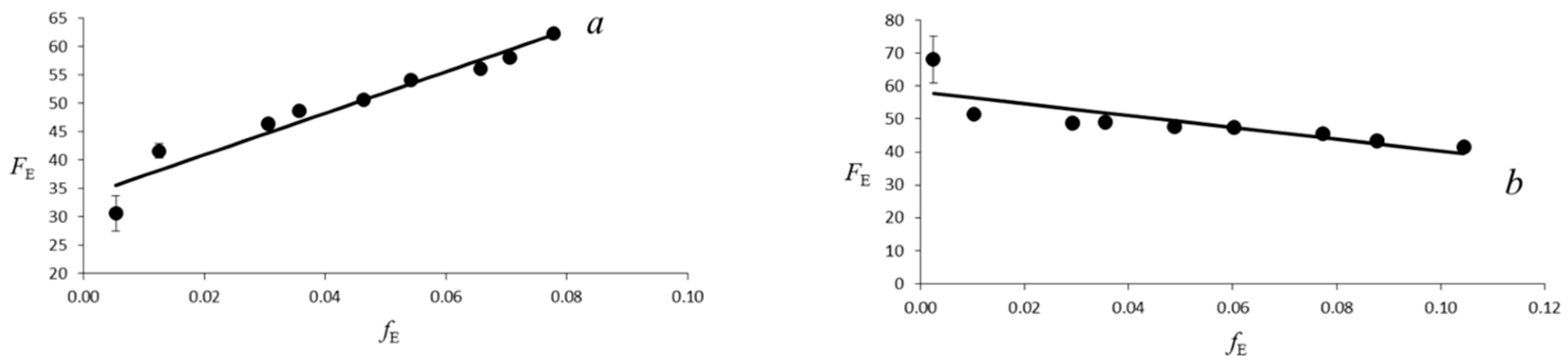
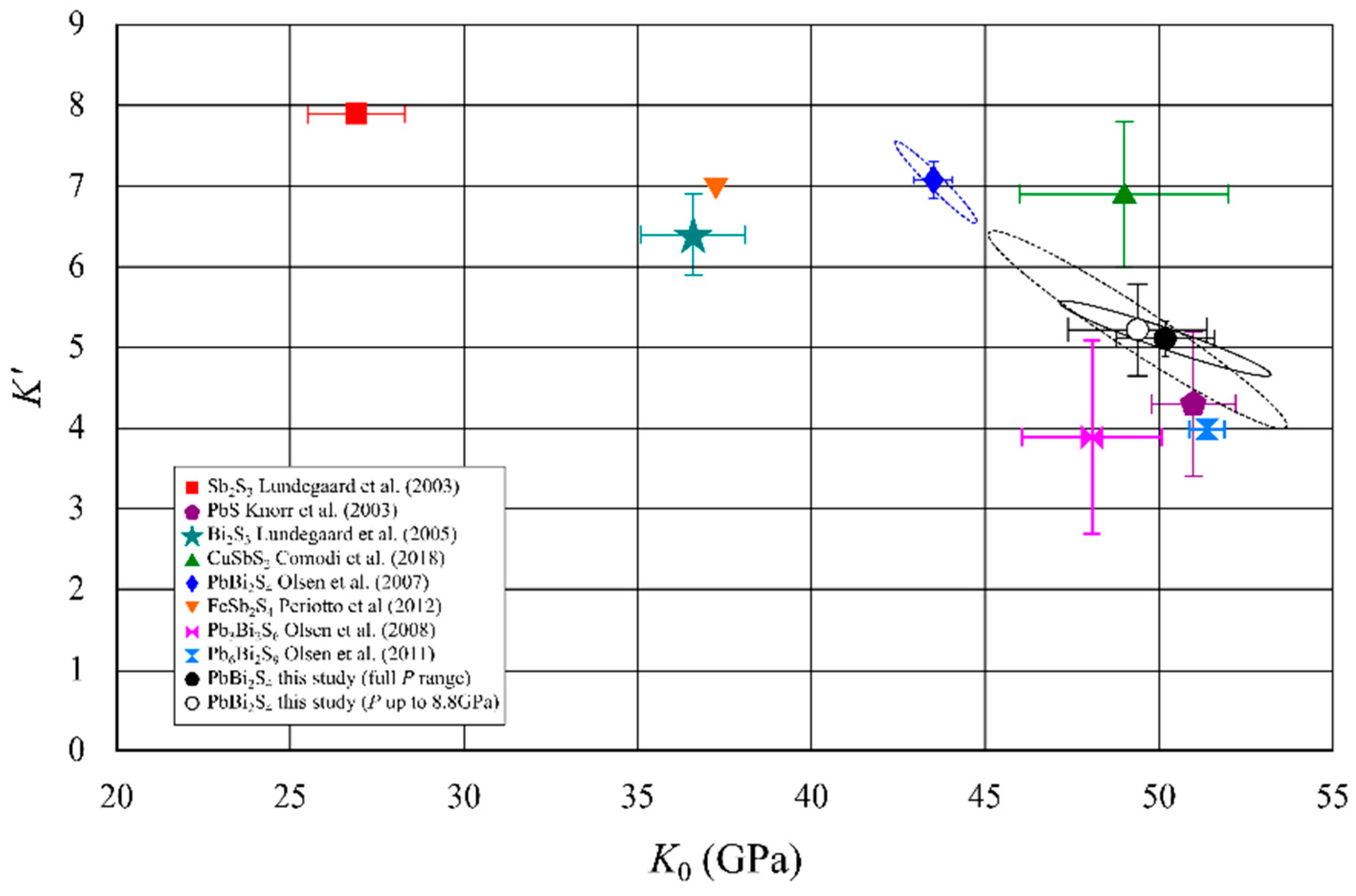
3.1. Compressibility
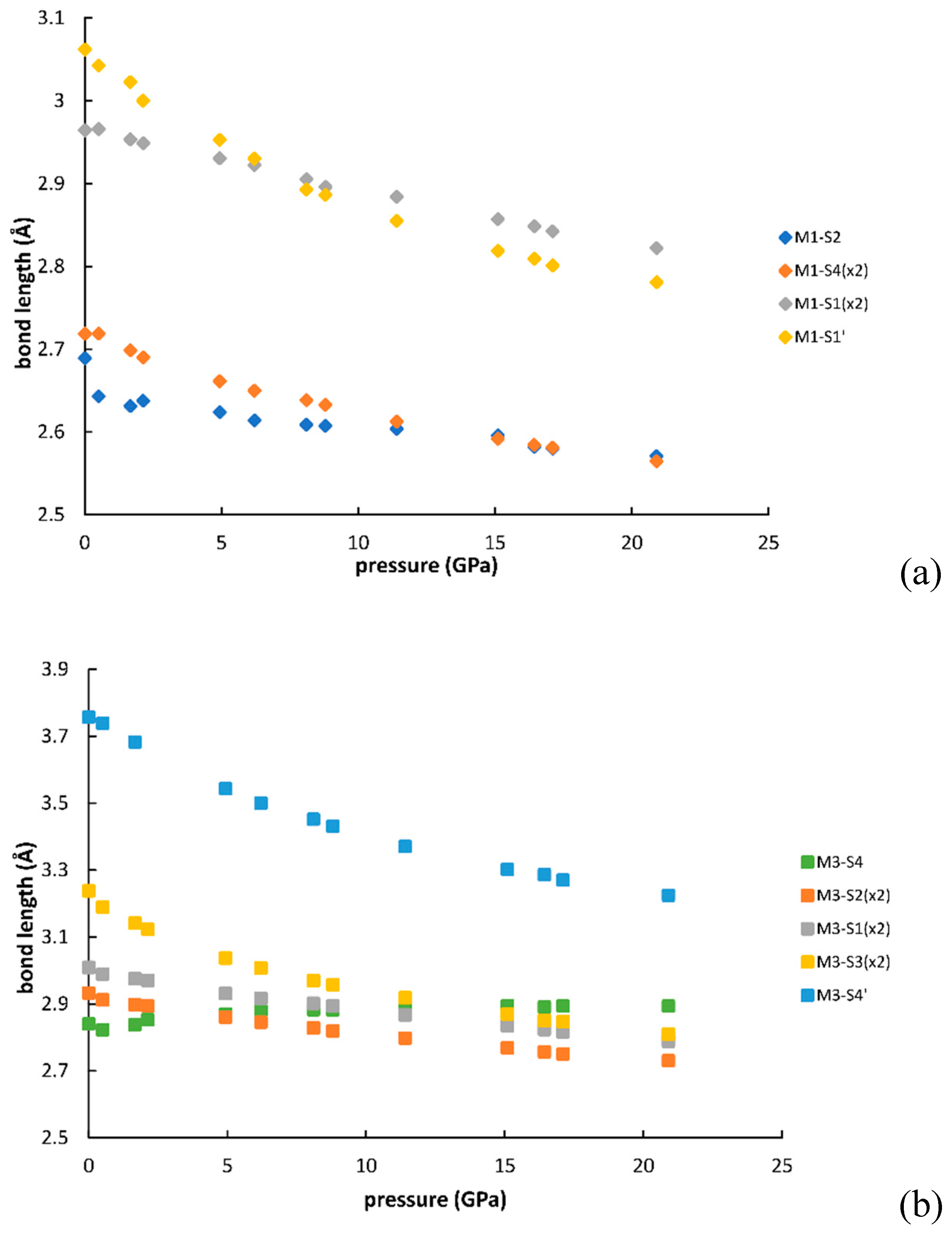
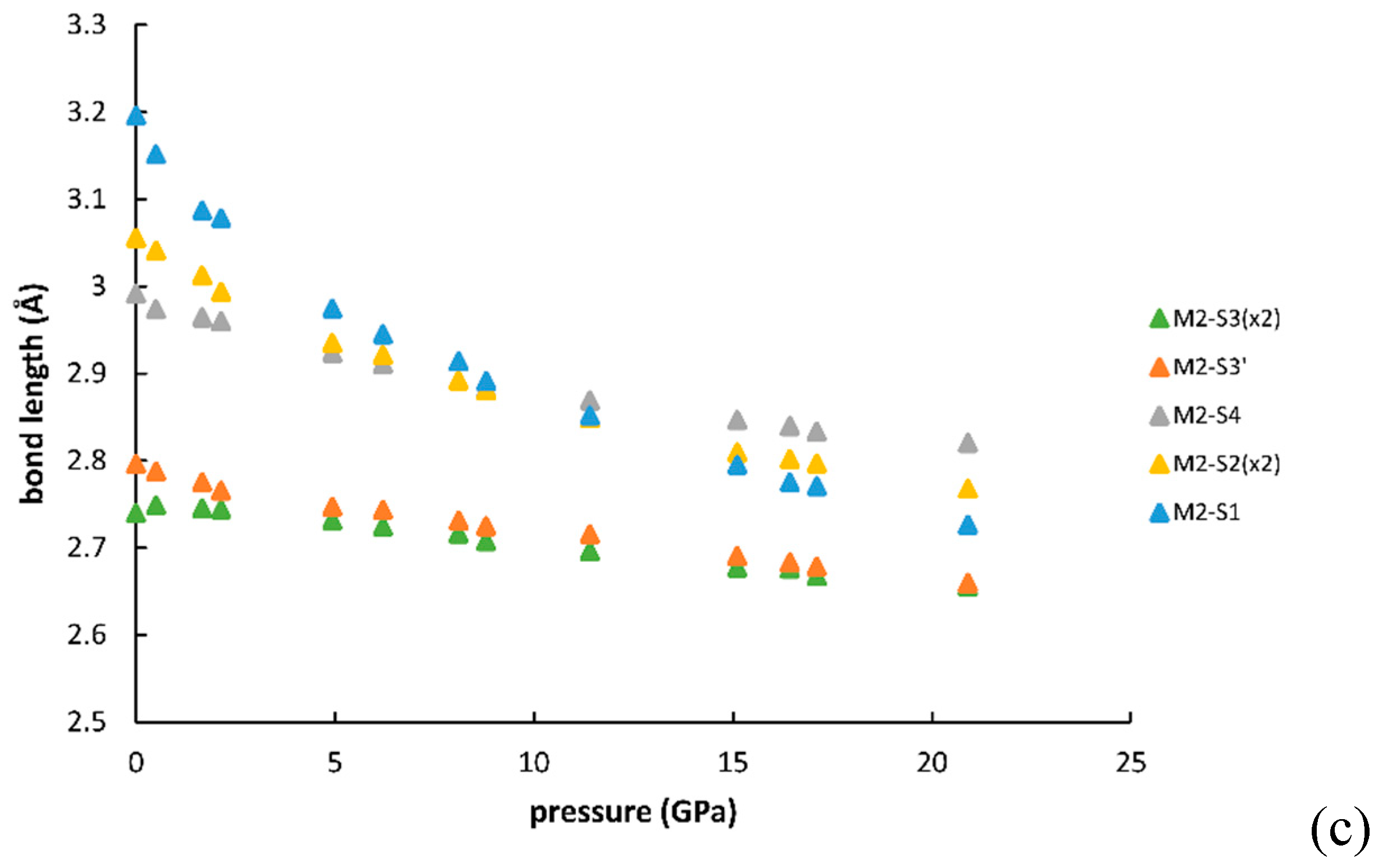
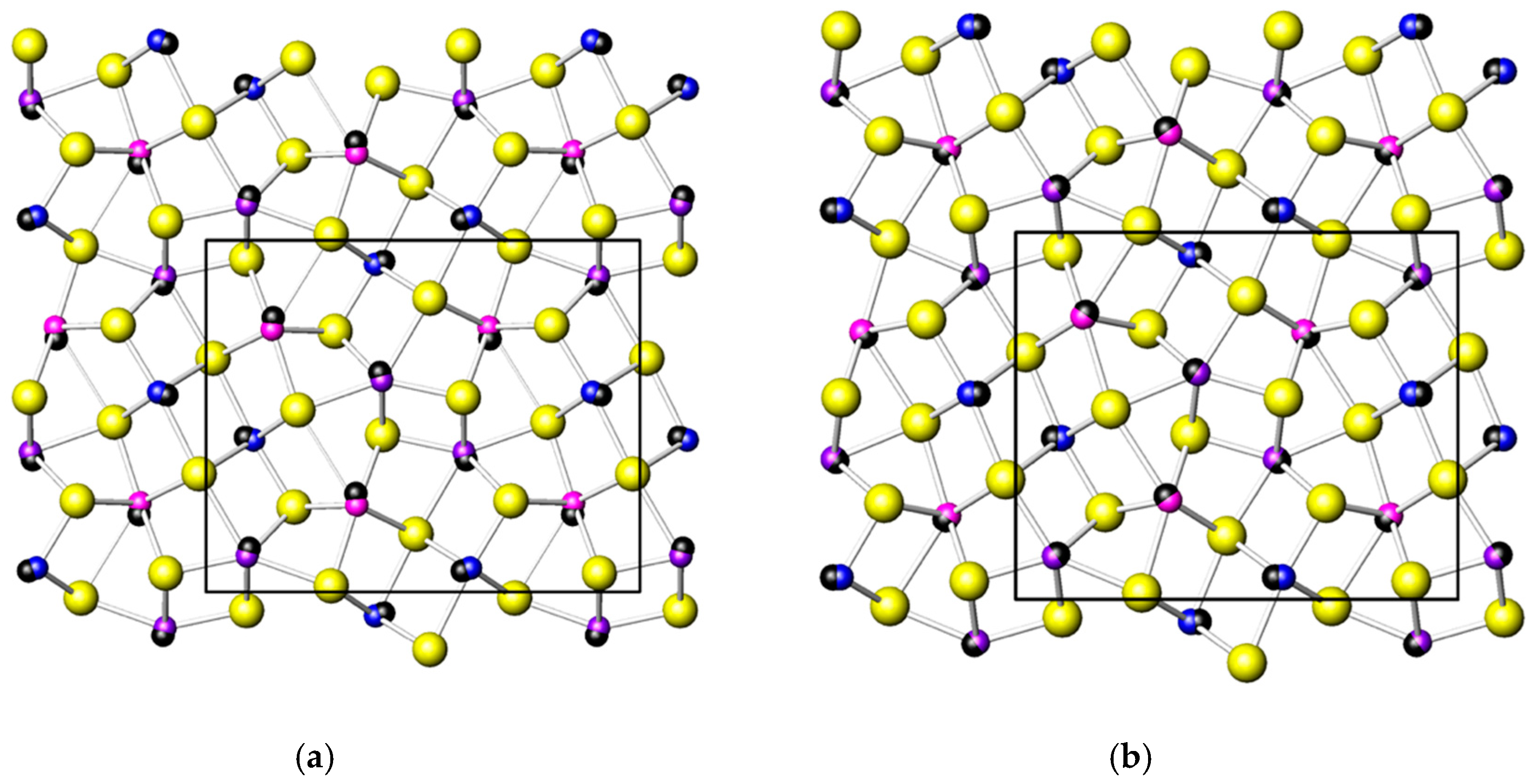
3.2. Structural Evolution with Pressure
4. Discussion and Conclusion
- (a)
- The structural evolution is completely reversible with pressure increase up to 20.9 GPa. The same values were measured increasing and decreasing the pressure and the same equation of state is measured by using values collected increasing or decreasing pressure. No evidence of hysteresis in the changes were observed, meaning that the changes are completely elastic.
- (b)
- The change in atomic coordinations bring the M3 coordination polyhedron closer to the shape observed in other members of the CF structural family (from CN7+1 to CN8). However, unlike other CF crystal structures, M2 keeps and even equalizes its seven-fold coordination with increasing pressure. This emphasizes the specific character of galenobismutite in this structural family. We suggest that the main reason is a comparatively large size of the M2 cation, comparable to that of the M3, unlike the other examples of CF structures, where M2 is significantly smaller than M3.
- (c)
- The structure remains stable at very high pressures (up to 20 GPa) notwithstanding the moderate bulk modulus, at least under the structural point of view, since there are no incompatible distances up to 20.9 GPa. All sulfur-sulfur distances, which could indicate instability of the structure, remained quite large with the shortest S3-S4 distance equal to 3.140 Å.
- (d)
- Calcium ferrite structure type reveals enough flexibility in incorporating various element combinations through the example of galenobismutite. Thus, not only Al and Na, incompatible in the periclase or perovskite crystal structures under the lower mantle conditions, can be considered to prefer this structure type, but it might incorporate also some other important or less abundant elements or combinations of elements.
Supplementary Materials
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Pinto, D.; Balic-Zunic, T.; Garavelli, A.; Makovicky, E.; Vurro, F. Comparative crystal-structure of Ag-free lillianite and galnbismutite from Vulcano, Aeolian Island, Italy. Can. Mineral. 2006, 44, 159–175. [Google Scholar] [CrossRef]
- Pinto, D.; Balic-Zunic, T.; Bonaccorsi, E.; Bordaev, Y.S.; Garavelli, A.; Garbarino, C.; Makovicky, E.; Mozgova, N.; Vurro, F. Rare Sulfosalts from Vulcano, Aeolian Island, Italy. VII. Cl-bearing galenobismutite. Can. Mineral. 2006, 44, 443–457. [Google Scholar] [CrossRef]
- Moëlo, Y.; Makovicky, E.; Mozgova, N.N.; Jambor, J.L.; Cook, N.; Pring, A.; Paar, W.; Nickel, E.H.; Graeser, S.; Karup-Møller, S.; et al. Sulfosalt systematics: A review. Report of the sulpfosalt sub-committee of the IMA Commission on Ore Mineralogy. Eur. J. Mineral. 2008, 20, 7–46. [Google Scholar]
- Brodtkorb, M.K.; Paar, W. Angelaíta en la paragénesis del distrito Los Manantiales, provincia del Chubut: Una nueva especie mineral. Rev. Assoc. Geol. Argent. 2004, 59, 787–789. [Google Scholar]
- Moëlo, Y.; Meerschaut, A.; Makovicky, E. Refinement of the crystal structure of nuffieldite, Pb2Cu1.4(Pb0.4Bi0.4Sb0.2)Bi2S7: Structural relationships and genesis of complex lead sulfosalt structures. Can. Mineral. 1997, 35, 1497–1508. [Google Scholar]
- Mumme, W.G. Seleniferous lead–bismuth sulphosalts from Falun, Sweden:Weibullite, wittite, and nordströmite. Am. Mineral. 1980, 65, 789–796. [Google Scholar]
- Buerger, M.J. The crystal structure of berthierite. Am. Mineral. 1936, 21, 205–206. [Google Scholar]
- Bindi, L.; Menchetti, S. Garavellite, FeSbBiS4, from the Caspari mine, North Rhine-Westphalia, Germany: Composition, physical properties and determination of the crystal structure, Locality: Caspari mine, North Rhine-Westphalia, Germany. Mineral. Petrol. 2005, 85, 131–139. [Google Scholar] [CrossRef]
- Bente, K.; Edenharter, A. Rontgenographische strukturanalyse von MnSb2S4 und strukturverfeinerung, von berthierit, FeSb2S4. Z. Krist. 1989, 185, 31–33. [Google Scholar]
- Zhou, H.; Sun, X.; Fu, Y.; Lin, H.; Jiang, L. Mineralogy and mineral chemistry: Constrains of ore genesis of the Beiya giant porphyry-skarn gold deposit, southwestern China. Ore Geol. Rev. 2016, 79, 408–424. [Google Scholar] [CrossRef]
- Wickman, F.E. The crystal structure of galenobismutite PbBi2S4. Ark. Miner. 1951, 1, 219. [Google Scholar]
- Iitaka, Y.; Nowacki, W. A re-determination of the crystal structure of galenobismutite PbBi2S4. Acta Crystallogr. 1962, 15, 691–698. [Google Scholar] [CrossRef]
- Makovicky, E. The building principles and classification of bismith-lead sulfosalts and related compounds. Fortschr. Miner. 1981, 59, 137–190. [Google Scholar]
- Finger, L.W.; Hazen, R.M. Systematic of high-pressure silicate structures. Rev. Mineral. Geochem. 2000, 41, 123–155. [Google Scholar] [CrossRef]
- Liu, L.G. High pressure NaAlSiO4: The first silicate calcium ferrite isotype. Geophys. Res. Lett. 1977, 4, 183–186. [Google Scholar] [CrossRef]
- Yamada, H.; Matsui, Y.; Ito, E. Crystal-chemical characterization of NaAlSiO4 with the CaFe2O4 structure. Mineral. Mag. 1983, 47, 177–181. [Google Scholar] [CrossRef][Green Version]
- Akaogi, M.; Tanaka, A.; Kobayashi, M.; Fikushima, N.; Suzuki, T. High pressure transformation in NaAlSiO4 and thermodynamic properties of jadeite, nepheline and calcium ferrite-type phase. Phys. Earth Plant. Inter. 2002, 130, 49–58. [Google Scholar] [CrossRef]
- Tutti, F.; Dubrovisky, L.; Saxena, S.K. High pressure phase transformation of jadeite and stability of NaAlSiO4 with calcium-ferrite type structure in the lower mantle conditions. Geophs. Res. Lett. 2000, 27, 2025–2028. [Google Scholar] [CrossRef]
- Shemet, V.; Gulay, L.; Stepien-Damm, J.; Pietraszko, A.; Olekseyuk, I. Crystal structure of the Sc2PbX4 (X = S and Se) compounds. J. Alloy. Compd. 2006, 407, 94–97. [Google Scholar] [CrossRef]
- Dubrovinsk, L.S.; Dubrovinskaia, N.A.; Prokopenko, V.B.; Le Bihan, T. Equation of state and crystal structure of NaAlSiO4 with calcium-ferrite type structure in the conditions of the lower mantle. High Press. Res. 2002, 22, 495–499. [Google Scholar] [CrossRef]
- Olsen, L.A.; Balic-Zunic, T.; Makovicky, E.; Ullrich, A.; Miletich, R. Hydrostatic compression of galenobismutite (PbBi2S4): Elastic properties and high-pressure crystal chemistry. Phys. Chem. Miner. 2007, 34, 467–475. [Google Scholar] [CrossRef]
- Ruiz-Fuertes, J.; Friedrich, A.; Errandonea, D.; Segura, A.; Morgenroth, W.; Rodríguez-Hernández, P.; Muñoz, A.; Meng, Y. Optical and structural study of the pressure-induced phase transition of CdWO4. Phys. Rev. B 2017, 95, 174105. [Google Scholar] [CrossRef]
- Comboni, D.; Lotti, P.; Gatta, G.D.; Lacalamita, M.; Mesto, E.; Merlini, M.; Hanfland, M. Amstrongite at non-ambient conditions: An in-situ high pressure single-crystal X-ray diffraction study. Microporous Mesoporous Mater. 2019, 274, 171–175. [Google Scholar] [CrossRef]
- Singh, A.K. Strength of solid helium under high pressure. J. Phys. Conf. Ser. 2012, 377, 012007. [Google Scholar] [CrossRef]
- Hammersley, A.P.; Svensson, S.O.; Hanfland, M.; Fitch, A.N.; Hausermann, D. Two-dimensional detector software: From real detector to idealized image or two-theta scan. High Press. Res. 1996, 14, 235–245. [Google Scholar] [CrossRef]
- Oxford Diffraction. CrysAlis(Pro); Oxford Diffraction Ltd.: Abingdon, UK, 2006. [Google Scholar]
- Angel, R.; Gonzalez-Platas, J. ABSORB–7 and ABSORB-GUI for single crystal absorption correction. J. Appl. Crystall. 2013, 46, 252–254. [Google Scholar] [CrossRef]
- Hubschle, C.B.; Sheldrick, G.M.; Dittrich, B. ShelXle: A Qt graphical user interface for29 SHELXL. J. Appl. Cryst. 2011, 44, 1281–1284. [Google Scholar] [CrossRef] [PubMed]
- Gonzalez-Platas, J.; Alvaro, M.; Nestola, F.; Angel, R. EOSFIT7-GUI: A new graphycal user interface for equation of state calculation, analyses and teaching. J. Appl. Cryst. 2016, 49, 1377–1382. [Google Scholar] [CrossRef]
- Angel, R.J. Equation of state. In High Temperature and High Pressure Crystal Chemistry; Hazen, R.M., Downs, R.T., Eds.; Reviews in Mineralogy and Geochemistry; Mineralogical Society of America: Chantilly, VA, USA, 2000; Volume 41, pp. 117–211. [Google Scholar]
- Knorr, K.; Ehm, L.; Hytha, M.; Winkler, B.; Depmeier, W. The high-pressure α/β phase transition in lead sulphide (PbS)—X-ray powder diffraction and quantum mechanical calculations. Eur. Phys. J. 2003, 31, 297–303. [Google Scholar] [CrossRef]
- Lundegaard, L.; Makovicky, E.; Boffa Ballaran, T.; Balic-Zunic, T. Crystal structure and cation lone electron pair activity of Bi2S3 between 0 and 10 GPa. Phys. Chem. Miner. 2005, 32, 578–584. [Google Scholar] [CrossRef]
- Lundegaard, L.F.; Miletich, R.; Balic-Zunic, T.; Makovicky, E. Equation of state and crystal structure of Sb2S3 between 0 and 10 GPa. Phys. Chem. Miner. 2003, 30, 463–468. [Google Scholar] [CrossRef]
- Comodi, P.; Guidoni, F.; Nazzareni, S.; Balic-Zunic, T.; Zucchini, A.; Makoviscky, E.; Prakapenka, V. A high-pressure phase transition in chalcostibite, CuSbS2. Eur. J. Miner. 2018, 30, 491–505. [Google Scholar] [CrossRef]
- Olsen, L.A.; Balić-Žunić, T.; Makovicky, E. High-pressure anisotropic distortion of Pb3Bi2S6: A pressure-induced, reversible phase transition with migration of chemical bonds. Inorg. Chem. 2008, 47, 6756–6762. [Google Scholar] [CrossRef]
- Olsen, L.A.; Friese, K.; Makovicky, E.; Balić-Žunić, T.; Morgenroth, W.; Grzechnik, A. Pressure induced phase transition in Pb6Bi2S9. Phys. Chem. Miner. 2011, 38, 1–10. [Google Scholar] [CrossRef]
- Periotto, B.; Balic-Zunic, T.; Nestola, F. The role of the Sb3+ lone electron pairs and Fe2+ coordination in the high-pressure behavior of berthierite. Can. Mineral. 2012, 50, 201–218. [Google Scholar] [CrossRef]
- Comodi, P.; Mellini, M.; Zanazzi, P.F. Scapolites: Variation of structure with pressure and possible role in the storage of fluids. Eur. J. Mineral. 1990, 2, 195–202. [Google Scholar] [CrossRef]
- Gatta, G.D.; Comodi, P.; Zanazzi, P.F.; Boffa Ballaran, T. Anomalous elastic behaviour and high-pressure structural evolution of zeolite levyne. Am. Mineral. 2005, 90, 645–652. [Google Scholar] [CrossRef]
- Mariolacos, K.; Kupčik, V.; Ohmasa, M.; Miehe, G. The Crystal Structure of Cu4Bi5S10 and its Relation to the Structures of Hodrushite and Cuprobismutite. Acta Cryst. 1975, 31, 703–708. [Google Scholar] [CrossRef]
- Topa, D.; Makovicky, E.; Balić-Žunić, T.; Paar, W.H. Kupčikite, Cu3.4Fe0.6Bi5S10, a new Cu-Bi sulfosalt from Felbertal, Austria, and its crystal structure. Can. Mineral. 2003, 41, 1155–1166. [Google Scholar] [CrossRef][Green Version]
- Grzechnik, A.; Friese, K. Pressure-induced orthorhombic structure of PbS. J. Phys. Condens. Matter 2010, 22, 095402. [Google Scholar] [CrossRef]
- Olsen, L.A.; Lopez-Solano, J.; Garcia, A.; Balic-Zinic, T.; Makovicky, E. Dependence of lone pair of bismuth on coordination environment and pressure: Ab Initio study on Cu4Bi5S10 and Bi2S3. J. Solid State Chem. 2010, 18, 2133–2143. [Google Scholar] [CrossRef]
- Yutani, M.; Yagi, T.; Yusa, H.; Irifune, T. Compressibility of calcium ferrite-type MgAl2O4. Phys. Chem. Miner. 1997, 24, 340–344. [Google Scholar] [CrossRef]
- Haavik, C.; Stolen, S.; Fjellvag, H.; Hanfland, M.; Häusermann, D. Equation of state of magnetite and its high-pressure modification: Thermodynamics of the Fe-O system at high pressure. Am. Mineral. 2000, 85, 514–523. [Google Scholar] [CrossRef]
- Anderson, O.L. Equation of State of Solids for Geophysics and Ceramic Science; Oxford University Press: New York, NY, USA, 1994; p. 495. [Google Scholar]
- Makovicky, E.; Balić-Žunić, T. New measure of distortion for coordination polyhedra. Acta Cryst. 1998, 54, 766–773. [Google Scholar] [CrossRef]
- Balić Žunić, T.; Makovicky, E. Determination of the Centroid or “the Best Centre” of a Coordination Polyhedron. Acta Cryst. 1996, 52, 78–81. [Google Scholar] [CrossRef]



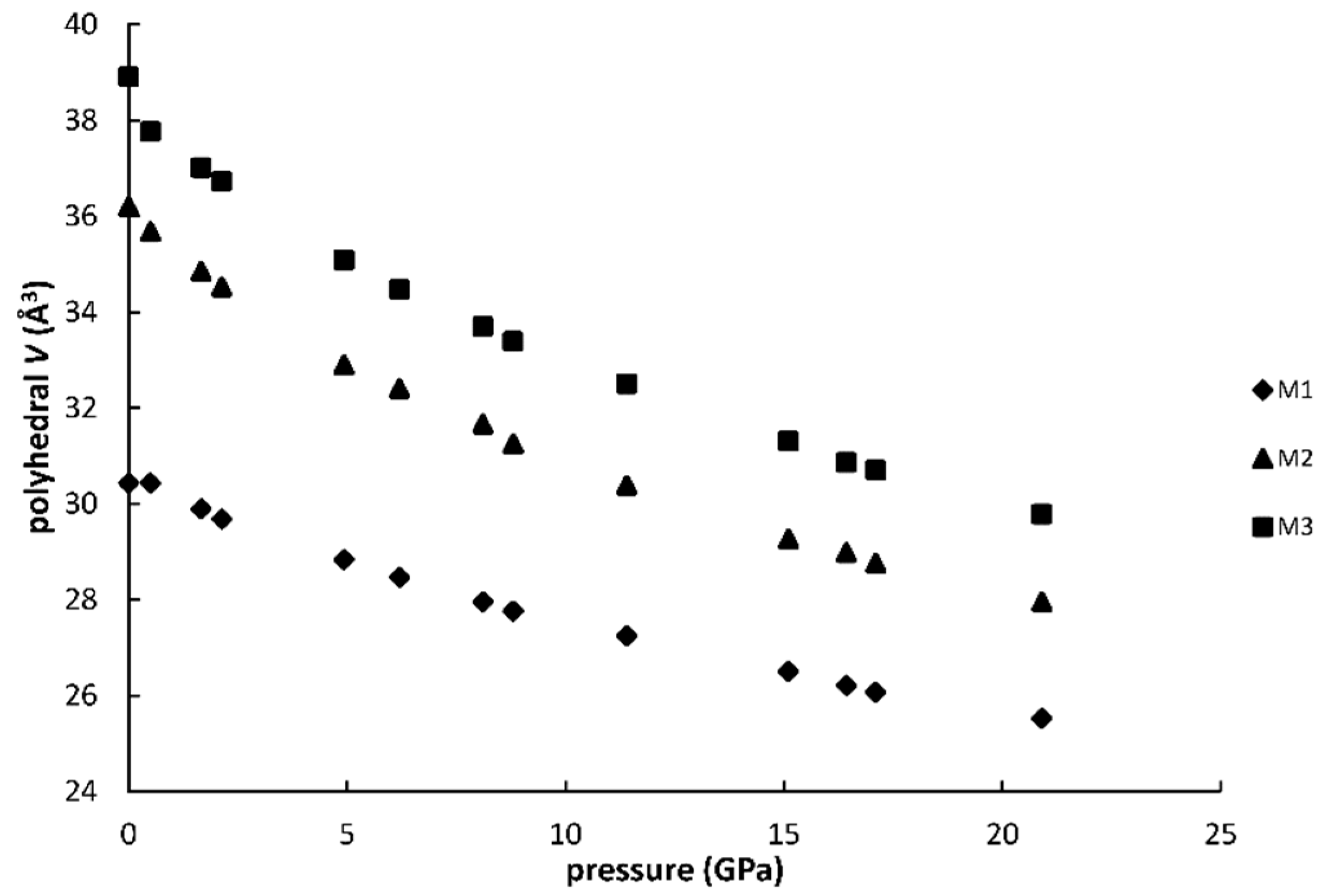
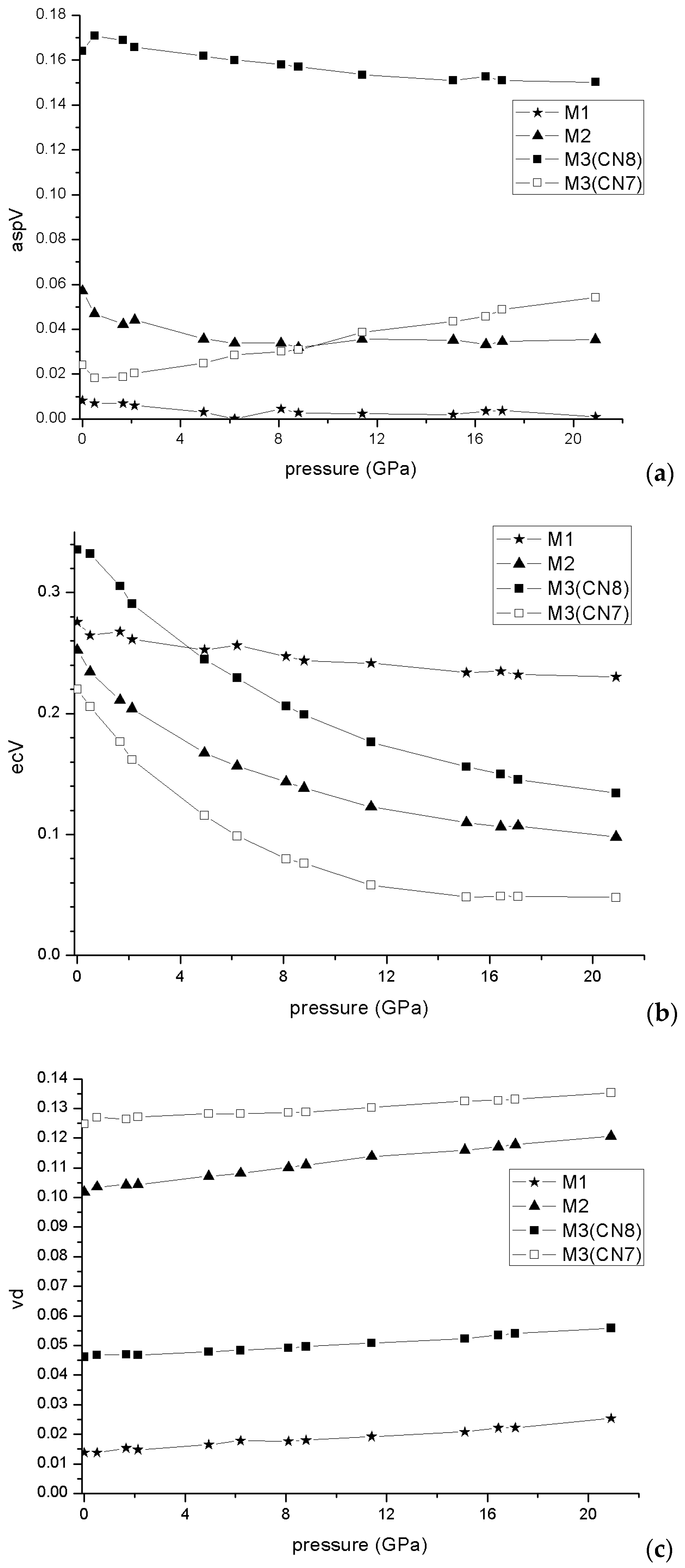
| P (GPa) | 0.50 | 1.66 | 2.13 * | 4.94 | 6.20 | 8.10 * | 8.80 | 11.40 | 15.10 | 16.43 * | 17.10 | 20.90 |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| a (Å) | 11.7338(7) | 11.6511(4) | 11.6167(7) | 11.4505(5) | 11.3956(6) | 11.3191(8) | 11.2841(6) | 11.2038(6) | 11.0890(8) | 11.0611(5) | 11.0423(5) | 10.9733(6) |
| b (Å) | 14.5066(4) | 14.3951(3) | 14.3541(3) | 14.1314(4) | 14.0521(4) | 13.9355(3) | 13.8803(3) | 13.7379(4) | 13.5330(5) | 13.4491(3) | 13.4115(3) | 13.2252(4) |
| c (Å) | 4.05910(6) | 4.03492(5) | 4.02572(4) | 3.97480(5) | 3.95717(5) | 3.93304(5) | 3.92120(5) | 3.89439(6) | 3.86003(7) | 3.84944(5) | 3.84254(5) | 3.81906(5) |
| V (Å3) | 690.93(5) | 676.73(3) | 671.28(4) | 643.17(3) | 633.67(4) | 620.39(5) | 614.16(4) | 599.41(4) | 579.26(5) | 572.65(3) | 569.06(3) | 554.24(4) |
| ρ (g/cm3) | 7.243 | 7.395 | 7.455 | 7.780 | 7.897 | 8.066 | 8.148 | 8.348 | 8.639 | 8.739 | 8.794 | 9.029 |
| Data collection | ||||||||||||
| # Meas. refl. | 1004 | 1091 | 1132 | 1056 | 1052 | 1026 | 1015 | 1052 | 1022 | 1080 | 1033 | 1106 |
| # Unique refl. | 536 | 581 | 551 | 555 | 549 | 510 | 536 | 531 | 501 | 500 | 504 | 511 |
| # Obs. ref. ** | 506 | 548 | 516 | 514 | 517 | 518 | 520 | 487 | 485 | 488 | 491 | |
| Rint | 0.0314 | 0.0314 | 0.0266 | 0.0284 | 0.0315 | 0.0265 | 0.0296 | 0.0321 | 0.0263 | 0.0247 | 0.0237 | 0.0222 |
| 2θmax (°) | 41.84 | 40.93 | 41.05 | 41.29 | 40.96 | 41.16 | 41.02 | 41.10 | 41.63 | 41.16 | 41.26 | 41.30 |
| Range hkl | −10 ≤ h ≤ 12 | −9 ≤ h ≤ 12 | −7 ≤ h ≤ 10 | −12 ≤ h ≤ 9 | −9 ≤ h ≤ 12 | −9 ≤ h ≤ 7 | −11 ≤ h ≤ 9 | −11 ≤ h ≤ 9 | −9 ≤ h ≤ 11 | −11 ≤ h ≤ 8 | −9 ≤ h ≤ 11 | −9 ≤ h ≤ 11 |
| −17 ≤ k ≤ 19 | −19 ≤ k ≤ 17 | −19 ≤ k ≤ 20 | −19 ≤ k ≤ 17 | −16 ≤ k ≤ 19 | −20 ≤ k ≤ 19 | −18 ≤ k ≤ 17 | −18 ≤ k ≤ 16 | −17 ≤ k ≤ 18 | −18 ≤ k ≤ 17 | −17 ≤ k ≤ 18 | −16 ≤ k ≤ 18 | |
| −6 ≤ l ≤ 5 | −5 ≤ l ≤ 5 | −5 ≤ l ≤ 5 | −5 ≤ l ≤ 5 | −5 ≤ l ≤ 5 | −5 ≤ l ≤ 5 | −5 ≤ l ≤ 5 | −5 ≤ l ≤ 5 | −5 ≤ l ≤ 5 | −5 ≤ l ≤ 5 | −5 ≤ l ≤ 5 | −5 ≤ l ≤ 5 | |
| senθ/λ (Å−1) | 0.87 | 0.85 | 0.85 | 0.86 | 0.85 | 0.85 | 0.85 | 0.85 | 0.86 | 0.85 | 0.86 | 0.86 |
| 2θ max | 41.8 | 40.9 | 41.1 | 41.3 | 41.0 | 41.2 | 41.0 | 41.1 | 41.6 | 41.2 | 41.3 | 41.3 |
| Completeness (%) *** | 25.76 | 30.11 | 29.09 | 29.32 | 30.13 | 28.73 | 29.98 | 30.18 | 28.53 | 29.58 | 29.66 | 30.75 |
| Rafinement | ||||||||||||
| R1 (|F0| > 4σ) | 0.0246 | 0.0213 | 0.0199 | 0.0223 | 0.0213 | 0.0221 | 0.0210 | 0.0221 | 0.0193 | 0.0163 | 0.0164 | 0.0158 |
| wR2 | 0.0626 | 0.0537 | 0.0489 | 0.0538 | 0.0539 | 0.0586 | 0.0552 | 0.0553 | 0.0442 | 0.0372 | 0.0367 | 0.0360 |
| Goodness of Fit (GooF) | 1.071 | 1.023 | 1.044 | 1.016 | 1.032 | 1.027 | 1.084 | 1.078 | 1.006 | 1.019 | 1.016 | 1.037 |
| # Parameters | 44 | 44 | 44 | 44 | 44 | 44 | 44 | 44 | 44 | 44 | 44 | 44 |
| GPa | 0.50 | 1.66 | 2.13 * | 4.94 | 6.20 | 8.10 * | 8.80 | 11.4 | 15.10 | 16.43 * | 17.10 | 20.90 | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Bi1 | x | 0.06702(6) | 0.06582(4) | 0.06536(7) | 0.06325(6) | 0.06267(6) | 0.06199(7) | 0.06172(6) | 0.06115(6) | 0.06082(6) | 0.06073(6) | 0.06076(6) | 0.06071(6) |
| y | 0.39075(3) | 0.39092(3) | 0.39102(3) | 0.39170(3) | 0.39196(3) | 0.39247(3) | 0.39268(3) | 0.39333(3) | 0.39446(3) | 0.39490(3) | 0.39508(3) | 0.39644(3) | |
| z | 0.25 | 0.25 | 0.25 | 0.25 | 0.25 | 0.25 | 0.25 | 0.25 | 0.25 | 0.25 | 0.25 | 0.25 | |
| Uiso | 0.0150(2) | 0.01358(19) | 0.0122(2) | 0.0112(2) | 0.0106(2) | 0.0108(3) | 0.0103(2) | 0.0102(2) | 0.01034(18) | 0.01120(17) | 0.01005(17) | 0.01091(16) | |
| U11 | 0.0144(7) | 0.0138(6) | 0.0117(8) | 0.0109(6) | 0.0090(6) | 0.0110(9) | 0.0095(6) | 0.0093(6) | 0.0108(6) | 0.0123(5) | 0.0113(5) | 0.0125(5) | |
| U22 | 0.0168(4) | 0.0149(3) | 0.0135(3) | 0.0128(4) | 0.0136(4) | 0.0123(3) | 0.0123(4) | 0.0127(4) | 0.0117(3) | 0.0120(3) | 0.0106(3) | 0.0115(3) | |
| U33 | 0.0139(2) | 0.0121(2) | 0.0115(2) | 0.0097(2) | 0.0093(2) | 0.0091(2) | 0.0091(2) | 0.0085(2) | 0.00849(18) | 0.00937(17) | 0.00829(17) | 0.00868(16) | |
| U23 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | |
| U13 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | |
| U12 | 0.00162(16) | 0.00131(13) | 0.00121(18) | 0.00082(16) | 0.00074(14) | 0.00024(18) | 0.00052(14) | 0.00024(14) | 0.00030(14) | 0.00024(13) | 0.00023(13) | 0.00013(13) | |
| Bi2 | x | 0.10329(6) | 0.10545(5) | 0.10621(7) | 0.10974(6) | 0.11084(6) | 0.11234(7) | 0.11270(6) | 0.11413(6) | 0.11562(6) | 0.11623(6) | 0.11627(5) | 0.11716(5) |
| y | 0.90590(3) | 0.90648(3) | 0.90666(3) | 0.90753(4) | 0.90789(3) | 0.90843(3) | 0.90869(3) | 0.90935(3) | 0.91036(3) | 0.91074(3) | 0.91097(3) | 0.91199(3) | |
| z | 0.25 | 0.25 | 0.25 | 0.25 | 0.25 | 0.25 | 0.25 | 0.25 | 0.25 | 0.25 | 0.25 | 0.25 | |
| Uiso | 0.0182(2) | 0.0158(2) | 0.0140(3) | 0.0123(2) | 0.0112(2) | 0.0106(3) | 0.0107(2) | 0.0101(2) | 0.00983(18) | 0.01075(17) | 0.00945(16) | 0.01001(16) | |
| U11 | 0.0193(7) | 0.0174(6) | 0.0141(9) | 0.0130(6) | 0.0100(6) | 0.0101(9) | 0.0111(6) | 0.0095(6) | 0.0104(6) | 0.0125(5) | 0.0113(5) | 0.0115(5) | |
| U22 | 0.0230(4) | 0.0190(3) | 0.0178(3) | 0.0146(3) | 0.0147(3) | 0.0132(3) | 0.0126(3) | 0.0126(3) | 0.0109(3) | 0.0107(3) | 0.0093(3) | 0.0099(2) | |
| U33 | 0.0122(2) | 0.0110(2) | 0.0103(2) | 0.0092(2) | 0.0090(2) | 0.0085(2) | 0.0086(2) | 0.0081(2) | 0.00821(19) | 0.00908(18) | 0.00768(17) | 0.00863(16) | |
| U23 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | |
| U13 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | |
| U12 | 0.00020(18) | 0.00009(14) | 0.00001(19) | 0.00000(16) | 0.00005(14) | −0.00003(17) | −0.00007(15) | −0.00010(15) | −0.00006(14) | −0.00009(13) | −0.00008(13) | −0.00030(13) | |
| Pb3 | x | 0.24848(7) | 0.25147(5) | 0.25280(7) | 0.25793(6) | 0.25963(6) | 0.26198(7) | 0.26265(6) | 0.26497(6) | 0.26766(6) | 0.26874(6) | 0.26905(5) | 0.27136(5) |
| y | 0.65233(4) | 0.65209(3) | 0.65203(3) | 0.65201(4) | 0.65203(3) | 0.65214(3) | 0.65219(4) | 0.65241(3) | 0.65264(3) | 0.65274(3) | 0.65279(3) | 0.65308(3) | |
| z | 0.25 | 0.25 | 0.25 | 0.25 | 0.25 | 0.25 | 0.25 | 0.25 | 0.25 | 0.25 | 0.25 | 0.25 | |
| Uiso | 0.0229(3) | 0.0198(2) | 0.0180(3) | 0.0153(2) | 0.0141(2) | 0.0132(3) | 0.0126(2) | 0.0118(2) | 0.01114(19) | 0.01206(18) | 0.01068(17) | 0.01094(17) | |
| U11 | 0.0274(8) | 0.0247(7) | 0.0219(9) | 0.0199(7) | 0.0164(7) | 0.0167(9) | 0.0160(6) | 0.0145(6) | 0.0142(6) | 0.0166(6) | 0.0149(5) | 0.0145(5) | |
| U22 | 0.0221(5) | 0.0185(4) | 0.0172(3) | 0.0141(4) | 0.0146(4) | 0.0131(3) | 0.0122(4) | 0.0125(3) | 0.0113(3) | 0.0109(3) | 0.0096(3) | 0.0104(3) | |
| U33 | 0.0192(2) | 0.0163(2) | 0.0150(2) | 0.0119(2) | 0.0111(2) | 0.0099(2) | 0.0096(2) | 0.0085(2) | 0.00793(19) | 0.00876(18) | 0.00746(17) | 0.00790(16) | |
| U23 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | |
| U13 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | |
| U12 | 0.0053(2) | 0.00440(17) | 0.0040(2) | 0.00297(18) | 0.00267(17) | 0.00241(18) | 0.00217(16) | 0.00197(15) | 0.00169(15) | 0.00157(14) | 0.00158(14) | 0.00153(13) | |
| S1 | x | 0.3343(4) | 0.3330(3) | 0.3335(5) | 0.3313(4) | 0.3306(4) | 0.3309(5) | 0.3303(4) | 0.3295(4) | 0.3285(4) | 0.3280(4) | 0.3281(4) | 0.3267(4) |
| y | 0.0167(3) | 0.0164(2) | 0.0169(2) | 0.0175(3) | 0.0182(2) | 0.0189(2) | 0.0187(2) | 0.0200(2) | 0.0210(2) | 0.0214(2) | 0.0217(2) | 0.0228(2) | |
| z | 0.25 | 0.25 | 0.25 | 0.25 | 0.25 | 0.25 | 0.25 | 0.25 | 0.25 | 0.25 | 0.25 | 0.25 | |
| Uiso | 0.0161(9) | 0.0148(8) | 0.0150(16) | 0.0124(10) | 0.0127(9) | 0.0108(16) | 0.0122(10) | 0.0113(10) | 0.0108(9) | 0.0117(8) | 0.0119(9) | 0.0110(8) | |
| U11 | 0.010(4) | 0.010(4) | 0.015(6) | 0.011(4) | 0.016(4) | 0.006(6) | 0.012(4) | 0.010(4) | 0.009(4) | 0.010(4) | 0.016(4) | 0.008(3) | |
| U22 | 0.021(2) | 0.0182(19) | 0.0141(19) | 0.012(2) | 0.0079(19) | 0.0134(16) | 0.013(2) | 0.0115(19) | 0.010(2) | 0.0106(18) | 0.0066(18) | 0.0117(17) | |
| U33 | 0.0178(10) | 0.0162(10) | 0.0163(11) | 0.0137(11) | 0.0147(10) | 0.0133(10) | 0.0122(10) | 0.0122(10) | 0.0134(10) | 0.0145(10) | 0.0128(10) | 0.0131(10) | |
| U23 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | |
| U13 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | |
| U12 | 0.0001(11) | 0.0025(10) | 0.0014(14) | 0.0008(11) | −0.0003(11) | 0.0002(13) | 0.0017(12) | 0.0014(11) | −0.0006(11) | −0.0002(10) | −0.0012(10) | 0.0000(10) | |
| S2 | x | 0.2595(4) | 0.2591(3) | 0.2601(5) | 0.2602(4) | 0.2597(4) | 0.2602(5) | 0.2605(4) | 0.2614(4) | 0.2626(4) | 0.2618(4) | 0.2620(4) | 0.2627(4) |
| y | 0.2961(2) | 0.29633(19) | 0.2965(2) | 0.2964(2) | 0.2967(2) | 0.2969(2) | 0.2969(2) | 0.2971(2) | 0.2972(2) | 0.2973(2) | 0.2973(2) | 0.2979(2) | |
| z | 0.25 | 0.25 | 0.25 | 0.25 | 0.25 | 0.25 | 0.25 | 0.25 | 0.25 | 0.25 | 0.25 | 0.25 | |
| Uiso | 0.0135(10) | 0.0123(8) | 0.0118(14) | 0.0091(9) | 0.0104(9) | 0.0105(14) | 0.0098(10) | 0.0085(8) | 0.0097(9) | 0.0098(8) | 0.0096(8) | 0.0098(8) | |
| U11 | 0.011(4) | 0.008(4) | 0.010(5) | 0.002(4) | 0.008(4) | 0.009(5) | 0.007(4) | 0.003(4) | 0.007(4) | 0.008(3) | 0.010(3) | 0.010(3) | |
| U22 | 0.017(2) | 0.0150(19) | 0.0140(17) | 0.014(2) | 0.013(2) | 0.0123(17) | 0.013(2) | 0.013(2) | 0.013(2) | 0.0107(18) | 0.0115(19) | 0.0103(18) | |
| U33 | 0.0127(10) | 0.0142(9) | 0.0113(10) | 0.0115(11) | 0.0106(9) | 0.0100(9) | 0.0092(10) | 0.0094(9) | 0.0091(9) | 0.0107(9) | 0.0078(9) | 0.0092(9) | |
| U23 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | |
| U13 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | |
| U12 | −0.0001(11) | 0.0011(9) | −0.0008(12) | −0.0010(11) | −0.0011(10) | −0.0002(12) | −0.0005(10) | −0.0005(11) | −0.0004(11) | 0.0002(9) | 0.0000(10) | −0.0009(9) | |
| S3 | x | 0.0547(4) | 0.0543(3) | 0.0543(5) | 0.0535(5) | 0.0534(4) | 0.0530(5) | 0.0525(4) | 0.0518(4) | 0.0510(4) | 0.0510(4) | 0.0504(4) | 0.0499(4) |
| y | 0.0940(2) | 0.0948(2) | 0.0947(2) | 0.0966(2) | 0.0975(2) | 0.0984(2) | 0.0988(2) | 0.1004(2) | 0.1020(2) | 0.1029(2) | 0.1032(2) | 0.1052(2) | |
| z | 0.25 | 0.25 | 0.25 | 0.25 | 0.25 | 0.25 | 0.25 | 0.25 | 0.25 | 0.25 | 0.25 | 0.25 | |
| Uiso | 0.0149(10) | 0.0139(9) | 0.0116(14) | 0.0112(10) | 0.0109(9) | 0.0121(17) | 0.0101(9) | 0.0096(9) | 0.0096(9) | 0.0116(9) | 0.0087(8) | 0.0099(8) | |
| U11 | 0.011(4) | 0.013(4) | 0.007(5) | 0.009(4) | 0.008(4) | 0.014(6) | 0.007(4) | 0.007(4) | 0.009(4) | 0.013(4) | 0.008(3) | 0.012(3) | |
| U22 | 0.017(2) | 0.0139(17) | 0.0148(17) | 0.013(2) | 0.015(2) | 0.0107(18) | 0.012(2) | 0.012(2) | 0.0096(19) | 0.0122(19) | 0.0094(18) | 0.0088(17) | |
| U33 | 0.0174(11) | 0.0152(9) | 0.0132(10) | 0.0116(11) | 0.0104(10) | 0.0117(9) | 0.0110(9) | 0.0091(9) | 0.0097(10) | 0.0099(9) | 0.0084(9) | 0.0093(9) | |
| U23 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | |
| U13 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | |
| U12 | 0.0007(10) | −0.0013(9) | −0.0011(12) | 0.0003(11) | −0.0003(10) | −0.0028(12) | 0.0000(10) | 0.0001(10) | 0.0002(9) | −0.0011(10) | −0.0007(9) | −0.0005(9) | |
| S4 | x | 0.0197(4) | 0.0197(4) | 0.0191(5) | 0.0194(4) | 0.0190(4) | 0.0194(5) | 0.0194(4) | 0.0185(4) | 0.0183(4) | 0.0188(4) | 0.0184(4) | 0.0185(4) |
| y | 0.7124(2) | 0.71258(19) | 0.7129(2) | 0.7142(2) | 0.7146(2) | 0.7151(2) | 0.7154(2) | 0.7156(2) | 0.7157(2) | 0.7154(2) | 0.7157(2) | 0.7151(2) | |
| z | 0.25 | 0.25 | 0.25 | 0.25 | 0.25 | 0.25 | 0.25 | 0.25 | 0.25 | 0.25 | 0.25 | 0.25 | |
| Uiso | 0.0143(9) | 0.0130(7) | 0.0109(13) | 0.0108(10) | 0.0106(9) | 0.0103(15) | 0.0110(9) | 0.0108(9) | 0.0098(9) | 0.0113(9) | 0.0105(8) | 0.0101(8) | |
| U11 | 0.018(4) | 0.015(3) | 0.011(5) | 0.011(4) | 0.010(4) | 0.009(5) | 0.010(4) | 0.010(4) | 0.007(4) | 0.013(3) | 0.012(3) | 0.010(3) | |
| U22 | 0.0123(19) | 0.0118(17) | 0.0100(16) | 0.013(2) | 0.0131(19) | 0.0118(16) | 0.014(2) | 0.0136(19) | 0.0135(19) | 0.0101(17) | 0.0101(17) | 0.0102(16) | |
| U33 | 0.0127(9) | 0.0122(8) | 0.0115(9) | 0.0081(10) | 0.0091(9) | 0.0101(9) | 0.0085(9) | 0.0091(9) | 0.0093(9) | 0.0108(9) | 0.0092(9) | 0.0101(9) | |
| U23 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | |
| U13 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | |
| U12 | −0.0031(11) | −0.0034(9) | −0.0023(12) | −0.0036(11) | −0.0040(10) | −0.0035(12) | −0.0040(11) | −0.0051(11) | −0.0037(11) | −0.0039(10) | −0.0040(10) | −0.0019(9) | |
| P (GPa) | M1-S2 | M1-S4(X2)’ | M1-S1(X2) | M1-S1’ | Vol | Mean M1-S |
|---|---|---|---|---|---|---|
| 0.0001 | 2.6890 | 2.1788 | 2.9646 | 3.0623 | 30.44 | 2.843 |
| 0.50 | 2.643 | 2.719 | 2.966 | 3.043 | 30.43 | 2.843 |
| 1.66 | 2.632 | 2.699 | 2.953 | 3.023 | 29.90 | 2.826 |
| 4.93 | 2.624 | 2.661 | 2.931 | 2.953 | 28.84 | 2.783 |
| 6.20 | 2.614 | 2.650 | 2.922 | 2.931 | 28.47 | 2.782 |
| 8.80 | 2.608 | 2.633 | 2.893 | 2.887 | 27.76 | 2.759 |
| 11.40 | 2.604 | 2.613 | 2.885 | 2.855 | 27.24 | 2.742 |
| 15.10 | 2.596 | 2.592 | 2.857 | 2.819 | 26.50 | 2.719 |
| 17.10 | 2.580 | 2.581 | 2.843 | 2.801 | 26.07 | 2.705 |
| 20.90 | 2.571 | 2.565 | 2.822 | 2.781 | 25.52 | 2.688 |
| 16.43 | 2.582 | 2.584 | 2.848 | 2.810 | 26.11 | 2.710 |
| 8.10 | 2.609 | 2.639 | 2.905 | 2.893 | 27.95 | 2.765 |
| 2.13 | 2.638 | 2.690 | 2.949 | 3.000 | 29.68 | 2.819 |
| P (GPa) | M2-S3 (x2) | M2-S3’ | M2-S4 | M2-S2(x2) | M2-S1 | Vol | Mean M2-S |
|---|---|---|---|---|---|---|---|
| 0.0001 | 2.7394 | 2.7962 | 2.9913 | 3.0559 | 3.1960 | 36.195 | 2.939 |
| 0.50 | 2.749 | 2.788 | 2.973 | 3.041 | 3.151 | 35.68 | 2.927 |
| 1.66 | 2.745 | 2.776 | 2.965 | 3.012 | 3.087 | 34.85 | 2.906 |
| 4.93 | 2.7319 | 2.747 | 2.924 | 2.935 | 2.974 | 32.89 | 2.854 |
| 6.20 | 2.725 | 2.744 | 2.911 | 2.921 | 2.945 | 32.40 | 2.842 |
| 8.80 | 2.707 | 2.725 | 2.882 | 2.881 | 2.891 | 31.25 | 2.811 |
| 11.40 | 2.695 | 2.716 | 2.869 | 2.849 | 2.852 | 30.38 | 2.789 |
| 15.10 | 2.677 | 2.691 | 2.847 | 2.810 | 2.795 | 29.26 | 2.758 |
| 17.10. | 2.667 | 2.679 | 2.833 | 2.797 | 2.771 | 28.76 | 2.744 |
| 20.90 | 2.656 | 2.660 | 2.820 | 2.768 | 2.727 | 27.96 | 2.722 |
| 16.43 | 2.676 | 2.683 | 2.840 | 2.802 | 2.775 | 28.98 | 2.751 |
| 8.10. | 2.716 | 2.731 | 2.892 | 2.892 | 2.914 | 31.67 | 2.822 |
| 2.13 | 2.744 | 2.766 | 2.960 | 2.994 | 3.078 | 34.52 | 2.897 |
| P (GPa) | M3-S4 | M3-S2(x2) | M2-S1(x2) | M3-S3(x2) | M2-S4’ | Vol | Mean M3-S |
|---|---|---|---|---|---|---|---|
| 0.0001 | 2.8400 | 2.9320 | 3.0083 | 3.2375 | 3.7572 | 38.91 | 3.028 |
| 0.50 | 2.822 | 2.912 | 2.989 | 3.189 | 3.739 | 37.78 | 3.000 |
| 1.66 | 2.837 | 2.898 | 2.976 | 3.142 | 3.683 | 37.01 | 2.981 |
| 4.93 | 2.869 | 2.860 | 2.931 | 3.037 | 3.543 | 35.08 | 2.933 |
| 6.20 | 2.880 | 2.845 | 2.917 | 3.007 | 3.500 | 34.47 | 2.917 |
| 8.80 | 2.882 | 2.819 | 2.894 | 2.957 | 3.431 | 33.39 | 2.889 |
| 11.40 | 2.895 | 2.798 | 2.867 | 2.918 | 3.370 | 32.50 | 2.866 |
| 15.10 | 2.894 | 2.768 | 2.835 | 2.870 | 3.301 | 31.30 | 2.834 |
| 17.10 | 2.893 | 2.751 | 2.817 | 2.848 | 3.270 | 30.70 | 2.818 |
| 20.90 | 2.893 | 2.730 | 2.788 | 2.810 | 3.224 | 29.78 | 2.793 |
| 16.43 | 2.890 | 2.757 | 2.823 | 2.851 | 3.286 | 30.86 | 2.822 |
| 8.10 | 2.883 | 2.828 | 2.902 | 2.969 | 3.451 | 33.70 | 2.897 |
| 2.13 | 2.852 | 2.894 | 2.970 | 3.122 | 3.600 | 36.72 | 2.975 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Comodi, P.; Zucchini, A.; Balić-Žunić, T.; Hanfland, M.; Collings, I. The High Pressure Behavior of Galenobismutite, PbBi2S4: A Synchrotron Single Crystal X-ray Diffraction Study. Crystals 2019, 9, 210. https://doi.org/10.3390/cryst9040210
Comodi P, Zucchini A, Balić-Žunić T, Hanfland M, Collings I. The High Pressure Behavior of Galenobismutite, PbBi2S4: A Synchrotron Single Crystal X-ray Diffraction Study. Crystals. 2019; 9(4):210. https://doi.org/10.3390/cryst9040210
Chicago/Turabian StyleComodi, Paola, Azzurra Zucchini, Tonci Balić-Žunić, Michael Hanfland, and Ines Collings. 2019. "The High Pressure Behavior of Galenobismutite, PbBi2S4: A Synchrotron Single Crystal X-ray Diffraction Study" Crystals 9, no. 4: 210. https://doi.org/10.3390/cryst9040210
APA StyleComodi, P., Zucchini, A., Balić-Žunić, T., Hanfland, M., & Collings, I. (2019). The High Pressure Behavior of Galenobismutite, PbBi2S4: A Synchrotron Single Crystal X-ray Diffraction Study. Crystals, 9(4), 210. https://doi.org/10.3390/cryst9040210





