First-Principles Investigation of the Effect of Vacancy Defects and Carbon Impurities on Thermal Conductivity of Uranium Mononitride (UN)

Abstract

Uranium mononitride (UN) is a promising nuclear fuel with a high melting point, high thermal conductivity, and low coefficient of thermal expansion. Theoretical studies of UN can provide insights on its thermal transport mechanism, which is of great significance for the design and application of UN fuel. During the processing and operation, crystal defects and impurities, such as vacancies and carbon impurities, potentially arise in the nuclear fuel, which probably affect the thermomechanical properties of UN. To figure out the effect of vacancy defects and carbon impurities on the thermal conductivity of UN, density functional theory and Boltzmann transport theory are applied to conduct a theoretical investigation on the mechanical and thermal properties of ideal and defective UN. The calculated results show that in the case of UN with a U or N vacancy, both the lattice and electronic thermal conductivity are decreased, compared with the ideal case. With a carbon atom occupying the N site in the lattice, the electronic thermal conductivity is reduced but the lattice thermal conductivity is increased. Combining the results of lattice and electronic thermal conductivity, the total thermal conductivities of three defective states are lower than the ideal UN. The thermal conductivities of UN with a U vacancy (13.91 W/mK), N vacancy (15.36 W/mK), and a carbon atom occupying the N site (15.14 W/mK) are, respectively, reduced by 25.7%, 18.0%, and 19.2%, in comparison with ideal result (18.73 W/mK) at 1000 K.

1. Introduction

Uranium dioxide (UO₂) has been widely used as nuclear fuel material in nuclear power reactors because of its good irradiation stability [1]. However, its thermal conductivity is relatively low, resulting in a high temperature gradient between the fuel surface and center. The corresponding thermal stress due to the temperature gradient would lead to cracks in fuel pellets and fuel performance degradation [2,3,4]. In addition, in some accident conditions, the heat generated by the fuel cannot be quickly removed, resulting in a rapid increase in temperature, which may eventually lead to fuel failure. Compared with traditional UO₂ fuel, UN fuel combines the advantages of UO₂ and uranium alloys, with a high melting point, high thermal conductivity, low coefficient of thermal expansion, and low fission gas release rate, which can provide higher safety performance under accident conditions [5].

The theoretical modeling of thermophysical properties of UN can provide critical thermophysical parameters for fuel performance modelling, as well as reveal key microstructural features that may affect the thermal properties of the fuel. Among the different computational methods, density functional theory (DFT), which has been extensively utilized for modeling various materials and their properties, has been shown to be useful for reproducing experimental results and predicting material properties and behavior. However, using the DFT method, it is difficult to accurately describe the strong electron correlation caused by 5f valence electrons in the U atom [6]. Therefore, researchers applied the DFT approach with the Hubbard U method (DFT + U) to study the properties of UN [7,8,9]. However, when it comes to the study of thermodynamic properties, some studies have reported that the DFT + U method predicts a dynamically unstable UN structure, in contradiction with experiments [6,10]. Researchers also assessed the performance of other new DFT approaches on UN simulation. Yang et al. calculated the magnetic and electronic properties of UN by different DFT approaches and concluded that conventional Generalized Gradient Approximation (GGA) functionals performed better than hybrid and meta-GGA functionals when studying the properties of UN [11].

Currently, simulation studies on the thermal conductivity of UN fuel are mainly focused on analyzing the thermal conductivity mechanism and calculating thermal physical quantities by calculation methods such as DFT and molecular dynamics (MD). For example, researchers used calculation methods such as DFT to study the electronic structure and transport properties of UN and compared the effect of electrons and phonons on the thermal conductivity, pointing out that the influence of electrons predominates in UN [12,13,14,15]. Kurosaki K et al. estimated the thermal conductivity of UN in the temperature range of 300~2000 K by molecular dynamics calculation [16]. Webb A J et al. evaluated the thermal conductivity of W-UN and W-UO₂ fuels by means of the Bruggeman–Fricke model and discussed its relationship with the particle geometry and fuel volume fraction [17].

During the processing of UN fuel, carbon atoms can be found in UN as one common type of impurity. The carbon atoms primarily come from the nuclear fuel raw materials and the graphite molds used in fuel processing. Previous investigations of UN show that the existence of carbon impurities may increase the creep and swelling of the fuel [9]. In addition, nuclear fuels are subjected to high temperatures, high pressures, and high radiation doses for a long time during the operational phase, and the crystal structure of the fuel is prone to various types of defects such as vacancy defects. The crystal defects may have a significant impact on the thermophysical properties of the fuel. However, current simulation studies on the effect of crystal defects and impurities on the thermal conductivity of UN are relatively few. Since Kocevski et al. reported that vacancies in UN had lower formation energies than interstitials, vacancies are chosen in this work to analyze their effect on thermal conductivity rather than interstitials [6].

In this study, an investigation of the effect of vacancy defects and carbon impurities on the thermal conductivity of UN is conducted based on the first-principles method and Boltzmann transport theory. The formation energy ($E_f$) and formation enthalpy ($\mathsf{\Delta }H$) are calculated to characterize the thermodynamic stability of the crystal structures. Further, the mechanical properties and thermal conductivity of different structures are calculated and compared.

2. Method

The structural optimization starts from the 8-atom UN cell with a face-centered cubic structure (FCC) (Figure 1). The simulation computations of this work are mainly conducted by the first-principles calculation software VASP (Vienna Ab-initio Simulation Package) version 6.2.1 [18,19,20,21]. The Generalized Gradient Approximation with the Perdew–Burke–Ernzerhof (GGA-PBE) functional is adopted for the exchange–correlation interaction [22]. Electrons within the ionic core are modeled with the projector augmented wave (PAW) pseudopotentials included in VASP [23,24]. The plane-wave kinetic energy cutoff is fixed at 500 eV. For all calculations, the Monkhorst–Pack scheme is used to sample the Brillouin zone (BZ), and a 3 × 3 × 3 k-point mesh is used. In the previous first-principles investigations on UN, the 2 × 2 × 2 supercell (64 atoms) was generally used for the defect calculation and was sufficient to avoid defect–defect interactions [1,6,8]. It was reported that for the PBE calculations, the fractional defect concentration difference between using a 2 × 2 × 2 supercell and using a 3 × 3 × 3 supercell was less than 10⁻⁷ [6]. Therefore, using a larger cell would not have a significant effect and the 2 × 2 × 2 supercell is used in our calculation. The cell structure is allowed to relax freely during the structural optimization. The energy and force convergence criteria are set to 10⁻⁵ eV and 0.01 eV/Å. Kocevski et al. reported that the GGA-PBE functional with and without spin-orbit coupling (SOC) gave similar lattice parameters, electronic properties, and phonon dispersions of UN; thus, SOC is not considered in this work [6].

When it comes to the magnetic property of UN, UN is an antiferromagnetic (AFM) material with a Néel temperature of 53 K, becoming paramagnetic above this temperature. It has been shown that setting UN to an antiferromagnetic or ferromagnetic (FM) material more accurately reflects its properties in simulation [6]. The magnetic moment of the U atom is set to 3 ${\mathsf{\mu }}_{\mathrm{B}}$ in this work, and the magnetism is set to AFM in the section on thermodynamic properties.

Researchers usually use the DFT + U method to characterize the properties of UN instead of conventional DFT because of the strong electron correlation caused by 5f valence electrons in the U atom. However, it has been reported that the DFT + U method, when employed in the study of the thermal conductivity of UN, has the tendency to result in the destabilization of the crystal structure during the calculation process, probably leading to the inability to obtain converged calculation results [6,10]. Therefore, in the calculations of structural and phonon properties in this work, the calculated results with and without the Hubbard U method are compared to verify that the Hubbard U method leads to the structural instability of UN cells. Subsequently, the Hubbard U method is not applied in the calculations of thermodynamic properties in this work.

In this work, the lattice parameter and cell volume of UN are firstly calculated by four methods: AFM, AFM + U, FM, and FM + U (“+U” means that the Hubbard U method is used). Dudarev’s rotationally invariant approach is used when using the DFT + U method in this work [25]. The parameter $U_{eff}=U-J$ is used to describe the Coulombic repulsion in the DFT + U calculation [7,8,9]. To determine the parameter $U_{eff}$, Lan et al. fixed the value of the exchange parameter $J=0.125 \mathrm{eV}$ and adjusted the value of $U_{eff}$ from 0 to 4 eV to describe the real ground state of UN. Their calculation results at $U_{eff}=1.85 \mathrm{eV}$ correctly describe the ground state and electronic structure of UN [8]. Therefore, the value for $U$ on U atom is set as 1.975 eV, which for $J$ is 0.125 eV in our work. The phonon spectra of UN crystals established by these four methods are calculated to analyze the structural stability using Phonopy [26,27]. The finite displacement method is employed to obtain the phonon properties. When discussing crystal defects in UN in this work, several kinds of typical point defects in the supercell are selected, including vacancies and carbon impurities (Figure 2). The carbon concentration is set to ~0.15 wt% (mass fraction). The thermodynamic stability of these defective systems is discussed by the calculated formation energy and formation enthalpy. The mechanical properties and thermal conductivity of these defective structures are calculated and compared with the ideal UN. Since the thermal conductivity of UN consists of two parts, the electronic thermal conductivity ${\kappa }_e$ and the lattice thermal conductivity ${\kappa }_l$, it is necessary to calculate the contributions of electrons and phonons to the thermal conductivity separately in the thermal conductivity calculation of UN [12]. Before calculating the electronic thermal conductivity, self-consistent calculation is performed using VASP. BoltzTraP2 is then used to analyze the electronic structure from the VASP output file, eventually generating the electronic thermal conductivities based on the Boltzmann transport theory of electrons [28]. By the Slack equation, the lattice thermal conductivity can be derived from mechanical properties obtained by VASPKIT software version 1.5.1 [3,29,30].

3. Results and Discussion

3.1. Structural Properties of Ideal UN

Firstly, the lattice parameters, system energies, and magnetic moments of the U atom of the UN unit cell are calculated by four methods: AFM, AFM + U, FM, and FM + U, compared with reference values (

Table 1. A comparison of the lattice parameters (a, b, and c), system energies, and magnetic moment of U atom of UN in this study and previous theoretical and experimental studies.

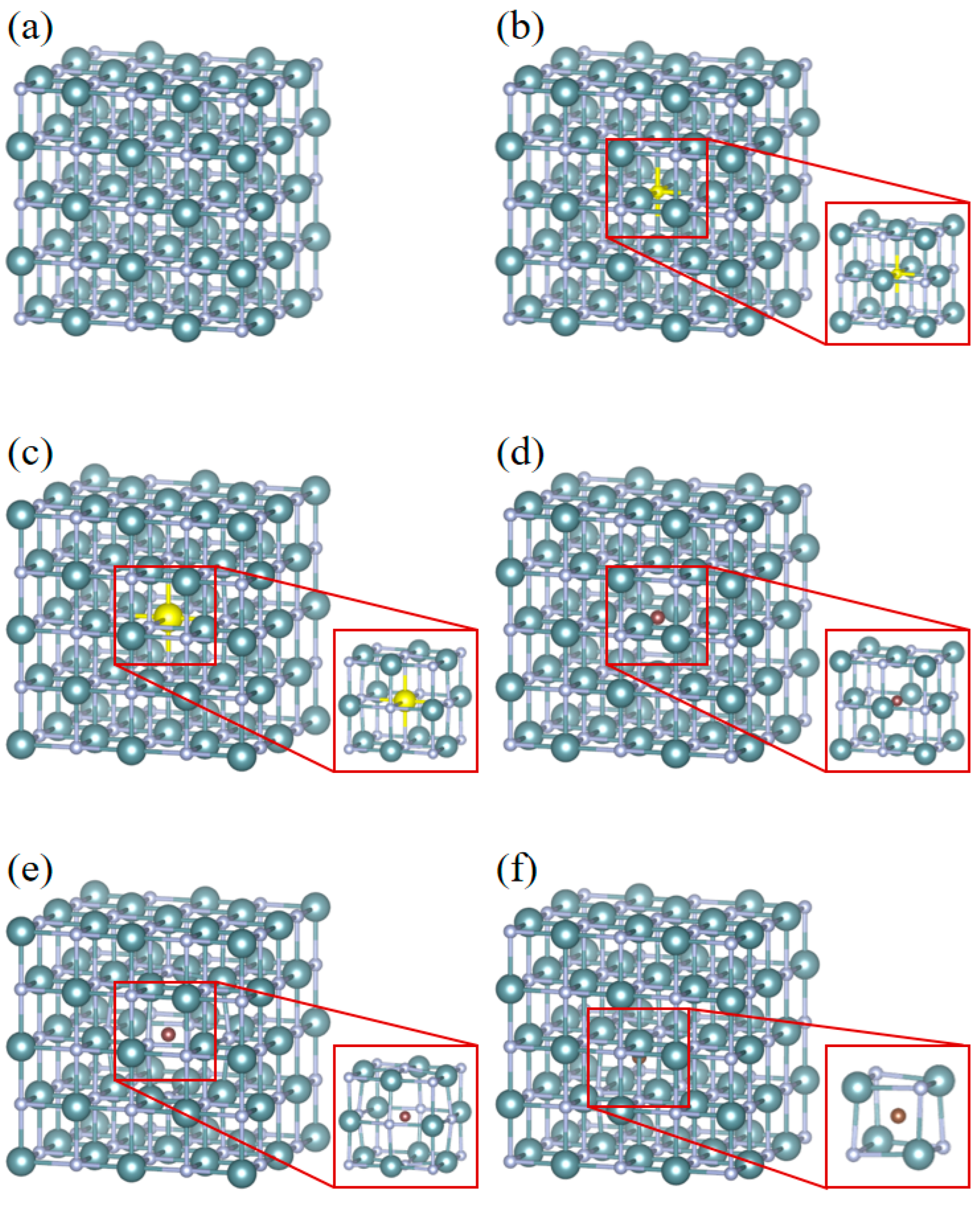
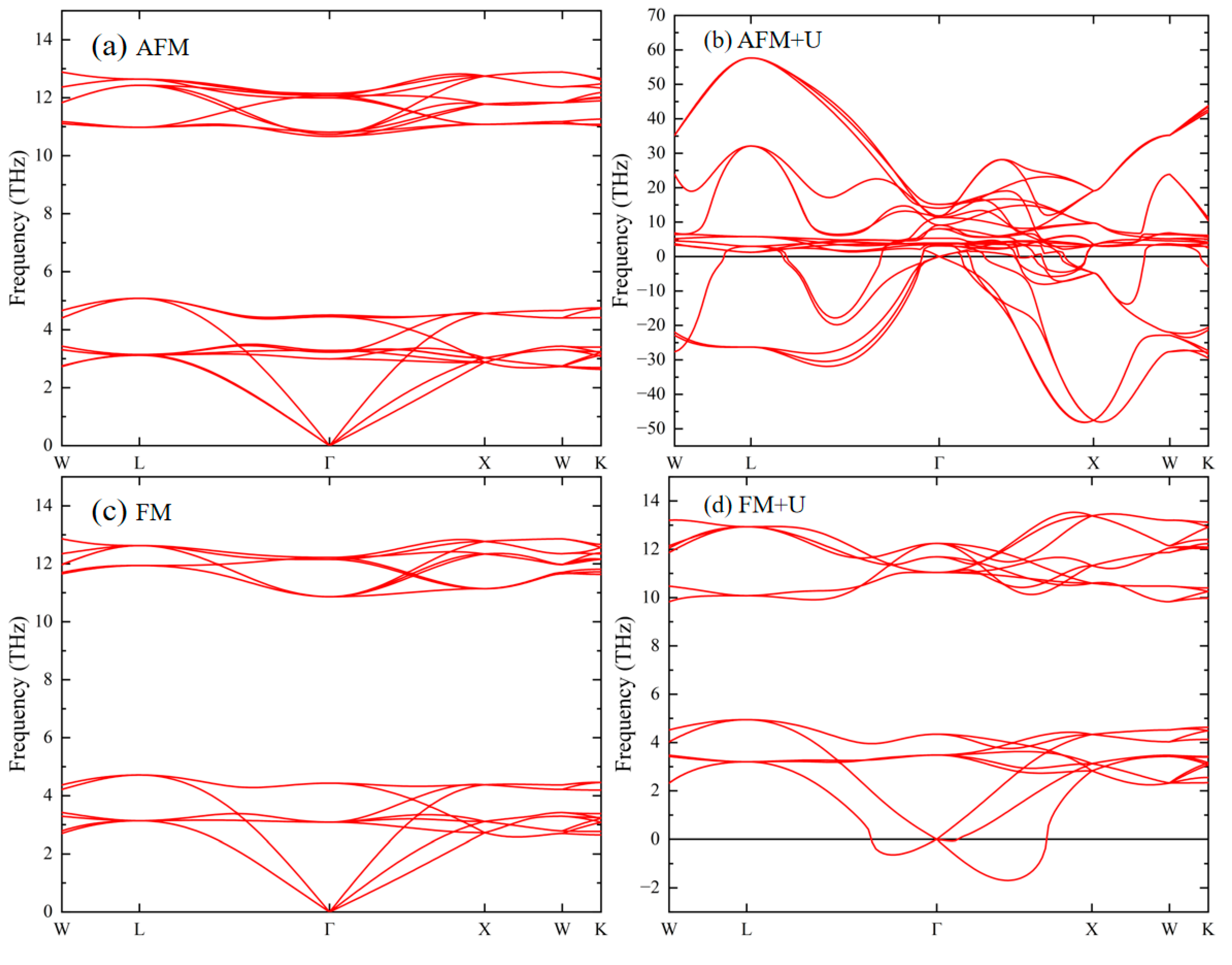
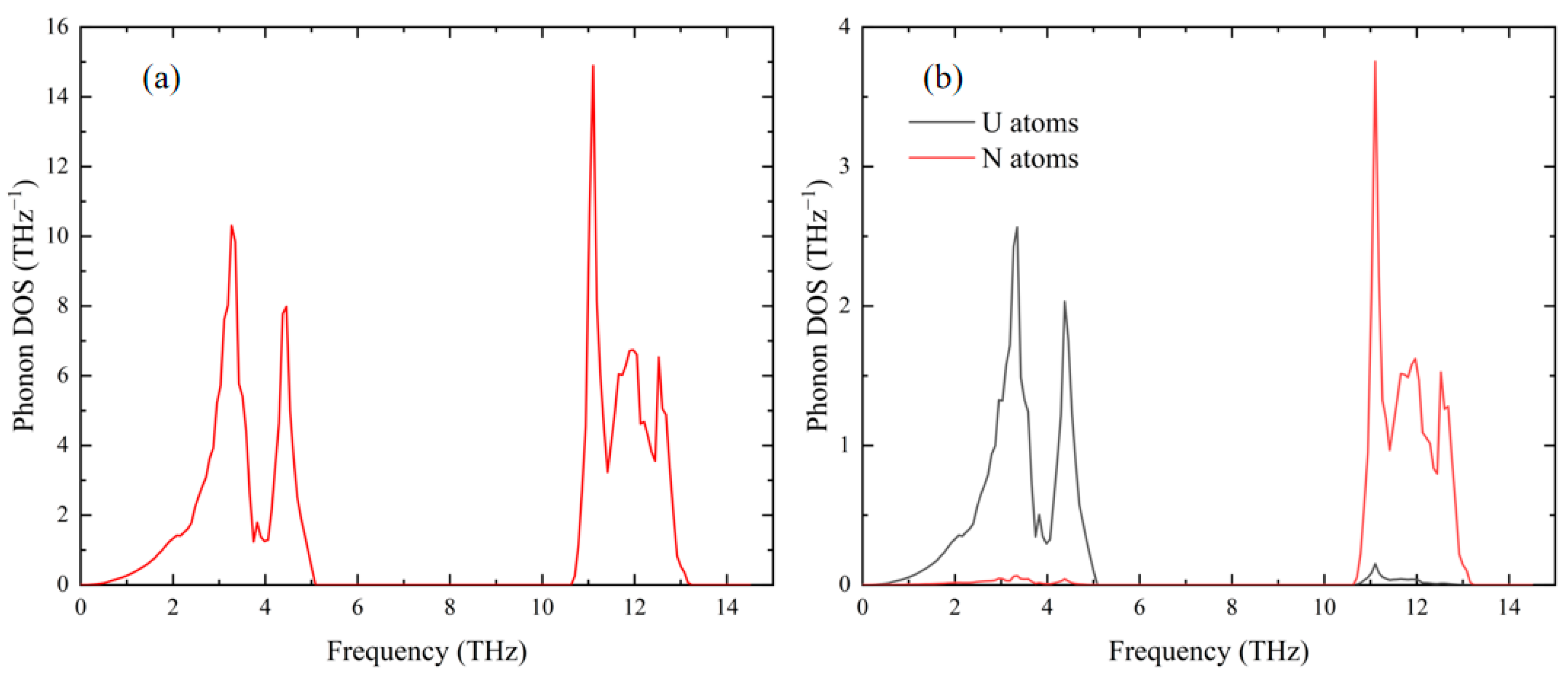
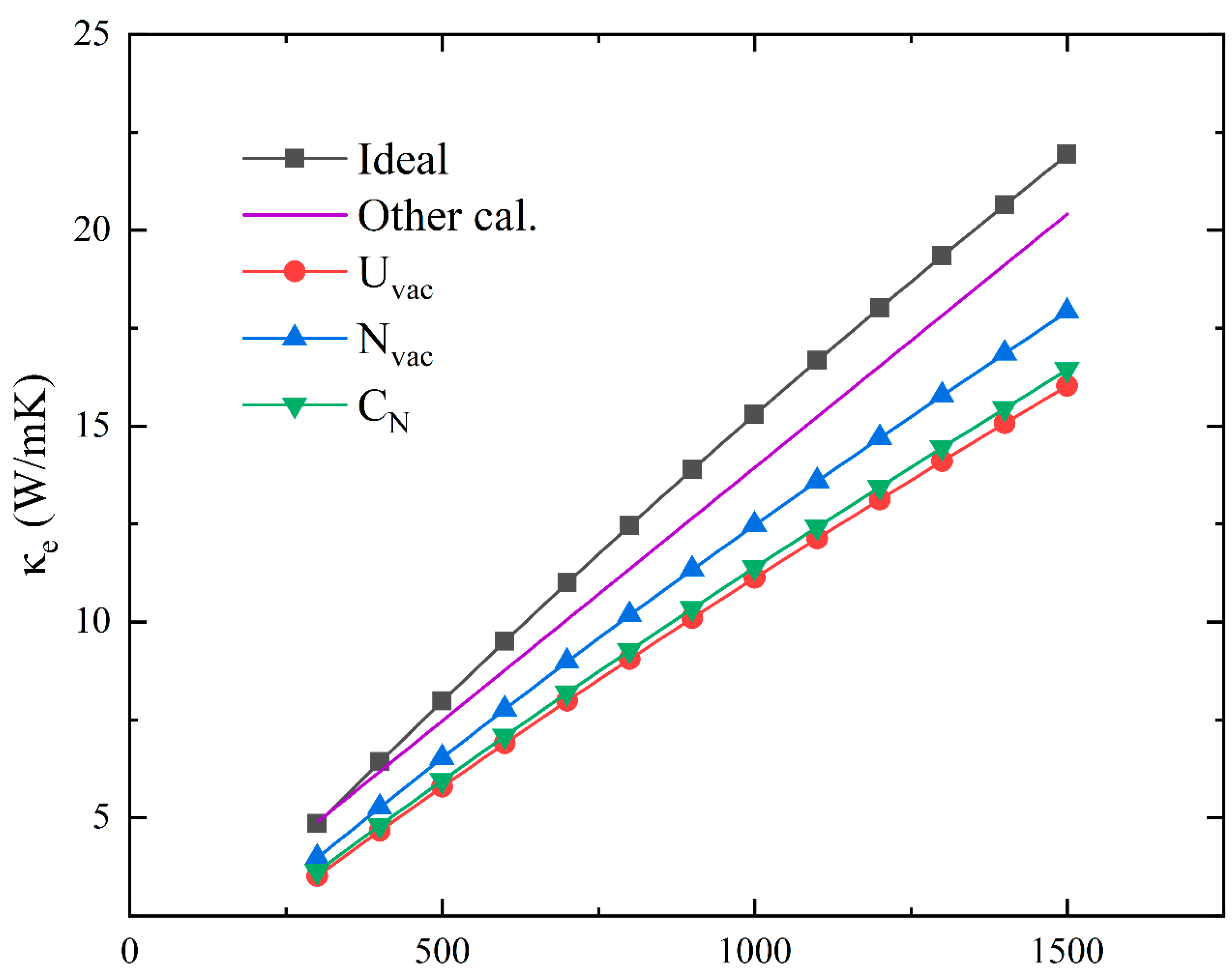
		Lattice Parameter (Å)	System Energy (eV/f.u.)	Magnetic Moment of U Atom (${\mathit{\mu }}_{\mathit{B}}$)
This study	AFM	4.858, 4.858, 4.882	−22.176	1.050
	AFM + U	4.962, 4.962, 4.848	−20.608	1.538
	FM	4.871	−22.229	1.347
	FM + U	4.920	−20.585	1.792
Other cal. [6]	AFM	4.853, 4.853, 4.872	/	0.99
	AFM + U	4.898, 5.009, 4.956	/	1.83
	FM	4.861	/	1.21
	FM + U	4.939, 4.940, 4.940	/	1.79
Other cal. [11]	AFM	4.865	/	1.05
	AFM + U	4.927	/	1.59
	FM	4.868	/	1.25
	FM + U	4.916	/	1.71
Exp. [32]		4.888	/	/

). The lattice parameters obtained by these four methods are close to the reference values from previous theoretical and experimental studies. The system energy of AFM and FM is slightly smaller than that of AFM + U and FM + U, indicating that UN unit cells modelled by AFM and FM are more stable than the latter two. The lattice constants a, b, and c of FM are equal, which shows that the high symmetry of the FCC structure is retained. However, the unit cells established by AFM are slightly distorted (a≠c), caused by the structural anisotropy generated by the AFM ordering of the U atom spins [9]. Goncharov et al. experimentally studied the local atomic structures of UN and confirmed the UN local environment distortion by the extended X-ray absorption fine structure (EXAFS) [31]. When the magnetism of UN is set as AFM, the obtained absolute values of magnetic moments of the U atom are larger than the value in the case of FM. When comparing the cases with or without the Hubbard U method, the former generate larger magnetic moments of the U atom.

3.2. Phonon Properties of Ideal UN

The phonon spectra of UN are shown in Figure 3, calculated along the W-L-Γ-X-W-K high symmetry k-vector path in the Brillouin zone by four methods: AFM, AFM + U, FM, and FM + U. The phonon spectra calculated by AFM and FM are free of imaginary frequencies, indicating that the structure is dynamically stable in these two cases. However, the presence of imaginary frequencies in the phonon spectra calculated by AFM + U and FM + U suggests that the structure is dynamically instable in these two cases, and this may lead to significant errors in the calculation of thermal conductivity [6,10]. Therefore, the DFT + U method was not applied in the following calculation in this work. As illustrated in Figure 3a, the highest frequency of the optical branch is 12.9 THz, while the highest frequency of the acoustic branch is 5.1 THz, which are consistent with previous calculations. The calculated optical phonon frequency at the Γ point is 12.1 THz, in line with the experimental value of 12.3 THz [33].

Figure 4 depicts the total and projected phonon density of states of UN. The band gap between 5.1 THz and 10.2 THz can be attributed to the significant mass disparity between the uranium and nitrogen atoms. As shown in Figure 4b, the projected phonon density of states reveals that the lattice vibrations of uranium atoms are predominantly concentrated in the low-frequency region between 0 and 5.1 THz, while those of nitrogen atoms are primarily distributed in the high-frequency region between 10.2 THz and 13.2 THz.

3.3. Energetics of Defect and Impurities

The formation energy of vacancies can be calculated by the following equation:

$$E_f=E_{UN\left(vac\right)}-E_{UN}+{\mu }_X$$

(1)

where $E_{UN\left(vac\right)}$ is the total energy of a UN unit cell with a U vacancy ($U_{vac}$) or a N vacancy ($N_{vac}$); $E_{UN}$ is the total energy of an ideal UN supercell; and ${\mu }_X$ is the chemical potential of U or N. A N₂ molecule and α-bulk U are taken as reference states to calculate the chemical potential [34,35]. The calculated ${\mu }_U$ and ${\mu }_N$ are −11.13 eV and −8.33 eV. The formation energy of $U_{vac}$ and $N_{vac}$ are 3.36 eV and 4.28 eV, which are close to the previous simulation work shown in

Table 2. The calculated formation energies of $U_{vac}$ and $N_{vac}$ compared with previous work.

	This Work (eV)	Other Cal. [11] (eV)	Other Cal. [35] (eV)
$U_{vac}$	3.36	3.17	3.74
$N_{vac}$	4.28	4.42	4.24

[35]. The $U_{vac}$ formation is slightly preferred (by 0.92 eV) over the $N_{vac}$ formation.

There are three possible occupational sites of a carbon atom in the UN lattice: a U site ($C_U$), N site ($C_N$), and interstitial site ($C_{int}$). To consider which site the carbon atoms prefer to occupy, it is necessary to compare the thermodynamic stability of UN with a carbon atom at different sites. The formation enthalpy ($\mathsf{\Delta }H$) can characterize the thermodynamic stability of UN structures with a carbon atom, which is given by the following [36]:

$$\mathsf{\Delta }\mathrm{H}={\displaystyle \frac{{\mathrm{E}}_{\mathrm{UN}}-{\mathrm{N}}_{\mathrm{U}}{\mathsf{\mu }}_{\mathrm{U}}-{\mathrm{N}}_{\mathrm{N}}{\mathsf{\mu }}_{\mathrm{N}}-{\mathrm{N}}_{\mathrm{C}}{\mathsf{\mu }}_{\mathrm{C}}}{{\mathrm{N}}_{\mathrm{U}}+{\mathrm{N}}_{\mathrm{N}}+{\mathrm{N}}_{\mathrm{C}}}}$$

(2)

where $N_U$, $N_N$, and $N_C$ are the number of U, N, and C atoms in the unit cell, and ${\mu }_U$, ${\mu }_N$, and ${\mu }_C$ correspond to the chemical potential of U, N, and C. Since the carbon impurities mainly come from the graphite molds [9], graphite is chosen as the reference state to calculate ${\mu }_C$, which results in −9.23 eV.

Table 3. The calculated formation enthalpies ($\mathsf{\Delta }H$) of UN structures with a carbon atom.

	$\mathsf{\Delta }\mathit{H}$ (eV/Atom)
$C_U$	−1.18
$C_N$	−1.29
$C_{int}$	−1.25

shows that when a carbon atom occupies the N site, $\mathsf{\Delta }H$ is negative and smaller than other cases, indicating that this structure has better thermodynamic stability. Lopes et al. calculated the incorporation energies ($E_I)$ of a carbon atom in the UN unit cell as follows [9]:

$${\mathrm{E}}_{\mathrm{I}}={\mathrm{E}}_{\mathrm{UN}\left(\mathrm{C}\right)}-{\mathrm{E}}_{\mathrm{UN}\left(\mathrm{vac}\right)}-{\mathsf{\mu }}_{\mathrm{X}}$$

(3)

The results showed that the $E_I$ of a carbon atom occupying the N site was the smallest among three cases [9]. The calculation of $\mathsf{\Delta }H$ reveals the influence of carbon impurities on the thermodynamic stability of UN. Further, the calculation of $E_I$ analyzes the physical process energetically by which the carbon atom occupies the vacancy. Both formulas indicate that carbon atoms prefer to occupy the N site. Thus, the other two structures with carbon atoms at the U site and interstitial site are not taken into account in the following calculations.

3.4. Mechanical Properties of Ideal and Defective UN

After structural optimization, the lattice volume changes of the defective UN are 0.21–0.58% relative to the ideal UN. To analyze the mechanical properties of ideal and defective UN, the elastic constants are calculated by the energy-strain method and the results are shown in

Table 4. The elastic constants of ideal and defective UN.

		${\mathit{C}}_{11}$ (GPa)	${\mathit{C}}_{12}$ (GPa)	${\mathit{C}}_{44}$ (GPa)
Ideal	This work	400.9	121.0	43.4
	Other cal. [33]	404.6	124.2	45.0
	Other cal. [38]	416.5	115.1	71.0
	Exp. [39]	423.9	98.1	75.7
$U_{vac}$		373.1	111.0	36.3
$N_{vac}$		371.6	116.5	39.4
$C_N$		409.3	123.9	45.7

. For the ideal UN, $C_{11}$, $C_{12}$, and $C_{44}$ are determined to be 400.9, 121.0, and 43.4 GPa, which are in line with previous calculations [33]. For the defective UN, elastic constants of $U_{vac}$ and $N_{vac}$ are all reduced as compared with the cases of the ideal UN; however, the elastic constants of $C_N$ are increased, suggesting that vacancies and carbon impurities affect the mechanical properties of UN to a certain degree. Calculated elastic constants of all structures can satisfy the Born stability criterion (Equation (4)) [37], indicating that these structures are mechanically stable.

$$C_{11}-C_{12}>0,C_{11}>0,C_{44}>0$$

(4)

After obtaining the elastic constants, the bulk modulus B, shear modulus G, Young’s modulus E, and Poisson’s ratio $\nu$ of UN are calculated by using the Voigt–Reuss–Hill approximation [40,41,42], and the results are summarized in

Table 5. The bulk modulus B, shear modulus G, Young’s modulus E, and Poisson’s ratio $\nu$ of ideal and defective UN.

		B (GPa)	G (GPa)	E (GPa)	$\mathit{\nu }$
Ideal	This work	216.6	69.8	189.2	0.354
	Other cal. [33]	217.7	72.4	195.1	0.350
	Exp. [39]	205.9	103.9	229	0.263
	Exp. [47]	194	/	/	/
$U_{vac}$		201.2	62.0	168.6	0.360
$N_{vac}$		203.4	63.4	172.3	0.359
$C_N$		220.4	72.8	196.8	0.351

. The results are found to agree well with previous calculations [33]. The bulk modulus B, shear modulus G, and Young’s modulus E characterize the ability to withstand compression, reversible deformation resistance under shear stress, and the stiffness of materials, respectively [36,43,44]. Compared with the ideal UN, the elastic moduli of $U_{vac}$ and $N_{vac}$ are all reduced, while the cases of $C_N$ are slightly increased. The results suggest that vacancies result in the lowering of resistance towards deformation and stiffness. On the contrary, ~0.15 wt% carbon impurities can improve these properties. Poisson’s ratio $\nu$ is a dimensionless parameter for characterizing the ductility of materials, and a higher $\nu$ represents stronger ductility [36]. The results show that unit cells with vacancies are more ductile than the ideal one, while the existence of carbon impurities weakens the ductility. Li, M. et al. researched the effects of point defects on the mechanical properties of U₃Si₂, and a similar phenomenon was reported; lattice point defects led to a decrease in the elastic moduli [45]. The vacancies reduce the bond density and eventually weaken the mechanical properties of the material. It is hypothesized that carbon atoms enhance the material by increasing the bond strength of UN and then improving its resistance to deformation [46].

3.5. Thermal Transport Properties of Ideal and Defective UN

After calculating the mechanical properties, the longitudinal ($v_l$) and transverse ($v_t$) sound velocities of materials can be calculated by using the bulk modulus B and shear modulus G; the equations are as follows [3]:

$$v_l={\left({\displaystyle \frac{B+\frac{4}{3}G}{\rho }}\right)}^{{\displaystyle \frac{1}{2}}},v_t={\left({\displaystyle \frac{G}{\rho }}\right)}^{{\displaystyle \frac{1}{2}}}$$

(5)

where $\rho$ is the mass density. The average sound wave velocity ($v_a$) is given as follows [3]:

$${\nu }_{\mathrm{a}}={\left[{\displaystyle \frac{1}{3}}\left({\displaystyle \frac{1}{{\nu }_l^3}}+{\displaystyle \frac{2}{{\nu }_t^3}}\right)\right]}^{-{\displaystyle \frac{1}{3}}}$$

(6)

The Debye temperature $\Theta$ is an important thermophysical parameter, which can be thought of as the temperature above which all vibrational modes in a crystal are excited, and can be derived as follows [3,29]:

$$\Theta ={\displaystyle \frac{h}{k_{\mathrm{B}}}}{\left({\displaystyle \frac{3n}{4\pi \Omega }}\right)}^{{\displaystyle \frac{1}{3}}}{\nu }_{\mathrm{a}}$$

(7)

where $h$, $k_{\mathrm{B}}$, and $\Omega$ are the Planck constant, Boltzmann constant, and volume of the unit cell, respectively. $n$ is the number of atoms in a primitive cell. The Grüneisen parameter $\gamma$ is used for describing the relationship between the lattice thermal vibration, heat capacity, and bulk expansion, and can be given by the following formula [3,29]:

$$\gamma ={\displaystyle \frac{9-12{\left(\frac{{\nu }_{\mathrm{t}}}{{\nu }_{\mathrm{l}}}\right)}^2}{2+4{\left(\frac{{\nu }_{\mathrm{t}}}{{\nu }_{\mathrm{l}}}\right)}^2}}$$

(8)

The lattice thermal conductivity ${\kappa }_l$ can be derived from the Slack equation [3,29]:

$${\kappa }_l=A{\displaystyle \frac{\overline{M}{\Theta }^3\delta }{{\gamma }^2{n}^{2/3}T}}$$

(9)

where $\overline{M}$, ${\delta }^3$, and $T$ are the average atomic mass, average volume per atom, and temperature, respectively. The dimensionless parameter $A$ is a function of the Grüneisen parameter $\gamma$ and is approximately equal to 3.04 × 10⁻⁸ as $\gamma \approx 2$.

The calculated thermal transport properties of the ideal and defective UN are summarized in

Table 6. The longitudinal sound velocity $v_l$, transverse sound velocity $v_t$, average sound velocity $v_a$, Grüneisen parameter $\gamma$, and Debye temperature $\Theta$ of ideal and defective UN.

		${\mathit{v}}_{\mathit{l}}$ (m/s)	${\mathit{v}}_{\mathit{t}}$ (m/s)	${\mathit{v}}_{\mathit{a}}$ (m/s)	$\mathit{\gamma }$	$\mathit{\Theta }$ (K)
Ideal	This work	4616.47	2192.11	2466.10	2.17	301.79
	Exp. [32,39]	4378	2507	/	1.98	282
$U_{vac}$		4473.05	2089.93	2353.04	2.22	287.01
$N_{vac}$		4447.95	2087.29	2349.56	2.21	286.33
$C_N$		4670.15	2236.47	2514.90	2.14	307.98

. The calculated $v_l$, $v_t$, $\gamma$, and $\Theta$ of the ideal UN are 4616.47 m/s, 2192.11 m/s, 2.17, and 301.79 K, respectively, while the experimental values are 4378 m/s, 2507 m/s, 1.98, and 282 K [32,39]. For $U_{vac}$ and $N_{vac}$, the Grüneisen parameter $\gamma$ is slightly increased compared to the ideal UN, indicating that phonon anharmonicity becomes enhanced on account of vacancies. In the meantime, the Debye temperature $\Theta$ is decreased, which suggests that the bonding interaction in the structure is weakened by vacancies [3]. However, for $C_N$, the change in thermal transport properties is contrary to $U_{vac}$ and $N_{vac}$, which means that ~0.15 wt% carbon impurities can advance the contribution of phonons to thermal transport. This phenomenon can be explained by an analysis of the chemical bonds, revealing that the C atom forms a very directional chemical bonding with the nearest U atoms and tends to rebuild the octahedral coordination, according to Lopes et al. [9].

Figure 5 shows the lattice thermal conductivities ${\kappa }_l$ of the ideal and defective UN as a function of temperature. The calculated ${\kappa }_l$ of the ideal and defective UN decreases as the temperature rises, which is due to the fact that the increasing temperature promotes the phonon–phonon scattering [12]. For the ideal UN, the calculated value of ${\kappa }_l$ is comparable to the experimental value, in the range of 400 K to 1000 K [48]. But the theoretical ${\kappa }_l$ of 11.43 W/mK at 300 K is larger than the experimental value of ~8 W/mK, resulting from the fact that acoustic–optical phonon scattering and optical–optical phonon scattering are not taken into account in this computing method [3]. For $U_{vac}$ and $N_{vac}$, the theoretical ${\kappa }_l$ is reduced by 18.8% and 16.0% in comparison to the ideal UN at the same temperature, on account of the phonon-defect scattering caused by vacancies. But for $C_N$, the calculated ${\kappa }_l$ is increased by 8.9% as a result of the weakened phonon anharmonicity.

3.6. Electronic Thermal Conductivity of Ideal and Defective UN

The electronic thermal conductivity ${\kappa }_e$ obtained by BoltzTrap2 is the ratio of the electronic thermal conductivity to the electron relaxation time (${\kappa }_e/\tau$). Therefore, it is necessary to figure out the electron relaxation time $\tau$ for calculating ${\kappa }_e$. The approximate value of $\tau$ (5.02 × 10⁻¹⁵ s) can be obtained by combining the experimental electrical resistivity at 300 K (148.8 $\mathsf{\mu }\mathsf{\Omega }·\mathrm{cm}$) with the calculated electrical conductivity in this study ($\sigma /\tau$) [48], which is close to reference value (4.98 × 10⁻¹⁵ s) from a previous theoretical study [5]. Due to the complex effect of defects on the electron relaxation time $\tau$, it is difficult to directly figure out the electron relaxation time $\tau$ of the defective UN, which is approximated as the result in the ideal case in this work.

As shown in Figure 6, the electronic thermal conductivities ${\kappa }_e$ of the ideal and defective UN increase with increasing temperature. This phenomenon can primarily be attributed to the fact that the increasing temperature enhances electronic transport, thereby facilitating the contribution of electrons to thermal transport [5]. The calculated ${\kappa }_e$ of the ideal UN is basically in line with reference data calculated from the experimental electrical resistivity via the Wiedemann–Franz law [15]. The ${\kappa }_e$ of ideal UN is calculated to be 4.84 W/mK at 300 K and 21.93 W/mK at 1500 K. For the defective UN, the ${\kappa }_e$ of $U_{vac}$, $N_{vac}$, and $C_N$ are, respectively, reduced by 27.0%, 18.2%, and 25.0% compared to the ideal case. A similar phenomenon was reported by Qi, H. et al.; Xe and Cs impurities reduced the ${\kappa }_e$ of U₃Si₂ [3]. This is caused by the destruction of structural symmetry, which enhanced the scattering of conduction electrons on lattice defects and impurities. The existence of vacancies and carbon atoms reduces the electron mobility and hence weakens electronic thermal conduction [12].

3.7. Total Thermal Conductivity of Ideal and Defective UN

Combining the results of lattice thermal conductivity ${\kappa }_l$ and electronic thermal conductivity ${\kappa }_e$, the total thermal conductivity ${\kappa }_{tot}$ of the ideal and defective UN in the temperature range of 300 K to 1500 K can be obtained, as shown in Figure 7. For the ideal UN, the ${\kappa }_{tot}$ is calculated to be 18.73 W/mK at 1000 K. When the temperature exceeds 500 K, the ${\kappa }_{tot}$ of the ideal and defective UN shows a roughly linear increase, resulting from the fact that the electronic thermal conductivity ${\kappa }_e$ forms the bulk of ${\kappa }_{tot}$ at a high temperature and increases roughly linearly with the temperature rise. And the calculated ${\kappa }_{tot}$ of the ideal UN is in excellent agreement with the experimental result over 500 K [48]. Nevertheless, the ${\kappa }_{tot}$ of the ideal UN is larger than the reference value in the low temperature range of 300 K to 500 K. This is mainly because acoustic–optical phonon scattering and optical–optical phonon scattering are not taken into account in the calculation of ${\kappa }_l$, which leads to an overestimation of ${\kappa }_l$ at a low temperature [3]. Meanwhile, since the ${\kappa }_l$ decreases significantly with an increasing temperature, there is a slight decline in the calculated ${\kappa }_{tot}$ of both the ideal and defective UN in the temperature range of 300 K to 500 K, in contrast to the upward trend of the experimental value [48].

For $U_{vac}$, $N_{vac}$, and $C_N$, the total thermal conductivity ${\kappa }_{tot}$ is, respectively, reduced to 13.91 W/mK, 15.36 W/mK, and 15.14 W/mK at 1000 K compared to the ideal UN. This phenomenon can be explained by the additional phonon-defect scattering and electron-defect scattering [12]. But it is noted that the variation in ${\kappa }_{tot}$ in the case of $C_N$ is extremely small at 300 K after ~0.15 wt% carbon impurity doping, due to the increased lattice thermal conductivity ${\kappa }_l$. The ${\kappa }_{tot}$ of $C_N$ is higher than $N_{vac}$ at a low temperature but becomes lower than $N_{vac}$ at a high temperature. This is because the advantage in the phonon thermal conductivity of $C_N$ is weakened after the temperature increases. The result of $U_{vac}$ is the lowest among three defect cases. This is because the destruction of the structural symmetry by $U_{vac}$ is more significant in comparison with other cases, enhancing the phonon-defect scattering and electron-defect scattering even more and leading to the lowest thermal conductivity.

Compared to traditional UO₂ fuel, the total thermal conductivity ${\kappa }_{tot}$ of idea UN (18.73 W/mK) is higher than the experimental value of UO₂ at 1000 K (3.68 W/mK) [2]. Meanwhile, the calculated results in three defective cases are still higher than UO₂. The higher thermal conductivity of UN indicates that heat can be expelled faster than UO₂ from the fuel pellet in operation or during accidents [2,3,4]. Moreover, the thermal conductivity of UO₂ decreases with increasing temperature, while that of UN shows an increasing trend. The difference in thermal conductivity with temperature further reflects the advantage of UN fuel in thermal transport properties at a high temperature [5].

4. Conclusions

In this work, density functional theory and Boltzmann transport theory were employed to conduct a computational analysis on the effect of vacancy defects and carbon impurities on the thermal conductivity of UN. The structural properties and phonon properties of UN cells were investigated firstly. The calculated formation energies of vacancies showed that the $U_{vac}$ formation is slightly preferred over the $N_{vac}$ formation. The computed formation enthalpies indicate that the carbon atom was energetically favorable at the N site. The elastic constants and elastic modulus were calculated to evaluate the defective effect on the mechanical properties of UN.

The computational study of thermal conductivity reveals that both $U_{vac}$ and $N_{vac}$ lead to the decrease in the lattice thermal conductivity ${\kappa }_l$ and electronic thermal conductivity ${\kappa }_e$. For $C_N$, there is also a decline of 25.0% in the electronic thermal conductivity ${\kappa }_e$ in comparison to the ideal UN. However, the calculated lattice thermal conductivity ${\kappa }_l$ of $C_N$ is higher than the ideal case. The calculation of the Grüneisen parameter $\gamma$ and Debye temperature $\Theta$ shows that the bonding interaction in the structure is promoted after the carbon atom occupying the N site, thereby enhancing the contribution of phonons to thermal transport. The total thermal conductivities ${\kappa }_{tot}$ of $U_{vac}$, $N_{vac}$, and $C_N$ are, respectively, reduced by 25.7%, 18.0%, and 19.2% at 1000 K. This work could provide theoretical support for evaluating the mechanical and thermal performance of UN.