Design, Simulation and Experimental Study of the Linear Magnetic Microactuator
Abstract
1. Introduction
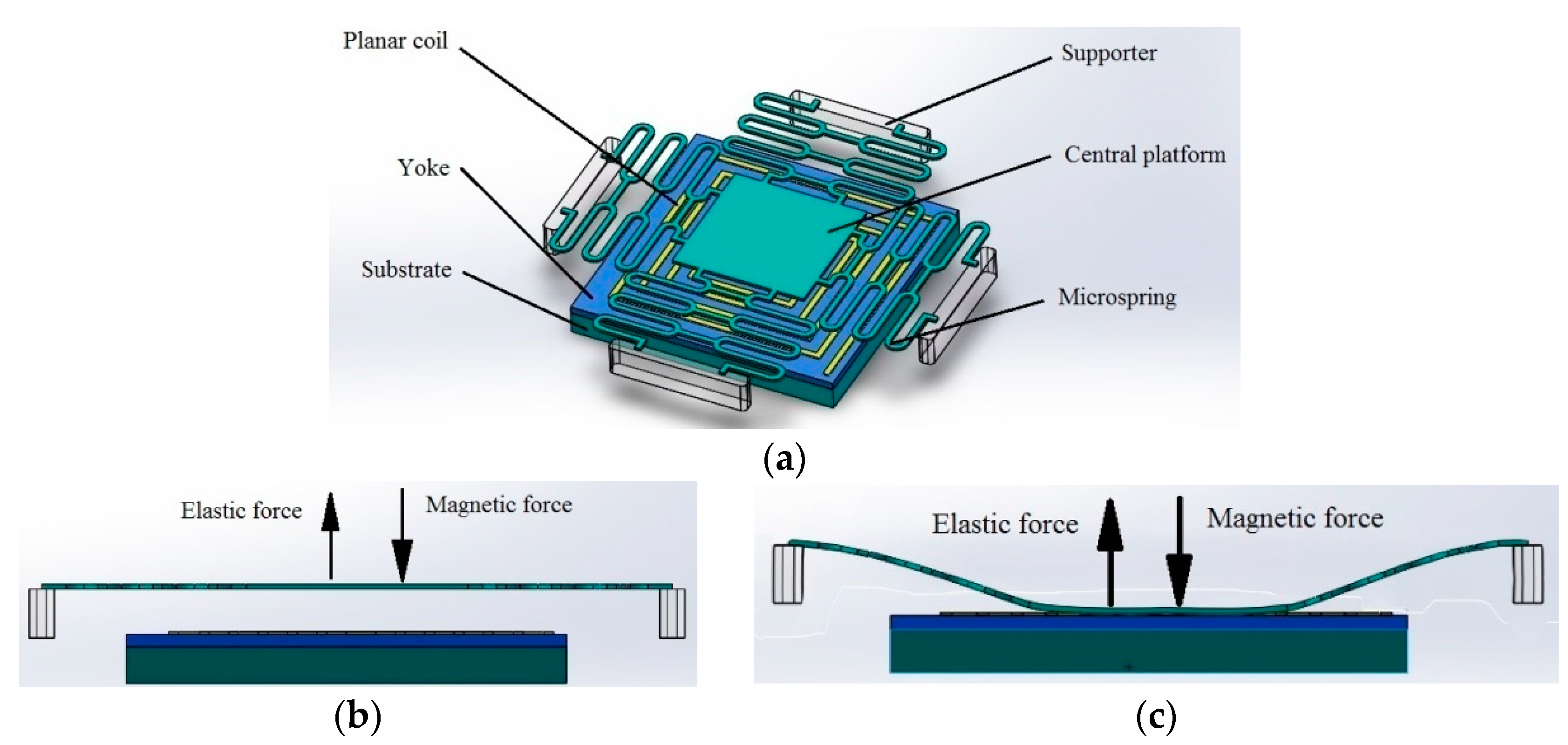
2. Structural Design of the Linear Magnetic Microactuator
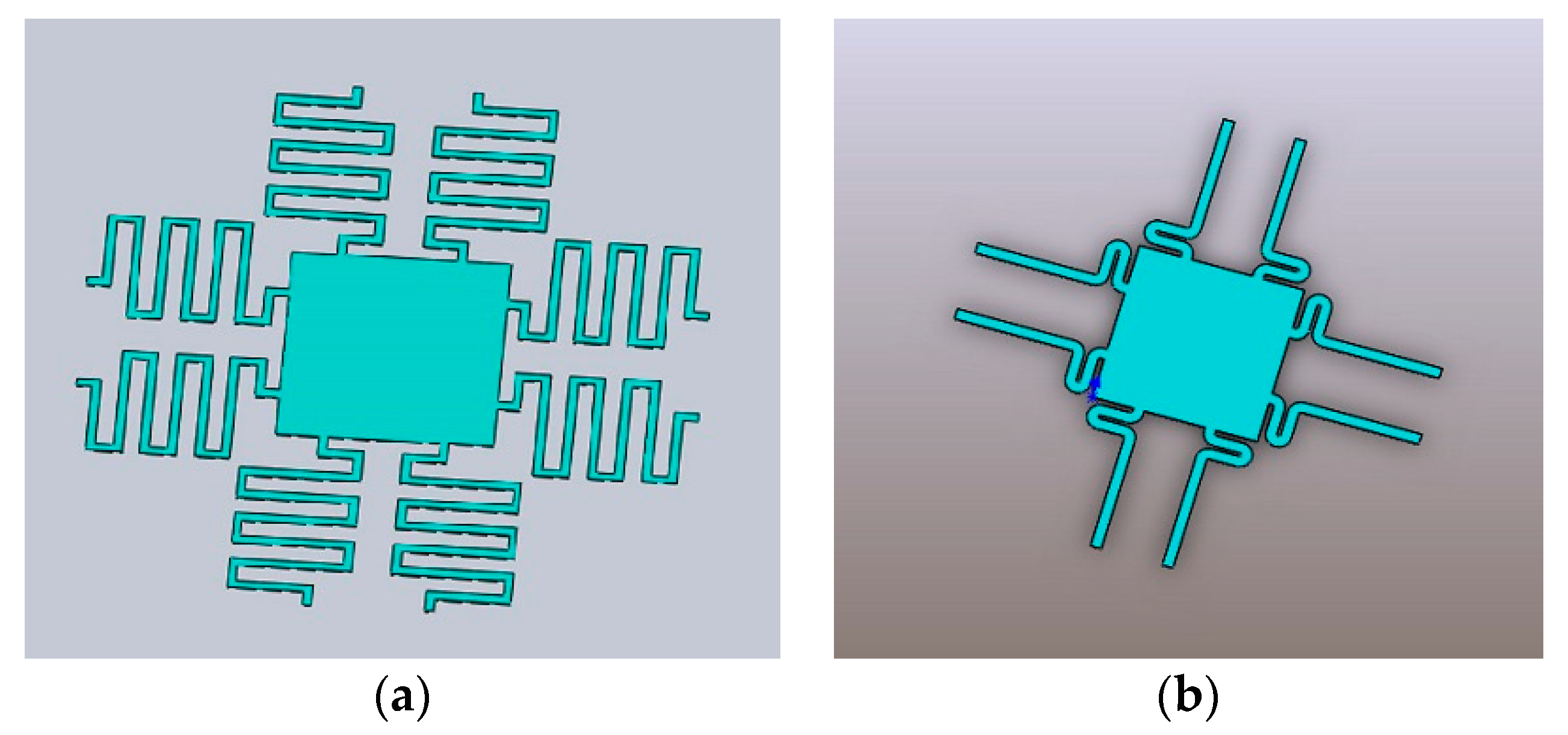
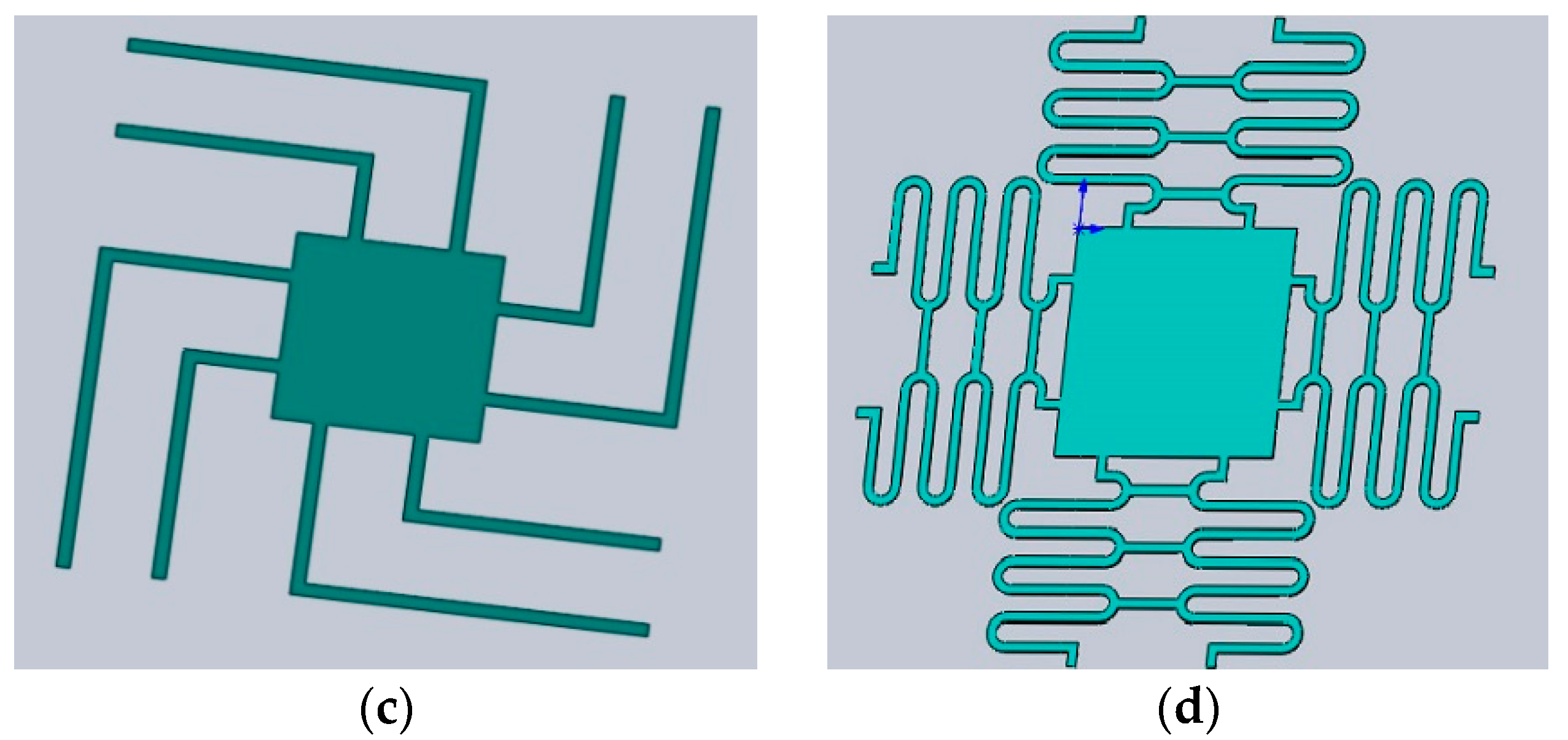
3. Topology Design and Simulation of the Microspring
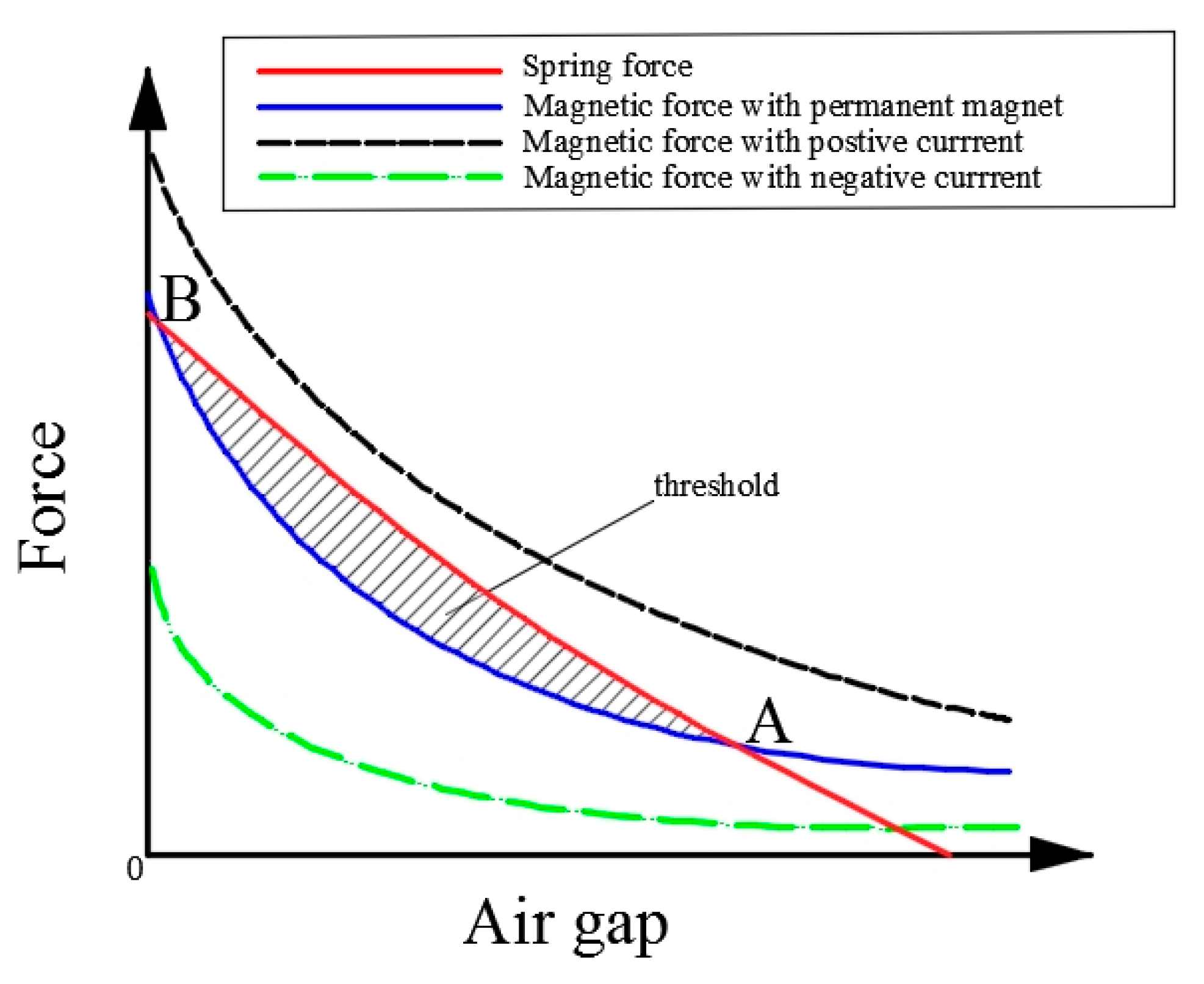
4. Simulation of the Bistable Mechanism of the Microactuator
5. Fabrication and Testing of the Bistable Microactuator
6. Conclusions
Author Contributions
Acknowledgments
Conflicts of Interest
References
- Judy, J.W. Microelectromechanical systems (MEMS): Fabrication, design and applications. Smart Mater. Struct. 2001, 10, 1115–1134. [Google Scholar] [CrossRef]
- Tanaka, M. An industrial and applied review of new MEMS devices features. Microelectron. Eng. 2007, 84, 1341–1344. [Google Scholar] [CrossRef]
- Chung, M.J.; Yee, Y.H.; Ahn, W.H. Development of compact camera module having auto focus actuator and mechanical shutter system for mobile phone. In Proceedings of the 2007 International Conference on Control, Automation and Systems, Seoul, Korea, 17–20 October 2007. [Google Scholar]
- Liu, C.S.; Ko, S.S.; Lin, P.D. Experimental characterization of high-performance miniature auto-focusing VCM actuator. IEEE Trans. Magn. 2011, 47, 738–745. [Google Scholar] [CrossRef]
- Tao, K.; Tang, L.; Wu, J.; Lye, S.W.; Chang, H.; Miao, J. Investigation of multimodal electret-based MEMS energy harvester with impact-induced nonlinearity. J. Microelectromech. Syst. 2018, 27, 276–288. [Google Scholar] [CrossRef]
- Wang, P.; Liu, R.; Ding, W.; Zhang, P.; Pan, L.; Dai, G.; Zou, H.; Dong, K.; Xu, C.; Wang, Z.L. Complementary electromagnetic-triboelectric active sensor for detecting multiple mechanical triggering. Adv. Funct. Mater. 2018, 28, 1705808. [Google Scholar] [CrossRef]
- Krebs, G.; Tounzi, A.; Pauwels, B.; Willemot, D.; Piriou, F. Modeling of a linear and rotary permanent magnet actuator. IEEE Trans. Magn. 2008, 44, 4357–4360. [Google Scholar] [CrossRef]
- Jin, P.; Yuan, Y.; Jian, G.; Lin, H.; Fang, S.; Yang, H. Static characteristics of novel air-cored linear and rotary Halbach permanent magnet actuator. IEEE Trans. Magn. 2014, 50, 977–980. [Google Scholar] [CrossRef]
- Chen, H.; Pallapa, M.; Sun, W.J.; Sun, Z.D.; Yeow, J.T.W. Nonlinear control of an electromagnetic polymer MEMS hard-magnetic micromirror and its imaging application. J. Micromech. Microeng. 2014, 24, 045004. [Google Scholar] [CrossRef]
- Bryzek, J.; Abbott, H.; Flannery, A.; Cagle, D.; Maitan, J. Control issues for MEMS. In Proceedings of the 42nd IEEE conference on Decision and Control, Maui, HI, USA, 9–12 December 2003. [Google Scholar]
- Peng, Y.; Cao, J.; Guo, Z.; Yu, H. A linear actuator for precision position of dual objects. Smart Mater. Struct. 2015, 24, 125039. [Google Scholar] [CrossRef]
- Borovic, B.; Hong, C.; Zhang, X.M.; Liu, A.Q.; Lewis, F.L. Open vs. closed-loop control of the MEMS electrostatic comb drive. In Proceedings of the 2005 IEEE International Symposium on Mediterrean Conference on Control and Automation Intelligent Control, Limassol, Cyprus, 27–29 June 2005. [Google Scholar]
- Yu, S.; Jang, K.; Cha, S.; Lee, Y.; Kwon, O.; Kwon, K.; Choi, J. A piezoelectric actuator driver circuit for automatic focusing of mobile phone cameras. In Proceedings of the2008 IEEE International Symposium on Circuits and Systems, Seattle, WA, USA, 18–21 May 2008. [Google Scholar]
- Park, C.; Cha, S.; Lee, Y.; Kwon, O.; Park, D.; Kwon, K.; Lee, J. A highly accurate piezoelectric actuator driver IC for auto-focus in camera module of mobile phone. In Proceedings of the 2010 IEEE International Symposium on Circuits and Systems, Paris, France, 30 May–2 June 2010. [Google Scholar]
- Watanabe, Y.; Kobayashi, S.; Iwamatsu, S.; Yahagi, T.; Sato, M.; Oizumi, N. Motion monitoring of MEMS actuator with electromagnetic induction. Electron. Commun. Jpn. 2014, 97, 52–57. [Google Scholar] [CrossRef]
- Cheng, H.D.; Hsiao, S.Y.; Wu, M.; Fang, W. Monolithic Bi-directional linear microactuator for light beam manipulation. In Proceedings of the IEEE/LEOS International Conference on Optical MEMS and Their Applications Conference, Big Sky, MT, USA, 21–24 August 2006. [Google Scholar]
- Choi, Y.M.; Gorman, J.J.; Dagalakis, N.G.; Yang, S.H.; Kim, Y.; Yoo, J.M. A high-bandwidth electromagnetic MEMS motion stage for scanning applications. J. Micromech. Microeng. 2012, 22, 105012. [Google Scholar] [CrossRef]
- Braune, S.; Liu, S. A novel linear actuator for variable valve actuation. In Proceedings of the 2005 IEEE International Conference on Industrial Technology, Hong Kong, China, 14–17 December 2005; pp. 377–382. [Google Scholar]
- Ruffert, C.; Gehrking, R.; Ponick, B.; Gatzen, H.H. Magnetic levitation assisted guide for a linear micro-actuator. IEEE Trans. Magn. 2006, 42, 3785–3787. [Google Scholar] [CrossRef]
- Sun, S.; Dai, X.; Wang, K.; Xiang, X.; Ding, G.; Zhao, X. Nonlinear electromagnetic vibration energy harvester with closed magnetic circuit. IEEE Magn. Lett. 2018, 9, 1–4. [Google Scholar] [CrossRef]
- Dai, X.; Miao, X.; Sui, L.; Zhou, H.; Zhao, X.; Ding, G. Tuning of nonlinear vibration via topology variation and its application in energy harvesting. Appl. Phys. Lett. 2012, 100, 031902. [Google Scholar] [CrossRef]
- Miao, X.; Dai, X.; Huang, Y.; Ding, G.; Zhao, X. Segmented magnetic circuit simulation of the large displacement planar micro-coil actuator with enclosed magnetic yokes. Microelectron. Eng. 2014, 129, 38–45. [Google Scholar] [CrossRef]
- Sun, S.; Dai, X.; Sun, Y.; Xiang, X.; Ding, G.; Zhao, X. MEMS-based wide-bandwidth electromagnetic energy harvester with electroplated nickel structure. J. Micromech. Microeng. 2017, 27, 115007. [Google Scholar] [CrossRef]
- Luharuka, R.; LeBlanc, S.; Bintoro, J.S.; Berthelot, Y.H.; Hesketh, P.J. Simulated and experimental dynamic response characterization of an electromagnetic valve. Sens. Actuators A 2008, 143, 399–408. [Google Scholar] [CrossRef]
- Wagner, B.; Benecke, W. Microfabricated actuator with moving permanent magnet. In Proceedings of the 1991 IEEE Micro Electro Mechanical Systems, Nara, Japan, 30 December 1990–2 January 1991. [Google Scholar]
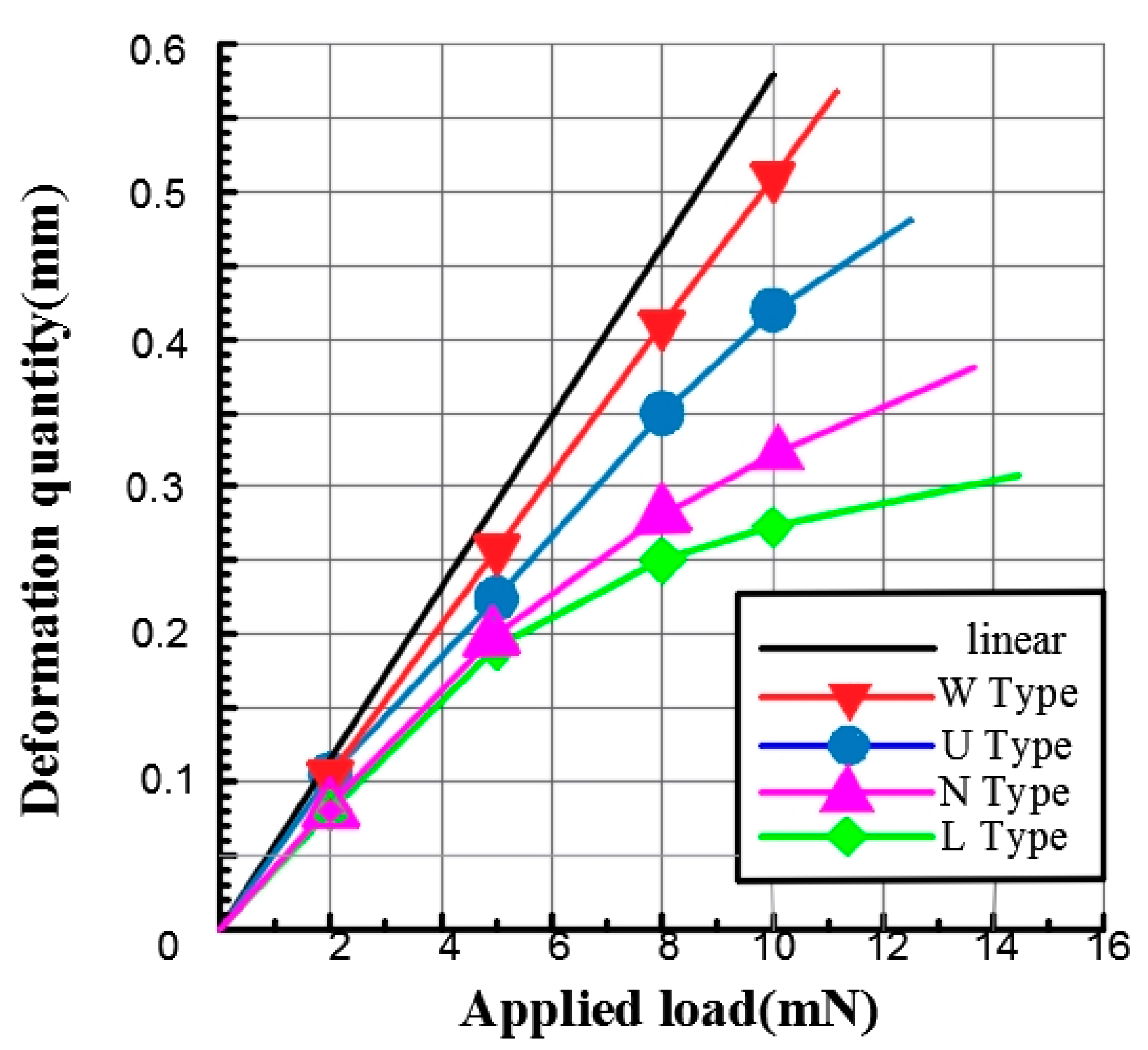
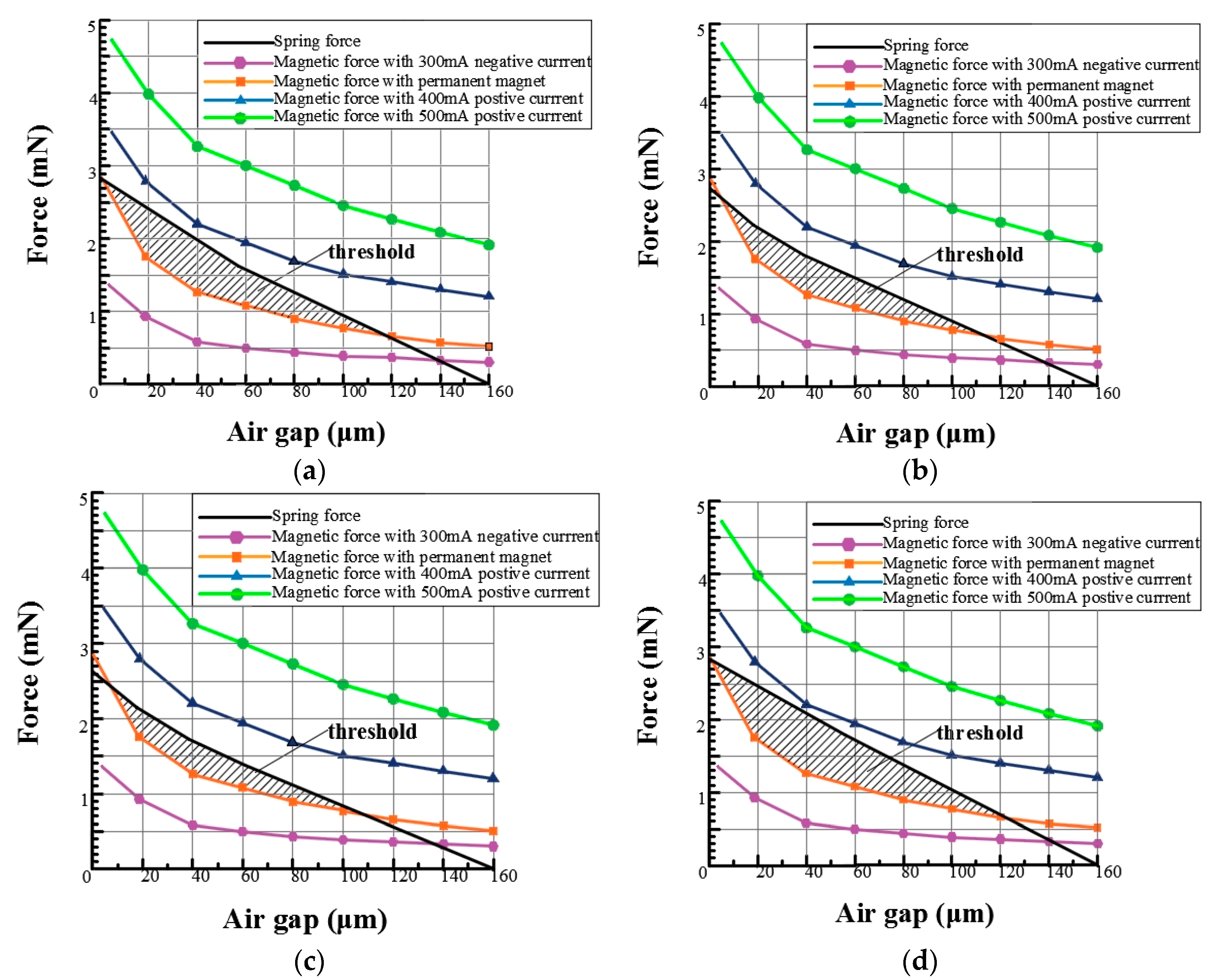
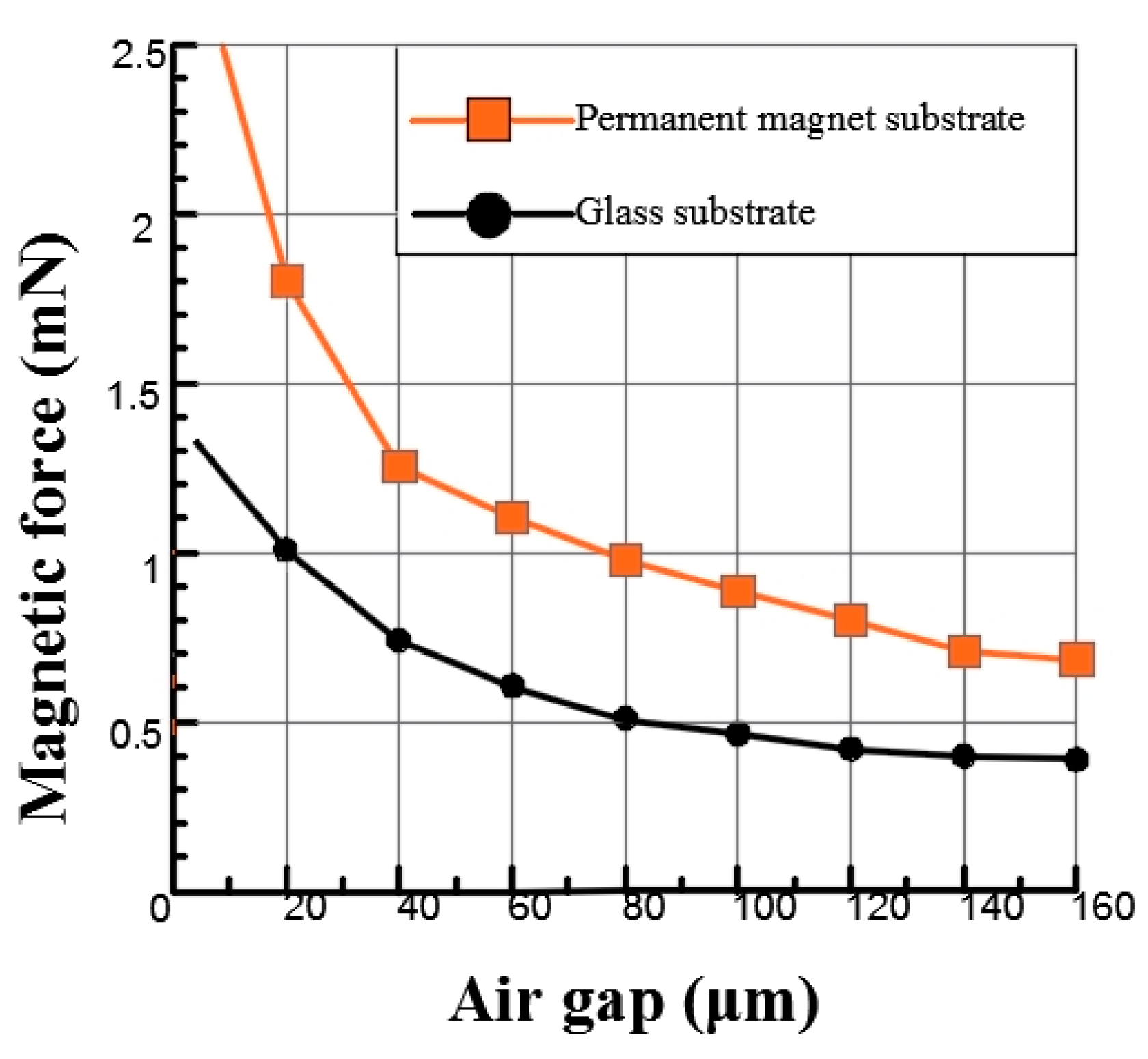


| Part | Material | Value |
|---|---|---|
| Central Platform | Permalloy | 1 mm × 1 mm × 15 µm |
| Planar Microcoil | Copper | 2.5 mm |
| Microspring | Nickel | 3 mm × 3 mm × 12 µm |
| Yoke | Permalloy | 2.8 mm × 2.8 mm × 50 µm |
| Substrate | Ferrite | 2.8 mm × 2.8 mm × 200 µm |
| Supporter | Nickel | 1.2 mm × 0.2 mm × 160 µm |
| p1 = −1.551 × e−10 | p2 = 8.225 × e−8 |
| p3 = −1.691 × e−5 | p4 = 0.0017 |
| p5 = −0.08869 | p6 = 3.003 |
| Type | Threshold Area |
|---|---|
| Da | 40.2 |
| Db | 23.45 |
| Dc | 22.74 |
| Dd | 53.48 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Feng, H.; Miao, X.; Yang, Z. Design, Simulation and Experimental Study of the Linear Magnetic Microactuator. Micromachines 2018, 9, 454. https://doi.org/10.3390/mi9090454
Feng H, Miao X, Yang Z. Design, Simulation and Experimental Study of the Linear Magnetic Microactuator. Micromachines. 2018; 9(9):454. https://doi.org/10.3390/mi9090454
Chicago/Turabian StyleFeng, Hanlin, Xiaodan Miao, and Zhuoqing Yang. 2018. "Design, Simulation and Experimental Study of the Linear Magnetic Microactuator" Micromachines 9, no. 9: 454. https://doi.org/10.3390/mi9090454
APA StyleFeng, H., Miao, X., & Yang, Z. (2018). Design, Simulation and Experimental Study of the Linear Magnetic Microactuator. Micromachines, 9(9), 454. https://doi.org/10.3390/mi9090454





