Modeling and Optimization of Structural Parameters for High-Efficiency Multi-Jet Polishing of Optical Glass
Abstract
1. Introduction
2. Theoretical Modeling
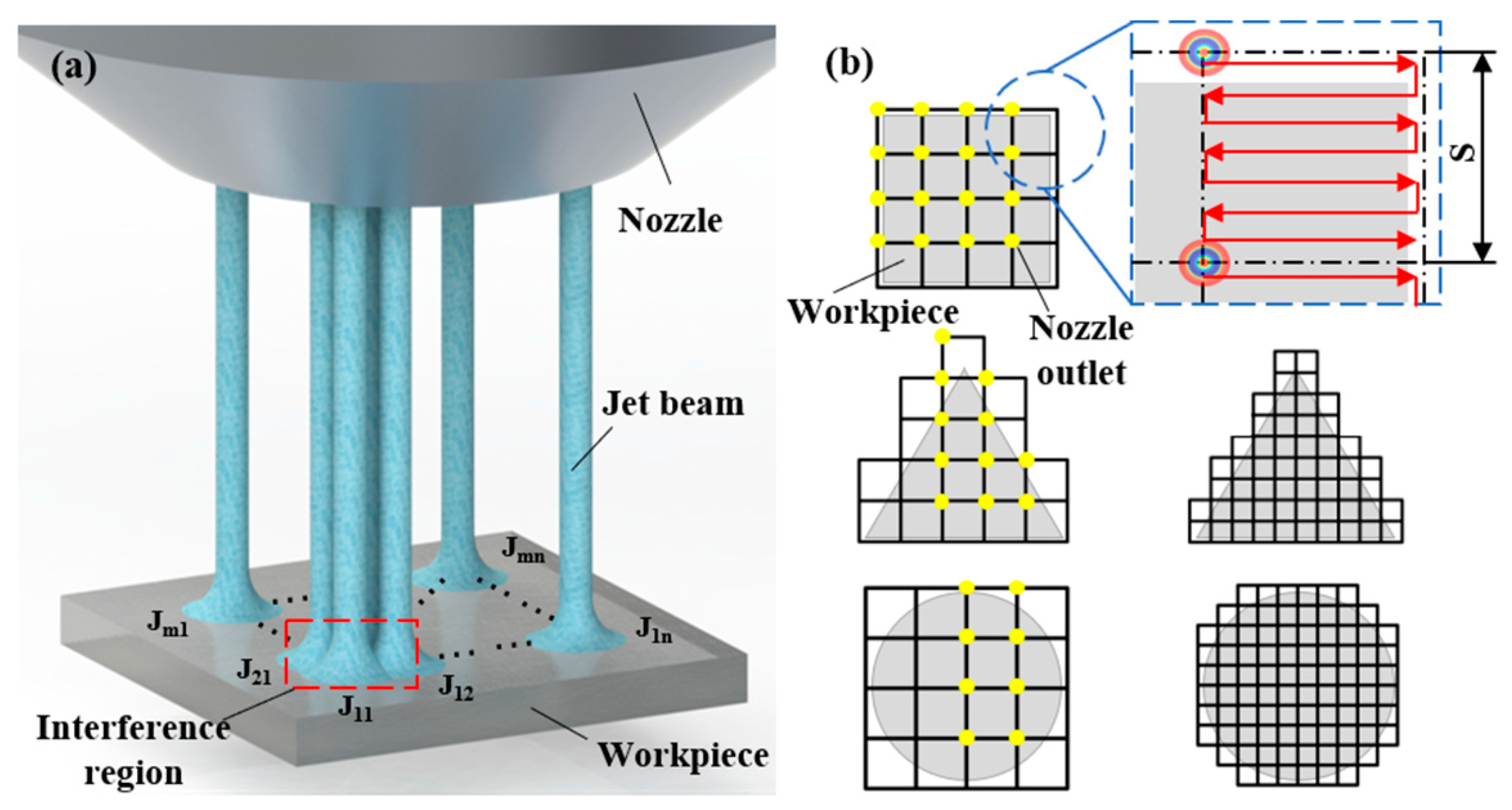
2.1. Geometric Modeling
2.2. Continuity Equation for the Continuous Phase
2.3. Discrete Phase Governing Equations
2.4. Particle Rebound Model
2.5. Abrasive Particle Erosion Theory
3. Numerical Simulation and Experimental Analysis
3.1. Numerical Simulation
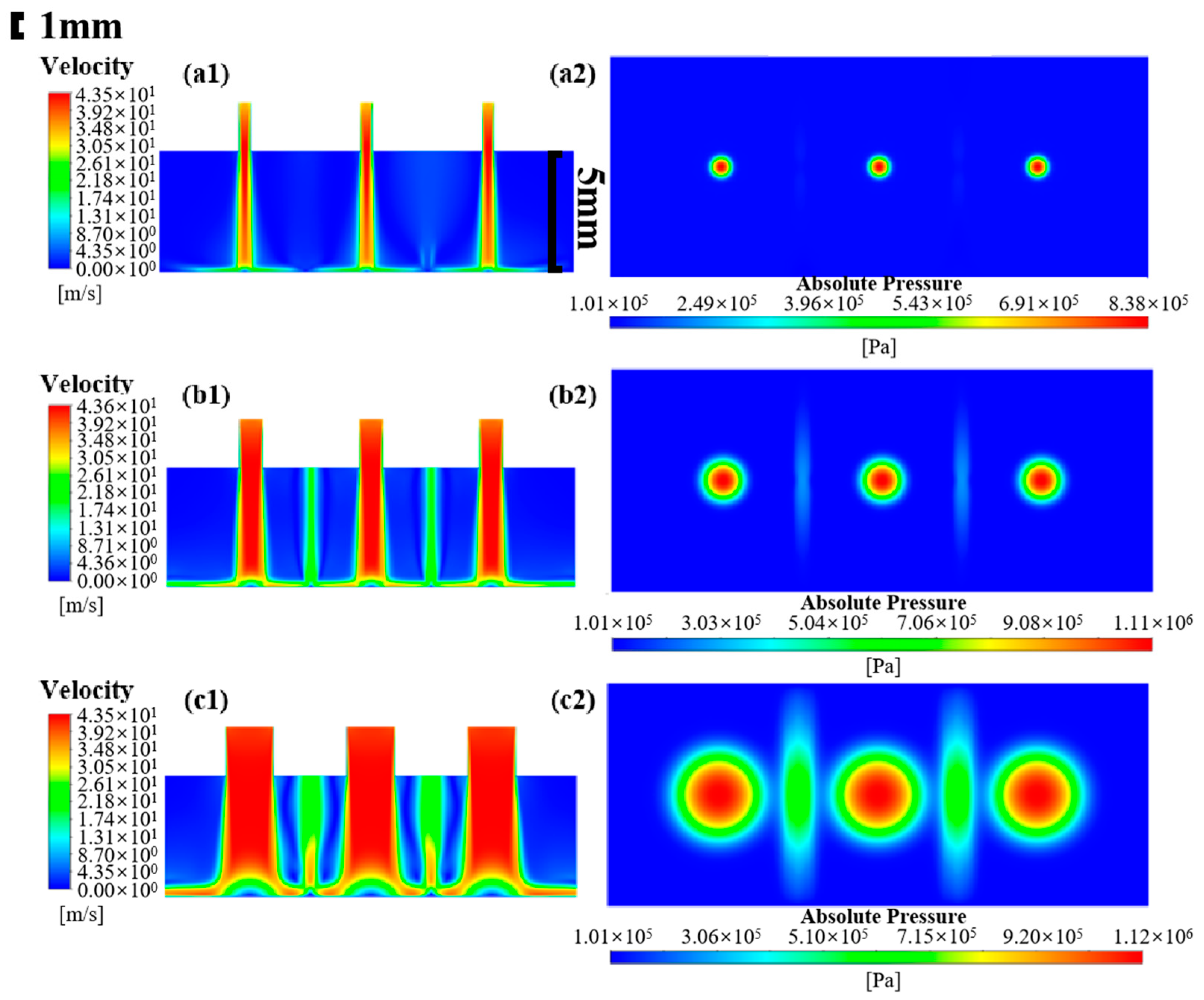
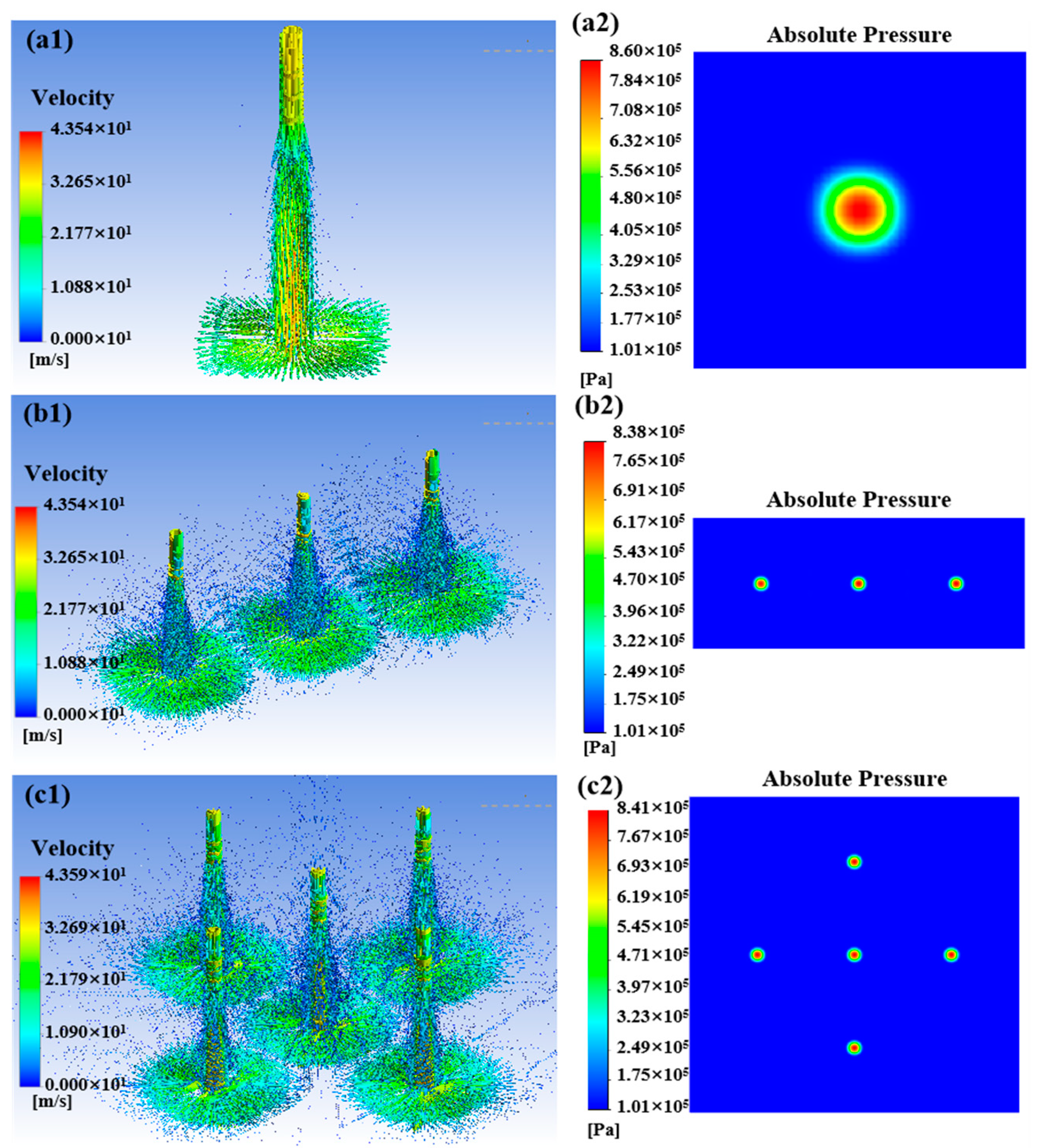
3.1.1. Effect of Nozzle Spacing
3.1.2. Effect of Outlet Diameter
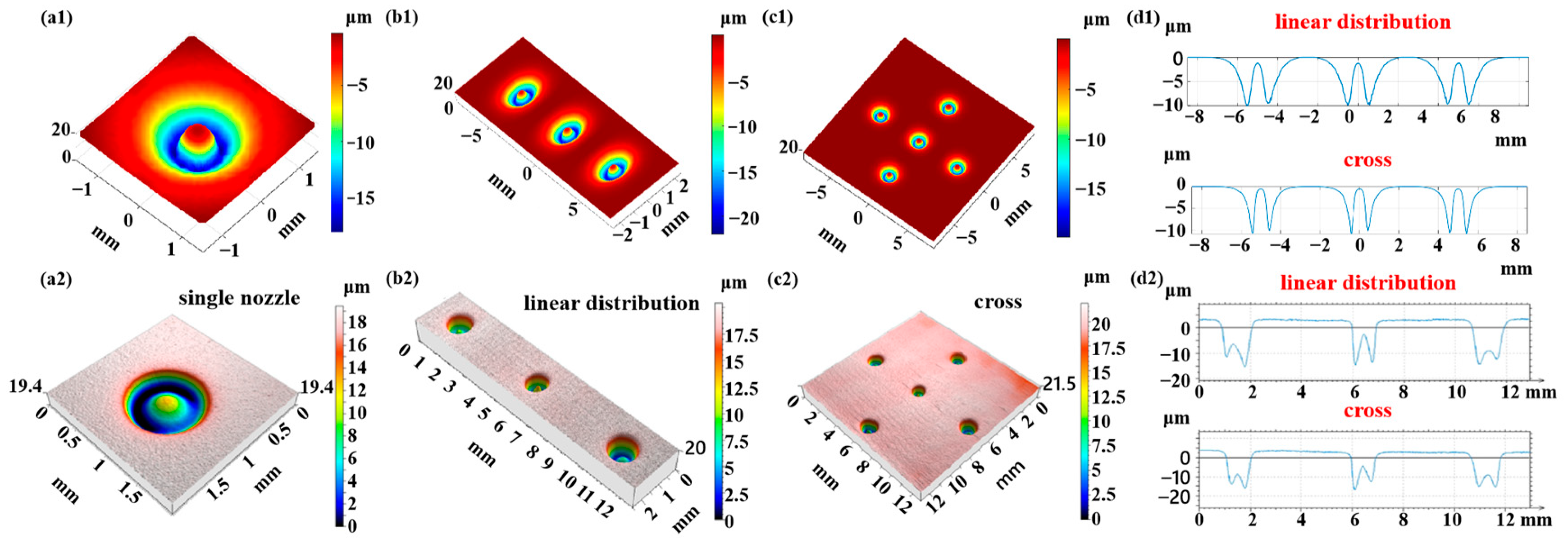
3.1.3. Effect of Distribution
3.2. Experiment
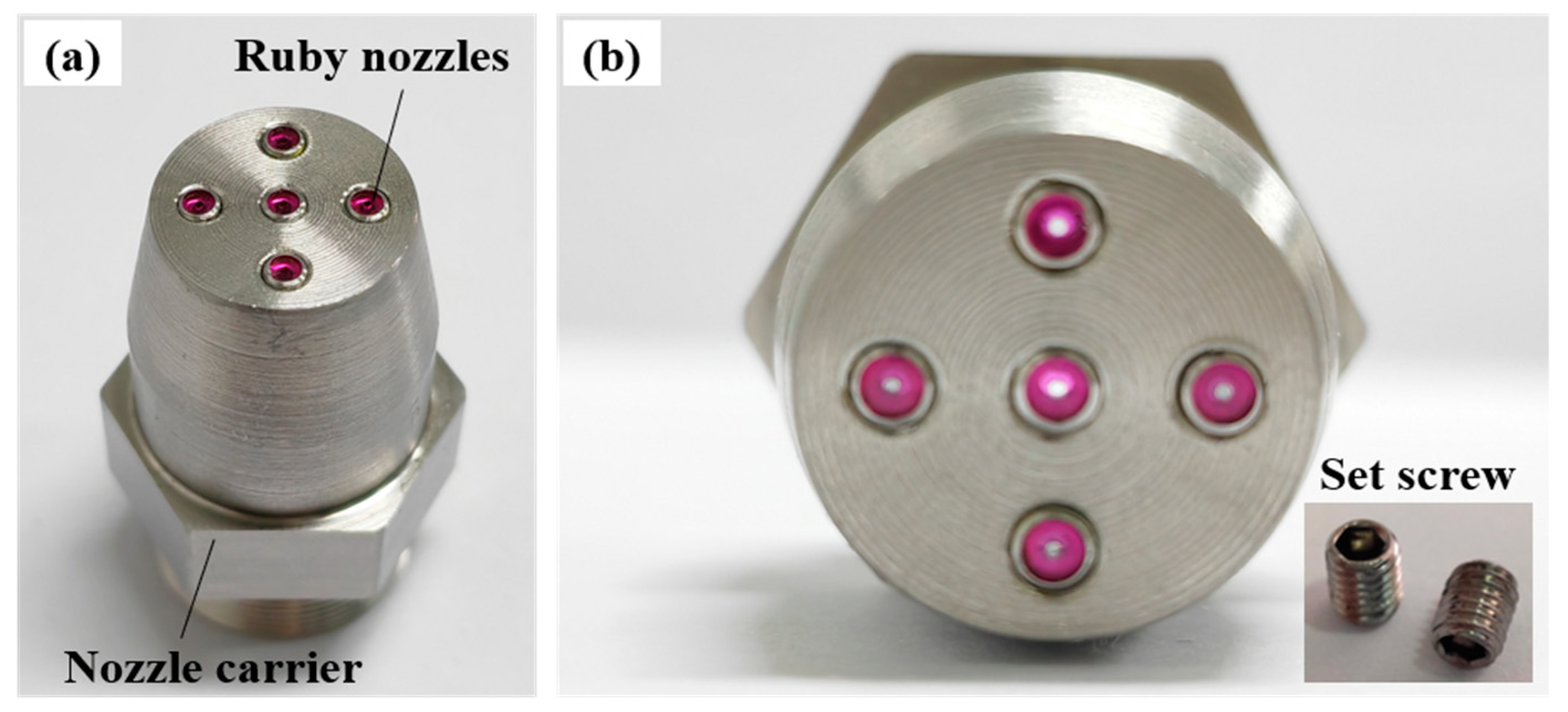
3.2.1. Experimental Design
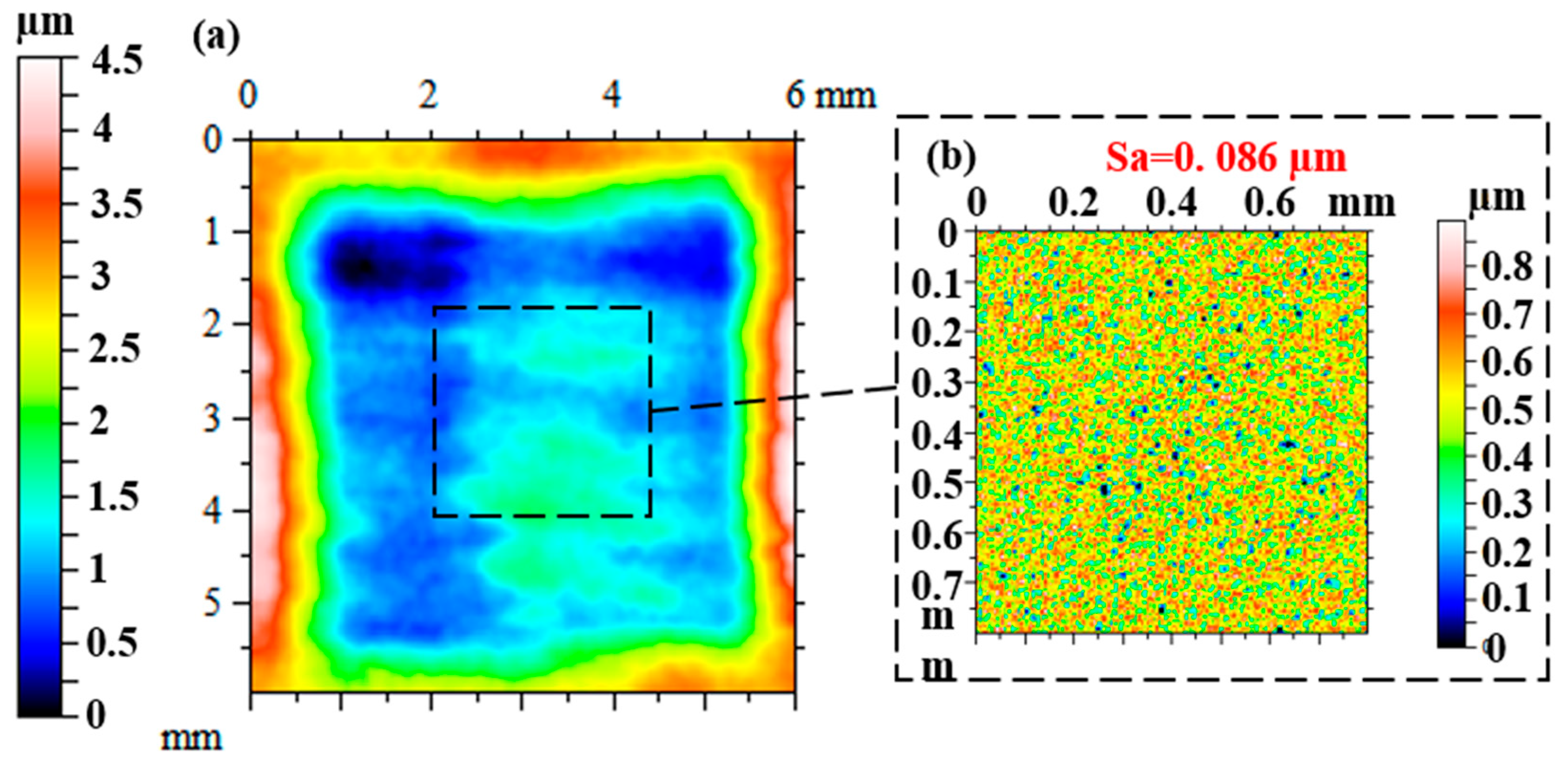
3.2.2. Results and Discussion
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Wang, C.J.; Cheung, C.F.; Liu, M.Y. Numerical modeling and experimentation of three dimensional material removal characteristics in fluid jet polishing. Int. J. Mech. Sci. 2017, 133, 568–577. [Google Scholar] [CrossRef]
- Fang, F.Z.; Zhang, X.D.; Weckenmann, A.; Zhang, G.X.; Evans, C. Manufacturing and measurement of freeform optics. CIRP Ann. 2013, 62, 823–846. [Google Scholar] [CrossRef]
- Ye, J.; Chen, L.; Li, X.; Yuan, Q.; Gao, Z. Review of optical freeform surface representation technique and its application. Opt. Eng. 2017, 56, 1. [Google Scholar] [CrossRef]
- Rolland, J.; Davies, M.; Suleski, T.; Evans, C.; Bauer, A.; Lambropoulos, J.; Falaggis, K. Freeform optics for imaging. Optica 2021, 8, 161–176. [Google Scholar] [CrossRef]
- Blaineau, P.; André, D.; Laheurte, R.; Darnis, P.; Darbois, N.; Cahuc, O.; Neauport, J. Subsurface mechanical damage during bound abrasive grinding of fused silica glass. Appl. Surf. Sci. 2015, 353, 764–773. [Google Scholar] [CrossRef]
- Fähnle, O.W.; Van Brug, H.; Frankena, H.J. Fluid Jet Polishing of Optical Surfaces. Appl. Opt. 1998, 37, 6771–6773. [Google Scholar] [CrossRef]
- Booij, S.M.; van Brug, H.; Fahnle, O.W. A mathematical model for machining spot in Fluid Jet Polishing. In Optical Fabrication and Testing; Optica Publishing Group: Québec City, QC, Canada, 2000. [Google Scholar]
- Zhao, X.; Ma, L.; Xu, X. Mode transition from adsorption removal to bombardment removal induced by nanoparticle-surface collisions in fluid jet polishing. Friction 2021, 9, 1127–1137. [Google Scholar] [CrossRef]
- Beaucamp, A.; Namba, Y. Super-Smooth finishing of diamond turned hard X-ray molding dies by combined fluid jet and bonnet polishing. CIRP Ann. 2013, 62, 315–318. [Google Scholar] [CrossRef]
- Beaucamp, A.; Katsuura, T.; Kawara, Z. A novel ultrasonic cavitation assisted fluid jet polishing system. CIRP Ann. 2017, 66, 301–304. [Google Scholar] [CrossRef]
- Wang, C.J.; Cheung, C.F.; Ho, L.T.; Liu, M.Y.; Lee, W.B. A novel multi-jet polishing process and tool for high-efficiency polishing. Int. J. Mach. Tools Manuf. 2017, 115, 60–73. [Google Scholar] [CrossRef]
- Cheung, B.C.F.; Wang, C.; Ho, L.; Lee, W.B. An investigation of multi-jet polishing of precision surfaces. In Proceedings of the ASPE 2017, Charlotte, NC, USA, 29 October–3 November 2017. [Google Scholar]
- Cao, Z.-C.; Wang, M.; Yan, S.; Zhao, C.; Liu, H. Surface integrity and material removal mechanism in fluid jet polishing of optical glass. J. Mater. Process. Technol. 2023, 311, 117798. [Google Scholar] [CrossRef]
- Luo, Y.; Li, X.; Zhang, R. Material removal rate analysis of multi-nozzle structure in FJP. Opt. Tech. 2015, 41, 360–364+368. [Google Scholar]
- Qiao, S.; Shi, F.; Tian, Y.; Song, C.; Tie, G.; Shen, X.; Song, J. Modeling and experiment on elastic region low defect jet polishing for fused quartz. J. Manuf. Process. 2022, 77, 831–837. [Google Scholar] [CrossRef]
- Qiao, S.; Shi, F.; Tian, Y.; Jiao, Z.; Yang, P.; Zhang, W. Numerical and experimental investigations on NANO-SIO2 jet polishing efficiency by different nozzle structures. Ceram. Int. 2022, 48, 15603–15612. [Google Scholar] [CrossRef]
- Cheung, C.F.; Wang, C.; Ho, L.T.; Chen, J. Curvature-adaptive multi-jet polishing of freeform surfaces. CIRP Ann. 2018, 67, 357–360. [Google Scholar] [CrossRef]
- Cheung, B.C.F.; Wang, C.; Cao, Z.-C.; Ho, L.; Liu, M. Development of a multi-jet polishing process for inner surface finishing. Precis. Eng. 2017, 52, 112–121. [Google Scholar] [CrossRef]
- Hirt, C.W.; Nichols, B.D. Volume of fluid (VOF) method for the dynamics of free boundaries. J. Comput. Phys. 1981, 39, 201–225. [Google Scholar] [CrossRef]
- Beaucamp, A.; Namba, Y.; Freeman, R. Dynamic multiphase modeling and optimization of fluid jet polishing process. CIRP Ann. 2012, 61, 315–318. [Google Scholar] [CrossRef]
- Sandilya, S.S.; Das, B.S.; Devi, K.; Khuntia, J.R.; Mohanty, M.P. CFD Simulation of Confluence of Flow Using Different Turbulence Models. In Hydraulics and Fluid Mechanics; Springer Nature: Singapore, 2025; Volume 1, pp. 205–217. [Google Scholar]
- Bulat, M.; Bulat, P. Comparison of Turbulence Models in the Calculation of Supersonic Separated Flows. World Appl. Sci. J. 2013, 27, 1263–1266. [Google Scholar]
- Ge, J.-Q.; Ren, Y.-L.; Xu, X.-S.; Li, C.; Li, Z.-A.; Xiang, W.-F. Numerical and experimental study on the ultrasonic-assisted soft abrasive flow polishing characteristics. Int. J. Adv. Manuf. Technol. 2021, 112, 3215–3233. [Google Scholar] [CrossRef]
- Ge, J.; Ren, Y.; Li, C.; Li, Z.; Yan, S.; Tang, P.; Xu, X.; Wang, Q. Ultrasonic coupled abrasive jet polishing (UC-AJP) of glass-based micro-channel for micro-fluidic chip. Int. J. Mech. Sci. 2023, 244, 108055. [Google Scholar] [CrossRef]
- Hoomans, B.P.B.; Kuipers, J.A.M.; Briels, W.J.; van Swaaij, W.P.M. Discrete particle simulation of bubble and slug formation in a two-dimensional gas-fluidised bed: A hard-sphere approach. Chem. Eng. Sci. 1996, 51, 99–118. [Google Scholar] [CrossRef]
- Hölzer, A.; Sommerfeld, M. New simple correlation formula for the drag coefficient of non-spherical particles. Powder Technol. 2008, 184, 361–365. [Google Scholar] [CrossRef]
- Hogue, C. Shape representation and contact detection for discrete element simulations of arbitrary geometries. Eng. Comput. 1998, 15, 374–390. [Google Scholar] [CrossRef]
- Grant, G.; Tabakoff, W. Erosion Prediction in Turbomachinery Resulting from Environmental Solid Particles. J. Aircr. 1975, 12, 471–478. [Google Scholar] [CrossRef]
- Cao, Z.-C.; Cheung, C.F. Theoretical modelling and analysis of the material removal characteristics in fluid jet polishing. Int. J. Mech. Sci. 2014, 89, 158–166. [Google Scholar] [CrossRef]
- Goodwin, J.E.; Sage, W.; Tilly, G.P. Study of Erosion by Solid Particles. Proc. Inst. Mech. Eng. 1969, 184, 279–292. [Google Scholar] [CrossRef]
- Huang, C.; Chiovelli, S.; Minev, P.; Luo, J.; Nandakumar, K. A comprehensive phenomenological model for erosion of materials in jet flow. Powder Technol. 2008, 187, 273–279. [Google Scholar] [CrossRef]
- Thongkaew, K.; Wang, J.; Yeoh, G.H. Impact characteristics and stagnation formation on a solid surface by a supersonic abrasive waterjet. Int. J. Extrem. Manuf. 2019, 1, 045004. [Google Scholar] [CrossRef]







| Parameters | Settings |
|---|---|
| Multiphase flow model | VOF |
| Volumetric | Implicit |
| Turbulence modelling | Realizable k-ε |
| Solver | Pressure-based |
| Solver algorithm | Coupled |
| Gradient | Least squares cell based |
| Experimental Parameters | Settings |
|---|---|
| Abrasive particles | CeO2 |
| Particle diameter (µm) | 6 |
| Jet pressure (bar) | 10 |
| Target distance (mm) | 5 |
| Impact angle (°) | 90 |
| Processing time (min) | 10 |
| Polishing fluid concentration | 1:12 (abrasive to water) |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cao, Z.; Miao, Y.; Wang, M.; Zhu, Z. Modeling and Optimization of Structural Parameters for High-Efficiency Multi-Jet Polishing of Optical Glass. Micromachines 2025, 16, 551. https://doi.org/10.3390/mi16050551
Cao Z, Miao Y, Wang M, Zhu Z. Modeling and Optimization of Structural Parameters for High-Efficiency Multi-Jet Polishing of Optical Glass. Micromachines. 2025; 16(5):551. https://doi.org/10.3390/mi16050551
Chicago/Turabian StyleCao, Zhongchen, Yiwei Miao, Ming Wang, and Zhenfeng Zhu. 2025. "Modeling and Optimization of Structural Parameters for High-Efficiency Multi-Jet Polishing of Optical Glass" Micromachines 16, no. 5: 551. https://doi.org/10.3390/mi16050551
APA StyleCao, Z., Miao, Y., Wang, M., & Zhu, Z. (2025). Modeling and Optimization of Structural Parameters for High-Efficiency Multi-Jet Polishing of Optical Glass. Micromachines, 16(5), 551. https://doi.org/10.3390/mi16050551






