Imbibition of Newtonian Fluids in Paper-like Materials with the Infinitesimal Control Volume Method
Abstract
:1. Introduction
2. Materials and Methods
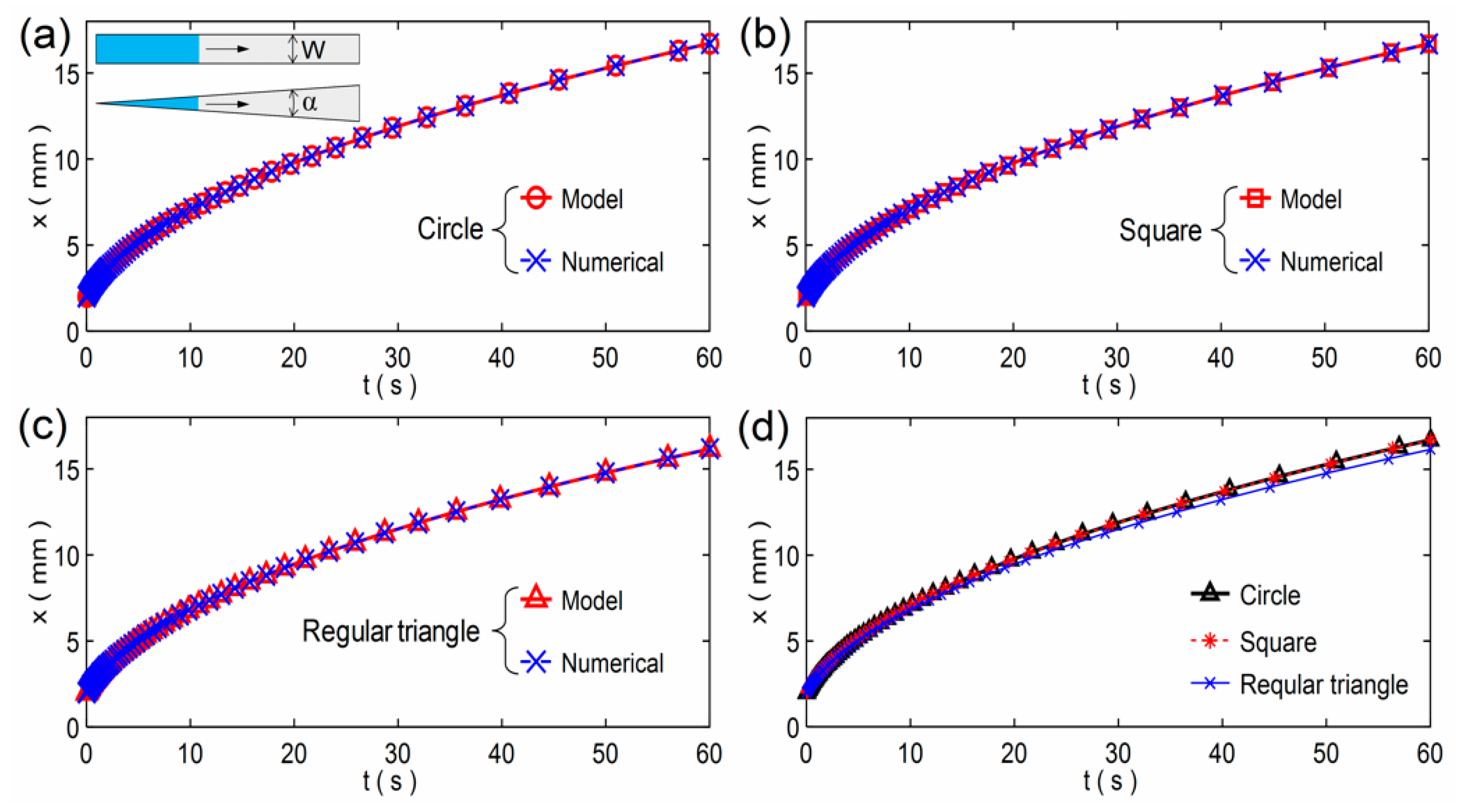
2.1. Imbibition Analysis with the Infinitesimal Control Volume Method
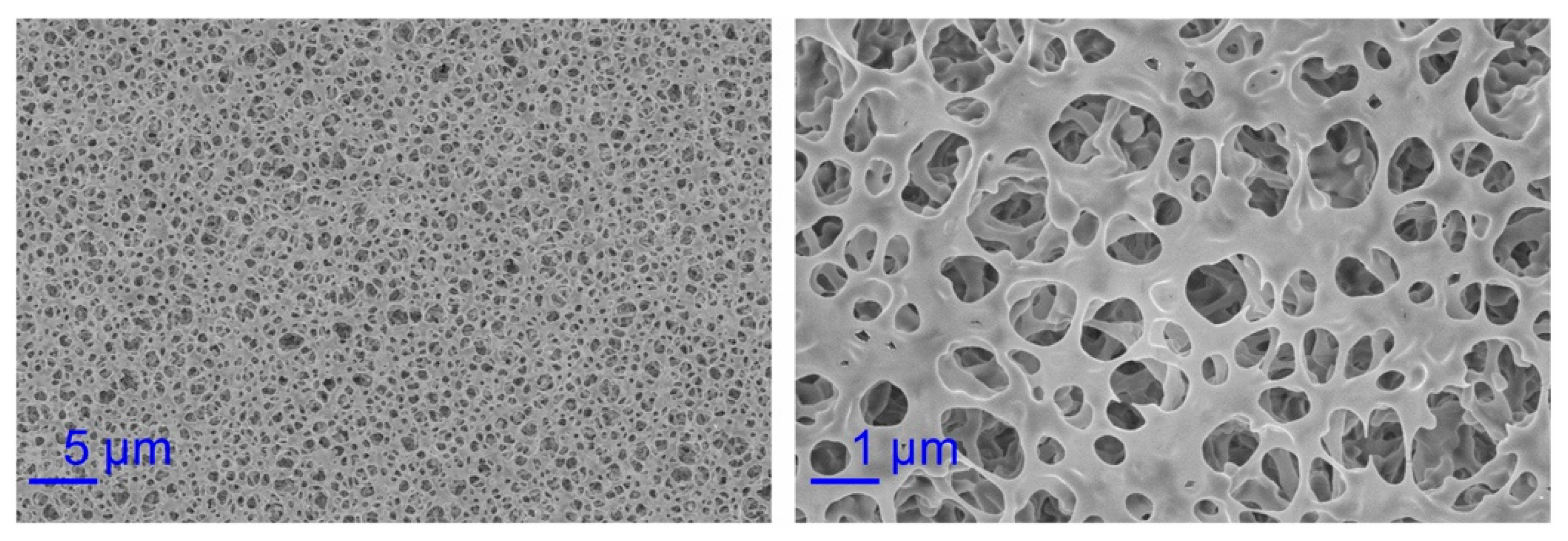
2.2. Imbibition Experiment
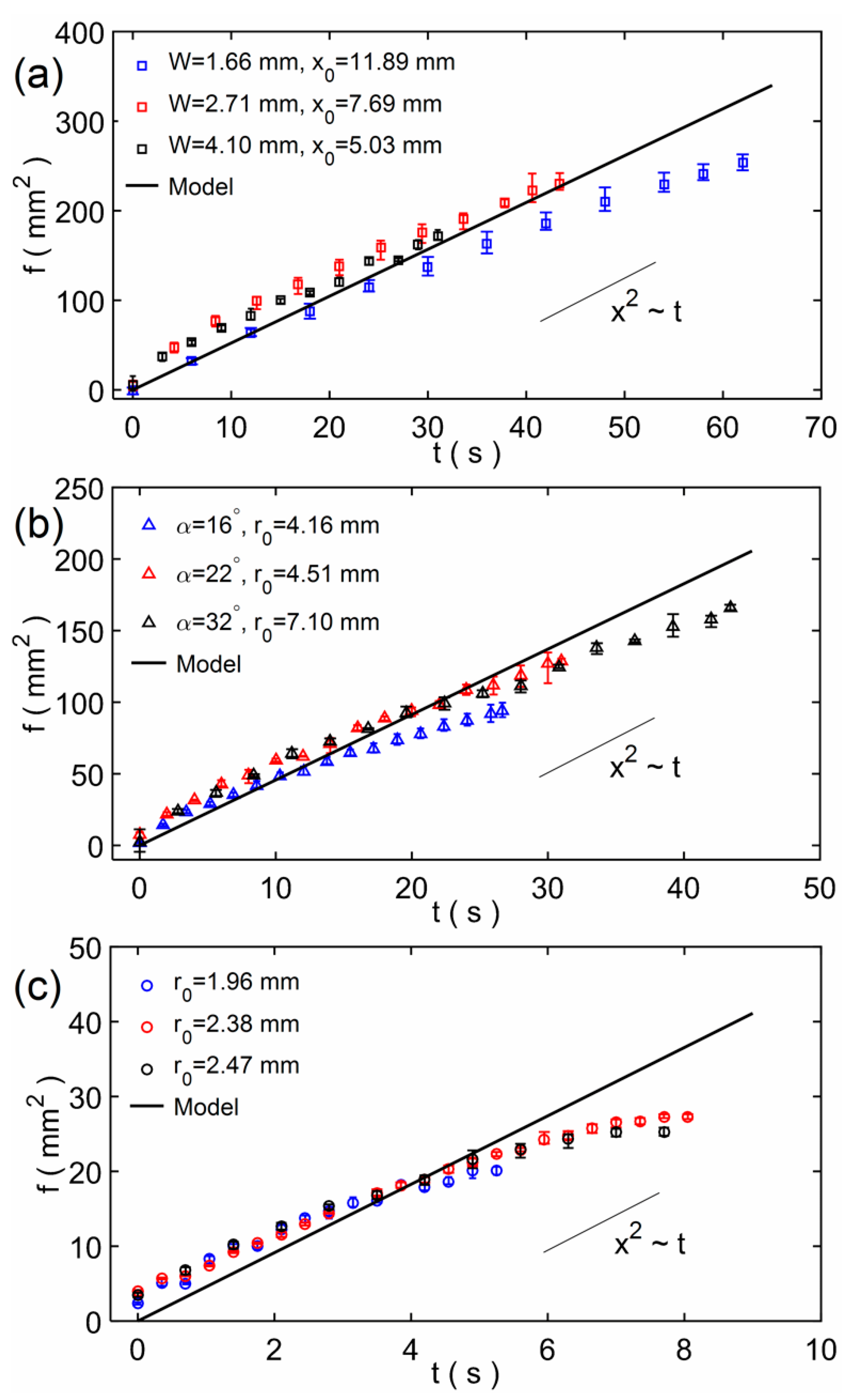
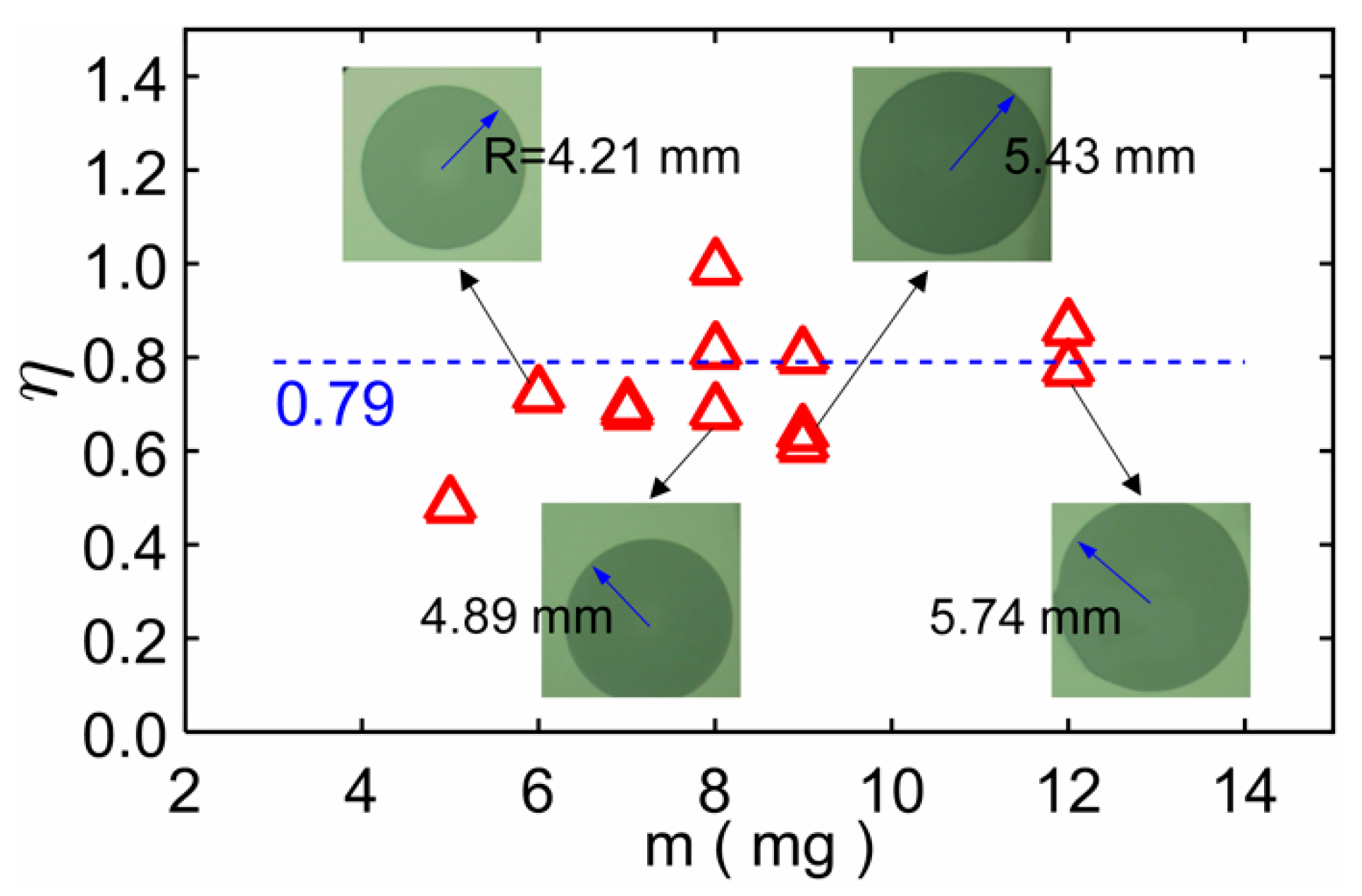
3. Results and Discussion
4. Conclusions
5. Patents
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Huang, C.H.; Lei, K.F.; Tsang, N.M. Paper-based microreactor array for rapid screening of cell signaling cascades. Lab Chip 2016, 16, 2911–2920. [Google Scholar] [CrossRef]
- Lantigua, D.; Kelly, Y.N.; Unal, B.; Camci-Unal, G. Engineered paper-based cell culture platforms. Adv. Healthc. Mater. 2017, 6, 1700619. [Google Scholar] [CrossRef] [PubMed]
- Hu, J.; Wang, S.; Wang, L.; Li, F.; Pingguan-Murphy, B.; Lu, T.; Xu, F. Advances in paper-based point-of-care diagnostics. Biosens. Bioelectron. 2014, 54, 585–597. [Google Scholar] [CrossRef]
- Martinez, A.W.; Phillips, S.T.; Whitesides, G.M. Three-dimensional microfluidic devices fabricated in layered paper and tape. Proc. Natl. Acad. Sci. USA 2008, 105, 19606–19611. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Yew, C.H.T.; Azari, P.; Choi, J.R.; Li, F.; Pingguan-Murphy, B. Electrospin-coating of nitrocellulose membrane enhances sensitivity in nucleic acid-based lateral flow assay. Anal. Chim. Acta 2018, 1009, 81–88. [Google Scholar] [CrossRef]
- Elizalde, E.; Urteaga, R.; Berli, C.L. Rational design of capillary-driven flows for paper-based microfluidics. Lab Chip 2015, 15, 2173–2180. [Google Scholar] [CrossRef] [PubMed]
- Martinez, A.W.; Phillips, S.T.; Whitesides, G.M.; Carrilho, E. Diagnostics for the developing world: Microfluidic paper-based analytical devices. Anal. Chem. 2010, 82, 3–10. [Google Scholar] [CrossRef]
- Li, X.; Ballerini, D.R.; Shen, W. A perspective on paper-based microfluidics: Current status and future trends. Biomicrofluidics 2012, 6, 011301. [Google Scholar] [CrossRef] [Green Version]
- Cate, D.M.; Adkins, J.A.; Mettakoonpitak, J.; Henry, C.S. Recent developments in paper-based microfluidic devices. Anal. Chem. 2015, 87, 19–41. [Google Scholar] [CrossRef]
- He, Y.; Wu, Y.; Fu, J.Z.; Wu, W.B. Fabrication of paper-based microfluidic analysis devices: A review. Rsc. Adv. 2015, 5, 78109–78127. [Google Scholar] [CrossRef]
- Soum, V.; Park, S.; Brilian, A.I.; Kwon, O.S.; Shin, K. Programmable paper-based microfluidic devices for biomarker detections. Micromachines 2019, 10, 516. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Martinez, A.W.; Phillips, S.T.; Butte, M.J.; Whitesides, G.M. Patterned paper as a platform for inexpensive, low-volume, portable bioassays. Angew. Chem. Int. Edit. 2007, 119, 1340–1342. [Google Scholar] [CrossRef]
- Agrawal, P.; Kumar, H.; Kumar, P. Rapid and even spreading of complex fluids over a large area in porous substrates. Appl. Phys. Lett. 2020, 117, 073703. [Google Scholar] [CrossRef]
- Busa, L.S.A.; Mohammadi, S.; Maeki, M.; Ishida, A.; Tani, H.; Tokeshi, M. Advances in microfluidic paper-based analytical devices for food and water analysis. Micromachines 2016, 7, 86. [Google Scholar] [CrossRef]
- He, Y.; Gao, Q.; Wu, W.; Nie, J.; Fu, J. 3D printed paper-based microfluidic analytical devices. Micromachines 2016, 7, 108. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Xu, H.; Li, J.; Tesler, A.B.; Yao, Y.; Aizenberg, J. Dynamic air/liquid pockets for guiding microscale flow. Nat. Commun. 2018, 9, 733. [Google Scholar]
- Fu, E.; Ramsey, S.A.; Kauffman, P.; Lutz, B.; Yager, P. Transport in two-dimensional paper networks. Microflud. Nanofluid. 2011, 10, 29–35. [Google Scholar] [CrossRef] [Green Version]
- Conrath, M.; Fries, R.; Zhang, R.; Dreyer, R.E. Radial capillary transport from an infinite reservoir. Transport. Porous Med. 2010, 84, 109–132. [Google Scholar] [CrossRef]
- Mendez, S.; Fenton, E.M.; Gallegos, G.R.; Petsev, D.N.; Sibbett, S.S.; Stone, H.A.; Zhang, Y.; López, G.P. Imbibition in porous membranes of complex shape: Quasi-stationary flow in thin rectangular segments. Langmuir 2010, 26, 1380–1385. [Google Scholar] [CrossRef]
- Xiao, W.; Hagen, J.A.; Papautsky, I. Paper pump for passive and programmable transport. Biomicrofluidics 2013, 7, 014107. [Google Scholar]
- Shou, D.; Ye, L.; Fan, J.; Fu, K.; Mei, M.; Wang, H.; Chen, Q. Geometry-induced asymmetric capillary flow. Langmuir 2014, 30, 5448–5454. [Google Scholar] [CrossRef] [PubMed]
- Kokalj, T.; Park, Y.; Vencelj, M.; Jenko, M.; Lee, L.P. Self-powered imbibing microfluidic pump by liquid encapsulation: SIMPLE. Lab Chip 2014, 14, 4329–4333. [Google Scholar] [CrossRef]
- Yang, S.; Yin, K.; Chu, D.; He, J.; Duan, J.A. Femtosecond laser structuring of Janus foam: Water spontaneous antigravity unidirectional penetration and pumping. Appl. Phys. Lett. 2018, 113, 203701. [Google Scholar] [CrossRef]
- Liu, Z.; He, X.; Han, J.; Zhang, X.; Li, F.; Li, A.; Qu, Z.; Xu, F. Liquid wicking behavior in paper-like materials: Mathematical models and their emerging biomedical applications. Microfluid. Nanofluid. 2018, 22, 132. [Google Scholar] [CrossRef]
- Liu, H.; Patil, P.R.; Narusawa, U. On Darcy-Brinkman equation: Viscous flow between two parallel plates packed with regular square arrays of cylinders. Entropy 2007, 9, 118–131. [Google Scholar] [CrossRef]
- Tang, K.P.M.; Kan, C.W.; Fan, J.T. Evaluation of water absorption and transport property of fabrics. Text. Prog. 2014, 46, 1–132. [Google Scholar] [CrossRef]
- Liou, W.W.; Peng, Y.; Parker, P.E. Analytical modeling of capillary flow in tubes of nonuniform cross section. J. Colloid Interfance Sci. 2009, 333, 389–399. [Google Scholar] [CrossRef]
- Ashari, A.; Bucher, T.M.; Tafreshi, H.V.; Tahir, M.A.; Rahman, M. Modeling fluid spread in thin fibrous sheets: Effects of fiber orientation. Int. J. Heat Mass Tran. 2010, 53, 1750–1758. [Google Scholar] [CrossRef]
- Chen, Q.; Tang, K.M.; Ma, P.; Jiang, G. Evaluation of water absorption and transport properties of weft knitted polyester fabrics by spontaneous uptake water transport tester and conventional test methods. Fiber. Polym. 2016, 17, 1287–1295. [Google Scholar] [CrossRef]
- Marmur, A. The radial capillary. J. Colloid Interfance Sci. 1988, 124, 301–308. [Google Scholar] [CrossRef]
- Kalogianni, E.P.; Savopoulos, T.; Karapantsios, T.D.; Raphaelides, S.N. A dynamic wicking technique for determining the effective pore radius of pregelatinized starch sheets. Colloid. Surf. B 2004, 35, 159–167. [Google Scholar] [CrossRef] [PubMed]
- Washburn, E.W. The dynamics of capillary flow. Phys. Rev. 1921, 17, 273. [Google Scholar] [CrossRef]
- Seker, E.; Begley, M.R.; Reed, M.L.; Utz, M. Kinetics of capillary wetting in nanoporous films in the presence of surface evaporation. Appl. Phys. Lett. 2008, 92, 013128. [Google Scholar] [CrossRef]
- Liu, M.; Wu, J.; Gan, Y.; Hanaor, D.A.H.; Chen, C.Q. Evaporation limited radial capillary penetration in porous media. Langmuir 2016, 32, 9899–9904. [Google Scholar] [CrossRef] [PubMed]
- Cencha1, L.G.; Huber, P.; Kappl, M.; Floudas, G.; Steinhart, M.; Berli, C.L.A.; Urteaga, R. Nondestructive high-throughput screening of nanopore geometry in porous membranes by imbibition. Appl. Phys. Lett. 2019, 115, 113701. [Google Scholar] [CrossRef]
- Fries, N.; Odic, K.; Conrath, M.; Dreyer, M. The effect of evaporation on the wicking of liquids into a metallic weave. J. Colloid Interfance Sci. 2008, 321, 118–129. [Google Scholar] [CrossRef]
- Hong, S.; Kim, W. Dynamics of water imbibition through paper channels with wax boundaries. Microflud. Nanofluid. 2015, 19, 845–853. [Google Scholar] [CrossRef]
- Cai, J.; Perfect, E.; Cheng, C.L.; Hu, X. Generalized modeling of spontaneous imbibition based on Hagen–Poiseuille flow in tortuous capillaries with variably shaped apertures. Langmuir 2014, 30, 5142–5151. [Google Scholar] [CrossRef] [PubMed]
- Patro, D.; Jayaram, V. Kinetics of pressureless infiltration of Al-Mg melts into porous alumina preforms. Metall. Mater. Trans. B 2008, 39, 108–115. [Google Scholar] [CrossRef]
- Chaudhury, K.; Kar, S.; Chakraborty, S. Diffusive dynamics on paper matrix. Appl. Phys. Lett. 2016, 109, 224101. [Google Scholar] [CrossRef]
- Li, K.; Zhao, H. Fractal prediction model of spontaneous imbibition rate. Transport. Porous Med. 2012, 91, 363–376. [Google Scholar] [CrossRef]
- Balankin, A.S.; Susarrey, O.; Gonzáles, J.M. Scaling properties of pinned interfaces in fractal media. Phys. Rev. Lett. 2003, 90, 096101. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Cai, J.; Yu, B. A discussion of the effect of tortuosity on the capillary imbibition in porous media. Transport. Porous Med. 2011, 89, 251–263. [Google Scholar] [CrossRef]
- Xiao, Z.; Yang, Y.; Zhang, X.; Guo, W. Controlling Capillary Flow Rate on Lateral Flow Test Substrates by Tape. Micromachines 2021, 12, 562. [Google Scholar] [CrossRef]
- Bruus, H. Theoretical Microfluidics; Oxford University Press: Oxford, UK, 2008. [Google Scholar]
- Böhm, A.; Carstens, F.; Trieb, C.; Schabel, S.; Biesalski, M. Engineering microfluidic papers: Effect of fiber source and paper sheet properties on capillary-driven fluid flow. Microflud. Nanofluid. 2014, 16, 789–799. [Google Scholar] [CrossRef]
- Shirasaki, N.; Matsushita, T.; Matsui, Y.; Murai, K. Assessment of the efficacy of membrane filtration processes to remove human enteric viruses and the suitability of bacteriophages and a plant virus as surrogates for those viruses. Water Res. 2017, 115, 29–39. [Google Scholar] [CrossRef]
- Lucas, R. Rate of capillary ascension of liquids. Kolloid-Z 1918, 23, 15–22. [Google Scholar] [CrossRef]




| Coefficient | Circle | Square | Regular Triangle |
|---|---|---|---|
| C1 | |||
| C2 | |||
| C3 |
| Paper Shape | Rectangle | Fan Shape | Circular |
|---|---|---|---|
| Q | Wδηv | αδηrv | 2πδηrv |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Song, K.; Huang, R.; Hu, X. Imbibition of Newtonian Fluids in Paper-like Materials with the Infinitesimal Control Volume Method. Micromachines 2021, 12, 1391. https://doi.org/10.3390/mi12111391
Song K, Huang R, Hu X. Imbibition of Newtonian Fluids in Paper-like Materials with the Infinitesimal Control Volume Method. Micromachines. 2021; 12(11):1391. https://doi.org/10.3390/mi12111391
Chicago/Turabian StyleSong, Kui, Ruijie Huang, and Xiaoling Hu. 2021. "Imbibition of Newtonian Fluids in Paper-like Materials with the Infinitesimal Control Volume Method" Micromachines 12, no. 11: 1391. https://doi.org/10.3390/mi12111391
APA StyleSong, K., Huang, R., & Hu, X. (2021). Imbibition of Newtonian Fluids in Paper-like Materials with the Infinitesimal Control Volume Method. Micromachines, 12(11), 1391. https://doi.org/10.3390/mi12111391






