A Nanomechanical Analysis of Deformation Characteristics of 6H-SiC Using an Indenter and Abrasives in Different Fixed Methods
Abstract
:1. Introduction
2. Experimental
2.1. Materials
2.2. Nanoindentation Experiment
2.3. Fixed Abrasive Machining
2.4. Free Abrasive Machining
2.5. Semi-fixed Abrasive Machining
3. Results and Discussion
3.1. Results and Theoretical Analysis of this Nanoindentation Experiment
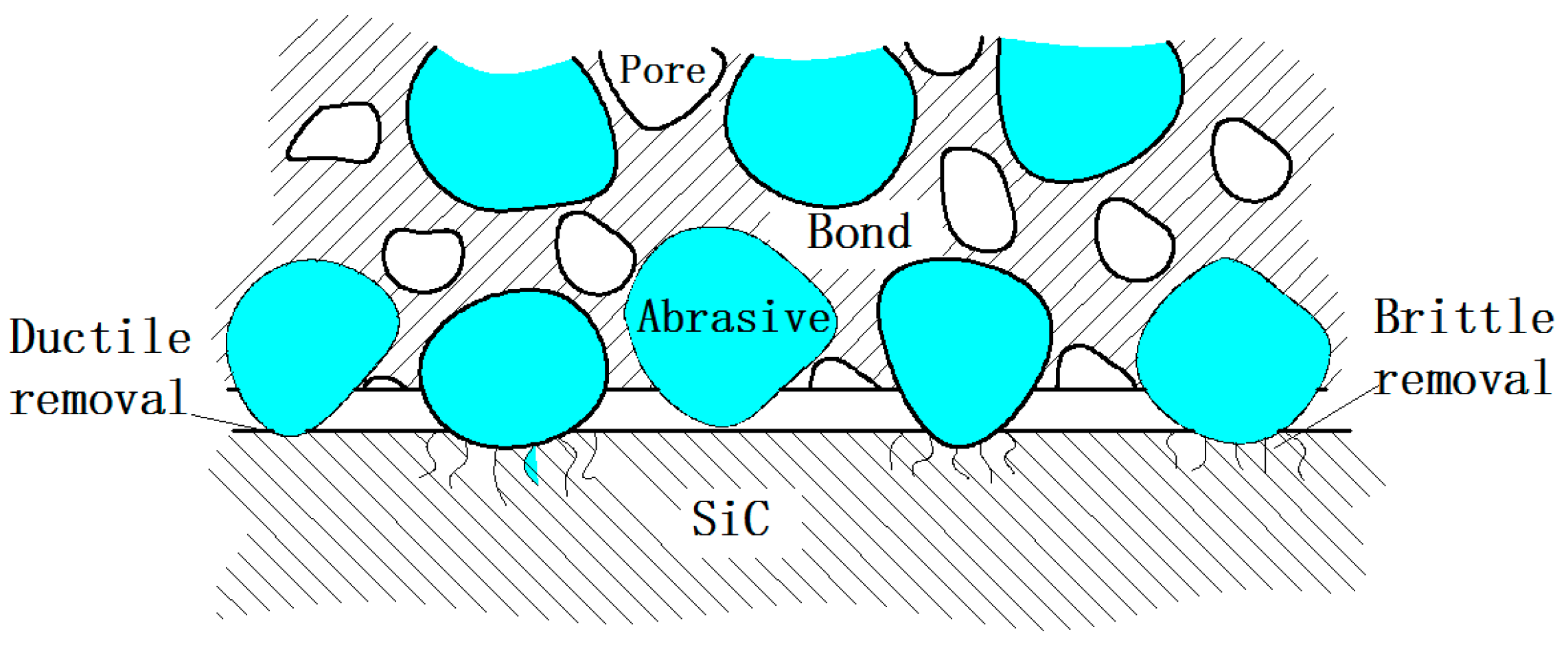
3.2. The Results of Grinding with Fixed Abrasives and Materials Removal Analysis
3.3. The Lapping Results of Free Abrasives and Analysis of Material Removal
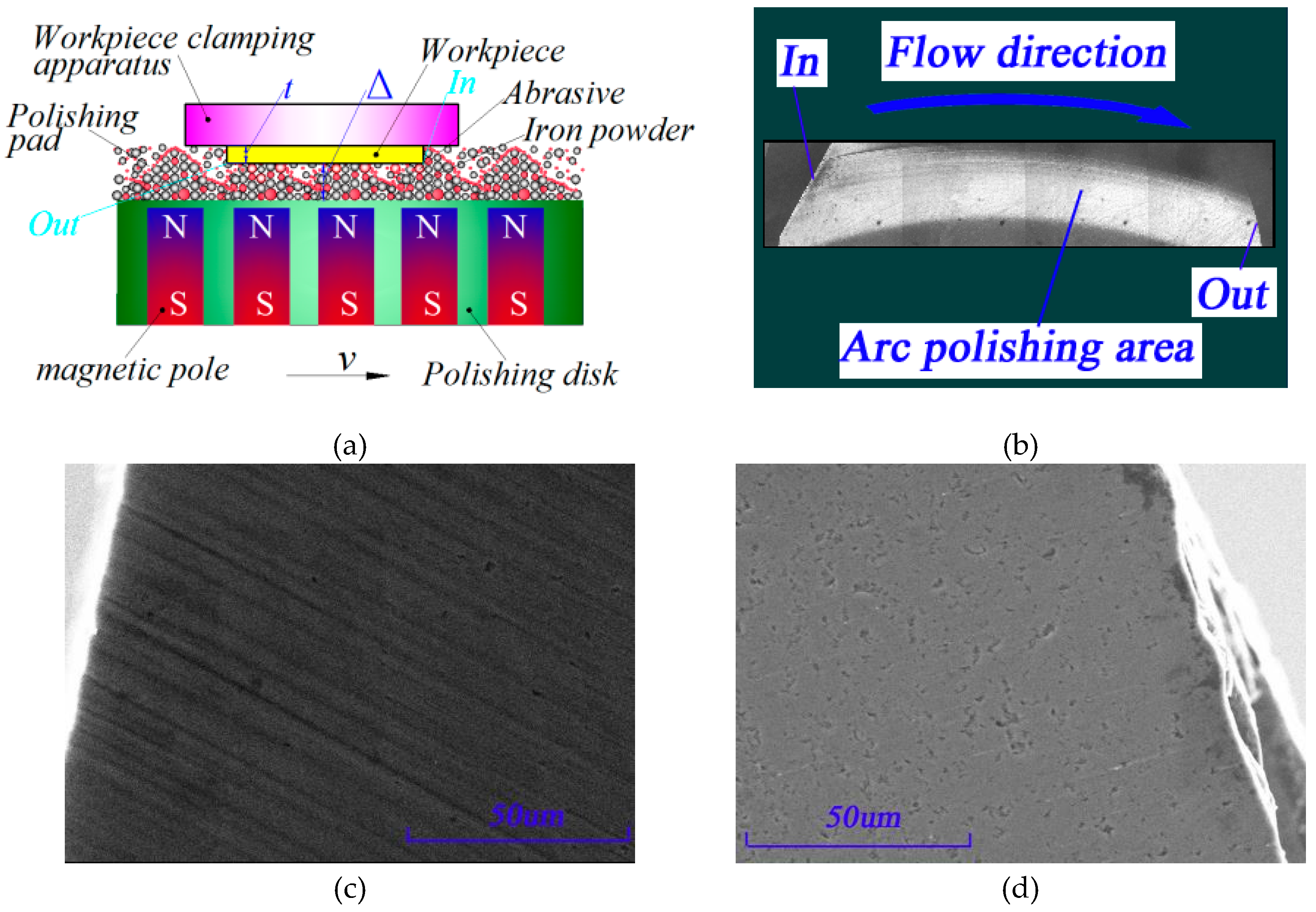
3.4. The Cluster MR Finishing Results of Semi-fixed Abrasives and Analysis of Material Removal
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
Nomenclature
| Symbol | Nomenclature |
| fs | Spindle feed(µm/s) |
| nw | The rotational speed of workpiece (rpm) |
| ns | The rotational speed of grinding wheel (rpm) |
| H | Hardness (Gpa) |
| E | Elasticity modulus (Gpa) |
| Kc | The fracture toughness (Mpa m1/2) |
| The radius of curvature of grinding grains on the materials (mm) | |
| F0 | The contact load (mN) |
| FC | The contact load of C face (mN) |
| FSi | The contact load of Si face (mN) |
| P* | The critical load for brittle materials changing from plastic deformation to brittle fracture (mN) |
| P*C | The critical load for C face changing from plastic deformation to brittle fracture (mN) |
| φ | The proportion of magnetic particles in the MR fluids |
| hmax | The maximum thickness of undeformed substrate (nm) |
| dc | The critical grit cutting depth (nm) |
| R | The radius of the grinding wheels (mm) |
| Lw | The circumference of layers of grinding material of the cupped grinding wheels (mm) |
| C | The concentration of grinding materials of diamond grinding wheels |
| Kβ | The coefficient relating to the shape of the grinding grains |
| dw | The radius of grinding grain (μm) |
| rm | The radius at any point on the surface of the workpieces |
| vd | The volume fraction of diamonds in the grinding wheel |
| hwp | The space between the workpieces and the lapping plates (mm) |
| Fin | The abrasive forces at the entrance of the polishing belts (μN) |
| S | The workpiece area (mm2) |
| PF | The polishing pressures (kPa) |
| Pm | The pressure produced by MR effects of the MR fluids (kPa) |
| Pd | The hydrodynamic pressure (kPa) |
| Pg | The buoyancy of MR fluids (kPa) |
| μ0 | The magnetic inductivity of a vacuum |
| μ | The magnetic inductivity of magnetic particles |
| η | The initial viscosity of the MR fluids (Pa.s) |
| v | Speed of polishing disk (m/s) |
| Fv | The volume fraction of the layers of material in the grinding wheels (g⁄cm3) |
| P*Si | The critical load for Si face changing from plastic deformation to brittle fracture (mN) |
| Kb | The distribution coefficient of abrasives between the workpieces and lapping plates |
| Hm | The strength of the external magnetic fields (Gs) |
| h0 | The distance from the polishing plates to the surfaces of workpieces (mm) |
| t | The thickness of workpieces (mm) |
| Δ | The machining gap (mm) |
| W | The tooth width of layers of grinding material of the cupped grinding wheels (mm) |
| Fw | The average pressure on single abrasive (mN) |
| SA | The actual contact area between a single abrasive and the workpieces (mm2) |
| p | The lapping pressure (kPa) |
| dg | The equivalent spherical diameter of diamond particles |
| f | The fraction of diamond particles that actively cut when grinding |
| dmax | The maximum diameter of abrasives (mm) |
| Fout | The abrasive forces at the exit of the polishing belts (μN) |
References
- Yang, Y.; Duan, B.; Yuan, S.; Jia, H. Novel Developments and Challenges for the SiC Power Devices. Advanced Silicon Carbide Devices and Processing. In Advanced Silicon Carbide Devices and Processing; IntechOpen: London, UK, 2015; pp. 175–195. [Google Scholar]
- Hamada, K.; Nagao, M.; Ajioka, M.; Kawai, F. SiC—Emerging Power Device Technology for Next-Generation Electrically Powered Environmentally Friendly Vehicles. IEEE Trans. Electron. Devices 2015, 62, 278–285. [Google Scholar] [CrossRef]
- Nishio, J.; Ota, C.; Hatakeyama, T.; Shinohe, T.; Kojima, K.; Nishizawa, S.-I.; Ohashi, H. Ultralow-Loss SiC Floating Junction Schottky Barrier Diodes (Super-SBDs). IEEE Trans. Electron. Devices 2008, 55, 1954–1960. [Google Scholar] [CrossRef]
- Neudeck, P.; Okojie, R.; Chen, L.-Y. High-temperature electronics—a role for wide bandgap semiconductors? Proc. IEEE 2002, 90, 1065–1076. [Google Scholar] [CrossRef]
- Li, C.H.; Jernigan, G.G.; Jonker, B.T.; Goswami, R.; Hellberg, C.S. Solid phase epitaxy of 3C-SiC on Si (001). US Patent 20,160,118,465, 2016. [Google Scholar]
- Pan, G.; Zhou, Y.; Luo, G.; Shi, X.; Zou, C.; Gong, H. Chemical mechanical polishing (CMP) of on-axis Si-face 6H-SiC wafer for obtaining atomically flat defect-free surface. J. Mater. Sci. 2013, 24, 5040–5047. [Google Scholar] [CrossRef]
- Tanaka, H.; Shimada, S. Damage-free machining of monocrystalline silicon carbide. CIRP Ann. 2013, 62, 55–58. [Google Scholar] [CrossRef]
- Yin, L.; Vancoille, E.Y.; Ramesh, K.; Huang, H. Surface characterization of 6H-SiC (0001) substrates in indentation and abrasive machining. Int. J. Mach. Tools Manuf. 2004, 44, 607–615. [Google Scholar] [CrossRef]
- Meng, B.; Zhang, F.; Li, Z. Deformation and removal characteristics in nanoscratching of 6H-SiC with Berkovich indenter. Mater. Sci. Semicond. Process. 2015, 31, 160–165. [Google Scholar] [CrossRef]
- Goel, S.; Luo, X.; Comley, P.; Reuben, R.L.; Cox, A. Brittle–ductile transition during diamond turning of single crystal silicon carbide. Int. J. Mach. Tools Manuf. 2013, 65, 15–21. [Google Scholar] [CrossRef]
- Yan, J.; Gai, X.; Harada, H. Subsurface Damage of Single Crystalline Silicon Carbide in Nanoindentation Tests. J. Nanosci. Nanotechnol. 2010, 10, 7808–7811. [Google Scholar] [CrossRef] [PubMed]
- Xiao, G.; To, S.; Zhang, G. The mechanism of ductile deformation in ductile regime machining of 6H SiC. Comput. Mater. Sci. 2015, 98, 178–188. [Google Scholar] [CrossRef]
- Lee, H.; Kim, D.; An, J.; Lee, H.; Kim, K.; Jeong, H. Hybrid polishing mechanism of single crystal SiC using mixed abrasive slurry (MAS). CIRP Ann. 2010, 59, 333–336. [Google Scholar] [CrossRef]
- Xiao, G.; Ren, M.; To, S. A Study of Mechanics in Brittle–Ductile Cutting Mode Transition. Micromachines 2018, 9, 49. [Google Scholar] [CrossRef]
- Li, C.; Zhang, F.; Meng, B.; Ma, Z. Simulation and Experiment on Surface Morphology and Mechanical Properties Response in Nano-Indentation of 6H-SiC. J. Mater. Eng. Perform. 2017, 26, 1000–1009. [Google Scholar] [CrossRef]
- Nawaz, A.; Mao, W.; Lu, C.; Shen, Y. Mechanical properties, stress distributions and nanoscale deformation mechanisms in single crystal 6H-SiC by nanoindentation. J. Alloy. Compd. 2017, 708, 1046–1053. [Google Scholar] [CrossRef]
- Pang, K.H.; Tymicki, E.; Roy, A. Indentation in single-crystal 6H silicon carbide: Experimental investigations and finite element analysis. Int. J. Mech. Sci. 2018, 144, 858–864. [Google Scholar] [CrossRef]
- Lu, J.; Luo, Q.; Xu, X.; Huang, H.; Jiang, F. Removal mechanism of 4H-and 6H-SiC substrates (0001 and 0001 over bar) in mechanical planarization machining. Proc. Inst. Mech. Eng. B 2019, 233, 69–76. [Google Scholar] [CrossRef]
- Chen, Y. Study on Mechanism and Key Techniques of Ion Implantation Surface Modification Method for Ultra-Precision Machining. PhD Thesis, University of Queensland, Brisbane, Australia, 2015. [Google Scholar]
- Mehregany, M.; Tong, L.; Matus, L.; Larkin, D. Internal stress and elastic modulus measurements on micromachined 3C-SiC thin films. IEEE Trans. Electron. Devices 1997, 44, 74–79. [Google Scholar] [CrossRef]
- Stevanovic, M. Modeling thermal contact resistance between elastic hemisphere and elastic layer bonded to elastic substrate. In Proceedings of the ITherm 2002. Eighth Intersociety Conference on Thermal and Thermomechanical Phenomena in Electronic Systems (Cat. No.02CH37258), San Diego, CA, USA, 30 May–1 June 2002; IEEE: Piscataway, NJ, USA, 2002. [Google Scholar]
- Ellyin, F. Fatigue Damage, Crack Growth and Life Prediction; Springer Science & Business Media: Berlin, Germany, 2012. [Google Scholar]
- Broek, D. Elementary Engineering Fracture Mechanics; Springer Science & Business Media: Berlin, Germany, 2012. [Google Scholar]
- Bradt, R.C.; Hasselman, D.P.H.; Munz, D.; Sakai, M.; Shevchenko, V.Y. (Eds.) Fracture Mechanics of Ceramics: Composites, R-Curve Behavior, and Fatigue; Springer Science & Business Media: Berlin, Germany, 2012. [Google Scholar]
- Arif, M.; Xinquan, Z.; Rahman, M.; Kumar, S. A predictive model of the critical undeformed chip thickness for ductile–brittle transition in nano-machining of brittle materials. Int. J. Mach. Tools Manuf. 2013, 64, 114–122. [Google Scholar] [CrossRef]
- Bifano, T.G.; Dow, T.A.; Scattergood, R.O. Ductile-regime grinding: a new technology for machining brittle materials. J. Eng. Ind. 1991, 113, 184–189. [Google Scholar] [CrossRef]
- Shang, G. Fundamental Research on Silicon Wafer Thinning by Ultra-precision Grinding; Dalian University of Technology: Dalian, China, 2013. [Google Scholar]
- Agarwal, S.; Rao, P.V. Experimental investigation of surface/subsurface damage formation and material removal mechanisms in SiC grinding. Int. J. Mach. Tools Manuf. 2008, 48, 698–710. [Google Scholar] [CrossRef]
- Ioan, D.M.; Eckart, U. Handbook of Lapping and Polishing (Manufacturing Engineering and Materials Processing); CRC Press: Boca Raton, FL, USA, 2006. [Google Scholar]
- Czado, C.; Schmidt, T. Mathematische Statistik; Springer: Berlin/Heidelberg, Germany, 2011. [Google Scholar]
- Evans, C.J.; Parks, R.E.; Roderick, D.J.; McGlauflin, M.L. Rapidly Renewable Lap: Theory and Practice. CIRP Ann. 1998, 47, 239–244. [Google Scholar] [CrossRef]
- Pan, J.; Yan, Q. Material removal mechanism of cluster magnetorheological effect in plane polishing. Int. J. Adv. Manuf. Technol. 2015, 81, 2017–2026. [Google Scholar] [CrossRef]
- Basim, G.B.; Vakarelski, I.U.; Moudgil, B.M. Role of interaction forces in controlling the stability and polishing performance of CMP slurries. J. Colloid Interface Sci. 2003, 263, 506–515. [Google Scholar] [CrossRef]
- Feng, Z. Foundation of mathematics model of magnetorheological finishing. Opt. Tech. 2000, 26, 190–192. [Google Scholar]
- Yang, P. Numerical Analysis of Fluid Lubrication; National Defense Industry Press: Beijing, China, 1998. [Google Scholar]
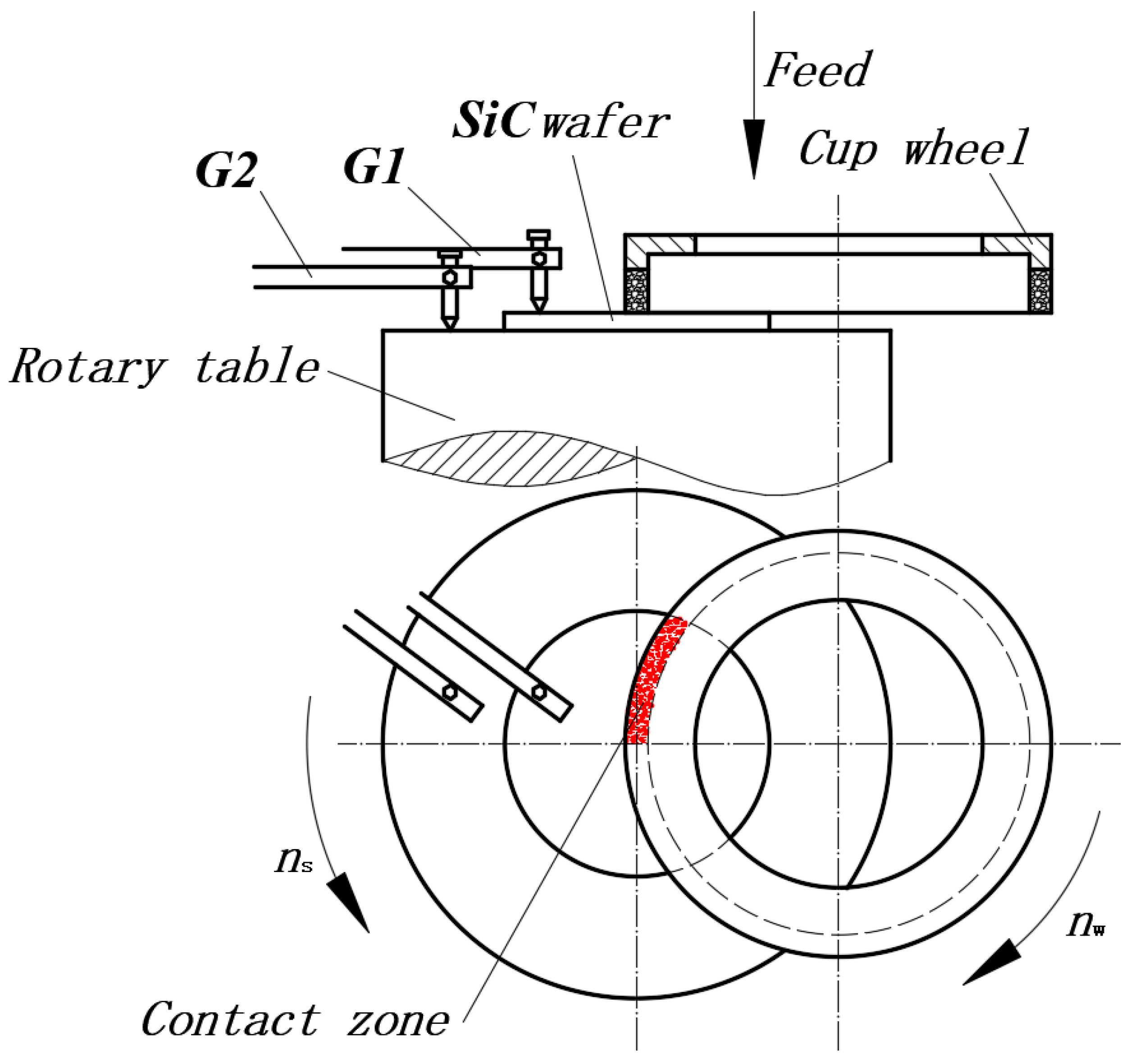

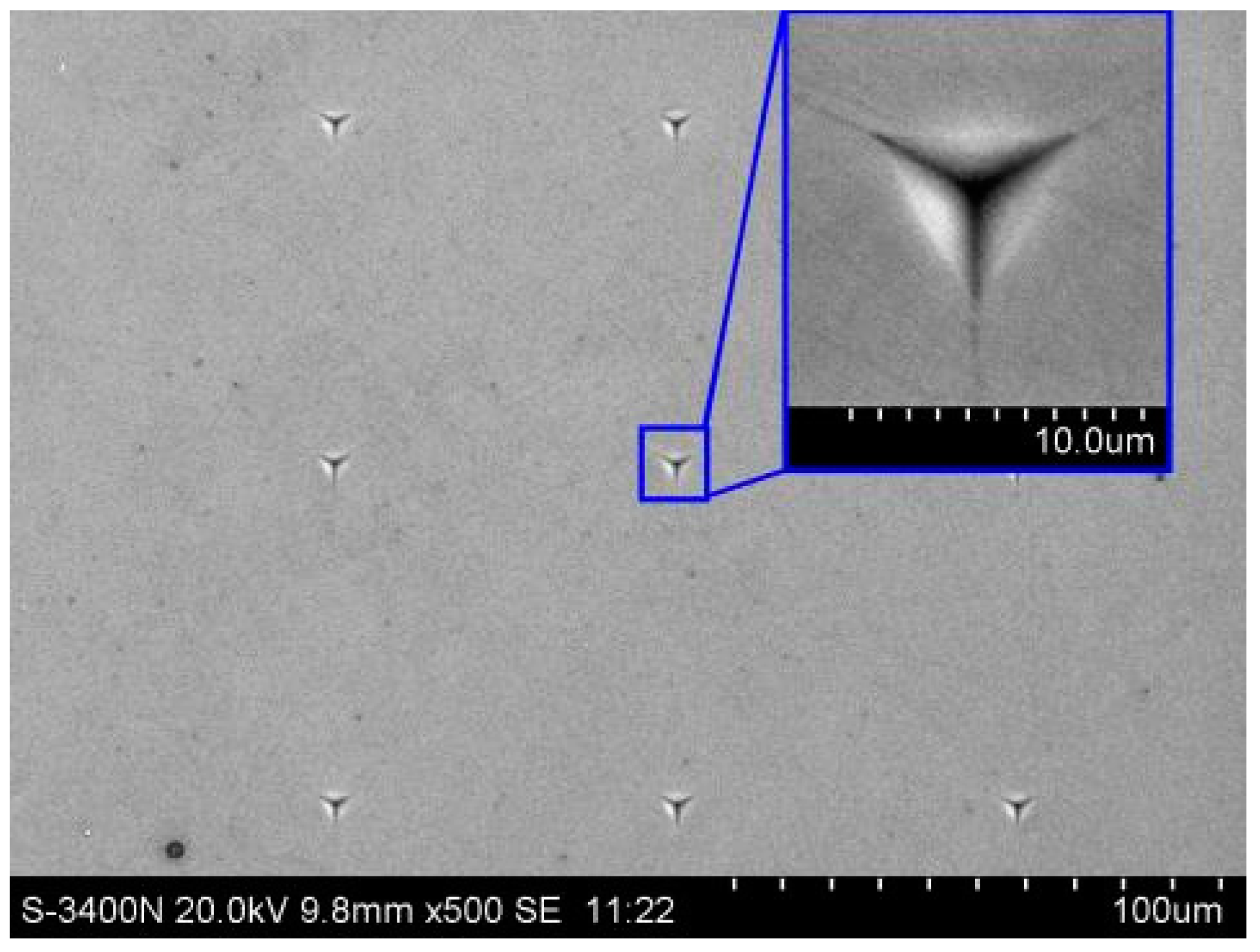
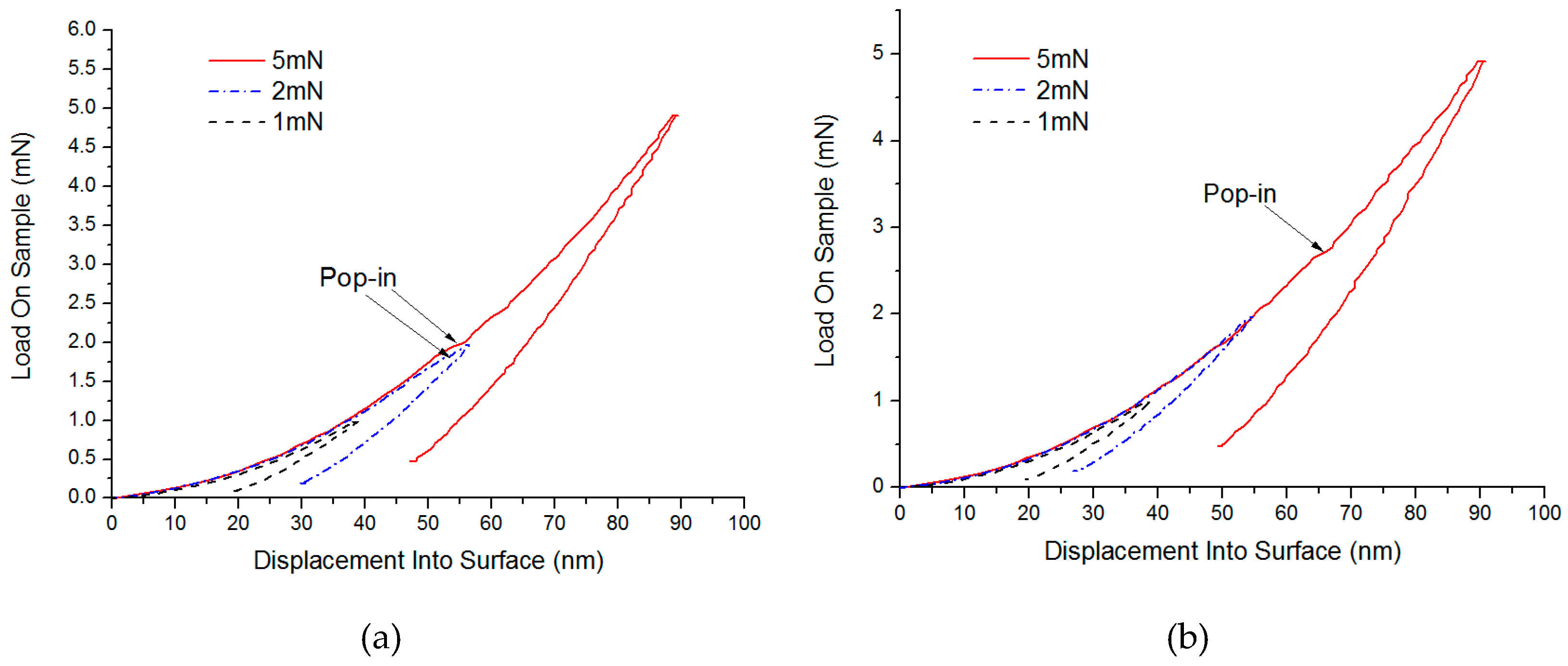
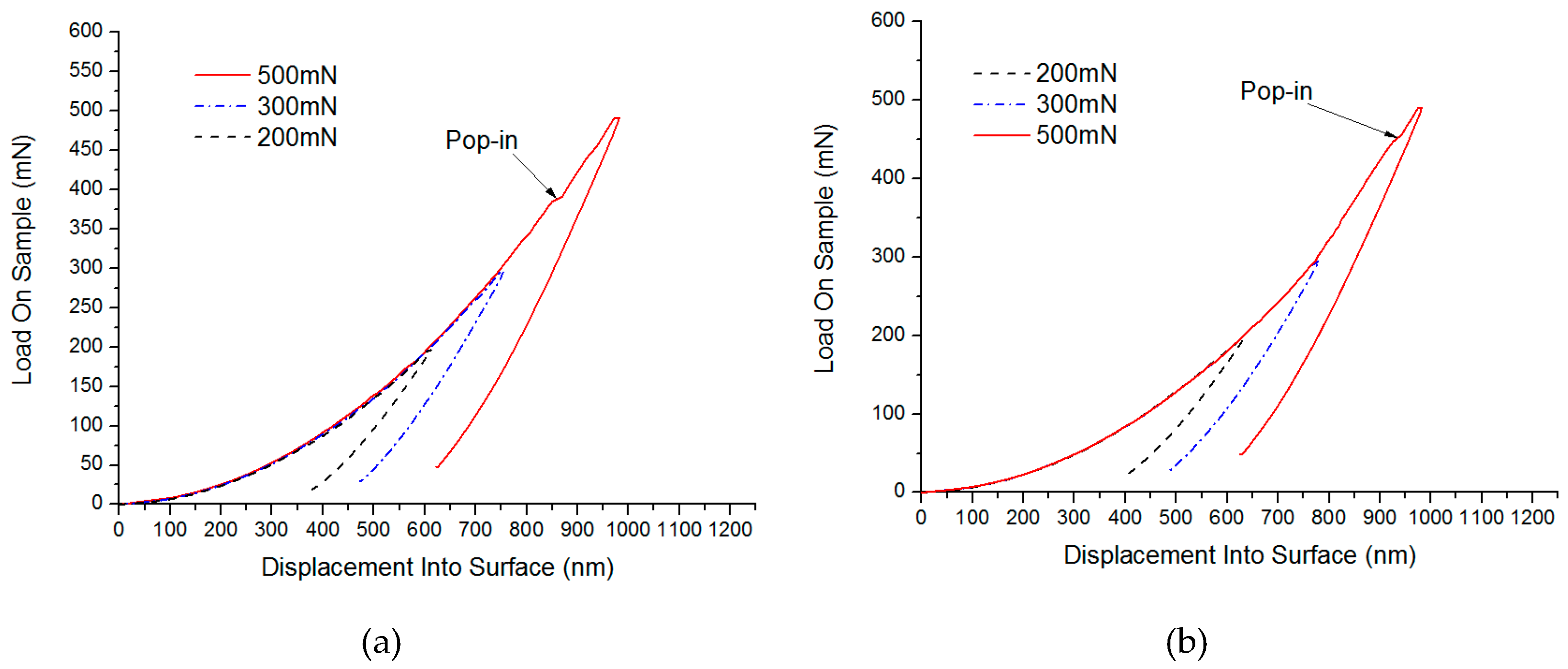




| Process No. | Grinding Wheel Type (Abrasive Grain Size) | Feed Rate fs (µm/s) | ||
|---|---|---|---|---|
| No.1 | 325# (45µm) | 5 | 151 | 1800 |
| No.2 | 325# (45µm) | 0.1 | 151 | 1800 |
| No.3 | 325# (45µm) | 0.1 | 151 | 3200 |
| No.4 | 8000# (1.6µm) | 0.1 | 151 | 3200 |
| Loads | 1 mN | 2 mN | 5 mN | 10 mN | 20 mN | 50 mN | 100 mN | 200 mN | 300 mN | 500 mN | |
|---|---|---|---|---|---|---|---|---|---|---|---|
| Hardness/ Elasticity Modulus | |||||||||||
| Hardness of C face (Gpa) | 47.867 | 55.418 | 55.825 | 52.596 | 50.514 | 46.745 | 43.741 | 41.435 | 39.861 | 38.596 | |
| Elasticity modulus of C face (Gpa) | 538.075 | 581.841 | 627.822 | 618.019 | 612.893 | 596.833 | 576.843 | 567.615 | 562.4 | 563.019 | |
| Hardness of Si face (Gpa) | 48.211 | 46.381 | 50.788 | 48.446 | 45.693 | 42.392 | 39.651 | 37.315 | 36.311 | 36.246 | |
| Elasticity modulus of Si face (Gpa) | 533.033 | 554.146 | 614.533 | 601.814 | 589.13 | 570.998 | 548.221 | 535.773 | 525.946 | 524.839 | |
| Parameter | Value |
|---|---|
| Thickness of workpiece t (mm) | 0.3 |
| Machining gap (mm) | 0.8 |
| Abrasive diameter (μm) | 2.8 |
| The initial viscosity of the MR fluid (Pa·s) | 0.5 |
| The magnetoconductivity of magnetic particles | 2000 |
| Vacuum permeability | 1 |
| Magnetic field intensity Hm (Gs) | 2000 |
| Speed of polishing disk v (m/s) | 1.27 |
| The proportion of magnetic particles in the MR fluid | 0.33 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Pan, J.; Yan, Q.; Li, W.; Zhang, X. A Nanomechanical Analysis of Deformation Characteristics of 6H-SiC Using an Indenter and Abrasives in Different Fixed Methods. Micromachines 2019, 10, 332. https://doi.org/10.3390/mi10050332
Pan J, Yan Q, Li W, Zhang X. A Nanomechanical Analysis of Deformation Characteristics of 6H-SiC Using an Indenter and Abrasives in Different Fixed Methods. Micromachines. 2019; 10(5):332. https://doi.org/10.3390/mi10050332
Chicago/Turabian StylePan, Jisheng, Qiusheng Yan, Weihua Li, and Xiaowei Zhang. 2019. "A Nanomechanical Analysis of Deformation Characteristics of 6H-SiC Using an Indenter and Abrasives in Different Fixed Methods" Micromachines 10, no. 5: 332. https://doi.org/10.3390/mi10050332
APA StylePan, J., Yan, Q., Li, W., & Zhang, X. (2019). A Nanomechanical Analysis of Deformation Characteristics of 6H-SiC Using an Indenter and Abrasives in Different Fixed Methods. Micromachines, 10(5), 332. https://doi.org/10.3390/mi10050332





