Classification of Hydration in Clinical Conditions: Indirect and Direct Approaches Using Bioimpedance
Abstract
1. Introduction
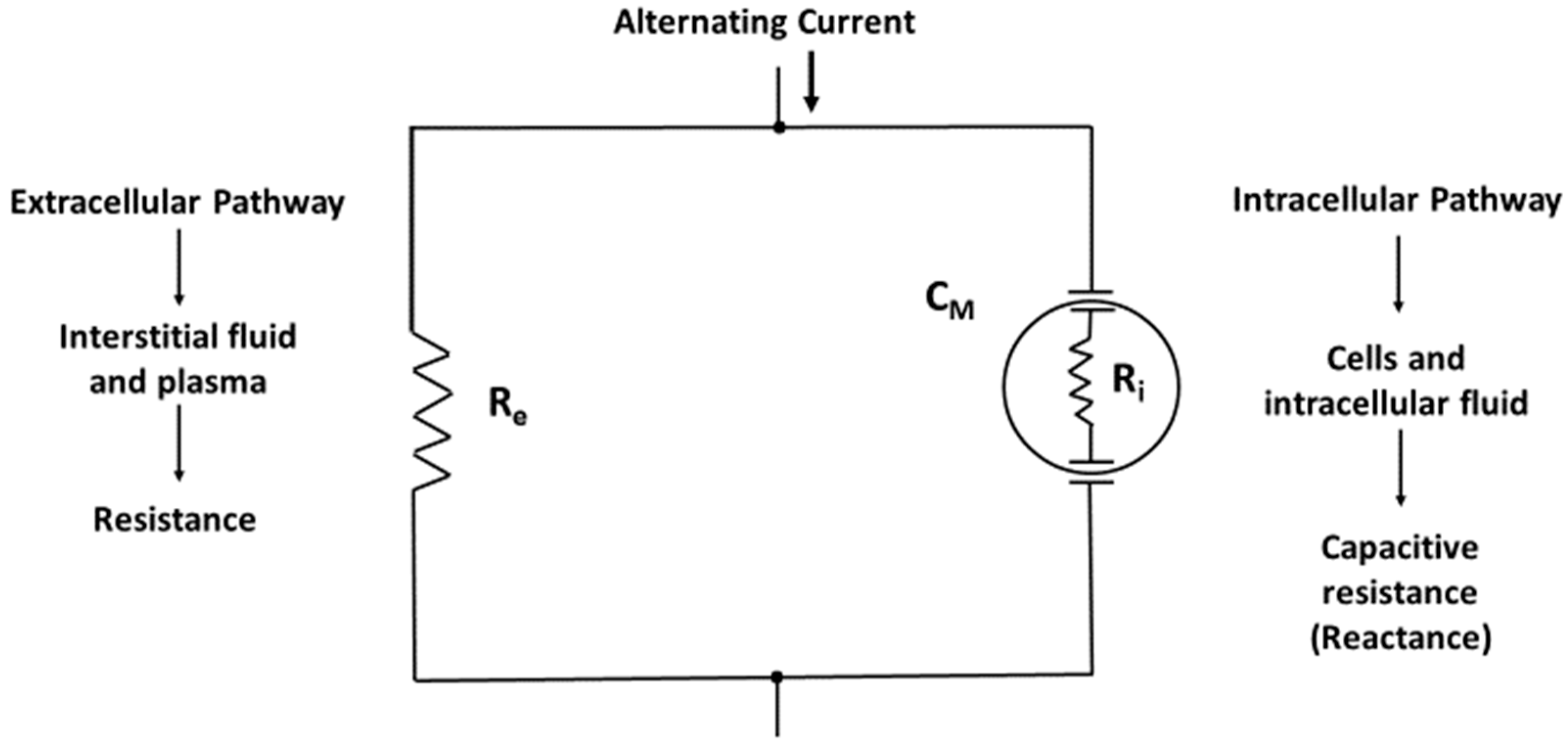
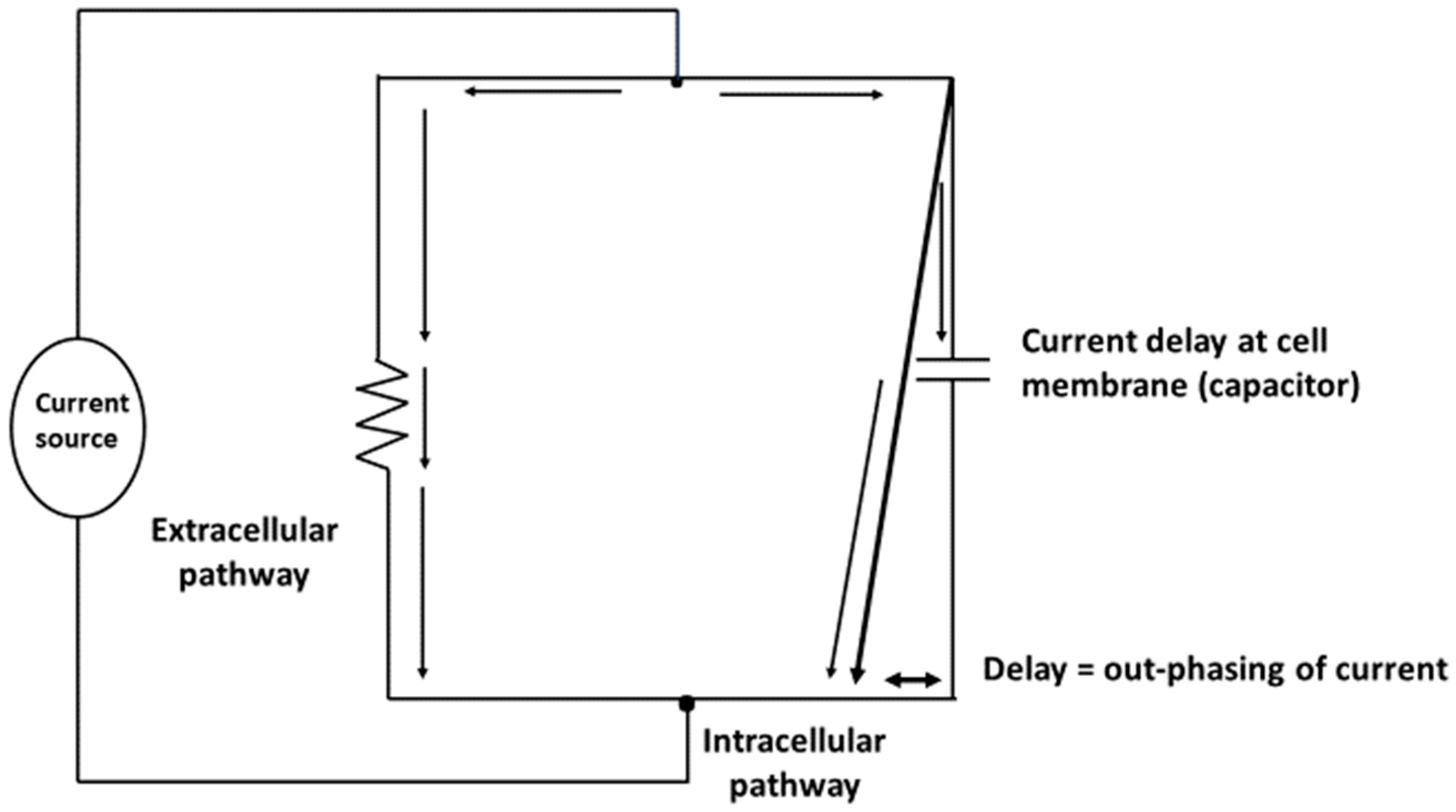
2. Bioelectrical Impedance
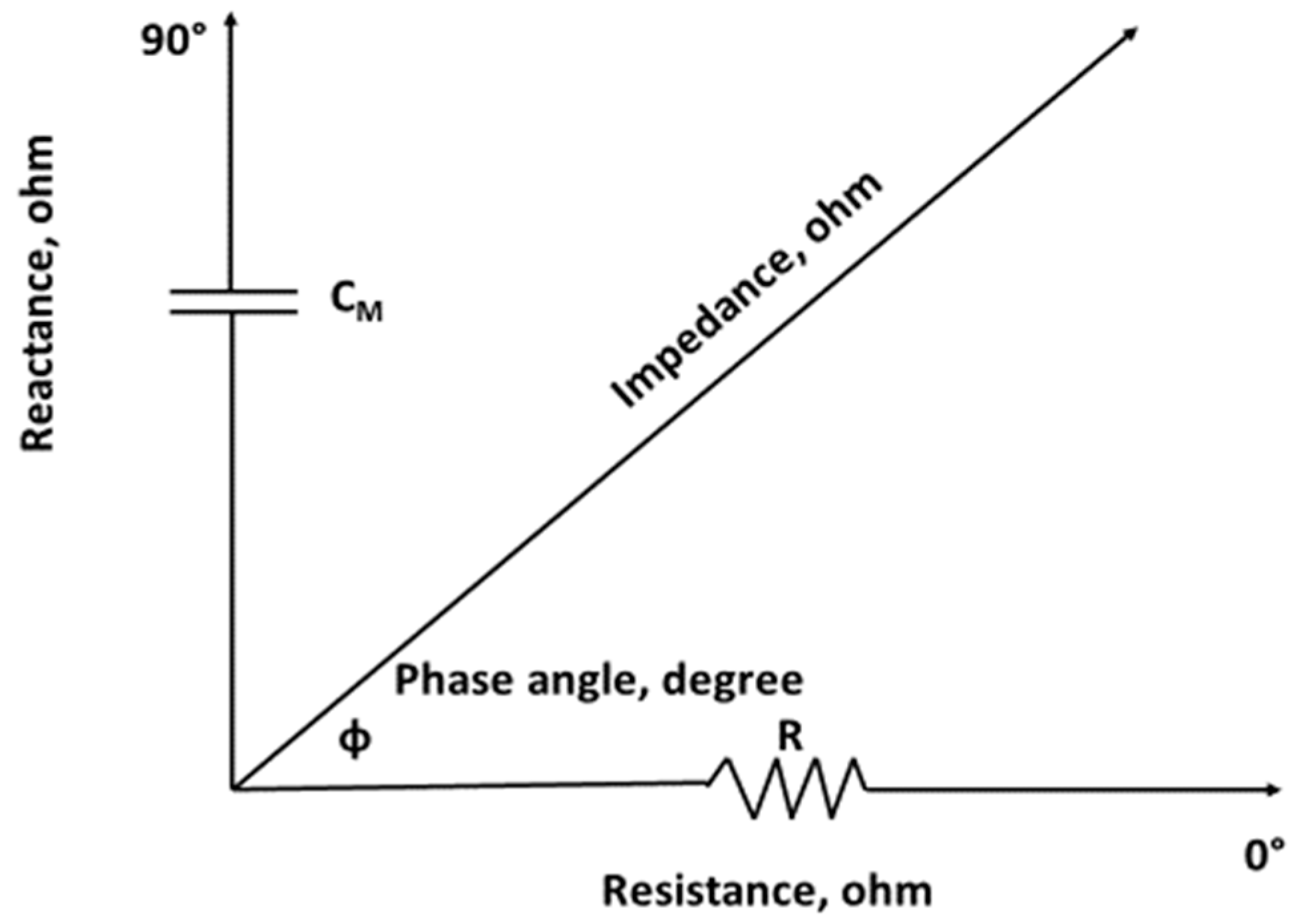
2.1. Bioimpedance Basics
2.2. Measurement of Bioelectrical Impedance
3. Volume Quantification in Hydration Assessment: Limitations and Imprecision
3.1. Single-Frequency Bioimpedance
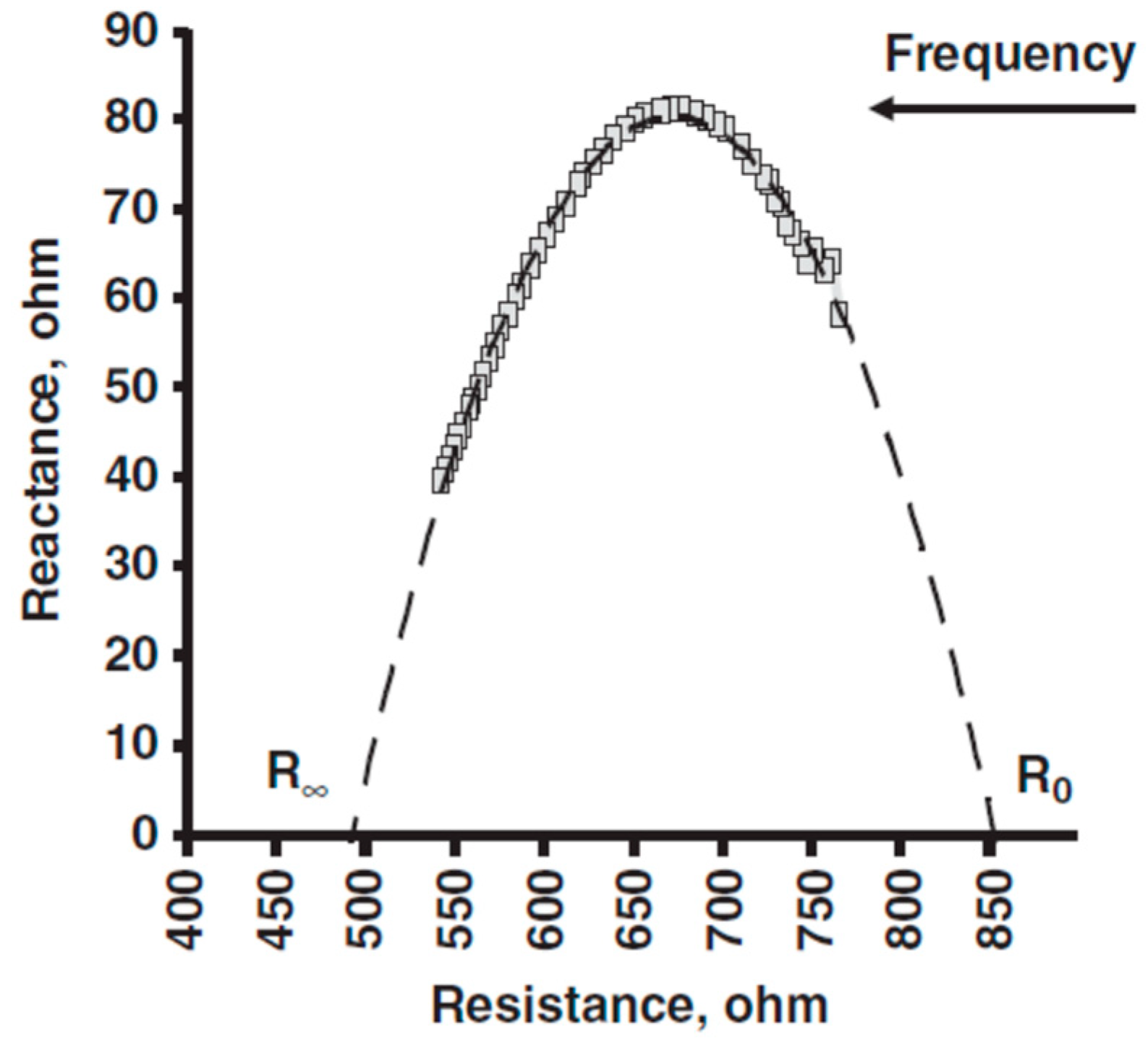
3.2. Bioelectrical Impedance Spectroscopy (BIS)
3.3. Comparison of Fluid Volumes Estimated with Single-Frequency Bioimpedance and Bioelectrical Impedance Spectroscopy
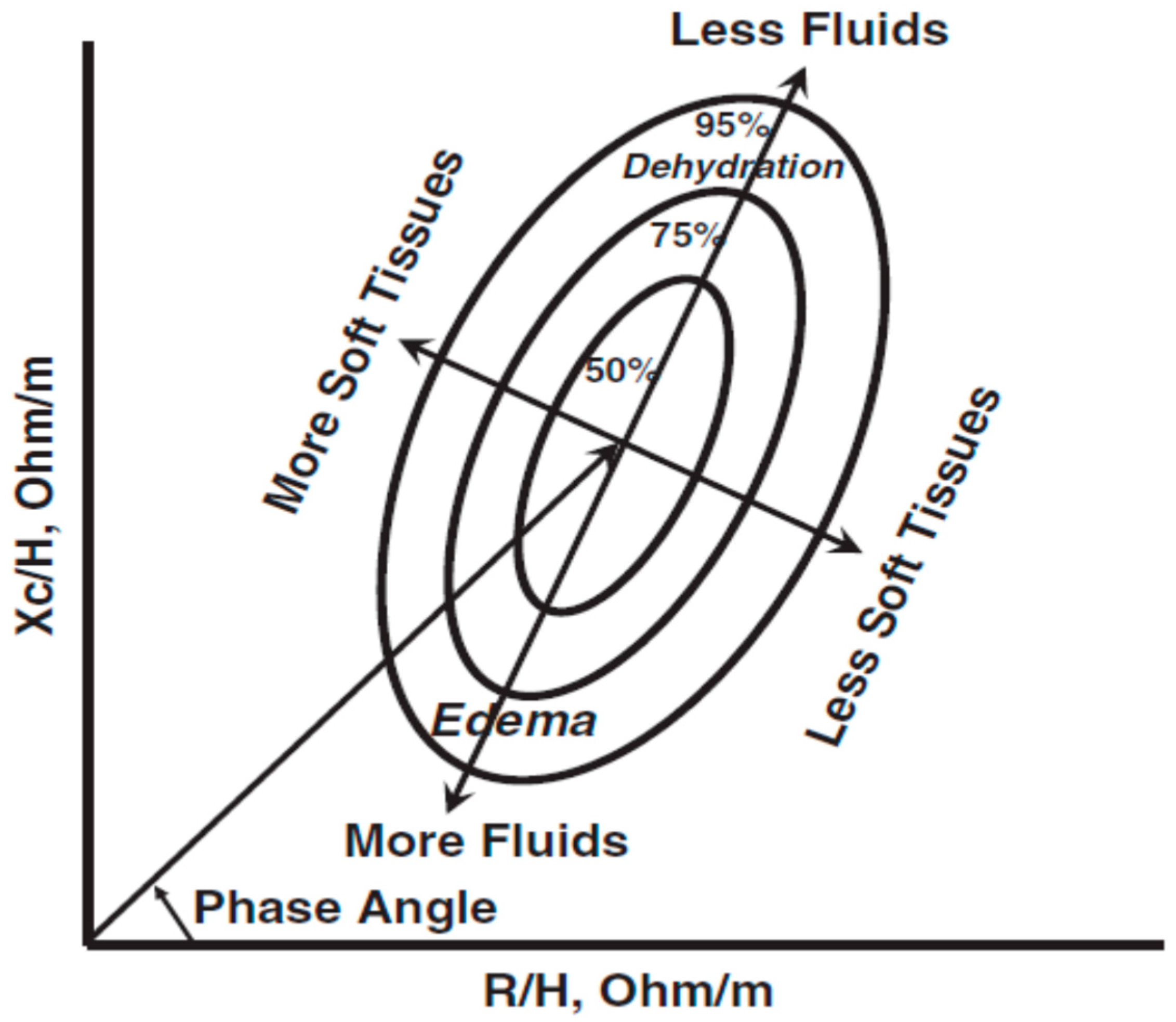
4. Classification of Hydration with Bioelectrical Impedance Vector Analysis (BIVA)
4.1. Clinical Applications of BIVA
4.1.1. Hydration Assessment in Hemodialysis (HD)
4.1.2. Hydration Changes and Vector Patterns During the HD Cycle
4.1.3. Vector Trajectories: Adequacy of Ultrafiltration (UF)
4.2. BIVA in Peritoneal Dialysis (PD)
4.3. BIVA in Critically Ill Patients
4.4. BIVA in Congested Heart Failure (HF)
5. Bioelectrical Impedance Spectroscopy (BIS)
BIS in Dialysis
6. BIVA in Nutrition Assessment
7. Equivalence of Bioimpedance Measurements
Standardization of BI Measurements
8. Summary and Conclusions
9. Future Directions
Author Contributions
Funding
Conflicts of Interest
References
- Armstrong, L.E. Assessing hydration status: The elusive gold standard. J. Am. Coll. Nutr. 2007, 26 (Suppl. 5), 575S–584S. [Google Scholar] [CrossRef] [PubMed]
- Di Somma, S.; Navarin, S.; Giordano, S.; Spadini, F.; Lippi, G.; Cervellin, G.; Dieffenbach, B.V.; Maisel, A.S. The emerging role of biomarkers and bio-impedance in evaluating hydration status in patients with acute heart failure. Clin. Chem. Lab. Med. 2012, 50, 2093–2105. [Google Scholar] [CrossRef]
- Moore, F.D.; Boyden, C.M. Body cell mass and limits of hydration of the fat-free body: Their relation to estimated skeletal weight. Ann. N. Y. Acad. Sci. 1963, 110, 62–71. [Google Scholar] [CrossRef] [PubMed]
- Snyder, W.S.; Cook, M.J.; Nasset, E.S.; Karhausen, L.R.; Howells, G.P.; Tipton, I.H. Report of the Task Group on Reference Man; Pergamon Press: Oxford, UK, 1975; pp. 27–32. [Google Scholar]
- Wang, Z.; Deurenberg, P.; Wang, W.; Pietrobelli, A.; Baumgartner, R.N.; Heymsfield, S.B. Hydration of fat-free body mass: Review and critique of a classic body-composition constant. Am. J. Clin. Nutr. 1999, 69, 833–841. [Google Scholar] [CrossRef] [PubMed]
- Chumlea, W.C.; Schubert, C.M.; Sun, S.S.; Demerath, E.; Towne, B.; Siervogel, R.M. A review of body water status and the effects of age and body fatness in children and adults. J. Nutr. Health Aging 2007, 11, 111–118. [Google Scholar]
- Armstrong, L.E.; Kavouras, S.A.; Walsh, N.P.; Roberts, W.O. Diagnosing dehydration? Blend evidence with clinical observations. Curr. Opin. Clin. Nutr. Metab. Care 2016, 19, 434–438. [Google Scholar] [CrossRef]
- Lukaski, H.C. Biological indexes considered in the derivation of the bioelectrical impedance analysis. Am. J. Clin. Nutr. 1996, 64 (Suppl. 3), 397S–404S. [Google Scholar] [CrossRef]
- Kyle, U.G.; Bosaeus, I.; De Lorenzo, A.D.; Deurenberg, P.; Elia, M.; Gómez, J.M.; Heitmann, B.L.; Kent-Smith, L.; Melchior, J.C.; Pirlich, M.; et al. Composition of the ESPEN Working Group. Bioelectrical impedance analysis-part I: Review of principles and methods. Eur. J. Clin. Nutr. 2004, 23, 1226–1243. [Google Scholar] [CrossRef]
- Lukaski, H.C. Evolution of bioimpedance: A circuitous journey from estimation of physiological function to assessment of body composition and a return to clinical research. Eur. J. Clin. Nutr. 2013, 67 (Suppl. 1), S2–S9. [Google Scholar] [CrossRef] [PubMed]
- Faes, T.J.; van der Meij, H.A.; de Munck, J.C.; Heethaar, R.M. The electric resistivity of human tissues (100 Hz–10 MHz): A meta-analysis of review studies. Physiol. Meas. 1999, 20, R1–R10. [Google Scholar] [CrossRef]
- Foster, K.R.; Lukaski, H.C. Whole-body impedance—What does it measure? Am. J. Clin. Nutr. 1996, 64 (Suppl. 3), 388S–396S. [Google Scholar] [CrossRef] [PubMed]
- Grimnes, S.; Martinsen, O. Bioimpedance and Bioelectricity Basics, 3rd ed.; Elsevier Ltd.: London, UK, 2015; pp. 1–8. [Google Scholar]
- Racette, S.B.; Schoeller, D.A.; Luke, A.H.; Shay, K.; Hnilicka, J.; Kushner, R.F. Relative dilution spaces of 2H-and 18O-labeled water in humans. Am. J. Physiol. 1994, 267, E585–E590. [Google Scholar] [CrossRef]
- Schoeller, D.A. Hydrometry. In Human Body Composition, 2nd ed.; Heymsfield, S.B., Lohman, T.G., Wang, Z.M., Going, S.B., Eds.; Human Kinetics: Champaign, IL, USA, 2005; pp. 35–50. [Google Scholar]
- Piccoli, A.; Pastori, G.; Guizzo, M.; Rebeschini, M.; Naso, A.; Cascone, C. Equivalence of information from single versus multiple frequency bioimpedance vector analysis in hemodialysis. Kidney Int. 2005, 67, 301–313. [Google Scholar] [CrossRef]
- Matthie, J.R. Bioimpedance measurements of human body composition: Critical analysis and outlook. Expert Rev. Med. Devices 2008, 5, 239–261. [Google Scholar] [CrossRef] [PubMed]
- Kyle, U.G.; Bosaeus, I.; De Lorenzo, A.D.; Deurenberg, P.; Elia, M.; Gómez, J.M.; Lilienthal Heitmann, B.; Kent-Smith, L.; Melchior, J.C.; Pirlich, M.; et al. Bioelectrical impedance analysis-part II: Utilization in clinical practice. Eur. J. Clin. Nutr. 2004, 23, 1430–1453. [Google Scholar] [CrossRef] [PubMed]
- Mulasi, U.; Kuchnia, A.J.; Cole, A.J.; Earthman, C.P. Bioimpedance at the bedside: Current applications, limitations, and opportunities. Nutr. Clin. Pract. 2015, 30, 180–193. [Google Scholar] [CrossRef] [PubMed]
- Sun, S.S.; Chumlea, W.C.; Heymsfield, S.B.; Lukaski, H.C.; Schoeller, D.; Friedl, K.; Kuczmarski, R.J.; Flegal, K.M.; Johnson, C.L.; Hubbard, V.S. Development of bioelectrical impedance analysis prediction equations for body composition with the use of a multicomponent model for use in epidemiological surveys. Am. J. Clin. Nutr. 2003, 77, 331–340. [Google Scholar] [CrossRef]
- Ellis, K.J.; Wong, W.W. Human hydrometry: Comparison of multifrequency bioelectrical impedance with 2H2O and bromine dilution. J. Appl. Physiol. 1998, 85, 1056–1062. [Google Scholar] [CrossRef]
- Ellis, K.J.; Bell, S.J.; Chertow, G.M.; Chumlea, W.C.; Knox, T.A.; Kotler, D.P.; Lukaski, H.C.; Schoeller, D.A. Bioelectrical impedance methods in clinical research: A follow-up to the NIH Technology Assessment Conference. Nutrition 1999, 15, 874–880. [Google Scholar] [CrossRef]
- Ellis, K.J. Human body composition: In vivo methods. Physiol. Rev. 2000, 80, 649–680. [Google Scholar] [CrossRef] [PubMed]
- Cole, K.S. Permeability and impermeability of cell membranes for ions. In Cold Spring Harbor Symposia on Quantitative Biology; Cold Spring Harbor Laboratory Press: Cold Spring Harbor, NY, USA, 1940; Volume 8, pp. 110–122. [Google Scholar]
- Hanai, T. Electrical properties of emulsions. In Emulsion Science; Sherman, P.H., Ed.; Academic Press: London, UK, 1968; pp. 354–477. [Google Scholar]
- De Lorenzo, A.; Andreoli, A.; Matthie, J.; Withers, P. Predicting body cell mass with bioimpedance by using theoretical methods: A technological review. J. Appl. Physiol. 1997, 82, 1542–1558. [Google Scholar] [CrossRef]
- Bolton, M.P.; Ward, L.C.; Khan, A.; Campbell, I.; Nightingale, P.; Dewit, O.; Elia, M. Sources of error in bioimpedance spectroscopy. Physiol. Meas. 1998, 19, 235–246. [Google Scholar] [CrossRef]
- Buendia, R.; Seoane, F.; Lindecrantz, K.; Bosaeus, I.; Gil-Pita, R.; Johannsson, G.; Ellegárd, L.; Ward, L.C. Estimation of body fluids with bioimpedance spectroscopy: State of the art methods and proposal of novel methods. Physiol. Meas. 2015, 36, 2171–2187. [Google Scholar] [CrossRef]
- Ward, L.C.; Isenring, E.; Dyer, J.M.; Kagawa, M.; Essex, T. Resistivity coefficients for body composition analysis using bioimpedance spectroscopy: Effects of body dominance and mixture theory algorithm. Physiol. Meas. 2015, 36, 1529–1549. [Google Scholar] [CrossRef]
- Cox-Reijven, P.L.; Soeters, P.B. Validation of bio-impedance spectroscopy: Effects of degree of obesity and ways of calculating volumes from measured resistance values. Int. J. Obes. Relat. Metab. Disord. 2000, 24, 271–280. [Google Scholar] [CrossRef]
- Cox-Reijven, P.L.; van Kreel, B.; Soeters, P.B. Accuracy of bioelectrical impedance spectroscopy in measuring changes in body composition during severe weight loss. J. Parenter. Enter. Nutr. 2002, 26, 120–127. [Google Scholar] [CrossRef]
- Mager, J.R.; Sibley, S.D.; Beckman, T.R.; Kellogg, T.A.; Earthman, C.P. Multifrequency bioelectrical impedance analysis and bioimpedance spectroscopy for monitoring fluid and body cell mass changes after gastric bypass surgery. Clin. Nutr. 2008, 27, 832–841. [Google Scholar] [CrossRef]
- Moissl, U.M.; Wabel, P.; Chamney, P.W.; Bosaeus, I.; Levin, N.W.; Bosy-Westphal, A.; Korth, O.; Müller, M.J.; Ellegård, L.; Malmros, V.; et al. Body fluid volume determination via body composition spectroscopy in health and disease. Physiol. Meas. 2006, 27, 921–933. [Google Scholar] [CrossRef]
- Gonzalez, M.C.; Correia, M.I.T.D.; Heymsfield, S.B. A requiem for BMI in the clinical setting. Curr. Opin. Clin. Nutr. Metab. Care 2017, 20, 314–321. [Google Scholar] [CrossRef]
- Raimann, J.G.; Zhu, F.; Wang, J.; Thijssen, S.; Kuhlmann, M.K.; Kotanko, P.; Levin, N.W.; Kaysen, G.A. Comparison of fluid volume estimates in chronic hemodialysis patients by bioimpedance, direct isotopic, and dilution methods. Kidney Int. 2014, 85, 898–908. [Google Scholar] [CrossRef]
- Raimann, J.G.; Abbas, S.R.; Liu, L.; Zhu, F.; Larive, B.; Kotanko, P.; Levin, N.W.; Kaysen, G.A.; FHN Trial. Agreement of single-and multi-frequency bioimpedance measurements in hemodialysis patients: An ancillary study of the Frequent Hemodialysis Network Daily Trial. Nephron Clin. Pract. 2014, 128, 115–126. [Google Scholar] [CrossRef]
- Piccoli, A. Estimation of fluid volumes in hemodialysis patients: Comparing bioimpedance with isotopic dilution methods. Kidney Int. 2014, 85, 738–741. [Google Scholar] [CrossRef]
- Seoane, F.; Abtahi, S.; Abtahi, F.; Ellegárd, L.; Johannsson, G.; Bosaeus, I.; Ward, L.C. Slightly superior performance of bioimpedance spectroscopy over single frequency regression equations for assessment of total body water. In Proceedings of the IEEE Engineering in Medicine and Biology Society (EMBC), Milan, Italy, 25–29 August 2015; pp. 3707–3710. [Google Scholar]
- Seoane, F.; Abtahi, S.; Abtahi, F.; Ellegárd, L.; Johannsson, G.; Bosaeus, I.; Ward, L.C. Mean expected error in prediction of total body water: A true accuracy comparison between bioimpedance spectroscopy and single frequency regression equations. Biomed. Res. Int. 2015, 11, 656323. [Google Scholar] [CrossRef]
- Piccoli, A.; Rossi, B.; Pillon, L.; Bucciante, G. A new method for monitoring body fluid variation by bioimpedance analysis: The RXc graph. Kidney Int. 1994, 46, 534–539. [Google Scholar] [CrossRef]
- Chumlea, W.C.; Guo, S.S.; Kuczmarski, R.J.; Johnson, C.L.; Flegel, K.; Heymsfield, S.; Lukaski, H.; Schoeller, D.; Friedl, K.; Hubbard, V.S. Body composition estimates from NHANES III bioelectrical impedance data. Int. J. Obes. 2002, 26, 1596–1609. [Google Scholar] [CrossRef]
- Nescolarde, L.; Lukaski, H.; De Lorenzo, A.; de-Mateo-Silleras, B.; Redondo-Del-Río, M.P.; Camina-Martín, M.A. Different displacement of bioimpedance vector due to Ag/AgCl electrode effect. Eur. J. Clin. Nutr. 2016, 70, 1401–1407. [Google Scholar] [CrossRef]
- Pillon, L.; Piccoli, A.; Lowrie, E.G.; Lazarus, J.M.; Chertow, G.M. Vector length as a proxy for the adequacy of ultrafiltration in hemodialysis. Kidney Int. 2004, 66, 1266–1271. [Google Scholar] [CrossRef]
- Piccoli, A.; Codognotto, M. Bioimpedance vector migration up to three days after the hemodialysis session. Kidney Int. 2004, 66, 2091–2092. [Google Scholar] [CrossRef]
- Piccoli, A. Whole body--single frequency bioimpedance. Contrib. Nephrol. 2005, 149, 150–161. [Google Scholar]
- Piccoli, A. Identification of operational clues to dry weight prescription in hemodialysis using bioimpedance vector analysis. The Italian Hemodialysis-Bioelectrical Impedance Analysis (HD-BIA) Study Group. Kidney Int. 1998, 53, 1036–1043. [Google Scholar] [CrossRef]
- Piccoli, A.; Italian CAPD-BIA Study Group. Bioelectric impedance vector distribution in peritoneal dialysis patients with different hydration status. Kidney Int. 2004, 65, 1050–1063. [Google Scholar] [CrossRef]
- Guyton, A.C. Textbook of Medical Physiology; Saunders: Philadelphia, PA, USA, 1991; p. 282. [Google Scholar]
- Piccoli, A.; Pittoni, G.; Facco, E.; Favaro, E.; Pillon, L. Relationship between central venous pressure and bioimpedance vector analysis in critically ill patients. Crit. Care Med. 2000, 28, 132–137. [Google Scholar] [CrossRef]
- Basso, F.; Berdin, G.; Virzì, G.M.; Mason, G.; Piccinni, P.; Day, S.; Cruz, D.N.; Wjewodzka, M.; Giuliani, A.; Brendolan, A.; et al. Fluid management in the intensive care unit: Bioelectrical impedance vector analysis as a tool to assess hydration status and optimal fluid balance in critically ill patients. Blood Purif. 2013, 36, 192–199. [Google Scholar] [CrossRef]
- Samoni, S.; Vigo, V.; Reséndiz, L.I.; Villa, G.; De Rosa, S.; Nalesso, F.; Ferrari, F.; Meola, M.; Brendolan, A.; Malacarne, P.; et al. Impact of hyperhydration on the mortality risk in critically ill patients admitted in intensive care units: Comparison between bioelectrical impedance vector analysis and cumulative fluid balance recording. Crit. Care 2016, 20, 95. [Google Scholar] [CrossRef]
- Valle, R.; Aspromonte, N.; Milani, L.; Peacock, F.W.; Maisel, A.S.; Santini, M.; Ronco, C. Optimizing fluid management in patients with acute decompensated heart failure (ADHF): The emerging role of combined measurement of body hydration status and brain natriuretic peptide (BNP) levels. Heart Fail. Rev. 2011, 16, 519–559. [Google Scholar] [CrossRef]
- Bouchard, J.; Soroko, S.B.; Chertow, G.M.; Himmelfarb, J.; Ikizler, T.A.; Paganini, E.P.; Mehta, R.L.; Program to Improve Care in Acute Renal Disease (PICARD) Study Group. Fluid accumulation, survival and recovery of kidney function in critically ill patients with acute kidney injury. Kidney Int. 2009, 76, 422–427. [Google Scholar] [CrossRef]
- Hise, A.C.D.R.; Gonzalez, M.C. Assessment of hydration status using bioelectrical impedance vector analysis in critical patients with acute kidney injury. Clin. Nutr. 2018, 37, 695–700. [Google Scholar] [CrossRef]
- Maioli, M.; Toso, A.; Leoncini, M.; Musilli, N.; Bellandi, F.; Rosner, M.H.; McCullough, P.A.; Ronco, C. Pre-procedural bioimpedance vectorial analysis of fluid status and prediction of contrast-induced acute kidney injury. J. Am. Coll. Cardiol. 2014, 63, 1387–1394. [Google Scholar] [CrossRef]
- Maioli, M.; Toso, A.; Leoncini, M.; Musilli, N.; Grippo, G.; Ronco, C.; McCullough, P.A.; Bellandi, F. Bioimpedance-Guided Hydration for the Prevention of Contrast-Induced Kidney Injury: The HYDRA Study. J. Am. Coll. Cardiol. 2018, 71, 2880–2889. [Google Scholar] [CrossRef]
- Di Somma, S.; Lalle, I.; Magrini, L.; Russo, V.; Navarin, S.; Castello, L.; Avanzi, G.C.; Di Stasio, E.; Maisel, A. Additive diagnostic and prognostic value of bioelectrical impedance vector analysis (BIVA) to brain natriuretic peptide ‘grey-zone’ in patients with acute heart failure in the emergency department. Eur. Heart J. Acute Cardiovasc. Care 2014, 3, 167–175. [Google Scholar] [CrossRef]
- Massari, F.; Scicchitano, P.; Ciccone, M.M.; Caldarola, P.; Aspromonte, N.; Iacoviello, M.; Barro, S.; Pantano, I.; Valle, R. Bioimpedance vector analysis predicts hospital length of stay in acute heart failure. Nutrition 2018, 61, 56–60. [Google Scholar] [CrossRef]
- Núñez, J.; Mascarell, B.; Stubbe, H.; Ventura, S.; Bonanad, C.; Bodí, V.; Núñez, E.; Miñana, G.; Fácila, L.; Bayés-Genis, A.; et al. Bioelectrical impedance vector analysis and clinical outcomes in patients with acute heart failure. J. Cardiovasc. Med. 2016, 17, 283–290. [Google Scholar] [CrossRef]
- Santarelli, S.; Russo, V.; Lalle, I.; De Berardinis, B.; Navarin, S.; Magrini, L.; Piccoli, A.; Codognotto, M.; Castello, L.M.; Avanzi, G.C.; et al. Usefulness of combining admission brain natriuretic peptide (BNP) plus hospital discharge bioelectrical impedance vector analysis (BIVA) in predicting 90 days cardiovascular mortality in patients with acute heart failure. Intern. Emerg. Med. 2017, 12, 445–451. [Google Scholar] [CrossRef]
- Piccoli, A.; Codognotto, M.; Cianci, V.; Vettore, G.; Zaninotto, M.; Plebani, M.; Maisel, A.; Peacock, W.F. Differentiation of cardiac and noncardiac dyspnea using bioelectrical impedance vector analysis (BIVA). J. Card. Fail. 2012, 18, 226–232. [Google Scholar] [CrossRef]
- Davies, S.J.; Davenport, A. The role of bioimpedance and biomarkers in helping to aid clinical decision-making of volume assessments in dialysis patients. Kidney Int. 2014, 86, 489–496. [Google Scholar] [CrossRef]
- Chamney, P.W.; Wabel, P.; Moissl, U.M.; Müller, M.J.; Bosy-Westphal, A.; Korth, O.; Fuller, N.J. A whole-body model to distinguish excess fluid from the hydration of major body tissues. Am. J. Clin. Nutr. 2007, 85, 80–89. [Google Scholar] [CrossRef]
- Wabel, P.; Moissl, U.; Chamney, P.; Jirka, T.; Machek, P.; Ponce, P.; Taborsky, P.; Tetta, C.; Velasco, N.; Vlasak, J.; et al. Towards improved cardiovascular management: The necessity of combining blood pressure and fluid overload. Nephrol. Dial. Transplant. 2008, 23, 2965–2971. [Google Scholar] [CrossRef]
- Oei, E.L.; Fan, S.L. Practical aspects of volume control in chronic kidney disease using whole body bioimpedance. Blood Purif. 2015, 39, 32–36. [Google Scholar] [CrossRef]
- O’Lone, E.L.; Visser, A.; Finney, H.; Fan, S.L. Clinical significance of multi-frequency bioimpedance spectroscopy in peritoneal dialysis patients: Independent predictor of patient survival. Nephrol. Dial. Transplant. 2014, 29, 1430–1437. [Google Scholar] [CrossRef]
- Wabel, P.; Chamney, P.; Moissl, U.; Jirka, T. Importance of whole-body bioimpedance spectroscopy for the management of fluid balance. Blood Purif. 2009, 7, 75–80. [Google Scholar] [CrossRef]
- Wizemann, V.; Wabel, P.; Chamney, P.; Zaluska, W.; Moissl, U.; Rode, C.; Malecka-Masalska, T.; Marcelli, D. The mortality risk of overhydration in haemodialysis patients. Nephrol. Dial. Transplant. 2009, 24, 1574–1579. [Google Scholar] [CrossRef]
- Tabinor, M.; Elphick, E.; Dudson, M.; Kwok, C.S.; Lambie, M.; Davies, S.J. Bioimpedance-defined overhydration predicts survival in end stage kidney failure (ESKF): Systematic review and subgroup meta-analysis. Sci. Rep. 2018, 13, 4441. [Google Scholar] [CrossRef]
- Hur, E.; Usta, M.; Toz, H.; Asci, G.; Wabel, P.; Kahvecioglu, S.; Kayikcioglu, M.; Demirci, M.S.; Ozkahya, M.; Duman, S.; et al. Effect of fluid management guided by bioimpedance spectroscopy on cardiovascular parameters in hemodialysis patients: A randomized controlled trial. Am. J. Kidney Dis. 2013, 61, 957–965. [Google Scholar] [CrossRef]
- Onofriescu, M.; Hogas, S.; Voroneanu, L.; Apetrii, M.; Nistor, I.; Kanbay, M.; Covic, A.C. Bioimpedance-guided fluid management in maintenance hemodialysis: A pilot randomized controlled trial. Am. J. Kidney Dis. 2014, 64, 111–118. [Google Scholar] [CrossRef]
- Van Biesen, W.; Williams, J.D.; Covic, A.C.; Fan, S.; Claes, K.; Lichodziejewska-Niemierko, M.; Verger, C.; Steiger, J.; Schoder, V.; Wabel, P.; et al. Fluid status in peritoneal dialysis patients: The European Body Composition Monitoring (EuroBCM) study cohort. PLoS ONE 2011, 6, e17148. [Google Scholar] [CrossRef]
- Ronco, C.; Verger, C.; Crepaldi, C.; Pham, J.; De Los Ríos, T.; Gauly, A.; Wabel, P.; Van Biesen, W.; IPOD-PD Study Group. Baseline hydration status in incident peritoneal dialysis patients: The initiative of patient outcomes in dialysis (IPOD-PD study). Nephrol. Dial. Transplant. 2015, 30, 849–858. [Google Scholar] [CrossRef]
- Organ, L.W.; Bradham, G.B.; Gore, D.T.; Lozier, S.L. Segmental bioelectrical impedance analysis: Theory and application of a new technique. J. Appl. Physiol. 1994, 77, 98–112. [Google Scholar] [CrossRef]
- Sipahi, S.; Hur, E.; Demirtas, S.; Kocayigit, I.; Bozkurt, D.; Tamer, A.; Gunduz, H.; Duman, S. Body composition monitor measurement technique for the detection of volume status in peritoneal dialysis patients: The effect of abdominal fullness. Int. Urol. Nephrol. 2011, 43, 1195–1199. [Google Scholar] [CrossRef]
- Parmentier, S.P.; Schirutschke, H.; Schmitt, B.; Schewe, J.; Herbrig, K.; Pistrosch, F.; Passauer, J. Influence of peritoneal dialysis solution on measurements of fluid status by bioimpedance spectroscopy. Int. Urol. Nephrol. 2013, 45, 229–232. [Google Scholar] [CrossRef]
- Arroyo, D.; Panizo, N.; Abad, S.; Vega, A.; Rincón, A.; de José, A.P.; López-Gómez, J.M. Intraperitoneal fluid overestimates hydration status assessment by bioimpedance spectroscopy. Perit. Dial. Int. 2015, 35, 85–89. [Google Scholar] [CrossRef]
- Oh, K.H.; Baek, S.H.; Joo, K.W.; Kim, D.K.; Kim, Y.S.; Kim, S.; Oh, Y.K.; Han, B.G.; Chang, J.H.; Chung, W.; et al. Control of fluid balance guided by body composition monitoring in patients on peritoneal dialysis (COMPASS) study. Does routine bioimpedance-guided fluid management provide additional benefit to non-anuric peritoneal dialysis patients? Results from COMPASS clinical trial. Perit. Dial. Int. 2018, 38, 131–138. [Google Scholar]
- Tabinor, M.; Davies, S.J. The use of bioimpedance spectroscopy to guide fluid management in patients receiving dialysis. Curr. Opin. Nephrol. Hypertens. 2018, 27, 406–412. [Google Scholar] [CrossRef]
- Zha, Y.; Qian, Q. Protein nutrition and malnutrition in CKD and ESRD. Nutrients 2017, 9, 208. [Google Scholar] [CrossRef]
- Cupisti, A.; Brunori, G.; Di Iorio, B.R.; D’Alessandro, C.; Pasticci, F.; Cosola, C.; Bellizzi, V.; Bolasco, P.; Capitanini, A.; Fantuzzi, A.L.; et al. Nutritional treatment of advanced CKD: Twenty consensus statements. J. Nephrol. 2018, 31, 457–473. [Google Scholar] [CrossRef]
- Bellizzi, V.; Scalfi, L.; Terracciano, V.; De Nicola, L.; Minutolo, R.; Marra, M.; Guida, B.; Cianciaruso, B.; Conte, G.; Di Iorio, B.R. Early changes in bioelectrical estimates of body composition in chronic kidney disease. J. Am. Soc. Nephrol. 2006, 17, 1481–1487. [Google Scholar] [CrossRef]
- Lukaski, H.C.; Kyle, U.G.; Kondrup, J. Assessment of adult malnutrition and prognosis with bioelectrical impedance analysis: Phase angle and impedance ratio. Curr. Opin. Clin. Nutr. Metab. Care 2017, 20, 330–339. [Google Scholar] [CrossRef]
- Piccoli, A.; Codognotto, M.; Piasentin, P.; Naso, A. Combined evaluation of nutrition and hydration in dialysis patients with bioelectrical impedance vector analysis (BIVA). Clin. Nutr. 2014, 33, 673–677. [Google Scholar] [CrossRef]
- Piccoli, A.; Pastori, G.; Codognotto, M.; Paoli, A. Equivalence of information from single frequency v. bioimpedance spectroscopy in bodybuilders. Br. J. Nutr. 2007, 97, 182–192. [Google Scholar] [CrossRef]
- Teruel-Briones, J.L.; Fernández-Lucas, M.; Ruiz-Roso, G.; Sánchez-Ramírez, H.; Rivera-Gorrin, M.; Gomis-Couto, A.; Rodríguez-Mendiola, N.; Quereda, C. Analysis of concordance between the bioelectrical impedance vector analysis and the bioelectrical impedance spectroscopy in haemodialysis patients. Nefrologia 2012, 32, 389–395. [Google Scholar]
- Gonzales-Correa, C.H.; Caicedo-Eraso, J.C. Bioelectrical impedance analysis (BIA): A proposal for the standardization of the classical method for adults. J. Phys. Conf. Ser. 2012, 407, 012018. [Google Scholar] [CrossRef]
- Genton, L.; Herrmann, F.R.; Spörri, A.; Graf, C.E. Association of mortality and phase angle measured by different bioelectrical impedance analysis (BIA) devices. Clin. Nutr. 2018, 37, 1066–1069. [Google Scholar] [CrossRef]
- Silva, A.M.; Matias, C.N.; Nunes, C.L.; Santos, D.A.; Marini, E.; Lukaski, H.C.; Sardinha, L.B. Lack of agreement of in vivo raw bioimpedance measurements obtained from two single and multi-frequency bioelectrical impedance devices. Eur. J. Clin. Nutr. 2018, 1, 22. [Google Scholar] [CrossRef]
- Di Somma, S.; Lukaski, H.C.; Codognotto, M.; Peacock, W.F.; Fiorini, F.; Aspromonte, N.; Ronco, C.; Santarelli, S.; Lalle, I.; Autunno, A.; et al. Consensus paper on the use of BIVA (Bioeletrical Impendance Vector Analysis) in medicine for the management of body hydration. Emerg. Care J. 2011, 4, 6–14. [Google Scholar] [CrossRef]
- Covic, A.; Ciumanghel, A.I.; Siriopol, D.; Kanbay, M.; Dumea, R.; Gavrilovici, C.; Nistor, I. Value of bioimpedance analysis estimated “dry weight” in maintenance dialysis patients: A systematic review and meta-analysis. Int. Urol. Nephrol. 2017, 49, 2231–2245. [Google Scholar] [CrossRef]
- Scotland, G.; Cruickshank, M.; Jacobsen, E.; Cooper, D.; Fraser, C.; Shimonovich, M.; Marks, A.; Brazzelli, M. Multiple-frequency bioimpedance devices for fluid management in people with chronic kidney disease receiving dialysis: A systematic review and economic evaluation. Health Technol. Assess. 2018, 22, 1–138. [Google Scholar] [CrossRef]
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lukaski, H.C.; Vega Diaz, N.; Talluri, A.; Nescolarde, L. Classification of Hydration in Clinical Conditions: Indirect and Direct Approaches Using Bioimpedance. Nutrients 2019, 11, 809. https://doi.org/10.3390/nu11040809
Lukaski HC, Vega Diaz N, Talluri A, Nescolarde L. Classification of Hydration in Clinical Conditions: Indirect and Direct Approaches Using Bioimpedance. Nutrients. 2019; 11(4):809. https://doi.org/10.3390/nu11040809
Chicago/Turabian StyleLukaski, Henry C., Nicanor Vega Diaz, Antonio Talluri, and Lexa Nescolarde. 2019. "Classification of Hydration in Clinical Conditions: Indirect and Direct Approaches Using Bioimpedance" Nutrients 11, no. 4: 809. https://doi.org/10.3390/nu11040809
APA StyleLukaski, H. C., Vega Diaz, N., Talluri, A., & Nescolarde, L. (2019). Classification of Hydration in Clinical Conditions: Indirect and Direct Approaches Using Bioimpedance. Nutrients, 11(4), 809. https://doi.org/10.3390/nu11040809




