Real-Time Precise Orbit Determination of Low Earth Orbit Satellites Based on GPS and BDS-3 PPP B2b Service
Abstract
1. Introduction
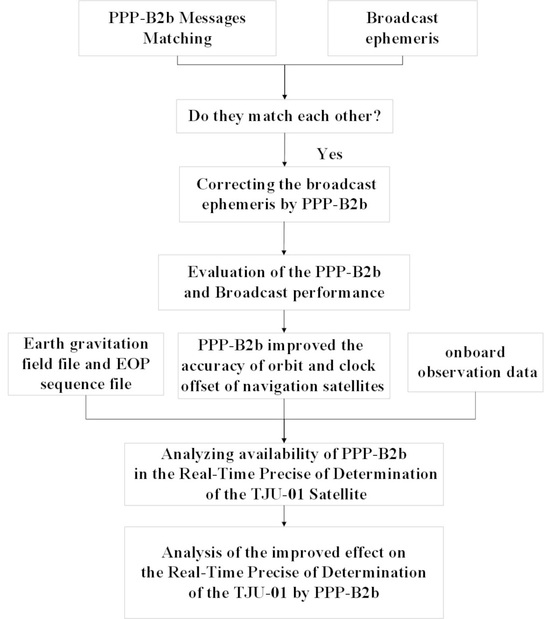
2. Principle of the Broadcast Ephemeris Correction with the PPP-B2b Service
2.1. Matching Algorithms of PPP-B2b Messages
2.2. Correction Algorithm
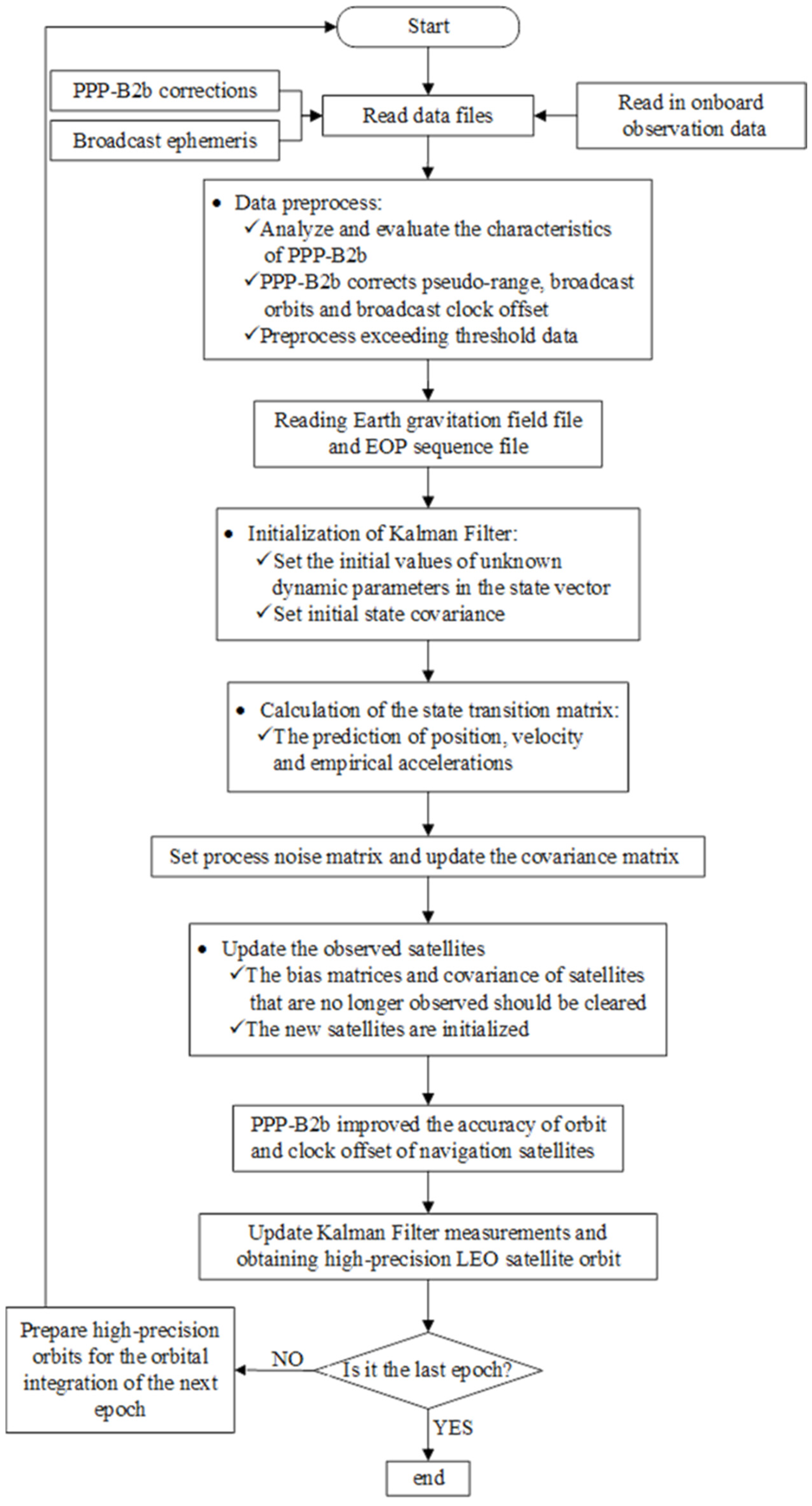
3. Mathematical Models for the RT POD of LEO Satellites
3.1. Preprocessing of Pseudo-Range and Carrier-Phase Measurements after B2b Correction
3.2. Observation Model
3.3. Kalman Filtering Model
3.4. Processing Strategy
4. Analysis and Evaluation of the PPP-B2b and Broadcast Ephemeris Performance
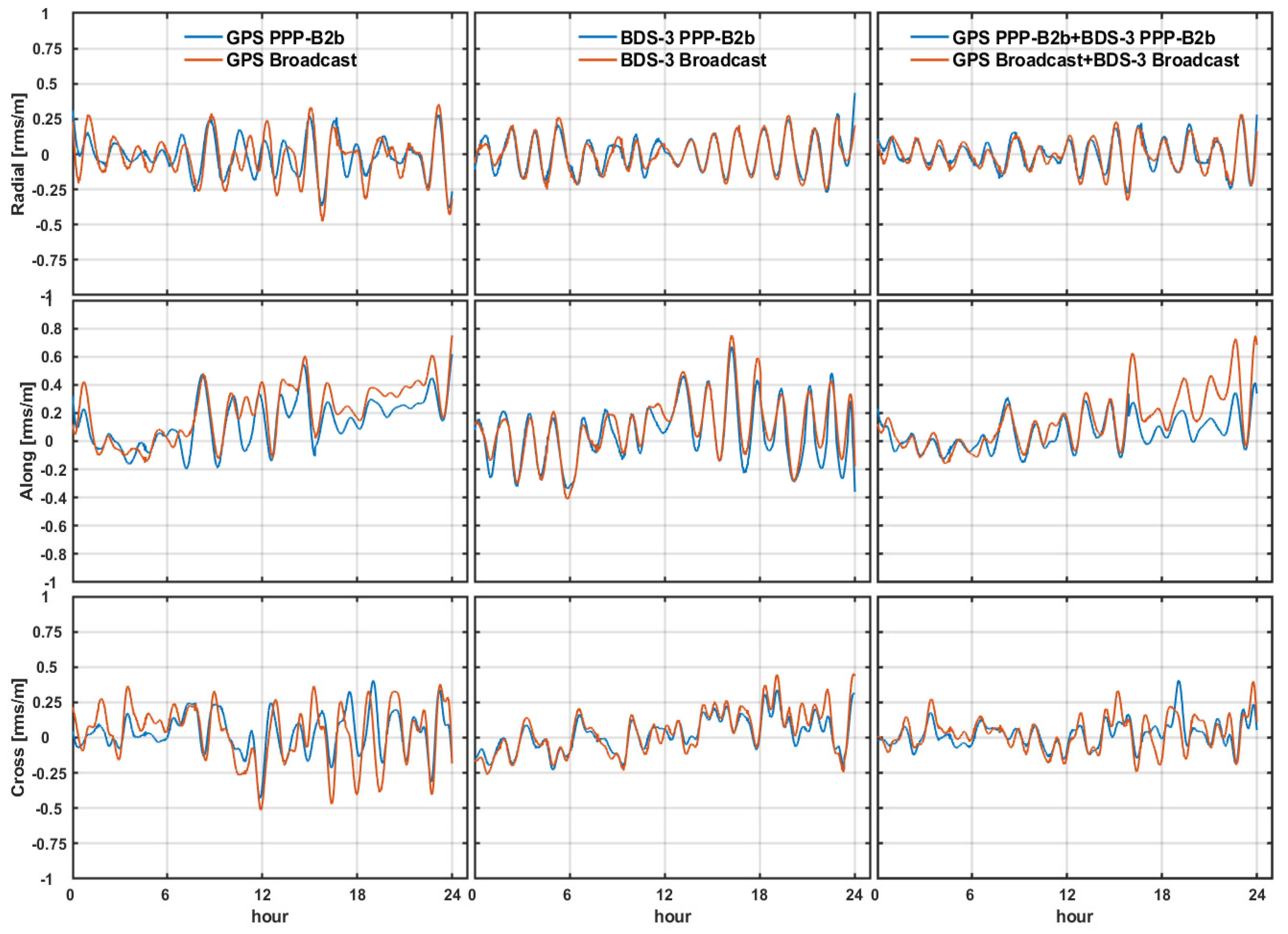
4.1. Analysis of the Characteristics of the PPP-B2b Correction Time Series
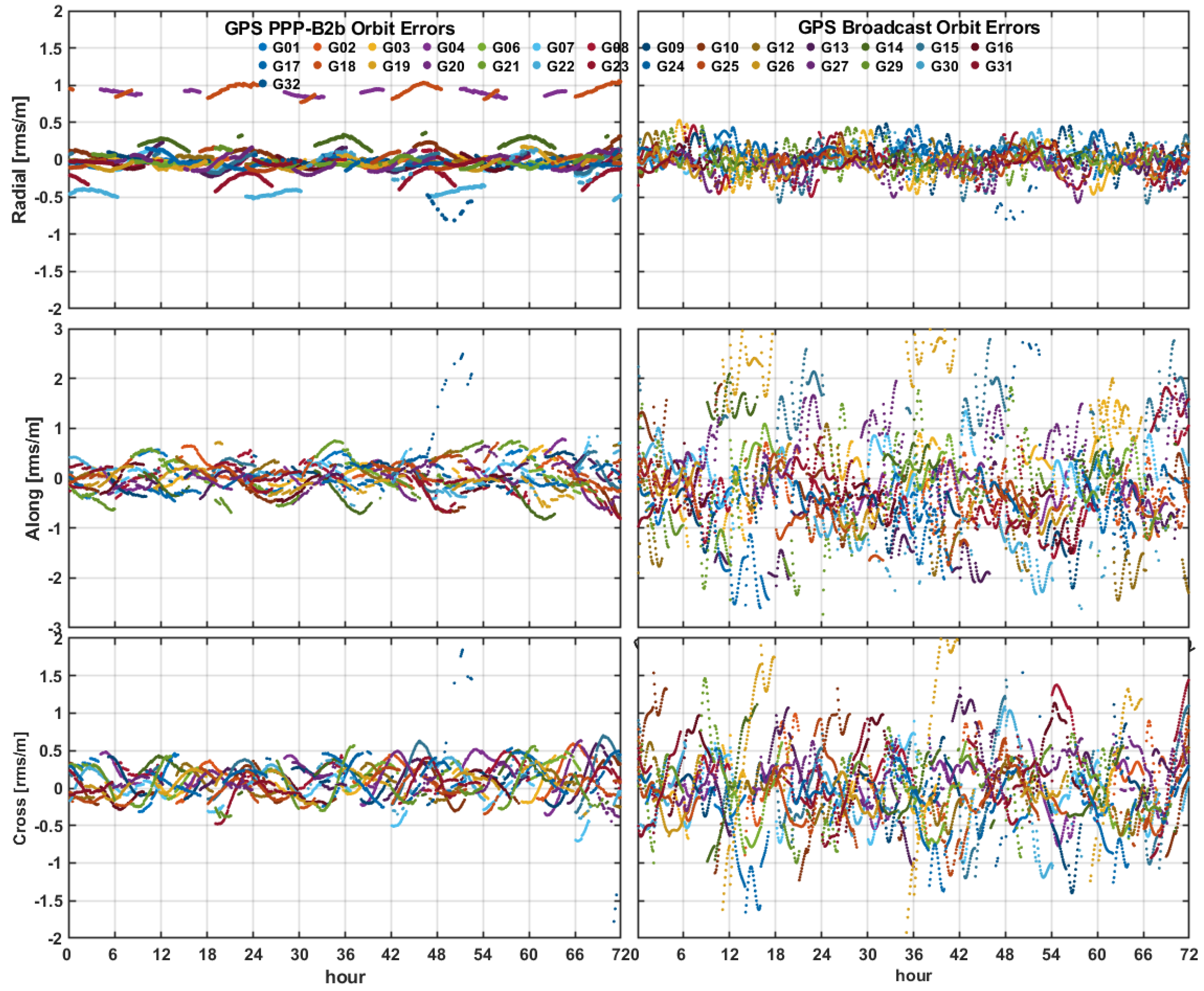
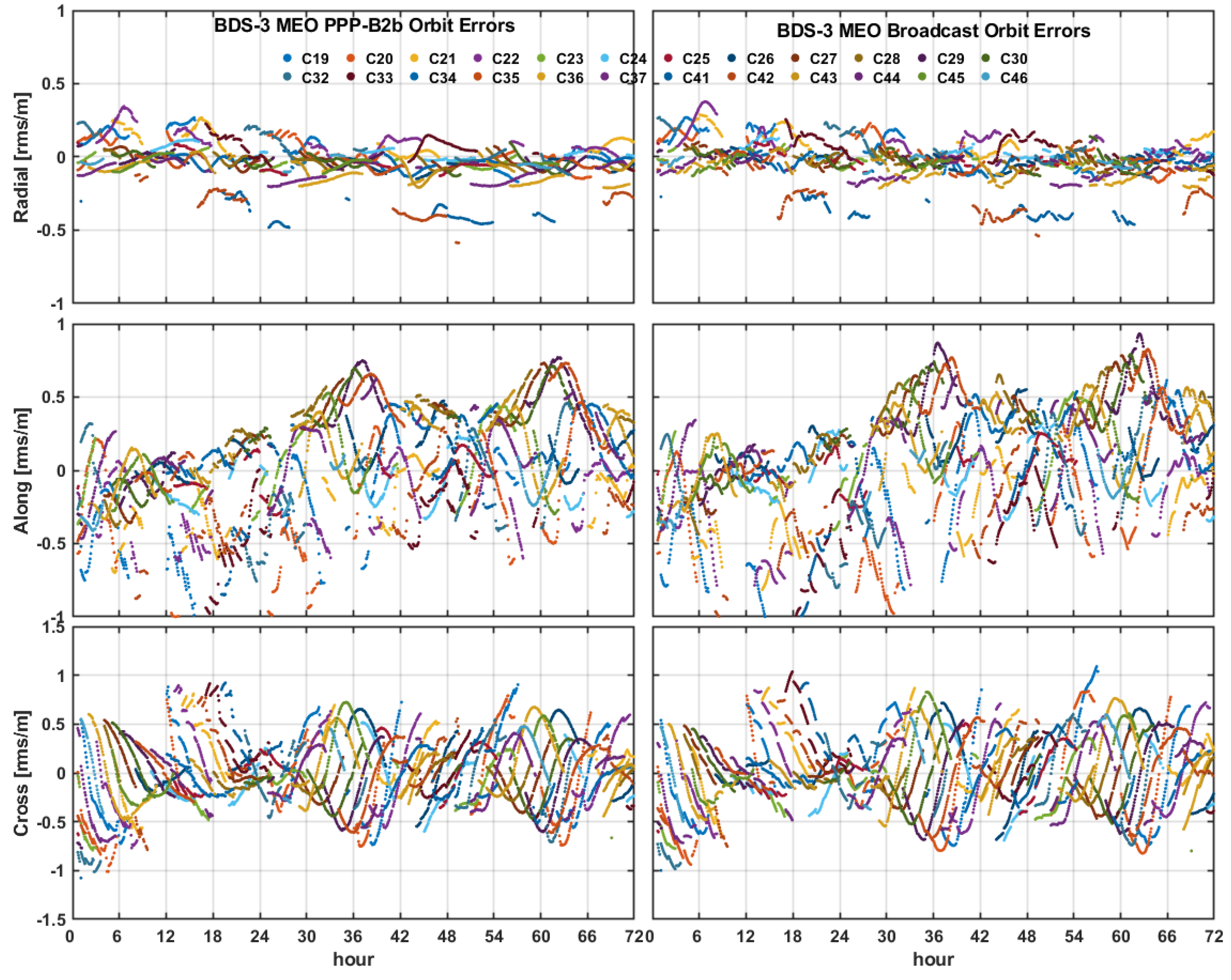
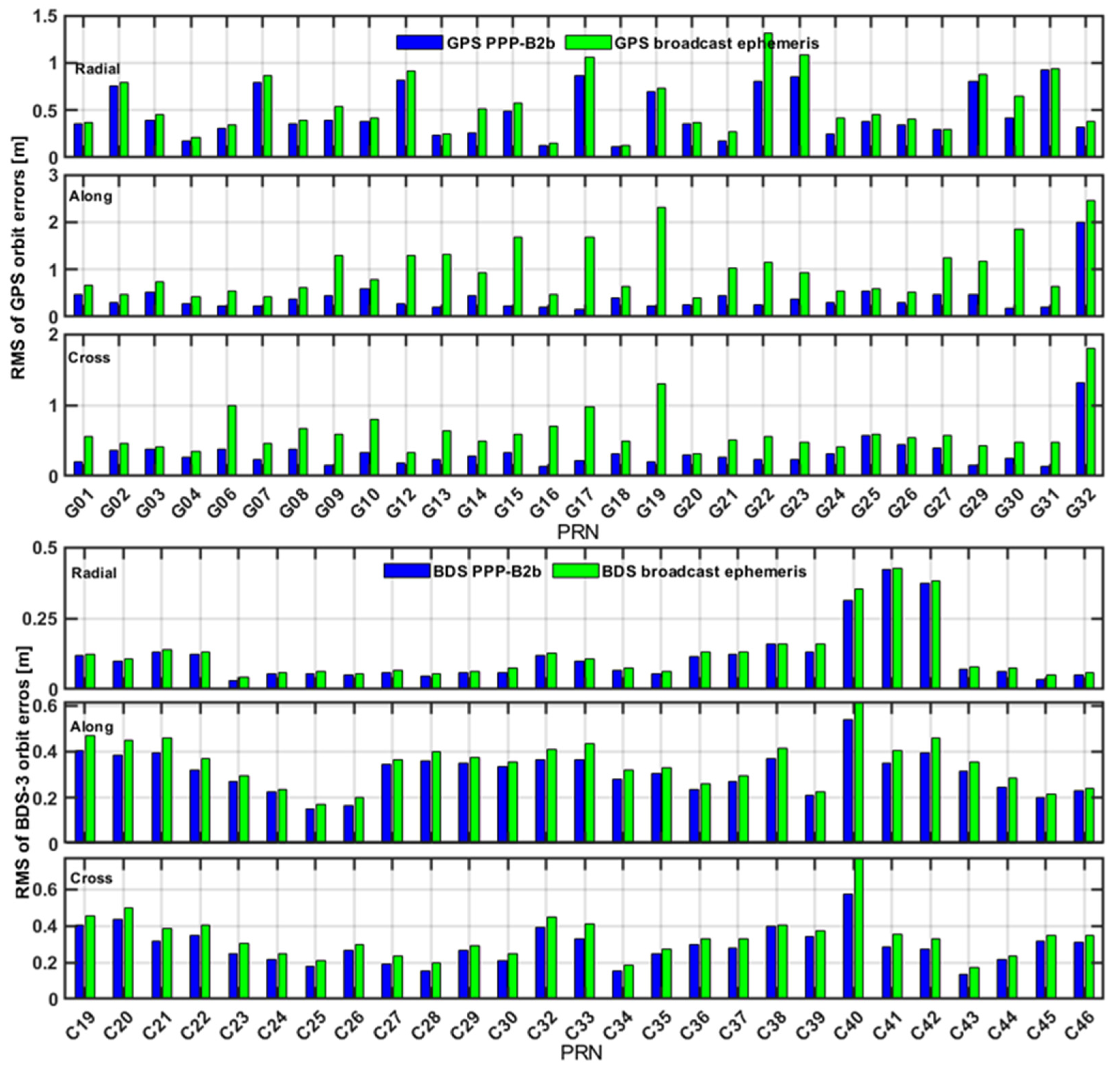
4.2. Evaluation of the PPP-B2b and Broadcast Orbit Accuracy
4.3. Evaluation of the RT Clock Offset Accuracy
4.4. Comparision of the Globally Averaged Signal in Space Range Error of PPP-B2b and Broadcast Empeheris
5. RT POD Processing and Analysis
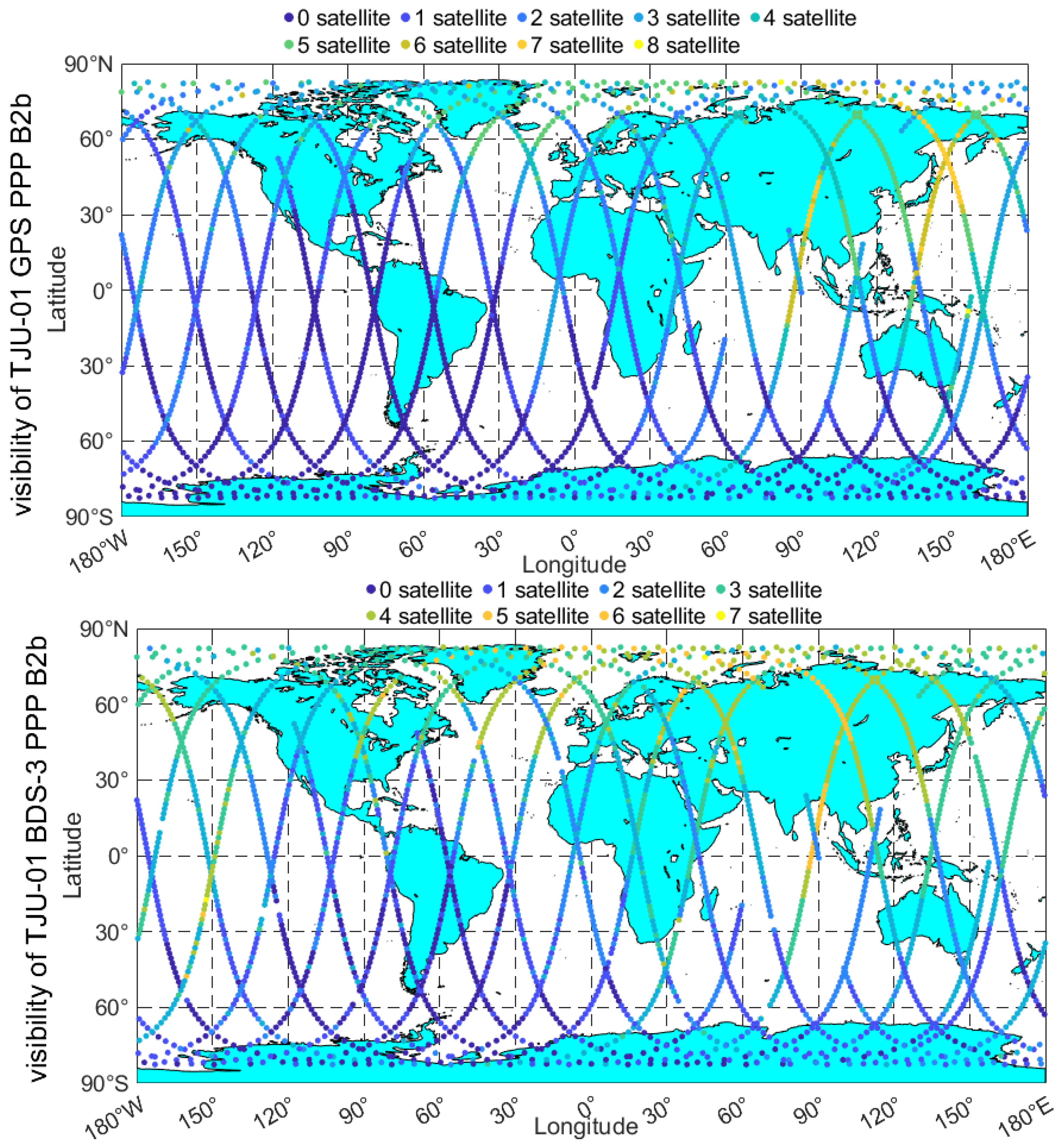
5.1. Availability Analysis of Onboard GNSS Data
5.2. Availability of PPP-B2b in the RT POD of the TJU-01 Satellite
5.3. Results and Analysis of the RT POD of the TJU-01 Satellite
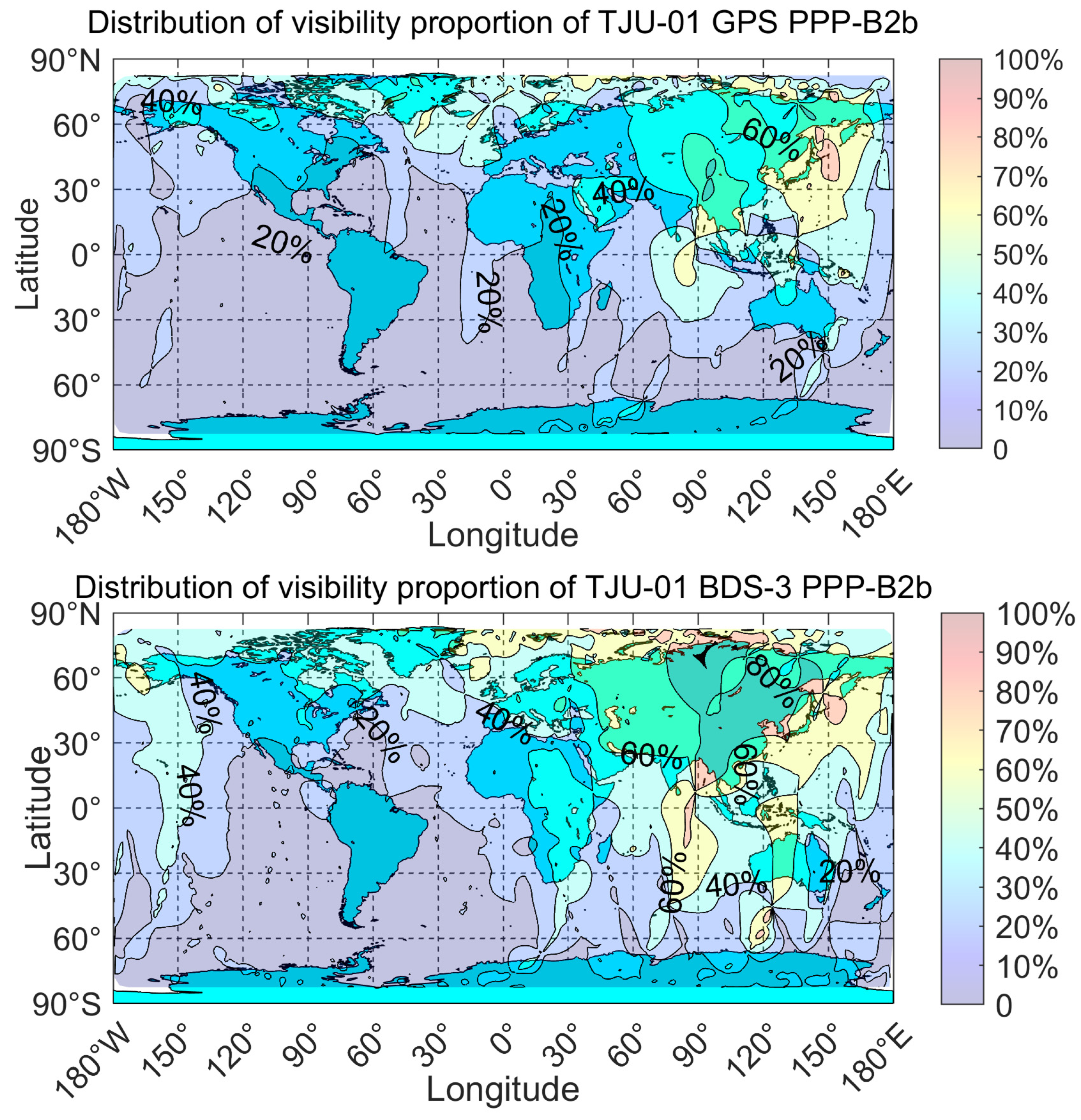
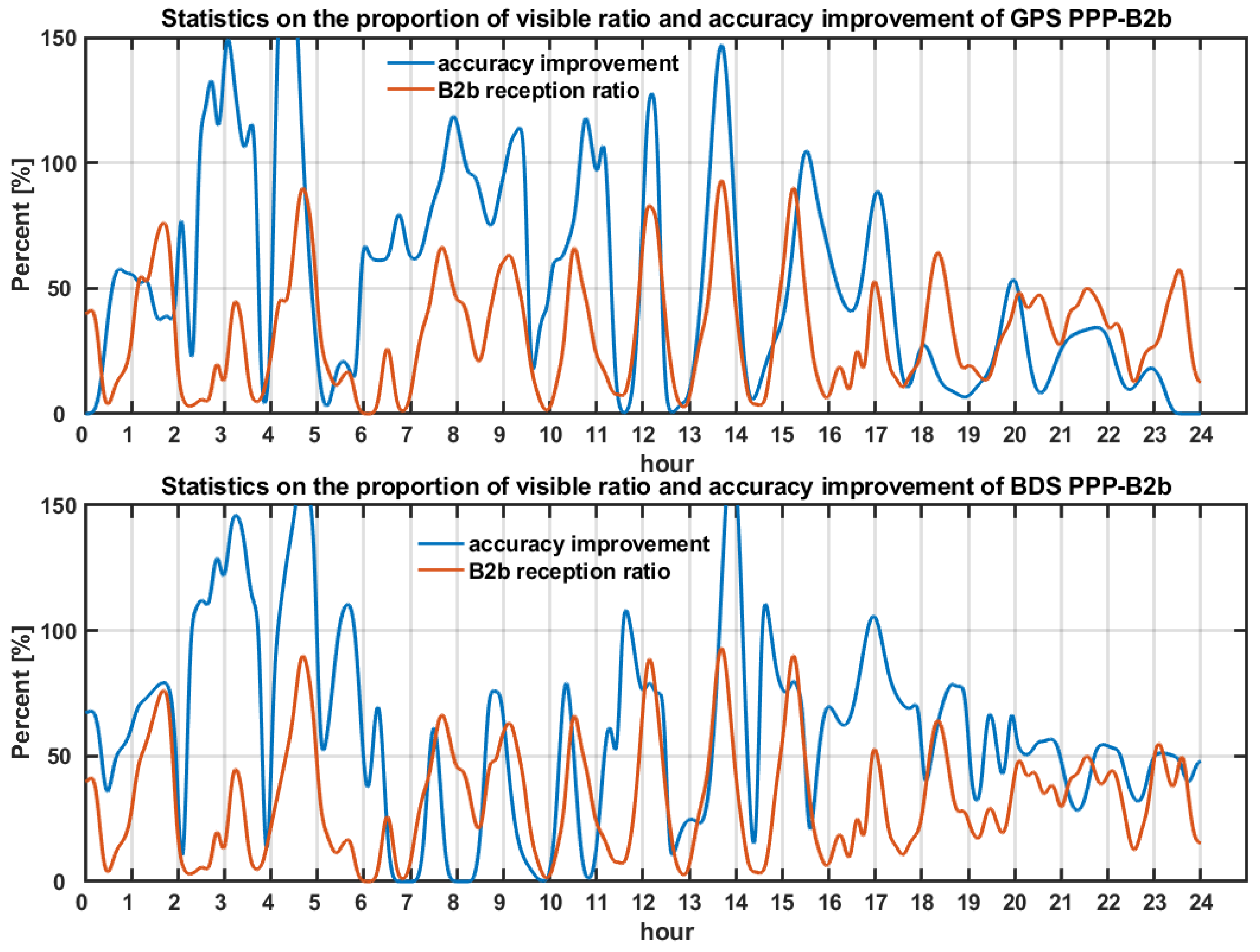
5.4. Correlation Analysis of PPP-B2b Reception Ratio and Accuracy Improvement Ratio
6. Conclusions
- The RMS of the GPS broadcast ephemeris orbit errors in the along direction (0.92 m) is greater than that in the radial direction (0.17 m) and that in the cross direction (0.49 m). In addition, errors in the along direction are corrected most by the GPS PPP-B2b (by approximately 60%) among the three directions. The RMS of the GPS PPP-B2b orbit errors in the radial, along, and cross directions is 0.12, 0.34, and 0.26 m, respectively.
- For BDS-3, the orbit errors of PPP-B2b are a few centimeters smaller than those of the broadcast ephemeris. The orbit errors of the IGSO satellite broadcast ephemeris are much greater than those of the MEO satellite broadcast ephemeris, and the orbit correction of the IGSO satellites is greater than the orbit corrections of the MEO satellites. The RMS of the MEO satellite PPP-B2b orbit errors in the radial, along, and cross directions is 0.10, 0.27, and 0.24 m, which is better than those of the IGSO satellites, 0.15, 0.32, and 0.41 m, respectively.
- PPP-B2b correction improves the broadcast clock offset accuracy remarkably. For BDS-3, the average value of the STDs of the PPP-B2b clock offset is approximately 0.07 m. The accuracy is 70% higher than that of the broadcast ephemeris clock offset. For the GPS, the average value of the STDs of the PPP-B2b clock offset is approximately 0.03 m, i.e., the accuracy is 50% better than that of the broadcast ephemeris clock offset.
- The smoothness and continuity of the navigation satellite orbit and clock offset error series are improved by the PPP-B2b service, which reduces the errors introduced by broadcast ephemeris data updates.
- As the TJU-01 satellite is much higher than ground stations, it has a wider geographical range of PPP-B2b reception. Up to eight GPS satellites can receive the PPP-B2b correction at a certain epoch whereas up to seven BDS-3 satellites can receive the PPP-B2b correction.
- More BDS-3 satellites than GPS satellites can receive PPP-B2b. The BDS-3 and GPS satellite constellations have different configurations, and the integrity of the GPS observations is lower than that of the BDS-3 observations.
- As PPP-B2b mainly reduces errors in the broadcast ephemeris orbit in the along direction, the errors in the RT POD of the TJU-01 satellite using PPP-B2b in the along direction are smaller than those when using the broadcast ephemeris.
- The accuracy of the RT POD of the TJU-01 satellite when using the GPS broadcast ephemeris is lower than that when using the BDS-3 broadcast ephemeris because the inter-satellite links improve the accuracy of the BDS-3 broadcast ephemeris, and more BDS-3 satellites than GPS satellites receive PPP-B2b. Moreover, the integrity of the GPS observations is lower than that of the BDS-3 observations.
- The most accurate scheme is using the GPS and BDS-3 PPP-B2b for the RT POD of the TJU-01 satellite. The accuracy is improved by 5.1%, 43.9%, and 28.7%, respectively, in the radial, along, and cross directions relative to using the GPS and BDS-3 broadcast ephemeris.
- As the proportion of PPP-B2b correction reception increases, the accuracy improvement of PPP-B2b in the RT POD of the TJU-01 satellite increases.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Lee, B.-S.; Yoon, J.-C.; Hwang, Y.; Kim, J. Orbit determination system for the kompsat-2 using gps measurement data. Acta Astronaut. 2005, 57, 747–753. [Google Scholar] [CrossRef]
- Montenbruck, O.; Ramos-Bosch, P. Precision real-time navigation of leo satellites using global positioning system measurements. GPS Solut. 2007, 12, 187–198. [Google Scholar] [CrossRef]
- Yang, Y.; Yue, X.; Dempster, A.G. Gps-based onboard real-time orbit determination for leo satellites using consider kalman filter. IEEE Trans. Aerosp. Electron. Syst. 2016, 52, 769–777. [Google Scholar] [CrossRef]
- Reichert, A.; Meehan, T.; Munson, T. Toward decimeter-level real-time orbit determination: A demonstration using the sac-c and champ spacecraft. In Proceedings of the 15th International Technical Meeting of the Satellite Division of The Institute of Navigation (ION GPS 2002), Los Angeles, CA, USA, 4–7 February 2002; pp. 1996–2003. [Google Scholar]
- Montenbruck, O.; Hauschild, A.; Andres, Y.; von Engeln, A.; Marquardt, C. (near-) real-time orbit determination for gnss radio occultation processing. GPS Solut. 2013, 17, 199–209. [Google Scholar] [CrossRef]
- Xiong, C.; Lu, C.; Zhu, J.; Ding, H. Orbit determination using real tracking data from fy3c-gnos. Adv. Space Res. 2017, 60, 543–556. [Google Scholar] [CrossRef]
- Tang, J.; Lyu, D.; Zeng, F.; Ge, Y.; Zhang, R. Comprehensive analysis of ppp-b2b service and its impact on bds-3/gps real-time ppp time transfer. Remote Sens. 2022, 14, 5366. [Google Scholar] [CrossRef]
- Liu, Y.; Yang, C.; Zhang, M. Comprehensive analyses of ppp-b2b performance in china and surrounding areas. Remote Sens. 2022, 14, 643. [Google Scholar] [CrossRef]
- Ren, Z.; Gong, H.; Peng, J.; Tang, C.; Huang, X.; Sun, G. Performance assessment of real-time precise point positioning using bds ppp-b2b service signal. Adv. Space Res. 2021, 68, 3242–3254. [Google Scholar] [CrossRef]
- Tang, C.; Hu, X.; Chen, J.; Liu, L.; Zhou, S.; Guo, R.; Li, X.; He, F.; Liu, J.; Yang, J. Orbit determination, clock estimation and performance evaluation of bds-3 ppp-b2b service. J. Geod. 2022, 96, 60. [Google Scholar] [CrossRef]
- Tao, J.; Liu, J.; Hu, Z.; Zhao, Q.; Chen, G.; Ju, B. Initial assessment of the bds-3 ppp-b2b rts compared with the cnes rts. GPS Solut. 2021, 25, 131. [Google Scholar] [CrossRef]
- Xu, Y.; Yang, Y.; Li, J. Performance evaluation of bds-3 ppp-b2b precise point positioning service. GPS Solut. 2021, 25, 142. [Google Scholar] [CrossRef]
- Yang, Y.; Ding, Q.; Gao, W.; Li, J.; Xu, Y.; Sun, B. Principle and performance of bdsbas and ppp-b2b of bds-3. Satell. Navig. 2022, 3, 5. [Google Scholar] [CrossRef]
- Yang, Y.; Liu, L.; Li, J.; Yang, Y.; Zhang, T.; Mao, Y.; Sun, B.; Ren, X. Featured services and performance of bds-3. Sci. Bull. 2021, 66, 2135–2143. [Google Scholar] [CrossRef]
- Chen, J.; Zhang, Y.; Yu, C.; Wang, A.; Song, Z.; Zhou, J. Models and performance of sbas and ppp of bds. Satell. Navig. 2022, 3, 4. [Google Scholar] [CrossRef]
- Wermuth, M.; Hauschild, A.; Montenbruck, O.; Kahle, R. Terrasar-x precise orbit determination with real-time gps ephemerides. Adv. Space Res. 2012, 50, 549–559. [Google Scholar] [CrossRef]
- Xiao, G.; Liu, G.; Ou, J.; Zhou, C.; He, Z.; Chen, R.; Guo, A.; Yang, Z. Real-time carrier observation quality control algorithm for precision orbit determination of leo satellites. GPS Solut. 2022, 26, 102. [Google Scholar] [CrossRef]
- Li, D.; Zhou, X.; Li, K. Centimeter-level orbit determination of grace-c using igs-rts data. Remote Sens. 2023, 15, 1832. [Google Scholar] [CrossRef]
- Yang, H.; He, X.; Ferreira, V.; Ji, S.; Xu, Y.; Song, S. Assessment of precipitable water vapor retrieved from precise point positioning with ppp-b2b service. Earth Sci. Inform. 2023, 16, 315–328. [Google Scholar] [CrossRef]
- Li, M.; Xu, T.; Shi, Y.; Wei, K.; Fei, X.; Wang, D. Adaptive kalman filter for real-time precise orbit determination of low earth orbit satellites based on pseudorange and epoch-differenced carrier-phase measurements. Remote Sens. 2022, 14, 2273. [Google Scholar] [CrossRef]
- Li, B.; Ge, H.; Ge, M.; Nie, L.; Shen, Y.; Schuh, H. Leo enhanced global navigation satellite system (legnss) for real-time precise positioning services. Adv. Space Res. 2019, 63, 73–93. [Google Scholar] [CrossRef]
- Förste, C.; Bruinsma, S.; Flechtner, F.; Abrykosov, O.; Dahle, C.; Marty, J.; Lemoine, J.; Biancale, R.; Barthelmes, F.; Neumayer, K. Eigen-6c3-the latest combined global gravity field model including goce data up to degree and order 1949 of gfz potsdam and grgs toulouse. In Proceedings of the AGU Fall Meeting Abstracts, San Francisco, CA, USA, 5–9 December 2011; p. G51A-0860. [Google Scholar]
- Luzum, B.; Petit, G. The iers conventions (2010): Reference systems and new models. In Proceedings of the IAU General Assembly, Beijing, China, 20–31 August 2012. [Google Scholar]
- Spiridonov, E.; Vinogradova, O.Y. Oceanic tide model fes2014b: Comparison with gravity measurements. Izv. Atmos. Ocean. Phys. 2020, 56, 1432–1446. [Google Scholar] [CrossRef]
- Altamimi, Z.; Rebischung, P.; Collilieux, X.; Métivier, L.; Chanard, K. Itrf2020: An augmented reference frame refining the modeling of nonlinear station motions. J. Geod. 2023, 97, 47. [Google Scholar] [CrossRef]
- Yang, Y.; Li, J.; Wang, A.; Xu, J.; He, H.; Guo, H.; Shen, J.; Dai, X. Preliminary assessment of the navigation and positioning performance of beidou regional navigation satellite system. Sci. China Earth Sci 2014, 57, 144–152. [Google Scholar] [CrossRef]
- Montenbruck, O.; Steigenberger, P.; Hauschild, A. Multi-gnss signal-in-space range error assessment–methodology and results. Adv. Space Res. 2018, 61, 3020–3038. [Google Scholar] [CrossRef]
- Xu, X.; Nie, Z.; Wang, Z.; Zhang, Y.; Dong, L. An improved bds-3 ppp-b2b positioning approach by estimating signal in space range errors. GPS Solut. 2023, 27, 110. [Google Scholar] [CrossRef]







| The Content of Correction | Message Type | Nominal Validity | Update Interval |
|---|---|---|---|
| Satellite mask | 1 | 86,400 s | 48 s |
| Orbit correction | 2, 6, 7 | 96 s | 48 s |
| Clock correction | 3 | 86,400 s | 12 s |
| Differential code bias | 4, 6, 7 | 12 s | 6 s |
| User ranging accuracy index | 2, 5, 6, 7 | 96 s | 48 s |
| Type of Force | Model Adopted |
|---|---|
| Earth gravity model | EIGEN-6C (70 × 70) [22] |
| N-body disturbance | JPL DE405 |
| Solid tide and pole tide | IERS 2010 [23] |
| Ocean tide | FES2014b [24] |
| Relative effect | IERS 2010 [23] |
| Solar radiation pressure | Macro Model [22] |
| Atmosphere drag | Harris–Priester density model, fixed area, estimating the drag parameter every 240 min [20] |
| Earth rotation parameter | Predicting EOP from Bulletin A in IERS2010 [23] |
| Empirical forces | Six piecewise periodical terms in the three (along, cross, and radial) directions, Cr and Sr represent radial periodical parameters, Ct and St represent that in the along direction, and Cn, Sn for the cross direction, estimated every 90 min |
| Items | Model Adopted |
|---|---|
| Observation model | Carrier-phase and pseudo-range undifferenced dual-frequency ionospheric-free combination |
| Cut-off elevation | 1° |
| Processing arc | 9-day arc |
| Sampling interval | 1 s |
| Terrestrial frame | ITRF2020 [25] |
| Observation weight | . represents the variance in code or carrier-phase measurements |
| Receiver clock offset | White noise estimation |
| Receiver ISB | Random walk process |
| Filtering method | Extended Kalman filter |
| Ambiguity parameter | Float solution |
| Parameter | The Value of Initial Variance | The Value of Steady-State Variance | Correlation Time |
|---|---|---|---|
| LEO satellite position [m] | 1.0 | - | - |
| LEO satellite velocity [m/s] | 1.0 | - | - |
| GPS receiver clock offset [m] | 500.0 | 50.0 | 1 s |
| ISB (G-C) [m] | 1.2 | 1 | 1 s |
| Empirical force acceleration in radial direction [nm/s2] | 100.0 | 200.0 | 1 s |
| Empirical force acceleration in along direction [nm/s2] | 400.0 | 800.0 | 1 s |
| Empirical force acceleration in cross direction [nm/s2] | 200.0 | 400.0 | 1 s |
| Ambiguity parameter | 0 | 0 | 1 s |
| Ephemeris Type | MEO Orbit (RMS/m) | IGSO Orbit (RMS/m) | ||||
|---|---|---|---|---|---|---|
| Radial | Along | Cross | Radial | Along | Cross | |
| BDS broadcast ephemeris | 0.11 | 0.31 | 0.28 | 0.17 | 0.34 | 0.47 |
| BDS PPP-B2b | 0.10 | 0.27 | 0.24 | 0.15 | 0.32 | 0.41 |
| GPS broadcast ephemeris | 0.17 | 0.92 | 0.49 | - | - | - |
| GPS PPP-B2b | 0.12 | 0.34 | 0.26 | - | - | - |
| Type | |||
|---|---|---|---|
| Ephemeris | |||
| GPS | 0.98 | 1/49 | |
| BDS-3 MEO | 0.98 | 1/54 | |
| BDS-3 IGSO | 0.98 | 1/126 | |
| Type | SISRE (ORB)/m | SISRE/m | |
|---|---|---|---|
| Ephemeris | |||
| GPS broadcast Ephemeris | 1.29 | 0.54 | |
| GPS PPP-B2b | 0.81 | 0.22 | |
| BDS-3 broadcast ephemeris | 1.77 | 0.14 | |
| BDS-3 PPP-B2b | 1.24 | 0.12 | |
| Number of Satellites | 0 | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 |
|---|---|---|---|---|---|---|---|---|---|
| Available epochs | 800 | 471 | 475 | 450 | 223 | 177 | 79 | 33 | 3 |
| Total number of GPS satellites | 6400 | 3768 | 3800 | 3600 | 1784 | 1416 | 632 | 264 | 24 |
| Epoch proportion (%) | 29.5 | 17.4 | 17.5 | 16.5 | 8.2 | 6.5 | 2.9 | 1.2 | 0.1 |
| Quantity proportion (%) | 0 | 12.5 | 25 | 37.5 | 50 | 67.3 | 75 | 87.3 | 100 |
| Number of Satellites | 0 | 1 | 2 | 3 | 4 | 5 | 6 | 7 |
|---|---|---|---|---|---|---|---|---|
| Available epochs | 338 | 543 | 462 | 520 | 436 | 291 | 68 | 2 |
| Total number of BDS3 satellites | 2628 | 4071 | 3493 | 3981 | 3334 | 2209 | 500 | 21 |
| Epoch proportion (%) | 12.7 | 20.3 | 17.3 | 19.5 | 16.3 | 10.9 | 2.5 | 0.1 |
| Quantity proportion (%) | 0 | 13.3 | 26.5 | 39.2 | 52.3 | 65.9 | 81.6 | 66.7 |
| Direction | Radial (RMS/m) | Along (RMS/m) | Cross (RMS/m) | ||||
|---|---|---|---|---|---|---|---|
| Ephemeris | Not Corrected | B2b Corrected | Not Corrected | B2b Corrected | Not Corrected | B2b Corrected | |
| GPS broadcast ephemeris | 0.15 | 0.12 | 0.28 | 0.19 | 0.19 | 0.14 | |
| BDS-3 broadcast ephemeris | 0.12 | 0.11 | 0.23 | 0.20 | 0.16 | 0.13 | |
| GPS + BDS-3 broadcast ephemeris | 0.11 | 0.10 | 0.21 | 0.13 | 0.12 | 0.09 | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shi, Y.; Xu, T.; Li, M.; Wei, K.; Wang, S.; Wang, D. Real-Time Precise Orbit Determination of Low Earth Orbit Satellites Based on GPS and BDS-3 PPP B2b Service. Remote Sens. 2024, 16, 833. https://doi.org/10.3390/rs16050833
Shi Y, Xu T, Li M, Wei K, Wang S, Wang D. Real-Time Precise Orbit Determination of Low Earth Orbit Satellites Based on GPS and BDS-3 PPP B2b Service. Remote Sensing. 2024; 16(5):833. https://doi.org/10.3390/rs16050833
Chicago/Turabian StyleShi, Yali, Tianhe Xu, Min Li, Kai Wei, Shuai Wang, and Dixing Wang. 2024. "Real-Time Precise Orbit Determination of Low Earth Orbit Satellites Based on GPS and BDS-3 PPP B2b Service" Remote Sensing 16, no. 5: 833. https://doi.org/10.3390/rs16050833
APA StyleShi, Y., Xu, T., Li, M., Wei, K., Wang, S., & Wang, D. (2024). Real-Time Precise Orbit Determination of Low Earth Orbit Satellites Based on GPS and BDS-3 PPP B2b Service. Remote Sensing, 16(5), 833. https://doi.org/10.3390/rs16050833