Advancing CubeSats Capabilities: Ground-Based Calibration of Uvsq-Sat NG Satellite’s NIR Spectrometer and Determination of the Extraterrestrial Solar Spectrum
Abstract
1. Introduction
2. Scientific Objectives of the UNIS NIR Spectrometer Ground-Based Measurements
3. Calibration of the UNIS NIR Spectrometer
- Pixel characterization: Each pixel of the InGaAs linear image sensor in the UNIS NIR spectrometer has unique characteristics such as dark current, dark offset, and gain. This characterization is essential to accurately determine the incident irradiance from the digital signal output, as pixels may respond non-linearly to changes in irradiance.
- Photosensitivity and quantum efficiency: This characterization is important for accurately converting the digital signals from the UNIS linear sensor into irradiance for all wavelengths.
- Wavelength calibration: It represents a procedure that assigns a specific wavelength to each pixel of the UNIS linear sensor.
- Linearity: A calibration to verify the linearity of the signal with respect to the source intensity.
- Spectrometer bandwidth: Accurate measurement of the bandwidth, determined by the slit width and the grating’s dispersion, is vital. This ensures the spectrometer’s capability to resolve closely spaced spectral lines, therefore delivering the required spectral resolution data.
- Absolute response: It represents an essential characterization for ensuring the spectrometer’s accuracy, reliability, and consistency of future UNIS SSI observations.
- Inverse Square Law Verification: Confirm that the intensity of light received by the spectrometer decreases inversely with the square of the distance from the light source.
- Bidirectional Reflectance Distribution Function (BRDF) and angular responses: Measurements to predict how light will be reflected or transmitted at varying angles.
- Straylight: Characterization of the stray light to ensure that the spectrometer maintains its sensitivity, dynamic range, and spectral resolution.
- Temperature response: This characterization is important to ensure accurate, stable, and reliable SSI observations of the UNIS NIR spectrometer under different thermal conditions.
3.1. Wavelength Calibration
3.2. Absolute Response
3.3. Bandwidth and Slit Functions
3.4. Temperature
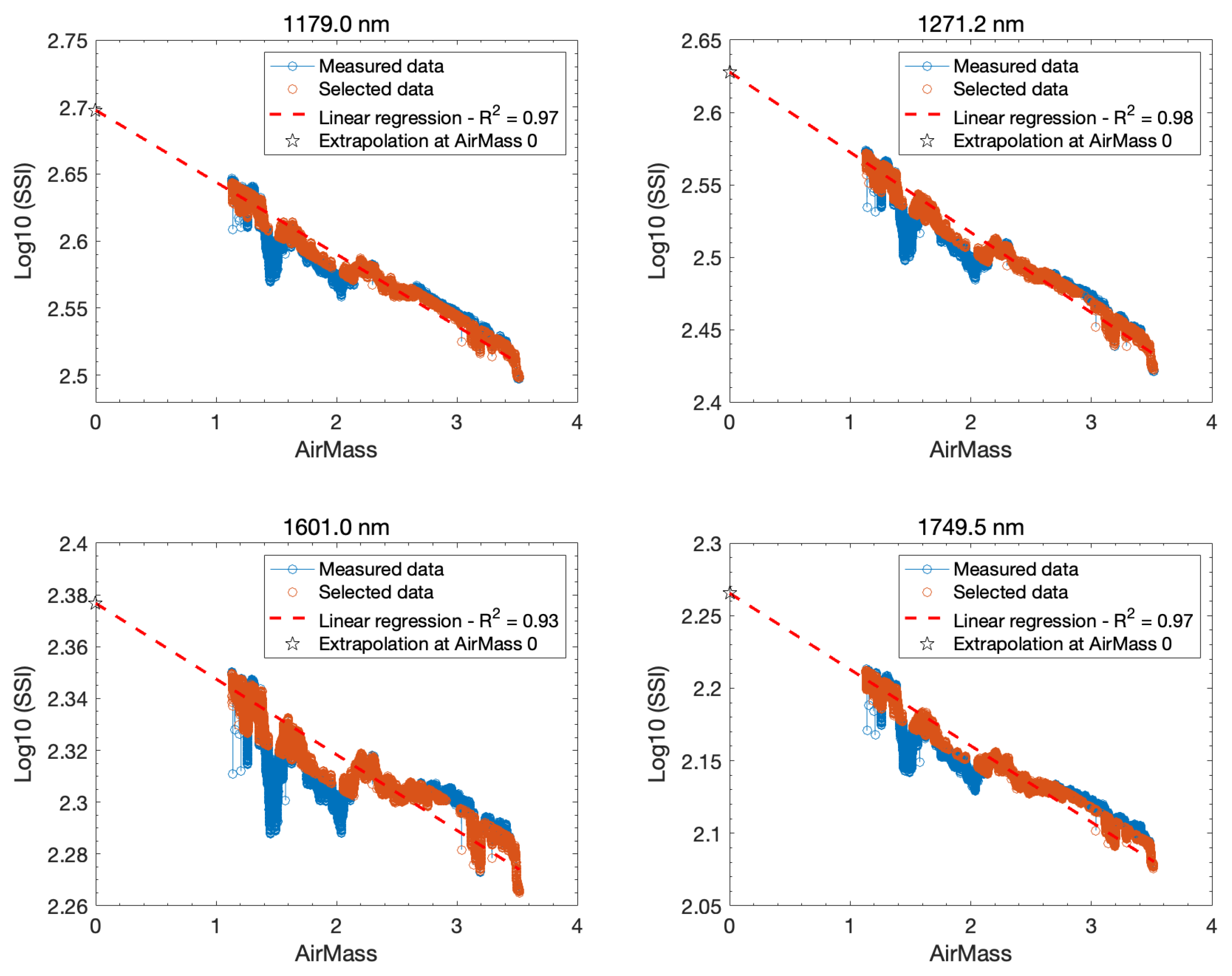
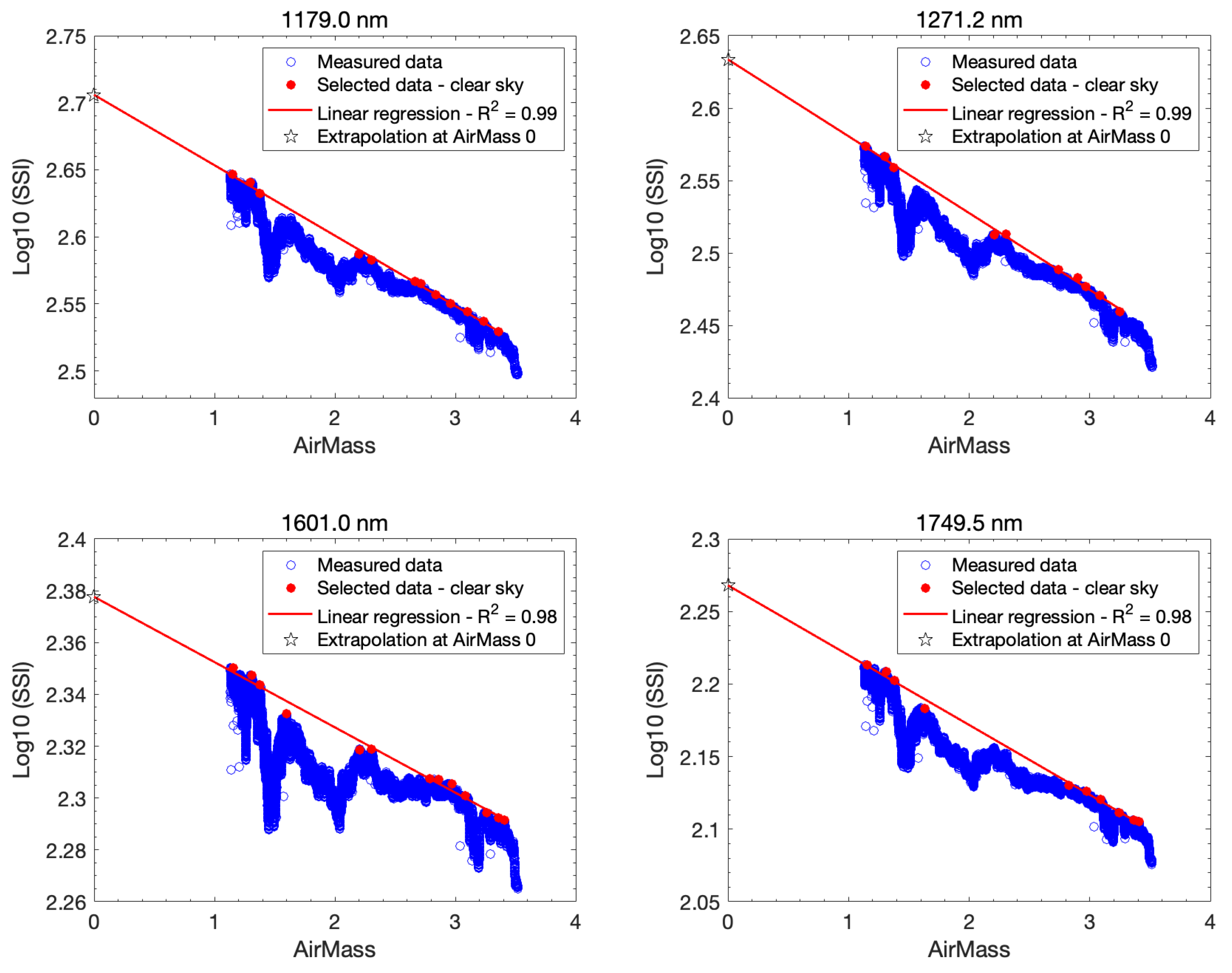
4. Determining Extraterrestrial Solar Spectrum Using the Langley-Plot Technique
4.1. Methodology
4.2. Consideration of Atmospheric Effects
4.2.1. Rayleigh Scattering
4.2.2. Ozone Absorption
4.2.3. Mie Scattering and Aerosols
4.2.4. Other Gases
4.3. Comprehensive Calculation of Optical Depth
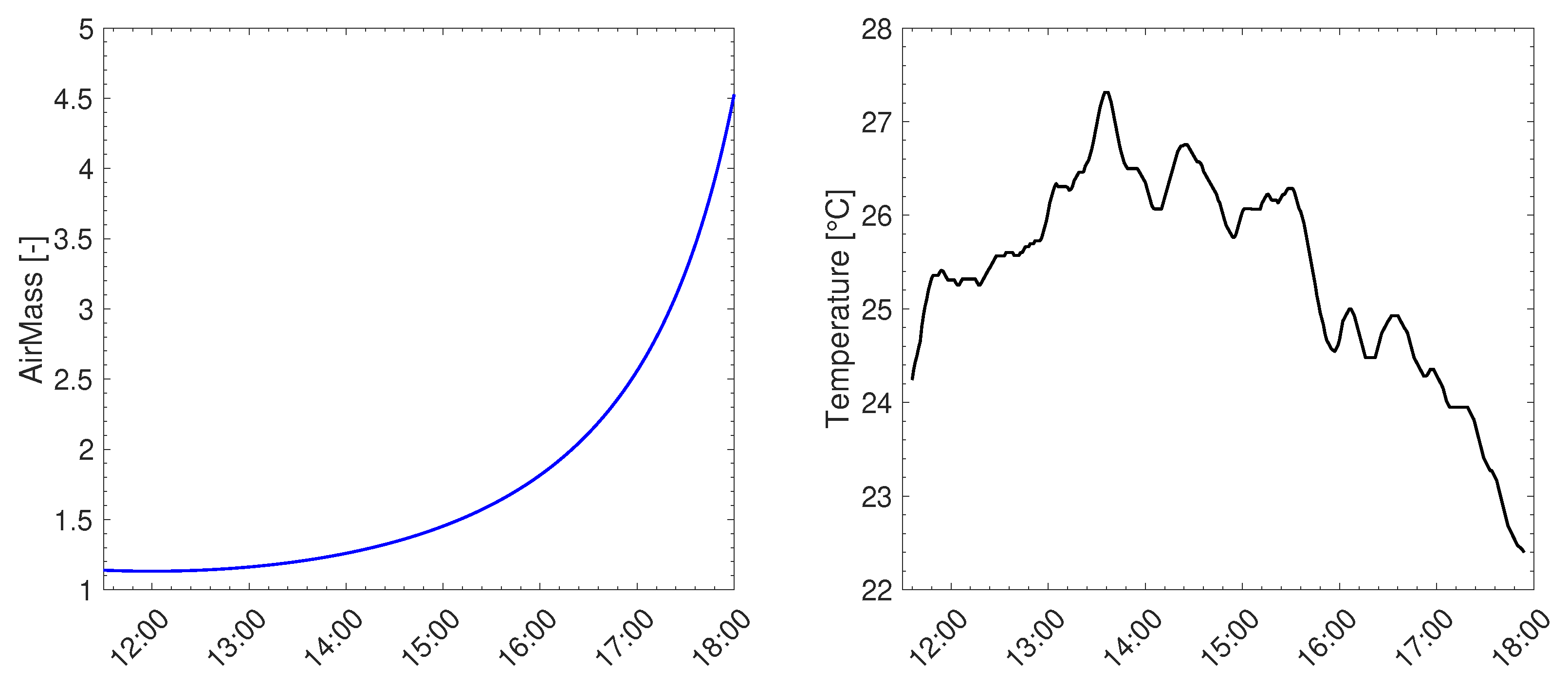
4.4. Conditions for Accurate Measurements Using the Langley Technique
5. Experiment Results and Discussion
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A. Optical Design of the Uvsq-Sat NG Spectrometer
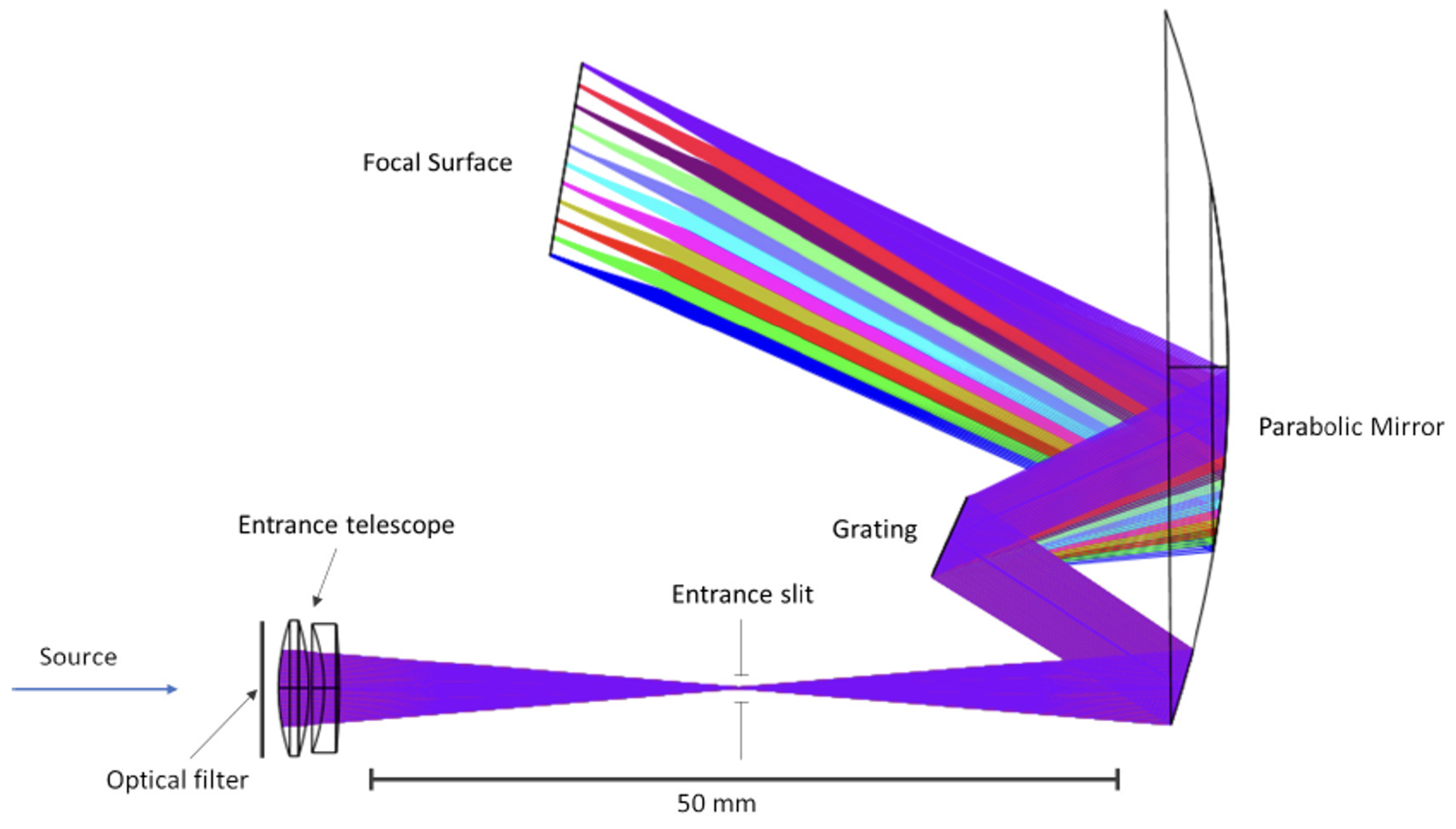
Appendix B. Observations Made with the Uvsq-Sat NG Spectrometer

References
- Meftah, M.; Clavier, C.; Sarkissian, A.; Hauchecorne, A.; Bekki, S.; Lefèvre, F.; Galopeau, P.; Dahoo, P.R.; Pazmino, A.; Vieau, A.J.; et al. Uvsq-Sat NG, a New CubeSat Pathfinder for Monitoring Earth Outgoing Energy and Greenhouse Gases. Remote Sens. 2023, 15, 4876. [Google Scholar] [CrossRef]
- Meftah, M.; Damé, L.; Bolsée, D.; Hauchecorne, A.; Pereira, N.; Sluse, D.; Cessateur, G.; Irbah, A.; Bureau, J.; Weber, M.; et al. SOLAR-ISS: A new reference spectrum based on SOLAR/SOLSPEC observations. Astron. Astrophys. 2018, 611, A1. [Google Scholar] [CrossRef]
- Coddington, O.M.; Richard, E.C.; Harber, D.; Pilewskie, P.; Woods, T.N.; Chance, K.; Liu, X.; Sun, K. The TSIS-1 Hybrid Solar Reference Spectrum. Geophys. Res. Lett. 2021, 48, e91709. [Google Scholar] [CrossRef] [PubMed]
- Meftah, M.; Sarkissian, A.; Keckhut, P.; Hauchecorne, A. The SOLAR-HRS New High-Resolution Solar Spectra for Disk-Integrated, Disk-Center, and Intermediate Cases. Remote Sens. 2023, 15, 3560. [Google Scholar] [CrossRef]
- Pasternak, F.; Bernard, P.; Georges, L.; Pascal, V. The microcarb instrument. In International Society for Optics and Photonics, Proceedings of the International Conference on Space Optics—ICSO 2016, Biarritz, France, 18–21 October 2016; Cugny, B., Karafolas, N., Sodnik, Z., Eds.; SPIE: Paris, France, 2017; Volume 10562, p. 105621P. [Google Scholar] [CrossRef][Green Version]
- Bojinski, S.; Verstraete, M.; Peterson, T.C.; Richter, C.; Simmons, A.; Zemp, M. The Concept of Essential Climate Variables in Support of Climate Research, Applications, and Policy. Bull. Am. Meteorol. Soc. 2014, 95, 1431–1443. [Google Scholar] [CrossRef]
- Stephens, G.L.; Li, J.; Wild, M.; Clayson, C.A.; Loeb, N.; Kato, S.; L’Ecuyer, T.; Stackhouse, P.W.; Lebsock, M.; Andrews, T. An update on Earth’s energy balance in light of the latest global observations. Nat. Geosci. 2012, 5, 691–696. [Google Scholar] [CrossRef]
- Arvesen, J.C.; Griffin, R.N.; Pearson, B.D. Determination of extraterrestrial solar spectral irradiance from a research aircraft. Appl. Opt. 1969, 8, 2215–2232. [Google Scholar] [CrossRef] [PubMed]
- Ångström, A. Apparent solar constant variations and their relation to the variability of atmospheric transmission. Tellus 1970, 22, 205–218. [Google Scholar] [CrossRef]
- Shaw, G.E. Sun Photometry. Bull. Am. Meteorol. Soc. 1983, 64, 4–10. [Google Scholar] [CrossRef]
- Gröbner, J.; Kerr, J.B. Ground-based determination of the spectral ultraviolet extraterrestrial solar irradiance: Providing a link between space-based and ground-based solar UV measurements. J. Geophys. Res. 2001, 106, 7211–7218. [Google Scholar] [CrossRef]
- Harrison, L.; Kiedron, P.; Berndt, J.; Schlemmer, J. Extraterrestrial solar spectrum 360–1050 nm from rotating shadowband spectroradiometer measurements at the Southern Great Plains (ARM) site. J. Geophys. Res. Atmos. 2003, 108. [Google Scholar] [CrossRef]
- Menang, K.P.; Coleman, M.D.; Gardiner, T.D.; Ptashnik, I.V.; Shine, K.P. A high-resolution near-infrared extraterrestrial solar spectrum derived from ground-based Fourier transform spectrometer measurements. J. Geophys. Res. Atmos. 2013, 118, 5319–5331. [Google Scholar] [CrossRef]
- Bolsée, D.; Pereira, N.; Decuyper, W.; Gillotay, D.; Yu, H.; Sperfeld, P.; Pape, S.; Cuevas, E.; Redondas, A.; Hernandéz, Y.; et al. Accurate Determination of the TOA Solar Spectral NIR Irradiance Using a Primary Standard Source and the Bouguer-Langley Technique. Sol. Phys. 2014, 289, 2433–2457. [Google Scholar] [CrossRef]
- Gröbner, J.; Kröger, I.; Egli, L.; Hülsen, G.; Riechelmann, S.; Sperfeld, P. The high-resolution extraterrestrial solar spectrum (QASUMEFTS) determined from ground-based solar irradiance measurements. Atmos. Meas. Tech. 2017, 10, 3375–3383. [Google Scholar] [CrossRef]
- Elsey, J.; Coleman, M.D.; Gardiner, T.; Shine, K.P. Can Measurements of the Near-Infrared Solar Spectral Irradiance be Reconciled? A New Ground-Based Assessment Between 4000 and 10,000 cm−1. Geophys. Res. Lett. 2017, 44, 10071–10080. [Google Scholar] [CrossRef]
- Pereira, N.; Bolsée, D.; Sperfeld, P.; Pape, S.; Sluse, D.; Cessateur, G. Metrology of solar spectral irradiance at the top of the atmosphere in the near infrared measured at Mauna Loa Observatory: The PYR-ILIOS campaign. Atmos. Meas. Tech. 2018, 11, 6605–6615. [Google Scholar] [CrossRef]
- Thuillier, G.; Hersé, M.; Labs, D.; Foujols, T.; Peetermans, W.; Gillotay, D.; Simon, P.; Mandel, H. The solar spectral irradiance from 200 to 2400 nm as measured by the SOLSPEC spectrometer from the ATLAS and EURECA missions. Sol. Phys. 2003, 214, 1–22. [Google Scholar] [CrossRef]
- BenMoussa, A.; Gissot, S.; Schühle, U.; Del Zanna, G.; Auchère, F.; Mekaoui, S.; Jones, A.R.; Walton, D.; Eyles, C.J.; Thuillier, G.; et al. On-Orbit Degradation of Solar Instruments. Sol. Phys. 2013, 288, 389–434. [Google Scholar] [CrossRef]
- Meftah, M.; Dominique, M.; BenMoussa, A.; Dammasch, I.E.; Bolsée, D.; Pereira, N.; Damé, L.; Bekki, S.; Hauchecorne, A. On-orbit degradation of recent space-based solar instruments and understanding of the degradation processes. In Sensors and Systems for Space Applications X, Proceedings of the Society of Photo-Optical Instrumentation Engineers (SPIE) Conference Series, Anaheim, CA, USA, 2017; Pham, K.D., Chen, G., Eds.; SPIE: Paris, France, 2017; Volume 10196, p. 1019606. [Google Scholar] [CrossRef]
- Schöll, M.; de Wit, T.D.; Kretzschmar, M.; Haberreiter, M. Making of a solar spectral irradiance dataset I: Observations, uncertainties, and methods. J. Space Weather Space Clim. 2016, 6, A14. [Google Scholar] [CrossRef][Green Version]
- Walker, J.H. Spectral Irradiance Calibrations; US Department of Commerce, National Bureau of Standards: Gaithersburg, MD, USA, 1987; Volume 250.
- Mielenz, K.D.; Saunders, R.D.; Parr, A.C.; Hsia, J.J. The 1990 NIST scales of thermal radiometry. J. Res. Natl. Inst. Stand. Technol. 1990, 95, 621. [Google Scholar] [CrossRef]
- Taylor, B.N.; Kuyatt, C.E. Guidelines for Evaluating and Expressing the Uncertainty of NIST Measurement Results; US Department of Commerce, Technology Administration, National Institute of Standards and Technology: Gaithersburg, MD, USA, 1994; Volume 1297.
- Crouzet, P.E.; Duvet, L.; De Wit, F.; Beaufort, T.; Blommaert, S.; Butler, B.; Van Duinkerken, G.; ter Haar, J.; Heijnen, J.; van der Luijt, K.; et al. Quantum efficiency test set up performances for NIR detector characterization at ESTEC. In Proceedings of the High Energy, Optical, and Infrared Detectors for Astronomy VI, Montréal, QC, Canada, 22–25 June 2014; Volume 9154, pp. 476–488. [Google Scholar]
- Langley, S.P. Research on Solar Heat and Its Absorption by the Earth’s Atmosphere; US Government Printing Office: Washington, DC, USA, 1884; Volume 15.
- Kasten, F.; Young, A.T. Revised optical air mass tables and approximation formula. Appl. Opt. 1989, 28, 4735–4738. [Google Scholar] [CrossRef] [PubMed]
- Bodhaine, B.A.; Wood, N.B.; Dutton, E.G.; Slusser, J.R. On Rayleigh optical depth calculations. J. Atmos. Ocean. Technol. 1999, 16, 1854–1861. [Google Scholar] [CrossRef]
- Harrison, L.; Michalsky, J.; Berndt, J. Automated multifilter rotating shadow-band radiometer: An instrument for optical depth and radiation measurements. Appl. Opt. 1994, 33, 5118–5125. [Google Scholar] [CrossRef] [PubMed]
- Liu, C.; Li, Y.; Gao, W.; Shi, R.; Bai, K. Retrieval of columnar water vapor using multispectral radiometer measurements over northern China. J. Appl. Remote Sens. 2011, 5, 053558. [Google Scholar] [CrossRef]
- Thome, K.J.; Smith, M.W.; Palmer, J.M.; Reagan, J.A. Three-channel solar radiometer for the determination of atmospheric columnar water vapor. Appl. Opt. 1994, 33, 5811–5819. [Google Scholar] [CrossRef]
- Bovensmann, H.; Burrows, J.; Buchwitz, M.; Frerick, J.; Noel, S.; Rozanov, V.; Chance, K.; Goede, A. SCIAMACHY: Mission objectives and measurement modes. J. Atmos. Sci. 1999, 56, 127–150. [Google Scholar] [CrossRef]
- Thuillier, G.; Harder, J.; Shapiro, A.; Woods, T.; Perrin, J.M.; Snow, M.; Sukhodolov, T.; Schmutz, W. SOLSPEC investigation on board the International Space Station: The Absolute Solar Spectral Irradiance in the Infrared Domain. In Proceedings of the EGU General Assembly Conference Abstracts, Vienna, Austria, 12–17 April 2015; p. 9663. [Google Scholar]
- Harder, J.W.; Lawrence, G.M.; Rottman, G.J.; Woods, T.N. Spectral Irradiance Monitor (SIM) for the SORCE mission. In Proceedings of the Earth Observing Systems V, San Diego, CA, USA, 2–4 August 2000; Volume 4135, pp. 204–214. [Google Scholar]
- Hilbig, T.; Weber, M.; Bramstedt, K.; Noël, S.; Burrows, J.; Krijger, J.; Snel, R.; Meftah, M.; Damé, L.; Bekki, S.; et al. The new SCIAMACHY reference solar spectral irradiance and its validation. Sol. Phys. 2018, 293, 1–26. [Google Scholar] [CrossRef]
- Avrett, E.H. The solar temperature minimum and chromosphere. In Proceedings of the Current Theoretical Models and Future High Resolution Solar Observations: Preparing for ATST, Sunspot, NM, USA, 11–15 March 2002; Volume 286, p. 419. [Google Scholar]



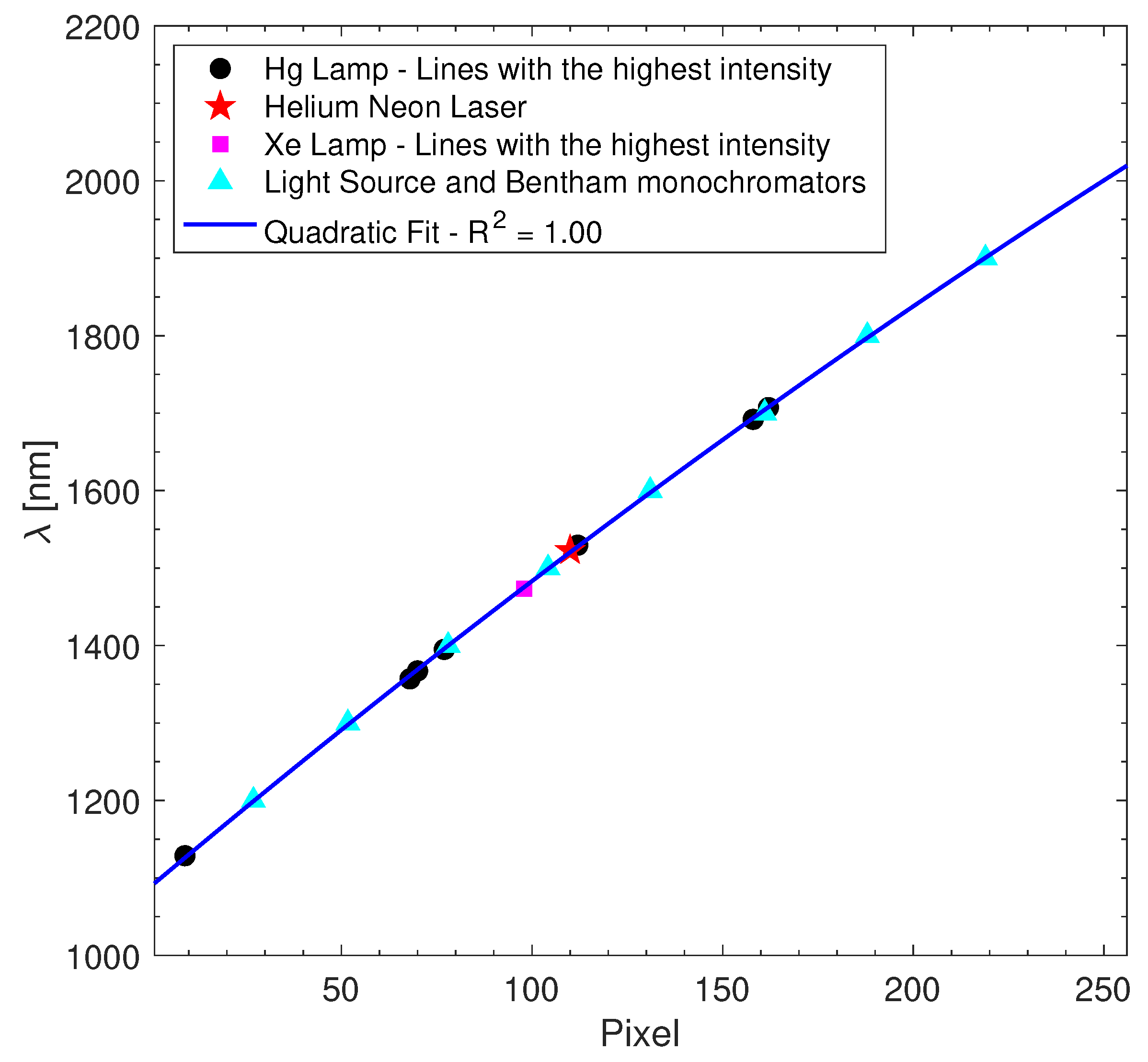
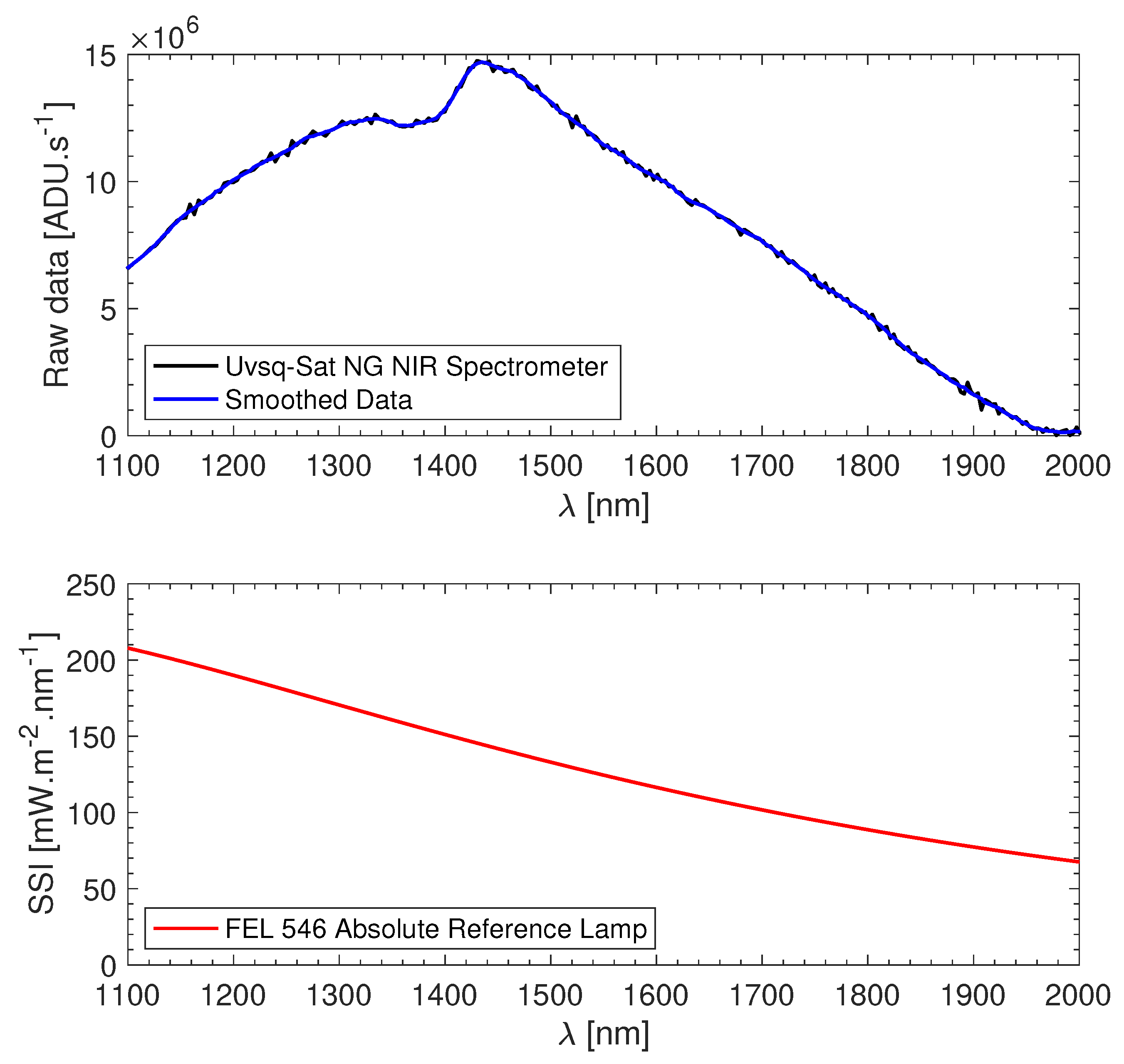
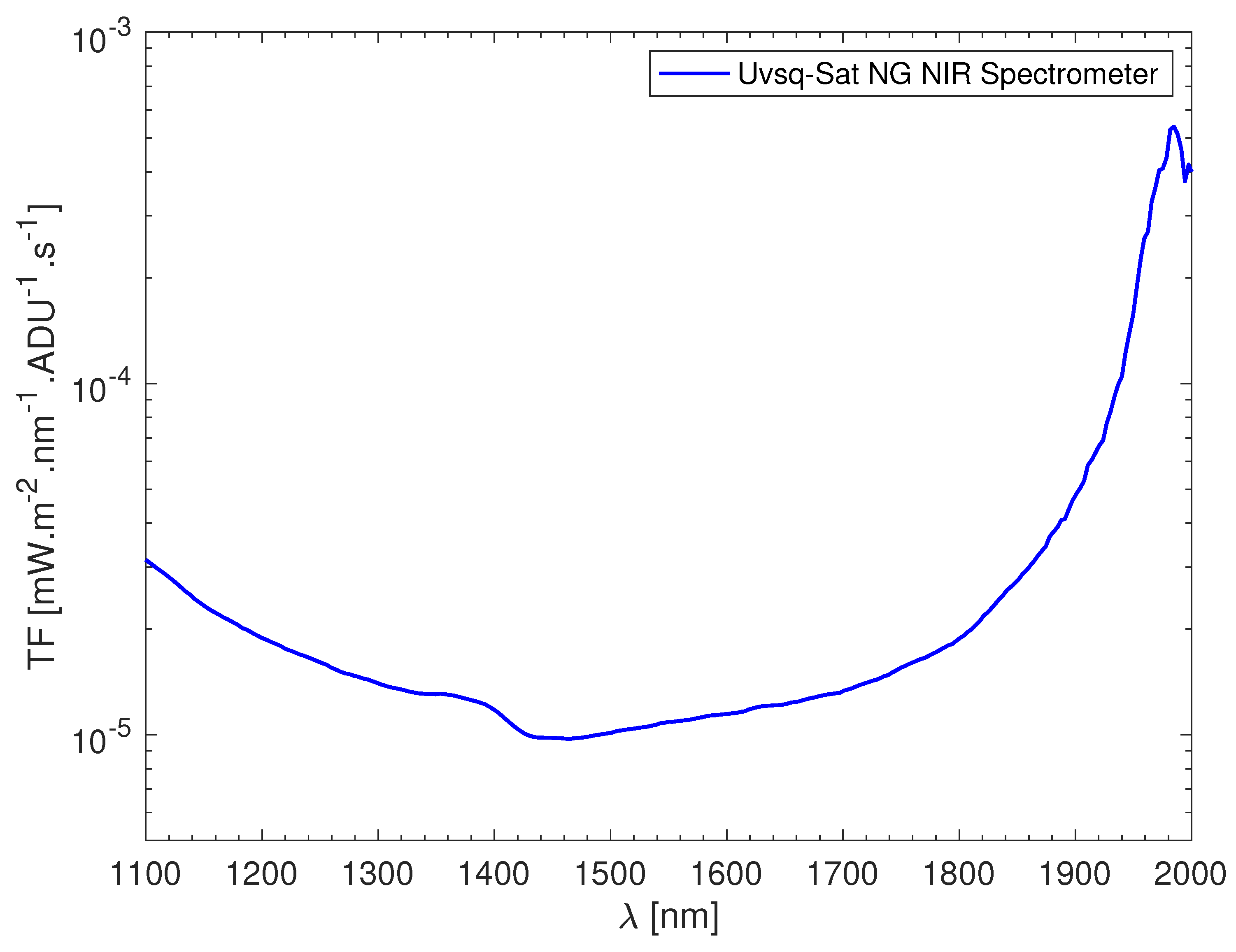
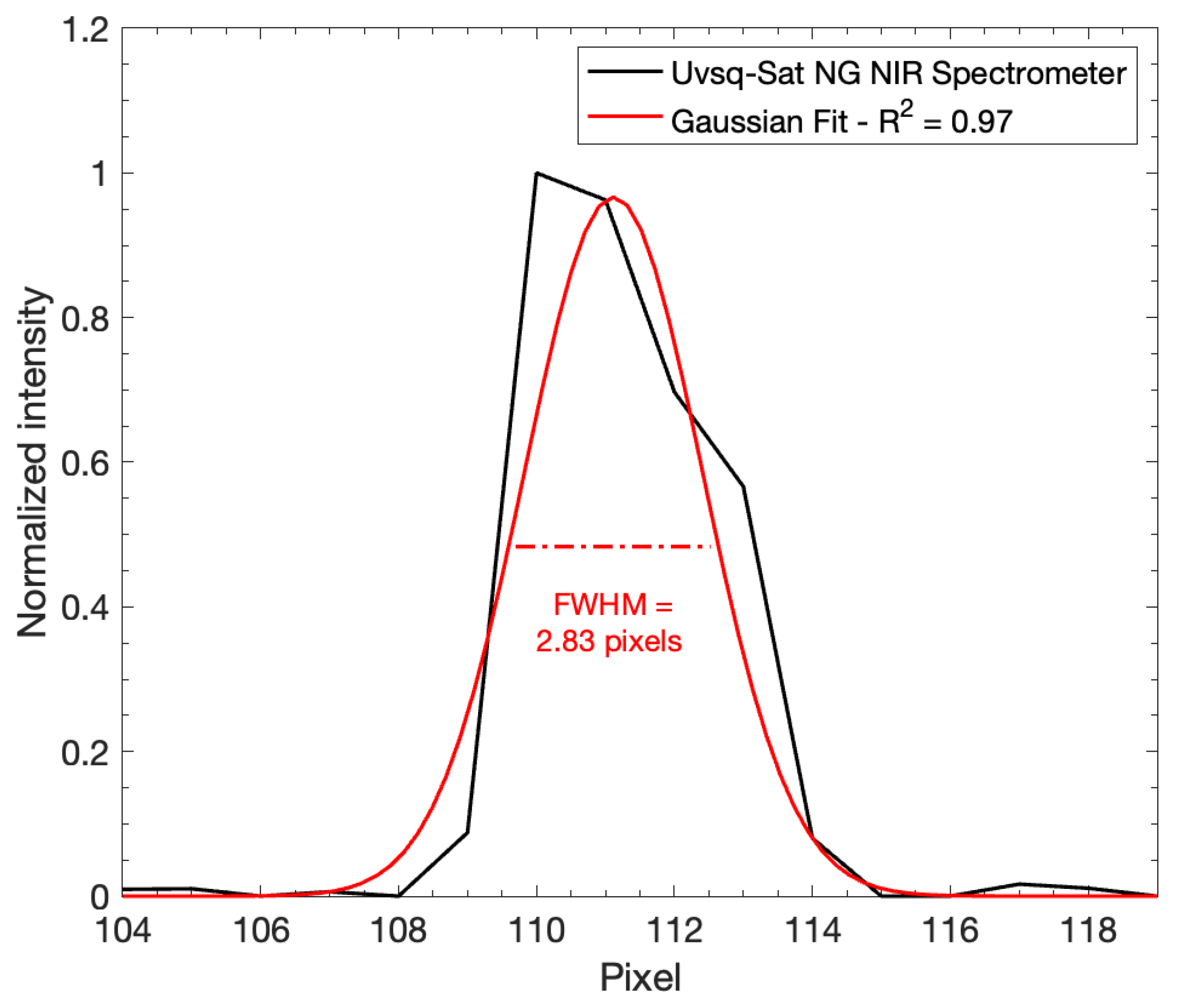
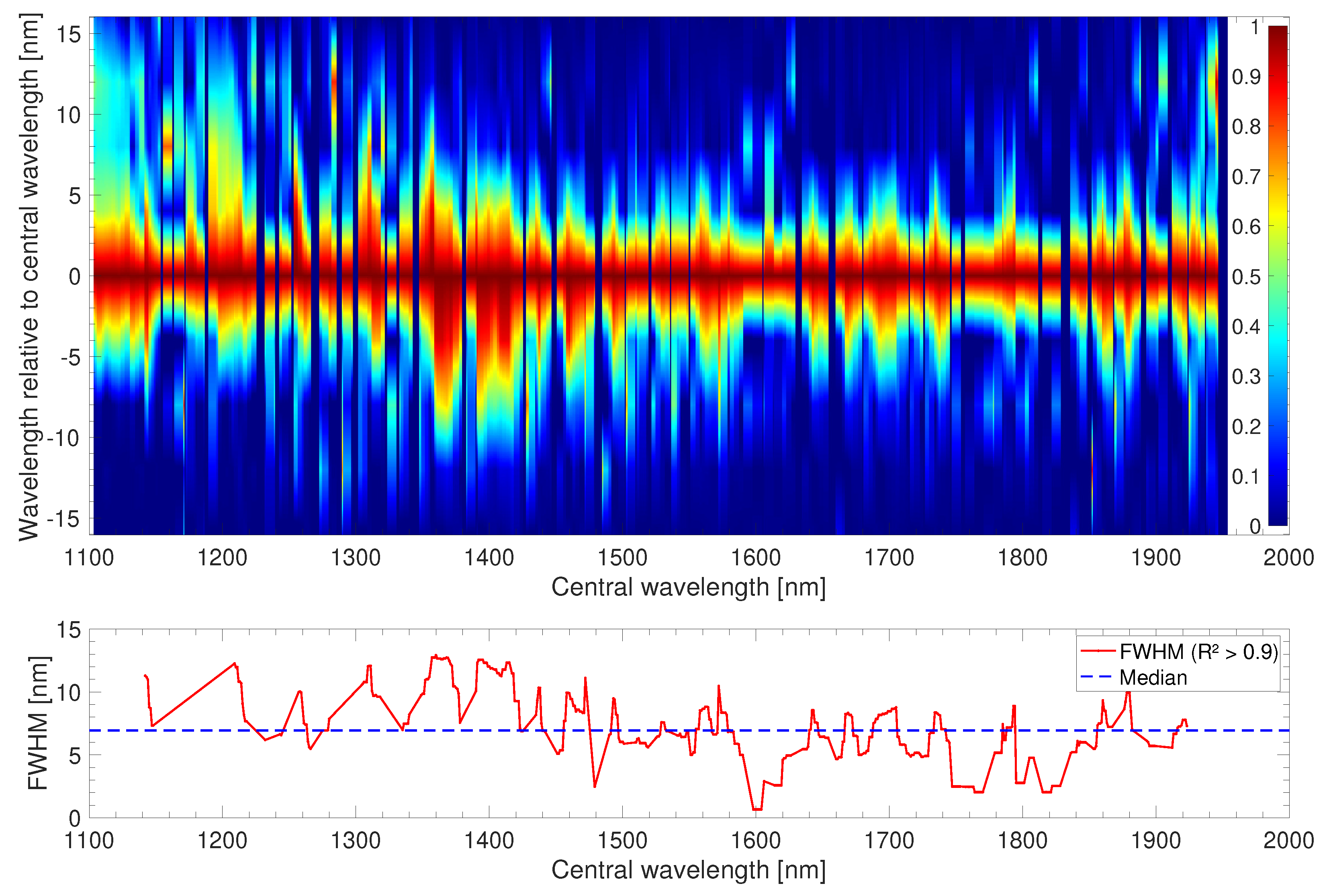

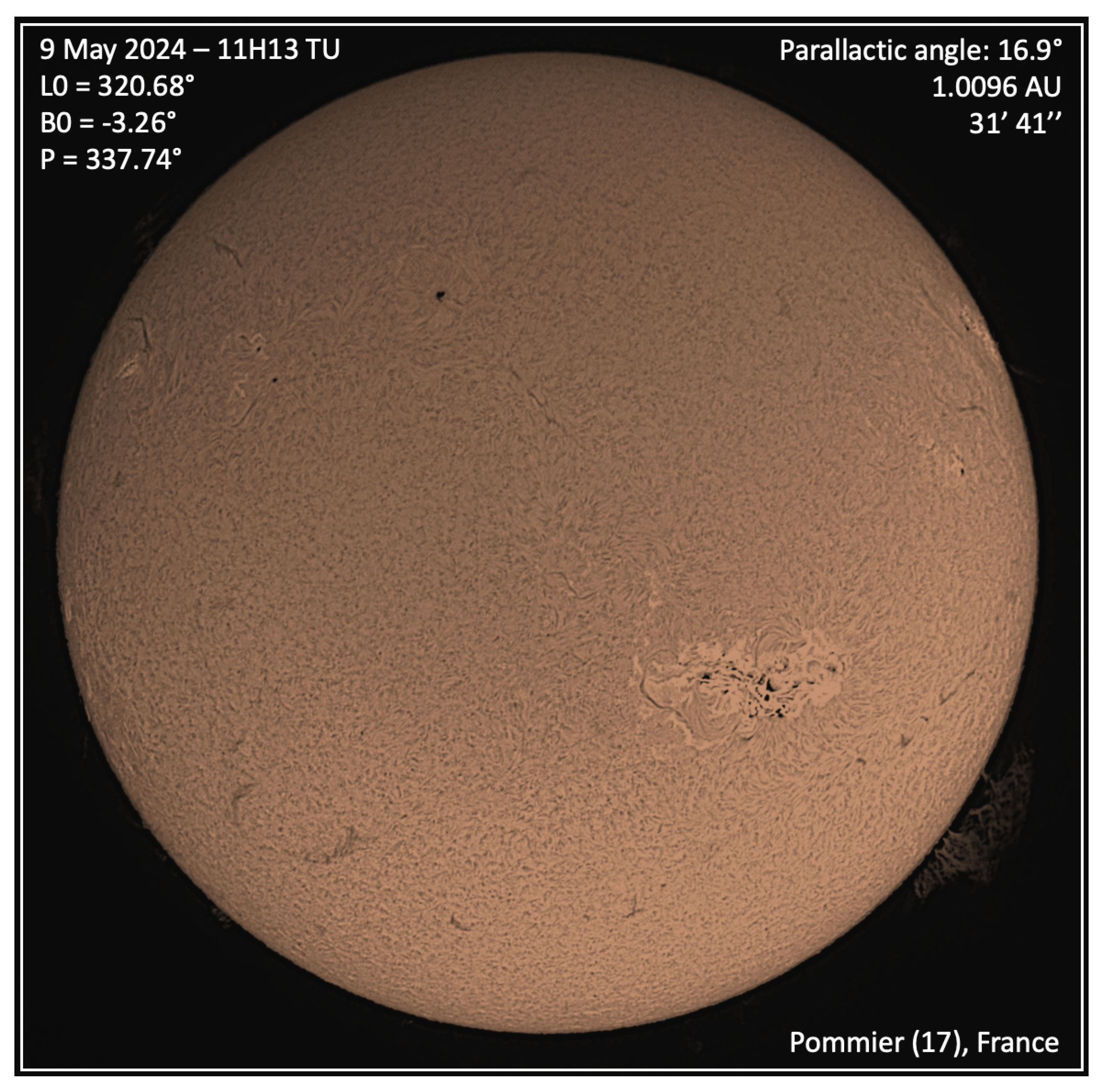


| Ion | –Air [nm] | Unc. [nm] | Rel. Int. |
|---|---|---|---|
| Hg I | 1128.710 | 0.0400 | 1000 |
| 1357.021 | 0.0200 | 200 | |
| 1367.351 | 0.0200 | 300 | |
| 1395.055 | 0.0200 | 200 | |
| 1529.582 | 0.0200 | 600 | |
| 1692.016 | 0.0200 | 1600 | |
| 1707.279 | 0.0200 | 80 | |
| Xe I | 1108.524 | 0.0004 | 1900 |
| 1174.224 | 0.0004 | 1750 | |
| 1295.644 | - | 2.3 | |
| 1314.671 | - | 1.5 | |
| 1473.238 | 0.0100 | 200 | |
| 1665.763 | - | 2.8 | |
| 1672.816 | 0.0008 | 5000 | |
| 1732.580 | 0.0009 | 1650 | |
| 1878.815 | 0.0011 | 860 | |
| Kr I | 1286.189 | 0.0004 | 76,000 |
| 1373.886 | 0.0004 | 310,000 | |
| 1404.566 | 0.0004 | 106,000 | |
| He-Ne | 1523.071 | - | - |
| [nm] | [mW.m−2.nm−1] | (k = 2) [%] |
|---|---|---|
| 1100 | 207.45 | 1.11 |
| 1200 | 189.90 | 1.12 |
| 1300 | 170.40 | 1.13 |
| 1540 | 126.10 | 1.36 |
| 1600 | 116.40 | 1.42 |
| 1700 | 101.60 | 1.65 |
| 2000 | 67.72 | 2.33 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Meftah, M.; Dufour, C.; Bolsée, D.; Van Laeken, L.; Clavier, C.; Chandran, A.; Chang, L.; Sarkissian, A.; Galopeau, P.; Hauchecorne, A.; et al. Advancing CubeSats Capabilities: Ground-Based Calibration of Uvsq-Sat NG Satellite’s NIR Spectrometer and Determination of the Extraterrestrial Solar Spectrum. Remote Sens. 2024, 16, 3655. https://doi.org/10.3390/rs16193655
Meftah M, Dufour C, Bolsée D, Van Laeken L, Clavier C, Chandran A, Chang L, Sarkissian A, Galopeau P, Hauchecorne A, et al. Advancing CubeSats Capabilities: Ground-Based Calibration of Uvsq-Sat NG Satellite’s NIR Spectrometer and Determination of the Extraterrestrial Solar Spectrum. Remote Sensing. 2024; 16(19):3655. https://doi.org/10.3390/rs16193655
Chicago/Turabian StyleMeftah, Mustapha, Christophe Dufour, David Bolsée, Lionel Van Laeken, Cannelle Clavier, Amal Chandran, Loren Chang, Alain Sarkissian, Patrick Galopeau, Alain Hauchecorne, and et al. 2024. "Advancing CubeSats Capabilities: Ground-Based Calibration of Uvsq-Sat NG Satellite’s NIR Spectrometer and Determination of the Extraterrestrial Solar Spectrum" Remote Sensing 16, no. 19: 3655. https://doi.org/10.3390/rs16193655
APA StyleMeftah, M., Dufour, C., Bolsée, D., Van Laeken, L., Clavier, C., Chandran, A., Chang, L., Sarkissian, A., Galopeau, P., Hauchecorne, A., Dahoo, P.-R., Damé, L., Vieau, A.-J., Bertran, E., Gilbert, P., Ferreira, F., Engler, J.-L., Montaron, C., Mangin, A., ... Keckhut, P. (2024). Advancing CubeSats Capabilities: Ground-Based Calibration of Uvsq-Sat NG Satellite’s NIR Spectrometer and Determination of the Extraterrestrial Solar Spectrum. Remote Sensing, 16(19), 3655. https://doi.org/10.3390/rs16193655









