An Improved Average Acceleration Approach of Modelling Earth Gravity Field Based on K-Band Range-Rate Observations
Abstract
1. Introduction
2. Methodology
3. Data Processing
| Observation Data | |
|---|---|
| GNV1B | Reduced dynamic orbit data with sample rate of 1 Hz |
| SCA1B | Rotation quaternion from inertial frame to GRACE science reference frame with sample rate of 1 Hz |
| ACT1B | Transplanted non-conservative acceleration data with sample rate of 1 Hz |
| KBR1B | K-band ranging data, including inter-satellite range, its first and second order derivatives and corresponding phase center and light-time corrections, with sample rate of 0.2 Hz |
| Back ground model | |
| Earth’s gravity field | GOCO06s model with static component up to degree and order 300 and temporal component up to degree and order 200 |
| Ocean tide | FES2014b model up to degree and order 180 with 34 major tidal constituents and 361 minor tidal constituents |
| N-body Perturbation | JPL DE430 planetary ephemerides, consider Sun, Moon, Venus, Mars, Saturn and Jupiter, direct and indirect J2 effect |
| Solid earth Tide | IERS Conventions 2010, include frequency independent term, frequency dependent term and permanent tide |
| Solid earth pole tide | IERS Conventions 2010 |
| Ocean pole tide | Desai [25], up to degree and order 180 |
| Atmospheric and oceanic variability | AOD1B RL06, linear interpolation, include 12 atmosphere tidal constituents |
| General relativistic effect | IERS Conventions 2010 |
| Estimated parameter | |
| Spherical harmonic coefficients | Complete to degree and order 96 |
| Accelerometer calibration parameters | Biases, once per revolution (1.5 h), include biases in along-track, cross-track and radial direction; Scales, once per day, full scale matrix |
| K-band ranging empirical parameters | Bias and slope, once per half revolution; One cycle-per-revolution (1CPR) components, once per revolution |
4. Results
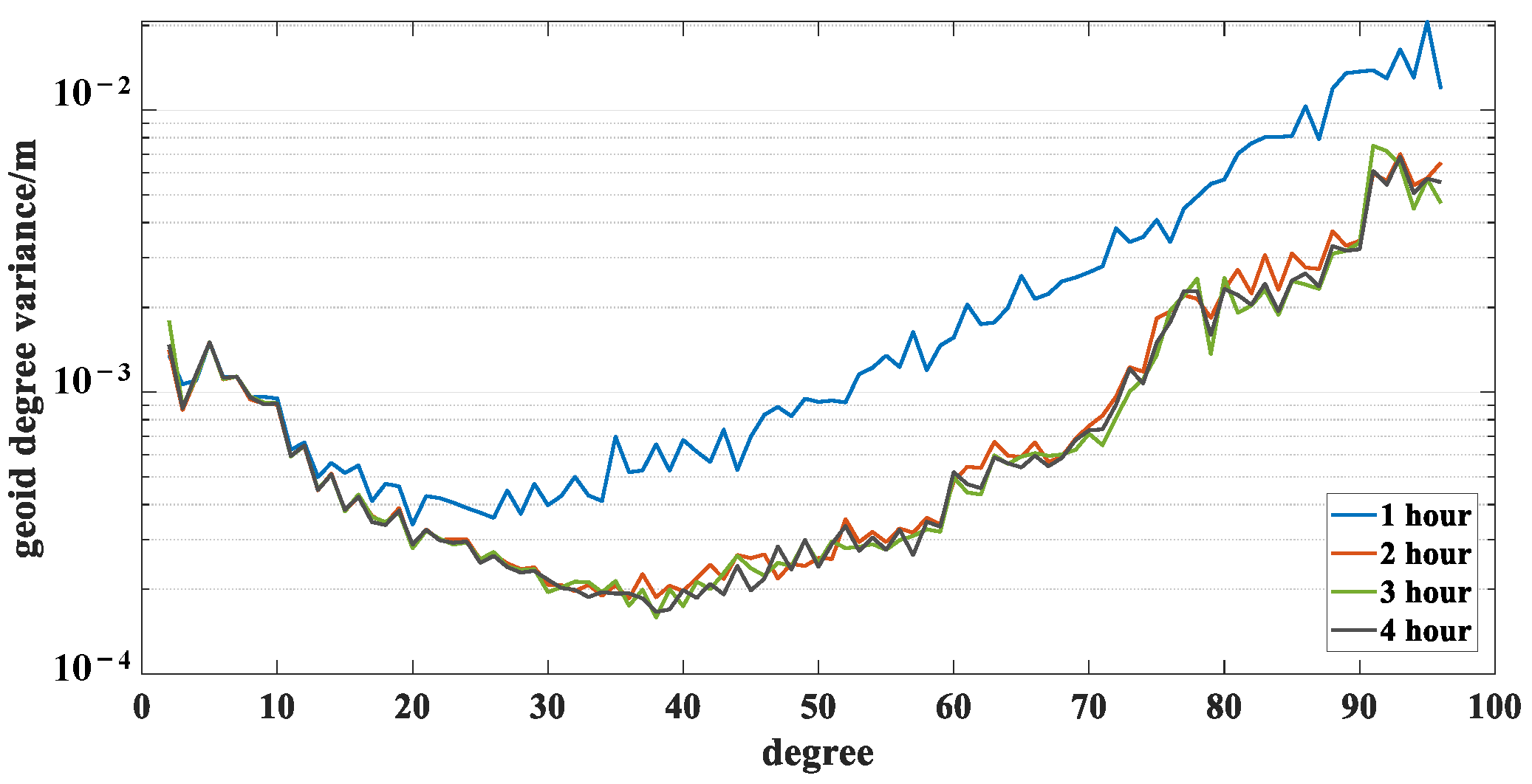
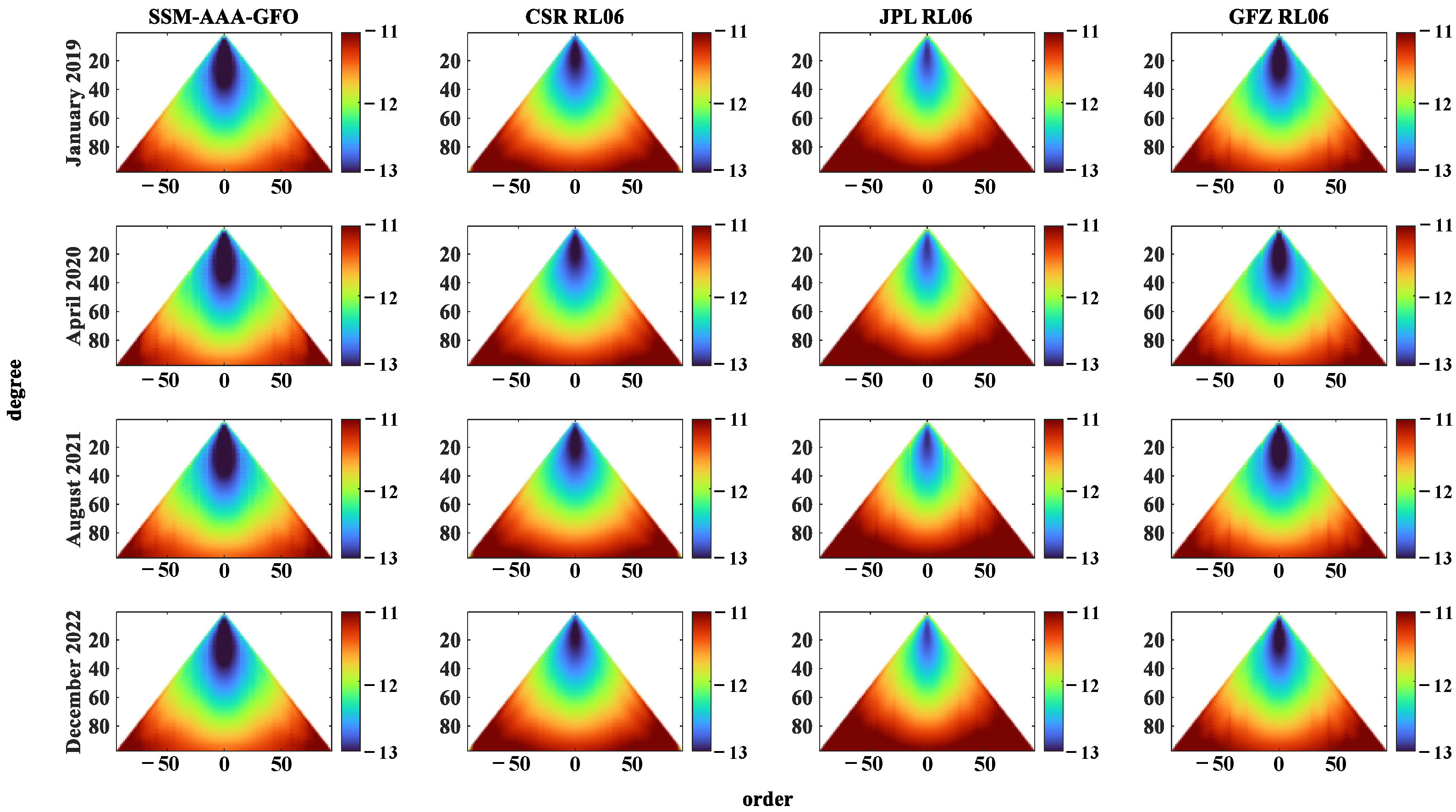
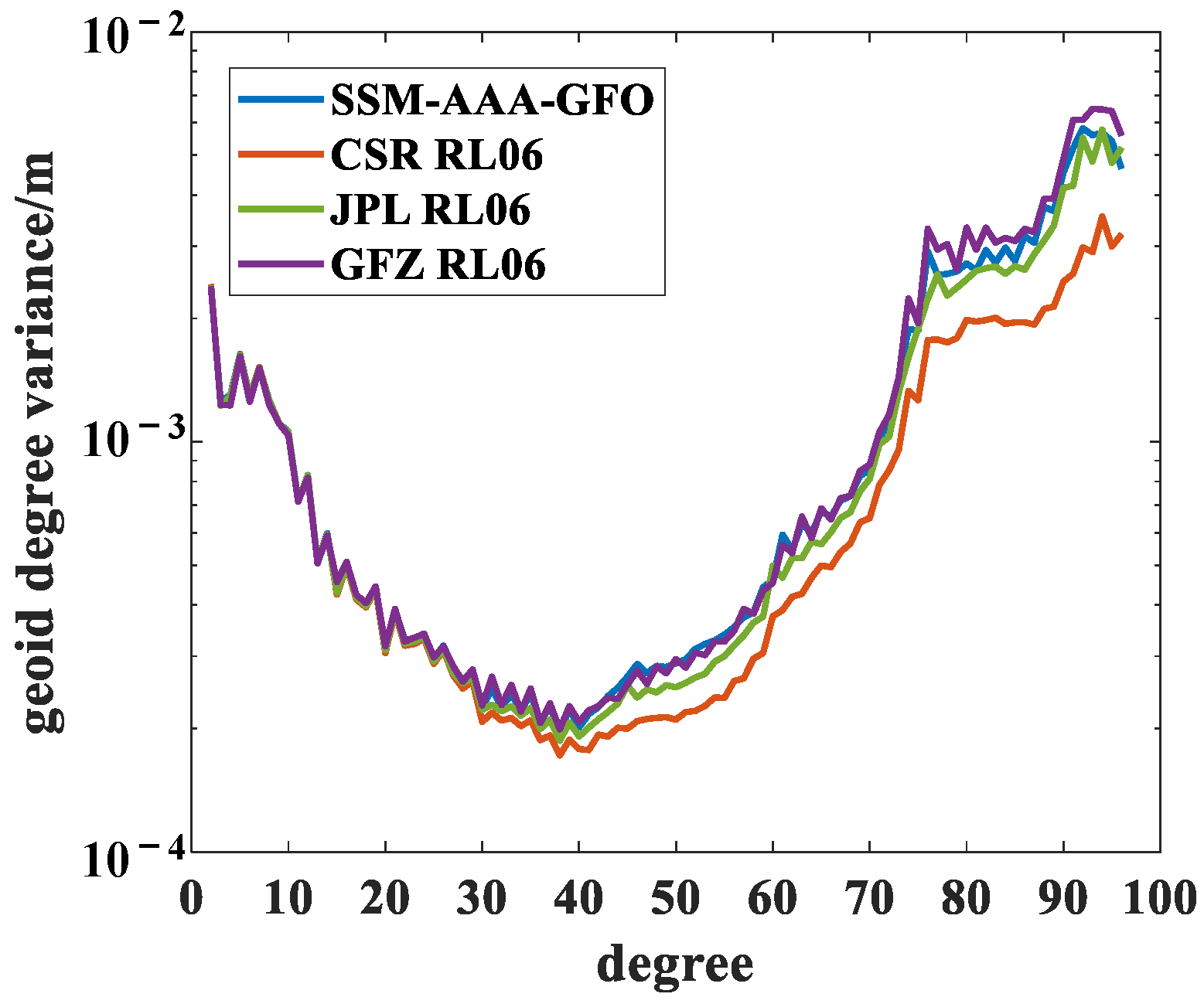
4.1. Spectral Analysis
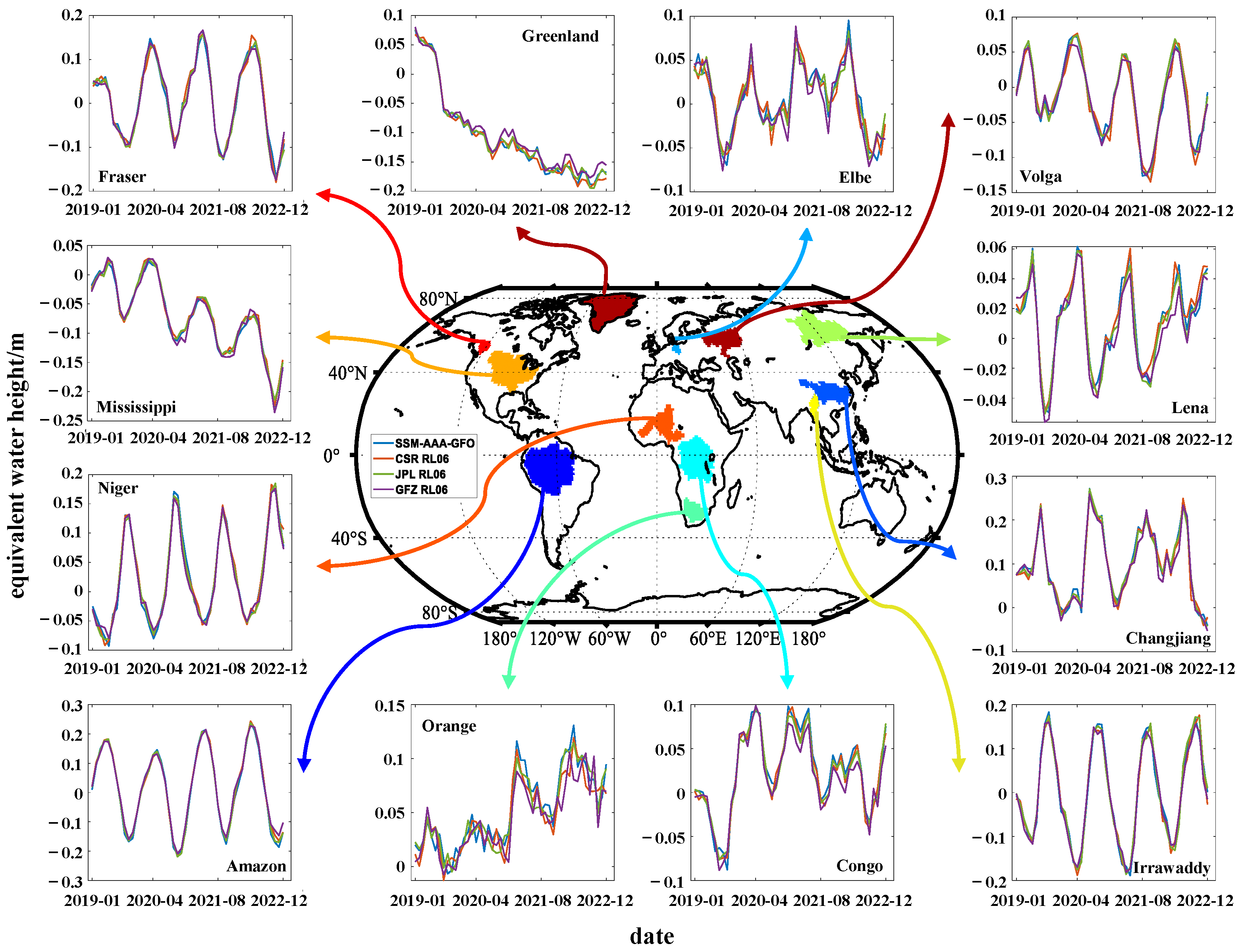
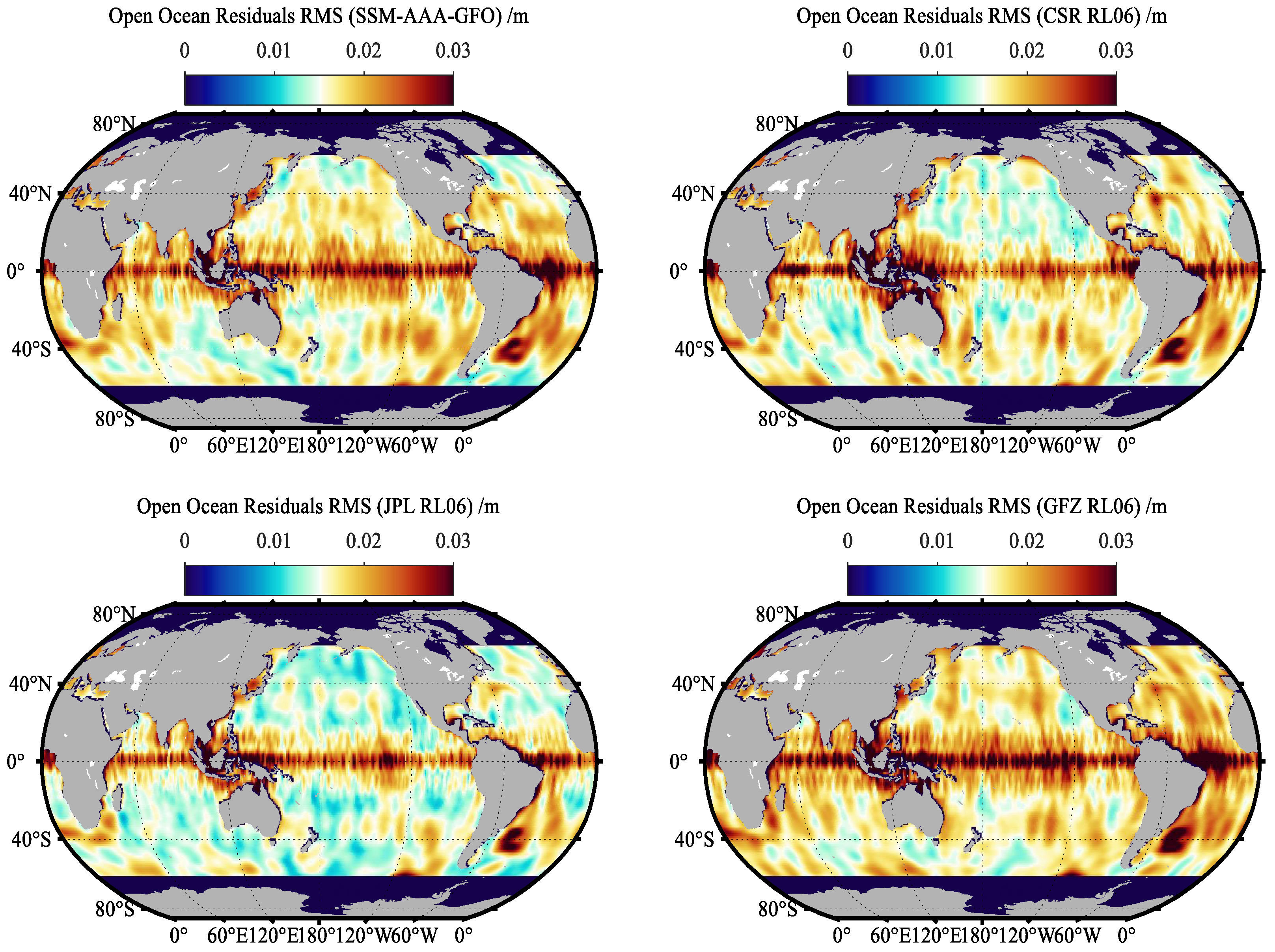
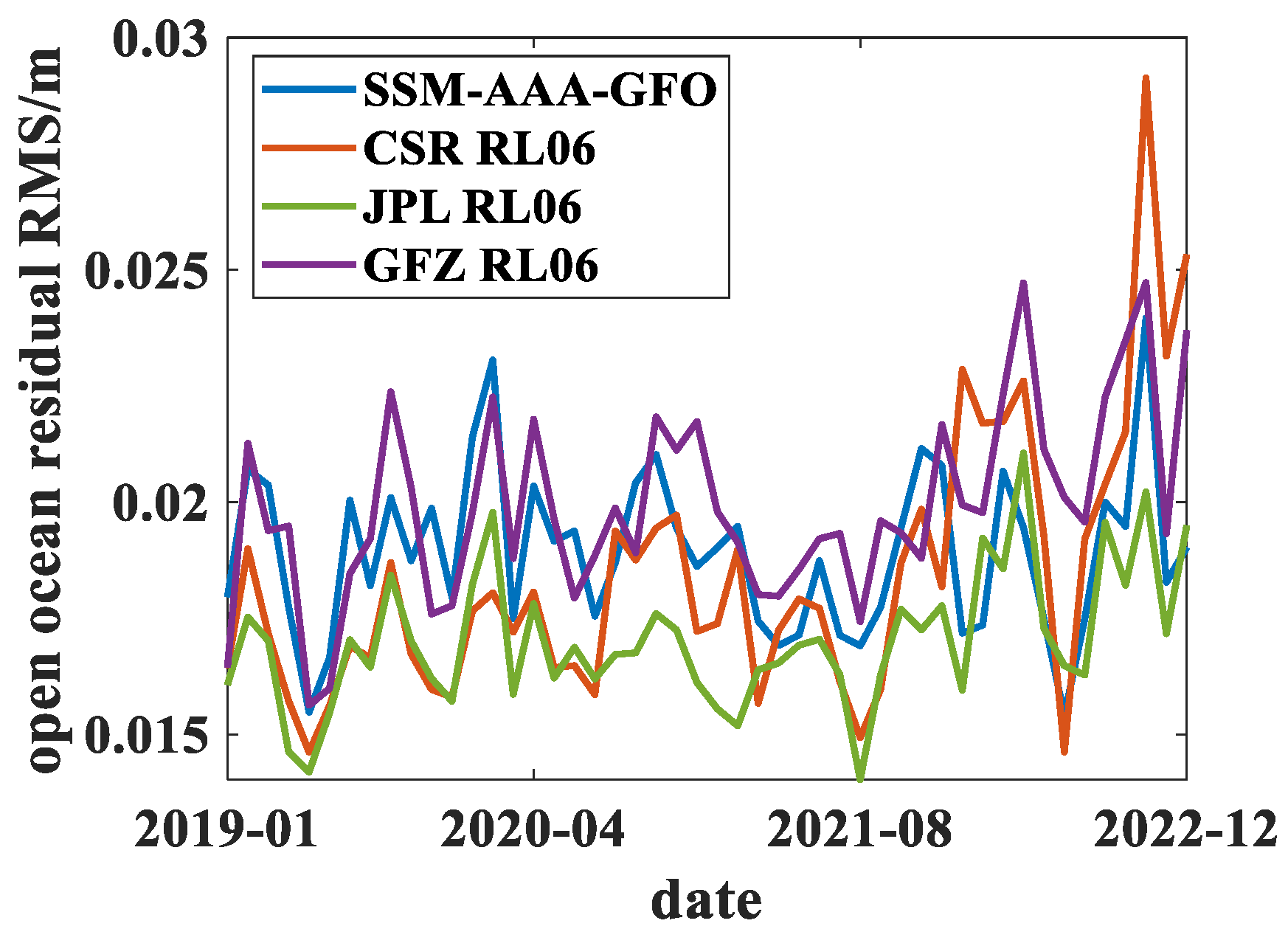
4.2. Spatial Analysis
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Tapley, B.D.; Bettadpur, S.; Watkins, M.; Reigber, C. The gravity recovery and climate experiment: Mission overview and early results. Geophys. Res. Lett. 2004, 31, L09607. [Google Scholar] [CrossRef]
- Tapley, B.D.; Watkins, M.M.; Flechtner, F.; Reigber, C.; Bettadpur, S.; Rodell, M.; Sasgen, I.; Famiglietti, J.S.; Landerer, F.W.; Chambers, D.P.; et al. Contributions of GRACE to understanding climate change. Nat. Clim. Chang. 2019, 9, 358–369. [Google Scholar] [CrossRef]
- Flechtner, F.; Morton, P.; Watkins, M.; Webb, F. Status of the GRACE follow-on mission. In Gravity, Geoid and Height Systems; Marti, U., Ed.; Springer: Berlin, Germany, 2014; pp. 117–121. [Google Scholar]
- Flechtner, F.; Neumayer, K.H.; Dahle, C.; Dobslaw, H.; Fagiolini, E.; Raimondo, J.C.; Güntner, A. What can be expected from the GRACE-FO laser ranging interferometer for earth science applications? In Remote Sensing and Water Resources; Cazenave, A., Champollion, N., Benveniste, J., Chen, J., Eds.; Springer: Berlin, Germany, 2016; pp. 263–280. [Google Scholar]
- Zhou, H.; Zheng, L.; Li, Y.; Guo, X.; Zhou, Z.; Luo, Z. HUST-Grace2024: A new GRACE-only gravity field time series based on more than 20 years of satellite geodesy data and a hybrid processing chain. Earth Syst. Sci. Data 2024, 16, 3261–3281. [Google Scholar] [CrossRef]
- Zhou, H.; Luo, Z.; Zhong, B. WHU-Grace01s: A new temporal gravity field model recovered from GRACE KBRR data alone. Geod. Geodyn. 2015, 6, 316–323. [Google Scholar] [CrossRef]
- Chen, Q.; Shen, Y.; Zhang, X.; Hsu, H.; Chen, W.; Ju, X.; Lou, L. Monthly gravity field models derived from GRACE Level 1B data using a modified short-arc approach. J. Geophys. Res. Solid Earth 2015, 120, 1804–1819. [Google Scholar] [CrossRef]
- Chen, Q.; Shen, Y.; Chen, W.; Francis, O.; Zhang, X.; Chen, Q.; Li, W.; Chen, T. An optimized short-arc approach: Methodology and application to develop refined time series of Tongji-Grace2018 GRACE monthly solutions. J. Geophys. Res. Solid Earth 2019, 124, 6010–6038. [Google Scholar] [CrossRef]
- Shang, K.; Guo, J.; Shum, C.K.; Dai, C.; Luo, J. GRACE time-variable gravity field recovery using an improved energy balance approach. Geophys. J. Int. 2015, 203, 1773–1786. [Google Scholar] [CrossRef]
- Zhong, B.; Li, X.; Chen, J.; Li, Q. WHU-GRACE-GPD01s: A Series of Constrained Monthly Gravity Field Solutions Derived From GRACE-Based Geopotential Differences. Earth Space Sci. 2023, 10, e2022EA002699. [Google Scholar] [CrossRef]
- Ditmar, P.; Sluijs, A.V.E.V.D. A technique for modeling the Earth’s gravity field on the basis of satellite accelerations. J. Geod. 2004, 78, 12–33. [Google Scholar] [CrossRef]
- Ditmar, P.; Sluijs, A.V.E.V.D.; Kuznetsov, V. Modeling the Earth’s gravity field from precise satellite orbit data: The acceleration approach works! In Proceedings of the “GOCE, The Geoid and Oceanography”, Frascati, Italy, 8–10 March 2004. [Google Scholar]
- Liu, X.; Ditmar, P.; Siemes, C.; Slobbe, D.C.; Revtova, E.; Klees, R.; Riva, R.; Zhao, Q. DEOS Mass Transport model (DMT-1) based on GRACE satellite data: Methodology and validation. Geophys. J. Int. 2010, 181, 769–788. [Google Scholar] [CrossRef]
- Ditmar, P.; Kuznetsov, V.; Sluijs, A.V.E.V.D.; Schrama, E.; Klees, R. ‘DEOS_CHAMP-01C_70’: A model of the Earth’s gravity field computed from accelerations of the CHAMP satellite. J. Geod. 2006, 79, 586–601. [Google Scholar] [CrossRef][Green Version]
- Farahani, H.H.; Ditmar, P.; Inácio, P.; Didova, O.; Gunter, B.; Klees, R.; Guo, X.; Guo, J.; Sun, Y.; Liu, X.; et al. A high resolution model of linear trend in mass variations from DMT-2: Added value of accounting for coloured noise in GRACE data. J. Geodyn. 2017, 103, 12–25. [Google Scholar] [CrossRef][Green Version]
- Farahani, H.H.; Ditmar, P.; Klees, R.; Liu, X.; Zhao, Q.; Guo, J. The static gravity field model DGM-1S from GRACE and GOCE data: Computation, validation and an analysis of GOCE mission’s added value. J. Geod. 2013, 87, 843–867. [Google Scholar] [CrossRef]
- Chen, Q.; Shen, Y.; Chen, W.; Zhang, X.; Hsu, H.; Ju, X. A modified acceleration-based monthly gravity field solution from GRACE data. Geophys. J. Int. 2015, 202, 1190–1206. [Google Scholar] [CrossRef]
- Shen, Z.; Chen, Q.; Shen, Y. An Improved Acceleration Approach by Utilizing K-Band Range Rate Observations. Remote Sens. 2023, 15, 5260. [Google Scholar] [CrossRef]
- Darbeheshti, N.; Lasser, M.; Meyer, U.; Arnold, D.; Jäggi, A. AIUB-GRACE gravity field solutions for G3P: Processing strategies and instrument parameterization. Earth Syst. Sci. Data 2024, 16, 1589–1599. [Google Scholar] [CrossRef]
- Kvas, A.; Brockmann, J.M.; Krauss, S.; Schubert, T.; Gruber, T.; Meyer, U. GOCO06s—A satellite-only global gravity field model. Earth Syst. Sci. Data 2021, 13, 99–118. [Google Scholar] [CrossRef]
- Lyard, F.H.; Allain, D.J.; Cancet, M.; Carrère, L.; Picot, N. FES2014 global ocean tide atlas: Design and performance. Ocean. Sci. 2021, 17, 615–649. [Google Scholar] [CrossRef]
- Dobslaw, H.; Bergmann-Wolf, I.; Dill, R.; Poropat, L.; Thomas, M.; Dahle, C.; Esselborn, S.; König, R.; Flechtner, F. A new high-resolution model of non-tidal atmosphere and ocean mass variability for de-aliasing of satellite gravity observations: AOD1B RL06. Geophys. J. Int. 2017, 211, 263–269. [Google Scholar] [CrossRef]
- Folkner, W.M.; Williams, J.G.; Boggs, D.H.; Park, R.S.; Kuchynka, P. The Planetary and Lunar Ephemerides DE430 and DE431. Available online: https://www.google.com/url?sa=t&source=web&rct=j&opi=89978449&url=https://naif.jpl.nasa.gov/pub/naif/generic_kernels/spk/planets/de430_and_de431.pdf&ved=2ahUKEwiwoITVgoCIAxVErokEHRS6PUkQFnoECBcQAQ&usg=AOvVaw2i8bA6P-_iLhX2Hkq3rCcl (accessed on 12 August 2024).
- Petit, G.; Luzum, B. IERS Conventions. (No. IERS-TN-36); Bureau International Des Poids et Mesures Sevres: Sèvres, France, 2010. [Google Scholar]
- Desai, S.D. Observing the pole tide with satellite altimetry. J. Geophys. Res. Ocean. 2002, 107, 7-1–7-13. [Google Scholar] [CrossRef]
- Chen, Q.; Shen, Y.; Francis, O.; Chen, W.; Zhang, X.; Hsu, H. Tongji-Grace02s and Tongji-Grace02k: High-precision static GRACE-only global Earth’s gravity field models derived by refined data processing strategies. J. Geophys. Res. Solid Earth 2018, 123, 6111–6137. [Google Scholar] [CrossRef]
- Meyer, U.; Jäggi, A.; Jean, Y.; Beutler, G. AIUB-RL02: An improved time-series of monthly gravity fields from GRACE data. Geophys. J. Int. 2016, 205, 1196–1207. [Google Scholar] [CrossRef]
- Duan, X.J.; Guo, J.Y.; Shum, C.K.; Van Der Wal, W. On the postprocessing removal of correlated errors in GRACE temporal gravity field solutions. J. Geod. 2009, 83, 1095–1106. [Google Scholar] [CrossRef]
- Bettadpur, S. UTCSR level-2 processing standards document for level-2 product release 0006. GRACE Rep. 2018, 327, 742. [Google Scholar]
- Chen, J.; Tapley, B.; Tamisiea, M.E.; Save, H.; Wilson, C.; Bettadpur, S.; Seo, K.W. Error assessment of GRACE and GRACE follow-on mass change. J. Geophys. Res. Solid Earth 2021, 126, e2021JB022124. [Google Scholar] [CrossRef]
- Feng, W. GRAMAT: A comprehensive Matlab toolbox for estimating global mass variations from GRACE satellite data. Earth Sci. Inform. 2018, 12, 389–404. [Google Scholar] [CrossRef]



Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tan, X.; Fan, D.; Feng, J.; Wan, H.; Xu, Z.; Li, S. An Improved Average Acceleration Approach of Modelling Earth Gravity Field Based on K-Band Range-Rate Observations. Remote Sens. 2024, 16, 3172. https://doi.org/10.3390/rs16173172
Tan X, Fan D, Feng J, Wan H, Xu Z, Li S. An Improved Average Acceleration Approach of Modelling Earth Gravity Field Based on K-Band Range-Rate Observations. Remote Sensing. 2024; 16(17):3172. https://doi.org/10.3390/rs16173172
Chicago/Turabian StyleTan, Xuli, Diao Fan, Jinkai Feng, Hongfa Wan, Zhenbang Xu, and Shanshan Li. 2024. "An Improved Average Acceleration Approach of Modelling Earth Gravity Field Based on K-Band Range-Rate Observations" Remote Sensing 16, no. 17: 3172. https://doi.org/10.3390/rs16173172
APA StyleTan, X., Fan, D., Feng, J., Wan, H., Xu, Z., & Li, S. (2024). An Improved Average Acceleration Approach of Modelling Earth Gravity Field Based on K-Band Range-Rate Observations. Remote Sensing, 16(17), 3172. https://doi.org/10.3390/rs16173172




