Technical Possibilities and Limitations of the DPS-4D Type of Digisonde in Individual Meteor Detections

Abstract

During the peak days of the 2019 Leonids and Geminids (16–19 November and 10–16 December), two ionograms/minute and one Skymap/minute campaign measurements were carried out at the Sopron (47.63°N, 16.72°E) and Průhonice (50.00°N, 14.60°E) Digisonde stations. The stations used frequencies between 1 and 17 MHz for the ionograms, and the Skymaps were made at 2.5 MHz. A temporary optical camera was also installed at Sopron with a lower brightness limit of +1 visual magnitude. The manual scaling of ionograms for November and December 2019 to study the behavior of the regular sporadic E layer was also completed. Although the distributions of the stations were similar, there were interesting differences despite the relative proximity of the stations. The optical measurements detected 88 meteors. A total of 376 meteor-induced traces were found on the Digisonde ionograms at a most probable amplitude (MPA) threshold of 4 dB and of these, 40 cases could be linked to reflections on the Skymaps, too. Of the 88 optical detections, 31 could be identified on the ionograms. The success of detections depends on the sensitivity of the instruments and the noise-filtering. Geometrically, meteors above 80 km and with an altitude angle of 40° or higher can be detected using the Digisondes.

1. Introduction

According to the definitions of the International Astronomical Union (IAU) in 2017, meteors are the light and associated physical phenomena (heat, shock, ionization, etc.), which result from the high-speed entry of a solid object from space into a gaseous atmosphere. The meteor phenomenon can be caused by a meteoroid, a comet, an asteroid or any solid matter with the appropriate combination of velocity, mass and mean-free-path in a planet’s atmosphere. A meteoroid’s size, by agreement, is roughly between 30 μm and 1 m. Meteor showers are groups of meteors produced by meteoroids of the same meteoroid stream.

The Leonid and Geminid meteor showers are widely known meteoroid swarms. The Leonids are associated with comet 55P/Temple-Tuttle [1], while the source of the Geminids is the asteroid-originated object 3200 Phaethon [2]. The Leonid meteor shower arrives between 6 November and 30 November, and the number of incoming meteors usually peaks on 17 November. The Geminid meteor shower takes place between 4 December and 17 December, usually peaking on 14 December.

The peak of the two main meteor showers overlaps with less-known, minor meteoroid swarms. The Leonids’ peak is simultaneously present with the Southern- and Northern-Taurids (active: 23 September–8 December; and 13 October–2 December, respectively) and the α-Monocerotids (active: 15 November–23 November). The σ-Hydrids (active: 22 November–4 January), the Monocerotids (active: 5 December–20 December), the Coma-Berenicids (active: 12 December–23 December) and the Antihelion Source (active: 10 December–20 September) swarms happen at the same time as the Geminids’ peak [3].

The Southern-Taurids originated from comet Encke and the Northern-Taurids are associated with asteroid 2004 TG₁₀, which, based on its orbital data, is probably a larger chunk of the comet Encke [4]. The origin of the α-Monocerotids and the Coma-Berenicids is unknown. The α-Monocerotids’ origin is presumably a long-period comet [5], while the Coma-Berenicids’ is probably a moderate-period comet. The Monocerotids are associated with a long-period comet C/1917 F1 (Mellish) [6]. The σ-Hydrids are suggested to originate from long-period comet C/2023 P1 (Nishimura) based on its orbit parameters, but this is not proven yet [7]. The Antihelion Source meteoroid stream is thought to originate from the Earth passing through the debris of comets and asteroids under Jupiter’s influence. The radiant point is in the direction opposite to the Sun in the sky, hence the name, antihelion [8].

Some meteors cannot be linked to a specific parental body based on their orbital data, and these are called sporadic meteors. At most, they can be classified as being of cometary or asteroidal origin. These can come from the remains of ancient, disintegrated comets, or from known meteor swarms that have left orbit.

When meteors enter or meteoric dust and metallic material is deposited in the Earth’s lower atmosphere, thin and faint layers of ionization can form via vertical ion convergence. This trapping mechanism is the combined effect of the vertical shear of the zonal winds, the meridional winds and the Lorentz force controlling the movement of ions through the local magnetic field. This ion convergence also creates the sporadic E layer (Es) phenomenon [9,10,11]. At mid-latitude the wind shear theory is also the currently accepted theory for the formation of sporadic E layers.

Under nighttime conditions, the meteoric smoke absorbs free electrons, significantly changing the usual balance between electrons and negative/positive ions [12]. Atomic oxygen also destroys negative ions, but this does not affect the electrons attached to meteoric smoke particles [13].

It should also be noted that Jacobi et al. [14] found a significant increase in sporadic E activity after the Geminids, with an average delay of 2.5 days. This is not the case for the Leonids, although a distinct increase of sporadic E activity in association with the Leonids event 1996 has been reported in low latitudes by Chandra et al. [15].

According to Stuart (Table 10-2) [16], the brighter a meteor, the higher the electron line density (electrons per meter of trail length). An expected value of roughly 10¹⁵ el/m is associated with a visual magnitude of 2.5, while −2.5 is associated with 10¹⁷ el/m. It should be noted that the Stuart’s [16] figures are based on the Sugar’s [17] tables, who based their figures on Manning and Eshleman’s [18] tables.

McKinley [19] noted that for bright meteors (below 0 magnitude), the enduring ionization’s height is greater than the corresponding height of the maximum light emitted. For 0 magnitude, these heights are roughly equal, while for fainter meteors (above 0 magnitude) the maximum of the visible light is above the radio-echo’s height.

Meteor trails are usually classified as underdense or overdense. A common approximation is to consider the meteor trail as an infinitely long right circular cylinder of electrons, through which an incident electromagnetic wave passes without being significantly modified. Stuart [16] defines underdense trails in this way. If this assumption is not valid and the incident wave is significantly modified, the trail is overdense. This arises if ionization occurs in the wake of a meteor to such an extent that it can be approximated as a metallic reflecting surface that expands and spreads out over time, causing the electron line density to decrease to the point where the underdense approximation is true. This is also discussed in Maruyama et al. [20]. The article states that in their campaign, the ionosondes that they use can only detect events with lifetimes of more than 15 s, which are considered overdense traces [21]. Kozlovsky et al. [22] give a more tangible definition. A meteor echo is underdense if the electron line density is below 2.4 × 10¹⁴ el/m. Above this quantity, it is an overdense meteor echo, and close to it is a transitional echo.

It is worth noting that polarization effects can also occur. For traces with a dielectric constant close to −1, a plasma resonance phenomenon can occur if the polarization of the incident electric field is such that it is perpendicular to the axis of the trail. In this case, the reflection coefficient can double or the reflected energy can quadruple [16]. The magnetic field may also play a role. Based on previous observations [23], if the meteor’s trail and the magnetic field lines align, this can stabilize the trail and increase its lifetime. Oppenheim and Dimant [24] found in 3D simulations that if the difference in the field alignment is 6^o or below, it can significantly enhance the lifetime of the meteoric trails.

The initial mass and the velocity of the meteor strongly influence the observable characteristics of meteor trails. For a given mass, the higher the velocity, the higher the altitude of the meteor trails. And at a given speed, the higher the mass, the lower the altitude of the trails [16]. Theories indicate that the length distribution of the meteor trails is independent of the electron line density that is designated as the criterion for terminating the trail and is also independent of the sensitivity of the radar receiver and the power output of the radar transmitter [25].

Leonid meteors have an average speed of 71 km/s (~40.7 degree/s for 100 km height) when entering the Earth’s atmosphere [26], and their average size is 10 mm [27]. The chemical composition is mainly Mg, Fe, Ca and Na, although it is perhaps worth noting that the Na content of the smaller meteors is ~30% lower [28,29] which is the same for the Perseids. However, Leonid meteors easily disintegrate in the atmosphere and the Na in them evaporate faster because of that, unlike in the Perseids [28].

Geminid meteors have an average speed of 35 km/s (~20.1 degree/s for 100 km height) when arriving in the atmosphere [30], and their average size is 1 mm [31]. The chemical composition is mainly Mg, Ca, Fe, Na and Ni [32,33,34]. It is worth noting that the Ni abundance is higher than in the other meteoroid showers, while the Na abundance is lower [34].

Fragmentation of the incoming meteor leads to the dispersion of smaller pieces over a larger area than the parent body [19]. Statistical analysis shows that the percentage of fragmentation [35] is less pronounced in the asteroid-originated Geminids than in the comet-originated Leonids [36].

Optical observations [37] and meteor radars [38,39] are the most often used meteor detecting methods, but during major meteor falls, meteor echo traces were often observed on ionograms, which were classified into separate categories [40]. However, this classification led to controversy, which was finally resolved by Maruyama et al. [20].

Maruyama et al. [20] analyzed data from a campaign session made during the Leonids of 2001, which was a peak number producing event [41,42]—three 10C-type ionosondes and one DPS4 Digisonde took campaign measurements over Japan from 03:00 (UT, 12:00 JST) on 17 November 2001 to 09:00 (UT, 18:00 JST) on 21 November 2001. The ionosonde stations were at Wakkanai (45.39°N, 141.69°E), Kokubunji/Tokyo (35.71°N, 139.49°E) and Yamagawa (31.20°N, 130.62°E) and they operated with a 1 ionogram/minute setting. At Ogimi/Okinawa (26.68°N, 128.16°E) the Digisonde was taking 11 ionogram/every 15 min interval (the number of its findings was multiplied by 15/11 for convenience). The frequency sweep to create the ionograms (see Section 2.3) went from 1 MHz to 30 MHz. The automatic gain control (AGC) was turned off; also no post processing or noise reductions were used on the data. Between 16:00 UT and 24:00 UT on 18 November, a significant number of meteor-induced echoes were observed, which corresponded to the predicted peak period of the Leonid meteor shower of 2001. Maruyama et al. [20] concluded that these meteor-induced echoes were backscattering (Fresnel scattering) from the region of increased electron line density along the meteor’s path. Since the measurements were made using ionosondes, because of the settings and the capabilities of the instruments, only traces longer than 15 s were recorded according to the article, and these were categorized as overdense echoes [21].

At Sodankylä Geophysical Observatory (67.37°N, 26.63°E), in a year of measurements of their frequency-modulated continuous-wave chirp sounder, 28 overdense cases around 90 km in height with 1 min time resolution ionograms were selected, which could be combined with optical measurements and a colocated all-sky interferometric meteor radar. Most of the events originated from the Geminids of 2015. The article itself [22] proves that their proprietary ionosonde can also detect the ionization caused by meteors. In addition, it was found in the analysis of overdense cases that the decay time of the meteor’s trail depends on the initial electron line density: a less dense trail decays slower, while a denser trail decays faster. They suggest that this might be the result of two competing mechanisms: the presence of meteoric dust may decrease the rate of diffusion and recombination, which can lead to an increased rate of decay for the denser trails.

The DPS-4D Digisonde is an advanced type of ionosonde, with added features and enhanced sounding ability [43] (see Section 2.3). In case studies [44,45], it was proven that these types of ionosondes can also detect individual meteor-induced traces, but the exact mapping of what these types of devices can do has not yet been conducted so far. The aim of this study is to explore the potential of meteor observation with the DPS-4D Digisonde, the technical details to look out for and the features that can be useful.

Following the Introduction, the data collection and processing methods—the camera and processing software used for the optical images, Metrec (Section 2.1), the data produced by the processing (Section 2.2) and the results from the processing of the Digisonde measurements (Section 2.3), the ionogram and the Skymap data (Section 2.4.1, Section 2.4.2 and Section 2.4.3)—will be presented. After the data summary, a detailed analysis of the measurements will be presented—what was found (Section 3.1.1), what was not found (Section 3.1.2) and the other features that were observed (Section 3.2.1, Section 3.2.2 and Section 3.2.3). The observations are followed by a discussion (Section 4) and finally conclusions (Section 5) are drawn.

2. Methods and Data

2.1. Meteor Camera and Optical Processing

Optical data were acquired using a Watec 902H2 Ultimate camera. It was equipped with a Computar HG2610AFCSHSP objective (2.6 mm focal length, 30 mm effective lens aperture and toward the zenith 122° × 97° field of view). This temporary station at Széchenyi István Geophysical Observatory (47.632°N, 16.718°E) was operated using the Metrec automatic meteor detection software [46]. In this work, the lower limit in brightness is roughly +1 magnitude. According to the software description, the magnitude estimation’s accuracy is ±0.5 magnitude. The process also includes position accuracy estimation.

The Metrec software determines the right ascension (degree) and the hour angle (h) in the equatorial coordinate system II using a reference file of bright stationary stars (281 references were used for this processing). Furthermore, it identifies the parental meteor shower based on the meteor’s orbit, calculates the velocity (degrees/s) and the duration of the event, and gives a rough estimate of the length of the meteor’s light trail based on the number of oversaturated pixels and the direction (degree).

It should be noted that this software is not suitable for high-precision orbit determination. However, it can tell where the meteor was in relation to the Digisonde and whether the instrument had any chance of detecting the object at all. So, for this study, it was decided to be precise enough.

2.2. Optical Data

During the campaign measurements (Leonid campaign: 16–19 November 2019 and Geminid campaign: 10–16 December 2019), most of the nights were overcast at Sopron, and thus, only the pictures from the night of 18 November and dawn of 19 November 2019 from the Leonid campaign, part of 13 December’s night and the night of 14 December—dawn of 15 December 2019 from the Geminid campaign could be used (

Table 1. The summary of the successful optical detections at Sopron for the campaign sessions.

Campaign	Number of Optical Detections
Leonid campaign of 2019 Night: 2019-11-18. (from: 17:05 UT) Dawn: 2019-11-19. (until 3:52 UT)	LEO: 3 NTA: 0 STA: 1 AMO: 0 SPO: 5
Geminid campaign of 2019 Nights: 2019-12-13. (22:40–23:22 UT)      2019-12-14. (from: 17:21 UT) Dawn: 2019-12-15 (until 4:48 UT)	GEM: 21 COM: 5 HYD: 4 MON: 6 ANT: 6 SPO: 37

).

The Metrec software took all occurring meteor showers into consideration when processing the picture. During the Leonid campaign, there were four active meteor showers: the Leonids (LEO), the Southern- and Northern-Taurids (STA, NTA) and the α-Monocerotids (AMO). During the Geminid campaign, there were five: the Geminids (GEM), the Coma-Berenicids (COM), the σ-Hydrids (HYD), the Monocerotids (MON) and the Antihelion Source (ANT).

The Metrec software detected 9 meteors during the Leonid campaign and 79 meteors during the Geminid campaign. According to the identification of the software, from the 9 meteors of the Leonid campaign, only 3 were from the Leonid meteor shower. One was from the Southern-Taurids, while the rest were detected as sporadic meteors (SPO). From the 79 meteors of the Geminid campaign, 21 meteors were from the Geminids, 5 from the Coma-Berenicids, 4 from the sigma-Hydrids, 6 from the Monocerotids and 6 from the Antihelion Source, and 37 were identified as sporadic meteors.

There was also an extra optical detection, thanks to the European Fireball Network. On 17 November 2019, at 4:15 a.m. (UT), a bright Leonid fireball passed not far from Průhonice’s Digisonde, and its signal was recorded on the ionograms and on the Skymaps. A separate case study was conducted of this [45]. Since the fireball is a separate phenomenon, although it is included in the tables and descriptions, it is omitted from the analyses and is not shown in the histograms.

The software determines the duration of the event, the parental meteor shower (given the time frame, it also lists the possible showers), the brightness (accuracy: ±0.5 magnitude), the right ascension and declination (equatorial coordinate system II) of the beginning and the end point, the number of pixels saturated by the meteor, the direction of the meteor, the velocity (degree/s), the position accuracy and the signal-to-noise ratio. From the right ascension and declination, the azimuth and altitude coordinates were also calculated in the horizontal coordinate system.

2.3. Digisonde Technique

As a consequence of Snell’s law, the electromagnetic wave that enters the environment with the changing electron concentration will refract or reflect. All electromagnetic waves with a frequency smaller than the plasma frequency entering the ionosphere at vertical incidence will be reflected back to the Earth (Equation (1)) [47]. The highest frequency for which the electromagnetic wave will return is the critical frequency of the layer.

The equation of the plasma frequency is:

$$f_p={\displaystyle \frac{{\omega }_{pe}}{2\pi }}{, \omega }_{pe}=\sqrt{{\displaystyle \frac{n_e{e}^2}{m^{*}{\epsilon }_0}}}$$

(1)

where f_p is the electron’s plasma frequency (Hz), ⍵_pe is the electron’s plasma angular frequency (rad/s), n_e is the electron density (number of electrons/m³), e is the electron’s charge (1.609 × 10⁻¹⁹ C), m* is the effective mass of the electron (9.11 × 10⁻³¹ kg) and ε₀ is the vacuum permittivity (8.85 × 10⁻¹² F/m).

When the sounding signal’s frequency reaches the plasma frequency, the stratification, electron content and virtual height of the different ionospheric layers can be derived from the measurements using inversion models. Typically, ionosondes use frequencies between 1 and 20 MHz, which makes the observation of the E and F layers possible. The Digisonde makes a pulse transmission with a peak pulse power of 300 W. Because of the pulsed nature of the signals, the average power will be lower. It should be noted that the precise power transmitted in a given direction is difficult to determine because of the complicated antenna pattern [48]. (The transmitter antenna has different gains for different elevation angles, as well as for different azimuths.)

The D region is undetectable for such frequencies due to its plasma frequency usually being below the minimum used sounding frequency. The upper sounding limit can be adjusted (for instance for DPS instruments) through the year according to the expected maximum value of the observed critical frequencies. The advantage of the sounding program adjustment could be the shortening of the sounding time.

The result of the measurements taken by an ionosonde—the virtual height frequency values—are called ionograms. The delays caused by the local atmospheric conditions are not taken into consideration in the making of the ionograms, and the conditions of a wave propagating in the vacuum are assumed at first. The difference in the delay in the time of flight is small for the E layer, but for the F layer, this difference is significant, and inversion is necessary for the layer’s true height.

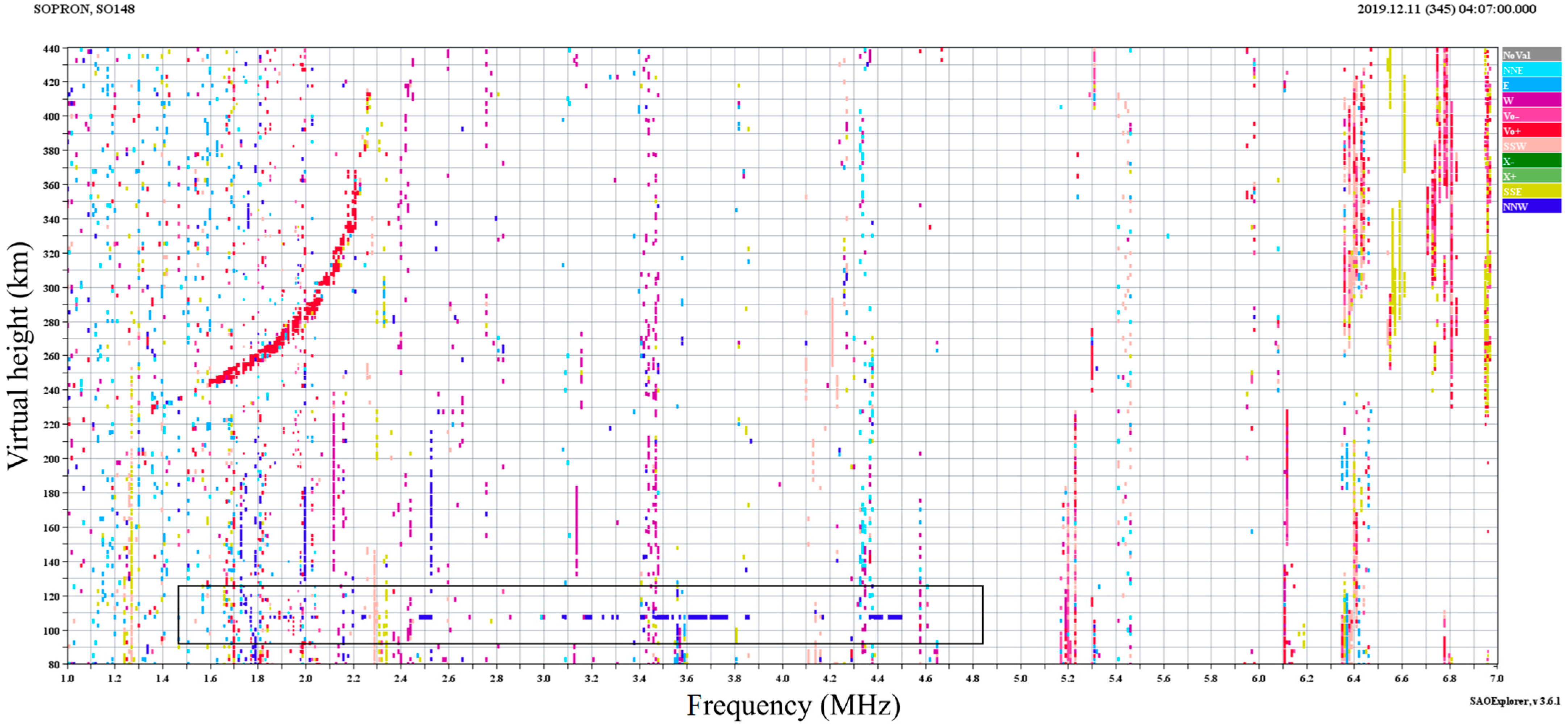
Deriving the real height from the virtual height is not necessarily trivial. At an 80 km altitude, the difference between the virtual and real height in the vertical direction is relatively small (~2–3 km), less than the vertical resolution of the Digisonde (~5 km). However, in the case of the reflections from the oblique direction (Figure 1a), both the virtual height and the critical frequency suffer a distortion and will appear at higher values compared to the values in the vertical direction. The difference between the virtual and real height derived from the oblique direction can be in the order of ~10 km, even at the height of 80 km.

At Průhonice (50.00°N, 14.60°E, station code: PQ052, geomagnetic coordinates for 2019: 49.33°N, 98.35°E) and at Sopron (47.63°N, 16.72°E, station code: SO148, geomagnetic coordinates for 2019: 46.70°N, 99.56°E), a Digisonde DPS-4D (hereinafter Digisonde) type of ionosonde station was installed. It has been providing measurements since January 2004 in Průhonice and since June 2018 in Sopron [49] as a part of the GIRO Network [50]. 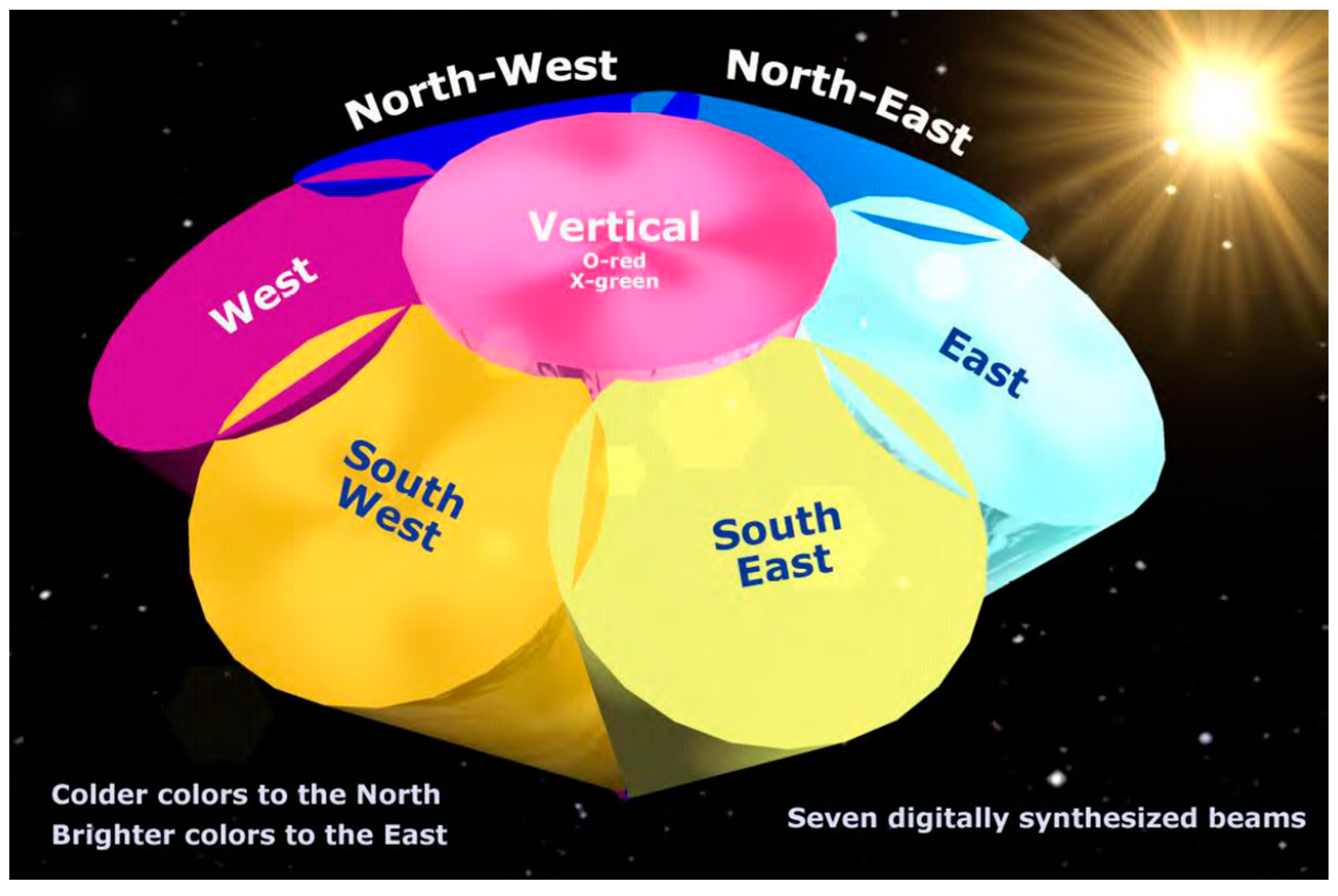Through the addition of four receiver antennas surrounding the central transmitter [43,51,52], the Digisonde is capable of not just the vertical sounding, but also the oblique sounding. This kind of arrangement makes the determination of a reflection’s direction possible, based on the phase difference of the reflected signal as observed by the four receiver antennas. On the ionograms, these are color-coded: warm colors for southern and western directions, and cold colors for northern and eastern directions (Figure 1a).

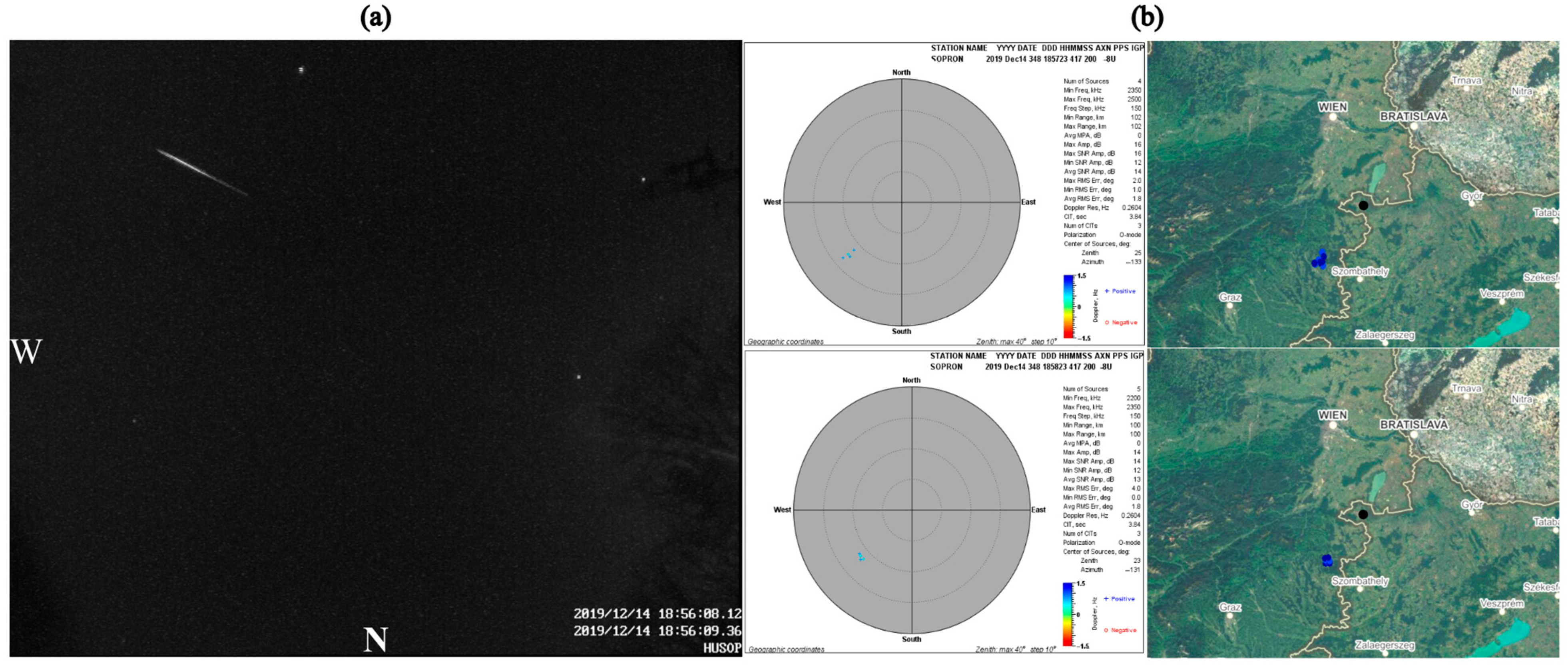
The regular ionograms are automatically scaled. This usually provides the necessary parameters (e.g., critical frequencies of the E (foE) and F (foF) layers) for the setup of the drift measurements. If there are movements in the plasma, the Doppler shift effect will be registered for the reflected sounding waves. Digisondes can estimate the speed and direction of plasma motions, by emitting signals of the same frequencies, and measuring the Doppler shift and the direction (made possible by the receiver antenna arrangements) of the reflected signals. The final product of the measurements is the so-called Skymap. The Skymap displays the measurements of the three velocity vector components, namely vertical (v_z), north (v_n) and east (v_e) directions and these are represented graphically in color-coded east–west and north–south planes (Figure 1b).

There were synchronized campaign measurements at both stations for the Leonids and Geminids in 2018 and 2019. Synchronization was important because the two stations are very close to each other in ionospheric terms, so local and regional effects could be easily separated by simultaneous measurements (Supplementary Figure S1). The campaigns of 2018 were used as a test. Further, the results were used in the settings for the campaigns of 2019 (

Table 2. The campaign settings and measurement times of 2019 at the different stations.

Campaign	Leonid Campaign		Geminid Campaign
2019 (UT)	Sopron	Průhonice	Sopron	Průhonice
Digisonde 2 ionogram/minute campaign dates: night-dawn from-till	11.16–11.17 16:30–6:30	11.16–11.17 16:30–6:30	12.10–12.11 19:40–20:00 21:00–06:00	-
			12.11–12.12 16:30–05:30	-
	11.17–11.18 16:30–6:30	11.17–11.18 16:30–6:30	12.12–12.13 17:30–05:30	-
			12.13–12.14 16:30–05:30	12.13–12.14 16:00–08:00
	11.18–11.19 16:30–6:30	–	12.14–12.15 16:30–05:30	12.14 18:00–20:00 12.15 0:00–03:00
			12.15–12.16 16:30–05:30	-
Skymap	1/minute	1/minute (until 6:30 17 November)	1/minute	-
Optical data	11.18–11.19 17:05–3:52 9 detections	–	12.13 22:40–23:22 3 detections	-
			12.14–12.15 17:21–4:48 76 detections

).

In all the cases, the campaigns started after sunset (roughly around 16:30 UT) and ended at sunrise (roughly around 6:30 UT). In 2018, the stations measured one ionogram/minute, but this setting was upgraded for the campaign of 2019 to two ionograms/minute. The drift measurements were not made in high time resolution in the 2018 campaign, but for the Leonids and Geminids of 2019, there was one Skymap/minute in Sopron (in Průhonice only during the Leonid campaign).

Meteor-induced traces were manually searched on the ionograms based on the following criteria: (1) The virtual height is between 80 km and ~160 km. (2) The reflections are well identified and clustered in a line. (3) They show no similarity to the regular sporadic E layer based on their lifetime and evolution. (4) In questionable situations, synchronized measurements from the other station were used to check the ionogram of the other station (Supplementary Figure S1), which effectively filtered out possible false hits caused by regular sporadic E.

Identifying the reflections from the meteor trails on Skymaps was more demanding, as usually 1–5 reflections/meteor were found, and not always. The height and direction had to match those seen on the ionograms or, where appropriate, optical sightings. Most nights, the Skymaps were empty, which simplified the identifications. However, there were also cases where other plasma flows were present, and reflections were observed in the right direction and altitude range. These were recorded as possible hits.

2.4. Digisonde Records

2.4.1. Background Sporadic E Activity

On ionograms, the faint meteor-induced traces and regular sporadic E layer can occur in the same height range, at altitudes of ~80–130 km. Although sporadic E is essentially considered a summer phenomenon, it can in fact occur at any time [11]. During the campaigns, there were also several occasions when the background sporadic E activity made it difficult to identify the meteoric traces.

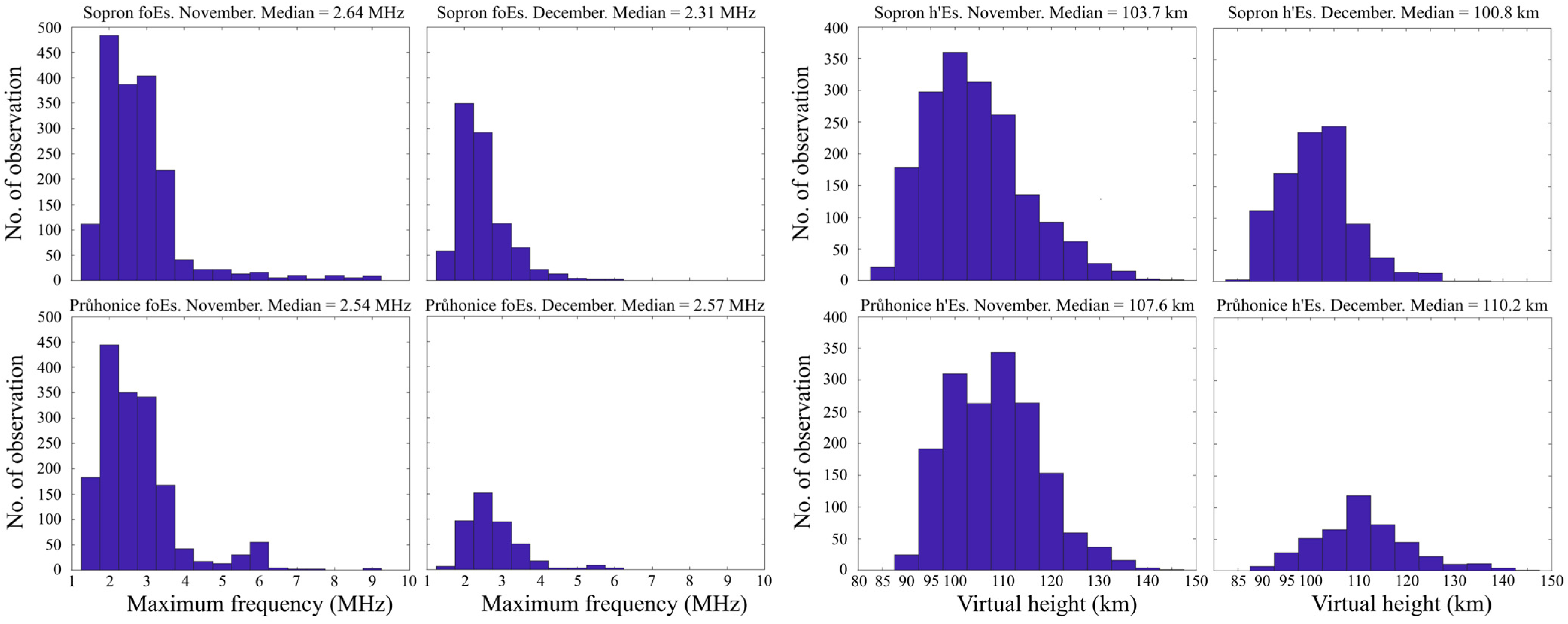
To make it easier to dissect the phenomena, for November and December 2019, the ionograms for the month were manually scaled, except for the days of the campaign measurements. In Průhonice, the ionograms were obtained every 5 min during November and every 15 min during December of 2019, while in Sopron, they were taken every 5 min in both months. A total of 10,406 ionograms were processed manually for Průhonice and 14,069 for Sopron (Figure 2, Supplementary Figure S2, ref. [53]). Of the processed ionograms, the phenomenon occurred in 20.2% over Průhonice and 19.1% over Sopron.

In Sopron, 65.61% of the detected sporadic E occurred in November and 34.39% in December. In Průhonice, 79.07% of the detected sporadic E occurred in November and 20.93% in December.

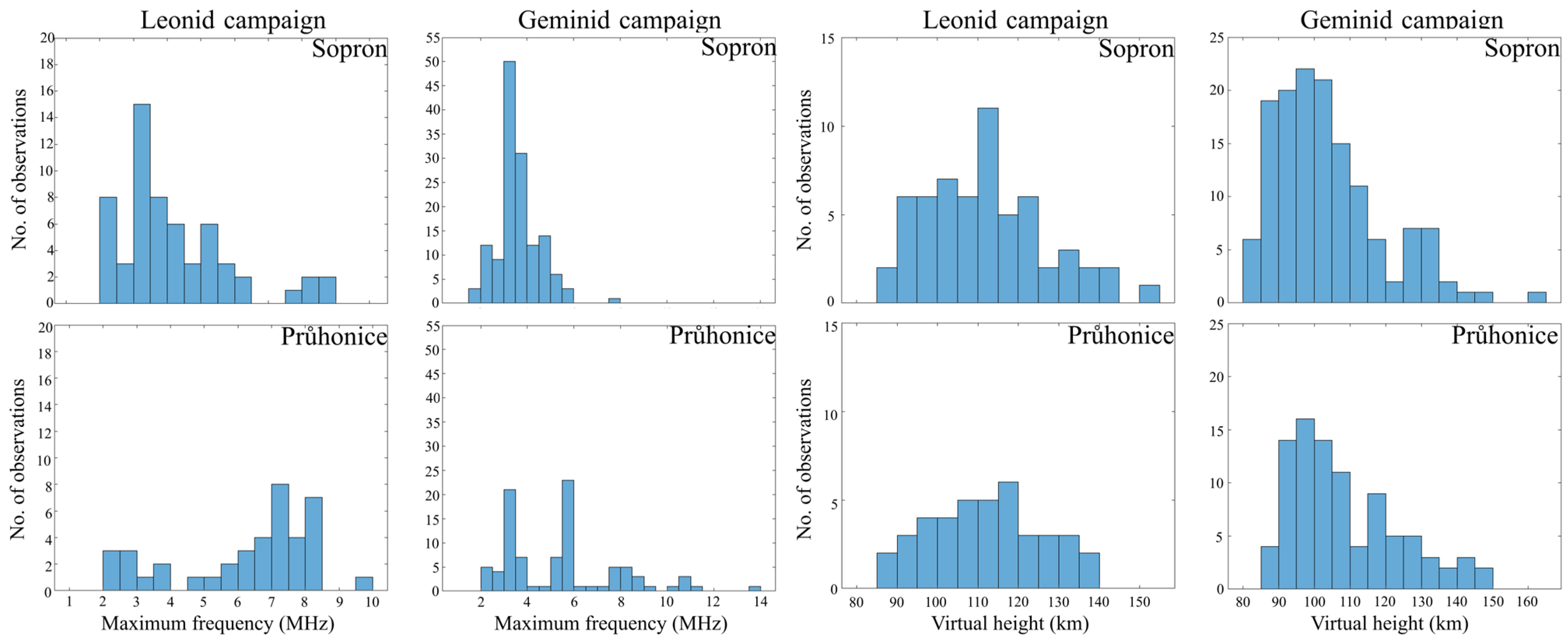
The distributions of foEs over the two stations and for the two months are very similar. The main difference is that at Průhonice, a second peak in foEs occurs at higher frequencies in both months, which is not present in Sopron’s distributions. It is also observed that while the median for Průhonice barely changes for the two months, Sopron shows a decrease of 0.3 MHz for December.

The distributions of virtual height (h’Es) for November are also very similar for the two stations, but a ~5 km shift in the values can be observed, which is within the vertical resolution of the Digisonde. Whether this is because of an instrumental setting or a physical phenomenon is still under investigation. However, the distributions of December show a significant divergence for the stations. In the case of Průhonice, the result is a flat, sloping curve, while in Sopron, a sharp rise is followed by a sharp fall, and the distribution has a significantly higher skew and kurtosis. The medians show a difference of 10 km which is significant for two stations in such close proximity.

2.4.2. Ionograms

The ionograms (see Section 2.3) for the 2 ionogram/minute campaign were manually reviewed for both stations. The campaign settings were not quite the same at the two stations—there were a lower number of performed high cadence measurements in Průhonice for various reasons (Table 2). For the times when there were no available 2 ionograms/minute measurements in Průhonice, the regular ionograms (taken every 5 or 15 min) were checked.

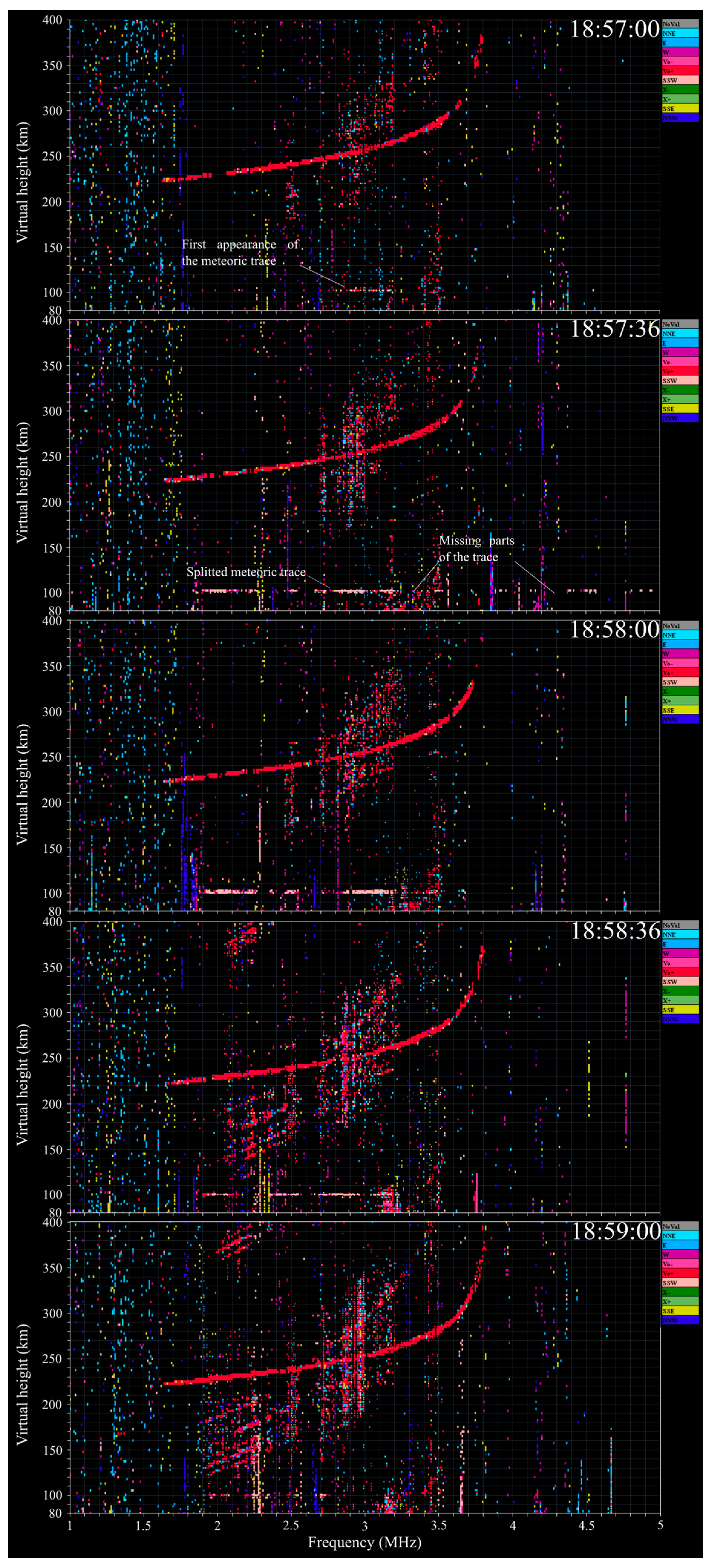
When the distinctive, meteor-induced traces appeared on the ionograms (Figure 3), the time of the first and last sighting of the trace was recorded. From these, the lifetime of the trace can be calculated. In rare cases, the trace disappeared for one ionogram, but reappeared on the following ionogram. Such picture jumps were flagged. The maximum and minimum frequencies reached during the trace lifetime were also recorded. The virtual height was read from the ionogram of the first appearance (vertical resolution ~5 km). The direction of reflections is also indicated [53].

According to the manuals [48], ionograms should be scaled at the 6 dB most probable amplitude (MPA) threshold noise reduction (see Supplementary Figure S3). But at the 6 dB MPA, the meteor-induced traces can be jagged, faint and often not even fully visible, probably due to the signal processing techniques. Therefore, the ionograms were reviewed at 4 dB, and the data were read at this threshold. A value of 2 dB would have been better, but due to the noise, most of the recorded ionograms could not be manually analyzed at that MPA threshold. The traces have been classified according to their appearance on different MPAs [53].

Two phenomena were commented on next to the ionograms. Sometimes, a meteor-induced trace split in two on the ionogram, and a distance of at least 5 km appears between the now split traces. This is recorded as a split in the trace. The other comment was added when the meteor-induced trace overlapped with a regular sporadic E, making the finding somewhat unreliable [53].

The detected meteor-induced traces in the ionograms and the resulting Skymaps are summarized in

Table 3. The detected meteor-induced traces on the ionograms and linked to them, the Skymap findings. The numbers in brackets are the amounts found on the regular ionograms for the ionograms when Průhonice did not have 2 ionogram/minute measurements. For the Skymaps, the bracketed, question marked numbers are the possible findings—there were reflections in the right direction and height on the Skymaps, but they overlapped with other plasma flows, making the detections uncertain [53].

Detection	Sopron	Průhonice
Leonid campaign on ionogram	59	41 (+4)
Leonid campaign on Skymap	12	6 (+4?)
Geminid campaign on ionogram	141	91 (+40)
Geminid campaign on Skymap	22 (+4?)	-

. During the Leonid campaign, at the 4 dB MPA threshold, Sopron registered 59 cases which could be identified as a meteor-induced trace, while Průhonice registered 41 with 4 additional findings in the regular ionograms (Table 3). The Geminid campaign yielded 141 meteor-induced traces for Sopron and 91 for Průhonice with 40 additional findings in regular ionograms (Table 3).

2.4.3. Skymap

The drift measurements (see Section 2.3) were performed at 2.5 MHz. The Sopron station took one Skymap/minute during both campaigns, while Průhonice station recorded them only during the Leonid campaign, and even then only until 06:30 of 17 November 2019.

Since this method is focused on plasma movements, only 1 Skymap was taken every minute, and the measurement was carried out at a specific frequency, so it is not surprising that few meteoric reflections were captured. However, regarding the fact that a fireball that lasted for 20 min while slowly dissipating and the Skymaps showed only 2–5 reflections [45], the number of successful detections with drift measurements is surprisingly high.

In the case of the Leonid campaign, for 59 identified cases on the ionograms, there were 12 events on the Skymaps at Sopron (see Table 3). When Průhonice was taking the drift measurements, for the 41 findings on the ionograms, there were 6 episodes on the Skymaps (see Table 3), followed by 4 other possible detections (the reflections’ direction and height range supports the assumption that they could have been from meteoric trails, but they were overlapping with other plasma flows).

In the case of the Geminid campaign (only Sopron took drift measurements) for the 141 findings on the ionograms, there were 22 identified records on the Skymaps with 4 additional possibilities or suspicious detections (see Table 3).

3. Results and Observations

3.1. Results of the Comparison between the Different Data

3.1.1. Optically Detected Meteors Present in the Digisonde Data

Of the 88 optically detected meteors, 31 were found by the Digisonde measurements [53]. In addition, another case has been identified as a fireball event [45] by the European Fireball Network [54].

During the Leonid campaign, there was one fireball detection near Průhonice and nine optical meteor records in Sopron. The fireball and eight from nine optical detections could be linked to the meteor-induced traces found on the ionograms. The effect of the fireball and one meteor could be found on the Skymaps, too. The meteors detected optically and also on the ionograms were as follows: one Southern-Taurid, four sporadic, three Leonid. The fireball was identified as a Leonid, too (see Supplementary Table S1).

During the Geminid campaign, from 79 optical detections 23 were found on the ionogram: 21 of them in Sopron’s measurements, 2 of them in Průhonice’s. Four of the detections could be linked to reflections on the Skymaps, too (Figure 4 and Figure 5). The meteors detected via the two different methods were as follows: one Antihelion Source, one σ-Hydrid, one Monocerotid, seven Geminid and thirteen sporadic [53]. Around the optical detections, the lowest noise reduction settings were checked too, and some of these findings were acquired at the 2 dB MPA threshold, though it should be noted that most of our manual review was with the 4 dB MPA threshold setting (see Section 2.4.2 and Supplementary Table S1).

3.1.2. Optically Detected Meteors Not Present in the Digisonde Data

In total, 57 of optical detections could not be linked with ionogram records [53]. It should be noted that the optical camera’s field of view was wider (122° × 97° field of view) and covered a larger area than the Digisonde can cover (field of view is ~45° × 45°) [48]. Particularly during the Geminids, it was common for sightings to occur at the very edge of the optical recordings, which was beyond the range of the Digisonde.

Of the 57 undetected cases, there were only six cases where Sopron’s Digisonde should have detected the meteor, but did not. Also, in nine other cases, there is a slight probability that Průhonice could have detected the meteor, but regular measurements were being taken at the time (one ionogram/15 or 5 min). In the other cases, there are circumstances that clarify or explain why no trace did appear on the ionograms (see Supplementary Table S2).

Such possible explanations are as follows: (1) the meteor was out of the range covered by the Digisonde, (2) it was at such a low altitude angle (horizontal coordinate system), that it was not detectable by the Digisonde, (3) regular sporadic E could cover up the traces, (4) the signal processing could filter out the faint traces (the received signals with an amplitude below the estimated MPA were not detected because of the Digisonde settings at Sopron, detailed in Section 2.4.2; also see Figure 5), and (5) additional circumstances for not detecting meteors with the Digisonde can be that its plasma trail was below 80 km in height (see Supplementary Table S2).

Also, there were cases where there were signals in the noise that seemed to be a good candidate (some points in line at the same height) but could not be clearly categorized as a meteor-induced trace (see Supplementary Table S2).

3.2. Observations on the Digisonde Data Regarding Meteors

3.2.1. Necessary Conditions for the Successful Detection of Meteors Using the Digisonde

The transmission antennas used for the ionosonde are optimized for vertical transmissions, but significant power is emitted even at zenith angles close to 90°. Thus, meteor traces close to the horizon are still illuminated by the ionosonde’s transmissions. However, the angle of arrival detection of received echoes used here works by applying digital beamforming with seven beams, namely one vertical and six at different azimuths and centered around a zenith angle of 30°, as shown in Figure 6 [48]. Thus, reflections with zenith angles much larger than 45° are harder to detect. All the detected meteors at Sopron had at least an ~40° altitude angle, which supports our original assumptions. This is also consistent with what was observed by Sodankylä [22].

The acquired raw data were subjected to a noise filtering method—this is the setting of the most probable amplitude (MPA) threshold (see Supplementary Figure S3). This method filters out reflections based on the estimation of how likely it is that the reflection is a real one (Figure 3 and Figure 5). At the 6 dB MPA threshold—the standard for manual scaling—only a fraction of the findings were present. For this reason, for the manual review of the data, the threshold was set at 4dB MPA. The setting of 2 dB MPA would have probably yielded even more, but that was too noisy to work with, while 2 dB data were checked in certain cases (around the time of optically detected meteors), but other than that, it was not reviewed methodically.

The presence of a regular sporadic E layer can also prevent the detection of meteors. For example, in Sopron station’s observation on 13 December 2019 (one day before the peak of the Geminid meteor shower) between 1:42:00 and 2:52:36 (UT), there were no detected meteor-induced traces on the ionograms, while a strong regular sporadic E layer was present.

3.2.2. Observations of the Digisonde Detections Regarding Meteors

In the case of the detected fireball [45], it was discussed that a huge part of the trace (between 5 and 14 MHz) consisted of W and SSW reflections, when physically the fireball was located in the E and NNE directions relative to the Digisonde of Průhonice. The explanation lies in the effect of the geometrical parameters of the antenna system that can cause 180° phase jumps. In light of this, it was surprising that there were only two cases where part of the signal suffered a phase jump. The direction determination of the Digisonde proved to be almost entirely reliable.

What also proved surprising was the delay in the appearance of the traces compared to the optical observations. This time delay ranged from 12 to 132 s. It is also worth noting that during the nine matches of the Leonid campaign (including the fireball’s case), these delay times were mostly in the explainable range, with a maximum of 62 s. However, for matches under the Geminid campaign, these numbers tended to be higher.

It should be noted that the virtual heights of the Leonids found by both measurement methods were surprisingly high: the values ranged from 113 to 137 km, with one exception (which was ~100 km high). In contrast, the Geminids were generally found at lower heights (~80–115 km) on the ionograms.

The number of detections may also depend largely on the specifications of the instruments. Although both stations use the DPS-4D type of Digisonde, Průhonice detected significantly more meteor-induced traces during the same time periods. For example, during the period 16:30–24:00 on 13 December 2019, Průhonice recorded 27 meteor trails, while Sopron recorded 12. Also, “high-frequency” traces (>5 MHz), which were very rare in Sopron, were often detected in Průhonice’s measurements (Figure 7, ref. [55]).

3.2.3. Other Observations

Compared to the optical observations, there was much more trace detection on the ionograms (altogether 376 [53], Table 3) taking into account the whole periods of the two meteor showers. The distribution of the maximum frequency was remarkably different for the stations. The distribution of the meteor-induced traces’ virtual heights was different during the two campaign sessions (but similar for the two stations at one given campaign). It was also distinct from the average behavior of sporadic E (Figure 8). Although the shape of the height distribution during the Leonid campaign was reminiscent of the regular ones, the height distribution of the Geminid campaign with their double peaks was reminiscent of the height distributions of the long-enduring echoes of McKinley (Figures 5–13) [19].

It should be noted that for the reflections from the oblique direction, both the height and the frequency are distorted, and higher values are obtained than what would be the case in vertical reflections. This can reach the values of ~10 km at height (Figure 9).

The most surprising finding was that the maximum frequency reached by the meteor-induced traces and the brightness showed anticorrelation—the brighter a meteor was, the lower the maximum frequencies that it reached. The original expectations were the opposite. (Technically, the correlation coefficient is positive—see Figure 10a—but in the case of brightness, the lower the number, the brighter the meteor is. For example, a +1 magnitude meteor is less bright than a −0.5 magnitude meteor. So, in this case, the positive correlation meant the dimmer the meteor, the higher the frequencies that its trace reaches.) It should be noted that this cannot be considered statistically conclusive on the basis of 31 cases (25 if only the 4 dB MPA cases are taken into account; see Figure 10c,d). In contrast, velocity (degree/s) was slightly correlated with the maximum frequency (Figure 10b)—the faster a meteor, the higher the maximum frequency that it tended to reach (Figure 10b,d). This correlation is far better pronounced when the data points acquired at the 2 dB MPA threshold are discarded and the data points used were all acquired at the 4 dB MPA threshold. In that case, the correlation is significant.

The meteor trail length does not show any correlation with any of the other measured parameters.

The lifetime of the trace does not seem to depend on the calculated or observed physical properties of the meteors (duration of the event, brightness, etc.). However, in the case where the trace split in two—presumably due to the wind shear caused by mesospheric winds (see Supplementary Figure S4)—the duration is generally longer.

4. Discussion

Using Sopron’s Digisonde, 29 optically detected meteors were found in the measurements. At Průhonice station, two of these meteors and one fireball were found (confirmed by the European Fireball Network). Of the remaining 57 optical detections, the meteors with too large zenith angles were not detected by the Digisonde. This is to be expected due to the applied method for the angle of arrival detection (as described in Section 3.2.1) and the fact that these signals would need to travel a long distance through the D-region of the ionosphere and can therefore be expected to experience more attenuation. Of these 57 optically detected meteors, there were a total of six meteors that were not detected by Sopron’s or Průhonice’s Digisonde, and no comprehensive explanation can be found to fully clarify the absence of echoes in the ionograms. One of the most probable explanations can be the fact that these meteors have not formed a stable ionization layer existing for a sufficient time so that the Digisonde could detect it. It is also possible that it did form, but the mesospheric winds and/or turbulence ripped it apart or “blew it out” of the Digisonde’s range. It is also possible that the meteor had its ionization effect below 80 km, which is below the detection range of the Digisonde.

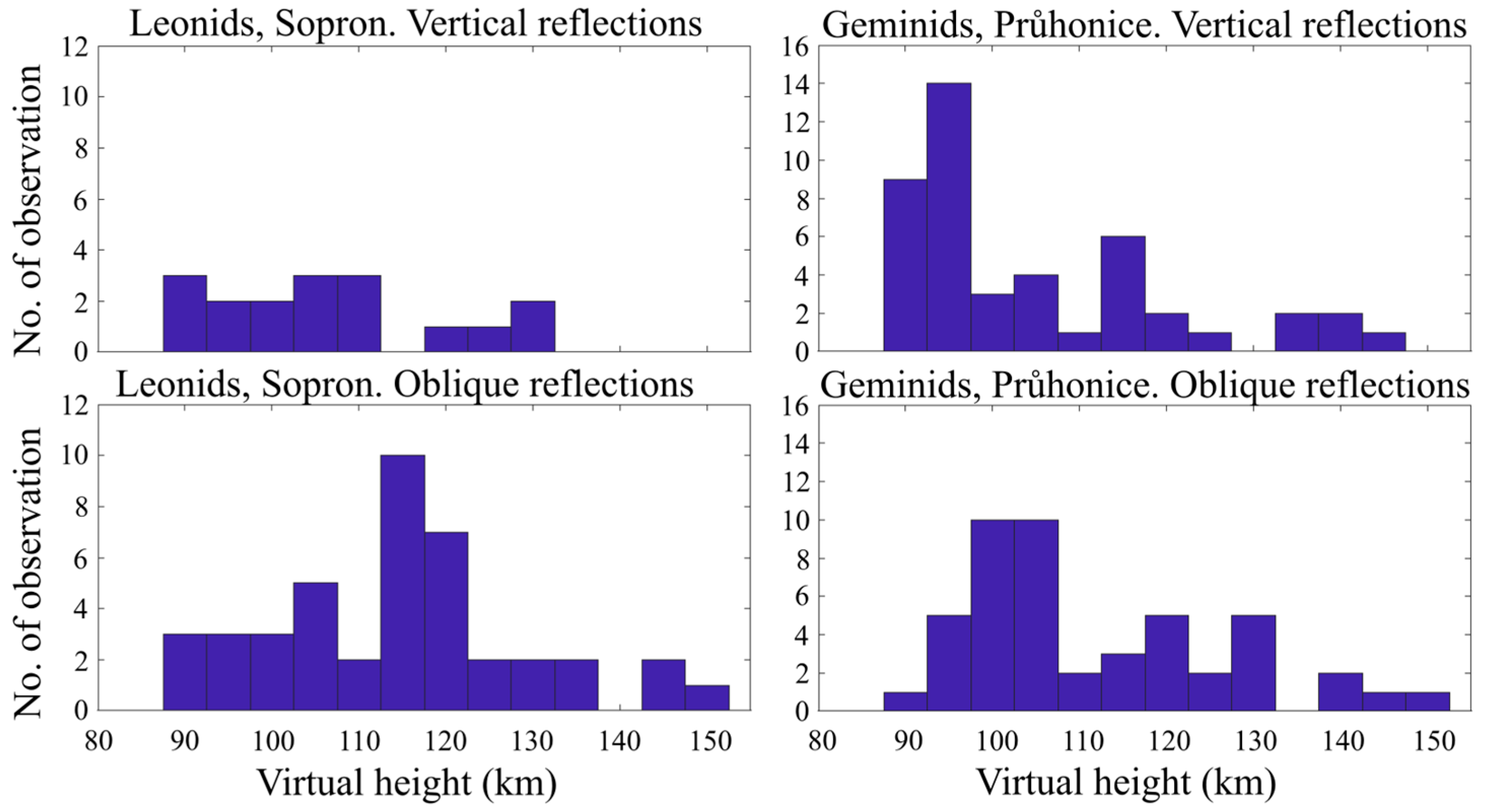
During the presence of regular sporadic E layers, for a couple of hours, no meteor-induced traces were recorded. Presumably, this is not because there were no incoming meteors—as it can be seen in the ionogram observations comparing the two stations—but because strong, stable and prominent reflections from the regular sporadic E layers dominated the ionograms in the same height region where meteor-induced traces are most likely to occur which prevents the identification of meteor-induced reflections. The traces that do appear tend to reach a higher maximum frequency than the regular sporadic E, or they are well separated from it in height, and usually come from an oblique direction, very rarely from a vertical direction (±15° from zenith).

During the Leonid campaign, Sopron’s Digisonde detected 59 and Průhonice detected 45 events, respectively, and during the Geminid campaign, Sopron detected 141 and Průhonice detected 131 events, respectively. A contributing factor to these numbers is that the 2019 Leonids produced anomalously few meteors [56]. The mass of bright meteors (−2.5–+2.5 visual magnitude) ranged from ~1 g to ~10⁻² g on average [16]. There are also smaller meteors, but these can only be detected via intensified video or meteor radar [57]. If these extra traces found on the ionograms are attributed to small meteors (below ~10⁻² g)—not detected via the +1 magnitude lower limit camera—then their abundance and ionization (which in most cases produced a more distinct trace and higher frequencies than those also detected optically) can be understood and explained.

The height distribution of the meteor-induced traces in the ionograms are different for the two campaign sessions, but quite similar for the two stations in a given campaign. This could be attributed to the fact that the higher a meteor’s velocity, the higher the ionization effect appears [16,19,58]. The Leonids enter the Earth’s atmosphere at 71 km/s velocity (~40.7 degree/s for a 100 km height), and the Geminids do so at 35 km/s (~20.1 degree/s for a 100 km height). Also, the shift between the vertical and oblique reflections should be taken into consideration. The difference in distributions may also be caused by the different material quality of the incoming meteoroids. Not only the composition but also the propensity for fragmentation differs between the two swarms. Leonids of cometary origin fragment more easily. Geminids of asteroidal origin, on the other hand, are more compacted in space because of the Sun, and are more difficult to fragment [34,36].

The virtual height values themselves are broadly consistent with those found by Maruyama et al. [20], except one particular type of observation. The study [20] reported that among the events that they identified as meteor echoes, there was one that appeared during the Leonid peak and was detected at all four stations. These echoes occurred at virtual heights of 200–250 km and, according to the reported images, slowly descended. Based on the observed high virtual height and hourly duration, this was probably not a meteor echo, but an intermediate descending layer; nevertheless it occurred well before the sunrise, while generally the descending layers appear at around 6:00 local time [59]. The phenomenon was not described in detail when Maruyama et al. [20] was published [60].

In contrast to the height distribution, the frequency distributions are very different from campaign to campaign and from station to station. Since Průhonice’s Digisonde has a quality antenna system resulting in a much higher sensitivity [55], the variation per station is not surprising, nor is the fact that Průhonice recorded higher frequencies. Also, the two stations applied different settings. At the Průhonice station, all the measurements were saved, while at the Sopron station, there were filtering parameters set, which could lead to data loss. This may also have contributed to the fact that within Průhonice’s data, higher average frequencies were identified.

Regarding the frequency distributions, another factor to consider is the radar backscatter cross section (σ, Stuart, p. 837, ref. [16]):

$$\sigma ={\displaystyle \frac{{\mu }_0^2{e}^2{\lambda }_0^4{N}_m^2{v}_m^2}{4096{\pi }^6{m}_e^2{D}^2}}{e}^{-{\displaystyle \frac{8{\pi }^2{r}_0^2}{{\lambda }_0^2}}}$$

(2)

N_m is the electron line density(el/m), μ₀ is the magnetic permeability of a vacuum (4π × 10⁻⁷ H/m or 1.26 × 10⁻⁶ N/A²), m_e is the mass of an electron (9.11 × 10⁻³¹ kg), e is the charge of an electron (1.609 × 10⁻¹⁹ C), λ₀ is the wavelength of the wave used in the measurements, D is the ambipolar diffusion coefficient, r₀ is the initial meteor trail radius (m), and v_m is the initial speed of the meteor.

Assume a situation in which two meteors from different meteor showers, but with the same physical parameters (N_m, r₀), arrive at the same altitude and face the same ambipolar diffusion coefficient (D). The radar cross section depends quadratically on the speed of the meteor (v_m). This means that Leonids at 71 km/s are four times more detectable than Geminids at 35 km/s (respectively ~40.7 degree/s and ~20.1 degree/s for a 100 km height). Fragmentation may also play a role, affecting the Leonids to a greater extent. Incoming meteors may break up into smaller pieces, which can then scatter over a larger area, making them easier for the Digisonde to detect [19]. This was also reflected somewhat in the data.

Based on the definitions of underdense and overdense meteor echoes of Kozlovsky et al. [22] and the typical signal strength–time plots of the radio signals reflected from meteor trails (see Supplementary Figure S5) [16], the Digisonde can detect overdense meteor trails, and presumable even underdense meteor echoes, too. Although the Digisonde transmits a relatively low power, various signal processing and noise and interference removal techniques are used to allow the detection of faint echoes [48]. Many detections endured for only one ionogram and reached low frequencies (below 3 MHz) [53]. However, in the absence of an independent measurement method (e.g., meteor radar) and a higher time resolution ($\tau ={\displaystyle \frac{r_e{c}^2{a}_l}{4{\pi }^2{f}^{2}D}}$ [22] where τ is the decay time [s], r_e is the classical electron radius ~2.82 × 10⁻¹⁵ m, c is the speed of light, D is the diffusion coefficient, a_l is the electron line density [el/m] and f is the frequency [Hz]), which could specify the decay time from which the electron line density could be derived, it was not possible to clearly categorize the meteor-induced traces found on the ionograms, and whether they were truly underdense or not is unknown.

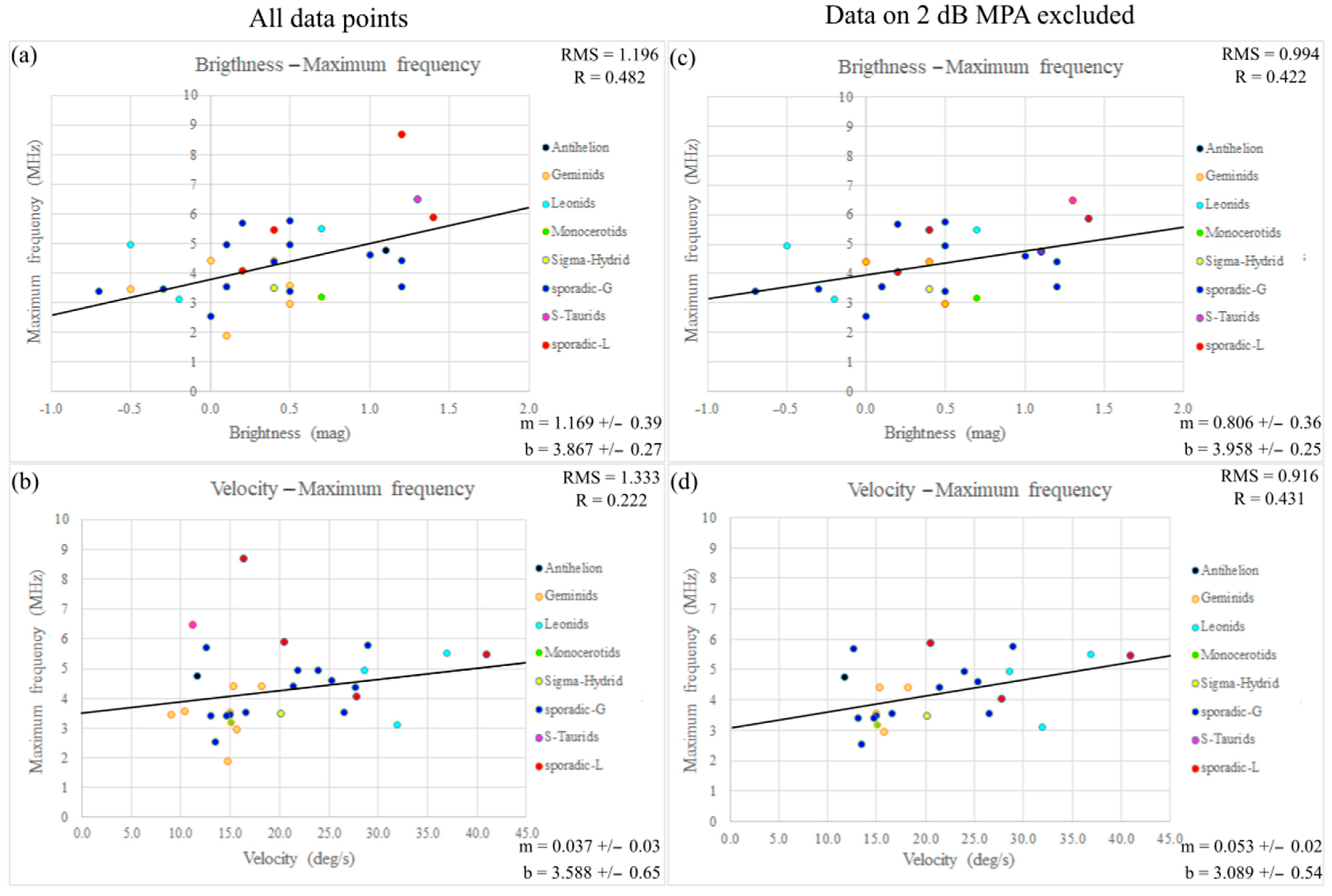
The analysis of the Digisonde measurements associated with the optical findings revealed that there is a slight correlation between the meteor’s speed and the maximum frequency reached by the occurring trace. In general, the faster a meteor was, the higher the frequency that the trace reached on the ionograms. This makes sense—the faster a meteor is, the greater the friction and the greater the ionization effect that it can have. For brightness, however, there was a slight anticorrelation with the Digisonde measurements. The brighter a meteor was, the lower the maximum echo frequencies that it reached on the ionograms. This is completely contrary to our preliminary expectations, as the expected electron line density should be higher the brighter that the meteor is [16,17,18]. It should be noted that 31 cases (25 if the data points acquired at 2 dB MPA are excluded; see Figure 10) do not allow statistically significant and robust conclusions to be drawn; however, this is a very interesting and surprising result. Further research and campaigns would be needed to be able to make statements with certainty.

Another interesting feature is the time-delayed appearance of the traces on the ionograms compared to the detection time of the optical measurements, which ranges from 12 to 132 s. Of this, up to ~40–60 s can be attributed to the measurement settings. The schedule of 1 min measurements was [hh:mm:00]—first ionogram, [hh:mm:20]—drift measurement, [hh:mm:40]—second ionogram. If a meteor arrives at say [hh:mm-1:55] and would reach say 5 MHz, but the Digisonde is currently transmitting and receiving a 6 MHz signal, the meteor’s trace will not show up on the [hh:mm:00] ionogram, and can appear only on the [hh:mm:40] ionogram. However, in comparison, it seems that there can be yet another extra ~60 s between the optical detection and Digisonde detection. The reason given for this can be that the Digisonde may have a limited horizontal resolution, and the meteor’s effect may need time to diffuse enough to be detected via the instrument (f.e. layer formation). The original incoming meteors can have a length in the kilometer range (for sporadic meteors: the most probable trail length is 15 km, while typical lengths are up to 50 km [17]) but with a diameter of only a few meters [24]. The meteor trail length does not show any correlation with any of the other measured parameters. In the case of the terminating electron line density, this is consistent with Eshleman’s theory [25]. Another factor may be the interaction between the angle of arrival and the direction of the magnetic field. In the case of the instrumental part, it is also possible that the meteor trace appears on the ionograms earlier, but only at the 2 dB MPA setting, and is therefore drowned in the noise. It is also curious that for the Leonids (all eight cases identified) this time delay is in the explainable range—the maximum delay is 62 s, which follows the case of the measurement setup described. These numbers are much higher for the Geminids. At this point, the role of the fragmentation—which is more pronounced in the Leonids—should also be mentioned. As a meteoroid breaks up, smaller pieces may scatter over an appreciable cross section larger than that of the parental meteoroid [19]. Whether this is the result of a physical process or an instrumental feature remains to be explained.

The lifetime of the traces on the ionograms is the most interesting result of all, because no correlation could be found with any of the other measured/observed parameters. It was noticed that when a meteor-induced trace splits in two on the ionograms, it tends to have a longer lifetime. These phenomena usually appear in clusters and are presumably related to mesospheric winds. Maruyama et al. [20] and Kozlovsky et al. [22] also describe that a meteor-induced trace can become a sporadic E patch-like formation due to wind shear (Supplementary Figure S4), which is observed for meteor-induced traces with long lifetimes (there, by the way, they refer to traces that persist for ~20–40 min). However, there were also traces with a lifetime of 2–3 min, which did not show any signs of wind shear (splitting). According to Oppenheim and Dimant [24], the evolution of meteor trails can be influenced by the magnetic field in addition to turbulence, which is in agreement with other previous observations [23]. Field alignment is when the direction of the meteor’s trace and the magnetic field are nearly the same, and this can increase the lifetime of the meteor’s trace. According to 3D simulations [24], near-perfect alignment (deviation below 6°) has a significant effect. A simplified comparison was also attempted in the present study, but the quality of the data is not sufficient for a credible comparison, so no significant conclusion can be drawn from that.

5. Conclusions

In 2019, on the days around the Leonids’ and Geminids’ peak, campaign measurements were performed using the DPS-4D Digisondes at the Sopron and Průhonice stations. During the sessions, two ionograms and one drift measurement were taken in one minute. The two stations are very close to each other in ionospheric terms. Synchronized measurements were made because in this way it is easy to separate regional and local effects. In addition, a temporary optical monitoring station was installed in Sopron.

Preliminarily, two months of ionograms (excluding the campaign periods) for the two stations were processed manually to study the behavior of the regular sporadic E layer. Although there are small differences (the median of the virtual height shows a 5 km difference, the maximum frequency of Průhonice displays a second peak at ~6 MHz), the distributions were mostly similar in both frequency and virtual height for the stations.

The main outputs of this study can be highlighted as follows:

• DPS-4D represents a convenient tool to detect meteor-induced traces. The settings of the instrument play a key role in the traces’ localization.
• A substantial time delay within the optical and Digisonde detection was identified and needs further investigation.
• The height distribution of the meteor-induced traces in the ionograms characterizes the particular campaigns (differs for the Geminis and the Leonids) but is quite similar for the two stations in a given campaign.
• The distributions of the maximum frequencies reached by the meteor-induced traces are very different from campaign to campaign and from station to station, unlike the height distribution. This is probably due to the different sensitivity of the antenna systems, with the handcrafted antennas of Průhonice being much more sensitive [55].
• A meteor’s speed and the maximum recorded frequency of the induced trace shows a slight positive correlation.
• The brightness of a meteor and the maximum echo frequencies detected on the ionogram are anticorrelated. This is contrary to the preliminary knowledge and needs further studies.
• The lifetime of the traces on the ionograms does not express any correlation with the other measured/observed parameters.
• A regular sporadic E activity can obscure the meteor-induced traces for the Digisonde measurements.

The analysis of the acquired data showed that it is possible to detect individual meteor-induced traces using the DPS-4D type of Digisonde, too. The meteors that were observed in the optical data but would have a large zenith angle seen by the ionosonde were not detected by the latter because of the applied method for the angle of arrival detection.

In these cases, where the instrument detects meteors, the Digisonde behaves as a meteor radar that operates on 1–17 MHz frequencies, though the upper limit depends on the actual setting and can be adjusted. At least a two ionogram/minute time resolution is needed for a proper study. The MPA threshold and the noise filtering mechanisms can have a huge effect on the detections. The Digisonde recorded more meteors’ traces than the optical instrument which had a lower brightness limit of +1 magnitude. The detection of the meteor-induced traces also depends on the sensitivity of the antenna systems. Průhonice’s Digisonde—which is modified and much more sensitive—generally detected more traces in a given time period. Because both stations are located close to each other, such a discrepancy should be attributed to the technical/instrumental differences between the two stations rather than the different physical processes above both stations.

The regular sporadic E activity can affect or even prevent successful meteor detections with the Digisondes.

Finding the reflections of a meteoric origin is also possible on Skymaps, but with a much lower probability with the current setting: only one Skymap was made every minute and they were measured/monitored with a constant 2.5 MHz frequency. For a successful detection, it was necessary that the meteor-induced traces were able to reflect the emitted signals at 2.5 MHz very briefly before the time of the measurement.

The distribution of the virtual heights of the meteor-induced traces detected on the ionograms showed that both stations obtained a similar distribution for a given campaign, but different distributions for the Leonids and Geminids campaigns. This is probably due to the different velocities and also probably a consequence of the different material quality of the meteoroid swarms.