Self-Calibration Strip Bundle Adjustment of High-Resolution Satellite Imagery
Abstract
1. Introduction
2. Methodology
2.1. Rigorous Sensor Model Taking into Account the Installation
2.2. Attitude Error Compensation
2.2.1. Satellite Attitude Representation
2.2.2. Attitude Compensation Model
2.3. Self-Calibration Strip Adjustment Taking into Account the Installation
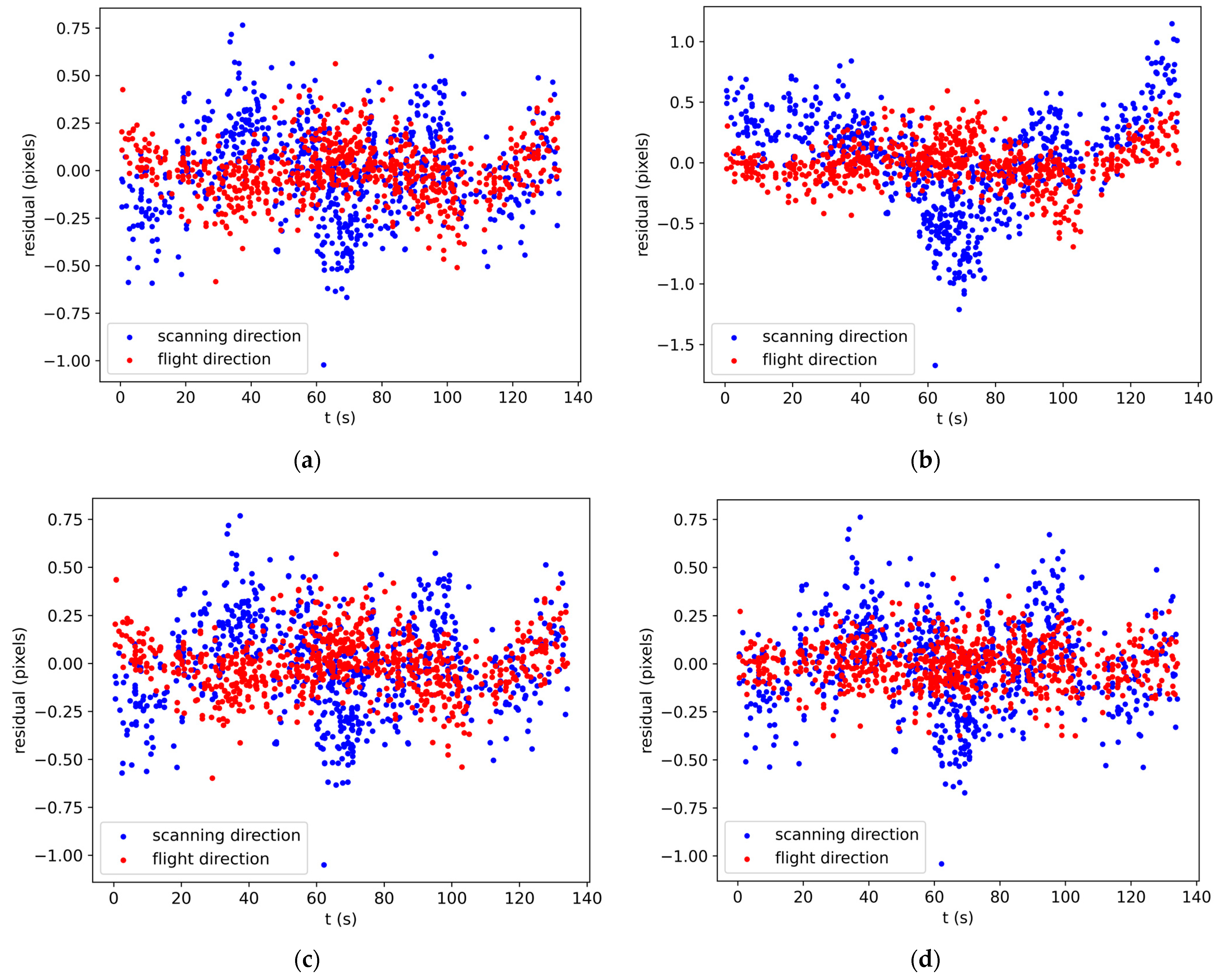
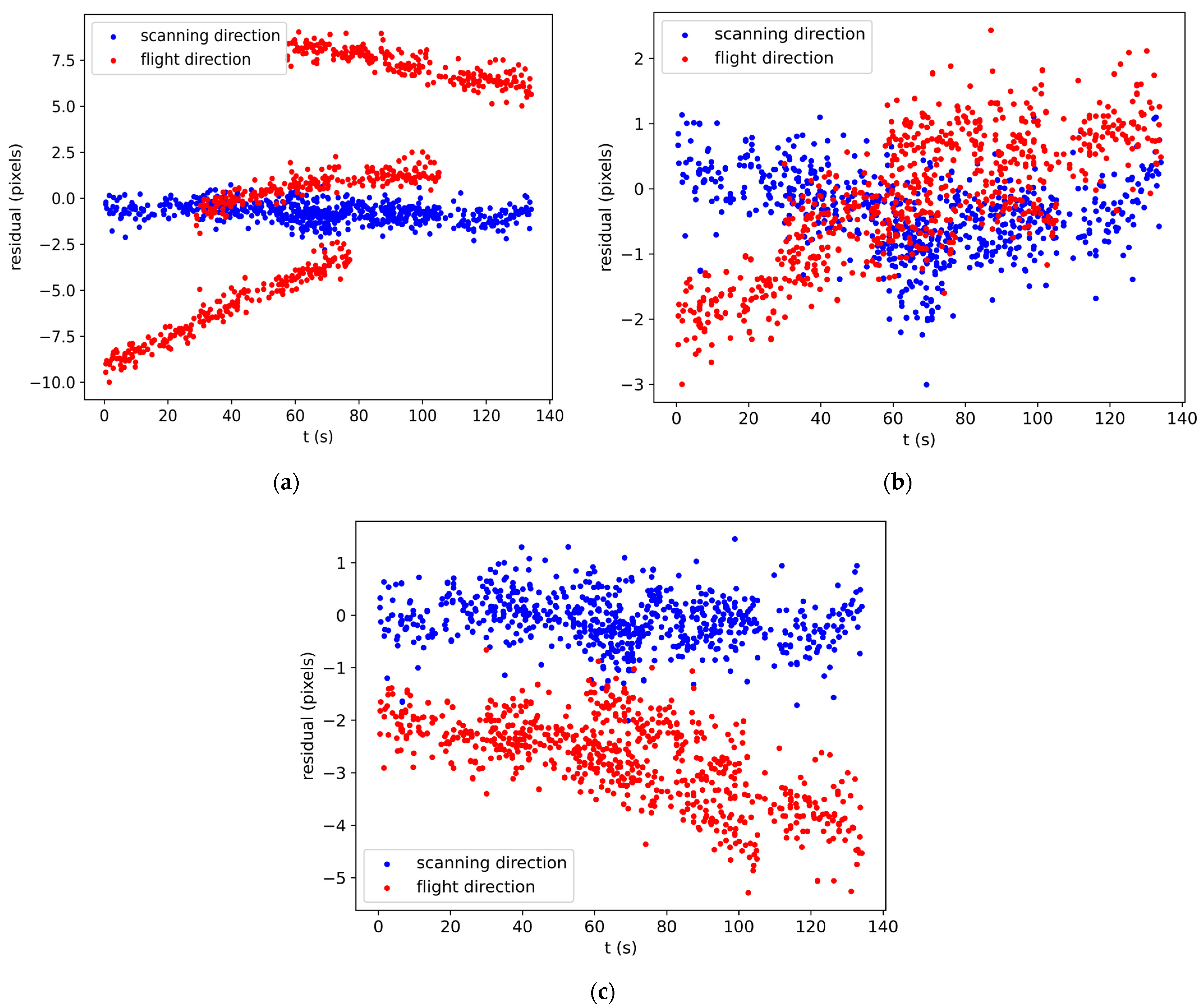
3. Experimental Results
3.1. Experimental Datasets
3.2. Bundle Adjustment without GCPs
- Plan A:
- Three-line-array-cameras independent adjustment with first-order attitude compensation model.
- Plan B:
- Self-calibration adjustment with translation attitude compensation model considering installation.
- Plan C:
- Self-calibration adjustment with first-order attitude compensation model considering installation.
- Plan D:
- Self-calibration adjustment with second-order attitude compensation model considering installation.
3.3. Bundle Adjustment with GCPs
4. Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Li, D.; Wang, M. A review of high resolution optical satellite surveying and mapping technology. Spacecr. Recovery Remote Sens. 2020, 41, 1–11. [Google Scholar]
- Tadono, T.; Shimada, M.; Watanabe, M.; Hashimoto, T.; Iwata, T. Calibration and validation of PRISM onboard ALOS. Int. Arch. Photogramm. Remote Sens. Spat. Inf. Sci. 2004, 35, 13–18. [Google Scholar]
- Bouillon, A.; Bernard, M.; Gigord, P.; Orsoni, A.; Rudowski, V.; Baudoin, A. SPOT 5 HRS geometric performances: Using block adjustment as a key issue to improve quality of DEM generation. ISPRS J. Photogramm. Remote Sens. 2006, 60, 134–146. [Google Scholar] [CrossRef]
- Muralikrishnan, S.; Pillai, A.; Narender, B.; Reddy, S.; Venkataraman, V.R.; Dadhwal, V. Validation of Indian national DEM from Cartosat-1 data. J. Indian Soc. Remote Sens. 2013, 41, 1–13. [Google Scholar] [CrossRef]
- Wang, R.; Hu, X.; Wang, J. Photogrammetry of mapping satellite-1 without ground control points. Acta Geod. Cartogr. Sin. 2013, 42, 1–5. [Google Scholar]
- Cao, H.; Zhang, X.; Zhao, C.; Xu, C.; Mo, F.; Dai, J. System design and key technolongies of the GF-7 satellite. Chin. Space Sci. Technol. 2020, 40, 1. [Google Scholar]
- Barazzetti, L.; Roncoroni, F.; Brumana, R.; Previtali, M. Georeferencing accuracy analysis of a single worldview-3 image collected over milan. Int. Arch. Photogramm. Remote Sens. Spat. Inf. Sci. 2016, XLI-B1, 429–434. [Google Scholar] [CrossRef]
- Greslou, D.; de Lussy, F.; Amberg, V.; Dechoz, C.; Lenoir, F.; Delvit, J.-M.; Lebègue, L. Pleiades-HR 1A&1B image quality commissioning: Innovative geometric calibration methods and results. In Proceedings of the Earth Observing Systems XVIII; SPIE: San Diego, CA, USA, 2013; p. 886611. [Google Scholar]
- Luthcke, S.; Zelensky, N.; Rowlands, D.; Lemoine, F.; Williams, T. The 1-centimeter orbit: Jason-1 precision orbit determination using GPS, SLR, DORIS, and altimeter data special issue: Jason-1 calibration/validation. Mar. Geod. 2003, 26, 399–421. [Google Scholar] [CrossRef]
- Zhao, C.; Tang, X. Precise orbit determination for the ZY-3 satellite mission using GPS receiver. J. Astronaut. 2013, 34, 1202–1206. [Google Scholar]
- Jiang, Y.; Zhang, G.; Tang, X.; Zhu, X.; Qin, Q.; Li, D.; Fu, X. High accuracy geometric calibration of ZY-3 three-line image. Acta Geod. Cartogr. Sin. 2013, 42, 523–529. [Google Scholar]
- Hofmann, O.; Navé, P.; Ebner, H. DPS-A Digital Photogrammetric System for producing digital elevation models and orthophotos by means of linear array scanner imagery. Photogramm. Eng. Remote Sens. 1984, 50, 1135–1142. [Google Scholar]
- Wang, R. Satellite Photogrammetric Principle for Three-Line-Array CCD Imagery; Surveying and Mapping Press: Beijing, China, 2016; pp. 53–54. [Google Scholar]
- Wang, R.; Hu, X.; Wang, X.; Yang, J. The construction and application of mapping satellite-1 engineering. J. Remote Sens. 2012, 16, 2–5. [Google Scholar]
- Ebner, H.; Kornus, W.; Ohlhof, T.; Putz, E. Orientation of MOMS-02/D2 and MOMS-2P/PRIRODA imagery. ISPRS J. Photogramm. Remote Sens. 1999, 54, 332–341. [Google Scholar] [CrossRef]
- Poli, D. A rigorous model for spaceborne linear array sensors. Photogramm. Eng. Remote Sens. 2007, 73, 187–196. [Google Scholar] [CrossRef]
- Weser, T.; Rottensteiner, F.; Willneff, J.; Poon, J.; Fraser, C.S. Development and testing of a generic sensor model for pushbroom satellite imagery. Photogramm. Rec. 2008, 23, 255–274. [Google Scholar] [CrossRef]
- Kim, T.; Dowman, I. Comparison of two physical sensor models for satellite images: Position–rotation model and orbit–attitude model. Photogramm. Rec. 2006, 21, 110–123. [Google Scholar] [CrossRef]
- Li, R.; Hwangbo, J.; Chen, Y.; Di, K. Rigorous photogrammetric processing of HiRISE stereo imagery for Mars topographic mapping. IEEE Trans. Geosci. Remote Sens. 2011, 49, 2558–2572. [Google Scholar]
- Radhadevi, P.; Nagasubramanian, V.; Mahapatra, A.; Solanki, S.; Sumanth, K.; Varadan, G. Potential of high-resolution Indian remote sensing satellite imagery for large scale mapping. In Proceedings of the ISPRS Hannover Workshop, High-Resolution Earth Imaging for Geospatial Information, Hannover, Germany, 2–5 June 2009. [Google Scholar]
- Rottensteiner, F.; Weser, T.; Lewis, A.; Fraser, C.S. A strip adjustment approach for precise georeferencing of ALOS optical imagery. IEEE Trans. Geosci. Remote Sens. 2009, 47, 4083–4091. [Google Scholar] [CrossRef]
- Di, K.; Liu, Y.; Liu, B.; Peng, M.; Hu, W. A Self-Calibration Bundle Adjustment Method for Photogrammetric Processing of Chang’E-2 Stereo Lunar Imagery. IEEE Trans. Geosci. Remote Sens. 2013, 52, 5432–5442. [Google Scholar]
- Zhang, Y.; Zheng, M.; Wang, X.; Huang, X. Strip-based bundle adjustment of Mapping Satellite-1 three-line array imagery. J. Remote Sens. Beijing 2012, 16, 84–89. [Google Scholar]
- Zheng, M.; Zhang, Y.; Zhu, J.; Xiong, X. Self-calibration adjustment of CBERS-02B long-strip imagery. IEEE Trans. Geosci. Remote Sens. 2015, 53, 3847–3854. [Google Scholar] [CrossRef]
- Cao, J.; Yuan, X.; Fu, J.; Gong, J. Precise sensor orientation of high-resolution satellite imagery with the strip constraint. IEEE Trans. Geosci. Remote Sens. 2017, 55, 5313–5323. [Google Scholar] [CrossRef]
- Li, D. China’s first civilian three-line-array stereo mapping satellite: ZY-3. Acta Geod. Cartogr. Sin. 2012, 41, 317–322. [Google Scholar]
- Grodecki, J.; Dial, G. Block adjustment of high-resolution satellite images described by rational polynomials. Photogramm. Eng. Remote Sens. 2003, 69, 59–68. [Google Scholar] [CrossRef]
- Zhang, Y.; Zheng, M.; Xiong, J.; Lu, Y.; Xiong, X. On-orbit geometric calibration of ZY-3 three-line array imagery with multistrip data sets. IEEE Trans. Geosci. Remote Sens. 2013, 52, 224–234. [Google Scholar] [CrossRef]
- Zhang, Y.; Zheng, M.; Xiong, X.; Xiong, J. Multistrip bundle block adjustment of ZY-3 satellite imagery by rigorous sensor model without ground control point. IEEE Geosci. Remote Sens. Lett. 2014, 12, 865–869. [Google Scholar] [CrossRef]
- Pan, H.; Tao, C.; Zou, Z. Precise georeferencing using the rigorous sensor model and rational function model for ZiYuan-3 strip scenes with minimum control. ISPRS J. Photogramm. Remote Sens. 2016, 119, 259–266. [Google Scholar] [CrossRef]
- Yuan, X.; Yu, X. Calibration of angular systematic errors for high resolution satellite imagery. Acta Geod. Cartogr. Sin. 2012, 41, 385–392. [Google Scholar]
- Cao, J.; Fu, J.; Yuan, X.; Gong, J. Nonlinear bias compensation of ZiYuan-3 satellite imagery with cubic splines. ISPRS J. Photogramm. Remote Sens. 2017, 133, 174–185. [Google Scholar] [CrossRef]
- Tao, C.V.; Hu, Y. A comprehensive study of the rational function model for photogrammetric processing. Photogramm. Eng. Remote Sens. 2001, 67, 1347–1358. [Google Scholar]
- Poli, D.; Toutin, T. Review of developments in geometric modelling for high resolution satellite pushbroom sensors. Photogramm. Rec. 2012, 27, 58–73. [Google Scholar] [CrossRef]
- Liu, C.; Zhang, Y.; Fan, D.; Lei, R.; Dai, H. Self-calibration Block Adjustment for Three-line-array Image of ZY-3. Acta Geod. Cartogr. Sin. 2014, 43, 1046. [Google Scholar]

| Number of Scenes | Image Acquisition Time | Image Coverage Ground Size | Image Coverage (Latitude and Longitude) |
|---|---|---|---|
| 12 × 3 | 3 February 2012 | 560 km × 51 km | E114.947709° N42.064374° |
| E115.547510° N41.956830° | |||
| E114.107452° N37.138009° | |||
| E113.544067° N37.226530° |
| Plan | The RMSE of TPs in the Image Space (Pixels) | ||
|---|---|---|---|
| Scanning Direction | Flight Direction | Plane | |
| Plan A | 0.240 | 0.145 | 0.280 |
| Plan B | 0.402 | 0.172 | 0.438 |
| Plan C | 0.241 | 0.145 | 0.281 |
| Plan D | 0.238 | 0.117 | 0.265 |
| Plan | The RMSE of CKPs in the Object Space (m) | |||
|---|---|---|---|---|
| East | North | Plane | Height | |
| Plan A | 3.873 | 2.553 | 4.639 | 53.270 |
| Plan B | 7.293 | 15.638 | 17.255 | 53.918 |
| Plan C | 2.308 | 3.217 | 3.960 | 53.287 |
| Plan D | 6.110 | 4.518 | 7.599 | 53.892 |
| GCP Layout Scheme | Plan | The RMSE of TPs in the Image Space (Pixels) | ||
|---|---|---|---|---|
| Scanning Direction | Flight Direction | Plane | ||
| 4 GCPs | Plan A | 0.248 | 0.145 | 0.287 |
| Plan C | 0.249 | 0.167 | 0.300 | |
| Plan D | 0.246 | 0.152 | 0.289 | |
| 16 GCPs | Plan A | 0.269 | 0.175 | 0.321 |
| Plan C | 0.269 | 0.194 | 0.331 | |
| Plan D | 0.263 | 0.181 | 0.319 | |
| 27 GCPs | Plan A | 0.290 | 0.218 | 0.363 |
| Plan C | 0.290 | 0.248 | 0.382 | |
| Plan D | 0.288 | 0.229 | 0.368 | |
| GCP Layout Scheme | Plan | The RMSE of CKPs in the Object Space (m) | |||
|---|---|---|---|---|---|
| East | North | Plane | Height | ||
| 4 GCPs | Plan A | 1.680 | 1.385 | 2.177 | 1.571 |
| Plan C | 1.679 | 1.355 | 2.158 | 2.313 | |
| Plan D | 1.297 | 1.332 | 1.859 | 1.876 | |
| 16 GCPs | Plan A | 1.333 | 1.331 | 1.884 | 1.505 |
| Plan C | 1.328 | 1.340 | 1.887 | 2.275 | |
| Plan D | 1.309 | 1.285 | 1.834 | 1.717 | |
| 27 GCPs | Plan A | 1.259 | 1.341 | 1.839 | 1.340 |
| Plan C | 1.260 | 1.343 | 1.841 | 2.224 | |
| Plan D | 1.233 | 1.298 | 1.790 | 1.360 | |
| Test Scheme | Comparison Plan | Plane | Height | ||
|---|---|---|---|---|---|
| ME (m) | p-Value | ME (m) | p-Value | ||
| Test 1 | Plan A | 1.904 | 0.924 | 0.751 | 0.455 |
| Plan C | 1.914 | 0.618 | |||
| Test 2 | Plan C | 1.914 | 2.190 × 10−4 | 0.618 | 0.194 |
| Plan D | 1.551 | 0.864 | |||
| Test Scheme | GCP Layout Scheme | Plane | Height | ||
|---|---|---|---|---|---|
| ME (m) | p-Value | ME (m) | p-Value | ||
| Test 3 | 4 GCPs | 1.551 | 0.895 | 0.864 | 0.009 |
| 16 GCPs | 1.538 | 0.436 | |||
| Test 4 | 16 GCPs | 1.538 | 0.676 | 0.436 | 0.020 |
| 27 GCPs | 1.496 | 0.079 | |||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, X.; Pan, H.; Zhou, S.; Zhu, X. Self-Calibration Strip Bundle Adjustment of High-Resolution Satellite Imagery. Remote Sens. 2024, 16, 2196. https://doi.org/10.3390/rs16122196
Zhang X, Pan H, Zhou S, Zhu X. Self-Calibration Strip Bundle Adjustment of High-Resolution Satellite Imagery. Remote Sensing. 2024; 16(12):2196. https://doi.org/10.3390/rs16122196
Chicago/Turabian StyleZhang, Xue, Hongbo Pan, Shun Zhou, and Xiaoyong Zhu. 2024. "Self-Calibration Strip Bundle Adjustment of High-Resolution Satellite Imagery" Remote Sensing 16, no. 12: 2196. https://doi.org/10.3390/rs16122196
APA StyleZhang, X., Pan, H., Zhou, S., & Zhu, X. (2024). Self-Calibration Strip Bundle Adjustment of High-Resolution Satellite Imagery. Remote Sensing, 16(12), 2196. https://doi.org/10.3390/rs16122196






