Spaceborne HRWS-SAR-GMTI System Design Method with Optimal Configuration
Abstract
1. Introduction
2. Problem Description
3. Proposed Method
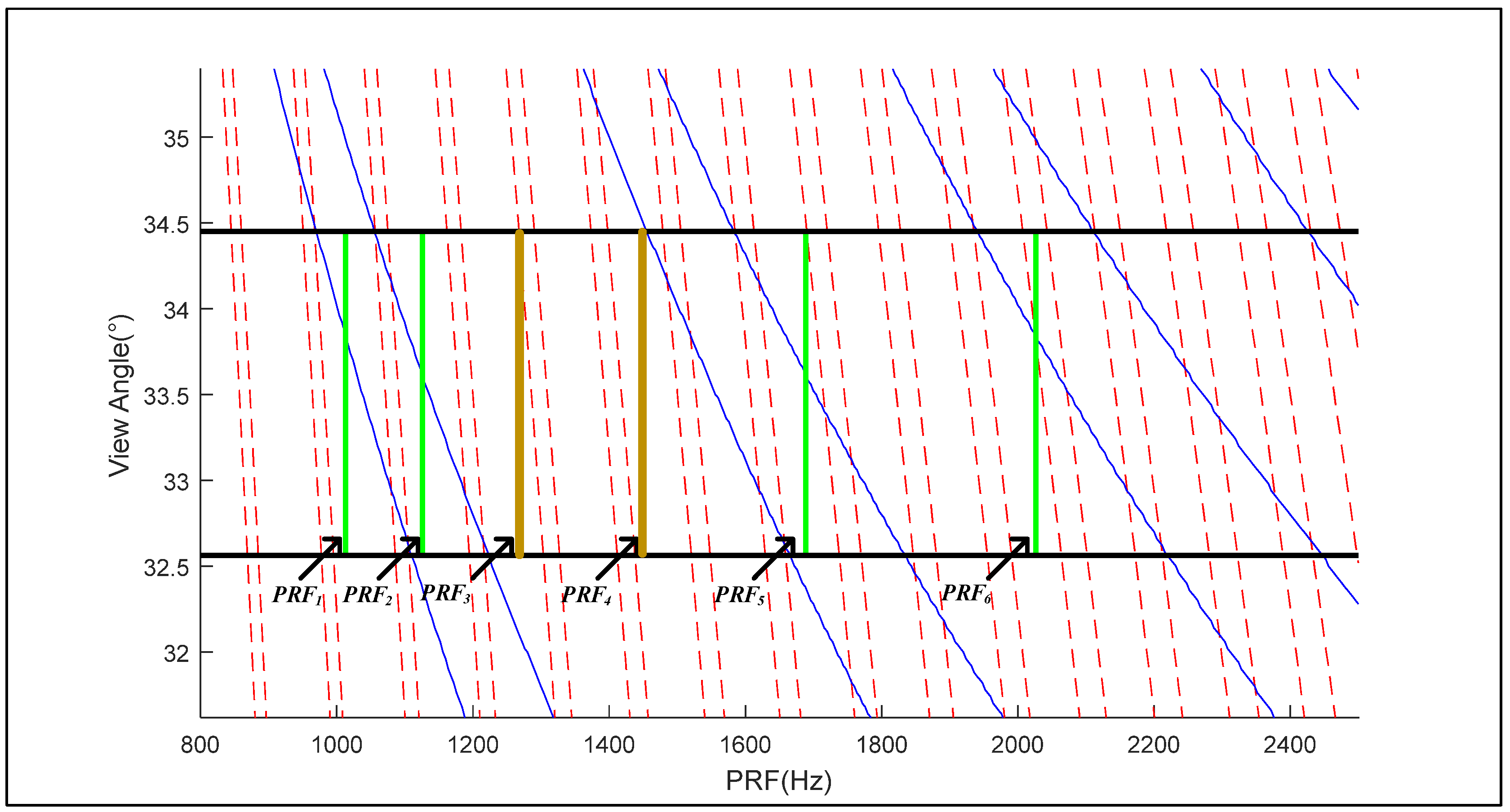
3.1. PRF Design for HRWS SAR-GMTI System with an Ideal Configuration
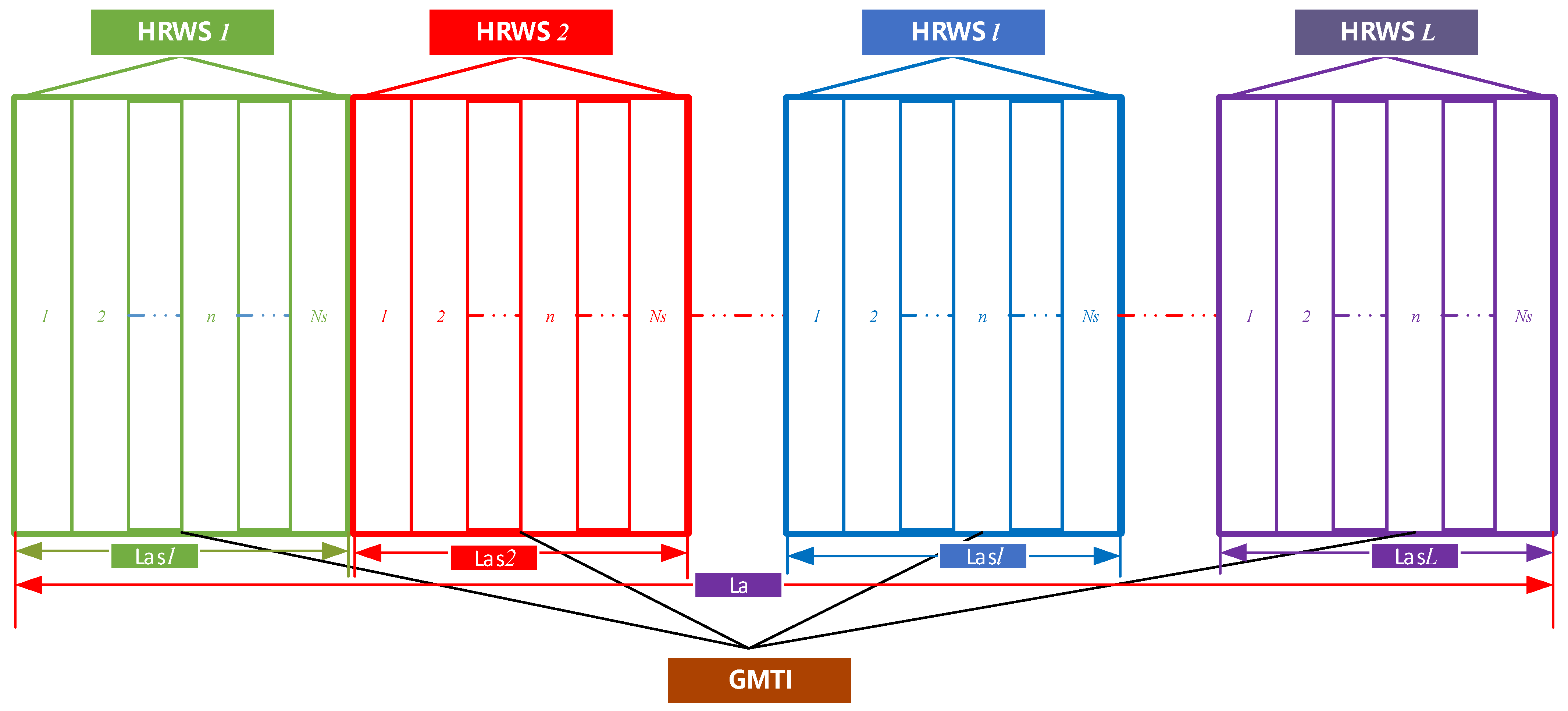
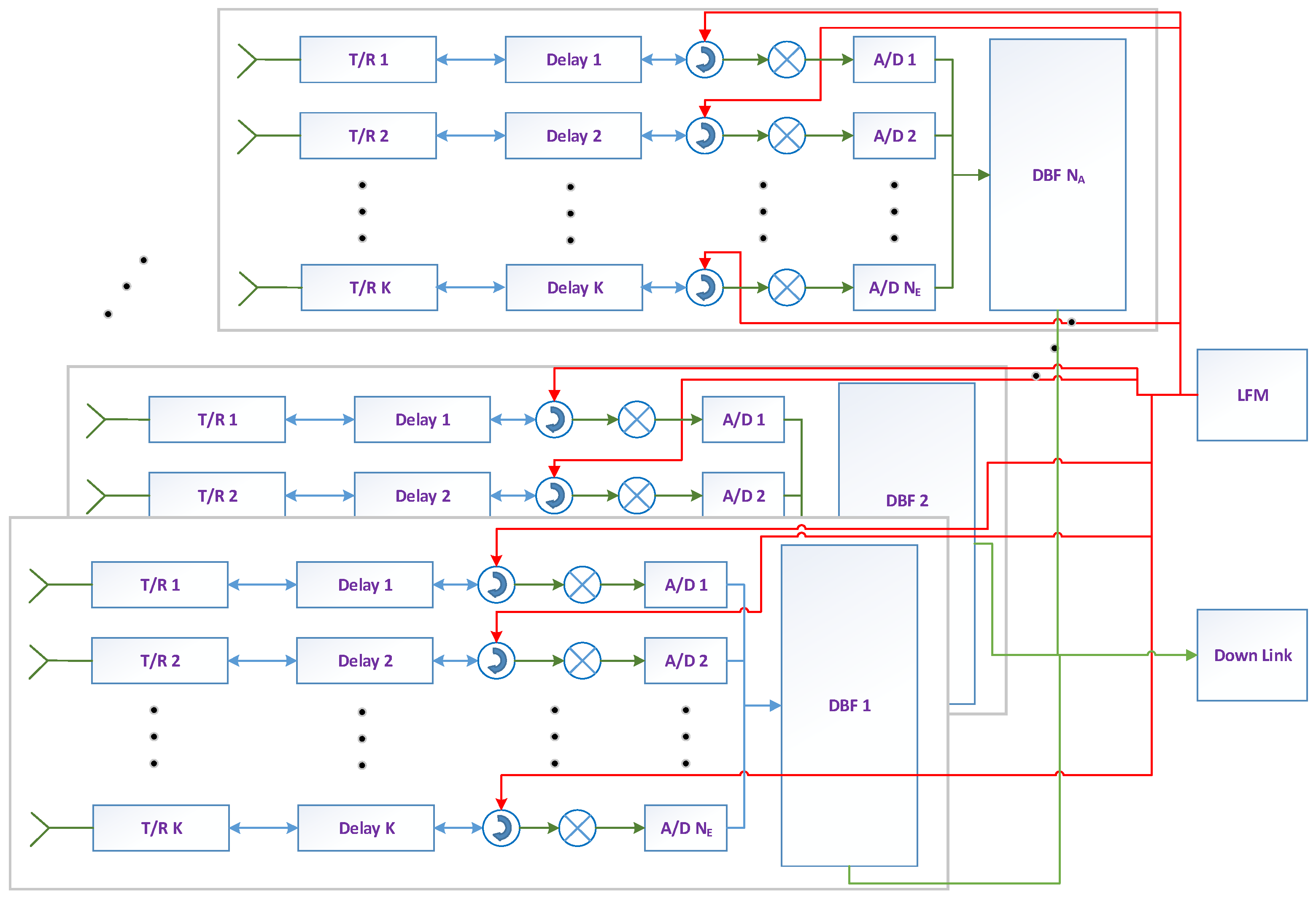
3.2. Wide-Swath Realization in a Spaceborne HRWS-SAR-GMTI System
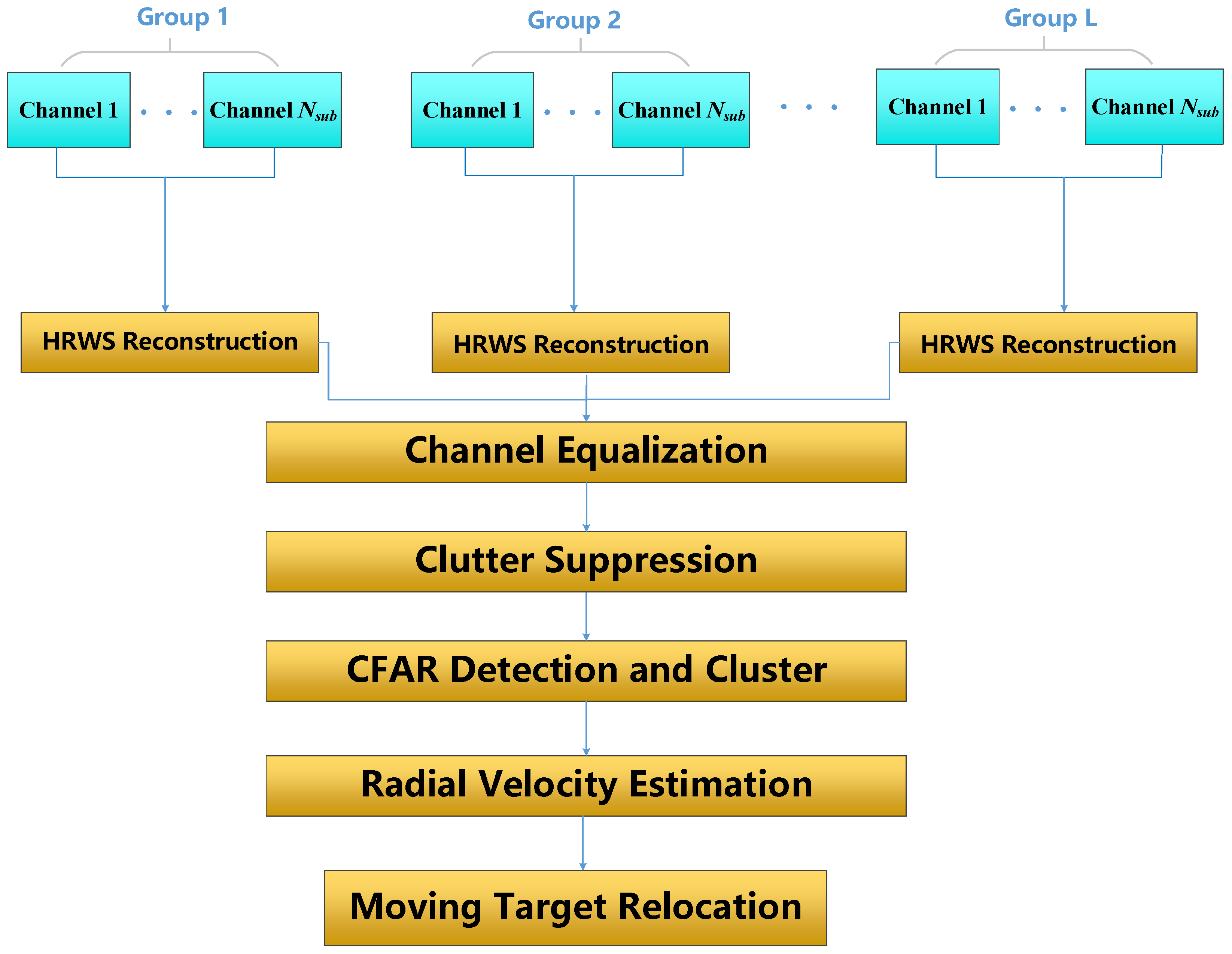
3.3. HRWS-GMTI Implementation
4. Simulation Results and Analysis
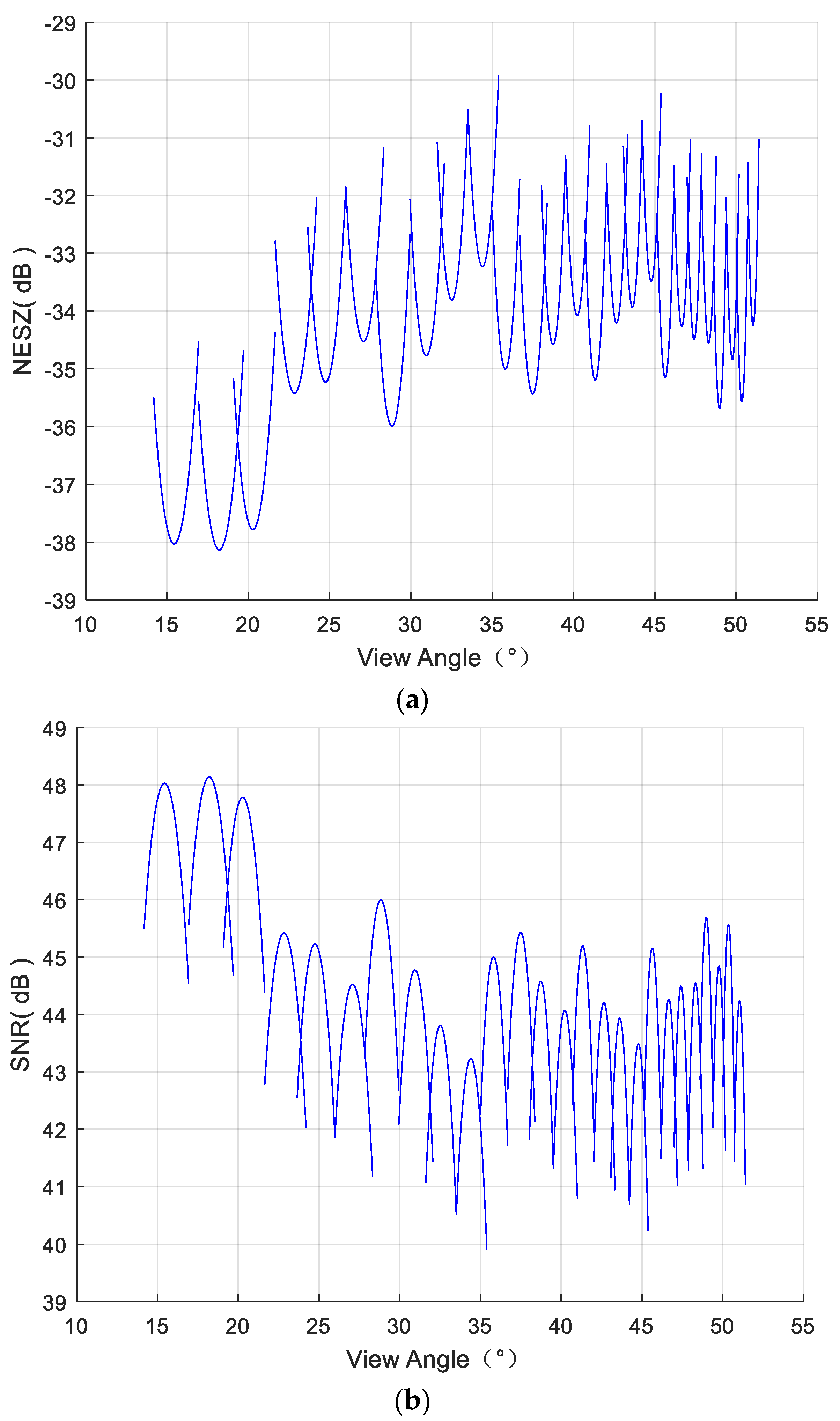
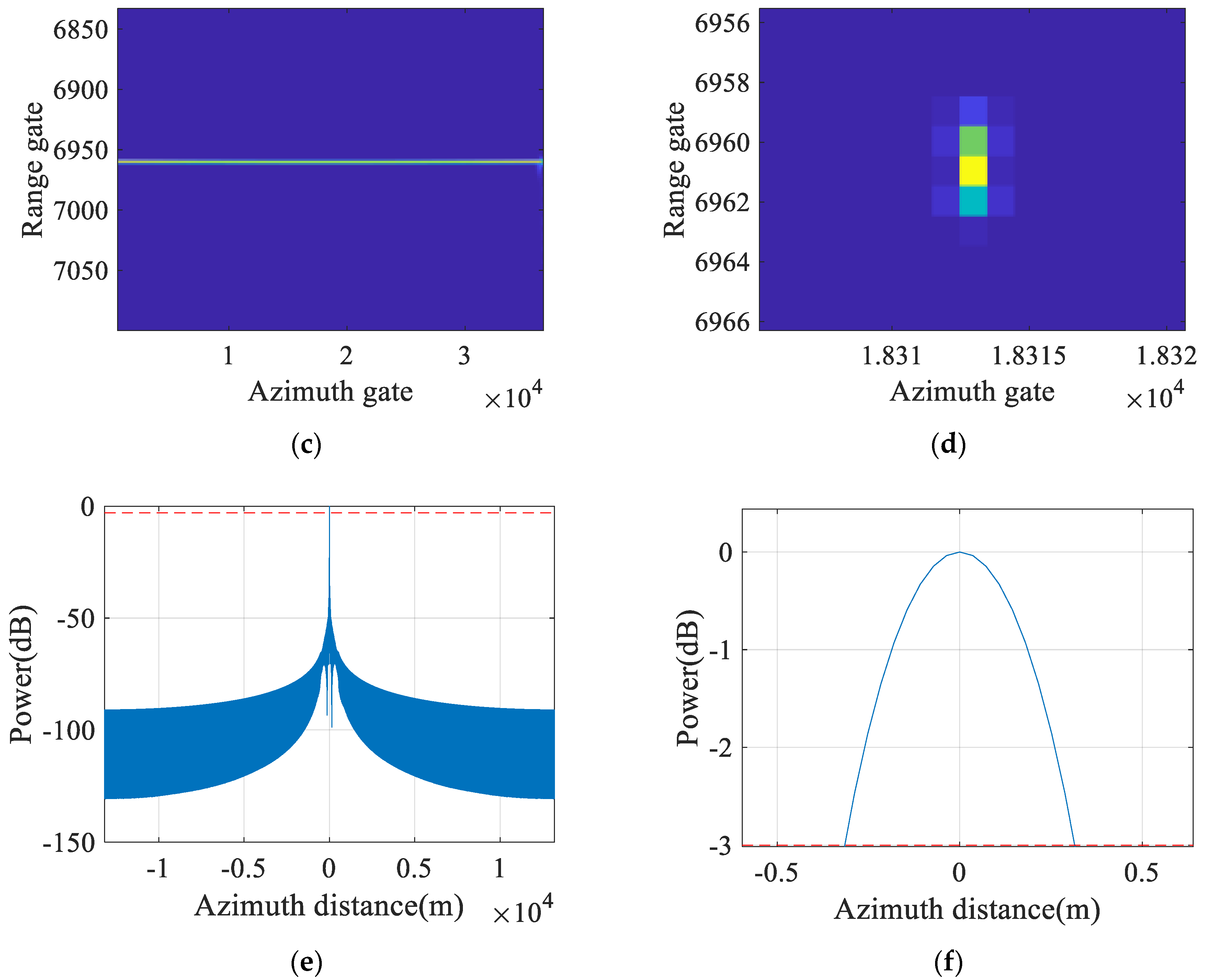
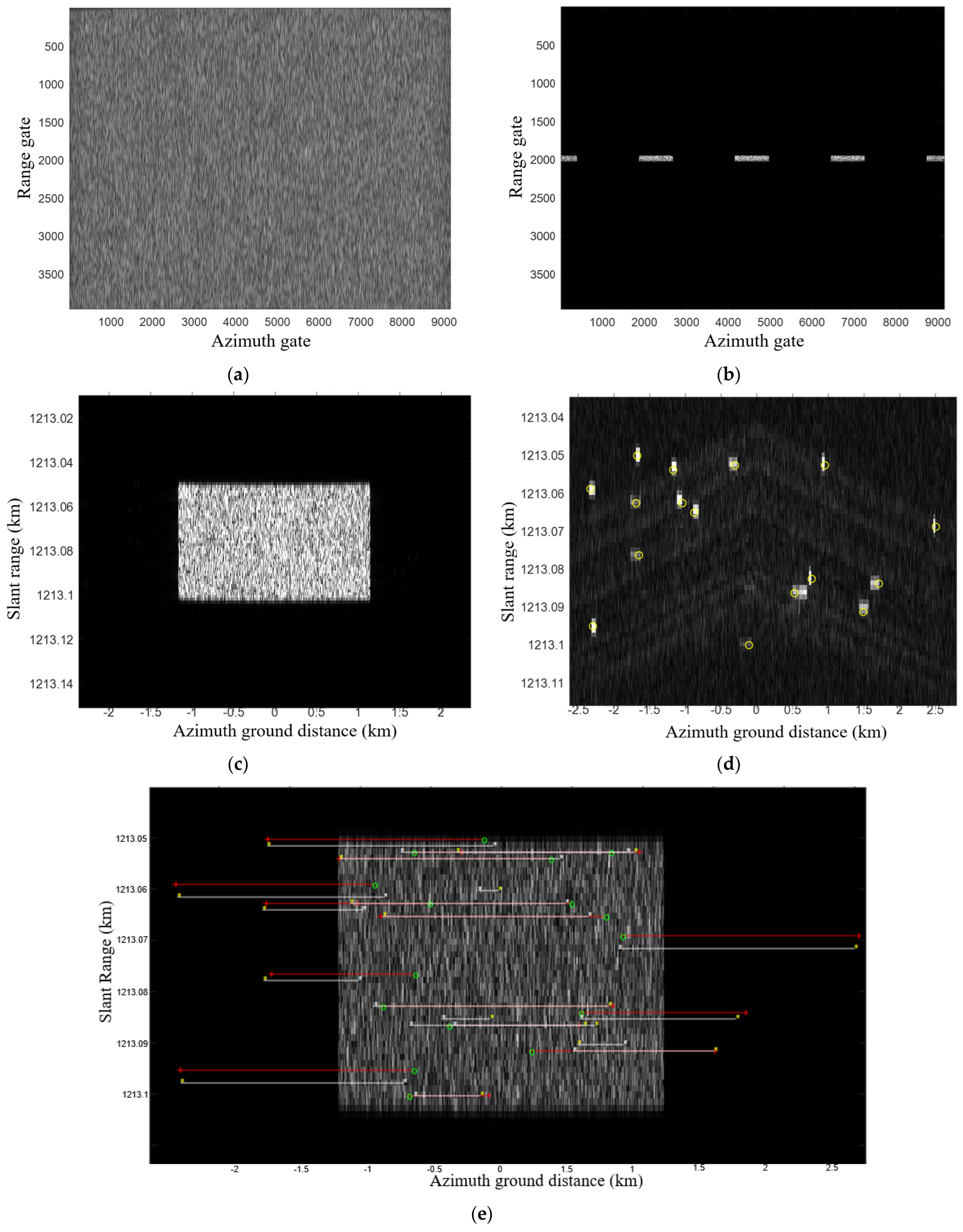
4.1. Experiment for Working Mode of the HRWS-GMTI
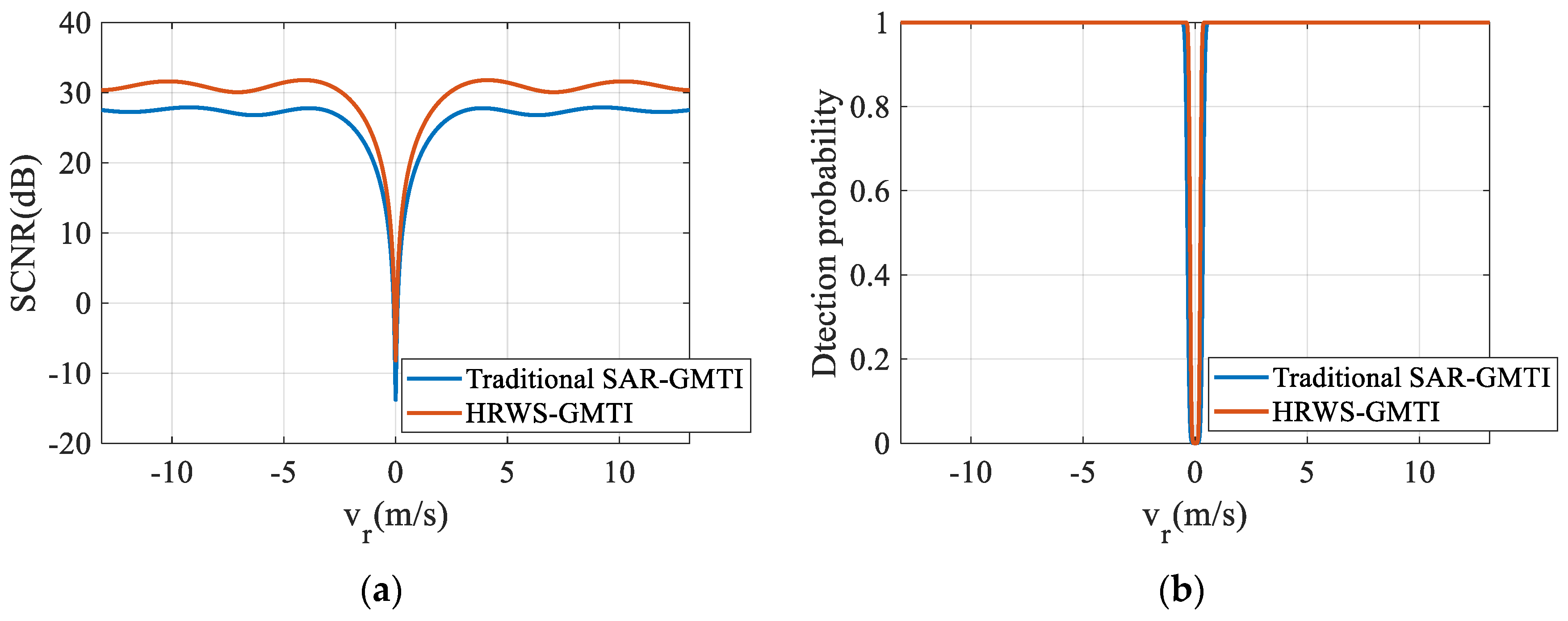
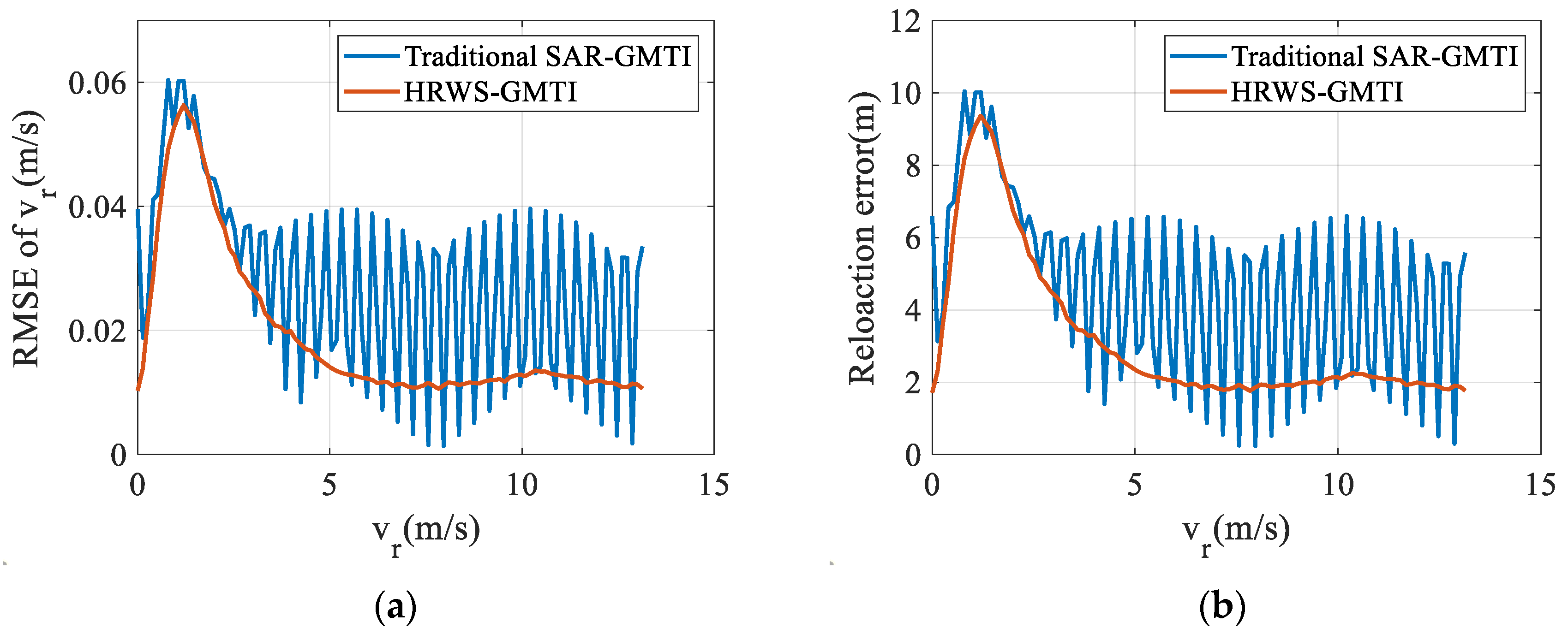
4.2. HRWS-GMTI Performance Analysis
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Abbreviations
| HRWS-SAR | High-Resolution Wide Swath Synthetic Aperture Rada |
| GMTI | Ground Moving Target Indication |
| DPCA | Displaced Phase Center Antenna |
| PRF | Pulse Repetition Frequency |
| DBF | Digital Beam Forming |
| SCORE | Scanning on Receive |
| NESZ | Noise equivalent scattering coefficient |
| ATI | Along-track interferometric |
| SCNR | Signal to clutter plus noise ratio |
| MRBF | Matched reconstruction filter bank |
| SNR | Signal-to-noise ratio |
References
- Raney, R.K. Synthetic Aperture Imaging Radar and Moving Targets. IEEE Trans. AES 1971, 3, 499–505. [Google Scholar] [CrossRef]
- Lee, J.S.; Hoppel, K.W.; Mango, S.A.; Miller, A.R. Intensity and phase statistics of multilook polarimetric and interferometric SAR imagery. IEEE Trans. Geosci. Remote Sens. 1994, 32, 1017–1028. [Google Scholar] [CrossRef]
- Budillon, A.; Evangelista, A.; Schirinzi, G. GLRT detection of moving targets via multi-baseline along track interferometric SAR systems. IEEE Geosci. Remote Sens. Lett. 2012, 9, 348–352. [Google Scholar] [CrossRef]
- Zheng, M.J.; Yang, R.L. An improved DPCA moving targets detecting algorithm. Acta Electron. Sin. 2004, 32, 1429–1432. [Google Scholar] [CrossRef]
- Yi, C.; Bo, Q.; Shengli, W. DPCA motion compensation technique based on multiple phase centers. In Proceedings of the 2011 IEEE CIE International Conference on Radar, Chengdu, China, 24–27 October 2011; pp. 711–714. [Google Scholar] [CrossRef]
- Brennan, L.E.; Reed, L.S. Theory of adaptive radar. IEEE Trans. Aerosp. Electron. Syst. 1973, 9, 237–252. [Google Scholar] [CrossRef]
- Shu, Y.; Liao, G.; Yang, Z. Design Considerations of PRF for Optimizing GMTI Performance in Azimuth Multichannel SAR Systems with HRWS Imaging Capability. IEEE Trans. Geosci. Remote Sens. 2014, 52, 2048–2063. [Google Scholar]
- Baumgartner, S.V.; Krieger, G. Simultaneous High-Resolution Wide-Swath SAR Imaging and Ground Moving Target Indication: Processing Approaches and System Concepts. IEEE J. Sel. Top. Appl. Earth Obs. Remote Sens. 2015, 8, 5015–5029. [Google Scholar] [CrossRef]
- Xing, M.; Sun, G.; Li, X. Study on SAR/GMTI Processing for High-Resolution Wide-Swath SAR System. J. Radars 2015, 4, 375–385. [Google Scholar]
- Zhang, S.X.; Xing, M.D.; Xia, X.G.; Guo, R.; Liu, Y.Y.; Bao, Z. Robust Clutter Suppression and Moving Target Imaging Approach for Multichannel in Azimuth High-Resolution and Wide-Swath Synthetic Aperture Radar. IEEE Trans. Geosci. Remote Sens. 2015, 53, 687–709. [Google Scholar] [CrossRef]
- Yang, T.; Wang, Y.; Li, W. A Moving Target Imaging Algorithm for HRWS SAR/GMTI Systems. IEEE Trans. Aerosp. Electron. Syst. 2017, 53, 1147–1157. [Google Scholar] [CrossRef]
- Hou, L.; Zhang, Q. GMTI Processing for Multi-Channel High-Resolution Wide-Swath SAR Systems. Remote Sens. Lett. 2019, 10, 21–29. [Google Scholar] [CrossRef]
- Zhang, S.; Jiang, Z.; Chen, J.; Li, S.; Liu, Y.; Guo, R.; Xing, M. An Effective Clutter Suppression Approach Based on Null-Space Technique for the Space-Borne Multichannel in Azimuth High-Resolution and Wide-Swath SAR System. IEEE Trans. Geosci. Remote Sens. 2022, 60, 5211428. [Google Scholar] [CrossRef]
- Zhang, Z.; Yu, W.; Zheng, M.; Zhao, L.; Zhou, Z.X. Robust Clutter Suppression and Radial Velocity Estimation for High-Resolution Wide-Swath SAR-GMTI. Remote Sens. 2022, 14, 1555. [Google Scholar] [CrossRef]
- Ender, J.; Klare, J. System architectures and algorithms for radar imaging by MIMO-SAR. In Proceedings of the 2009 IEEE Radar Conference, Pasadena, CA, USA, 4–8 May 2009. [Google Scholar]
- Huang, P.; Xia, X.G.; Wang, L.; Xu, H.; Liu, X.; Liao, G.; Jiang, X. Imaging and Relocation for Extended Ground Moving Targets in Multichannel SAR-GMTI Systems. IEEE Trans. Geosci. Remote Sens. 2021, 60, 5214024. [Google Scholar] [CrossRef]
- Ender, J.H.G. The airborne experimental multi-channel SAR system AER-II. In Proceedings of the EUSAR Conference, Koenigswinter, Germany, 26–28 March 1996; pp. 49–52. [Google Scholar]
- Ji, P.; Xing, S.; Dai, D.; Pang, B.; Feng, D. A Smart Multitransmitter Cooperative False Images Generation Method Against Multichannel SAR-GMTI. IEEE Trans. Geosci. Remote Sens. 2024, 62, 5204017. [Google Scholar] [CrossRef]
- Gebert, N.; Krieger, G.; Moreira, A. Digital Beamforming on Receive: Techniques and Optimization Strategies for High-Resolution Wide-Swath SAR Imaging. Aerosp. Electron. Syst. IEEE Trans. 2009, 45, 564–592. [Google Scholar] [CrossRef]
- Huang, H.; Huang, P.; Liu, X.; Xia, X.G.; Deng, Y.; Fan, H.; Liao, G. A Novel Channel Errors Calibration Algorithm for Multichannel High-Resolution and Wide-Swath SAR Imaging. IEEE Trans. Geosci. Remote Sens. 2021, 60, 5201619. [Google Scholar] [CrossRef]
- Raney, R.K.; Runge, H.; Bamler, R.; Cumming, I.G.; Wong, F.H. Precision SAR processing using chirp scaling. Geosci. Remote Sens. IEEE Trans. 1994, 32, 786–799. [Google Scholar] [CrossRef]



| Parameters | Values |
|---|---|
| Height of orbit | 1100 km |
| Carrier frequency | 9.6 GHz |
| Signal bandwidth | ≤400 MHz |
| Number of antenna channels (A × R) | 30 × 128 |
| Channel number of HRWS (A) | 4~10 |
| Channel number of GMTI (A) | ≥3 |
| Antenna size (A × R) | 43.2 m × 2.6 m |
| Range of view angle | 15.55°~51.05° |
| Resolution/swath | ≤1 m/(60 km~120 km) |
| Beam Number | View Angle | Azimuth Channel Number | Optimal PRF |
|---|---|---|---|
| 1 | 15.55° | 27 | 1126.6 Hz |
| 2 | 18.31° | 21 | 1448.4 Hz |
| 3 | 20.36° | 21 | 1448.4 Hz |
| 4 | 22.92° | 30 | 1013.3 Hz |
| 5 | 24.82° | 30 | 1013.3 Hz |
| 6 | 27.159° | 30 | 1013.3 Hz |
| 7 | 28.89° | 21 | 1448.4 Hz |
| 8 | 31° | 24 | 1266.3 Hz |
| 9 | 32.56° | 30 | 1013.3 Hz |
| 10 | 34.45° | 30 | 1013.3 Hz |
| 11 | 35.84° | 30 | 1013.3 Hz |
| 12 | 37.52° | 24 | 1266.3 Hz |
| 13 | 38.77° | 30 | 1013.3 Hz |
| 14 | 40.25° | 30 | 1013.3 Hz |
| 15 | 41.35° | 24 | 1266.3 Hz |
| 16 | 42.67° | 27 | 1126.6 Hz |
| 17 | 43.64° | 30 | 1013.3 Hz |
| 18 | 44.8° | 30 | 1013.3 Hz |
| 19 | 45.66° | 27 | 1126.6 Hz |
| 20 | 46.67° | 30 | 1013.3 Hz |
| 21 | 47.43° | 30 | 1013.3 Hz |
| 22 | 48.33° | 27 | 1126.6 Hz |
| 23 | 48.99° | 27 | 1126.6 Hz |
| 24 | 49.78° | 30 | 1013.3 Hz |
| 25 | 50.36° | 27 | 1126.6 Hz |
| 26 | 51.05° | 30 | 1013.3 Hz |
| Parameters | Values |
|---|---|
| Channel number of HRWS operation (A) | 6 |
| Channel number of GMTI operation (A) | 5 |
| Elevation angle of moving targets | 22.9° |
| The optimal PRF | 1694.4 Hz |
| RCS of moving point targets | 10 dBm2 |
| Clutter background | Grassland (RCS is considered with −10 dB) |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jiang, Y.; Wang, L.; Ling, Q.; Ma, J.; Huang, P.; Liu, X.; Fan, J. Spaceborne HRWS-SAR-GMTI System Design Method with Optimal Configuration. Remote Sens. 2024, 16, 2148. https://doi.org/10.3390/rs16122148
Jiang Y, Wang L, Ling Q, Ma J, Huang P, Liu X, Fan J. Spaceborne HRWS-SAR-GMTI System Design Method with Optimal Configuration. Remote Sensing. 2024; 16(12):2148. https://doi.org/10.3390/rs16122148
Chicago/Turabian StyleJiang, Yan, Lingyu Wang, Qing Ling, Jingtao Ma, Penghui Huang, Xingzhao Liu, and Jixia Fan. 2024. "Spaceborne HRWS-SAR-GMTI System Design Method with Optimal Configuration" Remote Sensing 16, no. 12: 2148. https://doi.org/10.3390/rs16122148
APA StyleJiang, Y., Wang, L., Ling, Q., Ma, J., Huang, P., Liu, X., & Fan, J. (2024). Spaceborne HRWS-SAR-GMTI System Design Method with Optimal Configuration. Remote Sensing, 16(12), 2148. https://doi.org/10.3390/rs16122148







