Recovering the Near-Surface Magnetic Image of Mercury from Satellite Observations
Abstract
1. Introduction
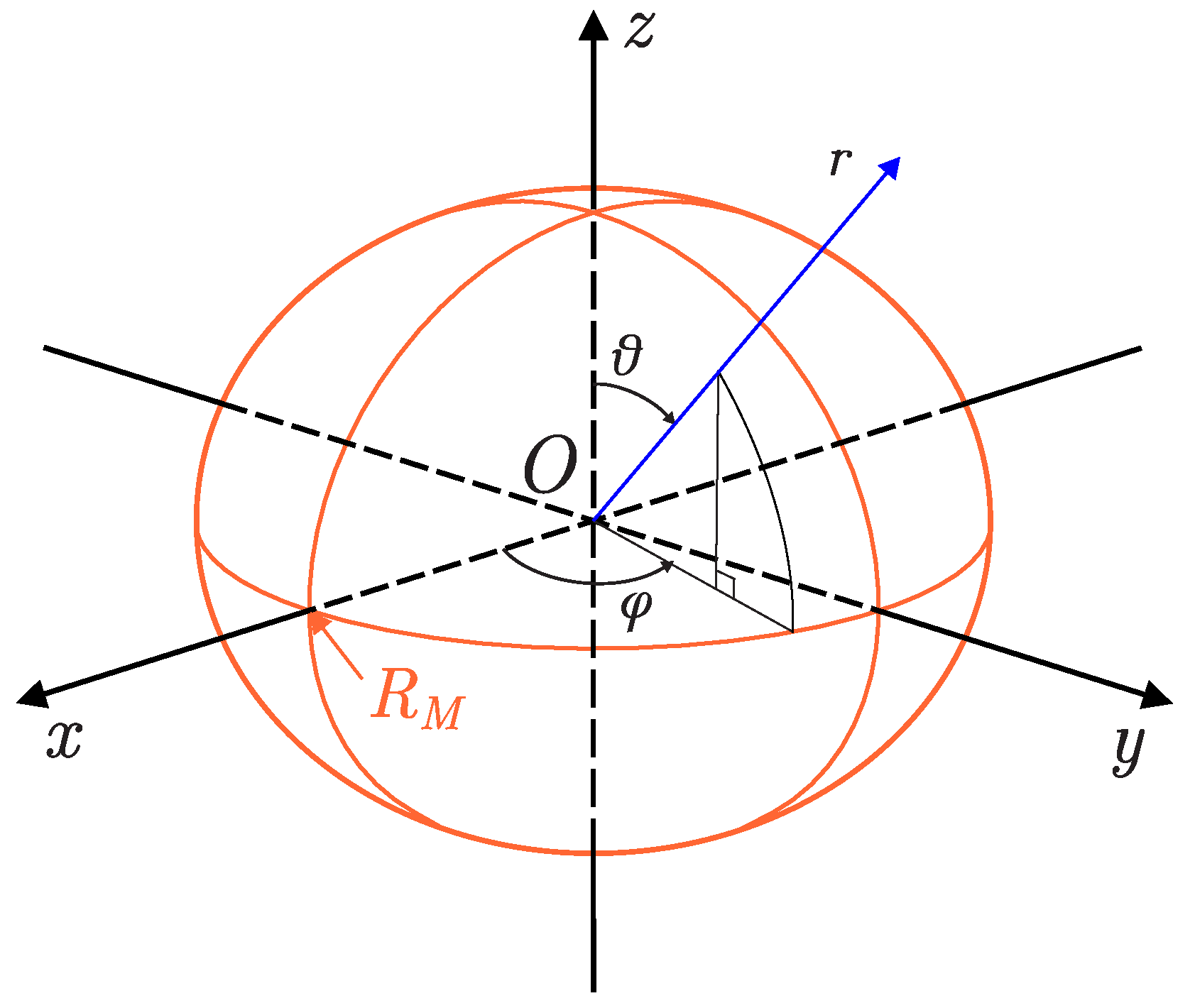
2. Problem Statement
2.1. Separation of the High-Frequency Component of the Internal Field
2.2. Reconstruction of the Near-Surface Magnetic Image of Mercury
3. Separation of the High-Frequency Component of Mercury’s Internal Magnetic Field
- .
- .
- .
4. Recovering of the Near-Surface Magnetic Image of Mercury
- The parameters of the spherical layer are set, in which, according to our assumption, there are magnetic masses that create the same external magnetic field with respect to them as real (unknown) sources.
- The inverse problem of finding the indicated magnetic masses is solved. The integral Equation (2) is discretized, and a system of linear algebraic equations is obtained with an approximately given right side (the right side contains the values of the components of the magnetic induction vector measured via the satellite during orbits near Mercury).
- The field is recalculated to another spherical shell.
- Again, the inverse problem of finding fictitious sources (magnetic masses) is solved.
- The results of the decisions of items 2 and 4 are compared qualitatively, according to the geometric properties of the distributions of magnetic masses. Quantitative comparison is expected to be carried out in the future. Those values of the regularization parameters for which the geometric characteristics are similar are considered admissible by us.
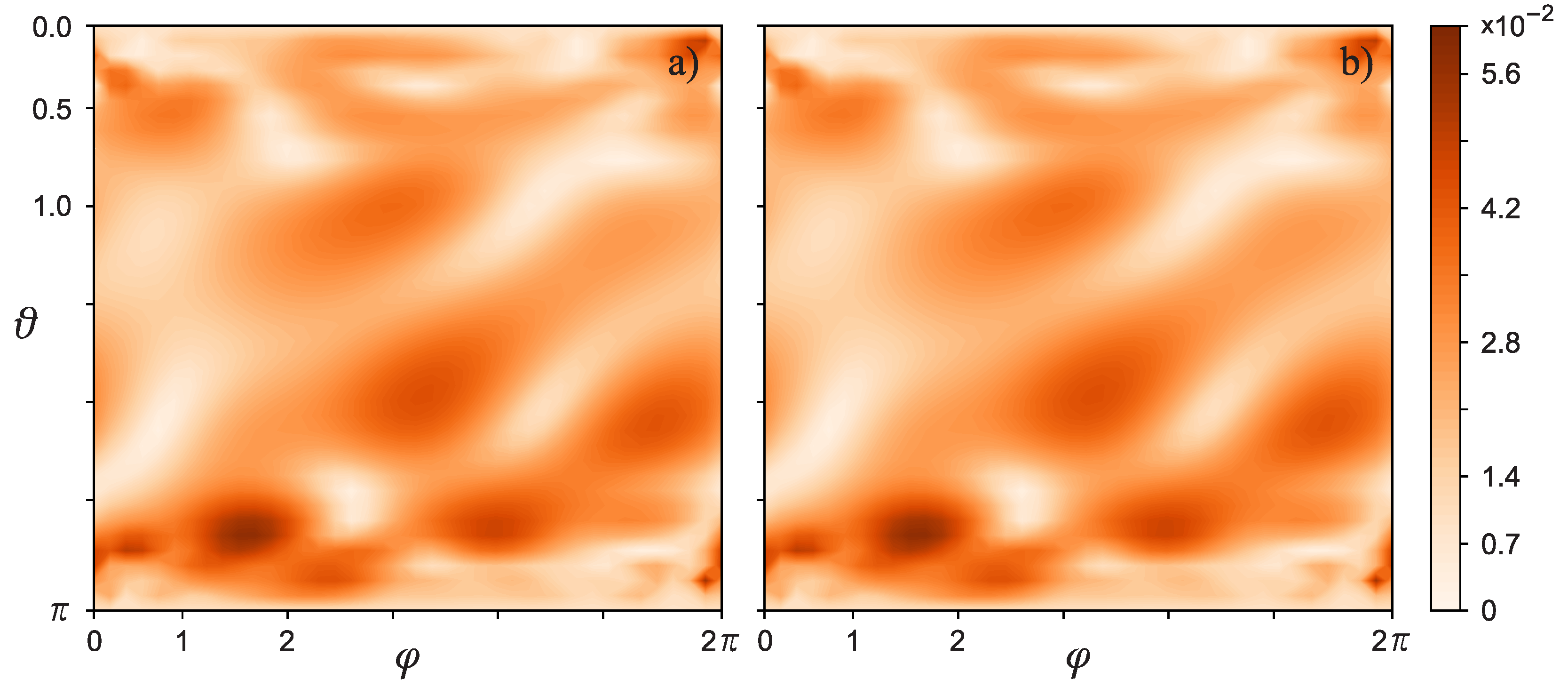
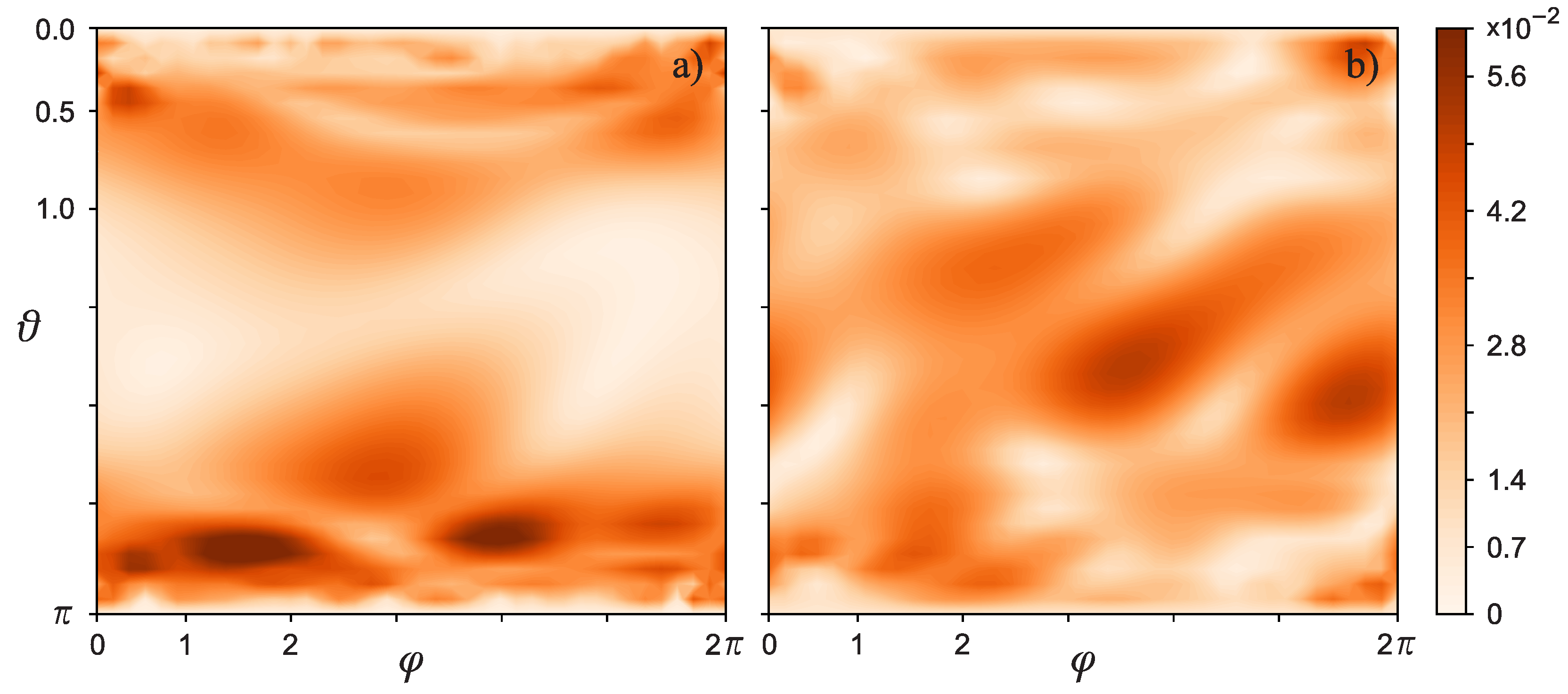
5. Results of Experimental Data Processing
- The magnetic field measurement points are located at an altitude of 10 (km) relative to the surface of Mercury. , where , , (m), = 10,001 (m). The value of the parameter + 10,000 (m).
- The magnetic field measurement points are located at an altitude of 50 (km) relative to the surface of Mercury. , where , , = 49,999 (m), = 50,001 (m). The value of the parameter + 50,000 (m).
- The magnetic field measurement points are located at an altitude of 100 (km) relative to the surface of Mercury. , where , , = 99,999 (m), = 100,001 (m). The value of the parameter + 100,000 (m).
6. Discussion
- It should be emphasized that outside the field sources (i.e., outside the outer liquid core of Mercury and other space objects with an internal magnetic field generator), the method of expanding signals into a series in terms of spherical harmonics, representations in the form of Fourier integrals, wavelets, etc. [38,39], is quite acceptable and can give good results when solving problems of analysis and synthesis. However, modeling of Mercury’s magnetic field adequate to reality cannot be obtained without taking into account magneto-hydrodynamic relations [40,41]. All mathematical models of physical fields must be properly tested: they must guarantee high accuracy in constructing linear field transforms, which include analytic continuation towards sources, higher derivatives of the potential, etc. As is known, the analytical continuation of the signal down, in the direction of singularities, is an ill posed problem and requires the development of efficient regularization algorithms. Otherwise, we get models that do not meet the requirement of adequacy to real physical data. When modeling the magnetic field of the planets of the Solar System, various methods of solving inverse problems were used (see, for example, [42,43]). In the latest work, a model of a continuously magnetized Mars was constructed. However, as we pointed out above, the reconstruction of the sources of physical fields from remote sensing data is an ill posed problem, so a variety of interpretations of satellite information are acceptable. The choice of the most adequate of the existing analytical models of the magnetic field can only be made with a comprehensive analysis of data from various geophysical surveys. Separately, the importance of separating the fields created by carriers occurring at different depths should be noted. Away from the surface of the planet, the “crustal” component of the magnetic field looks like a noise having a small amplitude compared to the time-dependent trend component. Therefore, new effective regularizing algorithms seem to be quite relevant, which allows one to obtain an approximate solution of the inverse problem of magnetic prospecting that is resistant to random noise in the input data.
- It can be noted that the spherical harmonic coefficients describing the distribution of the magnetization within a thin spherical layer can be obtained directly from the coefficients of the lithospheric magnetic field [44]. Of course, the expansion coefficients of the “core” magnetic field in a series of spherical harmonics can be useful for calculating the field values inside a thin spherical shell. We propose an alternative way of finding these coefficients to ensure the stability of the approximate solution of the inverse problem. With the method described in the article for finding the Gaussian coefficients inside a thin shell, more accurate values of the magnetic field in it are obtained: we do not need to look for the field expansion coefficients throughout the entire thickness of the crust. It can be expressed differently: the more “local” the inverse problem is (in our case, the search for expansion coefficients), the better the quality of the regularized solution.
- There are alternative models such as a possible remanent magnetization [45,46] of the crust and non-dipole component of Mercury’s magnetic field generated in the liquid core. These models are able to interpret the results in the context of Mercury’s crustal structure. However, detailed analyses of the structure of the crust and the distributions of magnetic masses found by us in the crust have not yet been carried out—this requires more accurate information about the magnetic field from orbits close to the surface.
7. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Alexeev, I.; Belenkaya, E.; Slavin, J.; Korth, H.; Anderson, B.; Baker, D.; Boardsen, S.; Johnson, C.; Purucker, M.; Sarantos, M.; et al. Mercury’s magnetospheric magnetic field after the first two MESSENGER flybys. Icarus 2010, 209, 23–39. [Google Scholar] [CrossRef]
- Anderson, B.; Acuna, M.; Korth, H.; Purucker, M.; Johnson, C.; Slavin, J.; Solomon, S.; McNutt, R. The structure of Mercury’s magnetic field from MESSENGER’s first flyby. Science 2008, 321, 82–85. [Google Scholar] [CrossRef]
- Anderson, B.; Acuna, M.; Korth, H.; Slavin, J.; Uno, H.; Johnson, C.; Purucker, M.; Solomon, S.; Raines, J.; Zurbuchen, T.; et al. The Magnetic Field of Mercury. Space Sci. Rev. 2010, 152, 307–339. [Google Scholar] [CrossRef]
- Anderson, B.; Johnson, C.; Korth, H.; Purucker, M.; Winslow, R.; Slavin, J.; Solomon, S.; McNutt, R.; Raines, J.; Zurbuchen, T. The Global Magnetic Field of Mercury from MESSENGER Orbital Observations. Science 2011, 333, 1859–1862. [Google Scholar] [CrossRef] [PubMed]
- Anderson, B.; Johnson, C.; Korth, H.; Winslow, R.; Borovsky, J.; Purucker, M.; Slavin, J.; Solomon, S.; Zuber, M.; McNutt, R. Low-degree structure in Mercury’s planetary magnetic field. J. Geophys. Res. 2012, 117. [Google Scholar] [CrossRef]
- Mayhew, M. Inversion of satellite magnetic anomaly data. J. Geophys. 1979, 45, 119–128. [Google Scholar]
- Ness, N.; Behannon, K.; Lepping, R.; Whang, Y.; Schatten, K. Magnetic Field Observations near Mercury: Preliminary Results from Mariner 10. Science 1974, 185, 151–160. [Google Scholar] [CrossRef]
- Ness, N.; Behannon, K.; Lepping, R.; Whang, Y. The magnetic field of Mercury, 1. J. Geophys. Res. 1975, 80, 2708–2716. [Google Scholar] [CrossRef]
- Solomon, S.; McNutt, R.; Gold, R.; Acuna, M.; Baker, D.; Boynton, W.; Chapman, C.; Cheng, A.; Andrew, F.; Gloeckler, G.; et al. The MESSENGER mission to Mercury: Scientific objectives and implementation. Planet. Space Sci. 2001, 49, 1445–1465. [Google Scholar] [CrossRef]
- Philpott, L.; Johnson, C.; Winslow, R.; Anderson, B.; Korth, H.; Purucker, M.; Solomon, S. Constraints on the secular variation of Mercury’s magnetic field from the combined analysis of MESSENGER and Mariner 10 data. Geophys. Res. Lett. 2014, 41, 6627–6634. [Google Scholar] [CrossRef]
- Wicht, J.; Heyner, D. Planetary Geodesy and Remote Sensing; CRC Press: Boca Raton, FL, USA, 2014. [Google Scholar]
- Kolotov, I.; Lukyanenko, D.; Stepanova, I.; Wang, Y.; Yagola, A. Recovering the magnetic image of Mars from satellite observations. J. Imaging 2021, 7, 234. [Google Scholar] [CrossRef]
- Milillo, A.; Fujimoto, M.; Murakami, G.; Benkhoff, J.; Zender, J.; Aizawa, S.; Dosa, M.; Griton, L.; Heyner, D.; Ho, G.; et al. Investigating Mercury’s Environment with the Two-Spacecraft BepiColombo Mission. Earth Planet. Sci. Lett. 2020, 216, 93. [Google Scholar] [CrossRef]
- Plagemann, S. Model of the internal constitution and temperature of the planet Mercury. J. Geophys. Res. 1965, 70, 985–993. [Google Scholar] [CrossRef]
- Smith, D.; Zuber, M.; Phillips, R.; Solomon, S.; Hauck, S.; Lemoine, F.; Mazarico, E.; Neumann, G.; Peale, S.; Margot, J.; et al. Gravity Field and Internal Structure of Mercury from MESSENGER. Science 2012, 336, 214–217. [Google Scholar] [CrossRef] [PubMed]
- Toepfer, S.; Narita, Y.; Glassmeier, K.H.; Heyner, D.; Kolhey, P.; Motschmann, U.; Langlais, B. The Mie representation for Mercury’s magnetic field. Earth Planets Space 2021, 73, 65. [Google Scholar] [CrossRef]
- Langlais, B.; Purucker, M.; Mandea, M. Crustal magnetic field of Mars. J. Geophys. Res. Planets 2004, 109, E02008. [Google Scholar] [CrossRef]
- Oliveira, J.; Langlais, B.; Pais, M.; Amit, H. A modified equivalent source dipole method to model partially distributed magnetic field measurements, with application to Mercury. J. Geophys. Res. Planets 2015, 120, 1075–1094. [Google Scholar] [CrossRef]
- Gudkova, T.; Stepanova, I.; Batov, A. Density anomalies in subsurface layers of Mars: Model estimates for the site of the InSight mission seismometer. Sol. Syst. Res. Vol. 2020, 54, 15–19. [Google Scholar] [CrossRef]
- Pan, L.; Quantin, C.; Tauzin, B.; Michaut, C.; Golombek, M.; Lognonne, P.; Grindrod, P.; Langlais, B.; Gudkova, T.; Stepanova, I.; et al. Crust heterogeneities and structure at the dichotomy boundary in western Elysium Planitia and Implications for InSight lander. Icarus 2020, 338, 113511. [Google Scholar] [CrossRef]
- Johnson, C.; Mittelholz, A.; Langlais, B.; Russell, C.; Ansan, V.; Banfield, D.; Chi, P.; Fillingim, M.; Forget, F.; Haviland, H.; et al. Crustal and time-varying magnetic fields at the InSight landing site on Mars. Nat. Geosci. 2020, 13, 199–204. [Google Scholar] [CrossRef]
- Stepanova, I. On the S-approximation of the Earth’s gravity field. Regional version. Inverse Probl. Sci. Eng. 2009, 16, 1095–1111. [Google Scholar] [CrossRef]
- Stepanova, I.; Shchepetilov, A.; Mikhailov, P. Analytical Models of the Physical Fields of the Earth in Regional Version with Ellipticity. Izv. Phys. Solid Earth 2022, 58, 406–419. [Google Scholar] [CrossRef]
- Stepanova, I.; Kerimov, I.; Raevskiy, D.; Shchepetilov, A. Improving the methods for processing large data in geophysics and geomorphology based on the modified S- and F-approximations. Izv. Phys. Solid Earth 2020, 16, 1095–1111. [Google Scholar] [CrossRef]
- Salnikov, A.; Batov, A.; Gudkova, T.; Stepanova, I. Analysis of the Magnetic Field Data of Mars. 2020. Available online: https://www.elibrary.ru/item.asp?id=45672757 (accessed on 15 February 2023).
- Salnikov, A.; Stepanova, I.; Gudkova, T.; Batov, A. Analytical modeling of the magnetic field of Mars from satellite data using modified S-approximations. Dokl. Earth Sci. 2021, 499, 575–579. [Google Scholar] [CrossRef]
- Lowes, F.; Duka, B. Magnetic multipole moments (Gauss coefficients) and vector potential given by an arbitrary current distribution. Earth Planets Space 2011, 63, i–vi. [Google Scholar] [CrossRef]
- Hood, L.; Oliveira, J.; Galluzzi, V.; Rothery, D. Investigating sources of Mercury’s crustal magnetic field: Further mapping of Messenger magnetometer data. JGR Planets 2018, 123, 2647–2666. [Google Scholar] [CrossRef]
- Lukyanenko, D.; Yagola, A.; Evdokimova, N. Application of inversion methods in solving ill-posed problems for magnetic parameter identification of steel hull vessel. J. Inverse-Ill-Posed Probl. 2011, 18, 1013–1029. [Google Scholar] [CrossRef]
- Lukyanenko, D.; Yagola, A. Some methods for solving of 3d inverse problem of magnetometry. Eurasian J. Math. Comput. Appl. 2016, 4, 4–14. [Google Scholar] [CrossRef]
- Wang, Y.; Lukyanenko, D.; Yagola, A. Magnetic parameters inversion method with full tensor gradient data. Inverse Probl. Imaging 2019, 13, 745–754. [Google Scholar] [CrossRef]
- Wang, Y.; Rong, L.; Qiu, L.; Lukyanenko, D.; Yagola, A. Magnetic susceptibility inversion method with full tensor gradient data using low temperature SQUIDs. Pet. Sci. 2019, 16, 794–807. [Google Scholar] [CrossRef]
- Wang, Y.; Kolotov, I.; Lukyanenko, D.; Yagola, A. Reconstruction of magnetic susceptibility using full magnetic gradient data. Comput. Math. Math. Phys. 2020, 60, 1000–1007. [Google Scholar] [CrossRef]
- Wang, Y.; Leonov, A.; Lukyanenko, D.; Yagola, A. General Tikhonov regularization with applications in geoscience. CSIAM Trans. Appl. Math. 2020, 1, 53–85. [Google Scholar] [CrossRef]
- Kolotov, I.; Lukyanenko, D.; Stepanova, I.; Wang, Y.; Yagola, A. Recovering the magnetic properties of Mercury from satellite observations. Eurasian J. Math. Comput. Appl. 2022, 10, 26–41. [Google Scholar] [CrossRef]
- Toepfer, S.; Oertel, I.; Schiron, V.; Narita, Y.; Glassmeier, K.H.; Heyner, D.; Kolhey, P.; Motschmann, U. Reconstruction of Mercury’s internal magnetic field beyond the octupole. Ann. Geophys. 2022, 40, 91–105. [Google Scholar] [CrossRef]
- Tikhonov, A.; Goncharsky, A.; Stepanov, V.; Yagola, A. Numerical Methods for the Solution of Ill-Posed Problems; Kluwer Academic Publishers: Dordrecht, The Netherlands, 1995. [Google Scholar]
- Frick, P.; Sokoloff, D.; Stepanov, R. Wavelets for the space-time structure analysis of physical fields. Phys. Uspekhi 2020, 65, 62. [Google Scholar] [CrossRef]
- Kazantsev, S.; Kardakov, V. Poloidal-Toroidal Decomposition of Solenoidal Vector Fields in the Ball. J. Appl. Ind. Math. 2019, 13, 480–499. [Google Scholar] [CrossRef]
- Reshetnyak, M. Spatial Spectra of the geomagnetic Field in the Observations and Geodynamo Models. Izv. Phys. Solid Earth 2015, 51, 354–361. [Google Scholar] [CrossRef]
- Reshetnyak, M. Inverse problem in Parker’s dynamo. Russ. J. Earth Sci. 2015, 15, ES4001. [Google Scholar] [CrossRef]
- Uno, H.; Anderson, B.; Korth, H.; Johnson, C.; Solomon, S. Modeling Mercury’s internal magnetic field with smooth inversions. Earth Planet. Sci. Lett. 2009, 285, 328–339. [Google Scholar] [CrossRef]
- Whaler, K.; Purucker, M. A spatially continuous magnetization model for Mars. Earth Planet. Sci. Lett. 2005, 110. [Google Scholar] [CrossRef]
- Gubbins, D.; Ivers, D.; Masterton, S.M.; Winch, D.E. Analysis of lithospheric magnetization in vector spherical harmonics. Geophys. J. Int. 2011, 187, 99–117. [Google Scholar] [CrossRef]
- Johnson, C.L.; Purucker, M.E.; Korth, H.; Anderson, B.J.; Winslow, R.M.; Al Asad, M.M.H.; Slavin, J.A.; Alexeev, I.I.; Phillips, R.J.; Zuber, M.T.; et al. MESSENGER observations of Mercury’s magnetic field structure. J. Geophys. Res. Planets 2012, 117. [Google Scholar] [CrossRef]
- Winslow, R.M.; Anderson, B.J.; Johnson, C.L.; Slavin, J.A.; Korth, H.; Purucker, M.E.; Baker, D.N.; Solomon, S.C. Mercury’s magnetopause and bow shock from MESSENGER Magnetometer observations. J. Geophys. Res. Space Phys. 2013, 118, 2213–2227. [Google Scholar] [CrossRef]
- Voevodin, V.; Antonov, A.; Nikitenko, D.; Shvets, P.; Sobolev, S.; Sidorov, I.; Stefanov, K.; Voevodin, V.; Zhumatiy, S. Supercomputer Lomonosov-2: Large Scale, Deep Monitoring and Fine Analytics for the User Community. Supercomput. Front. Innov. 2019, 6, 4–11. [Google Scholar]


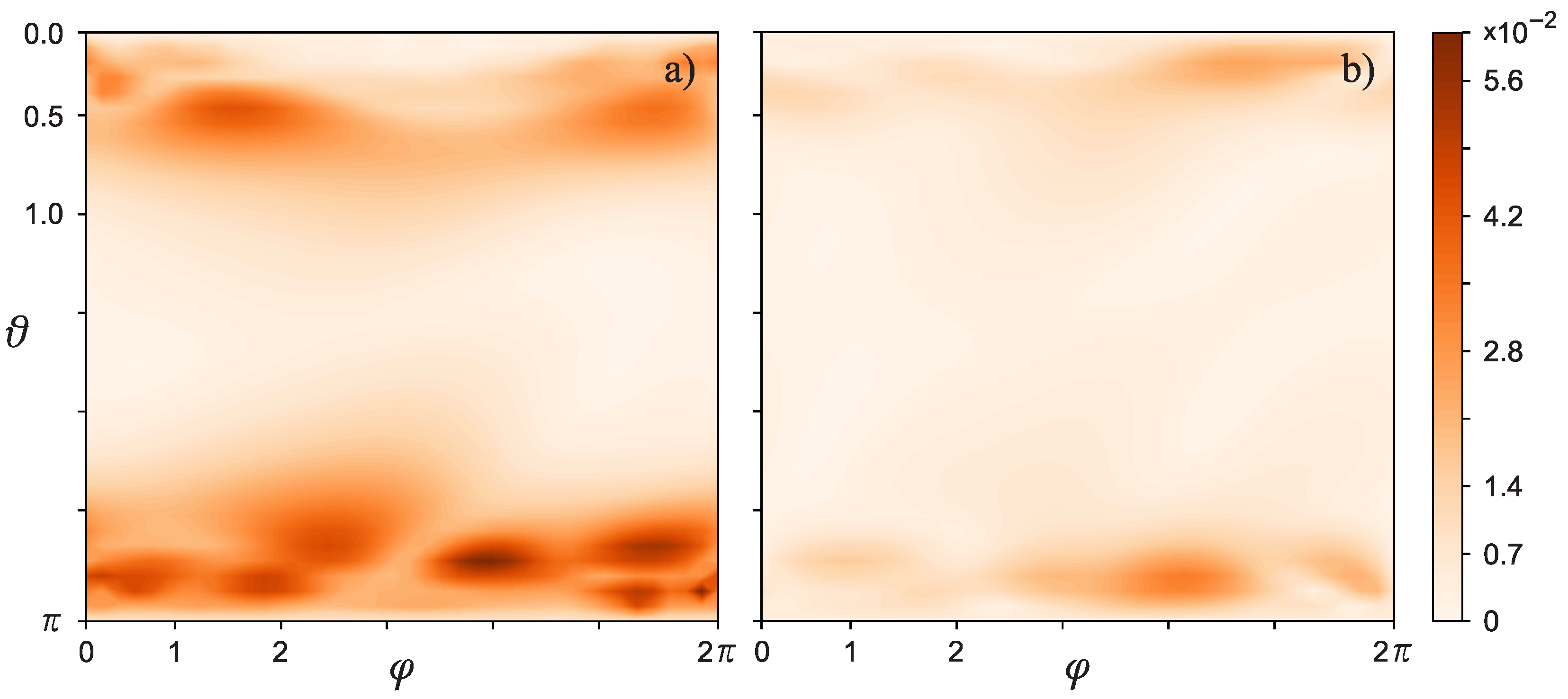

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kolotov, I.; Lukyanenko, D.; Stepanova, I.; Wang, Y.; Yagola, A. Recovering the Near-Surface Magnetic Image of Mercury from Satellite Observations. Remote Sens. 2023, 15, 2125. https://doi.org/10.3390/rs15082125
Kolotov I, Lukyanenko D, Stepanova I, Wang Y, Yagola A. Recovering the Near-Surface Magnetic Image of Mercury from Satellite Observations. Remote Sensing. 2023; 15(8):2125. https://doi.org/10.3390/rs15082125
Chicago/Turabian StyleKolotov, Igor, Dmitry Lukyanenko, Inna Stepanova, Yanfei Wang, and Anatoly Yagola. 2023. "Recovering the Near-Surface Magnetic Image of Mercury from Satellite Observations" Remote Sensing 15, no. 8: 2125. https://doi.org/10.3390/rs15082125
APA StyleKolotov, I., Lukyanenko, D., Stepanova, I., Wang, Y., & Yagola, A. (2023). Recovering the Near-Surface Magnetic Image of Mercury from Satellite Observations. Remote Sensing, 15(8), 2125. https://doi.org/10.3390/rs15082125






