Decadal Quasi-2-Day Wave Observations in the Equatorial Mesopause Region by a Meteor Radar over Kototabang (0.2°S, 100.3°E) and TIMED/TIDI and Comparison with Quasi-2-Day Wave Observations at Mid-Latitudes
Abstract
1. Introduction
2. Materials and Methods
2.1. Meteor Radar
2.2. TIDI
2.3. Methodology
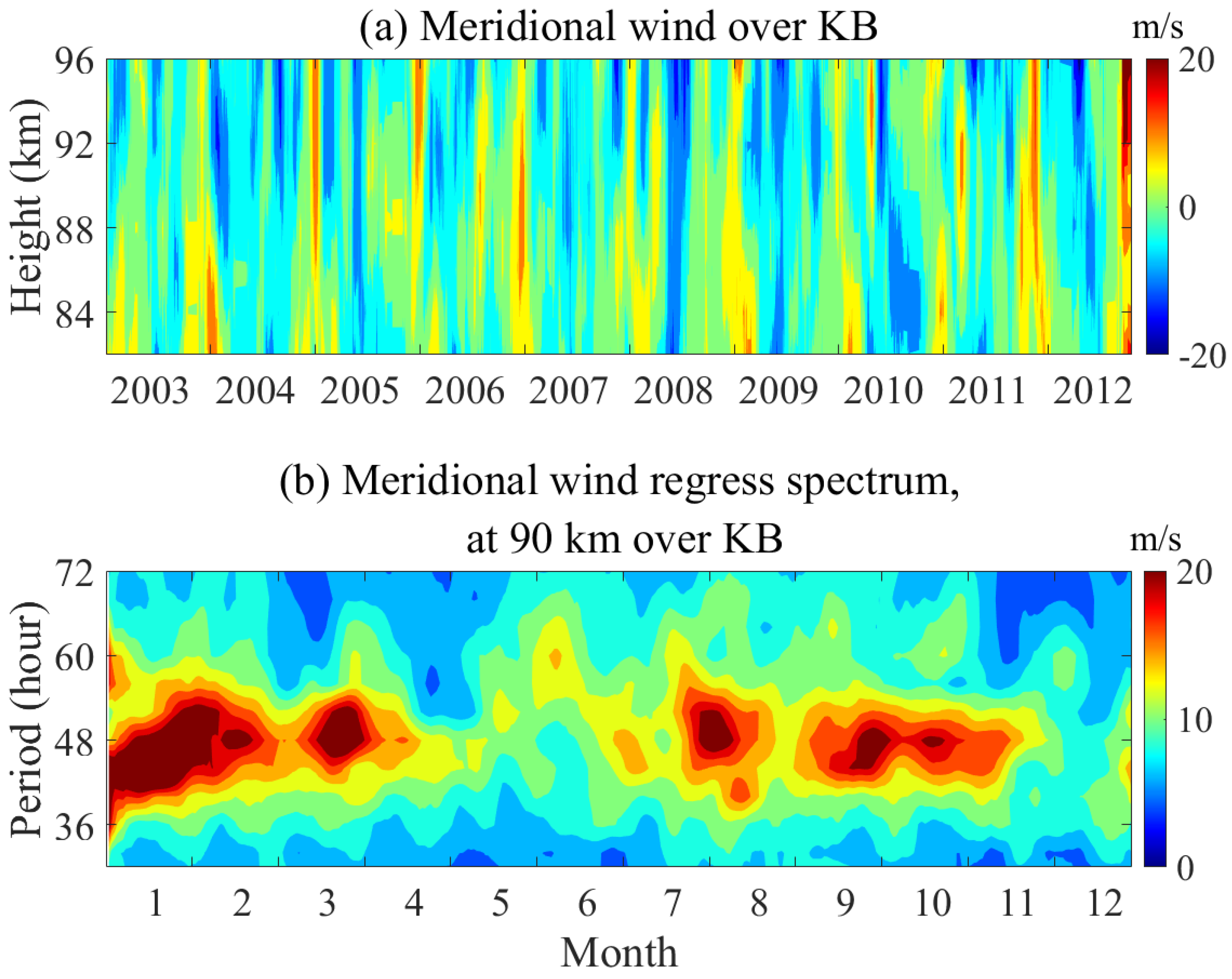
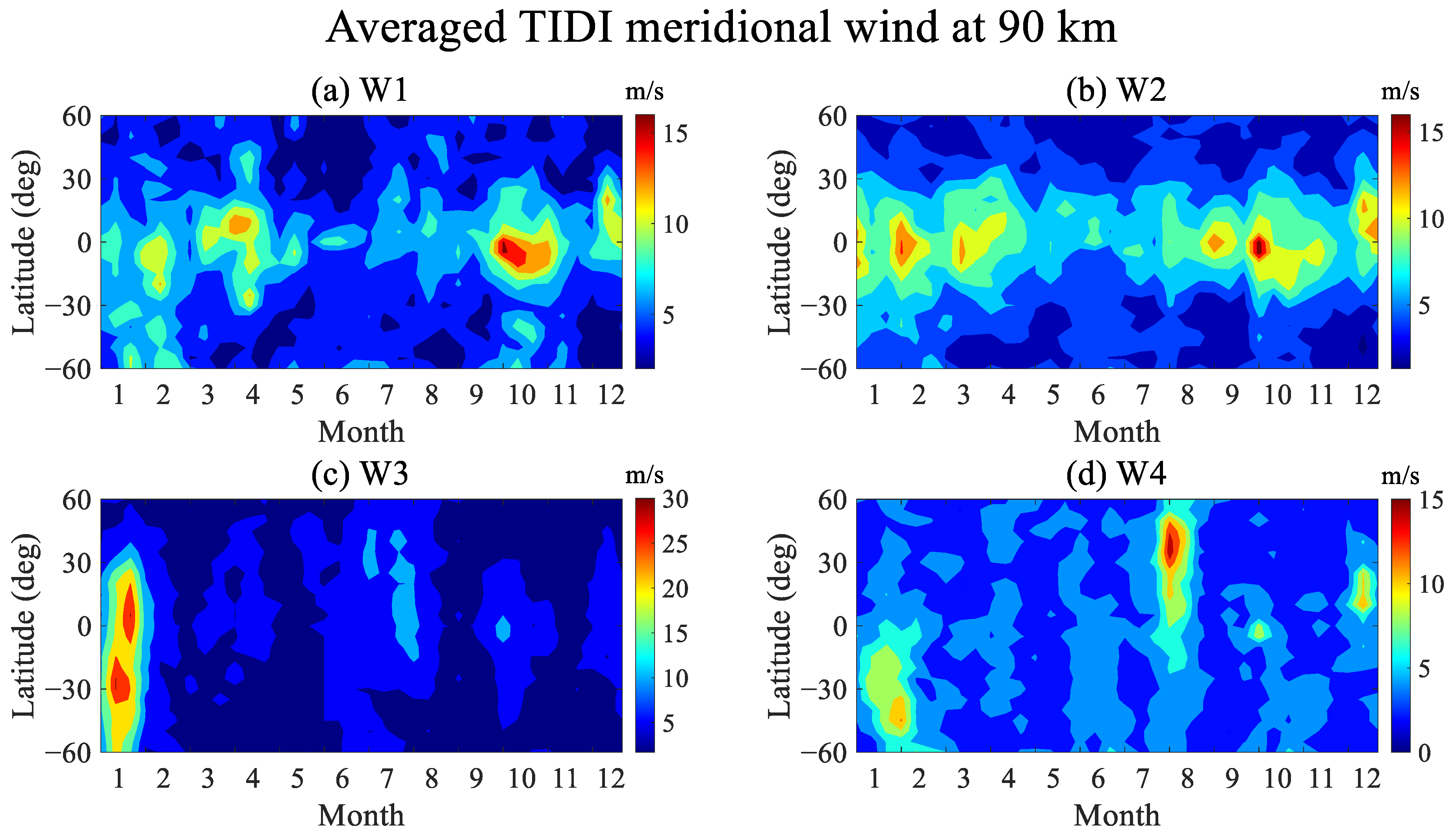
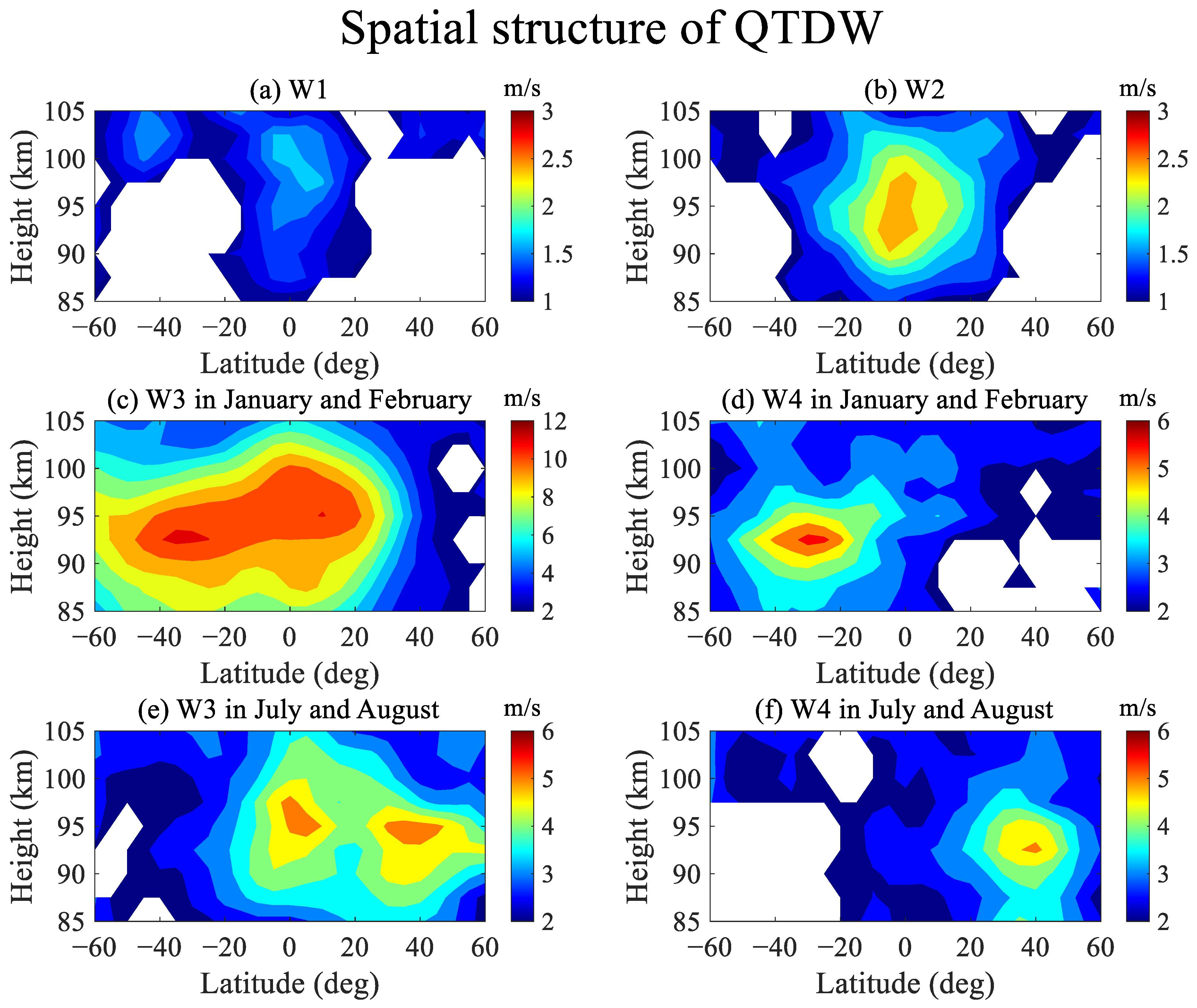
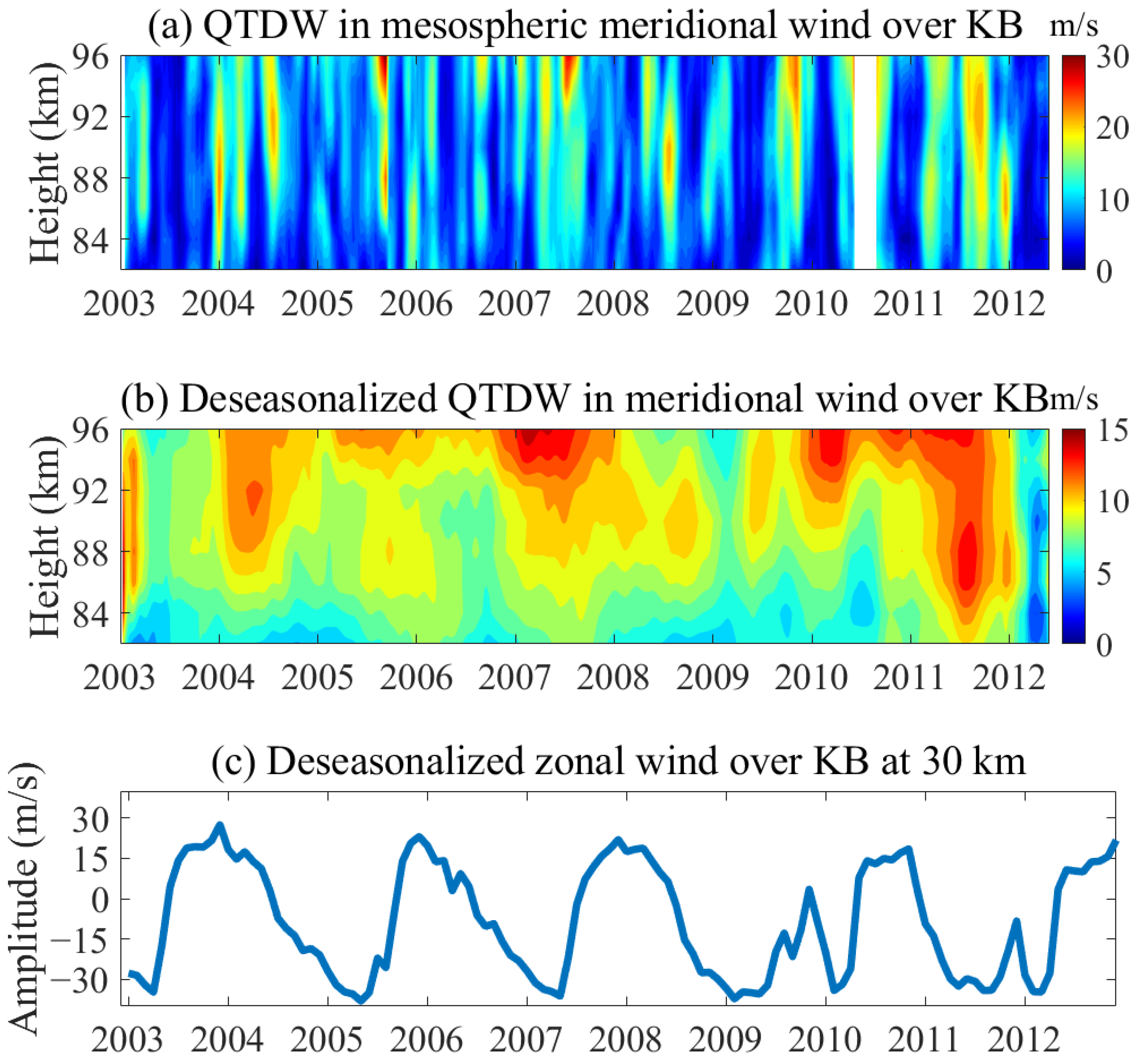
3. Results
3.1. Statistics on the Seasonal Features
3.2. QBO Influence
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Yue, J.; Wang, W.; Ruan, H.; Chang, L.C.; Lei, J. Impact of the interaction between the quasi-2 day wave and tides on the ionosphere and thermosphere. J. Geophys. Res. Space Phys. 2016, 121, 3555–3563. [Google Scholar] [CrossRef]
- Fröhlich, K.; Pogoreltsev, A.; Jacobi, C. Numerical simulation of tides, Rossby and Kelvin waves with the COMMA-LIM model. Adv. Space Res. 2003, 32, 863–868. [Google Scholar] [CrossRef]
- Chang, L.C.; Palo, S.E.; Liu, H.L. Short-term variability in the migrating diurnal tide caused by interactions with the quasi 2 day wave. J. Geophys. Res. Atmos. 2011, 116, D12112. [Google Scholar] [CrossRef]
- Yang, C.; Li, T.; Lai, D.; Wang, X.; Xue, X.; Dou, X. The Delayed Response of the Troposphere-Stratosphere-Mesosphere Coupling to the 2019 Southern SSW. Geophys. Res. Lett. 2022, 49, e2022GL101759. [Google Scholar] [CrossRef]
- Muller, H.G. A discussion on D and E region winds over Europe-Long-period meteor wind oscillations. Philos. Trans. R. Soc. London Ser. A Math. Phys. Sci. 1972, 271, 585–599. [Google Scholar] [CrossRef]
- Kumar, K.K.; Subrahmanyam, K.V.; Mathew, S.S.; Koushik, N.; Ramkumar, G. Simultaneous observations of the quasi 2-day wave climatology over the low and equatorial latitudes in the mesosphere lower thermosphere. Clim. Dyn. 2018, 51, 221–233. [Google Scholar] [CrossRef]
- Lilienthal, F.; Jacobi, C. Meteor radar quasi 2-day wave observations over 10 years at Collm (51.3°N, 13.0°E). Atmos. Chem. Phys. 2015, 15, 9917–9927. [Google Scholar] [CrossRef]
- Pancheva, D.; Mitchell, N.J.; Manson, A.H.; Meek, C.E.; Jacobi, C.; Portnyagin, Y.; Merzlyakov, E.; Hocking, W.K.; MacDougall, J.; Singer, W.; et al. Variability of the quasi-2-day wave observed in the MLT region during the PSMOS campaign of June–August 1999. J. Atmos. Sol. -Terr. Phys. 2004, 66, 539–565. [Google Scholar] [CrossRef]
- Yue, J.; Liu, H.-L.; Chang, L.C. Numerical investigation of the quasi 2 day wave in the mesosphere and lower thermosphere. J. Geophys. Res. Atmos. 2012, 117, D05111. [Google Scholar] [CrossRef]
- Yang, C.; Ren, B.; Li, G.; Zheng, J. Change of the wintertime SSTA variability over the West Pacific after the mid-1980s: Effect of the increasing El Niño Modoki. J. Geophys. Res. Atmos. 2014, 119, 5204–5225. [Google Scholar] [CrossRef]
- Harris, T.J.; Vincent, R.A. The quasi-two-day wave observed in the equatorial middle atmosphere. J. Geophys. Res. Atmos. 1993, 98, 10481–10490. [Google Scholar] [CrossRef]
- Rao, N.V.; Ratnam, M.V.; Vedavathi, C.; Tsuda, T.; Murthy, B.V.K.; Sathishkumar, S.; Gurubaran, S.; Kumar, K.K.; Subrahmanyam, K.V.; Rao, S.V.B. Seasonal, inter-annual and solar cycle variability of the quasi two day wave in the low-latitude mesosphere and lower thermosphere. J. Atmos. Sol. -Terr. Phys. 2017, 152–153, 20–29. [Google Scholar] [CrossRef]
- Lima, L.M.; Medeiros, A.F.; Buriti, R.A.; Batista, P.P.; Clemesha, B.R.; Takahashi, H. Mesospheric 2-Day waves observed simultaneously in the equatorial and low latitudes regions of Brazil. Rev. Bras. Geofís. 2007, 25, 43–48. [Google Scholar] [CrossRef]
- Jacobi, C.; Hoffmann, P.; Kürschner, D. Trends in MLT region winds and planetary waves, Collm (52°N, 15°E). Ann. Geophys. 2008, 26, 1221–1232. [Google Scholar] [CrossRef]
- Nozawa, S.; Imaida, S.; Brekke, A.; Hall, C.M.; Manson, A.; Meek, C.; Oyama, S.; Dobashi, K.; Fujii, R. The quasi 2-day wave observed in the polar mesosphere. J. Geophys. Res. Atmos. 2003, 108, ACL 3-1–ACL 3-12. [Google Scholar] [CrossRef]
- Araújo, L.R.; Lima, L.M.; Batista, P.P.; Clemesha, B.R.; Takahashi, H. Planetary wave seasonality from meteor wind measurements at 7.4°S and 22.7°S. Ann. Geophys. 2014, 32, 519–531. [Google Scholar] [CrossRef]
- Rodgers, C.; Prata, A.J. Evidence for a traveling two-day wave in the middle atmosphere. J. Geophys. Res. Atmos. 1981, 86, 9661–9664. [Google Scholar] [CrossRef]
- Wu, D.; Hays, P.; Skinner, W.; Marshall, A.; Burrage, M.; Lieberman, R.; Ortland, D.A. Observations of the quasi 2-day wave from the High Resolution Doppler Imager on UARS. Geophys. Res. Lett. 1993, 20, 2853–2856. [Google Scholar] [CrossRef]
- Yang, C.; Li, T.; Smith, A.K.; Dou, X. The response of the Southern Hemisphere middle atmosphere to the Madden–Julian oscillation during austral winter using the Specified-Dynamics Whole Atmosphere Community Climate Model. J. Clim. 2017, 30, 8317–8333. [Google Scholar] [CrossRef]
- Palo, S.E.; Roble, R.G.; Hagan, M. Middle atmosphere effects of the quasi-two-day wave determined from a General Circulation Model. Earth Planets Space 1999, 51, 629–647. [Google Scholar] [CrossRef]
- Gu, S.-Y.; Li, T.; Dou, X.; Wu, Q.; Mlynczak, M.G.; Russell III, J.M. Observations of Quasi-Two-Day wave by TIMED/SABER and TIMED/TIDI. J. Geophys. Res. Atmos. 2013, 118, 1624–1639. [Google Scholar] [CrossRef]
- Tunbridge, V.M.; Sandford, D.J.; Mitchell, N.J. Zonal wave numbers of the summertime 2 day planetary wave observed in the mesosphere by EOS Aura Microwave Limb Sounder. J. Geophys. Res. Atmos. 2011, 116, D11103. [Google Scholar] [CrossRef]
- Huang, Y.Y.; Zhang, S.D.; Yi, F.; Huang, C.M.; Huang, K.M.; Gan, Q.; Gong, Y. Global climatological variability of quasi-two-day waves revealed by TIMED/SABER observations. Ann. Geophys. 2013, 31, 1061–1075. [Google Scholar] [CrossRef]
- Pancheva, D.; Mukhtarov, P.; Siskind, D.E. Climatology of the quasi-2-day waves observed in the MLS/Aura measurements (2005–2014). J. Atmos. Sol. -Terr. Phys. 2018, 171, 210–224. [Google Scholar] [CrossRef]
- Garcia, R.R.; Lieberman, R.; Russell, J.M.; Mlynczak, M.G. Large-scale waves in the mesosphere and lower thermosphere observed by SABER. J. Atmos. Sci. 2005, 62, 4384–4399. [Google Scholar] [CrossRef]
- Liu, G.; England, S.L.; Janches, D. Quasi Two-, Three-, and Six-Day Planetary-Scale Wave Oscillations in the Upper Atmosphere Observed by TIMED/SABER Over ~17 Years During 2002–2018. J. Geophys. Res. Space Phys. 2019, 124, 9462–9474. [Google Scholar] [CrossRef]
- Gu, S.-Y.; Dou, X.-K.; Yang, C.-Y.; Jia, M.; Huang, K.-M.; Huang, C.-M.; Zhang, S.-D. Climatology and Anomaly of the Quasi-Two-Day Wave Behaviors During 2003–2018 Austral Summer Periods. J. Geophys. Res. Space Phys. 2019, 124, 544–556. [Google Scholar] [CrossRef]
- Gu, S.Y.; Liu, H.L.; Pedatella, N.; Dou, X.; Li, T.; Chen, T. The quasi 2 day wave activities during 2007 austral summer period as revealed by Whole Atmosphere Community Climate Model. J. Geophys. Res. Space Phys. 2016, 121, 2743–2754. [Google Scholar] [CrossRef]
- Madhavi, G.; Kishore, P.; Rao, S.; Velicogna, I.; Basha, G. Two-day wave observations over the middle and high latitudes in the NH and SH using COSMIC GPSRO measurements. Adv. Space Res. 2015, 55, 722–731. [Google Scholar] [CrossRef]
- Merzlyakov, E.; Jacobi, C. Quasi-two-day wave in an unstable summer atmosphere-some numerical results on excitation and propagation. Ann. Geophys. 2004, 22, 1917–1929. [Google Scholar] [CrossRef]
- Pancheva, D.; Mukhtarov, P.; Siskind, D.E.; Smith, A.K. Global distribution and variability of quasi 2 day waves based on the NOGAPS-ALPHA reanalysis model. J. Geophys. Res. Space Phys. 2016, 121, 11422–411449. [Google Scholar] [CrossRef]
- Andrews, D.G.; Holton, J.R.; Leovy, C.B. Middle Atmosphere Dynamics; Academic Press: Cambridge, MA, USA, 1987. [Google Scholar] [CrossRef]
- Salby, M.L. The 2-day wave in the middle atmosphere: Observations and theory. J. Geophys. Res. Ocean. 1981, 86, 9654–9660. [Google Scholar] [CrossRef]
- Plumb, R.A. Baroclinic instability of the summer mesosphere: A mechanism for the quasi-two-day wave? J. Atmos. Sci. 1983, 40, 262–270. [Google Scholar] [CrossRef]
- Li, T.; She, C.-Y.; Palo, S.E.; Wu, Q.; Liu, H.-L.; Salby, M.L. Coordinated lidar and TIMED observations of the quasi-two-day wave during August 2002–2004 and possible quasi-biennial oscillation influence. Adv. Space Res. 2008, 41, 1463–1471. [Google Scholar] [CrossRef]
- Yang, C.; Smith, A.K.; Li, T.; Dou, X. The effect of the Madden-Julian oscillation on the mesospheric migrating diurnal tide: A study using SD-WACCM. Geophys. Res. Lett. 2018, 45, 5105–5114. [Google Scholar] [CrossRef]
- Guharay, A.; Batista, P.; Andrioli, V. Study of solar cycle dependence of the quasi-two-day wave in the MLT from an extratropical station. J. Earth Syst. Sci. 2020, 129, 38. [Google Scholar] [CrossRef]
- Batubara, M.; Suryana, R.; Manik, T.; Sitompul, P. Kototabang—West Sumatera meteor radar: System design and initial results of a large scale meteor echo. In Proceedings of the 2011 6th International Conference on Telecommunication Systems, Services, and Applications (TSSA), Denpasar, Indonesia, 20–21 October 2011; pp. 17–21. [Google Scholar] [CrossRef]
- Matsumoto, N.; Shinbori, A.; Riggin, D.M.; Tsuda, T. Measurement of momentum flux using two meteor radars in Indonesia. Ann. Geophys. 2016, 34, 369–377. [Google Scholar] [CrossRef]
- Jia, M.; Xue, X.; Gu, S.; Chen, T.; Ning, B.; Wu, J.; Zeng, X.; Dou, X. Multiyear Observations of Gravity Wave Momentum Fluxes in the Midlatitude Mesosphere and Lower Thermosphere Region by Meteor Radar. J. Geophys. Res. Space Phys. 2018, 123, 5684–5703. [Google Scholar] [CrossRef]
- Killeen, T.L.; Wu, Q.; Solomon, S.C.; Ortland, D.A.; Skinner, W.R.; Niciejewski, R.J.; Gell, D.A. TIMED Doppler Interferometer: Overview and recent results. J. Geophys. Res. Space Phys. 2006, 111, A10S01. [Google Scholar] [CrossRef]
- Dhadly, M.S.; Englert, C.R.; Drob, D.P.; Emmert, J.T.; Niciejewski, R.; Zawdie, K.A. Comparison of ICON/MIGHTI and TIMED/TIDI Neutral Wind Measurements in the Lower Thermosphere. J. Geophys. Res. Space Phys. 2021, 126, e2021JA029904. [Google Scholar] [CrossRef]
- Oberheide, J.; Wu, Q.; Killeen, T.; Hagan, M.; Roble, R.G. A climatology of nonmigrating semidiurnal tides from TIMED Doppler Interferometer (TIDI) wind data. J. Atmos. Sol. -Terr. Phys. 2007, 69, 2203–2218. [Google Scholar] [CrossRef]
- Wu, D.L.; Hays, P.B.; Skinner, W.R. A Least Squares Method for Spectral Analysis of Space-Time Series. J. Atmos. Sci. 1995, 52, 3501–3511. [Google Scholar] [CrossRef]
- Yang, C.; Li, T.; Xue, X.; Gu, S.Y.; Yu, C.; Dou, X. Response of the northern stratosphere to the Madden-Julian oscillation during boreal winter. J. Atmos. Sci. 2019, 124, 5314–5331. [Google Scholar] [CrossRef]
- Merzlyakov, E.; Pancheva, D.; Mitchell, N.; Forbes, J.; Portnyagin, Y.I.; Palo, S.; Makarov, N.; Muller, H. High-and mid-latitude quasi-2-day waves observed simultaneouslyby four meteor radars during summer 2000. Ann. Geophys. 2004, 22, 773–788. [Google Scholar] [CrossRef]
- Ern, M.; Preusse, P.; Kalisch, S.; Kaufmann, M.; Riese, M. Role of gravity waves in the forcing of quasi two-day waves in the mesosphere: An observational study. J. Geophys. Res. Atmos. 2013, 118, 3467–3485. [Google Scholar] [CrossRef]
- Wu, D.L.; Schwartz, M.J.; Waters, J.W.; Limpasuvan, V.; Wu, Q.; Killeen, T.L. Mesospheric Doppler wind measurements from aura microwave limb sounder (MLS). Adv. Space Res. 2008, 42, 1246–1252. [Google Scholar] [CrossRef]
- Koval, A.V.; Gavrilov, N.M.; Didenko, K.A.; Ermakova, T.; Kandieva, K. Numerical simulation of stratospheric QBO impact on the meridional circulation up to the thermosphere. Sci. Rep. 2022, 44, 882. [Google Scholar] [CrossRef]




Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sun, R.; Gu, S.-Y.; Dou, X.; Wei, Y.; Qin, Y.; Yang, Z. Decadal Quasi-2-Day Wave Observations in the Equatorial Mesopause Region by a Meteor Radar over Kototabang (0.2°S, 100.3°E) and TIMED/TIDI and Comparison with Quasi-2-Day Wave Observations at Mid-Latitudes. Remote Sens. 2023, 15, 1122. https://doi.org/10.3390/rs15041122
Sun R, Gu S-Y, Dou X, Wei Y, Qin Y, Yang Z. Decadal Quasi-2-Day Wave Observations in the Equatorial Mesopause Region by a Meteor Radar over Kototabang (0.2°S, 100.3°E) and TIMED/TIDI and Comparison with Quasi-2-Day Wave Observations at Mid-Latitudes. Remote Sensing. 2023; 15(4):1122. https://doi.org/10.3390/rs15041122
Chicago/Turabian StyleSun, Ruidi, Sheng-Yang Gu, Xiankang Dou, Yafei Wei, Yusong Qin, and Zhenlin Yang. 2023. "Decadal Quasi-2-Day Wave Observations in the Equatorial Mesopause Region by a Meteor Radar over Kototabang (0.2°S, 100.3°E) and TIMED/TIDI and Comparison with Quasi-2-Day Wave Observations at Mid-Latitudes" Remote Sensing 15, no. 4: 1122. https://doi.org/10.3390/rs15041122
APA StyleSun, R., Gu, S.-Y., Dou, X., Wei, Y., Qin, Y., & Yang, Z. (2023). Decadal Quasi-2-Day Wave Observations in the Equatorial Mesopause Region by a Meteor Radar over Kototabang (0.2°S, 100.3°E) and TIMED/TIDI and Comparison with Quasi-2-Day Wave Observations at Mid-Latitudes. Remote Sensing, 15(4), 1122. https://doi.org/10.3390/rs15041122






