Euphotic Zone Depth Anomaly in Global Mesoscale Eddies by Multi-Mission Fusion Data
Abstract
1. Introduction
2. Materials and Methods
2.1. ZEU Datasets
2.2. Eddy Datasets
2.3. ZEU Data Preprocessing
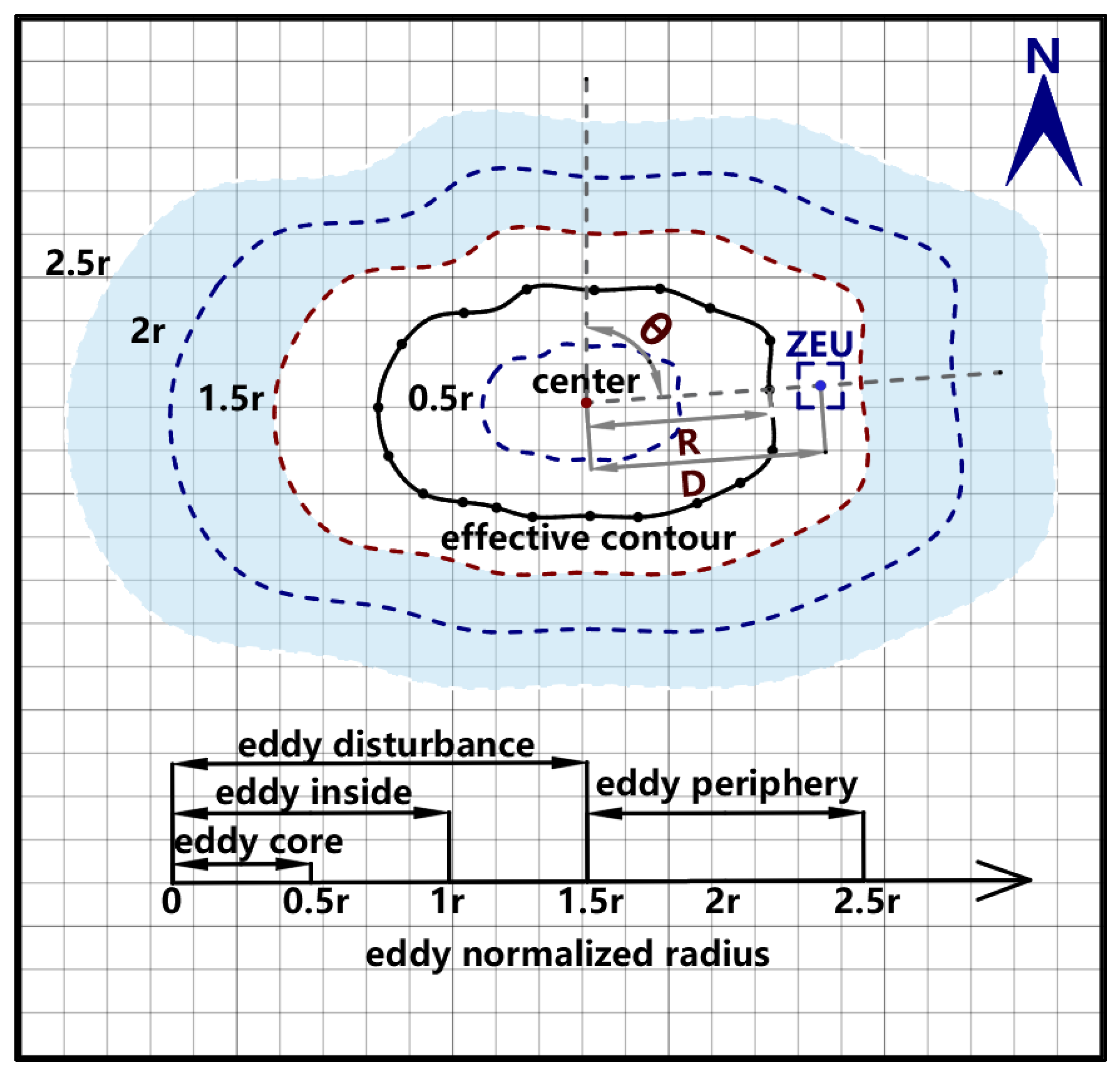
2.4. Effective Eddy Matching Using the ZEU Data
2.5. Anomalies of ZEU
3. Results
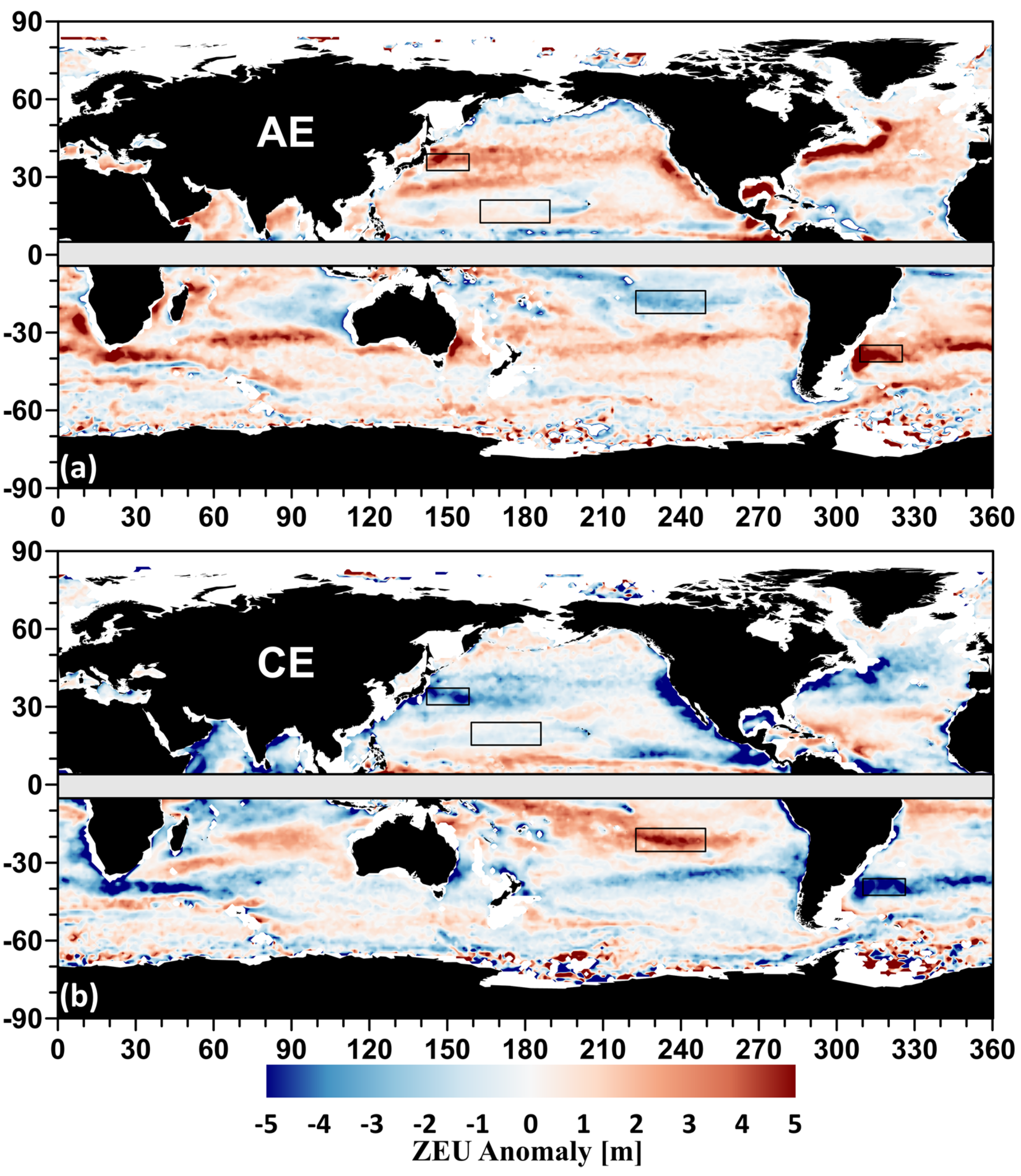
3.1. Global Distribution of the ZEU′
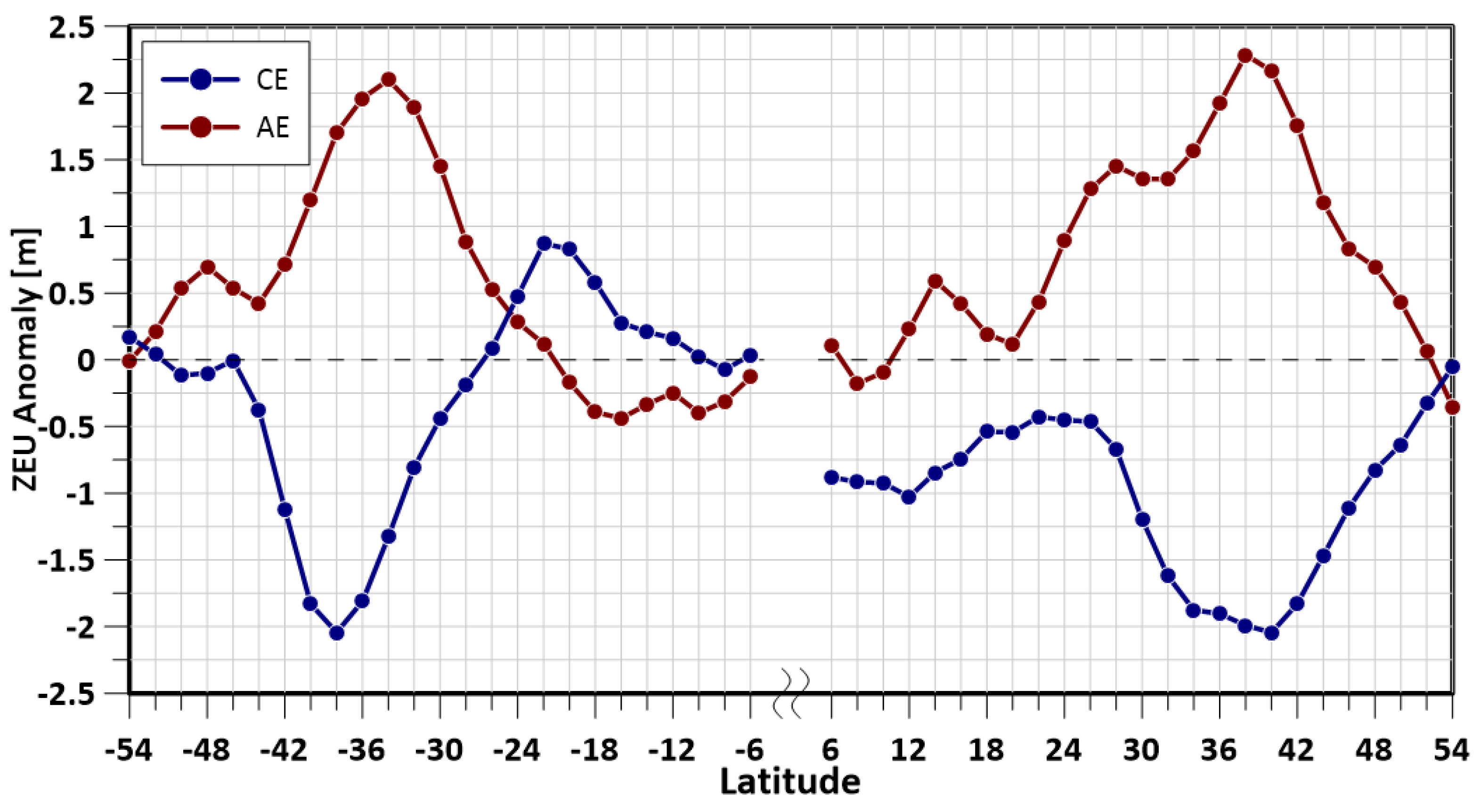
3.2. Zonal Variation of ZEU′
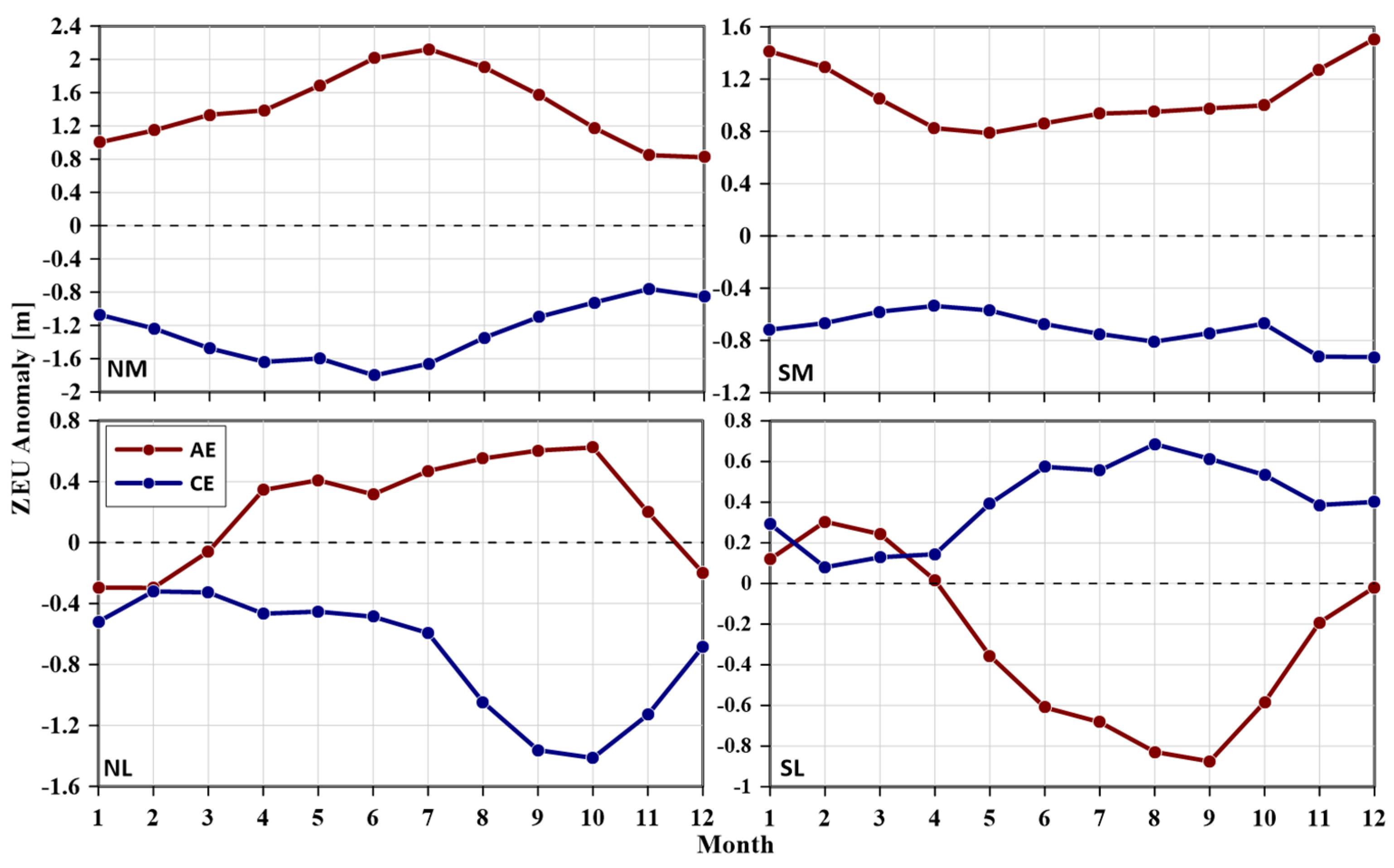
3.3. Seasonal Variation of the ZEU’
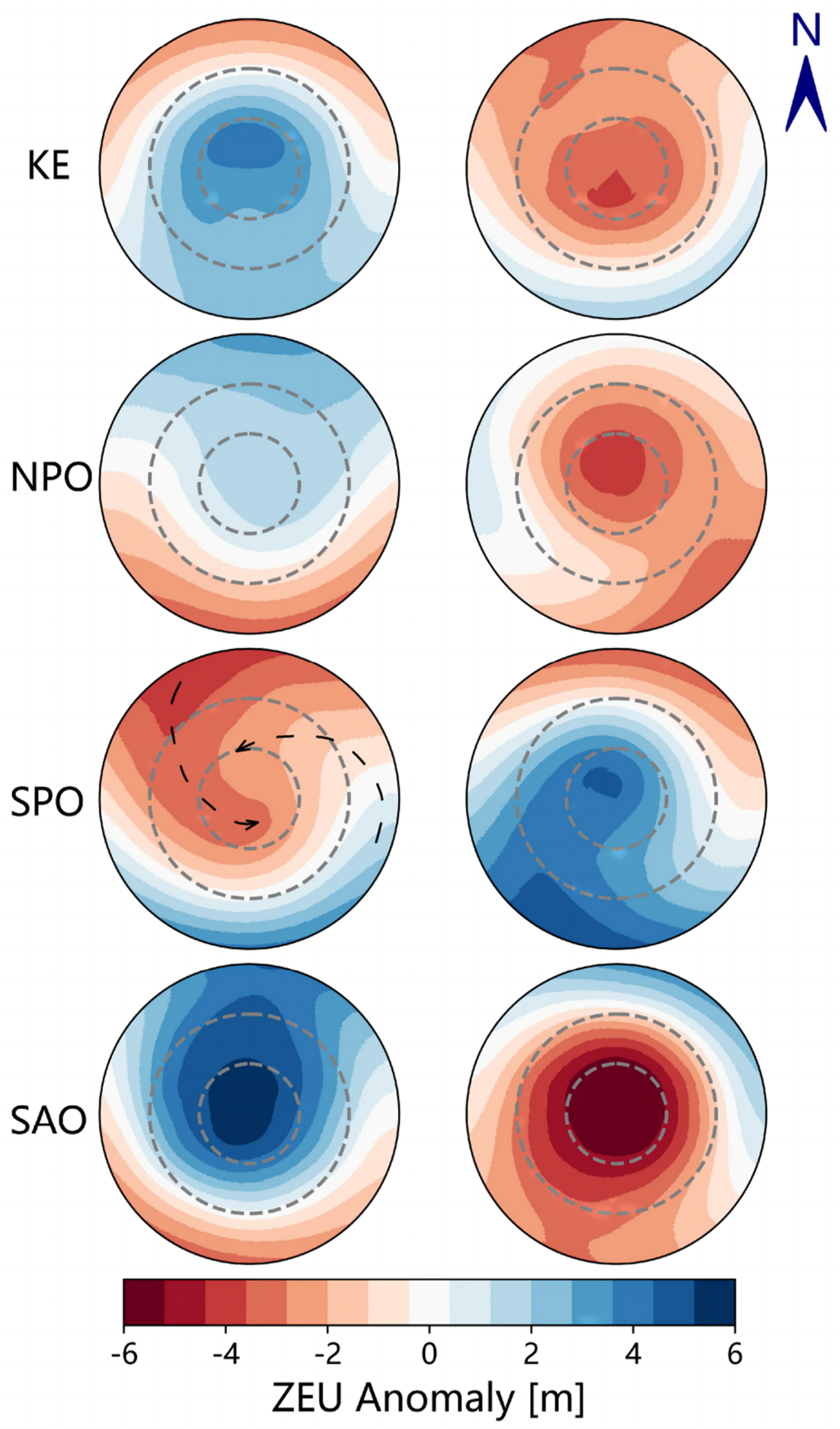
3.4. Variation of ZEU′ with Normalized Radius in a Two-Dimensional Eddy Coordinate System
3.5. Variation of ZEU′ with Normalized Radius in Eddy Coordinate System
3.6. ZEU′ Quantization in Eddy
4. Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Kirk, J.T.O. Light and Photosynthesis in Aquatic Ecosystems; 2019/10/09 ed.; Cambridge University Press: Cambridge, UK, 1994; Volume 74, p. 987. [Google Scholar]
- Behrenfeld, M.J.; Falkowski, P.G. A consumer’s guide to phytoplankton primary productivity models. Limnol. Oceanogr. 1997, 42, 1479–1491. [Google Scholar] [CrossRef]
- Behrenfeld, M.J.; Falkowski, P.G. Photosynthetic rates derived from satellite-based chlorophyll concentration. Limnol. Oceanogr. 1997, 42, 1–20. [Google Scholar] [CrossRef]
- Shang, S.L.; Lee, Z.P.; Wei, G.M. Characterization of MODIS-derived euphotic zone depth: Results for the China Sea. Remote Sens. Environ. 2011, 115, 180–186. [Google Scholar] [CrossRef]
- Behrenfeld, M.J.; Gaube, P.; Della Penna, A.; O’Malley, R.T.; Burt, W.J.; Hu, Y.; Bontempi, P.S.; Steinberg, D.K.; Boss, E.S.; Siegel, D.A.; et al. Global satellite-observed daily vertical migrations of ocean animals. Nature 2019, 576, 257–261. [Google Scholar] [CrossRef] [PubMed]
- Khanna, D.R.; Bhutiani, R.; Chandra, K.S. Effect of the Euphotic Depth and Mixing Depth on Phytoplanktonic Growth Mechanism. Int. J. Environ. Res. 2009, 3, 223–228. [Google Scholar]
- Falkowski, P.G. The role of phytoplankton photosynthesis in global biogeochemical cycles. Photosynth. Res. 1994, 39, 235–258. [Google Scholar] [CrossRef]
- Wu, J.; Lee, Z.; Xie, Y.; Goes, J.; Shang, S.; Marra, J.F.; Lin, G.; Yang, L.; Huang, B. Reconciling Between Optical and Biological Determinants of the Euphotic Zone Depth. J. Geophys. Res. Ocean. 2021, 126, e2020JC016874. [Google Scholar] [CrossRef]
- Morel, A.; Huot, Y.; Gentili, B.; Werdell, P.J.; Hooker, S.B.; Franz, B.A. Examining the consistency of products derived from various ocean color sensors in open ocean (Case 1) waters in the perspective of a multi-sensor approach. Remote Sens. Environ. 2007, 111, 69–88. [Google Scholar] [CrossRef]
- Mueller, J.L.; Lange, R.E. Bio-optical provinces of the Northeast Pacific Ocean: A provisional analysis. Limnol. Oceanogr. 2003, 34, 1572–1586. [Google Scholar] [CrossRef]
- Lee, Z.; Weidemann, A.; Kindle, J.; Arnone, R.; Carder, K.L.; Davis, C. Euphotic zone depth: Its derivation and implication to ocean-color remote sensing. J. Geophys. Res.-Ocean. 2007, 112, C03009. [Google Scholar] [CrossRef]
- Kratzer, S.; Hakansson, B.; Sahlin, C. Assessing Secchi and photic zone depth in the Baltic Sea from satellite data. Ambio 2003, 32, 577–585. [Google Scholar] [CrossRef]
- Zhang, Z.; Tian, J.; Qiu, B.; Zhao, W.; Chang, P.; Wu, D.; Wan, X. Observed 3D Structure, Generation, and Dissipation of Oceanic Mesoscale Eddies in the South China Sea. Sci. Rep. 2016, 6, 24349. [Google Scholar] [CrossRef]
- Danabasoglu, G.; McWilliams, J.C.; Gent, P.R. The role of mesoscale tracer transports in the global ocean circulation. Science 1994, 264, 1123–1126. [Google Scholar] [CrossRef] [PubMed]
- Zhao, D.D.; Xu, Y.S.; Zhang, X.G.; Huang, C. Global chlorophyll distribution induced by mesoscale eddies. Remote Sens. Environ. 2021, 254, 112245. [Google Scholar] [CrossRef]
- Gaube, P.; Chelton, D.B.; Strutton, P.G.; Behrenfeld, M.J. Satellite observations of chlorophyll, phytoplankton biomass, and Ekman pumping in nonlinear mesoscale eddies. J. Geophys. Res. -Ocean. 2013, 118, 6349–6370. [Google Scholar] [CrossRef]
- Dufois, F.; Hardman-Mountford, N.J.; Greenwood, J.; Richardson, A.J.; Feng, M.; Matear, R.J. Anticyclonic eddies are more productive than cyclonic eddies in subtropical gyres because of winter mixing. Sci. Adv. 2016, 2, e1600282. [Google Scholar] [CrossRef]
- He, Q.Y.; Zhan, H.G.; Cai, S.Q.; Zhan, W.K. Eddy-Induced Near-Surface Chlorophyll Anomalies in the Subtropical Gyres: Biomass or Physiology? Geophys. Res. Lett. 2021, 48, e2020GL091975. [Google Scholar] [CrossRef]
- Benitez-Nelson, C.R.; Bidigare, R.R.; Dickey, T.D.; Landry, M.R.; Leonard, C.L.; Brown, S.L.; Nencioli, F.; Rii, Y.M.; Maiti, K.; Becker, J.W.; et al. Mesoscale eddies drive increased silica export in the subtropical Pacific Ocean. Science 2007, 316, 1017–1021. [Google Scholar] [CrossRef]
- Dawson, H.R.S.; Strutton, P.G.; Gaube, P. The Unusual Surface Chlorophyll Signatures of Southern Ocean Eddies. J. Geophys. Res.-Ocean. 2018, 123, 6053–6069. [Google Scholar] [CrossRef]
- Frenger, I.; Munnich, M.; Gruber, N. Imprint of Southern Ocean mesoscale eddies on chlorophyll. Biogeosciences 2018, 15, 4781–4798. [Google Scholar] [CrossRef]
- Cornec, M.; Laxenaire, R.; Speich, S.; Claustre, H. Impact of Mesoscale Eddies on Deep Chlorophyll Maxima. Geophys. Res. Lett. 2021, 48, e2021GL093470. [Google Scholar] [CrossRef]
- Gaube, P.; Braun, C.D.; Lawson, G.L.; McGillicuddy, D.J., Jr.; Penna, A.D.; Skomal, G.B.; Fischer, C.; Thorrold, S.R. Mesoscale eddies influence the movements of mature female white sharks in the Gulf Stream and Sargasso Sea. Sci. Rep. 2018, 8, 7363. [Google Scholar] [CrossRef]
- McGillicuddy, D.J., Jr.; Anderson, L.A.; Bates, N.R.; Bibby, T.; Buesseler, K.O.; Carlson, C.A.; Davis, C.S.; Ewart, C.; Falkowski, P.G.; Goldthwait, S.A.; et al. Eddy/wind interactions stimulate extraordinary mid-ocean plankton blooms. Science 2007, 316, 1021–1026. [Google Scholar] [CrossRef]
- Chow, C.H.; Shih, Y.Y.; Chien, Y.T.; Chen, J.Y.; Fan, N.; Wu, W.C.; Hung, C.C. The Wind Effect on Biogeochemistry in Eddy Cores in the Northern South China Sea. Front. Mar. Sci. 2021, 8, 717576. [Google Scholar] [CrossRef]
- Arostegui, M.C.; Gaube, P.; Woodworth-Jefcoats, P.A.; Kobayashi, D.R.; Braun, C.D. Anticyclonic eddies aggregate pelagic predators in a subtropical gyre. Nature 2022, 609, 535–540. [Google Scholar] [CrossRef] [PubMed]
- Fanton, O.; Mangin, A.; Lavender, S.; Antoine, D.; Maritorena, S.; Morel, A.; Barrot, G.; Demaria, J.; Pinnock, S. GlobColour-the European Service for Ocean Colour. In Proceedings of the 2009 IEEE International Geoscience & Remote Sensing Symposium (IGARSS), Cape Town, South Africa, 12–17 July 2009. [Google Scholar]
- Maritorena, S.; d’Andon, O.H.F.; Mangin, A.; Siegel, D.A. Merged satellite ocean color data products using a bio-optical model: Characteristics, benefits and issues. Remote Sens. Environ. 2010, 114, 1791–1804. [Google Scholar] [CrossRef]
- Schlax, M.G.; Chelton, D.B. The “Growing Method” of Eddy Identification and Tracking in Two and Three Dimensions; College of Earth, Ocean and Atmospheric Sciences, Oregon State University: Corvallis, OR, USA, 2016; Volume 8. [Google Scholar]
- Pujol, M.I.; Faugere, Y.; Taburet, G.; Dupuy, S.; Pelloquin, C.; Ablain, M.; Picot, N. DUACS DT2014: The new multi-mission altimeter data set reprocessed over 20 years. Ocean Sci. 2016, 12, 1067–1090. [Google Scholar] [CrossRef]
- Mason, E.; Pascual, A.; McWilliams, J.C. A New Sea Surface Height-Based Code for Oceanic Mesoscale Eddy Tracking. J. Atmos. Ocean. Tech. 2014, 31, 1181–1188. [Google Scholar] [CrossRef]
- Pegliasco, C.; Delepoulle, A.; Mason, E.; Morrow, R.; Faugere, Y.; Dibarboure, G. META3.1exp: A new global mesoscale eddy trajectory atlas derived from altimetry. Earth Syst. Sci. Data 2022, 14, 1087–1107. [Google Scholar] [CrossRef]
- Amante, C.; Eakins, B.W. ETOPO1 1 Arc-Minute Global Relief Model: Procedures, Data Sources and Analysis. In NOAA Technical Memorandum NESDIS NGDC-24; National Geophysical Data Center, NOAA: Boulder, CO, USA, 2009. [Google Scholar]
- McGillicuddy, D.J., Jr. Mechanisms of Physical-Biological-Biogeochemical Interaction at the Oceanic Mesoscale. Ann. Rev. Mar. Sci. 2016, 8, 125–159. [Google Scholar] [CrossRef]
- McGillicuddy, D.J.; Robinson, A.R. Eddy-induced nutrient supply and new production in the Sargasso Sea. Deep-Sea Res. Part I 1997, 44, 1427–1450. [Google Scholar] [CrossRef]
- Chelton, D.B.; Gaube, P.; Schlax, M.G.; Early, J.J.; Samelson, R.M. The influence of nonlinear mesoscale eddies on near-surface oceanic chlorophyll. Science 2011, 334, 328–332. [Google Scholar] [CrossRef] [PubMed]
- Dufois, F.; Hardman-Mountford, N.J.; Greenwood, J.; Richardson, A.J.; Feng, M.; Herbette, S.; Matear, R. Impact of eddies on surface chlorophyll in the South Indian Ocean. J. Geophys. Res.-Ocean. 2014, 119, 8061–8077. [Google Scholar] [CrossRef]
- Dewar, W.K.; Flierl, G.R. Some Effects of the Wind on Rings. J. Phys. Oceanogr. 1987, 17, 1653–1667. [Google Scholar] [CrossRef]
- Travis, S.; Qiu, B. Seasonal Reversal of the Near-Surface Chlorophyll Response to the Presence of Mesoscale Eddies in the South Pacific Subtropical Countercurrent. J. Geophys. Res.-Ocean. 2020, 125, e2019JC015752. [Google Scholar] [CrossRef]
- Gaube, P.; McGillicuddy, D.J.; Moulin, A.J. Mesoscale Eddies Modulate Mixed Layer Depth Globally. Geophys. Res. Lett. 2019, 46, 1505–1512. [Google Scholar] [CrossRef]
- Gaube, P.; McGillicuddy, D.J.; Chelton, D.B.; Behrenfeld, M.J.; Strutton, P.G. Regional variations in the influence of mesoscale eddies on near-surface chlorophyll. J. Geophys. Res.-Ocean. 2014, 119, 8195–8220. [Google Scholar] [CrossRef]
- Guo, M.X.; Xiu, P.; Chai, F.; Xue, H.J. Mesoscale and Submesoscale Contributions to High Sea Surface Chlorophyll in Subtropical Gyres. Geophys. Res. Lett. 2019, 46, 13217–13226. [Google Scholar] [CrossRef]
- Chelton, D.B.; Schlax, M.G.; Samelson, R.M.; de Szoeke, R.A. Global observations of large oceanic eddies. Geophys. Res. Lett. 2007, 34, L15606. [Google Scholar] [CrossRef]
- Martínez-Moreno, J.; Hogg, A.M.; England, M.H.; Constantinou, N.C.; Kiss, A.E.; Morrison, A.K. Global changes in oceanic mesoscale currents over the satellite altimetry record. Nat. Clim. Chang. 2021, 11, 397–403. [Google Scholar] [CrossRef]


| Raw Data | Processed Data | |
|---|---|---|
| MODIS | 15.61% | 28.90% |
| Multi-sensor fusion | 61.23% | 74.49% |
| AE | AE Means | AE Maximum/Latitude | CE | CE Means | CE Maximum/Latitude | |||
|---|---|---|---|---|---|---|---|---|
| SL | − | −0.26 | 0.51 | −0.44/16°S | + | 0.32 | −0.26 | +0.87/22°S |
| SM | + | 0.94 | 2.10/34°S | − | −0.59 | −2.04/38°S | ||
| NL | + | 0.20 | 0.83 | 0.59/14°N | − | −0.76 | −1.01 | −1.03/12°N |
| NM | + | 1.18 | 2.29/38°N | − | −1.15 | −2.05/40°N | ||
| AE Maximum | AE | CE Maximum | CE | |
|---|---|---|---|---|
| SL | 0 | ~1.5r | ~0.5r | >2.0r |
| SM | ~0.2r | ~1.6r | ~0.2r | ~1.8r |
| NL | ~0.2r | 1.1r | ~0.2r | ~1.5r |
| NM | ~0.2r | 1.7r | ~0.2r | ~1.7r |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, Y.; Yang, J.; Chen, G. Euphotic Zone Depth Anomaly in Global Mesoscale Eddies by Multi-Mission Fusion Data. Remote Sens. 2023, 15, 1062. https://doi.org/10.3390/rs15041062
Wang Y, Yang J, Chen G. Euphotic Zone Depth Anomaly in Global Mesoscale Eddies by Multi-Mission Fusion Data. Remote Sensing. 2023; 15(4):1062. https://doi.org/10.3390/rs15041062
Chicago/Turabian StyleWang, Yan, Jie Yang, and Ge Chen. 2023. "Euphotic Zone Depth Anomaly in Global Mesoscale Eddies by Multi-Mission Fusion Data" Remote Sensing 15, no. 4: 1062. https://doi.org/10.3390/rs15041062
APA StyleWang, Y., Yang, J., & Chen, G. (2023). Euphotic Zone Depth Anomaly in Global Mesoscale Eddies by Multi-Mission Fusion Data. Remote Sensing, 15(4), 1062. https://doi.org/10.3390/rs15041062






