Atmospheric Gravity Wave Derived from the Neutral Wind with 5-Minute Resolution Routinely Retrieved by the Meteor Radar at Mohe
Abstract
1. Introduction
2. Data and Methodology
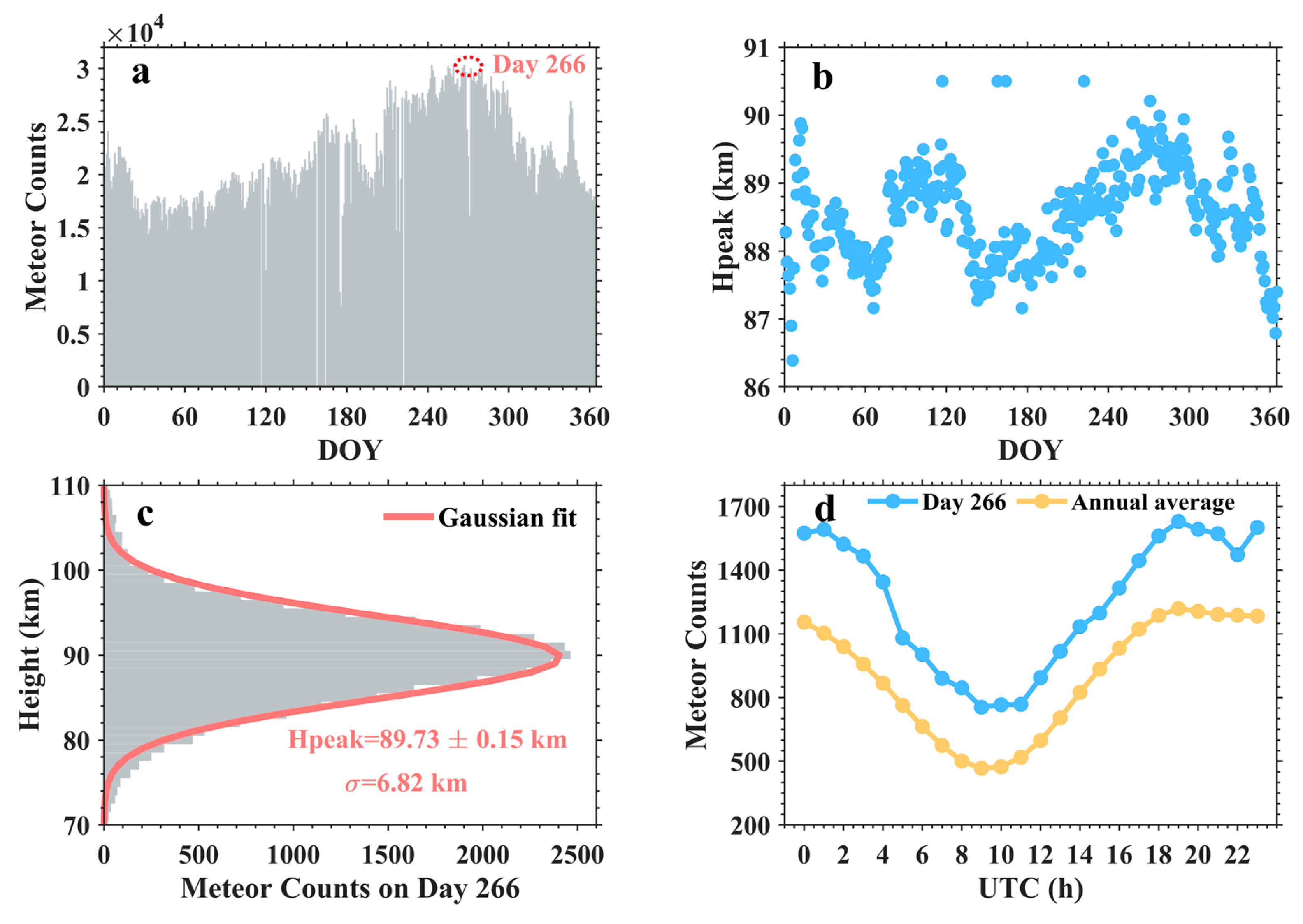
2.1. Meteor Data Description
2.2. Least Square Algorithm Method
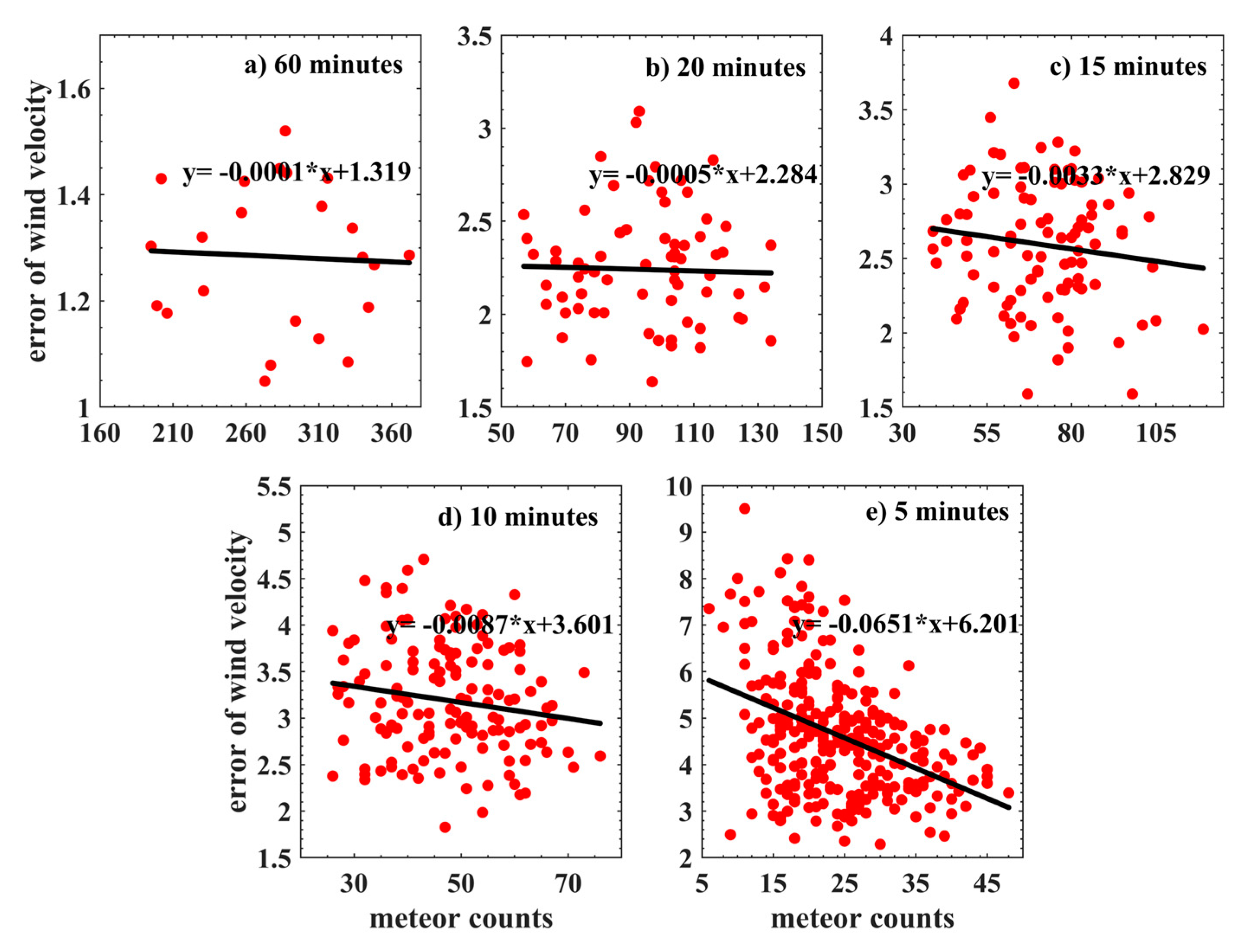
2.3. Error for Estimating the Meteor Wind
2.4. GW Kinetic Energy in the MLT Region
2.5. GW Total Energy in the Troposphere
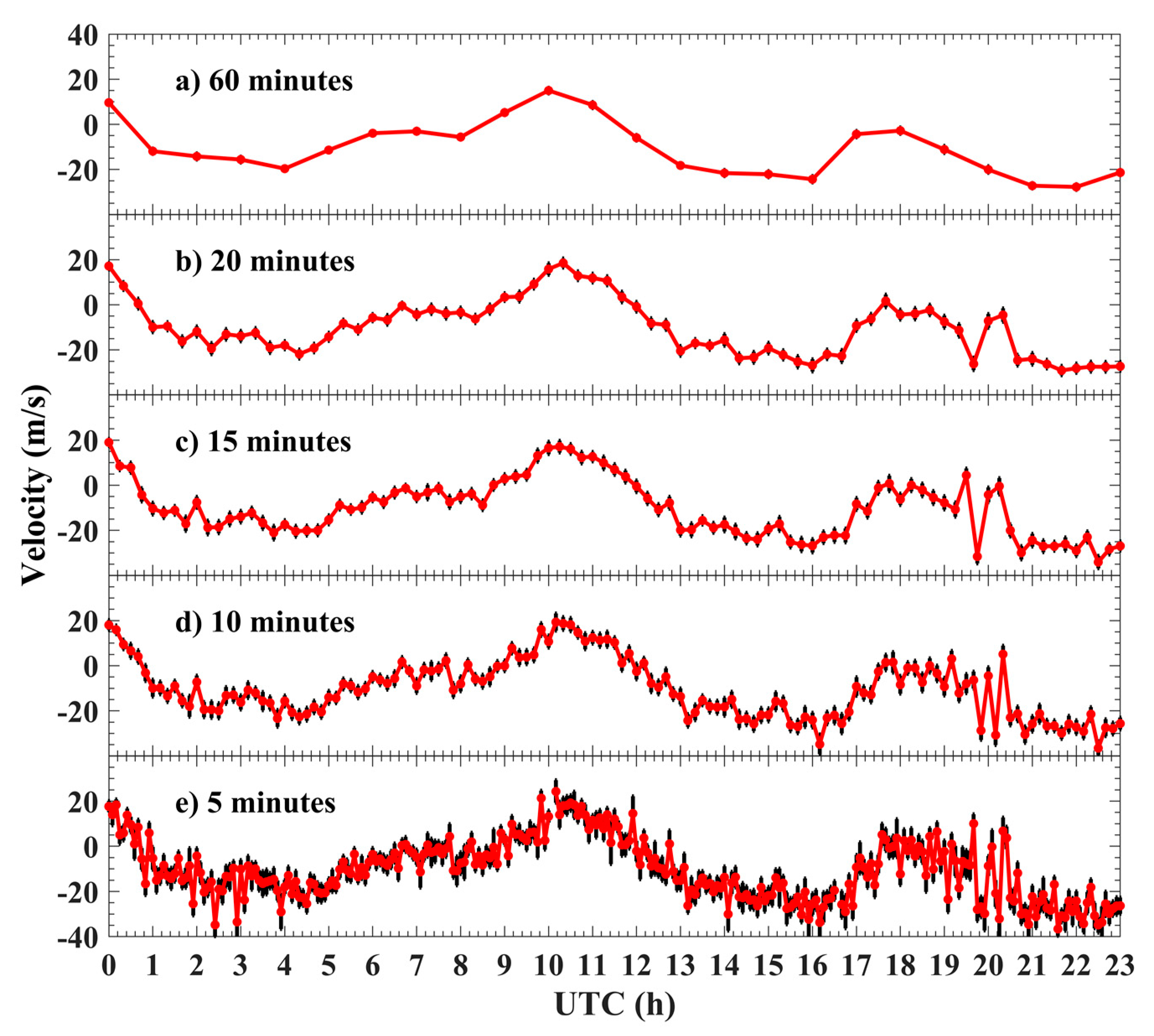
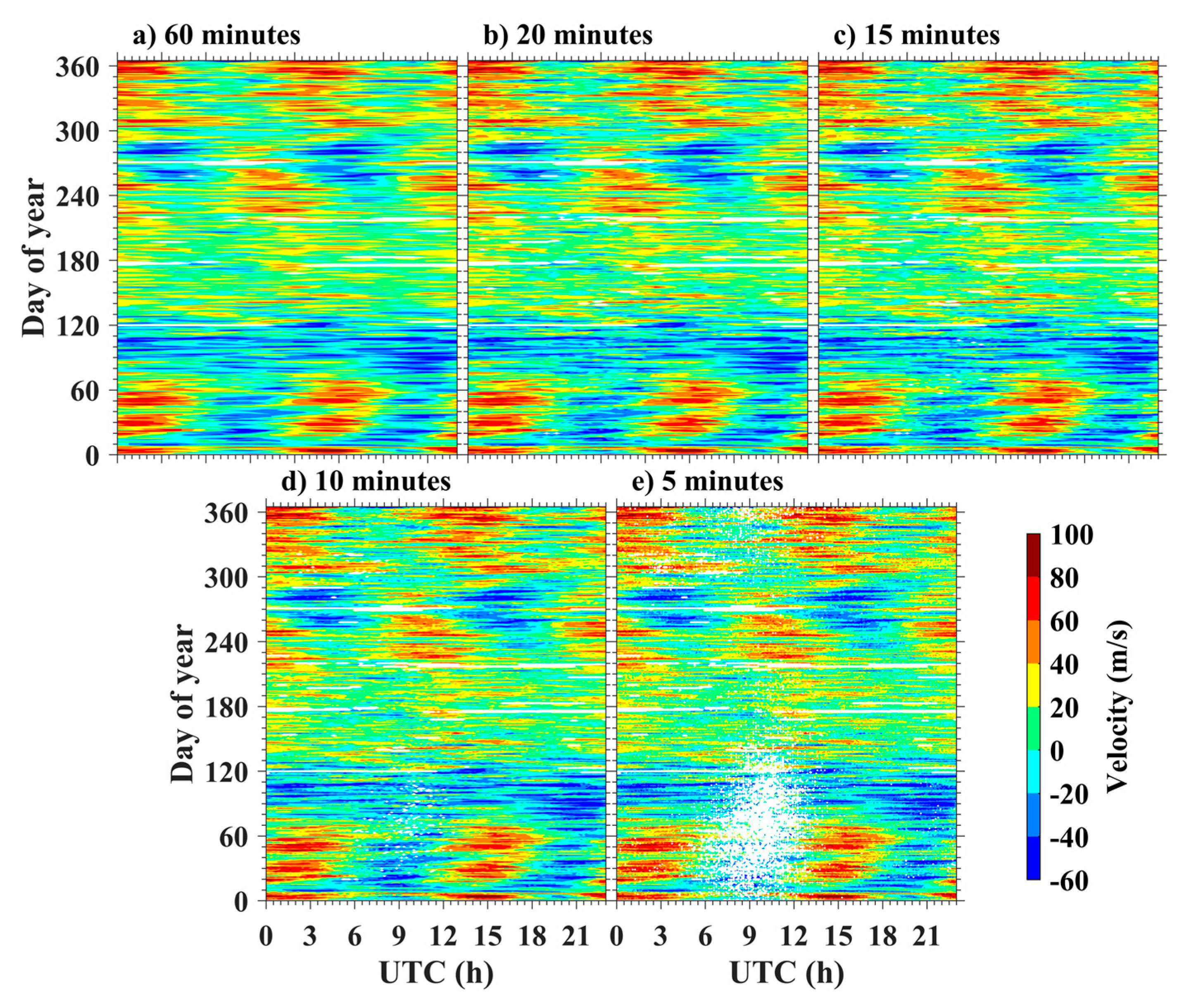
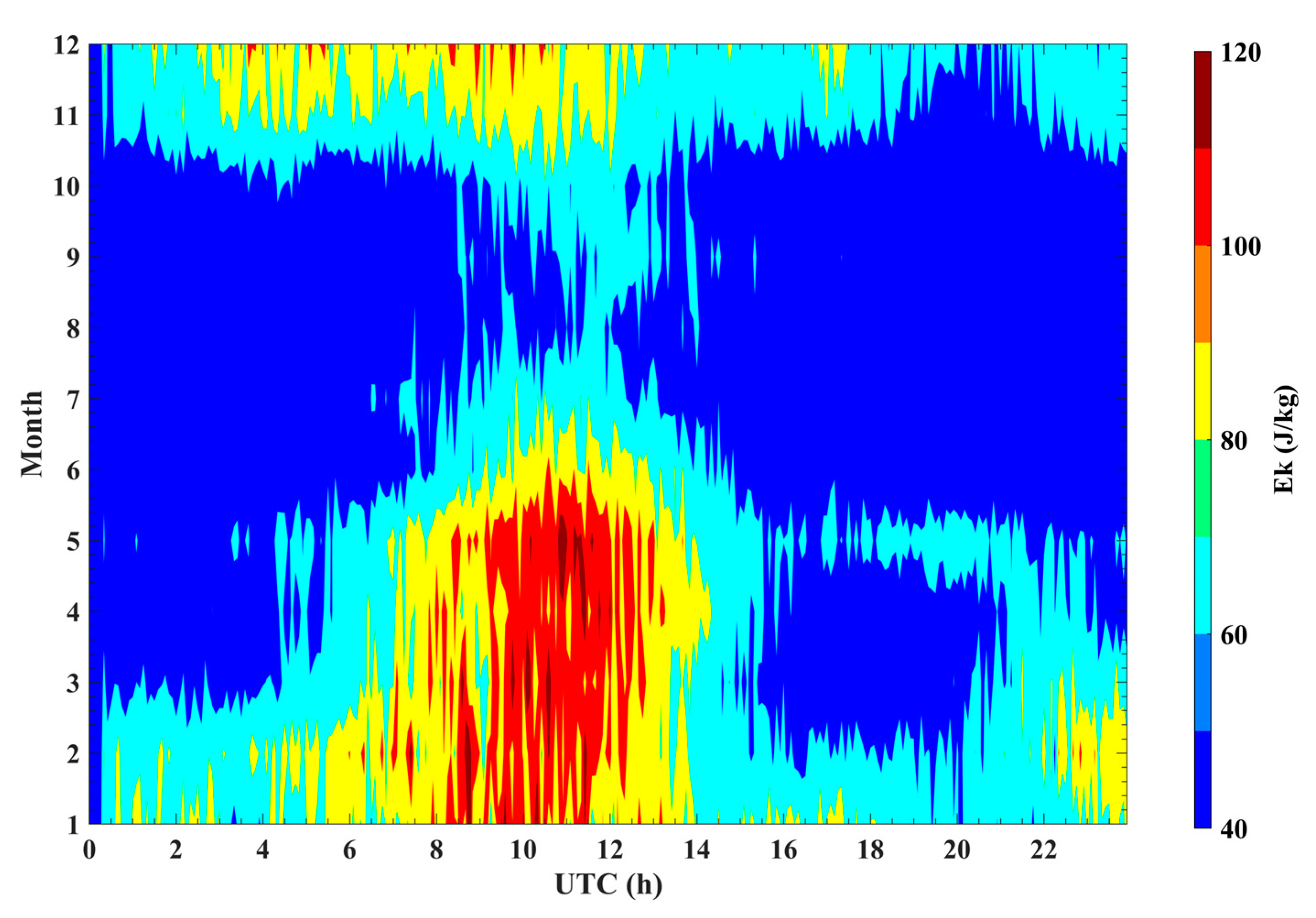
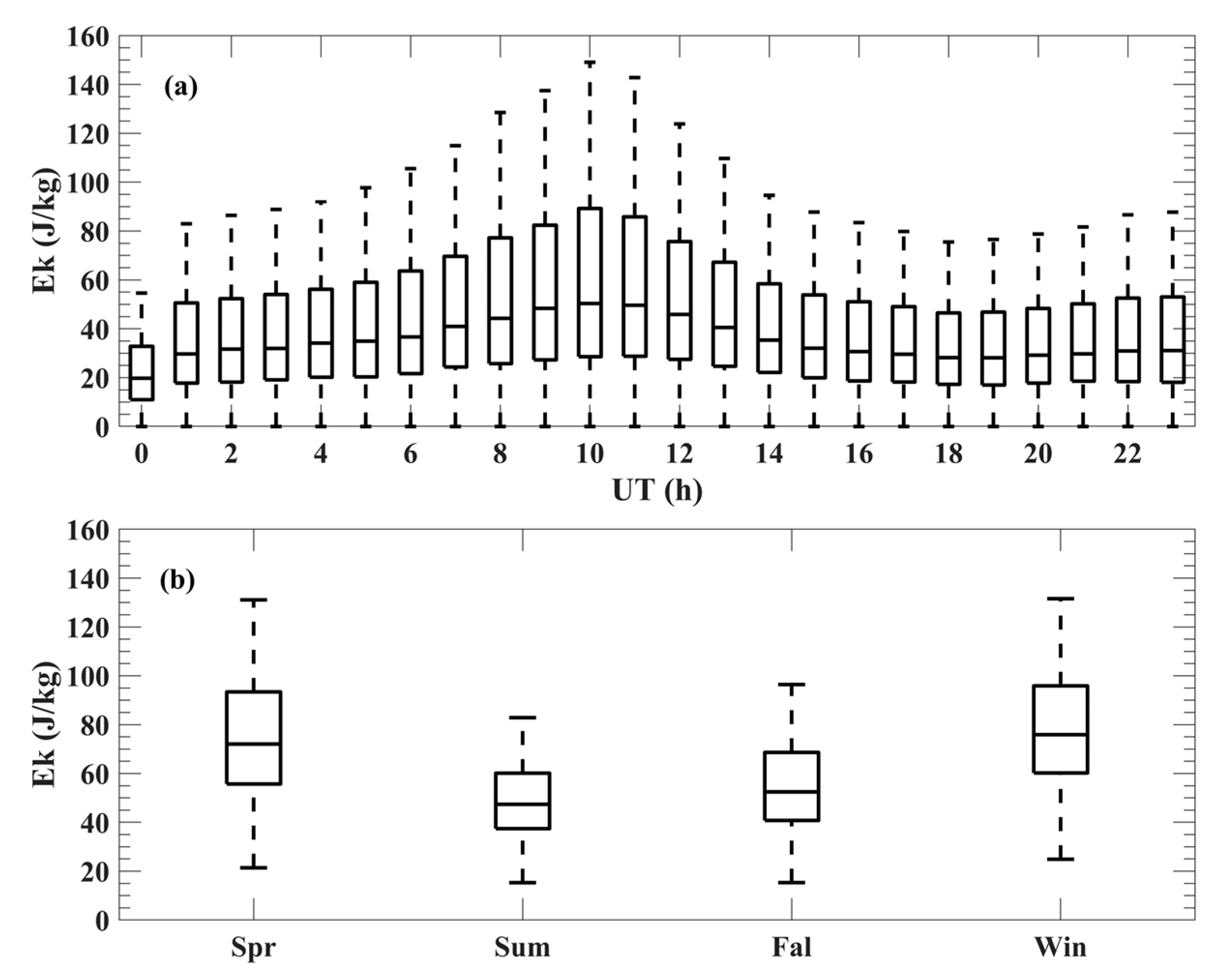
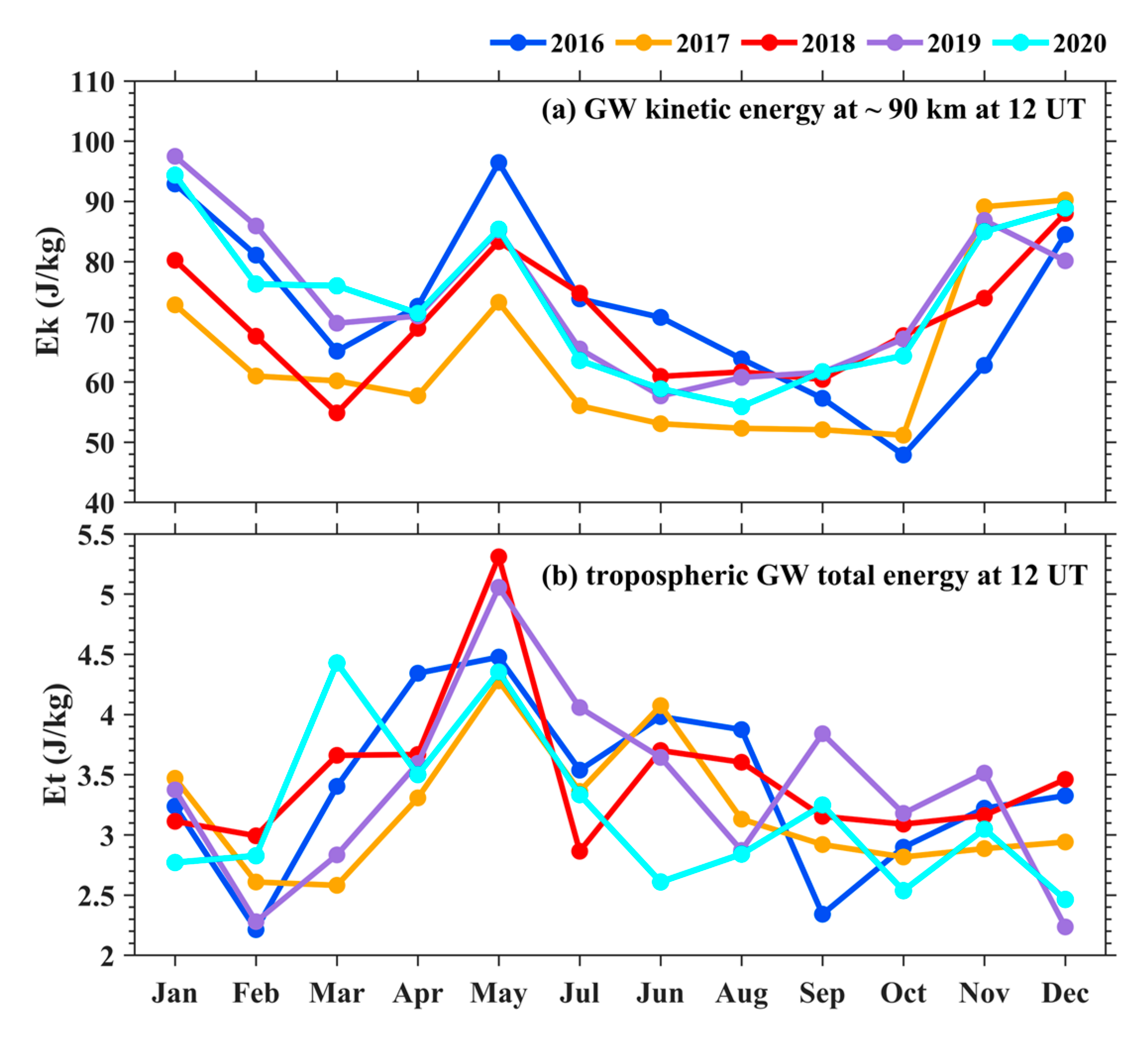
3. Results
4. Discussion and Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Smith, A.K.; Pedatella, N.M.; Marsh, D.R.; Matsuo, T. On the dynamical control of the mesosphere–lower thermosphere by the lower and middle atmosphere. J. Atmos. Sci. 2017, 74, 933–947. [Google Scholar] [CrossRef]
- Fritts, D.C.; Alexander, M.J. Gravity wave dynamics and effects in the middle atmosphere. Rev. Geophys. 2003, 41, 1003. [Google Scholar] [CrossRef]
- Dong, W.; Hickey, M.P.; Zhang, S. A numerical study of gravity waves propagation characteristics in the mesospheric Doppler duct. J. Geophys. Res. Atmos. 2021, 126, e2021JD034680. [Google Scholar] [CrossRef]
- Dong, W.; Fritts, D.C.; Hickey, M.P.; Liu, A.Z.; Lund, T.S.; Zhang, S.; Yan, Y.; Yang, F. Modeling studies of gravity wave dynamics in highly structured environments: Reflection, trapping, instability, momentum transport, secondary gravity waves, and induced flow responses. J. Geophys. Res. Atmos. 2022, 127, e2021JD035894. [Google Scholar] [CrossRef]
- Holdsworth, D.A.; Reid, I.M.; Cervera, M.A. Buckland Park all-sky interferometric meteor radar. Radio Sci. 2004, 39, RS5009. [Google Scholar] [CrossRef]
- Fritts, D.C.; Janches, D.; Hocking, W.K. Southern Argentina agile meteor radar: Initial assessment of gravity wave momentum fluxes. J. Geophys. Res. 2010, 115, D19123. [Google Scholar] [CrossRef]
- Gu, S.; Hou, X.; Li, N.; Yi, W.; Ding, Z.; Chen, J.; Hu, G.; Dou, X. First Comparative Analysis of the Simultaneous Horizontal Wind Observations by Collocated Meteor Radar and FPI at Low Latitude through 892.0-nm Airglow Emission. Remote Sens. 2021, 13, 4337. [Google Scholar] [CrossRef]
- Gavrilov, N.M.; Riggin, D.M.; Fritts, D.C. Medium-frequency radar studies of gravity-wave seasonal variations over Hawaii (22 N, 160 W). J. Geophys. Res. 2003, 108, 4655. [Google Scholar] [CrossRef]
- Manson, A.H.; Meek, C.; Koshyk, J.; Franke, S.; Fritts, D.; Riggin, D.; Hall, C.; Hocking, W.; MacDougall, J.; Igarashi, K.; et al. Gravity wave activity and dynamical effects in the middle atmosphere (60–90 km): Observations from an MF/MLT radar network, and results from the Canadian Middle Atmosphere Model (CMAM). J. Atmos. Sol. Terr. Phys. 2002, 64, 65–90. [Google Scholar] [CrossRef]
- Cai, X.; Yuan, T.; Zhao, Y.; Pautet, P.-D.; Taylor, M.J.; Pendleton, W.R., Jr. A coordinated investigation of the gravity wave breaking and the associated dynamical instability by a Na lidar and an advanced mesosphere temperature mapper over Logan, UT (41.7° N, 111.8° W). J. Geophys. Res. Space Phys. 2014, 119, 6852–6864. [Google Scholar] [CrossRef]
- Cai, X.; Yuan, T.; Liu, H.-L. Large-scale gravity wave perturbations in the mesopause region above Northern Hemisphere midlatitudes during autumnal equinox: A joint study by the USU Na lidar and Whole Atmosphere Community Climate Model. Ann. Geophys. 2017, 35, 181–188. [Google Scholar] [CrossRef]
- Jia, M.; Yuan, J.; Wang, C.; Xia, H.; Wu, Y.; Zhao, L.; Wei, T.; Wu, J.; Wang, L.; Gu, S.-Y.; et al. Long-lived high-frequency gravity waves in the atmospheric boundary layer: Observations and simulations. Atmos. Chem. Phys. 2019, 19, 15431–15446. [Google Scholar] [CrossRef]
- Baumgarten, K.; Gerding, M.; Baumgarten, G.; Lübken, F.-J. Temporal variability of tidal and gravity waves during a record long 10-day continuous lidar sounding. Atmos. Chem. Phys. 2018, 18, 371–384. [Google Scholar] [CrossRef]
- Li, F.; Liu, A.Z.; Swenson, G.R.; Hecht, J.H.; Robinson, W.A. Observations of gravity wave breakdown into ripples associated with dynamical instabilities. J. Geophys. Res. 2005, 110, D09S11. [Google Scholar] [CrossRef]
- Yang, F.; Liu, A.Z. Stability characteristics of the mesopause region above the Andes. J. Geophys. Res. Space Phys. 2022, 127, e2022JA030315. [Google Scholar] [CrossRef]
- Reichert, R.; Kaifler, B.; Kaifler, N.; Rapp, M.; Pautet, P.-D.; Taylor, M.J.; Kivi, R. Retrieval of intrinsic mesospheric gravity wave parameters using lidar and airglow temperature and meteor radar wind data. Atmos. Meas. Tech. 2019, 12, 5997–6015. [Google Scholar] [CrossRef]
- Yamashita, C.; England, S.L.; Immel, T.J.; Chang, L.C. Gravity wave variations during elevated stratopause events using SABER observations. J. Geophys. Res. Atmos. 2013, 118, 5287–5303. [Google Scholar] [CrossRef]
- Thurairajah, B.; Cullens, C.Y. On the downward progression of stratospheric temperature anomalies using long-term SABER observations. J. Geophys. Res. Atmos. 2022, 127, e2022JD036487. [Google Scholar] [CrossRef]
- John, S.R.; Kumar, K.K. TIMED/SABER observations of global gravity wave climatology and their interannual variability from stratosphere to mesosphere lower thermosphere. Clim. Dyn. 2012, 39, 1489–1505. [Google Scholar] [CrossRef]
- Elford, W.G.; Robertson, D.S. Measurements of winds in the upper atmosphere by means of drifting meteor trails II. J. Atmos. Terr. Phys. 1953, 4, 271–284. [Google Scholar] [CrossRef]
- Hocking, W.K.; Thayaparan, T.; Jones, J. Meteor decay times and their use in determining a diagnostic mesospheric temperature-pressure parameter: Methodology and one year of data. Geophys. Res. Lett. 1997, 24, 2977–2980. [Google Scholar] [CrossRef]
- Hocking, W.K.; Thayaparan, T. Simultaneous and colocated observation of winds and tides by MF and meteor radars over London, Canada (43°N, 81°W), during 1994–1996. Radio Sci. 1997, 32, 833–865. [Google Scholar] [CrossRef]
- Miyahara, S.; Forbes, J. Interaction between gravity waves and the diurnal tide in the mesosphere and lower thermosphere. J. Meteorol. Soc. Jpn. 1991, 69, 523–531. [Google Scholar] [CrossRef]
- Fritts, D.C.; Iimura, H.; Janches, D.; Lieberman, R.S.; Riggin, D.M.; Mitchell, N.J.; Vincent, R.A.; Reid, I.M.; Murphy, D.J.; Tsutsumi, M.; et al. Structure, variability, and mean-flow interactions of the January 2015 quasi-2-day wave at middle and high southern latitudes. J. Geophys. Res. Atmos. 2019, 124, 5981–6008. [Google Scholar] [CrossRef]
- Gong, Y.; Xue, J.; Ma, Z.; Zhang, S.; Zhou, Q.; Huang, C.; Huang, K.; Yu, Y.; Li, G. Strong quarterdiurnal tides in the mesosphere and lower thermosphere during the 2019 Arctic sudden stratospheric warming over Mohe, China. J. Geophys. Res. Space Phys. 2021, 126, e2020JA029066. [Google Scholar] [CrossRef]
- Hocking, W.K. A new approach to momentum flux determinations using SKiYMET meteor radars. Ann. Geophys. 2005, 23, 2433–2439. [Google Scholar] [CrossRef]
- de Wit, R.J.; Janches, D.; Fritts, D.C.; Stockwell, R.G.; Coy, L. Unexpected climatological behavior of MLT gravity wave momentum flux in the lee of the Southern Andes hot spot. Geophys. Res. Lett. 2017, 44, 1182–1191. [Google Scholar] [CrossRef]
- Wilhelm, S.; Stober, G.; Brown, P. Climatologies and long-term changes in mesospheric wind and wave measurements based on radar observations at high and mid latitudes. Ann. Geophys. 2019, 37, 851–875. [Google Scholar] [CrossRef]
- Yamamoto, M.; Tsuda, T.; Kato, S. Gravity waves observed by the Kyoto meteor radar in 1983-1985. J. Atmos. Sol.-Terr. Phys. 1986, 48, 597–603. [Google Scholar] [CrossRef]
- Suzuki, S.; Nakamura, T.; Ejiri, M.K.; Tsutsumi, M.; Shiokawa, K.; Kawahara, T.D. Simultaneous airglow, lidar, and radar measurements of mesospheric gravity waves over Japan. J. Geophys. Res. 2010, 115, D24113. [Google Scholar] [CrossRef]
- Kumar, K.K.; Antonita, T.M.; Shelbi, S.T. Initial results from SKiYMET meteor radar at Thumba (8.5°N, 77°E): 2. Gravity wave observations in the MLT region. Radio Sci. 2007, 42, RS6009. [Google Scholar] [CrossRef]
- Liu, L.; Liu, H.; Chen, Y.; Le, H.; Sun, Y.-Y.; Ning, B.; Hu, L.; Wan, W. Variations of the meteor echo heights at Beijing and Mohe, China. J. Geophys. Res. Space Phys. 2016, 122, 1117–1127. [Google Scholar] [CrossRef]
- Liu, L.; Liu, H.; Le, H.; Chen, Y.; Sun, Y.-Y.; Ning, B.; Hu, L.; Wan, W.; Li, N.; Xiong, J. Mesospheric temperatures estimated from the meteor radar observations at Mohe, China. J. Geophys. Res. Space Phys. 2017, 122, 2249–2259. [Google Scholar] [CrossRef]
- Yu, Y.; Wan, W.; Ren, Z.; Xiong, B.; Zhang, Y.; Hu, L.; Ning, B.; Liu, L. Seasonal variations of MLT tides revealed by a meteor radar chain based on Hough mode decomposition. J. Geophys. Res. Space Phys. 2015, 120, 7030–7048. [Google Scholar] [CrossRef]
- Hocking, W.K.; Fuller, B.; Vandepeer, B. Real-time determination of meteor-related parameters utilizing modern digital technology. J. Atmos. Sol.-Terr. Phys. 2001, 63, 155–169. [Google Scholar] [CrossRef]
- Zhang, J.; Guo, J.; Xue, H.; Zhang, S.; Huang, K.; Dong, W.; Shao, J.; Yi, M.; Zhang, Y. Tropospheric gravity waves as observed by the high-resolution China radiosonde network and their potential sources. J. Geophys. Res. Atmos. 2022, 127, e2022JD037174. [Google Scholar] [CrossRef]
- Zhang, J.; Guo, J.; Li, J.; Zhang, S.; Tong, B.; Shao, J.; Li, H.; Zhang, Y.; Cao, L.; Zhai, P.; et al. A climatology of merged daytime planetary boundary layer height over China from radiosonde measurements. J. Geophys. Res. Atmos. 2022, 127, e2021JD036367. [Google Scholar] [CrossRef]
- Holdsworth, D.A.; Reid, I.M. The Buckland Park MF radar: Routine observation scheme and velocity comparisons. Ann. Geophys. 2004, 22, 3815–3828. [Google Scholar] [CrossRef]
- Ehard, B.; Kaifler, B.; Kaifler, N.; Rapp, M. Evaluation of methods for gravity wave extraction from middle-atmospheric lidar temperature measurements. Atmos. Meas. Tech. 2015, 8, 4645–4655. [Google Scholar] [CrossRef]
- Baumgarten, K.; Gerding, M.; Lübken, F.J. Seasonal variation of gravity wave parameters using different filter methods with daylight lidar measurements at midlatitudes. J. Geophys. Res. 2017, 122, 2683–2695. [Google Scholar] [CrossRef]
- Wang, L.; Geller, M.A. Morphology of gravity-wave energy as observed from 4 years (1998–2001) of high vertical resolution U.S. radiosonde data. J. Geophys. Res. 2003, 108, 4489. [Google Scholar] [CrossRef]
- Wang, L.; Geller, M.A.; Alexander, M.J. Spatial and temporal variations of gravity wave parameters, Part I: Intrinsic frequency, wavelength, and vertical propagation direction. J. Atmos. Sci. 2005, 62, 125–142. [Google Scholar] [CrossRef]
- Zhang, S.D.; Yi, F. Latitudinal and seasonal variations of inertial gravity wave activity in the lower atmosphere over central China. J. Geophys. Res. 2007, 112, D05109. [Google Scholar] [CrossRef]
- Alexander, S.P.; Klekociuk, A.R.; Murphy, D.J. Rayleigh lidar observations of gravity wave activity in the winter upper stratosphere and lower mesosphere above Davis, Antarctica (69°S, 78°E). J. Geophys. Res. Atmos. 2011, 116, 1–12. [Google Scholar] [CrossRef]
- Kopp, M.; Gerding, M.; Höffner, J.; Lübken, F.-J. Tidal signatures in temperatures derived from daylight lidar sound- ings above Kühlungsborn (54°N, 12°E). J. Atmos. Sol.-Terr. Phys. 2015, 127, 37–50. [Google Scholar] [CrossRef]
- Jacobi, C. 6 year mean prevailing winds and tides measured by VHF meteor radar over Collm (51.3°N, 13.0°E). J. Atmos. Sol. -Terr. Phys. 2012, 78, 8–18. [Google Scholar] [CrossRef]
- VanZandt, T.E. A model for gravity wave spectra observed by Doppler sounding systems. Radio Sci. 1985, 20, 1323–1330. [Google Scholar] [CrossRef]
- Jia, M.; Xue, X.; Gu, S.; Chen, T.; Ning, B.; Wu, J.; Zeng, X.; Dou, X.; Mingjiao, J.; Xianghui, X.; et al. Multiyear observations of gravity wave momentum fluxes in the midlatitude mesosphere and lower thermosphere region by meteor radar. J. Geophys. Res. Space Phys. 2018, 123, 5684–5703. [Google Scholar] [CrossRef]
- Fritts, D.C.; Wang, L.; Taylor, M.J.; Pautet, P.-D.; Criddle, N.R.; Kaifler, B.; Eckermann, S.D.; Liley, B. Large-amplitude mountain waves in the mesosphere observed on 21 June 2014 during DEEPWAVE: 2. Nonlinear dynamics, wave breaking, and instabilities. J. Geophys. Res. Atmos. 2019, 124, 10006–10032. [Google Scholar] [CrossRef]
- Hunsucker, R.D. Atmospheric gravity waves generated in the high-latitude ionosphere: A review. Rev. Geophys. 1982, 20, 293–315. [Google Scholar] [CrossRef]
- Hines, C.O. Internal atmospheric gravity waves at ionospheric height. Can. J. Phys. 1960, 38, 1441–1481. [Google Scholar] [CrossRef]
- Otsuka, Y.; Shinbori, A.; Tsugawa, T.; Nishioka, M. Solar activity dependence of medium-scale traveling ionospheric disturbances using GPS receivers in Japan. Earth Planets Space 2021, 73, 22. [Google Scholar] [CrossRef]
- Cheng, P.H.; Lin, C.; Otsuka, Y.; Liu, H.; Rajesh, P.K.; Chen, C.-H.; Lin, J.-T.; Chang, M.T. Statistical study of medium-scale traveling ionospheric disturbances in low-latitude ionos-phere using an automatic algorithm. Earth Planets Space 2021, 73, 105. [Google Scholar] [CrossRef]
- Huang, F.; Lei, J.; Otsuka, Y.; Luan, X.; Liu, Y.; Zhong, J.; Dou, X. Characteristics of medium-scale traveling ionospheric disturbances and ionospheric irregularities at mid-latitudes revealed by the total electron content associated with the Beidou geostationary satellite. IEEE Trans. Geosci. Remote Sens. 2020, 59, 6424–6430. [Google Scholar] [CrossRef]



Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Long, C.; Yu, T.; Sun, Y.-Y.; Yan, X.; Zhang, J.; Yang, N.; Wang, J.; Xia, C.; Liang, Y.; Ye, H. Atmospheric Gravity Wave Derived from the Neutral Wind with 5-Minute Resolution Routinely Retrieved by the Meteor Radar at Mohe. Remote Sens. 2023, 15, 296. https://doi.org/10.3390/rs15020296
Long C, Yu T, Sun Y-Y, Yan X, Zhang J, Yang N, Wang J, Xia C, Liang Y, Ye H. Atmospheric Gravity Wave Derived from the Neutral Wind with 5-Minute Resolution Routinely Retrieved by the Meteor Radar at Mohe. Remote Sensing. 2023; 15(2):296. https://doi.org/10.3390/rs15020296
Chicago/Turabian StyleLong, Chi, Tao Yu, Yang-Yi Sun, Xiangxiang Yan, Jian Zhang, Na Yang, Jin Wang, Chunliang Xia, Yu Liang, and Hailun Ye. 2023. "Atmospheric Gravity Wave Derived from the Neutral Wind with 5-Minute Resolution Routinely Retrieved by the Meteor Radar at Mohe" Remote Sensing 15, no. 2: 296. https://doi.org/10.3390/rs15020296
APA StyleLong, C., Yu, T., Sun, Y.-Y., Yan, X., Zhang, J., Yang, N., Wang, J., Xia, C., Liang, Y., & Ye, H. (2023). Atmospheric Gravity Wave Derived from the Neutral Wind with 5-Minute Resolution Routinely Retrieved by the Meteor Radar at Mohe. Remote Sensing, 15(2), 296. https://doi.org/10.3390/rs15020296






