Optimal Estimation Inversion of Ionospheric Electron Density from GNSS-POD Limb Measurements: Part II-Validation and Comparison Using NmF2 and hmF2
Abstract
:1. Introduction
2. Materials and Methods
2.1. Space-Borne Data Sets
- 1.
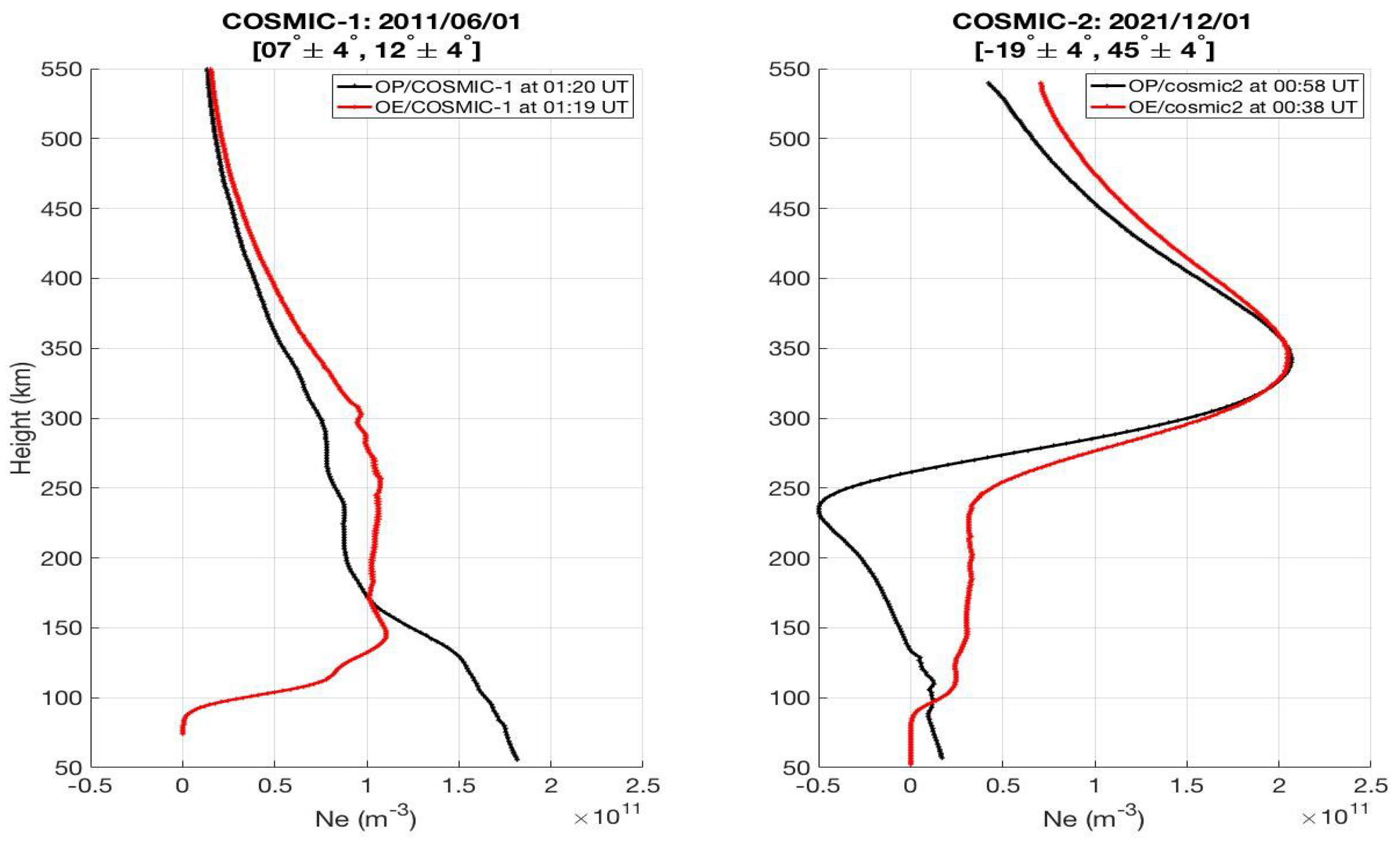
- We determine the initial NmF2 and hmF2 values for retrieved profiles within an altitude range. Although F2 peak altitudes tend to stay generally below 400 km, previous studies indicate occasionally higher values, especially at equatorial latitudes [24,25]. Hence, we set this range as 150–500 km. In some cases, the profiles are modulated with saw-tooth-like perturbation. Figure 2 shows such an example for the OE-retrieved N profile from the Spire constellation during June 2021. We apply a 7-point sliding window filter to smooth all profiles (shown in red), and re-evaluate NmF2 and hmF2 values to ensure that the initial and final values of hmF2 are not exceeded by 10 km.
- 2.
- In the OE inversion algorithm, the input parameter hTECs are fed only when their measurements are present for all tangent heights from the satellite orbital altitudes (∼450–600 km) down to ∼100 km. However, as shown above, such strict criteria are not generally applied in the case of OP inversion, and hence, large spatial gaps are present in some of the OP-retrieved N profiles. To ensure that our data sets are not affected by this, we check the continuity of these profiles within the selected altitude range. If any missing data are evident for more than a 10 km altitude range, the respective profile is discarded from the analysis. To further strengthen our selection criteria, we also discard any monotonically increasing or decreasing N profile, i.e., if the detected hmF2 occurs within 10 km of the lowest or highest edge of the set altitude range (150–500 km), the profile is discarded.
- 3.
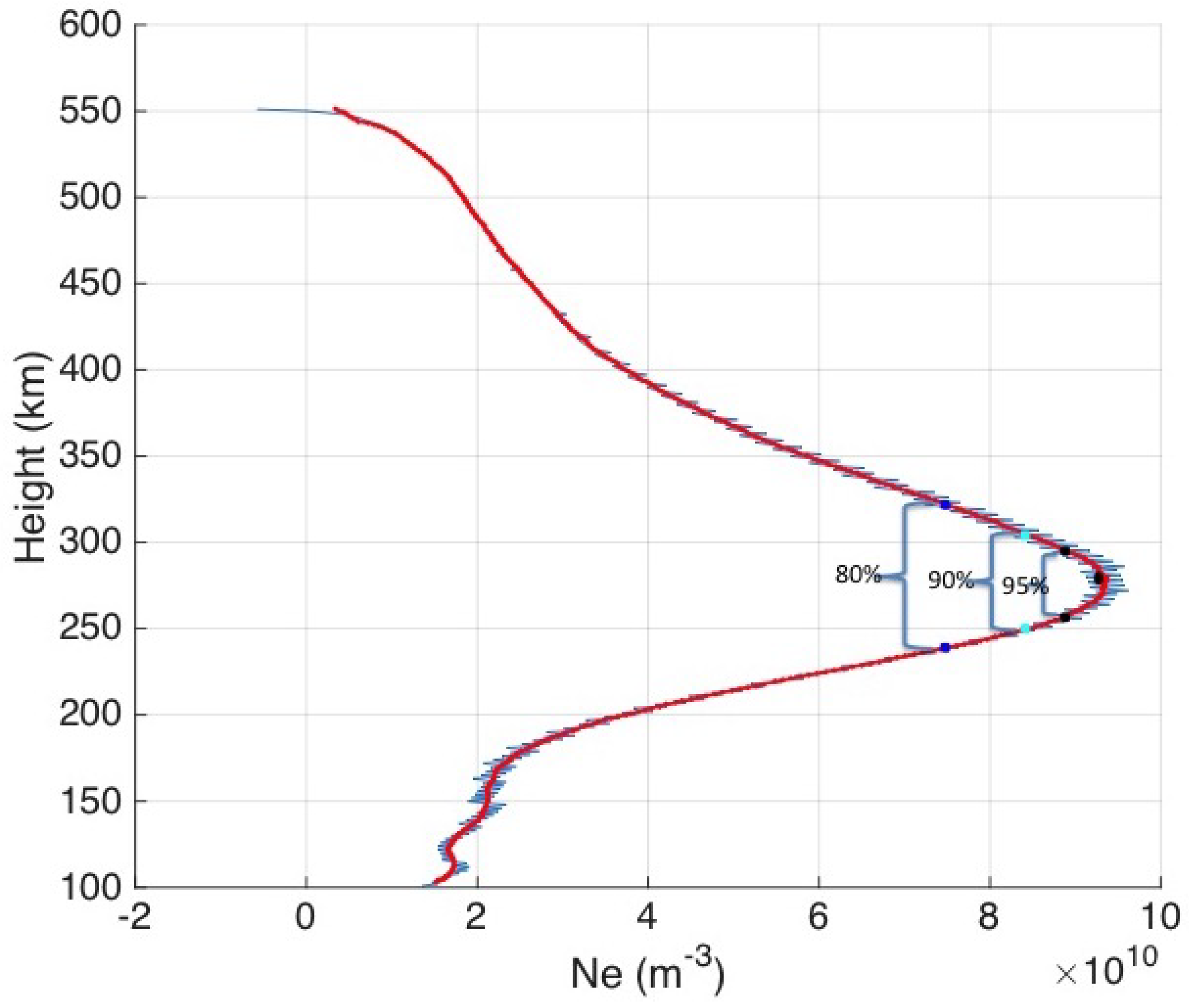
- The estimated NmF2 and hmF2 values are then binned into a 5 latitude by 10 longitude grid for each hour. To perform a comparison study with Digisondes and radars with meaningful statistics, we need to maintain the above grid size for OE-retrieved measurements. In addition, we also identify the thickness of the retrieved F2 layer with 95%, 90%, and 80% of NmF2. The estimated F2 layer thicknesses from the OE retrieval are later used for comparison with Digisonde measurements.
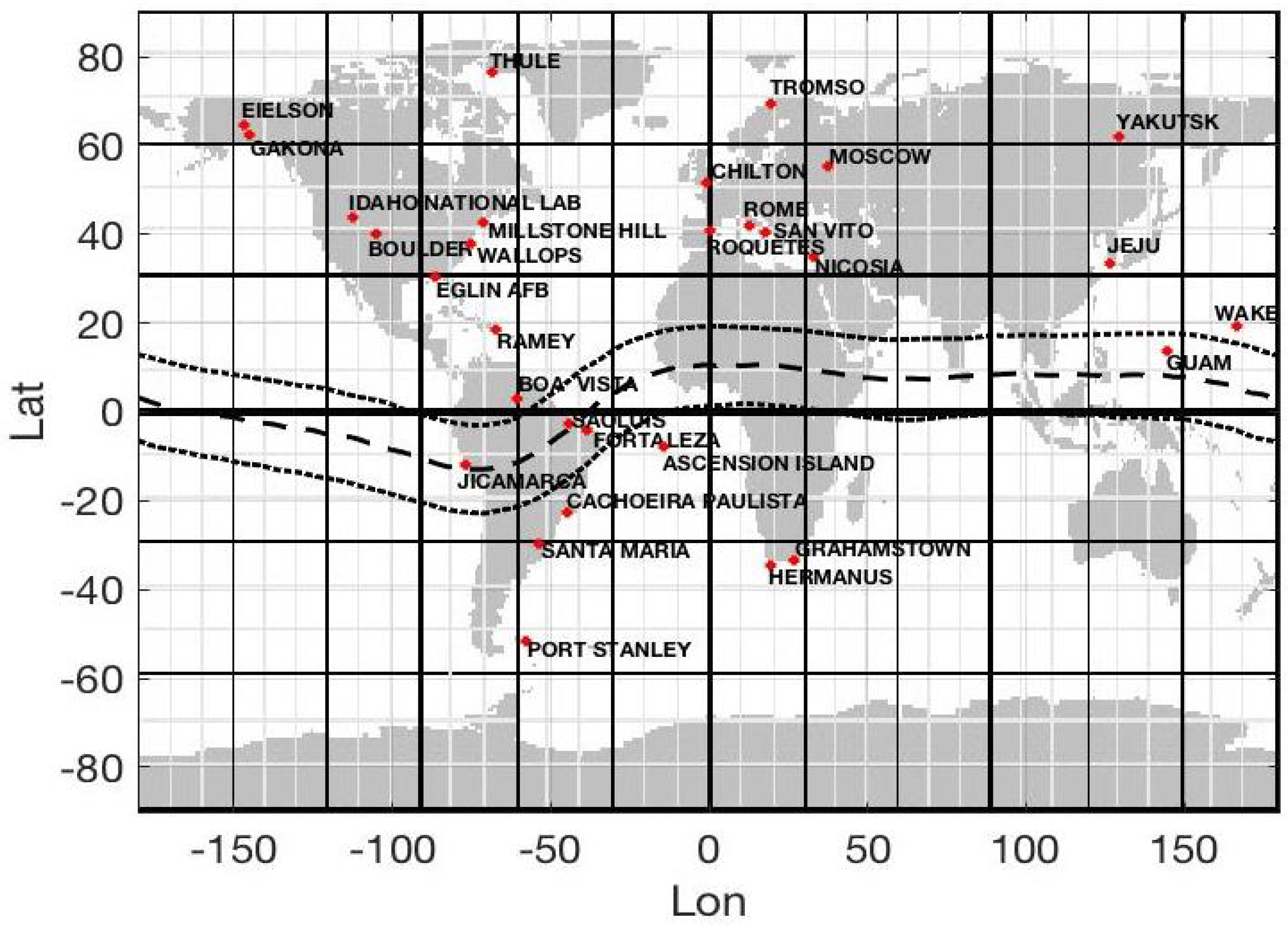
2.2. Ground-Based Data Sets
2.2.1. Digisondes
2.2.2. Incoherent Scatter Radar
3. Results
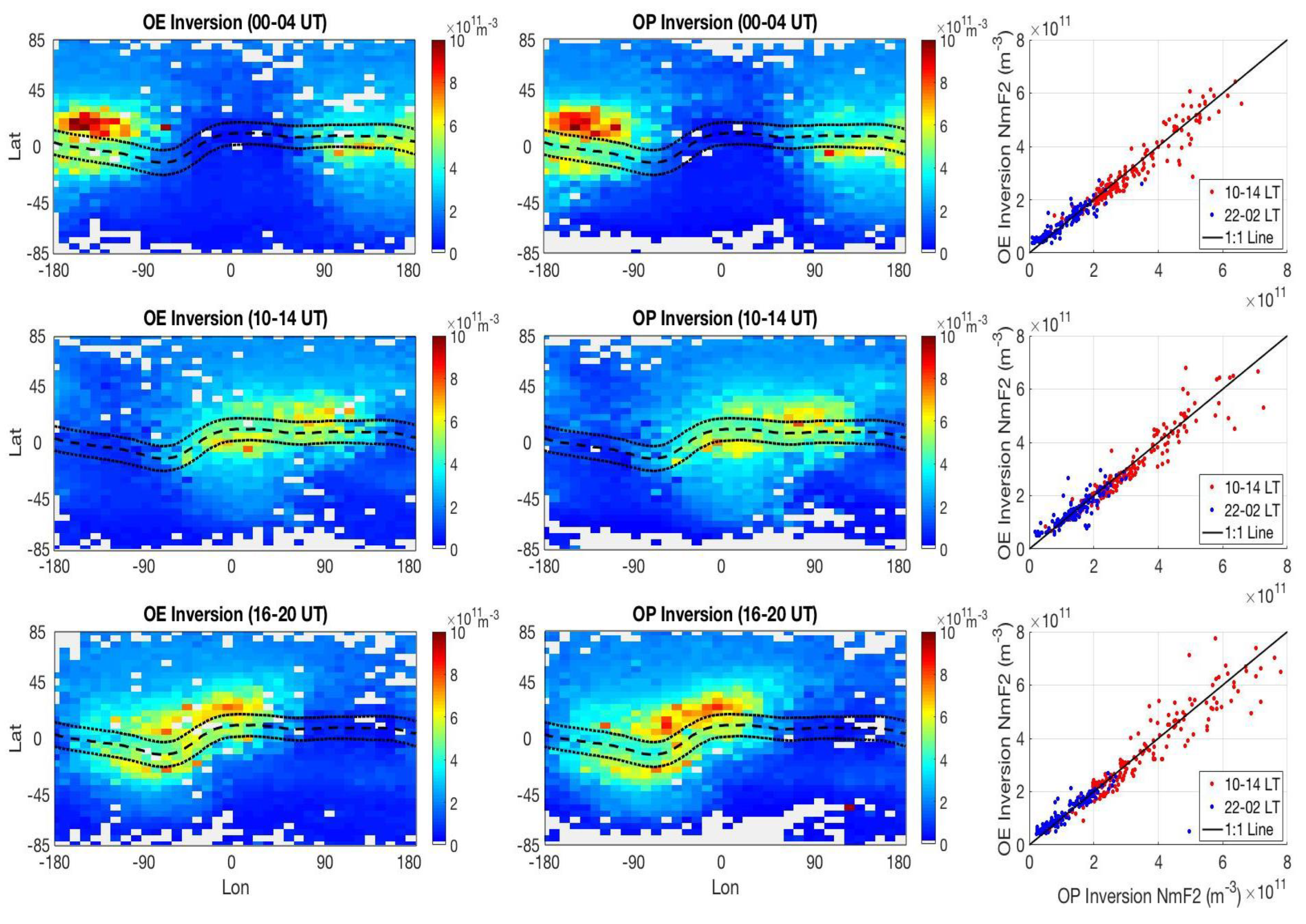
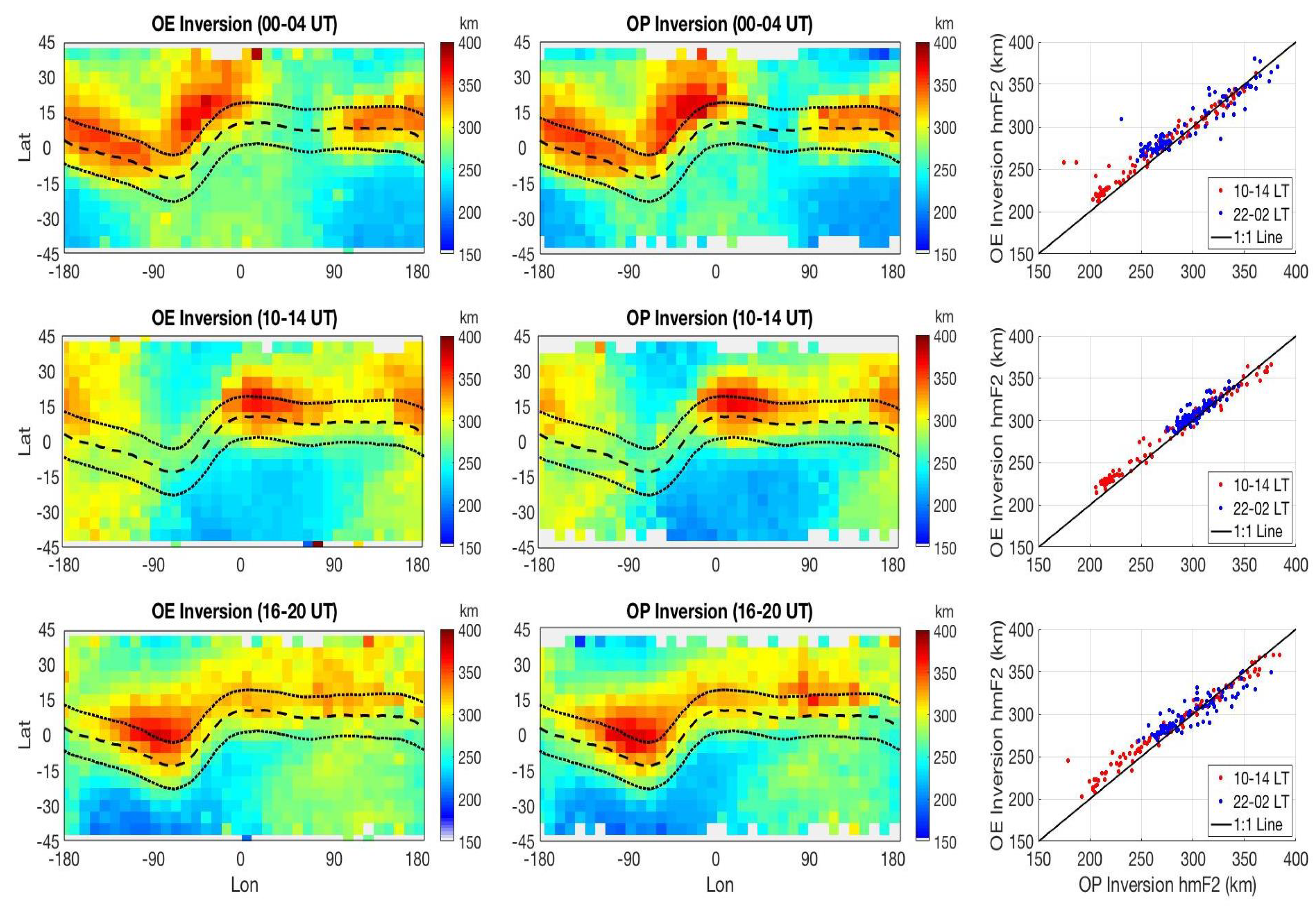
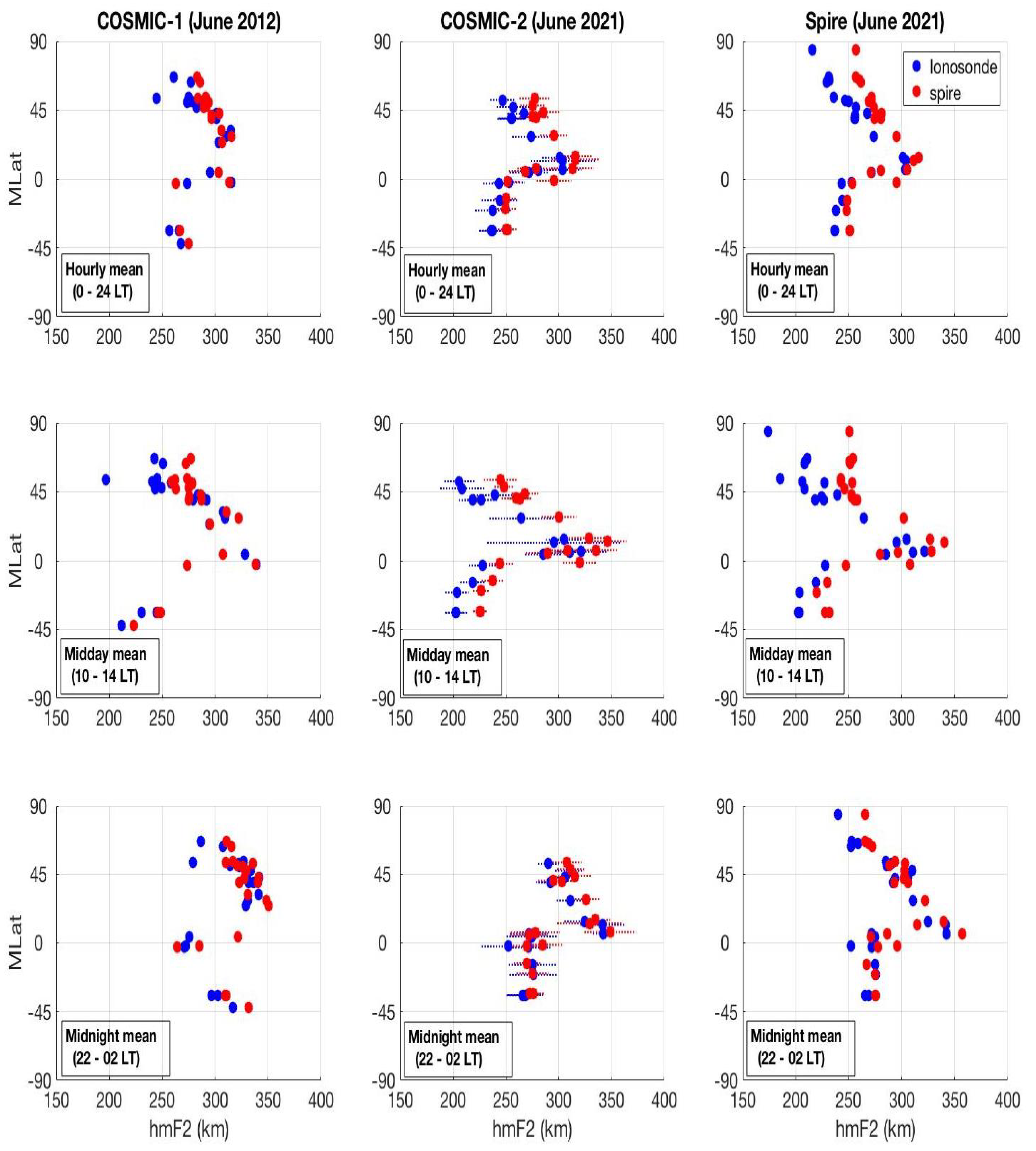
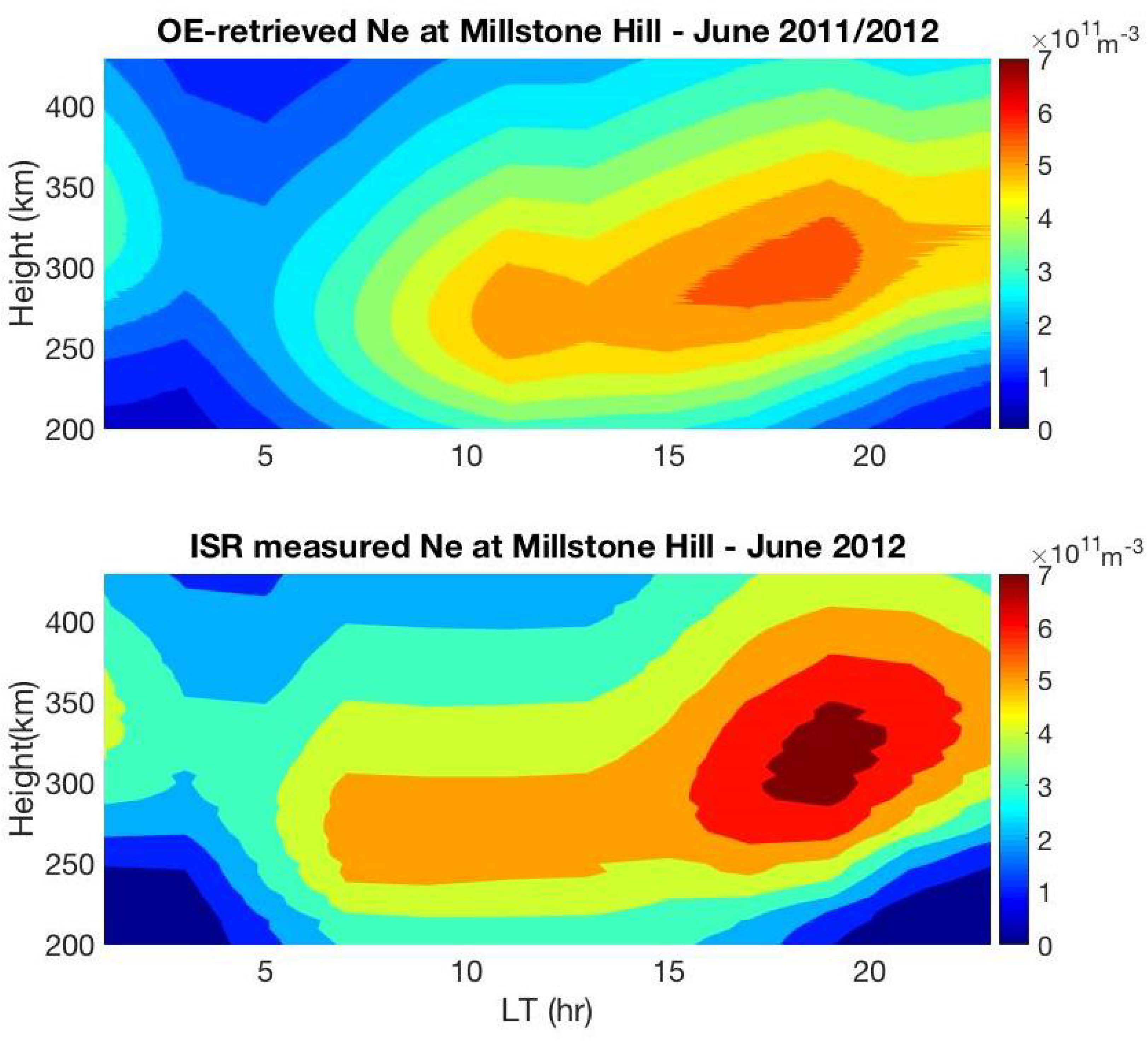
3.1. Climatological Comparison of Global NmF2 and hmF2 Maps
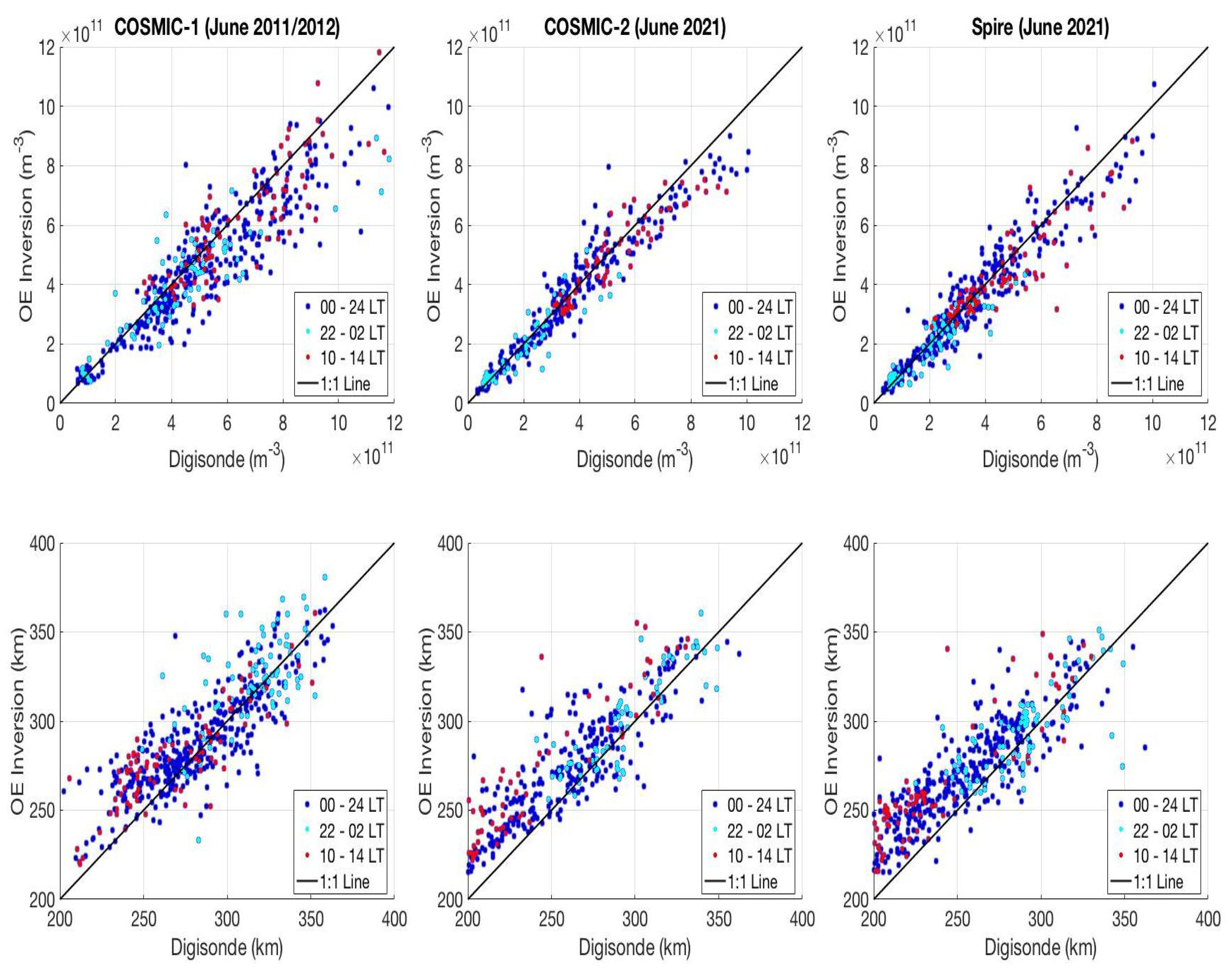
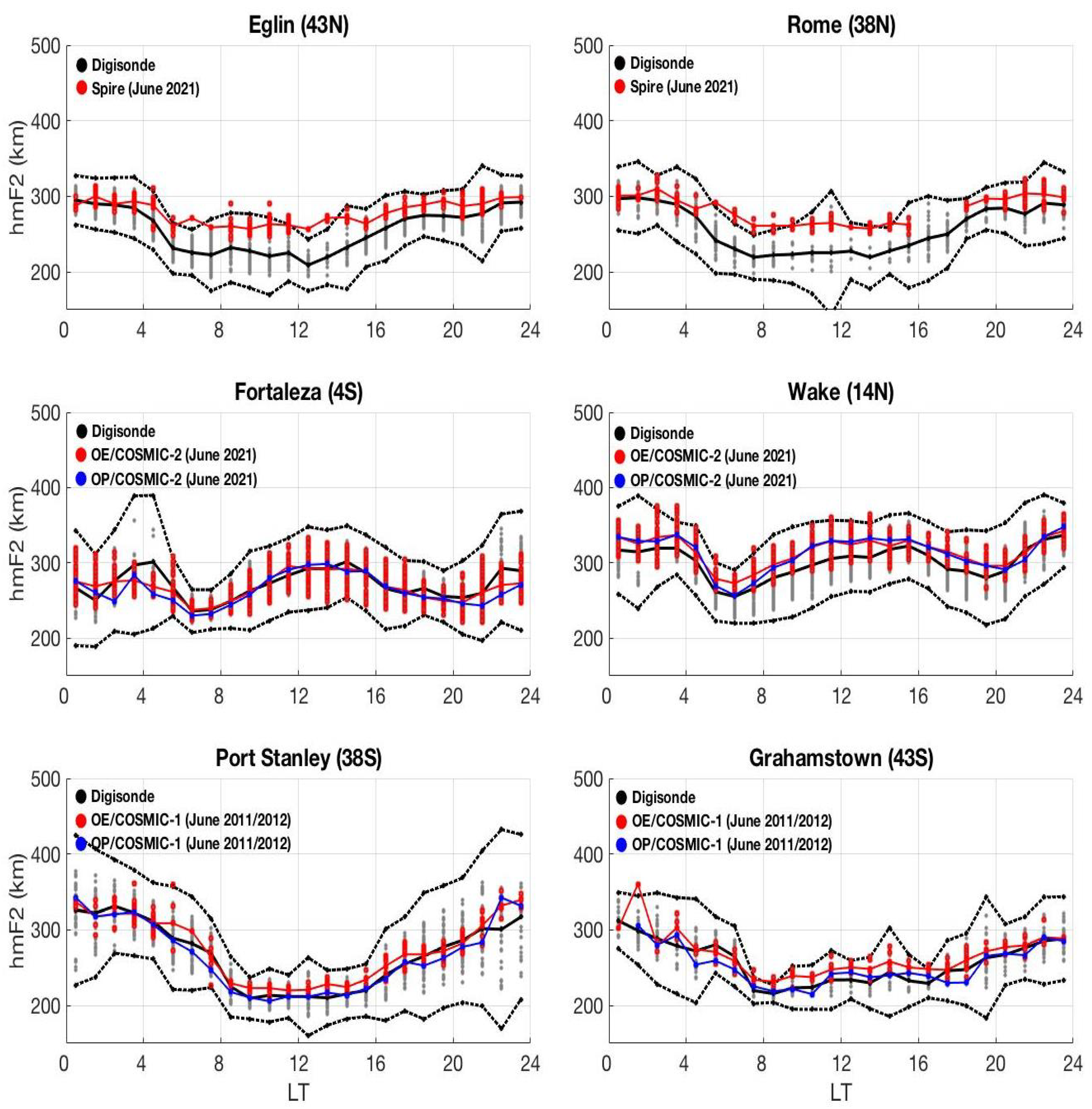
3.2. Comparison of OE-Retrieved F2 Peak Properties to Digisonde Measurements
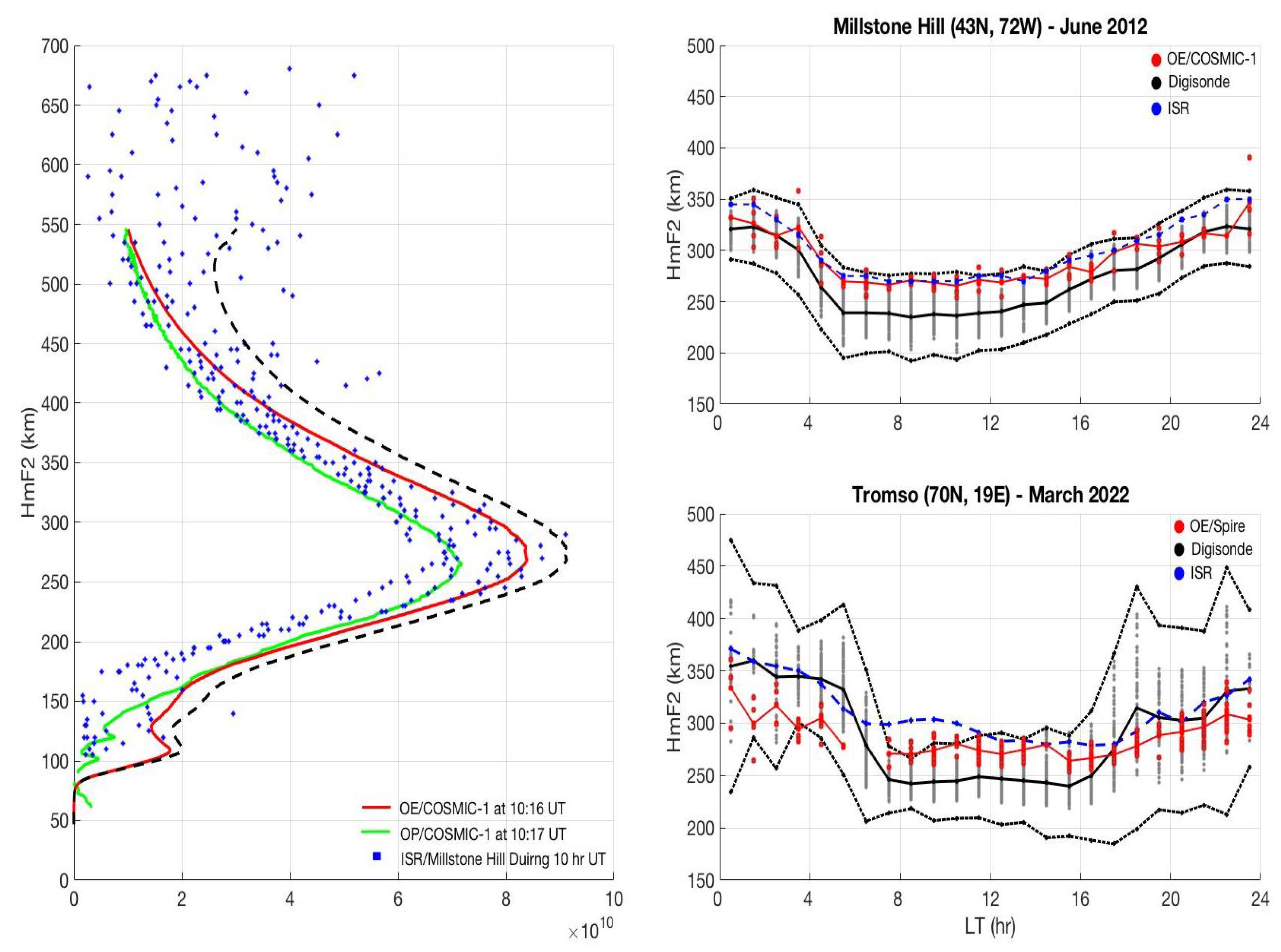
3.3. Comparison of OE-Retrieved hmF2 with Incoherent Scatter Radar Measurements
4. Discussion
4.1. Differences in OE-Retrieved and Digisonde-Measured hmF2s
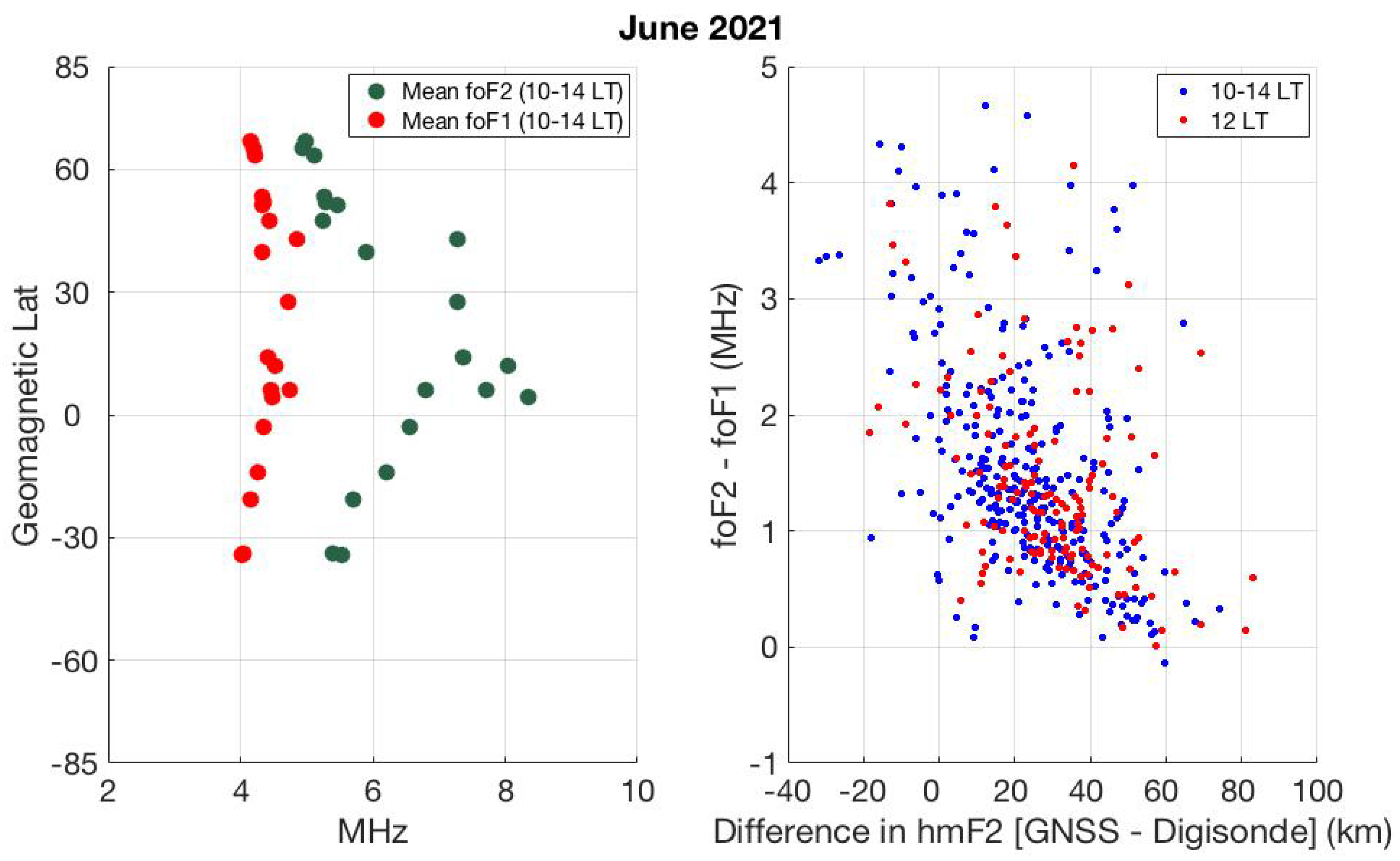
4.1.1. Does the F1 Valley Cause Underestimations in Digisonde Autoscaled hmF2?
4.2. F2 Layer Thickness and the Shape of the N Profile
5. Concluding Summary
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Balan, N.; Souza, J.; Bailey, G.J. Recent developments in the understanding of equatorial ionization anomaly: A review. J. Atmos. Sol.-Terr. Phys. 2018, 171, 3–11. [Google Scholar] [CrossRef]
- Rishbeth, H.; Garriott, O.K. Introduction to Ionospheric Physics, 1st ed.; Academic Press: New York, NY, USA, 1969; Volume 47. [Google Scholar]
- Reinisch, B.W. Digisonde 4D Technical Manual (Version 1.2.11); Lowell Digisonde International: Lowell, MA, USA, 2021. [Google Scholar]
- Reinisch, B.W.; Huang, X. Automatic calculation of electron density profiles from digital ionograms. III—Processing of bottomside ionograms. Radio Sci. 1983, 18, 477–492. [Google Scholar] [CrossRef]
- Titheridge, J.E. Ionogram Analysis with the Generalised Program POLAN; Report UAG; UAG: Irvine, CA, USA, 1985; Volume 93. [Google Scholar]
- Roininen, L.; Laine, M.; Ulich, T. Time-varying ionosonde trend: Case study of Sodankylä hmF2 data 1957–2014. J. Geophys. Res. Space Phys. 2015, 120, 6851–6859. [Google Scholar] [CrossRef]
- Galkin, I.A.; Reinisch, B.W. The New ARTIST 5 for all Digisondes. Ionosonde Netw. Advis. Group Bull. 2008, 69, 1–8. [Google Scholar]
- Reinisch, B.W.; Galkin, I.A. Global Ionospheric Radio Observatory (GIRO). Earth Planets Space 2011, 63, 377–381. [Google Scholar] [CrossRef]
- Gordon, W.E. Incoherent Scattering of Radio Waves by Free Electrons with Applications to Space Exploration. Proc. IRE 1958, 46, 1824–1829. [Google Scholar] [CrossRef]
- Hargreaves, J.K. The Solar-Terrestrial Environment; Cambridge University Press: Cambridge, UK, 1995. [Google Scholar]
- Rocken, C.; Kuo, Y.H.; Schreiner, W.S.; Hunt, D.; Sokolovskiy, S.; McCormick, C. COSMIC System Description. Terr. Atmos. Ocean. Sci. 2000, 11, 021. [Google Scholar] [CrossRef]
- Heise, S.; Jakowski, N.; Wehrenpfennig, A.; Reigber, C.; Lühr, H. Sounding of the topside ionosphere/plasmasphere based on GPS measurements from CHAMP: Initial results. J. Geophys. Res. Lett. 2002, 29, 1699. [Google Scholar] [CrossRef]
- Pedatella, N.M.; Forbes, J.M.; Maute, A.; Richmond, A.D.; Fang, T.W.; Larson, K.M.; Millward, G. Longitudinal variations in the F region ionosphere and the topside ionosphere-plasmasphere: Observations and model simulations. J. Geophys. Res. Space Phys. 2011, 116, A12309. [Google Scholar] [CrossRef]
- Schreiner, W.S.; Sokolovskiy, S.V.; Rocken, C.; Hunt, D.C. Analysis and validation of GPS/MET radio occultation data in the ionosphere. Radio Sci. 1999, 34, 949–966. [Google Scholar] [CrossRef]
- Tsai, L.C.; Tsai, W.H.; Schreiner, W.S.; Berkey, F.T.; Liu, J.Y. Comparisons of GPS/MET retrieved ionospheric electron density and ground based ionosonde data. Earth Planets Space 2001, 53, 193–205. [Google Scholar] [CrossRef]
- Yue, X.; Schreiner, W.S.; Lei, J.; Sokolovskiy, S.V.; Rocken, C.; Hunt, D.C.; Kuo, Y.H. Error analysis of Abel retrieved electron density profiles from radio occultation measurements. Ann. Geophys. 2010, 28, 217–222. [Google Scholar] [CrossRef]
- Pedatella, N.M.; Yue, X.; Schreiner, W.S. An improved inversion for FORMOSAT-3/COSMIC ionosphere electron density profiles. J. Geophys. Res. Space Phys. 2015, 120, 8942–8953. [Google Scholar] [CrossRef]
- Tulasi Ram, S.; Su, Y.; Tsai, L.; Liu, C. A self-contained GIM-aided Abel retrieval method to improve GNSS-radio occultation retrieved electron density profiles. GPS Solut. 2016, 20, 825–836. [Google Scholar] [CrossRef]
- Chou, M.Y.; Lin, C.C.H.; Tsai, H.F.; Lin, C.Y. Ionospheric electron density inversion for Global Navigation Satellite Systems radio occultation using aided Abel inversions. J. Geophys. Res. Space Phys. 2017, 122, 1386–1399. [Google Scholar] [CrossRef]
- Wu, D.L.; Emmons, D.J.; Swarnalingam, N. Global GNSS-RO Electron Density in the Lower Ionosphere. Remote Sens. 2022, 14, 1577. [Google Scholar] [CrossRef]
- Lin, C.Y.; Lin, C.C.H.; Liu, J.Y.; Rajesh, P.K.; Matsuo, T.; Chou, M.Y.; Tsai, H.F.; Yeh, W.H. The Early Results and Validation of FORMOSAT-7/COSMIC-2 Space Weather Products: Global Ionospheric Specification and Ne-Aided Abel Electron Density Profile. J. Geophys. Res. Space Phys. 2020, 125, e28028. [Google Scholar] [CrossRef]
- Swarnalingam, N.; Wu, D.L.; Gopalswamy, N. Interhemispheric Asymmetries in Ionospheric Electron Density Responses During Geomagnetic Storms: A Study Using Space-Based and Ground-Based GNSS and AMPERE Observations. J. Geophys. Res. Space Phys. 2022, 127, e30247. [Google Scholar] [CrossRef]
- Wu, D.L.; Swarnalingam, N.; Emmons, D.J.; Summers, T.C. Optimal Estimation Inversion of Electron Density from GNSS-POD Limb Measurements: Part I—Algorithm Description. Remote Sens. 2023, 15, 3245. [Google Scholar]
- Abdu, M.A.; Alam Kherani, E.; Batista, I.S.; de Paula, E.R.; Fritts, D.C.; Sobral, J.H.A. Gravity wave initiation of equatorial spread F/plasma bubble irregularities based on observational data from the SpreadFEx campaign. Ann. Geophys. 2009, 27, 2607–2622. [Google Scholar] [CrossRef]
- Bilitza, D.; Altadill, D.; Truhlik, V.; Shubin, V.; Galkin, I.; Reinisch, B.; Huang, X. International Reference Ionosphere 2016: From ionospheric climate to real-time weather predictions. Space Weather 2017, 15, 418–429. [Google Scholar] [CrossRef]
- VanZandt, T.; Clark, W.; Warnock, J. Magnetic Apex Coordinates: A Magnetic Coordinate System for the Ionospheric F2 Laye. J. Geophys. Res. 1972, 77, 2406–2411. [Google Scholar] [CrossRef]
- Gilbert, J.D.; Smith, R.W. A comparison between the automatic ionogram scaling system ARTIST and the standard manual method. Radio Sci. 1988, 23, 968–974. [Google Scholar] [CrossRef]
- McNamara, L.F. Quality figures and error bars for autoscaled Digisonde vertical incidence ionograms. Radio Sci. 2006, 41, RS4011. [Google Scholar] [CrossRef]
- Enell, C.F.; Kozlovsky, A.; Turunen, T.; Ulich, T.; Välitalo, S.; Scotto, C.; Pezzopane, M. Comparison between manual scaling and Autoscala automatic scaling applied to Sodankylä Geophysical Observatory ionograms. Geosci. Instrum. Methods Data Syst. 2016, 5, 53–64. [Google Scholar] [CrossRef]
- Pezzopane, M.; Scotto, C. Automatic scaling of critical frequency foF2 and MUF(3000)F2: A comparison between Autoscala and ARTIST 4.5 on Rome data. Radio Sci. 2007, 42, RS4003. [Google Scholar] [CrossRef]
- Galkin, I.A.; Reinisch, B.W.; Huang, X.; Khmyrov, G.M. Confidence Score of ARTIST-5 Ionogram Autoscaling; INAG Technical Memorandum; INAG: Laguna Hills, CA, USA, 2013. [Google Scholar]
- Burns, A.G.; Solomon, S.C.; Wang, W.; Qian, L.; Zhang, Y.; Paxton, L.J. Daytime climatology of ionospheric NmF2 and hmF2 from COSMIC data. J. Geophys. Res. Space Phys. 2012, 117, A09315. [Google Scholar] [CrossRef]
- Kil, H.; Talaat, E.R.; Oh, S.J.; Paxton, L.J.; England, S.L.; Su, S.Y. Wave structures of the plasma density and vertical E × B drift in low-latitude F region. J. Geophys. Res. Space Phys. 2008, 113, A09312. [Google Scholar] [CrossRef]
- Luan, X.; Wang, P.; Dou, X.; Liu, Y.C.M. Interhemispheric asymmetry of the equatorial ionization anomaly in solstices observed by COSMIC during 2007–2012. J. Geophys. Res. Space Phys. 2015, 120, 3059–3073. [Google Scholar] [CrossRef]
- Krankowski, A.; Zakharenkova, I.; Krypiak-Gregorczyk, A.; Shagimuratov, I.I.; Wielgosz, P. Ionospheric electron density observed by FORMOSAT-3/COSMIC over the European region and validated by ionosonde data. J. Geod. 2011, 85, 949–964. [Google Scholar] [CrossRef]
- Cherniak, I.; Zakharenkova, I.; Braun, J.; Wu, Q.; Pedatella, N.; Schreiner, W.; Weiss, J.P.; Hunt, D. Accuracy assessment of the quiet-time ionospheric F2 peak parameters as derived from COSMIC-2 multi-GNSS radio occultation measurements. J. Space Weather Space Clim. 2021, 11, 18. [Google Scholar] [CrossRef]
- Themens, D.R.; Reid, B.; Elvidge, S. ARTIST Ionogram Autoscaling Confidence Scores: Best Practices. URSI Radio Sci. Lett. 2022, 4, 1. [Google Scholar] [CrossRef]
- Scotto, C.; Sabbagh, D. The Accuracy of Real-time hmF2 Estimation from Ionosondes. Remote Sens. 2020, 12, 2671. [Google Scholar] [CrossRef]
- Krasheninnikov, I.V.; Leshchenko, L.N. Errors in Estimating of the F2-Layer Peak Parameters in Automatic Systems for Processing the Ionograms in the Vertical Radio Sounding of the Ionosphere under Low Solar Activity Conditions. Geomagn. Aeron. 2021, 61, 703–712. [Google Scholar] [CrossRef]
- Stankov, S.M.; Jodogne, J.C.; Kutiev, I.; Stegen, K.; Warnant, R. Evaluation of automatic ionogram scaling for use in real-time ionospheric density profile specification: Dourbes DGS-256/ARTIST-4 performance. Ann. Geophys. 2012, 55, 283–291. [Google Scholar]
- McNamara, L.F.; Cooke, D.L.; Valladares, C.E.; Reinisch, B.W. Comparison of CHAMP and Digisonde plasma frequencies at Jicamarca, Peru. Radio Sci. 2007, 42, RS2005. [Google Scholar] [CrossRef]
- Jacobs, L.; Poole, A.W.V.; McKinnell, L.A. An analysis of automatically scaled F1 layer data over Grahamstown, South Africa. Adv. Space Res. 2004, 34, 1949–1952. [Google Scholar] [CrossRef]
- Pezzopane, M.; Scotto, C. A method for automatic scaling of F1 critical frequencies from ionograms. Radio Sci. 2008, 43, RS2S91. [Google Scholar] [CrossRef]
- Reinisch, B.W.; Huang, X. Deducing topside profiles and total electron content from bottomside ionograms. Adv. Space Res. 2001, 27, 23–30. [Google Scholar] [CrossRef]
- Luan, X.; Liu, L.; Wan, W.; Lei, J.; Zhang, S.R.; Holt, J.M.; Sulzer, M.P. A study of the shape of topside electron density profile derived from incoherent scatter radar measurements over Arecibo and Millstone Hill. Radio Sci. 2006, 41, RS4006. [Google Scholar] [CrossRef]
- Tulasi Ram, S.; Su, S.Y.; Liu, C.H.; Reinisch, B.W.; McKinnell, L.A. Topside ionospheric effective scale heights (HT) derived with ROCSAT-1 and ground-based ionosonde observations at equatorial and midlatitude stations. J. Geophys. Res. Space Phys. 2009, 114, A10309. [Google Scholar] [CrossRef]
- Themens, D.R.; Jayachandran, P.T.; Bilitza, D.; Erickson, P.J.; Haggstrom, I.; Lyashenko, M.V.; Reid, B.; Varney, R.H.; Pustovalova, L. Topside Electron Density Representations for Middle and High Latitudes: A Topside Parameterization for E-CHAIM Based On the NeQuick. J. Geophys. Res. Space Phys. 2018, 123, 1603–1617. [Google Scholar] [CrossRef]
- Pignalberi, A.; Pietrella, M.; Pezzopane, M.; Nava, B.; Cesaroni, C. The Ionospheric Equivalent Slab Thickness: A Review Supported by a Global Climatological Study Over Two Solar Cycles. Space Sci. Rev. 2022, 218, 37. [Google Scholar] [CrossRef]
- Bilitza, D.; Pezzopane, M.; Truhlik, V.; Altadill, D.; Reinisch, B.W.; Pignalberi, A. The International Reference Ionosphere Model: A Review and Description of an Ionospheric Benchmark. Rev. Geophys. 2022, 60, e2022RG000792. [Google Scholar] [CrossRef]
- Shubin, V.N.; Karpachev, A.T.; Tsybulya, K.G. Global model of the F2 layer peak height for low solar activity based on GPS radio-occultation data. J. Atmos. Sol.-Terr. Phys. 2013, 104, 106–115. [Google Scholar] [CrossRef]








| Height (km) | Optimal Estimation Mean / Std (m−3) | Onion Peeling Mean and Std (m−3) |
|---|---|---|
| 150 | 1.7 × /1.0× (5998) | 2.2 × /1.2 × (3788) |
| 120 | 8.5 × /7.1 × (5998) | 1.3 × / 1.2 × (3751) |
| 100 | 2.7 × /1.3 × (5998) | 9.1 × /1.0 × (3596) |
| 90 | 3.2 × /1.9 × (5998) | −6.8 ×/9.5 × (3542) |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Swarnalingam, N.; Wu, D.L.; Emmons, D.J.; Gardiner-Garden, R. Optimal Estimation Inversion of Ionospheric Electron Density from GNSS-POD Limb Measurements: Part II-Validation and Comparison Using NmF2 and hmF2. Remote Sens. 2023, 15, 4048. https://doi.org/10.3390/rs15164048
Swarnalingam N, Wu DL, Emmons DJ, Gardiner-Garden R. Optimal Estimation Inversion of Ionospheric Electron Density from GNSS-POD Limb Measurements: Part II-Validation and Comparison Using NmF2 and hmF2. Remote Sensing. 2023; 15(16):4048. https://doi.org/10.3390/rs15164048
Chicago/Turabian StyleSwarnalingam, Nimalan, Dong L. Wu, Daniel J. Emmons, and Robert Gardiner-Garden. 2023. "Optimal Estimation Inversion of Ionospheric Electron Density from GNSS-POD Limb Measurements: Part II-Validation and Comparison Using NmF2 and hmF2" Remote Sensing 15, no. 16: 4048. https://doi.org/10.3390/rs15164048
APA StyleSwarnalingam, N., Wu, D. L., Emmons, D. J., & Gardiner-Garden, R. (2023). Optimal Estimation Inversion of Ionospheric Electron Density from GNSS-POD Limb Measurements: Part II-Validation and Comparison Using NmF2 and hmF2. Remote Sensing, 15(16), 4048. https://doi.org/10.3390/rs15164048






