The SOLAR-HRS New High-Resolution Solar Spectra for Disk-Integrated, Disk-Center, and Intermediate Cases
Abstract
1. Introduction
- The disk-integrated solar spectrum (Figure 1) and its variations over time, which are important for understanding the solar variability and its underlying mechanisms.
- The disk-center solar spectrum (with the deepest and warmest layers at the center) and limb darkening, which are important for the construction and verification of solar model atmospheres and therefore for a better understanding of the solar atmosphere.
- Solar spectra for different solar view angles, which are crucial for our understanding of both spatially resolved solar spectral radiance and full-disk spectral irradiance.
2. MicroCarb’s Scientific Needs Regarding the Disk-Integrated Solar Spectrum
3. Importance of Solar Spectra and Their Determination Methods
3.1. The Theoretical Approach
- The radiative transfer code developed by R.-L. Kurucz (http://kurucz.harvard.edu/sun/irradiance2008/, accessed on 13 April 2011), which calculated radiative transfer under the assumption of local thermodynamic equilibrium (LTE).
- The Solar Radiation Physical Modeling (SRPM) radiative transfer code developed by J.-M. Fontenla [29,30], which is a computing system for the quantitative evaluation of physical process modeling in the context of high-spectral-resolution solar observations. As with all other radiative codes, SRPM takes into account various physical processes, such as the absorption, scattering, and emission of radiation. SRPM (200 nm to 100 m) is extensively used in the study of the solar atmosphere and its effects on the Earth’s climate.
- The COSI spherical radiative transfer code [31], which stands for COde for Solar Irradiance. It solves both the radiative transfer equation and the equations of statistical equilibrium simultaneously. This is essential for accurately determining the solar spectral irradiance in the ultraviolet region (partially formed in the chromosphere), where the LTE assumption is not valid.
- The NESSY radiative transfer code [32], which stands for Non-Local Thermodynamic Equilibrium (NLTE) Spectrum SYnthesis. It represents an improvement of the COSI code, where the entire solar spectrum (ultraviolet, visible, infrared) can be obtained. The computation time of NESSY is significantly faster than that of COSI, making it a more efficient tool for analyzing star spectra.
- The MPS-ATLAS code [33], which was developed by the Max Planck Institute for Solar System Research (Germany). It is based on the ATLAS 9 code [34] and combines the efficient generation of opacity distribution functions (ODFs), atmosphere modeling, and spectral synthesis in local thermodynamic equilibrium into a single efficient package. MPS-ATLAS has been validated against previous ATLAS 9 calculations, the PHOENIX code calculations [35], and high-quality observations. It provides more numerical functionality and is substantially faster compared to other available codes.
3.2. Ground-Based Measurements and the Bouguer–Langley Method Extrapolation
3.3. Ground-Based and Airplane Measurements and the Telluric Subtraction Method
- Measuring solar spectra: The first step is to measure the solar spectrum on the ground or with an airplane. The presence of atmospheric absorption lines (e.g., from water vapor, oxygen, and ozone) distorts the solar spectrum. Thus, these atmospheric lines need to be removed before the solar spectrum can be analyzed.
- Removing atmospheric absorption lines: This can be carried out by comparing the measured solar spectrum to a reference spectrum that has been corrected for atmospheric absorption. The reference spectrum can be obtained from a solar atlas or a model of the Earth’s atmosphere.
- Modeling solar spectral lines: After removing atmospheric absorption lines, the remaining lines can be attributed to the Sun. However, some of these lines may also be due to atmospheric pollution or instrumental effects. To ensure that only solar spectral lines are analyzed, a detailed model of solar spectral lines must be used. These models (Kurucz, SRPM, COSI, NESSY, MPS-ATLAS, etc.) take into account the physical properties of the Sun, such as temperature, pressure, and composition.
- Analyzing solar spectral lines: Once the solar spectral lines have been isolated, they can be analyzed to extract information about the Sun’s physical properties. The intensity and width of the spectral lines can be used to determine the temperature and pressure of the Sun’s atmosphere. The positions of the spectral lines allow the composition of the Sun’s atmosphere to be determined, including the abundance of elements, such as hydrogen, helium, oxygen, carbon, iron, neon, nitrogen, silicon, magnesium, sulfur, calcium, and chromium.
3.4. Space-Based Measurements
- Solar irradiance measurement using a photometer, radiometer, or telescope: This method involves the measurement of the total amount of solar radiation received by a sensor in space (e.g., the PREcision Monitoring Sensor (PREMOS) photometer or the SOlar Diameter Imager and Surface Mapper (SODISM) telescope [40,41] onboard the French PICARD microsatellite). By analyzing the spectral content of the radiation, the solar spectrum can be derived. This method has the advantage of being relatively simple and robust, but it does not provide detailed spectral information.
- Spectroscopy using diffraction grating: This method involves the use of optical parts to disperse the incoming solar radiation into its component wavelengths and then measuring the intensity of each wavelength using a detector such as a photomultiplier detector (SOLar SPECtrum (SOLSPEC) onboard the International Space Station). This method can provide detailed spectral information, but it requires precise calibration and is sensitive to instrument drift.
- Spectroscopy using a Fabry–Perot interferometer: This method involves the use of an interferometer to selectively pass only certain wavelengths of the incoming solar radiation while blocking out all others. By scanning the interferometer over a range of wavelengths, the solar spectrum can be reconstructed. This method can provide very high spectral resolution, but it requires very precise alignment and calibration.
- Spectroscopy using a Fourier transform interferometer: This method involves the use of a special interferometer to measure the interference pattern of the incoming solar radiation as a function of wavelength. By applying a Fourier transform to the interference pattern, the solar spectrum can be reconstructed. This method can provide very high spectral resolution and is relatively simple to implement, but it is sensitive to instrument vibrations and requires careful calibration.
4. Methodology for Determining the SOLAR-HRS and MPS-ATLAS Spectra
4.1. Methodology for Obtaining the SOLAR-HRS Spectra Based on Ground and Space Observations
- Convolve a high-resolution spectrum with lower radiometric calibration accuracy and the best slit function that is available for the lower-resolution solar measure or reference spectrum :The high-resolution solar spectrum () based on very high spectral resolution solar line data is degraded to SOLAR-SOLSPEC instrument resolution using the chosen slit function settings (). The convolution of the spectrum by the instrument slit function is given by the integral described in Equation (1). The integration interval () is defined by the width of the slit function.
- Interpolate the obtained high-sampling low-resolution spectrum on the wavelength grid of the low-resolution reference spectrum:The solar spectrum obtained in step 1 has low spectral resolution, but it has preserved the sampling of the initial spectrum at very high spectral resolution (). Therefore, it is necessary to resample the spectrum to make it compatible with the absolute reference solar spectrum for obtaining the scaling factor.
- Divide that spectrum by the low-resolution spectrum to obtain the fraction by which to multiply the original high-resolution spectrum, used in the first step to obtain the scaling factor (Q):
- Interpolate the low-sampling scaling factor to the high-resolution wavelength grid:
- The new high-resolution solar reference spectrum is defined by:
- The choice of the best ground-based solar high-resolution spectra (e.g., QASUMEFTS) and/or pseudo-transmittance spectra (e.g., SPTS) that have a radiometric calibration with lower accuracy.
- The choice of a lower-resolution reference spectrum that is considered the most realistic for radiometric calibration accuracy and based on space measurements (e.g., SOLAR-ISS).
- An accurate knowledge of the spectral response function of the instrument (e.g., slit function of SOLAR-SOLSPEC) providing the low-resolution reference spectrum, as well as careful attention to the details of the interpolation process.
- An accurate knowledge of the sources of uncertainty (reference spectrum’s radiometric calibration, original high-resolution spectrum’s radiometric calibration, etc.).
4.2. Methodology for Obtaining the MPS-ATLAS Solar Spectra Based on Kurucz and Vald3 Solar Linelists
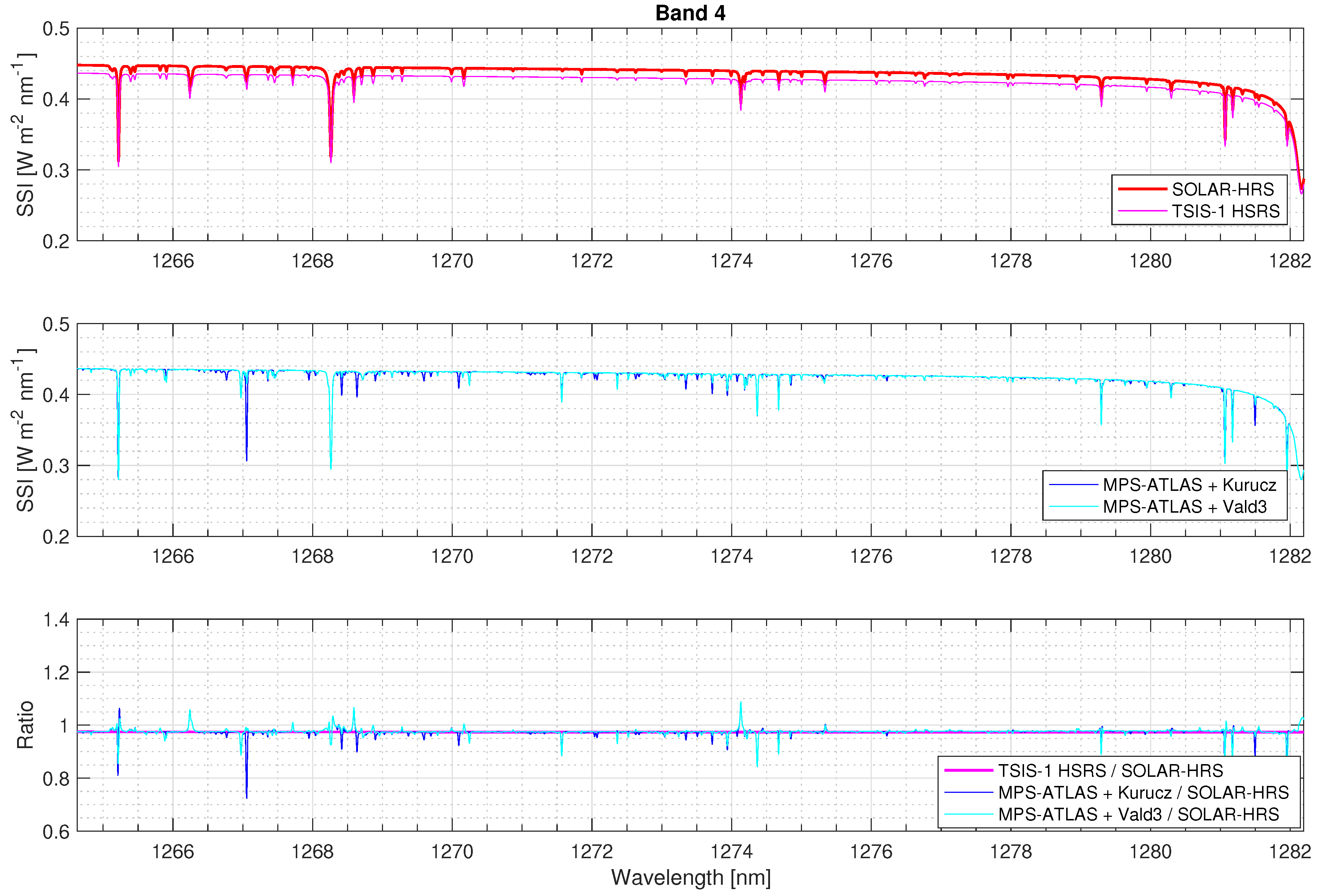
5. Results
6. Discussion and Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Meftah, M.; Jouglet, D.; Revel, C. The SSHR Solar Reference Spectrum. In Proceedings of the AGU Fall Meeting Abstracts, New Orleans, LA, USA, 13–17 December 2021; Volume 2021, p. SH45B-2379. [Google Scholar]
- Moore, C.E.; Minnaert, M.G.J.; Houtgast, J. The Solar Spectrum 2935 A to 8770 A; National Bureau of Standards Monograph: Washington, DC, USA, 1966.
- Delbouille, L.; Roland, G.; Neven, L. Atlas Photometrique du Spectre Solaire de [lambda] 3000 a [lambda] 10000; Université de Liège: Liège, Belgium, 1973. [Google Scholar]
- Willson, R.C. Status of knowledge of the extraterrestrial solar ’constant’ and spectral distribution. In Proceedings of the Seminar on Testing Solar Energy Materials and Systems, Gaithersburg, MD, USA, 22–24 May 1978; pp. 75–82. [Google Scholar]
- Kurucz, R.L.; Furenlid, I.; Brault, J.; Testerman, L. Solar Flux Atlas from 296 to 1300 nm; National Solar Observatory: Sunspot, NM, USA, 1984. [Google Scholar]
- Fontenla, J.; White, O.R.; Fox, P.A.; Avrett, E.H.; Kurucz, R.L. Calculation of Solar Irradiances. I. Synthesis of the Solar Spectrum. Astrophys. J. 1999, 518, 480–499. [Google Scholar] [CrossRef]
- Thuillier, G.; Hersé, M.; Labs, D.; Foujols, T.; Peetermans, W.; Gillotay, D.; Simon, P.C.; Mandel, H. The Solar Spectral Irradiance from 200 to 2400 nm as Measured by the SOLSPEC Spectrometer from the Atlas and Eureca Missions. Solar Phys. 2003, 214, 1–22. [Google Scholar] [CrossRef]
- Chance, K.; Kurucz, R.L. An improved high-resolution solar reference spectrum for earth’s atmosphere measurements in the ultraviolet, visible, and near infrared. J. Quant. Spectrosc. Radiat. Transf. 2010, 111, 1289–1295. [Google Scholar] [CrossRef]
- Meftah, M.; Damé, L.; Bolsée, D.; Hauchecorne, A.; Pereira, N.; Sluse, D.; Cessateur, G.; Irbah, A.; Bureau, J.; Weber, M.; et al. SOLAR-ISS: A new reference spectrum based on SOLAR/SOLSPEC observations. Astron. Astrophys. 2018, 611, A1. [Google Scholar] [CrossRef]
- Coddington, O.M.; Richard, E.C.; Harber, D.; Pilewskie, P.; Woods, T.N.; Chance, K.; Liu, X.; Sun, K. The TSIS-1 Hybrid Solar Reference Spectrum. Geophys. Res. Lett. 2021, 48, e91709. [Google Scholar] [CrossRef]
- Gueymard, C.A. The sun’s total and spectral irradiance for solar energy applications and solar radiation models. Sol. Energy 2004, 76, 423–453. [Google Scholar] [CrossRef]
- Bhatt, R.; Doelling, D.R.; Coddington, O.; Scarino, B.; Gopalan, A.; Haney, C. Quantifying the Impact of Solar Spectra on the Inter-Calibration of Satellite Instruments. Remote Sens. 2021, 13, 1438. [Google Scholar] [CrossRef]
- Bolsee, D.; Pereira, N.; Decuyper, W.; Gillotay, D.; Yu, H.; Sperfeld, P.; Pape, S.; Cuevas, E.; Redondas, A.; Hernandez, Y. Accurate Determination of the TOA Solar Spectral NIR Irradiance Using a Primary Standard Source and Bouguer-Langley Technique. Sol. Phys. 2014, 289, 2433–2457. [Google Scholar] [CrossRef]
- Elsey, J.; Coleman, M.D.; Gardiner, T.; Shine, K.P. Can Measurements of the Near-Infrared Solar Spectral Irradiance be Reconciled? A New Ground-Based Assessment Between 4000 and 10,000 cm−1. Geophys. Res. Lett. 2017, 44, 10071–10080. [Google Scholar] [CrossRef]
- Pasternak, F.; Bernard, P.; Georges, L.; Pascal, V. The microcarb instrument. In Proceedings of the International Conference on Space Optics—ICSO 2016, Biarritz, France, 18–21 October 2016; Cugny, B., Karafolas, N., Sodnik, Z., Eds.; International Society for Optics and Photonics, SPIE: Bellingham, WA, USA, 2017; Volume 10562, p. 105621P. [Google Scholar] [CrossRef]
- Bréon, F.M. Monitoring the Carbon Cycle from Space. In Proceedings of the 40th COSPAR Scientific Assembly, Moscow, Russia, 2–10 August 2014; Volume 40, p. SIDL7-1-14. [Google Scholar]
- Bertaux, J.L.; Hauchecorne, A.; Lefèvre, F.; Bréon, F.M.; Blanot, L.; Jouglet, D.; Lafrique, P.; Akaev, P. The use of the 1.27 µm O2 absorption band for greenhouse gas monitoring from space and application to MicroCarb. Atmos. Meas. Tech. 2020, 13, 3329–3374. [Google Scholar] [CrossRef]
- Wu, K.; Palmer, P.I.; Wu, D.; Jouglet, D.; Feng, L.; Oda, T. Theoretical assessment of the ability of the MicroCarb satellite city-scan observing mode to estimate urban CO2 emissions. Atmos. Meas. Tech. 2023, 16, 581–602. [Google Scholar] [CrossRef]
- Shapiro, A.I.; Solanki, S.K.; Krivova, N.A.; Tagirov, R.V.; Schmutz, W.K. The role of the Fraunhofer lines in solar brightness variability. Astron. Astrophys. 2015, 581, A116. [Google Scholar] [CrossRef]
- Sukhodolov, T.; Rozanov, E.; Shapiro, A.I.; Anet, J.; Cagnazzo, C.; Peter, T.; Schmutz, W. Evaluation of the ECHAM family radiation codes performance in the representation of the solar signal. Geosci. Model Dev. 2014, 7, 2859–2866. [Google Scholar] [CrossRef]
- Sukhodolov, T.; Rozanov, E.; Ball, W.T.; Bais, A.; Tourpali, K.; Shapiro, A.I.; Telford, P.; Smyshlyaev, S.; Fomin, B.; Sander, R.; et al. Evaluation of simulated photolysis rates and their response to solar irradiance variability. J. Geophys. Res. 2016, 121, 6066–6084. [Google Scholar] [CrossRef]
- Shapiro, A.I.; Peter, H.; Solanki, S.K. Chapter 3—The Sun’s Atmospher. In The Sun as a Guide to Stellar Physics; Engvold, O., Vial, J.C., Skumanich, A., Eds.; Elsevier: Amsterdam, The Netherlands, 2019; pp. 59–85. [Google Scholar] [CrossRef]
- Borucki, W.J.; Koch, D.; Basri, G.; Batalha, N.; Brown, T.; Caldwell, D.; Caldwell, J.; Christensen-Dalsgaard, J.; Cochran, W.D.; DeVore, E.; et al. Kepler Planet-Detection Mission: Introduction and First Results. Science 2010, 327, 977–980. [Google Scholar] [CrossRef]
- Ricker, G.R.; Winn, J.N.; Vanderspek, R.; Latham, D.W.; Bakos, G.Á.; Bean, J.L.; Berta-Thompson, Z.K.; Brown, T.M.; Buchhave, L.; Butler, N.R.; et al. Transiting Exoplanet Survey Satellite (TESS). In Proceedings of the Space Telescopes and Instrumentation 2014: Optical, Infrared, and Millimeter Wave, Montreal, QC, Canada, 22–27 June 2014; SPIE: Bellingham, WA, USA, 2014; Volume 9143, p. 914320. [Google Scholar] [CrossRef]
- Sing, D.K. Stellar limb-darkening coefficients for CoRot and Kepler. Astron. Astrophys. 2010, 510, A21. [Google Scholar] [CrossRef]
- Claret, A.; Hauschildt, P.H.; Witte, S. New limb-darkening coefficients for Phoenix/1d model atmospheres. II. Calculations for 5000 K ≤ Teff≤ 10,000 K Kepler, CoRot, Spitzer, uvby, UBVRIJHK, Sloan, and 2MASS photometric systems. Astron. Astrophys. 2013, 552, A16. [Google Scholar] [CrossRef]
- Claret, A. Limb and Gravity-darkening Coefficients for the Space Mission CHEOPS. Res. Notes Am. Astron. Soc. 2021, 5, 13. [Google Scholar] [CrossRef]
- Kostogryz, N.M.; Witzke, V.; Shapiro, A.; Solanki, S.K.; Maxted, P.; Kurucz, R.; Gizon, L. Stellar limb darkening. I. A new MPS-ATLAS library for Kepler, TESS, CHEOPS, and PLATO passbands. Astron. Astrophys. 2022, 8, 14. [Google Scholar]
- Fontenla, J.M.; Curdt, W.; Haberreiter, M.; Harder, J.; Tian, H. Semiempirical Models of the Solar Atmosphere. III. Set of Non-LTE Models for Far-Ultraviolet/Extreme-Ultraviolet Irradiance Computation. Astrophys. J. 2009, 707, 482–502. [Google Scholar] [CrossRef]
- Fontenla, J.M.; Stancil, P.C.; Landi, E. Solar Spectral Irradiance, Solar Activity, and the Near-Ultra-Violet. Astrophys. J. 2015, 809, 157. [Google Scholar] [CrossRef]
- Shapiro, A.I.; Schmutz, W.; Schoell, M.; Haberreiter, M.; Rozanov, E. NLTE solar irradiance modeling with the COSI code. Astron. Astrophys. 2010, 517, A48. [Google Scholar] [CrossRef]
- Tagirov, R.V.; Shapiro, A.I.; Schmutz, W. NESSY: NLTE spectral synthesis code for solar and stellar atmospheres. Astron. Astrophys. 2017, 603, A27. [Google Scholar] [CrossRef]
- Witzke, V.; Shapiro, A.I.; Cernetic, M.; Tagirov, R.V.; Kostogryz, N.M.; Anusha, L.S.; Unruh, Y.C.; Solanki, S.K.; Kurucz, R.L. MPS-ATLAS: A fast all-in-one code for synthesising stellar spectra. Astron. Astrophys. 2021, 653, A65. [Google Scholar] [CrossRef]
- Kurucz, R. CD-ROM 13, ATLAS9 Stellar Atmosphere Programs and 2 km/s Grid; SAO: Cambridge, MA, USA, 1993. [Google Scholar]
- Husser, T.O.; Wende-von Berg, S.; Dreizler, S.; Homeier, D.; Reiners, A.; Barman, T.; Hauschildt, P.H. A new extensive library of PHOENIX stellar atmospheres and synthetic spectra. Astron. Astrophys. 2013, 553, A6. [Google Scholar] [CrossRef]
- Gröbner, J.; Kröger, I.; Egli, L.; Hülsen, G.; Riechelmann, S.; Sperfeld, P. The high-resolution extraterrestrial solar spectrum (QASUMEFTS) determined from ground-based solar irradiance measurements. Atmos. Meas. Tech. 2017, 10, 3375–3383. [Google Scholar] [CrossRef]
- Langley, S. The Bolometer and Radiant Energy; American Academy of Arts and Sciences: Cambridge, MA, USA, 1881. [Google Scholar]
- Toon, G.C. Solar Line List for the TCCON 2014 Data Release; California Institute of Technology: Pasadena, CA, USA, 2015. [Google Scholar] [CrossRef]
- Schöll, M.; Dudok de Wit, T.; Kretzschmar, M.; Haberreiter, M. Making of a solar spectral irradiance dataset I: Observations, uncertainties, and methods. J. Space Weather Space Clim. 2016, 6, A14. [Google Scholar] [CrossRef]
- Meftah, M.; Hochedez, J.F.; Irbah, A.; Hauchecorne, A.; Boumier, P.; Corbard, T.; Turck-Chièze, S.; Abbaki, S.; Assus, P.; Bertran, E.; et al. Picard SODISM, a Space Telescope to Study the Sun from the Middle Ultraviolet to the Near Infrared. Solar Phys. 2014, 289, 1043–1076. [Google Scholar] [CrossRef]
- Meftah, M.; Hauchecorne, A.; Irbah, A.; Cessateur, G.; Bekki, S.; Damé, L.; Bolsée, D.; Pereira, N. Solar Spectral Irradiance at 782 nm as Measured by the SES Sensor Onboard Picard. Solar Phys. 2016, 291, 1043–1057. [Google Scholar] [CrossRef]
- Witzke, V.; Duehnen, H.; Shapiro, A.; Przybylski, D.; Bhatia, T.; Cameron, R.; Solanki, S. Small-scale Dynamo in Cool Stars. II. The effect of metallicity. Astron. Astrophys. 2023, 669, A157. [Google Scholar] [CrossRef]
- Cernetic, M.; Shapiro, A.I.; Witzke, V.; Krivova, N.A.; Solanki, S.K.; Tagirov, R.V. Opacity distribution functions for stellar spectra synthesis. Astron. Astrophys. 2019, 627, A157. [Google Scholar] [CrossRef]
- Kurucz, R.L. ATLAS12, SYNTHE, ATLAS9, WIDTH9, et cetera. Mem. Della Soc. Astron. Ital. Suppl. 2005, 8, 14. [Google Scholar]
- Yelles Chaouche, L.; Cameron, R.H.; Solanki, S.K.; Riethmüller, T.L.; Anusha, L.S.; Witzke, V.; Shapiro, A.I.; Barthol, P.; Gandorfer, A.; Gizon, L.; et al. Power spectrum of turbulent convection in the solar photosphere. Astron. Astrophys. 2020, 644, A44. [Google Scholar] [CrossRef]
- Anusha, L.S.; Shapiro, A.I.; Witzke, V.; Cernetic, M.; Solanki, S.K.; Gizon, L. Radiative Transfer with Opacity Distribution Functions: Application to Narrowband Filters. Astrophys. J. Suppl. Ser. 2021, 255, 3. [Google Scholar] [CrossRef]
- Vögler, A.; Shelyag, S.; Schüssler, M.; Cattaneo, F.; Emonet, T.; Linde, T. Simulations of magneto-convection in the solar photosphere. Equations, methods, and results of the MURaM code. Astron. Astrophys. 2005, 429, 335–351. [Google Scholar] [CrossRef]
- Kurucz, R.L. Including all the lines. Can. J. Phys. 2011, 89, 417–428. [Google Scholar] [CrossRef]
- Ryabchikova, T.; Piskunov, N.; Kurucz, R.L.; Stempels, H.C.; Heiter, U.; Pakhomov, Y.; Barklem, P.S. A major upgrade of the VALD database. JPhysica Scr. 2015, 90, 054005. [Google Scholar] [CrossRef]
- Meftah, M.; Sarkissian, A.; Reberac, A. SOLAR-HRS: The HRS High-Resolution Extraterrestrial Solar Reference Spectra for Disk-Integrated, Disk-Center, and Intermediate Cases; LATMOS: Paris, France, 2022. [Google Scholar] [CrossRef]
- Kuze, A.; Suto, H.; Nakajima, M.; Hamazaki, T. Thermal and near infrared sensor for carbon observation Fourier-transform spectrometer on the Greenhouse Gases Observing Satellite for greenhouse gases monitoring. Appl. Opt. 2009, 48, 6716–6733. [Google Scholar] [CrossRef]
- Uitenbroek, H. Multilevel Radiative Transfer with Partial Frequency Redistribution. Astrophys. J. 2001, 557, 389–398. [Google Scholar] [CrossRef]
- Meftah, M.; Damé, L.; Keckhut, P.; Bekki, S.; Sarkissian, A.; Hauchecorne, A.; Bertran, E.; Carta, J.P.; Rogers, D.; Abbaki, S.; et al. UVSQ-SAT, a Pathfinder CubeSat Mission for Observing Essential Climate Variables. Remote Sens. 2019, 12, 92. [Google Scholar] [CrossRef]
- Meftah, M.; Boust, F.; Keckhut, P.; Sarkissian, A.; Boutéraon, T.; Bekki, S.; Damé, L.; Galopeau, P.; Hauchecorne, A.; Dufour, C.; et al. INSPIRE-SAT 7, a Second CubeSat to Measure the Earth’s Energy Budget and to Probe the Ionosphere. Remote Sens. 2022, 14, 186. [Google Scholar] [CrossRef]
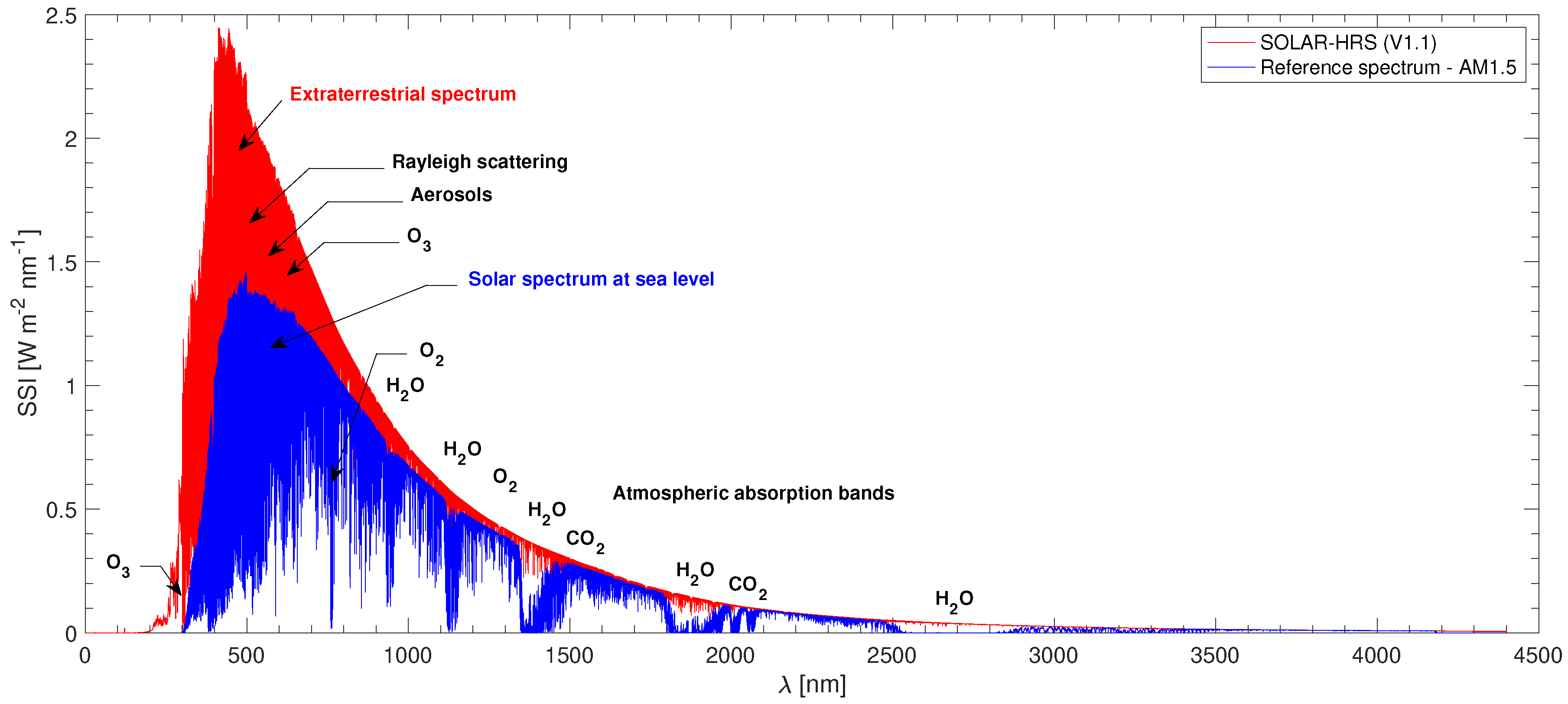
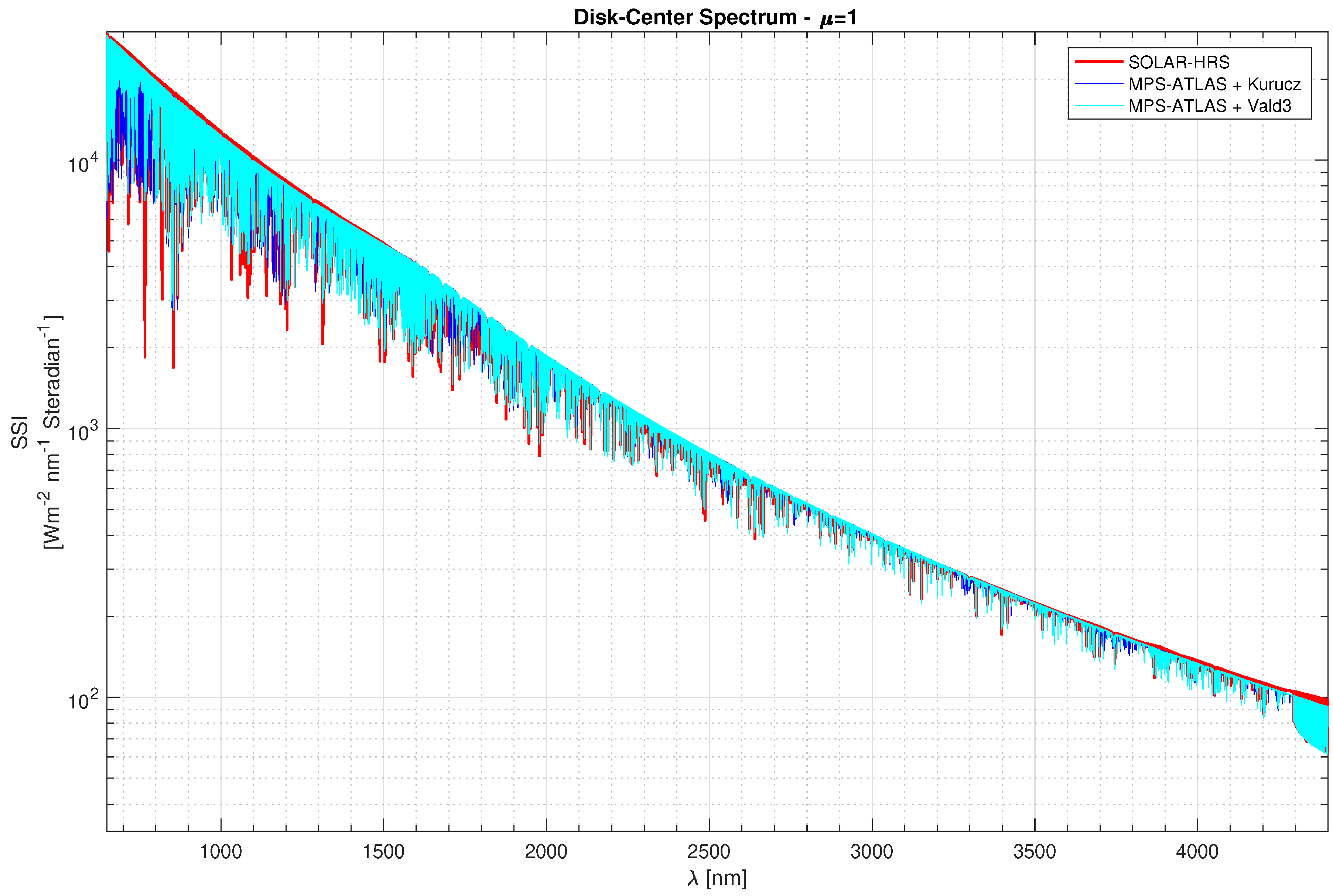

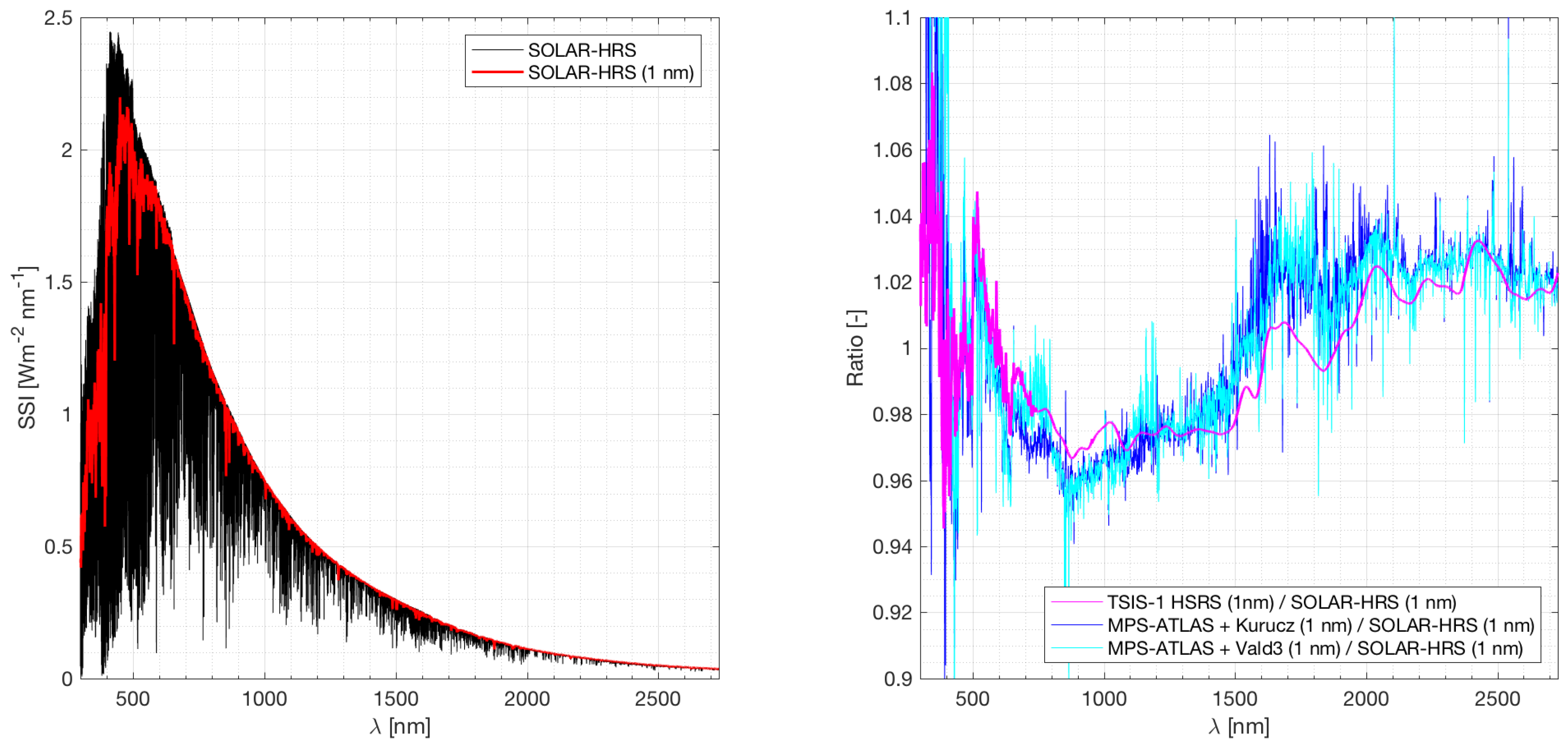


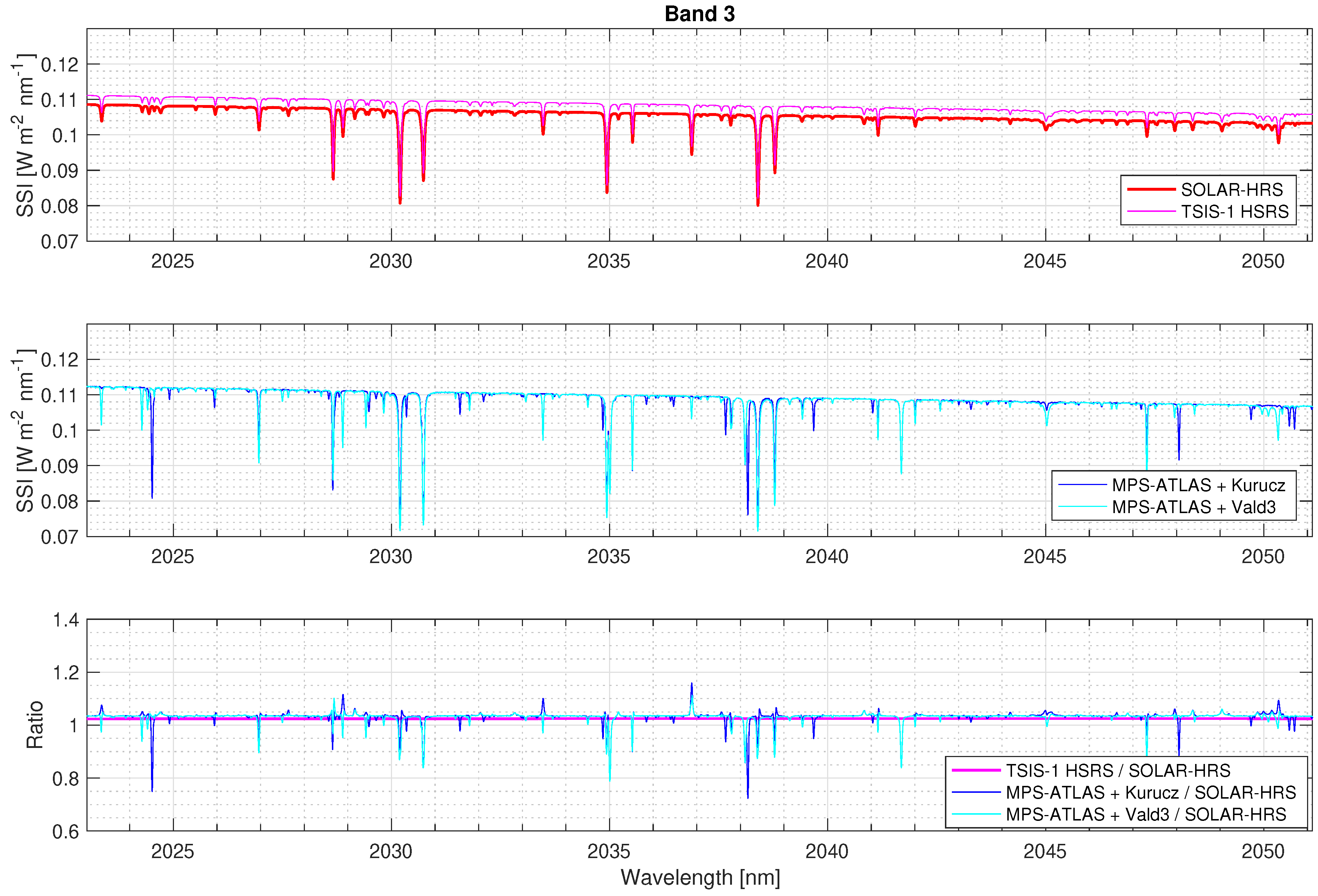


| Parameter | B1 | B2 | B3 | B4 |
|---|---|---|---|---|
| (nm) | 758.281 | 1596.772 | 2023.018 | 1264.630 |
| (nm) | 763.500 | 1607.900 | 2037.100 | 1273.400 |
| (nm) | 768.817 | 1618.946 | 2051.116 | 1282.191 |
| FWHM (nm) | 0.02966200 | 0.06226379 | 0.07900023 | 0.04932027 |
| Sampling (nm) | 0.01033519 | 0.02169470 | 0.02752621 | 0.01724485 |
| Atmospheric gas | O | CO | CO | O |
| Parameter | SOLAR-HRS | B1, B2, B3, and B4 |
|---|---|---|
| Wavelength range (nm) | 0.5–4400 | 763.5, 1607.9, 2037.1, and 1273.4 |
| Sampling resolution (nm) | <0.1 | <0.004 |
| Spectral resolution (nm) | <0.1 | 0.004 |
| Absolute uncertainty (%) | <2 | 1 |
| Central line position (nm) | <10 | 10 |
| Fraunhofer line shape (%) | <1 | 0.1 |
| Dataset Name | Data Type | Wavelength Coverage | Spectral Resolution | Sampling |
|---|---|---|---|---|
| SOLAR-HRS Disk-integrated spectrum | Composite Solar spectral irradiance | 0.5–4399.1 nm | SOLAR-ISS (<300 nm): <1.0 nm QASUMEFTS (300–380 nm): <0.025 nm SPTS (>380 nm): <0.01 nm | <0.02 nm |
| SOLAR-HRS Disk-center ( = 1.0) | Composite Solar spectral irradiance | 650.0–4399.1 nm | SPTS: <0.01 nm | <0.02 nm |
| SOLAR-HRS Intermediate cases Solar positions = 1.0, 0.9, 0.8, 0.7, 0.6, 0.5, 0.4, 0.3, 0.2, 0.1, 0.05 | Composite Solar spectral irradiance | 650.0–4399.1 nm | SPTS: <0.01 nm | <0.02 nm |
| SOLAR-HRS AM1.5 Disk-integrated spectrum | Composite Solar spectral irradiance | 0.5–4399.1 nm | SOLAR-ISS (<300 nm): <0.1 nm QASUMEFTS (300–380 nm): <0.025 nm SPTS (>380 nm): <0.01 nm | <0.02 nm |
| SOLAR-HRS AM1.5 (air) Disk-integrated spectrum | Composite Solar spectral irradiance | 0.5–4399.1 nm | SOLAR-ISS (<300 nm): <0.1 nm QASUMEFTS (300–380 nm): <0.025 nm SPTS (>380 nm): <0.01 nm | <0.02 nm |
| MPS-ATLAS-Kurucz Disk-integrated spectrum | Solar model | 250.0–5000.0 nm | <0.01 nm | <0.01 nm |
| MPS-ATLAS-Kurucz Disk-center ( = 1.0) | Solar model | 250.0–5000.0 nm | <0.01 nm | <0.01 nm |
| MPS-ATLAS-Vald3 Disk-integrated spectrum | Solar model | 250.0–5000.0 nm | <0.01 nm | <0.01 nm |
| MPS-ATLAS-Vald3 Disk-center ( = 1.0) | Solar model | 250.0–5000.0 nm | <0.01 nm | <0.01 nm |
| Parameter | B1, B2, B3 and B4 | Current Status |
|---|---|---|
| Sampling resolution (nm) | <0.004 | OK |
| Spectral resolution (nm) | 0.004 | OK |
| Absolute uncertainty (%) | 1 | NOK ⟹ 1.94% for B2 |
| Central line position (nm) | 10 | Difficult to assess |
| Fraunhofer line shape (%) | 0.1 | NOK ⟹ 0.5% for all bands |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Meftah, M.; Sarkissian, A.; Keckhut, P.; Hauchecorne, A. The SOLAR-HRS New High-Resolution Solar Spectra for Disk-Integrated, Disk-Center, and Intermediate Cases. Remote Sens. 2023, 15, 3560. https://doi.org/10.3390/rs15143560
Meftah M, Sarkissian A, Keckhut P, Hauchecorne A. The SOLAR-HRS New High-Resolution Solar Spectra for Disk-Integrated, Disk-Center, and Intermediate Cases. Remote Sensing. 2023; 15(14):3560. https://doi.org/10.3390/rs15143560
Chicago/Turabian StyleMeftah, Mustapha, Alain Sarkissian, Philippe Keckhut, and Alain Hauchecorne. 2023. "The SOLAR-HRS New High-Resolution Solar Spectra for Disk-Integrated, Disk-Center, and Intermediate Cases" Remote Sensing 15, no. 14: 3560. https://doi.org/10.3390/rs15143560
APA StyleMeftah, M., Sarkissian, A., Keckhut, P., & Hauchecorne, A. (2023). The SOLAR-HRS New High-Resolution Solar Spectra for Disk-Integrated, Disk-Center, and Intermediate Cases. Remote Sensing, 15(14), 3560. https://doi.org/10.3390/rs15143560










