Characteristics of Regional GPS Crustal Deformation before the 2021 Yunnan Yangbi Ms 6.4 Earthquake and Its Implications for Determining Potential Areas of Future Strong Earthquakes
Abstract
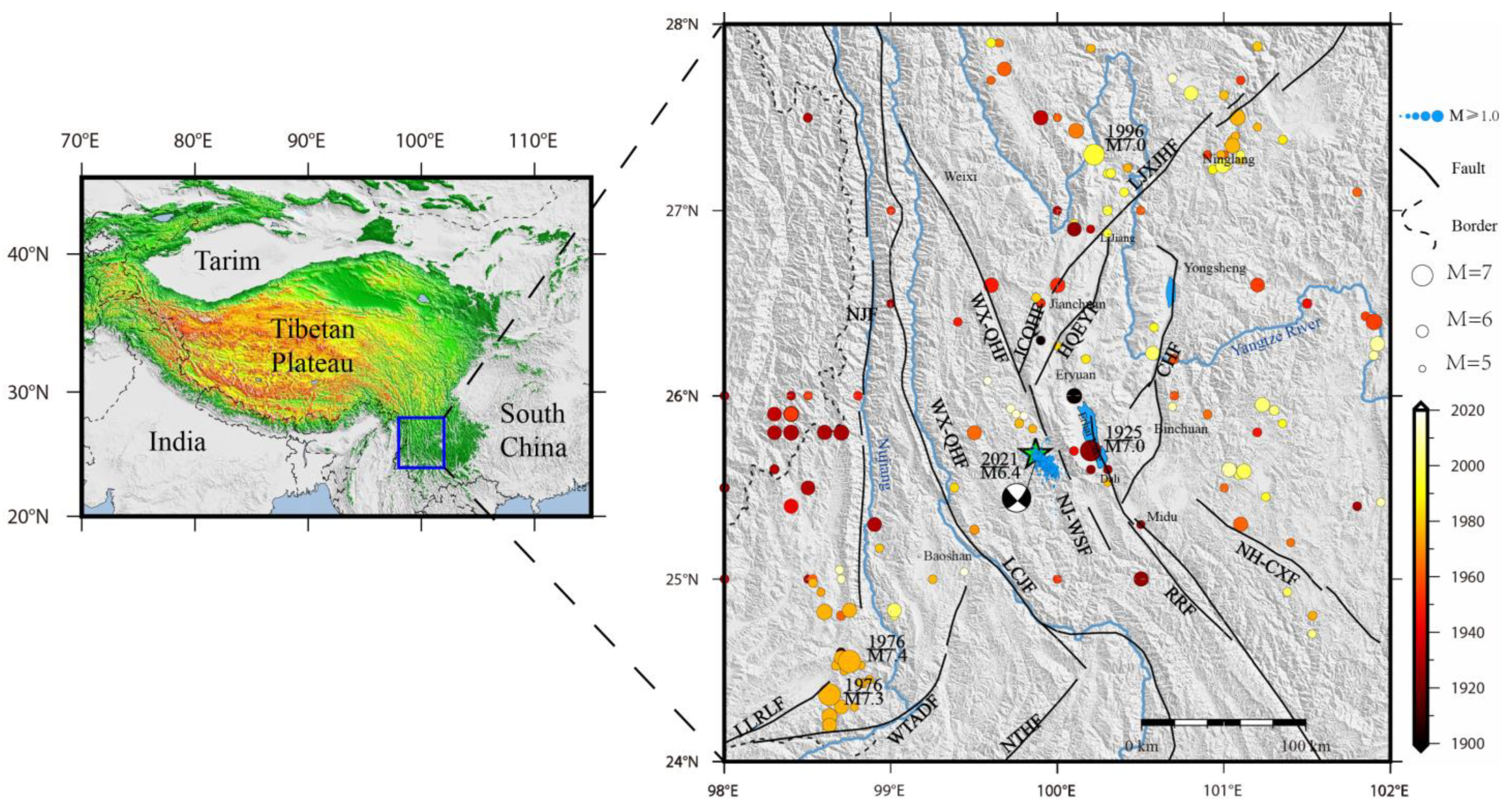
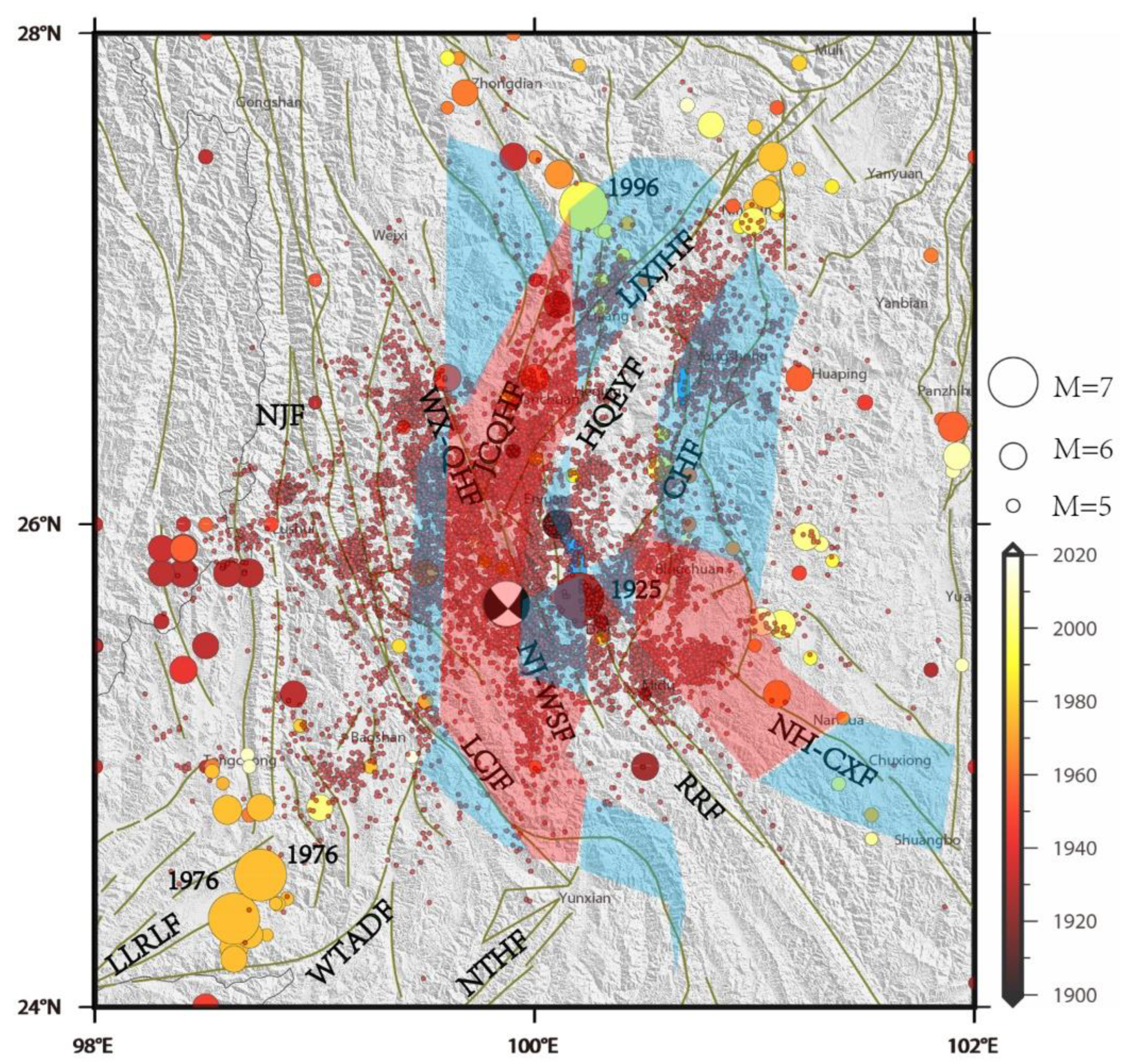
1. Introduction
2. GPS Observation Data and Data Processing
- Normalize the collected velocity vector data into a unified recording format;
- Find the common data points and ‘quasi common points’. The former refers to a point where the station name or point coordinates completely coincide; the latter refers to two points on the same tectonic block that are not completely coincident in coordinates, but are not far apart (within a few tens of meters);
- Solve the Euler vector of the difference of the velocity vector at common stations. The least squares method is used to solve the Euler rotation parameters based on the best fit of all common or “quasi common” velocity difference vectors;
- Eliminate outliers. For stations with large fitting residuals (greater than 2 times the mean square error), remove them and repeat step 3 until all residuals meet the requirements.
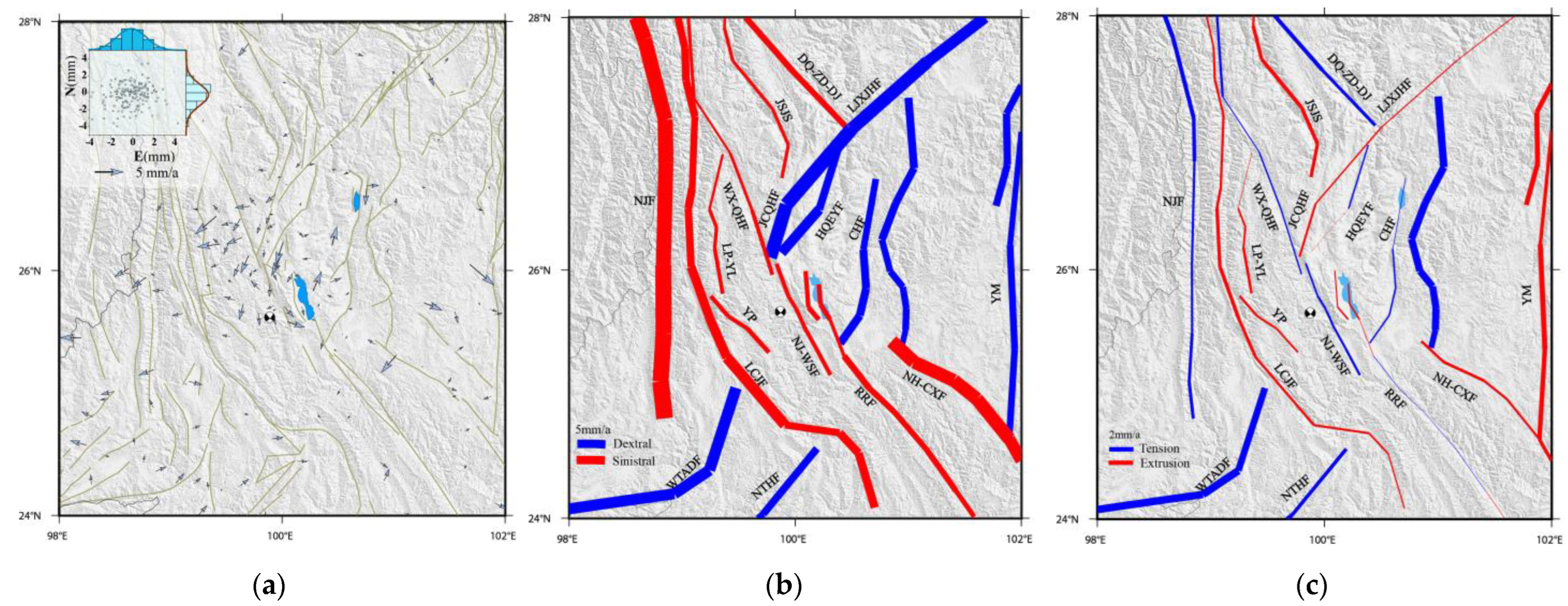
3. Regional Crustal Strain Rate Field and Its Characteristics before Earthquake
4. GPS-Constrained Inversion of Regional Main Fault Slip Rate
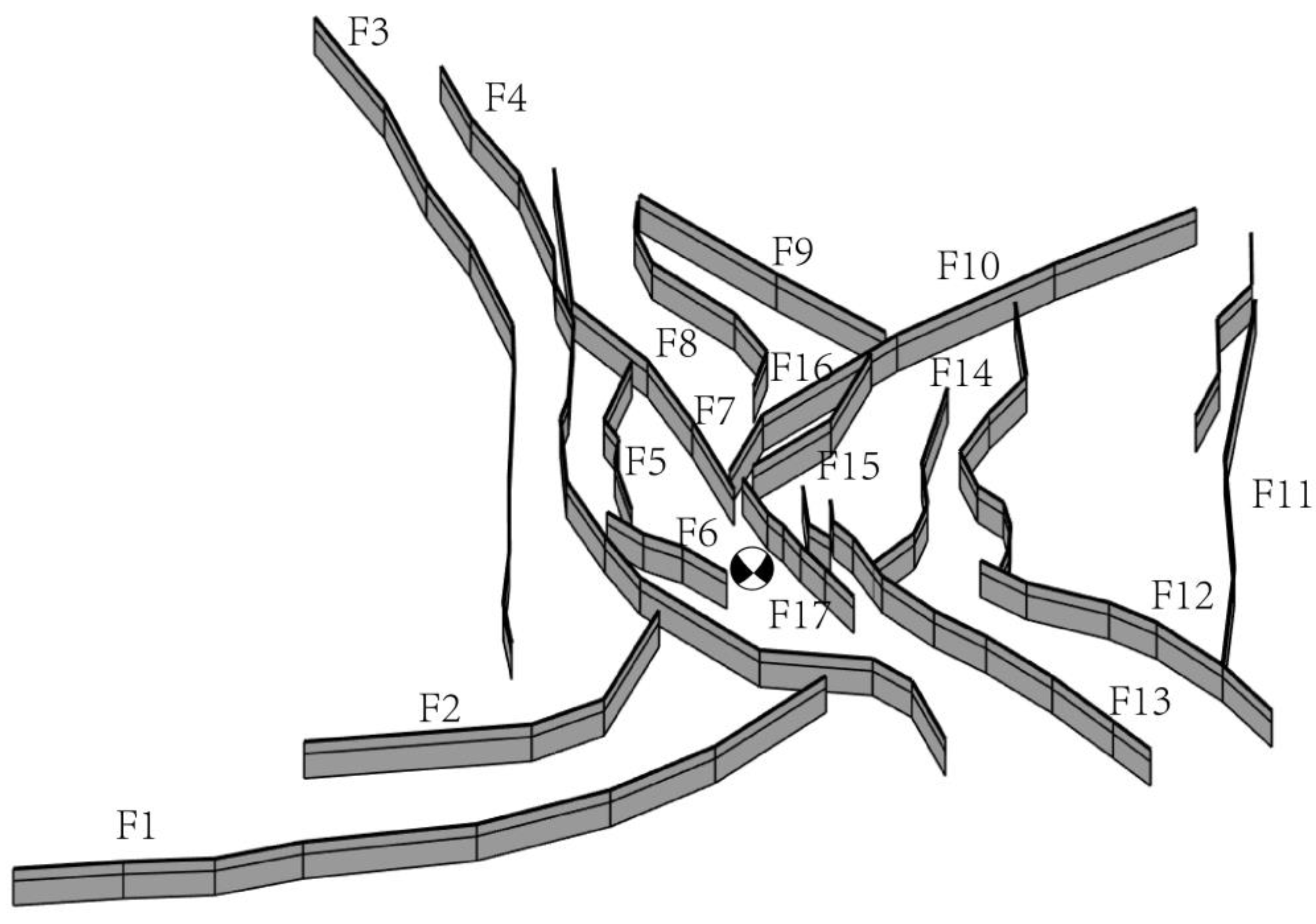
4.1. Construction of Regional Main Fault Geometry Model
4.2. GPS-Constrained Inversion Method of Fault Slip Rate
4.3. Inversion Results
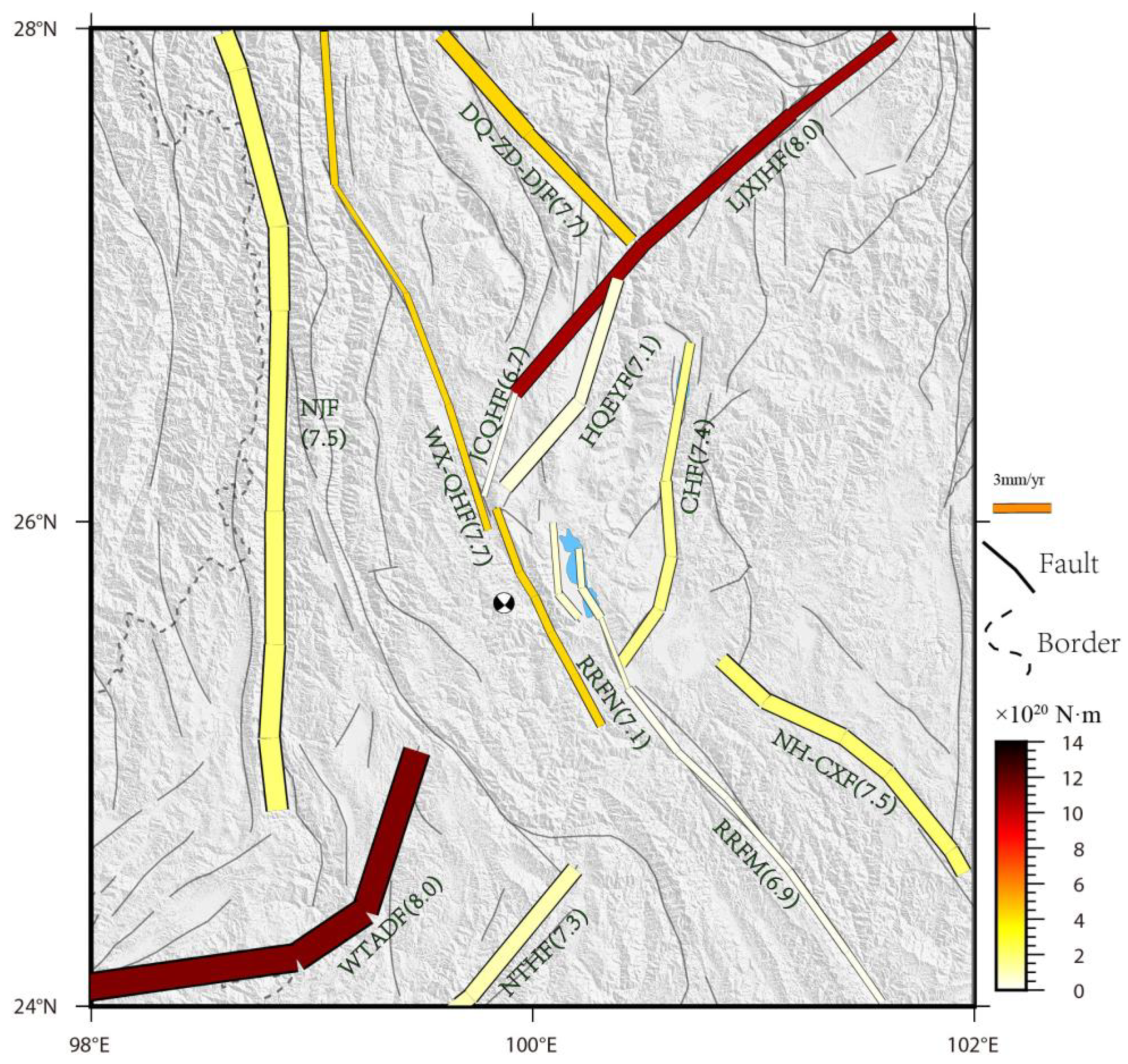
5. Seismic Moment Balance
5.1. Moment Accumulation Rate
5.2. Seismic Moment Release
5.3. Seismic Moment Deficit
6. Discussion
6.1. Seismic Hazard Analysis
- Located in the transition zone where the strain direction of the principal strain field has changed;
- Located at the junction between the high value of maximum shear strain and fault;
- Located at the edge of the transition between the surface dilatation and compression;
- Located at the position where the rotation rates on both sides are quite different.
6.2. Analysis of Fault Locking Degree
7. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Duan, M.; Zhao, C.; Zhou, L.; Zhao, C.; Zuo, K. Seismogenic structure of the 21 May 2021 MS6.4 Yunnan Yangbi earthquake sequence. Chin. J. Geophys. 2021, 64, 3111–3125. [Google Scholar] [CrossRef]
- Li, C.; Zhang, J.; Wang, W.; Sun, K.; Dan, X. The Seismogenic Fault of the 2021Yunnan Yangbi Ms6.4 Earthquake. Seismol. Geol. 2021, 43, 706–721. [Google Scholar] [CrossRef]
- Wang, G.; Wu, Z.; Peng, G.; Liu, Z.; Luo, R.; Huang, X.; Chen, H. Seismogenic fault and it’s rupture characteristics of the 21 May, 2021 Yangbi MS6.4 earthquake: Analysis results from the relocation of the earthquake sequence. J. Geomech. 2021, 27, 662–678. [Google Scholar]
- Zhang, K.; Gan, W.; Liang, S.; Xiao, G.; Dai, C.; Wang, Y.; Li, Z.; Zhang, L.; Ma, G. Coseismic displacement and slip distribution of the 21 May 2021, MS6.4, Yangbi Earthquake derived from GNSS observations. Chin. J. Geophys. 2021, 64, 2253–2266. [Google Scholar] [CrossRef]
- Wu, Z.; Zhao, X.; Fan, T.; Ye, P.; Tong, Y.; Yang, Z. Active faults and seismologic characteristics along the Dali-Ruili railway in western Yunnan Province. Geol. Bull. Chin. 2012, 31, 191–217. [Google Scholar]
- Wang, M.; Shen, Z. Present-day crustal deformation of continental China derived from GPS and its tectonic implications. J. Geophys. Res. Solid Earth 2020, 125, e2019JB018774. [Google Scholar] [CrossRef]
- Zhao, G.; Meng, G.; Wu, W.; Su, X.; Pan, Z. Earthquake Potential Assessment Around the Southeastern Tibetan Plateau Based on Seismic and Geodetic Data. Pure Appl. Geophys. 2022, 179, 11–44. [Google Scholar] [CrossRef]
- Yang, T.; Li, B.; Fang, L.; Su, Y.; Zhong, Y.; Yang, J.; Qin, M.; Xu, Y. Relocation of the Foreshocks and Aftershocks of the 2021 Ms 6.4 Yangbi Earthquake Sequence, Yunnan, China. J. Earth Sci. 2022, 33, 892–900. [Google Scholar] [CrossRef]
- Xu, W.; Gao, M. Statistical analysis of the completeness of earthquake catalogs in China mainland. Chin. J. Geophys. 2014, 57, 2802–2812. [Google Scholar] [CrossRef]
- Gan, W.; Zhang, R.; Zhang, Y.; Tang, F. Development of the Crustal Movement Observation Network in China and its Applications. Recent Dev. World Seismol. 2007, 7, 43–52. [Google Scholar]
- Gan, W.; Li, Q.; Zhang, R.; Shi, H. Construction and Application of Tectonic and Environmental Observation Network of China’s mainland. J. Eng. Stud. 2012, 4, 324–331. [Google Scholar]
- Liang, S.; Gan, W.; Xiao, G.; Wang, G.; Dai, C.; Zhang, K.; Dai, D.; Li, Z.; Zhang, L.; Zhang, Y.; et al. Strong Ground Motion Recorded by High-Rate GPS during the 2021 Ms 6.4 Yangbi, China, Earthquake. Seismol. Res. Lett. 2022, 93, 3219–3233. [Google Scholar] [CrossRef]
- Webb, F.; Zumberge, J. An Introduction to GIPSY/OASIS-II Precision Software for the Analysis of Data from GLobal Positioning System; JPL Publ.: La Cañada Flintridge, CA, USA, 1993; p. D-11088. [Google Scholar]
- Zumberge, J.; Heflin, M.; Jefferson, D.; Watkins, M.; Webb, F. Precise point positioning for the efficient and robust analysis of GPS data from large networks. J. Geophys. Res. Solid Earth 1997, 102, 5005–5017. [Google Scholar] [CrossRef]
- Dong, D.; Fang, P.; Bock, Y.; Webb, F.; Prawirodirdjo, L.; Kedar, S.; Jamason, P. Spatiotemporal filtering using principal component analysis and Karhunen-Loeve expansion approaches for regional GPS network analysis. J. Geophys. Res. Solid Earth 2006, 111, B03405. [Google Scholar] [CrossRef]
- Altamimi, Z.; Metivier, L.; Rebischung, P.; Rouby, H.; Collilieux, X. ITRF2014 plate motion model. Geophys. J. Int. 2017, 209, 1906–1912. [Google Scholar] [CrossRef]
- Gan, W.; Zhang, P.; Shen, Z.; Niu, Z.; Wang, M.; Wan, Y.; Zhou, D.; Cheng, J. Present-day crustal motion within the Tibetan Plateau inferred from GPS measurements. J. Geophys. Res. Solid Earth 2007, 112, B08416. [Google Scholar] [CrossRef]
- Shen, Z.K. Crustal deformation across and beyond the Los Angeles. basin from geodetic measurements. J. Geophys. Res. Solid Earth 1996, 101, 27957–27980. [Google Scholar] [CrossRef]
- Shen, Z.; Wang, M.; Zeng, Y.; Wang, F. Optimal Interpolation of Spatially Discretized Geodetic Data. Bull. Seismol. Soc. Amer. 2015, 105, 2117–2127. [Google Scholar] [CrossRef]
- Wu, Y.; Jiang, Z.; Yang, G.; Wei, W.; Liu, X. Comparison of GPS strain rate computing methods and their reliability. Geophys. J. Int. 2011, 185, 703–717. [Google Scholar] [CrossRef]
- Jiang, Z.; Liu, J. The method in establishing strain field and velocity field of crustal movement using least squares collocation. Chin. J. Geophys. 2010, 53, 1109–1116. [Google Scholar] [CrossRef]
- Liu, X.; Jiang, Z.; Wu, Y. The applicability of kriging interpolation method in GPS velocity gridding and strain calculating. Geomat. Inf. Sci. Wuhan Univ. 2014, 39, 457–461. [Google Scholar] [CrossRef]
- Wu, Y.; Jiang, Z.; Yang, G. The Application and Method of GPS Strain Calculation in Whole Mode Using Least Square Collocation in Sphere Surface. Chin. J. Geophys. 2009, 32, 306–311. [Google Scholar] [CrossRef]
- Su, X.; Yao, L.; Wu, W.; Meng, G.; Su, L.; Xiong, R.; Hong, S. Crustal Deformation on the Northeastern Margin of the Tibetan Plateau from Continuous GPS Observations. Remote Sens. 2019, 11, 34. [Google Scholar] [CrossRef]
- Wu, Y.; Jiang, Z.; Zhao, J.; Liu, X.; Wei, W.; Liu, Q.; Li, Q.; Zou, Z.; Zhang, L. Crustal deformation before the 2008 Wenchuan MS8. 0 earthquake studied using GPS data. J. Geodyn. 2015, 85, 11–23. [Google Scholar] [CrossRef]
- Jiang, Z.; Ma, Z.; Zhang, X.; Wang, Q.; Wang, S. Horizontal Strain Field and Tectonic Deformation of China Mainland Revealed by Preliminary Gps Result. Chin. J. Geophys. 2003, 46, 352–358. [Google Scholar] [CrossRef]
- Savage, J.; Simpson, R. Surface strain accumulation and the seismic moment tensor. Bull. Seismol. Soc. Amer. 1997, 87, 1345–1353. [Google Scholar] [CrossRef]
- Li, Z.; Wang, Y.; Gan, W.; Fang, L.; Zhou, R.; Seagren, E.G.; Zhang, H.; Liang, S.; Zhuang, W.; Yang, F. Diffuse Deformation in the SE Tibetan Plateau: New Insights from Geodetic Observations. J. Geophys. Res. Solid Earth 2020, 125, e2020JB019383. [Google Scholar] [CrossRef]
- Meade, B.; Hager, B. Block models of crustal motion in southern California constrained by GPS measurements. J. Geophys. Res. Solid Earth 2005, 110, B03403. [Google Scholar] [CrossRef]
- Meade, B. Present-day kinematics at the India-Asia collision zone. Geology 2007, 35, 81–84. [Google Scholar] [CrossRef]
- Li, Y.; Hao, M.; Ji, L.; Qin, S. Fault slip rate and seismic moment deficit on major active faults in mid and south part of the Eastern margin of Tibet plateau. Chin. J. Geophys. 2014, 57, 1062–1078. [Google Scholar] [CrossRef]
- Wang, Y.; Wang, E.; Shen, Z.; Wang, M.; Gan, W.; Qiao, X.; Meng, G.; Li, T.; Tao, W.; Yang, Y.; et al. GPS-constrained inversion of present-day slip rates along major faults of the Sichuan-Yunnan region, China. Sci. China Ser. D Earth Sci. 2008, 51, 582–597. [Google Scholar] [CrossRef]
- Liu, Y. Fine Velocity Structure and Earthquake Relocation in Source and Peripheral Area of Eryuan Earthquake. Master’s Thesis, China University of Geosciences, Beijing, China, 2020. [Google Scholar]
- Okada, Y. Internal deformation due to shear and tensile faults in a half-space. Bull. Seismol. Soc. Amer. 1992, 82, 1018–1040. [Google Scholar] [CrossRef]
- Comninou, M.; Dundurs, J. The angular dislocation in a half space. J. Elast. 1975, 5, 203–216. [Google Scholar] [CrossRef]
- Mansinha, L.; Symlie, D.E. The displacement fields of inclined faults. Bull. Seismol. Soc. Amer. 1971, 61, 1433–1440. [Google Scholar] [CrossRef]
- Maruyama, T. Statical elastic dislocation in an infinite and semi-infinite medium. Bull. Earthq. Res. Inst. 1964, 42, 289–368. [Google Scholar]
- Smylie, D.E.; Mansinha, L. The Elasticity Theory of Dislocations in Real Earth Models and Changes in the Rotation of the Earth. Geophys. J. R. Astron. Soc. 1971, 23, 329–354. [Google Scholar] [CrossRef]
- Wang, Y.; Wang, M.; Shen, Z.; Shao, D.; Shi, F. Present-Day Slip Rates and Potential Earthquake Risks along The Nujiang Fault. Seismol. Geol. 2015, 37, 374–383. [Google Scholar] [CrossRef]
- Chang, Z.; An, X.; Zhang, Y. Study on Late-Quaternary Activity and Displacement of Drainage Systems along the Wanding Fault. Seismol. Geol. 2012, 34, 228–239. [Google Scholar] [CrossRef]
- Shi, F. Tectonic Geomorphology of the Nantinghe Fault in Southwestern Yunnan. Doctoral Dissertations, Institute of Geology, China Earthquake Administrator, Beijing, China, 2014. [Google Scholar]
- Chang, Z.; Zhang, Y.; Li, J.; Zang, Y. The Geological and Geomorphic Characteristic of Late Quaternary Activity of the Deqin-Zhongdian-Daju Fault. J. Seismol. Res. 2014, 37, 46–52. [Google Scholar]
- Xu, X.; Wen, X.; Zheng, R.; Ma, W.; Song, F.; Yu, G. The latest tectonic change pattern and its dynamic source of active blocks in Sichuan-Yunnan region. Sci. China Ser. D Earth Sci. 2003, 33, 151–162. [Google Scholar] [CrossRef]
- Guo, S.; Zhang, J.; Li, X.; Xiang, H.; Chen, T.; Zhang, G. Fault Displacement and Recurrence Intervals of Earthquakes at the Northern Segment of The Honghe Fault Zone, Yunnan Province. Seismol. Geol. 1984, 6, 1–12. [Google Scholar]
- Shi, X.; Wang, Y.; Sieh, K.; Weldon, R.; Feng, L.; Chan, C.; Liu-Zeng, J. Fault Slip and GPS Velocities Across the Shan Plateau Define a Curved Southwestward Crustal Motion Around the Eastern Himalayan Syntaxis. J. Geophys. Res. Solid Earth 2018, 123, 2502–2518. [Google Scholar] [CrossRef]
- Li, Z.; Gan, W.; Qin, S.; Hao, M.; Liang, S.; Yang, F. Present-day deformation characteristics of the southeast borderland of the Tibetan Plateau. Chin. J. Geophys. 2019, 62, 4540–4553. [Google Scholar]
- Xiang, H.; Xu, X.; Guo, S.; Zhang, W.; Li, H.; Yu, G. Sinistral Thrusting Along the Lijiang-Xiaojinhe Fault Since Quaternary and Its Geologic-Tectonic Significance—Shielding Effect of Transverse Structure of Intracontinental Active Block. Seismol. Geol. 2002, 24, 188–198. [Google Scholar]
- Huang, X.; Wu, Z.; Liu, F.; Tian, T.; Huang, X.; Zhang, D. Tectonic interpretation of the main paleoseismic landslides and their distribution characteristics in the Chenghai fault zone, Northwest Yunnan. Earth Sci. Front. 2021, 28, 125–139. [Google Scholar] [CrossRef]
- Huang, X.; Wu, Z.; Huang, X.; Luo, R. Tectonic geomorphology constrains on quaternary activity and segmentation along Chenghai-Binchuan fault zone in Northwest Yunnan, China. Earth Sci. 2018, 43, 4651–4670. [Google Scholar] [CrossRef]
- Fang, Y.; Luo, W.; Ye, H.; Zhou, Y. Late Quaternary Activity of Heqing-Eryuan Fault and Its Impact on Water Diversion Project in the Central Yunnan. S. China J. Seismol. 2019, 39, 6–13. [Google Scholar]
- Sun, C.; Li, D.; Shen, X.; Kang, Y.; Liu, R.; Zhang, Y. Holocene activity evidence on the southeast boundary fault of Heqing basin, middle segment of Heqing-Eryuan fault zone, West Yunnan Province, China. J. Mt. Sci. 2017, 14, 1445–1453. [Google Scholar] [CrossRef]
- Wang, Y.; Zhang, B.; Hou, J.; Ai, S. Late Quaternary Activity of the Qujiang Fault and Analysis of the Slip Rate. Seismol. Geol. 2015, 37, 1177–1192. [Google Scholar]
- Wang, H.; Liu, M.; Cao, J.; Shen, X.; Zhang, G. Slip rates and seismic moment deficits on major active faults in mainland China. J. Geophys. Res. Solid Earth 2011, 116, B02405. [Google Scholar] [CrossRef]
- Liu, R.; Chen, Y.; Bormann, P.; Ren, X.; Hou, J.; Zou, L.; Yang, H. Comparison between Earthquake Magnitudes Determined by China Seismograph Network and U.S. Seismograph Network (II): Surface Wave Magnitude. Acta Seismol. Sin. 2006, 19, 1–7. [Google Scholar] [CrossRef]
- Wu, Z.; Zhao, G.; Long, Z.; Zhou, C.; Fan, T. The Seismic Hazard Assessment around South-East Area of Qinghai-Xizang Plateau: A Preliminary Results from Active Tectonics System Analysis. Acta Geol. Sin. 2014, 88, 1401–1416. [Google Scholar]
- Ding, R.; Ren, J.; Zhang, S.; Lu, Y.; Liu, H. Late Quaternary Paleoearthquakes on the Middle Segment of the Lijiang-Xiaojinhe Fault, Southeastern Tibet. Seismol. Geol. 2018, 40, 622–640. [Google Scholar]
- Wang, K.; Jin, M.; Huang, Y.; Dang, W.; Li, W.; Zhuo, Y.; He, C. Temporal and spatial evolution of the 2021 Yangbi (Yunnan China) Ms6.4 earthquake sequence. Seismol. Geol. 2021, 43, 1030–1039. [Google Scholar] [CrossRef]
- Zhou, Q.; Guo, S.; Xiang, H. Principle and Method of Delineation of Potential Seismic Sources in Northeastern Yunnan Province. Seismol. Geol. 2004, 26, 761–771. [Google Scholar]
- Cheng, J.; Liu, J.; Gan, W.; Li, G. Influence of coseismic deformation of the Wenchuan earthquake on the occurrence of earthquakes on active faults in Sichuan-Yunnan region. Acta Seismol. Sin. 2009, 31, 477–490. [Google Scholar]
- Li, L.; Liu, X.; Wan, Y.; Zheng, Z. Crustal deformation characteristics and recent movement changes of main fault zones in northwest Yunnan revealed by GPS and numerical simulation. Acta Geol. Sin. 2021, 95, 3205–3219. [Google Scholar]
- Zhao, B.; Bürgmann, R.; Wang, D.; Zhang, J.; Yu, J.; Li, Q. Aseismic slip and recent ruptures of persistent asperities along the Alaska-Aleutian subduction zone. Nat. Commun. 2022, 13, 3098. [Google Scholar] [CrossRef] [PubMed]
- McCaffrey, R.; Qamar, A.; King, R.; Wells, R.; Khazaradze, G.; Williams, C.; Stevens, C.; Vollick, J.; Zwick, P. Fault locking, block rotation and crustal deformation in the Pacific Northwest. Geophys. J. Int. 2007, 169, 1315–1340. [Google Scholar] [CrossRef]
- Mccaffrey, R. Time-dependent inversion of three-component continuous GPS for steady and transient sources in northern Cascadia. Geophys. Res. Lett. 2009, 36, 2497–2502. [Google Scholar] [CrossRef]
- Li, Y.C.; Dan, X.J.; Song, X.G.; Jiang, Y.; Gan, W.J.; Qu, C.Y.; Wang, Z.J. Fault locking and slip rate deficit on the middle and southern segment of the Tancheng-Lujiang fault inverted from GPS data. Chin. J. Geophys. 2016, 59, 4022–4034. [Google Scholar] [CrossRef]
- Yang, J.; Wen, Y.; Xu, C. The 21 May 2021 MS6.4 Yangbi (Yunnan) earthquake: A shallow strike-slip event rupturing in a blind fault. Chin. J. Geophys. 2021, 64, 3101–3110. [Google Scholar] [CrossRef]
- Xu, X.; Ji, L.; Zhu, L.; Wang, G.; Zhang, W.; Li, N. The Co-Seismic Deformation Characteristics and Seismogenic Structure of the Yangbi Ms6.4 Earthquake. Seismol. Geol. 2021, 43, 771–789. [Google Scholar] [CrossRef]




| Site | Longitude | Latitude | Ve (mm/yr) | Vn (mm/yr) | Se (mm/yr) | Sn (mm/yr) | Cen |
|---|---|---|---|---|---|---|---|
| EYFY | 99.92 | 25.99 | 3.40 | −12.61 | 0.94 | 0.90 | −0.04 |
| EYNJ | 99.98 | 26.06 | 2.29 | −12.39 | 0.93 | 0.90 | −0.06 |
| EYSY | 100.01 | 26.23 | 2.42 | −15.71 | 0.93 | 0.90 | −0.01 |
| EYHH | 99.73 | 26.00 | 1.88 | −12.82 | 0.93 | 0.90 | −0.01 |
| EYLG | 99.89 | 25.89 | 2.41 | −13.14 | 0.70 | 0.69 | 0.09 |
| EYTZ | 99.84 | 26.17 | 2.93 | −13.32 | 0.70 | 0.69 | −0.01 |
| EYXP | 99.61 | 26.05 | 1.10 | −14.21 | 0.70 | 0.69 | −0.03 |
| EYYX | 99.70 | 26.12 | 0.99 | −15.11 | 0.70 | 0.69 | 0.04 |
| JCMS | 99.64 | 26.27 | −0.34 | −13.63 | 0.58 | 0.58 | 0.10 |
| JCXT | 99.52 | 26.22 | 1.19 | −13.97 | 0.58 | 0.58 | 0.03 |
| JCYC | 99.71 | 26.52 | 0.89 | −13.31 | 0.58 | 0.57 | 0.00 |
| JCHC | 99.94 | 26.38 | 2.62 | −13.33 | 0.72 | 0.71 | −0.07 |
| JCMD | 99.56 | 26.39 | 0.64 | −12.95 | 0.58 | 0.57 | −0.02 |
| JCSH | 99.86 | 26.25 | 3.77 | −12.62 | 0.58 | 0.57 | −0.06 |
| JCSL | 99.80 | 26.38 | 2.88 | −14.53 | 0.71 | 0.70 | −0.04 |
| YBZM | 100.02 | 25.71 | 3.77 | −12.24 | 0.71 | 0.70 | 0.01 |
| YBZZ | 99.79 | 25.66 | 2.12 | −12.69 | 0.70 | 0.69 | 0.01 |
| YBXL | 99.91 | 25.64 | 2.52 | −11.94 | 0.70 | 0.69 | 0.00 |
| YLCX | 99.43 | 26.05 | 0.39 | −12.54 | 0.58 | 0.58 | −0.13 |
| YLZH | 99.44 | 26.21 | 0.85 | −11.03 | 1.28 | 1.41 | 0.07 |
| YLGP | 99.56 | 25.90 | 0.97 | −11.18 | 0.58 | 0.58 | −0.03 |
| YLTJ | 99.65 | 25.73 | 1.09 | −13.84 | 0.58 | 0.58 | −0.11 |
| YSXG | 100.45 | 26.20 | 5.44 | −8.71 | 1.35 | 1.47 | −0.04 |
| BCHQ | 100.43 | 25.91 | 5.72 | −10.66 | 1.33 | 1.47 | 0.08 |
| DLWS | 100.28 | 25.83 | 4.65 | −7.58 | 1.28 | 1.40 | −0.03 |
| HQHP | 100.26 | 26.10 | 2.42 | −11.67 | 1.28 | 1.20 | −0.03 |
| EYAJ | 99.80 | 25.84 | 0.70 | −9.33 | 0.67 | 0.69 | −0.07 |
| HQBX | 100.14 | 26.32 | 5.42 | −12.76 | 0.72 | 0.70 | −0.10 |
| Fault | Dip Angle | Locking Depth | Slip Rate (mm/yr) | Extrusion/Tension Rate (mm/yr) | ||
|---|---|---|---|---|---|---|
| Inversion | Geological | Inversion | Geological | |||
| Nantinghe | 90 | 25 | −2.8 ± 1.1 | −3.6 ± 0.4 | −1.6 ± 0.7 | −1.1 ± 0.3 |
| Wanting | 90 | 25 | −4.6 ± 1.0 | −1.7–3 | −2.9 ± 1.0 | |
| Nujiang | 90 | 25 | 5.5 ± 1.1 | −2.2 ± 1.0 | ||
| Northern segment of Lancangjiang fault | 90 | 25 | 3.3 ± 0.8 | 1.0 ± 0.7 | ||
| Lanping–Yunlong | 90 | 25 | 1.4 ± 0.6 | 0.1–0.19 | 0.7 ± 0.6 | |
| Yongping | 90 | 25 | 1.8 ± 0.7 | 0.1–0.19 | 0.8 ± 0.7 | |
| Yuanmou | 90 | 25 | −2.7 ± 0.7 | 1–2 | 1.9 ± 0.5 | 0.1–0.3 |
| Deqin–Zhongdian–Daju | 90 | 25 | 2.7 ± 0.6 | −1.5 ± 0.6 | ||
| South section of Jinshajiang fault (Jirada section) | 90 | 25 | 1.4 ± 0.8 | 1.5 ± 0.7 | ||
| Lijiang–Xiaojinhe | 90 | 25 | −4 ± 0.8 | −3.8 ± 0.7 | 0.6 ± 0.6 | 0.64 ± 0.14 |
| Jianchuan–Qiaohou | 90 | 25 | 0.8 ± 0.3 | 1.1 ± 0.5 | ||
| Heqing–Eryuan | 90 | 25 | −3.6 ± 0.6 | −1.8–2.0 | −0.54 ± 1.0 | |
| Chenghai | 90 | 25 | −3.1 ± 0.7 | −1–1.2 | −0.6 ± 0.7 | |
| Chuxiong–Nanhua | 90 | 25 | 5.4 ± 1.0 | 2.3–4 | 1.1 ± 0.7 | |
| Northern segment of Red River fault | 90 | 25 | 1.9 ± 0.7 | 1.0 ± 0.9 | ||
| Middle section of Red River fault | 90 | 25 | 2.5 ± 0.7 | −0.3 ± 0.6 | ||
| Weixi–Qiaohou | 90 | 25 | 1.1 ± 0.7 | 1–3 | −0.75 ± 0.7 | |
| Fault | Elapsed Time/a | Moment Accumulation Rate (1017 N·m/a) | Moment Accumulation (1020 N·m) | Moment Release (1020 N·m) | Moment Deficit (1020 N·m) | Equivalent Earthquake Magnitude |
|---|---|---|---|---|---|---|
| NTH | 81 | 13.415 | 1.087 | 0 | 1.087 | 7.3 |
| WD-AD | 1022 | 11.171 | 11.417 | 0.025 | 11.392 | 8.0 |
| NJ | 92 | 20.889 | 1.922 | 0 | 1.922 | 7.5 |
| DQ-ZD-DJ | 1022 | 4.444 | 4.542 | 0.099 | 4.353 | 7.7 |
| JC-QH | 271 | 0.542 | 0.147 | 0 | 0.147 | 6.7 |
| LJ-XJH | 1700 | 6.576 | 11.18 | 0.194 | 10.986 | 8.0 |
| HQ-EY | 183 | 3.004 | 0.550 | 0 | 0.550 | 7.1 |
| CH | 507 | 3.310 | 1.678 | 0.054 | 1.624 | 7.4 |
| NH-CX | 342 | 5.748 | 1.966 | 0.025 | 1.941 | 7.5 |
| RRN | 97 | 1.536 | 0.568 | 0 | 0.568 | 7.1 |
| RRM | 370 | 2.778 | 0.269 | 0.036 | 0.233 | 6.9 |
| WX-QH | 600 | 5.724 | 3.434 | 0.036 | 3.398 | 7.7 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Dai, C.; Gan, W.; Li, Z.; Liang, S.; Xiao, G.; Zhang, K.; Zhang, L. Characteristics of Regional GPS Crustal Deformation before the 2021 Yunnan Yangbi Ms 6.4 Earthquake and Its Implications for Determining Potential Areas of Future Strong Earthquakes. Remote Sens. 2023, 15, 3195. https://doi.org/10.3390/rs15123195
Dai C, Gan W, Li Z, Liang S, Xiao G, Zhang K, Zhang L. Characteristics of Regional GPS Crustal Deformation before the 2021 Yunnan Yangbi Ms 6.4 Earthquake and Its Implications for Determining Potential Areas of Future Strong Earthquakes. Remote Sensing. 2023; 15(12):3195. https://doi.org/10.3390/rs15123195
Chicago/Turabian StyleDai, Chenglong, Weijun Gan, Zhangjun Li, Shiming Liang, Genru Xiao, Keliang Zhang, and Ling Zhang. 2023. "Characteristics of Regional GPS Crustal Deformation before the 2021 Yunnan Yangbi Ms 6.4 Earthquake and Its Implications for Determining Potential Areas of Future Strong Earthquakes" Remote Sensing 15, no. 12: 3195. https://doi.org/10.3390/rs15123195
APA StyleDai, C., Gan, W., Li, Z., Liang, S., Xiao, G., Zhang, K., & Zhang, L. (2023). Characteristics of Regional GPS Crustal Deformation before the 2021 Yunnan Yangbi Ms 6.4 Earthquake and Its Implications for Determining Potential Areas of Future Strong Earthquakes. Remote Sensing, 15(12), 3195. https://doi.org/10.3390/rs15123195






