3D Airborne EM Forward Modeling Based on Finite-Element Method with Goal-Oriented Adaptive Octree Mesh
Abstract
1. Introduction
2. Methods
2.1. AEM forward Modeling Based on Octree Mesh
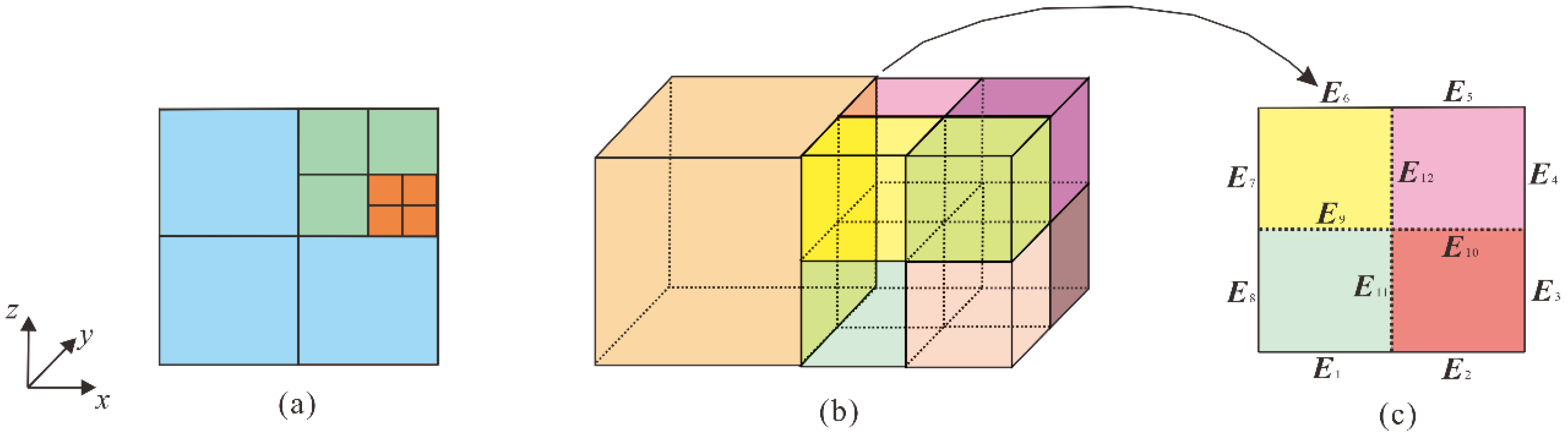
2.2. Adaptive Scheme for Octree Mesh
2.2.1. Posterior Errors Estimation for Octree Meshes
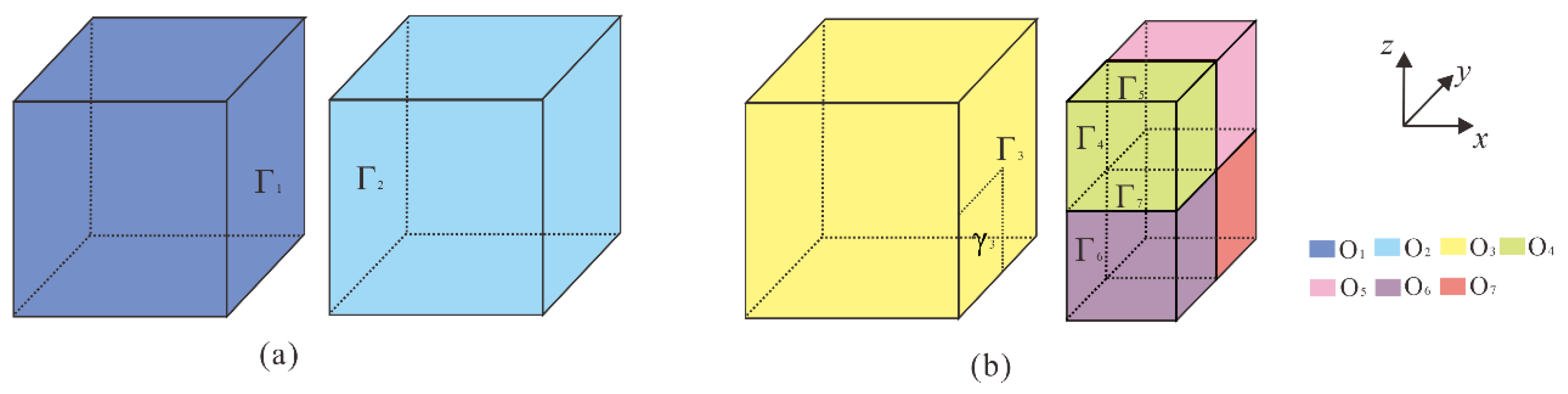
- For element O1 in Figure 2a, the relationship between elements O1 and O2 is one face to one face. Thus, when calculating the posterior error, only the differences of normal current densities between Г1 and Г2 need to be calculated.
- For element O3 in Figure 2b, the relationships with elements O4, O5, O6, and O7 are one face to four faces. Thus, when calculating the posterior error, the differences of normal current densities between Г3 and Г4, Г5, Г6, and Г7 need to be calculated.
- For elements O4, O5, O6, or O7 in Figure 2b, the relationship between them and O3 is one face to one face. Thus, when calculating the posterior errors, the differences of normal current densities between the faces Г4, Г5, Г6, Г7, and their corresponding areas on Г3 need to be calculated.
2.2.2. Influence Function
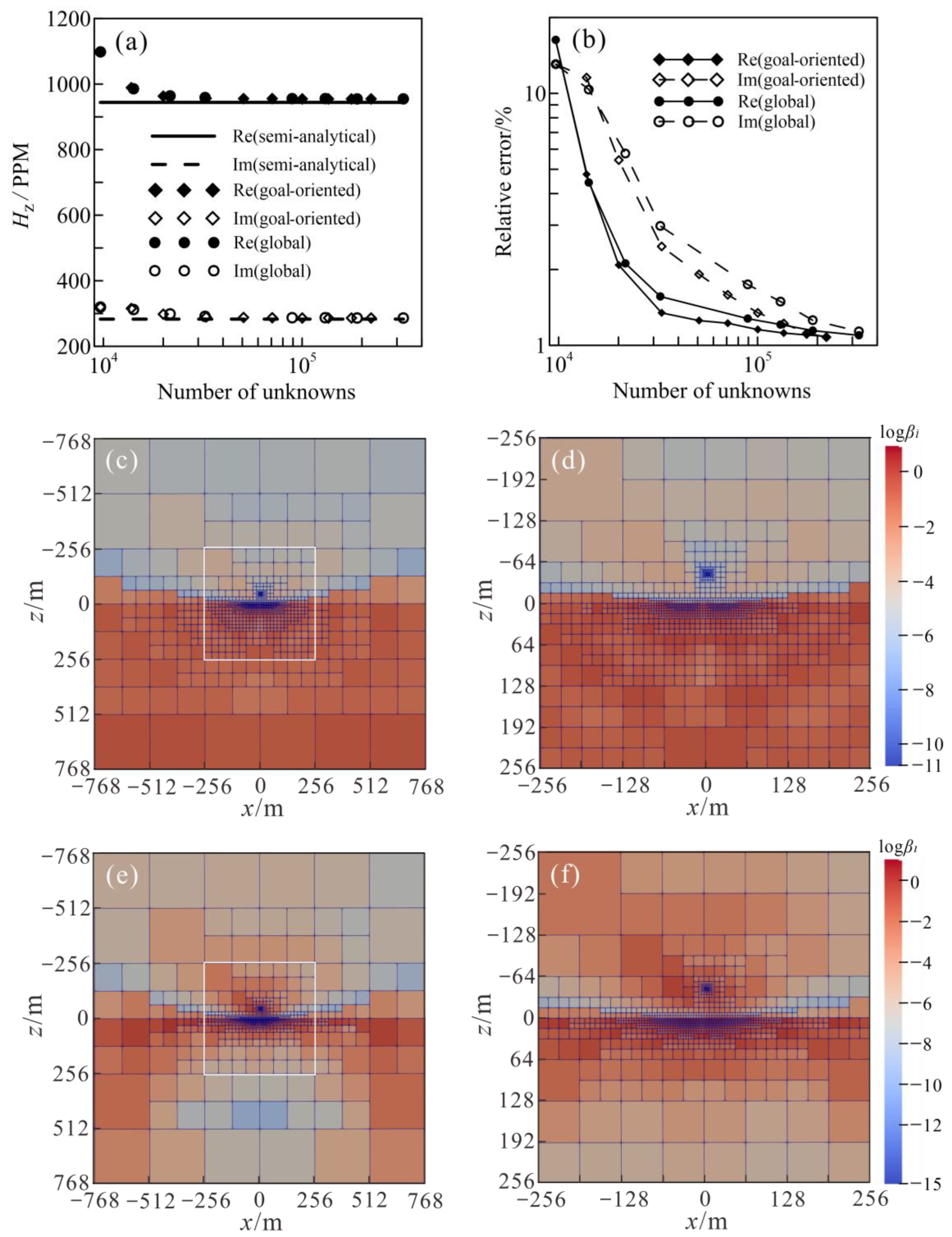
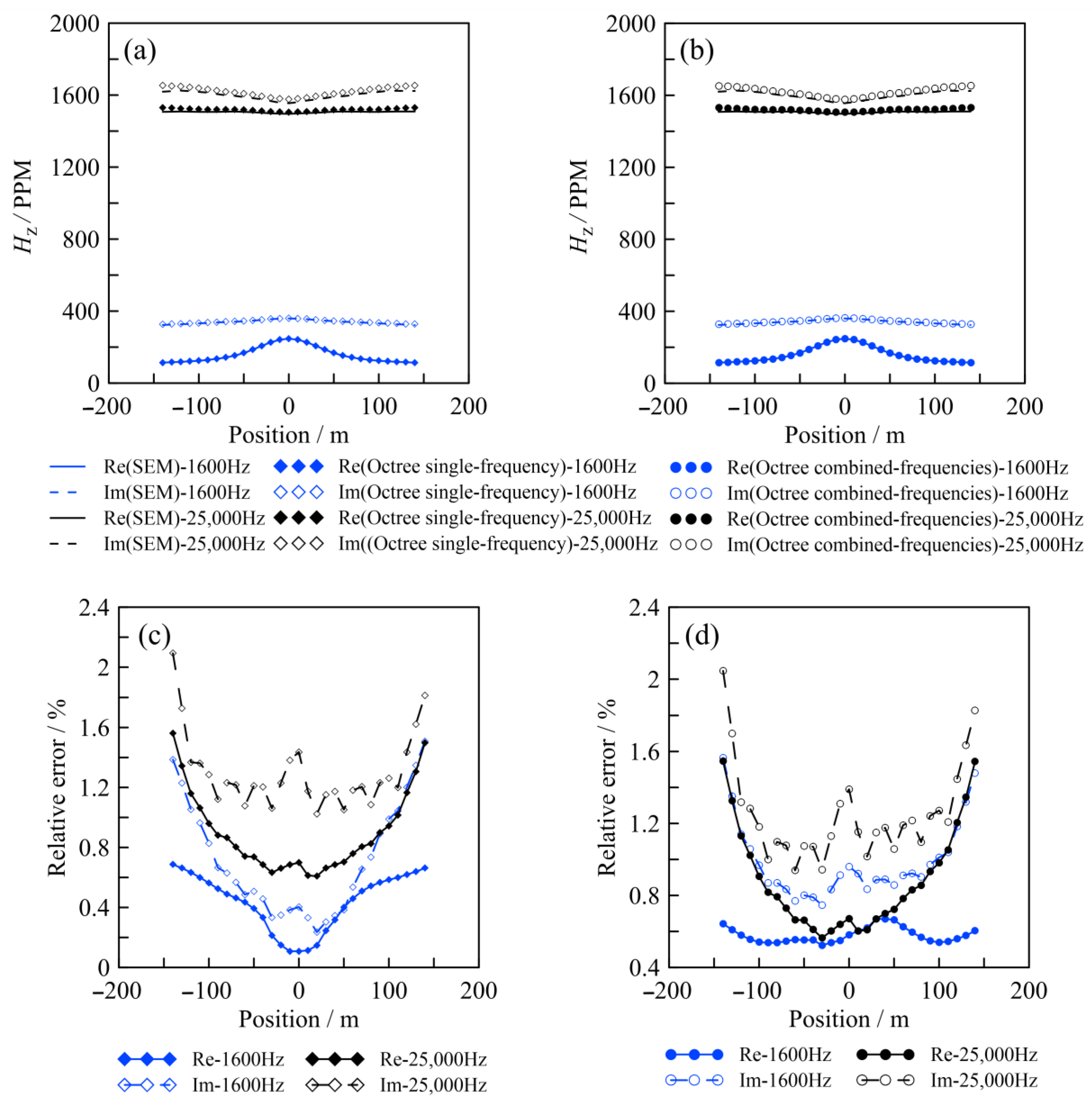
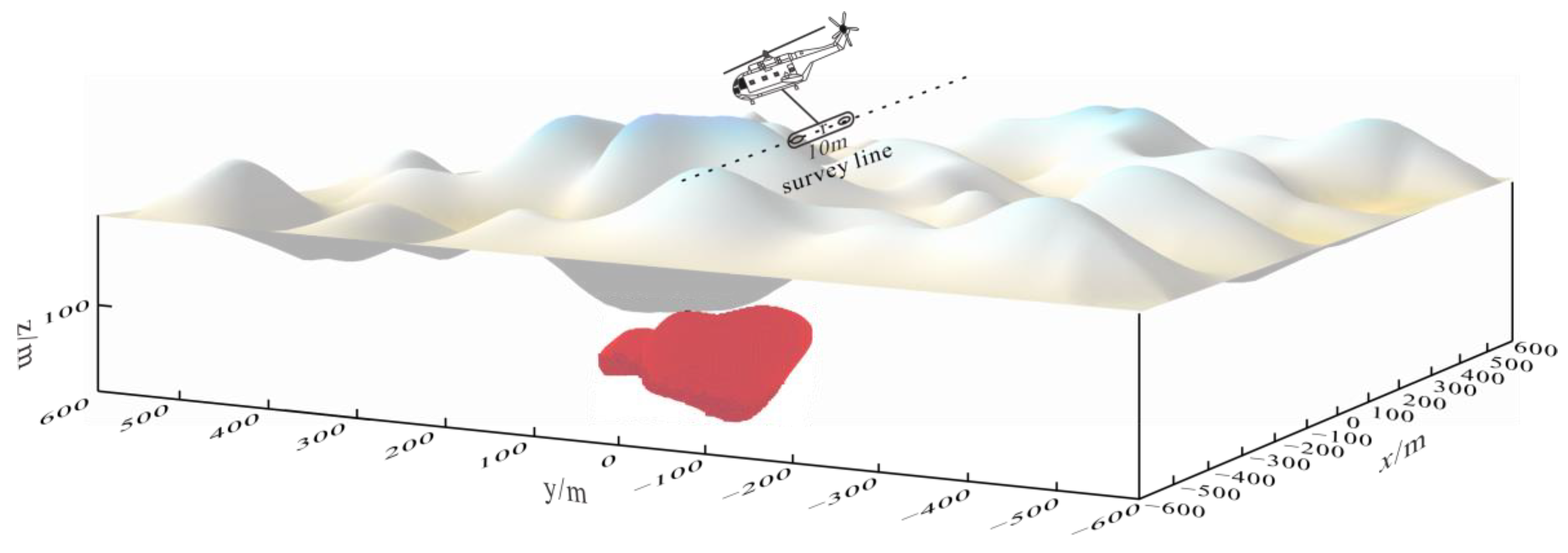
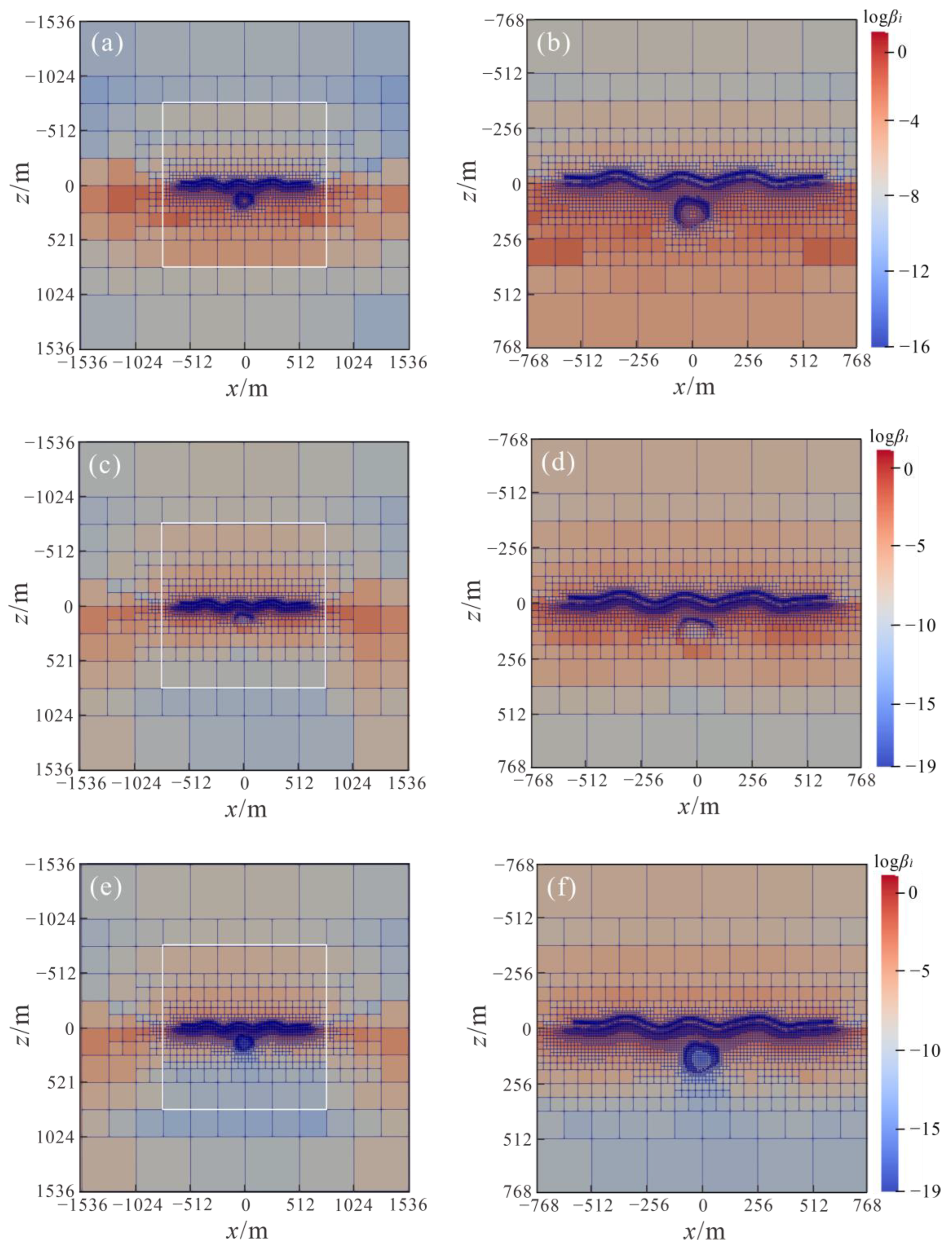
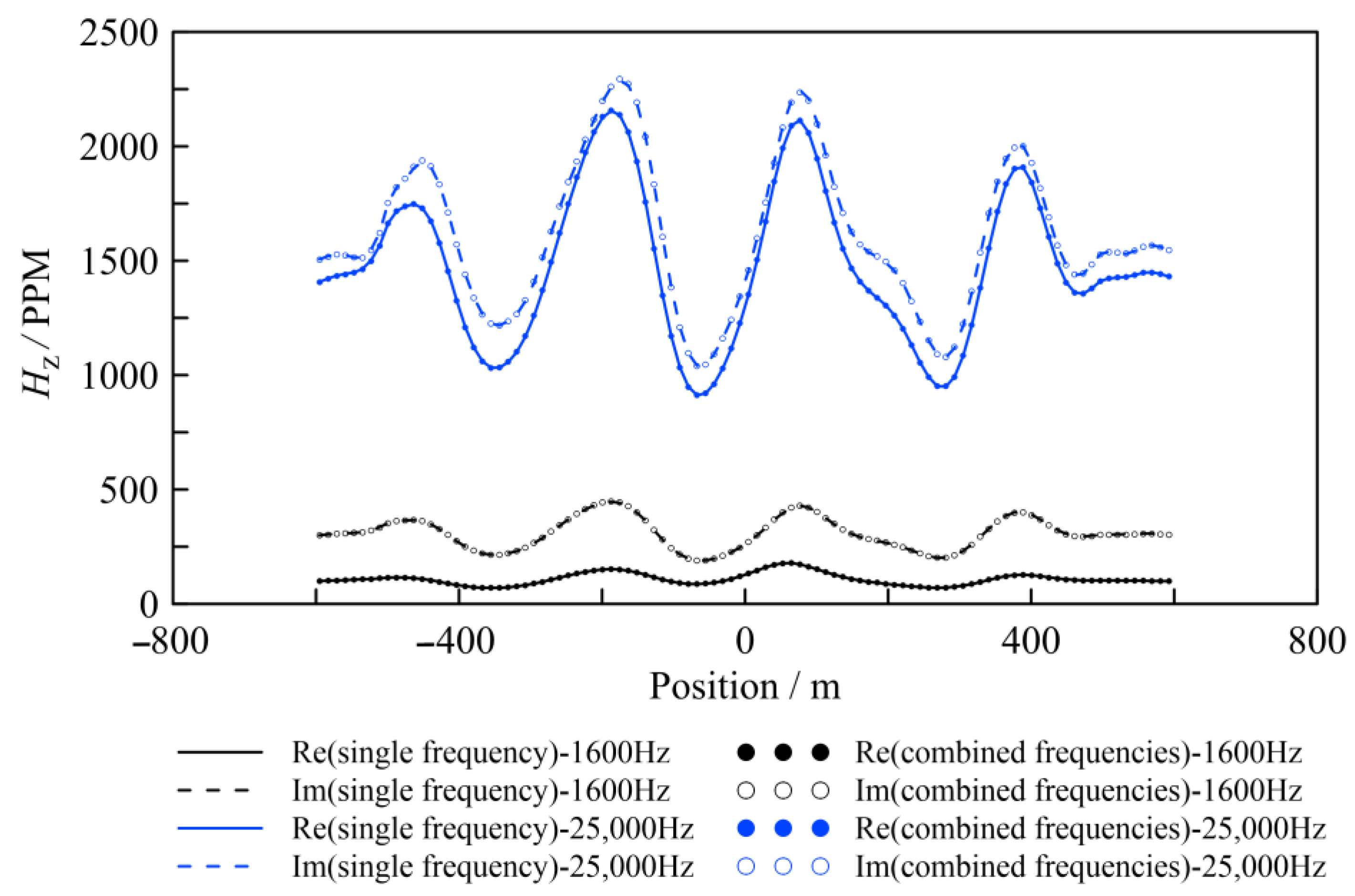
3. Numerical Experiments
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Pfaffhuber, A.A.; Monstad, S.; Rudd, J. Airborne electromagnetic hydrocarbon mapping in Mozambique. Explor. Geophys. 2009, 40, 237–245. [Google Scholar] [CrossRef]
- Yin, C.; Zhang, B.; Liu, Y.H.; Ren, X.; Cai, J. Review on airborne EM technology and developments. Chin. J. Geophys. 2015, 58, 2637–2653. [Google Scholar]
- Gottschalk, I.; Knight, R.; Asch, T.; Abraham, J.; Cannia, J. Using an airborne electromagnetic method to map saltwater intrusion in the northern Salinas Valley, California. Geophysics 2020, 85, B119–B131. [Google Scholar] [CrossRef]
- Walker, P.W.; West, G.F. A robust integral equation solution for electromagnetic scattering by a thin plate in conductive media. Geophysics 1991, 56, 1140–1152. [Google Scholar] [CrossRef]
- Zhdanov, M.S.; Lee, S.K.; Yoshioka, K. Integral equation method for 3D modeling of electromagnetic fields in complex structures with inhomogeneous background conductivity. Geophysics 2006, 71, G333–G345. [Google Scholar] [CrossRef]
- Yee, K. Numerical solution of initial boundary value problems involving Maxwell’s equations in isotropic media. IEEE Trans. Antennas Propag. 1966, 14, 302–307. [Google Scholar] [CrossRef]
- Newman, G.A.; Alumbaugh, D.L. Frequency-domain modelling of airborne electromagnetic responses using staggered finite differences. Geophys. Prospect. 1995, 43, 1021–1042. [Google Scholar] [CrossRef]
- Frank, A.M. Fast finite-difference time-domain modeling for marine-subsurface electromagnetic problem. Geophysics 2007, 72, A19–A23. [Google Scholar] [CrossRef]
- Streich, R. 3D finite-difference frequency-domain modeling of controlled-source electromagnetic data: Direct solution and optimization for high accuracy. Geophysics 2009, 74, F95–F105. [Google Scholar] [CrossRef]
- Haber, E.; Ascher, U.M. Fast finite volume simulation of 3Delectromagnetic problems with highly discontinuous coefficients. SIAM J. Sci. Comput. 2001, 22, 1943–1961. [Google Scholar] [CrossRef]
- Yang, B.; Xu, Y.; He, Z.; Sun, W. 3D frequency-domain modeling of marine controlled source electromagnetic response with topography using finite volume method. Chin. J. Geophys. 2012, 55, 1390–1399. [Google Scholar]
- Jahandari, H.; Farquharson, C.G. A finite-volume solution to the geophysical electromagnetic forward problem using unstructured grids. Geophysics 2014, 79, E287–E302. [Google Scholar] [CrossRef]
- Mitsuhata, Y. 2-D electromagnetic modeling by finite-element method with a dipole source and topography. Geophysics 2000, 65, 465–475. [Google Scholar] [CrossRef]
- Schwarzbach, C.; Haber, E. Finite element-based inversion for time-harmonic electromagnetic problems. Geophys. J. Int. 2013, 193, 615–634. [Google Scholar] [CrossRef]
- Yin, C.; Zhang, B.; Liu, Y.; Cai, J. 2.5-D forward modeling of the time-domain airborne EM system in areas with topographic relief. Chin. J. Geophys. 2015, 58, 1411–1424. [Google Scholar]
- Huang, X.; Yin, C.; Farquharson, C.G.; Cao, X.; Zhang, B.; Huang, W.; Cai, J. Spectral element method with arbitrary hexahedron meshes for time-domain 3D airborne electromagnetic forward modeling. Geophysics 2019, 84, E37–E46. [Google Scholar] [CrossRef]
- Young, D.P.; Melvin, R.G.; Bieterman, M.B.; Johnson, F.T.; Samant, S.S.; Bussoletti, J.E. A locally refined rectangular grid finite element method-Application to computational fluid dynamics and computational physics. J. Comput. Phys. 1991, 92, 1–66. [Google Scholar] [CrossRef]
- Ren, Z.; Kalscheuer, T.; Greenhalgh, S.; Maurer, H. A goal-oriented adaptive finite-element approach for plane wave 3D electromagnetic modeling. Geophys. J. Int. 2013, 187, 63–74. [Google Scholar] [CrossRef]
- Key, K.; Weiss, C. Adaptive finite-element modeling using unstructured grids: The 2D magnetotelluric example. Geophysics 2006, 71, G291–G299. [Google Scholar] [CrossRef]
- Ren, Z.; Tang, J. 3D direct current resistivity modeling with unstructured mesh by adaptive finite-element method. Geophysics 2010, 75, H7–H17. [Google Scholar] [CrossRef]
- Schwarzbach, C.; Borner, R.; Spitzer, K. Three-dimensional adaptive higher order finite element simulation for geo-electromagnetics-Amarine CSEM example. Geophys. J. Int. 2011, 187, 63–74. [Google Scholar] [CrossRef]
- Brenner, S.; Scott, L. The Mathematical Theory of Finite Element Methods; Springer: New York, NY, USA, 2008. [Google Scholar]
- Sasaki, Y.; Nakazato, H. Topographic effects in frequency-domain helicopter-borne electromagnetic. Explor. Geophys. 2003, 34, 24–28. [Google Scholar] [CrossRef]
- Nam, M.J.; Kim, H.J.; Song, Y.; Lee, T.J.; Son, J.S. 3D magneto-telluric modelling including surface topography. Geophys. Prospect. 2007, 55, 277–287. [Google Scholar] [CrossRef]
- Liu, Y.; Yin, C. 3D inversion for frequency-domain HEM data. Chin. J. Geophys. 2013, 56, 4278–4287. [Google Scholar]
- Ward, S.H.; Hohmann, G.W. Electromagnetic Theory for Geophysical. In Applications Electromagnetic Method in Applied Geophysics; Nabighian, M.N., Ed.; Society of Exploration Geophysicists: Houston, TX, USA, 1988; Volume 1, p. 176. [Google Scholar]
- Jin, J. The Finite Element Method in Electromagnetics; John Wiley & Sons: Hoboken, NJ, USA, 2014. [Google Scholar]
- Cai, H.; Xiong, B.; Han, M.; Zhdanov, M. 3D controlled-source electromagnetic modeling in anisotropic medium using edge-based finite element method. Comput. Geosci. 2014, 73, 164–176. [Google Scholar] [CrossRef]
- Legrain, G.; Allais, R.; Cartraud, P. On the use of the extended finite element method with quadtree/octree meshes. Int. J. Numerical. Methods Eng. 2011, 86, 717–743. [Google Scholar] [CrossRef]
- Bollhöfer, M.; Eftekhari, A.; Scheidegger, S.; Schenk, O. Large-Scale Sparse Inverse Covariance Matrix Estimation. SIAM J. Sci. Comput. 2019, 41, A380–A401. [Google Scholar] [CrossRef]
- Oden, J.T.; Prudhomme, S. Goal-oriented error estimation and adaptive for the finite element method. Comput. Math. Appl. 2001, 41, 735–756. [Google Scholar] [CrossRef]
- Zdunek, A.; Rachowicz, W. A goal-oriented hp-adaptive finite element approach to radar scattering problems. Comput. Methods Appl. Mech. Eng. 2005, 194, 657–674. [Google Scholar] [CrossRef]
- Kobayashi, T.; Oya, H.; Ono, T. A-scope analysis of subsurface radar sounding of lunar mare region. Earth Planets Space 2002, 54, 973–982. [Google Scholar] [CrossRef]



| Method | Frequency (Hz) | Number of Unknowns | Runtime (s) | Memory (MB) |
|---|---|---|---|---|
| SE method | 1600 | 521,352 | 31.56 | 14,806 |
| 25,000 | 31.38 | 14,818 | ||
| FE method with adaptive octree mesh | 1600 | 255,325 | 13.21 | 4857 |
| 25,000 | 13.10 | 4859 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Han, X.; Ni, J.; Yin, C.; Zhang, B.; Huang, X.; Zhu, J.; Liu, Y.; Ren, X.; Su, Y. 3D Airborne EM Forward Modeling Based on Finite-Element Method with Goal-Oriented Adaptive Octree Mesh. Remote Sens. 2023, 15, 2816. https://doi.org/10.3390/rs15112816
Han X, Ni J, Yin C, Zhang B, Huang X, Zhu J, Liu Y, Ren X, Su Y. 3D Airborne EM Forward Modeling Based on Finite-Element Method with Goal-Oriented Adaptive Octree Mesh. Remote Sensing. 2023; 15(11):2816. https://doi.org/10.3390/rs15112816
Chicago/Turabian StyleHan, Xue, Jianfu Ni, Changchun Yin, Bo Zhang, Xin Huang, Jiao Zhu, Yunhe Liu, Xiuyan Ren, and Yang Su. 2023. "3D Airborne EM Forward Modeling Based on Finite-Element Method with Goal-Oriented Adaptive Octree Mesh" Remote Sensing 15, no. 11: 2816. https://doi.org/10.3390/rs15112816
APA StyleHan, X., Ni, J., Yin, C., Zhang, B., Huang, X., Zhu, J., Liu, Y., Ren, X., & Su, Y. (2023). 3D Airborne EM Forward Modeling Based on Finite-Element Method with Goal-Oriented Adaptive Octree Mesh. Remote Sensing, 15(11), 2816. https://doi.org/10.3390/rs15112816







