Inverse Scattering Series Internal Multiple Attenuation in the Common-Midpoint Domain
Abstract
1. Introduction
2. Plane-Wave Domain Inverse Scattering Series Internal Multiple Prediction Algorithm (2D): Review
3. Theory
3.1. 1.5D Plane-Wave Domain Prediction Algorithm
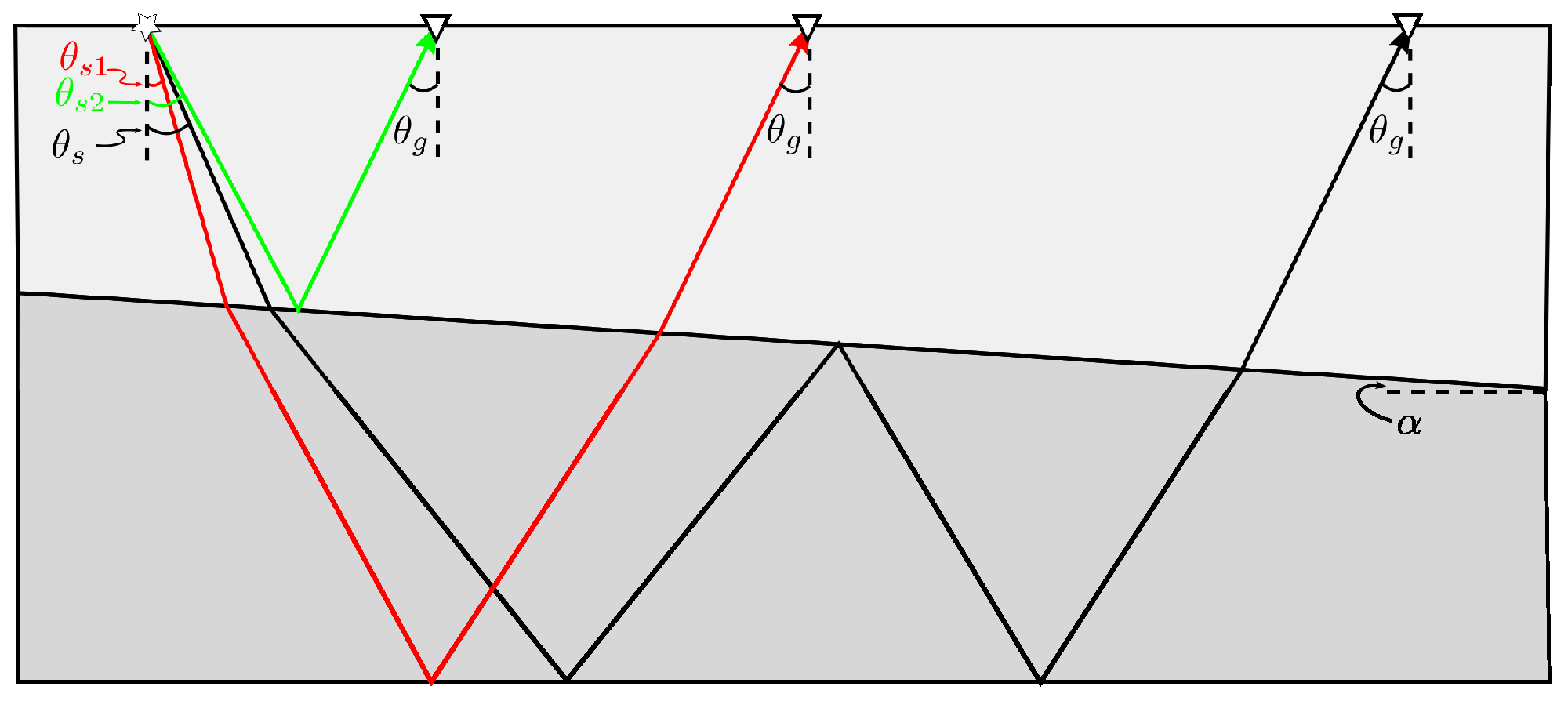
3.2. 1.5D Prediction Scheme with the Common Shot Gathers
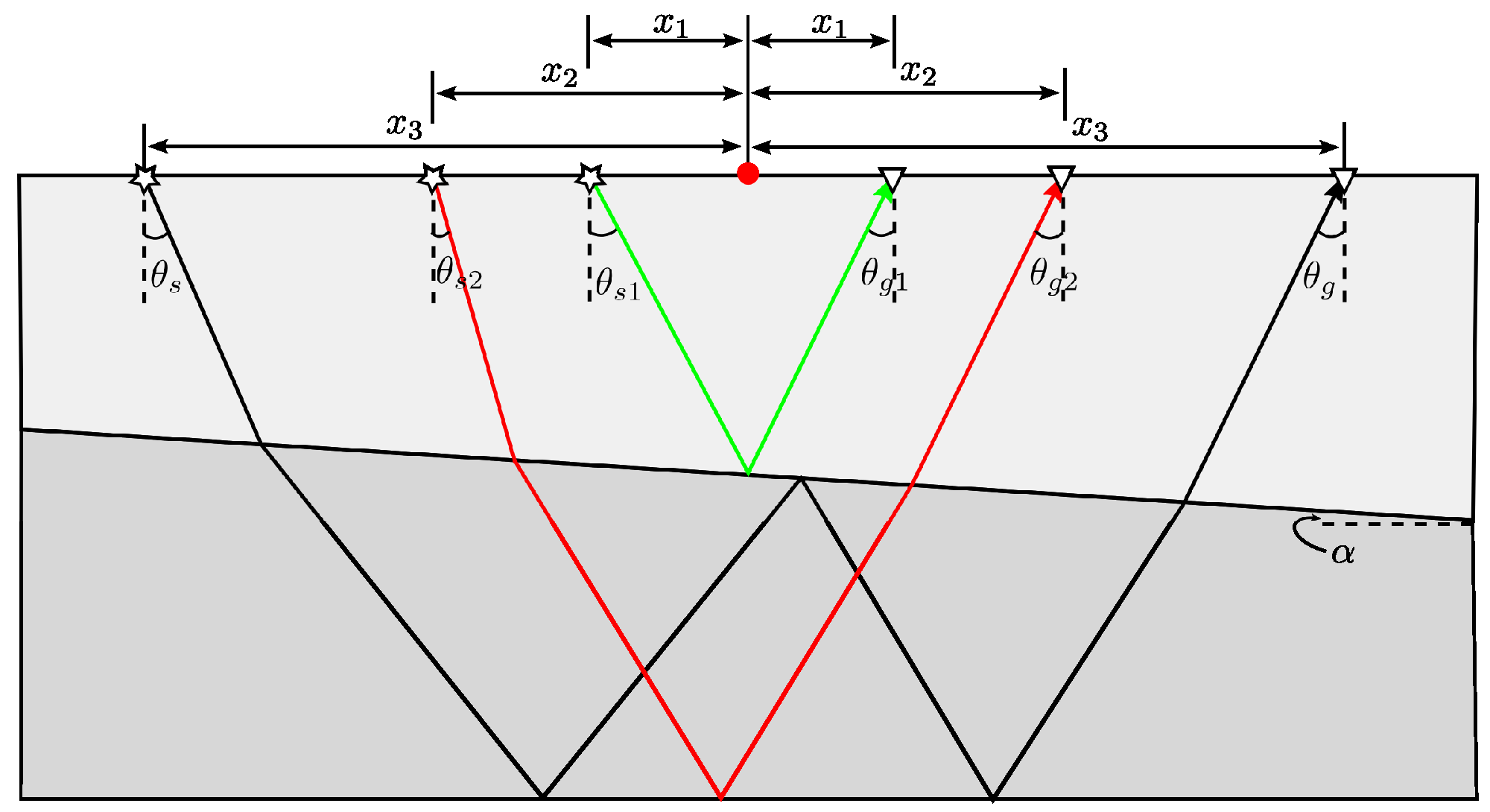
3.3. 1.5D Prediction Scheme with the CMP Gathers
3.4. Two-Dimensional (2D) Plane-Wave Domain Prediction Algorithm in the CMP Domain
4. Numerical Analysis of the 1.5D Prediction Algorithm on the 2D Cases
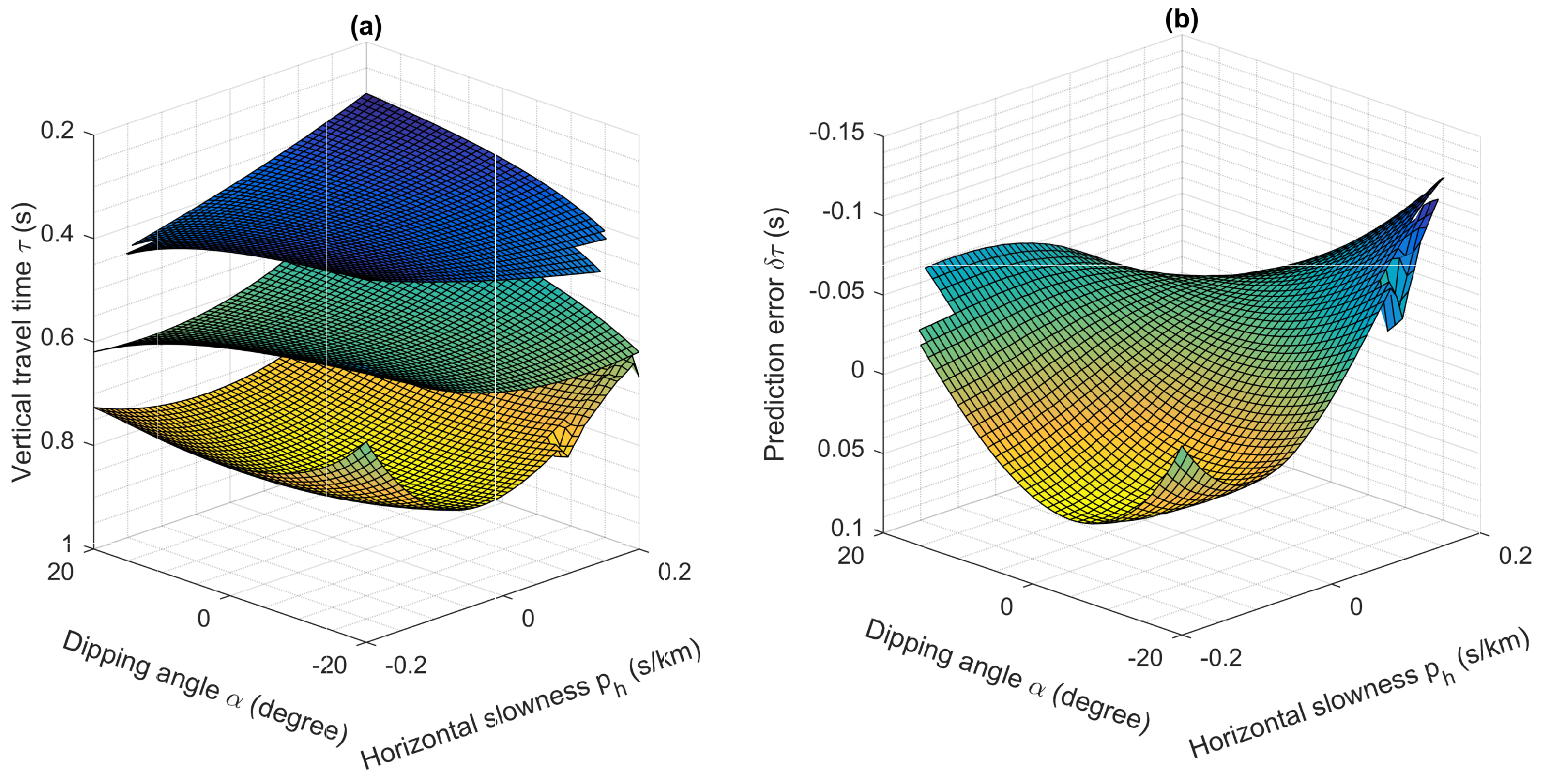
4.1. Error Analysis with the Shot Gathers
4.2. Error Analysis with the CMP Gathers
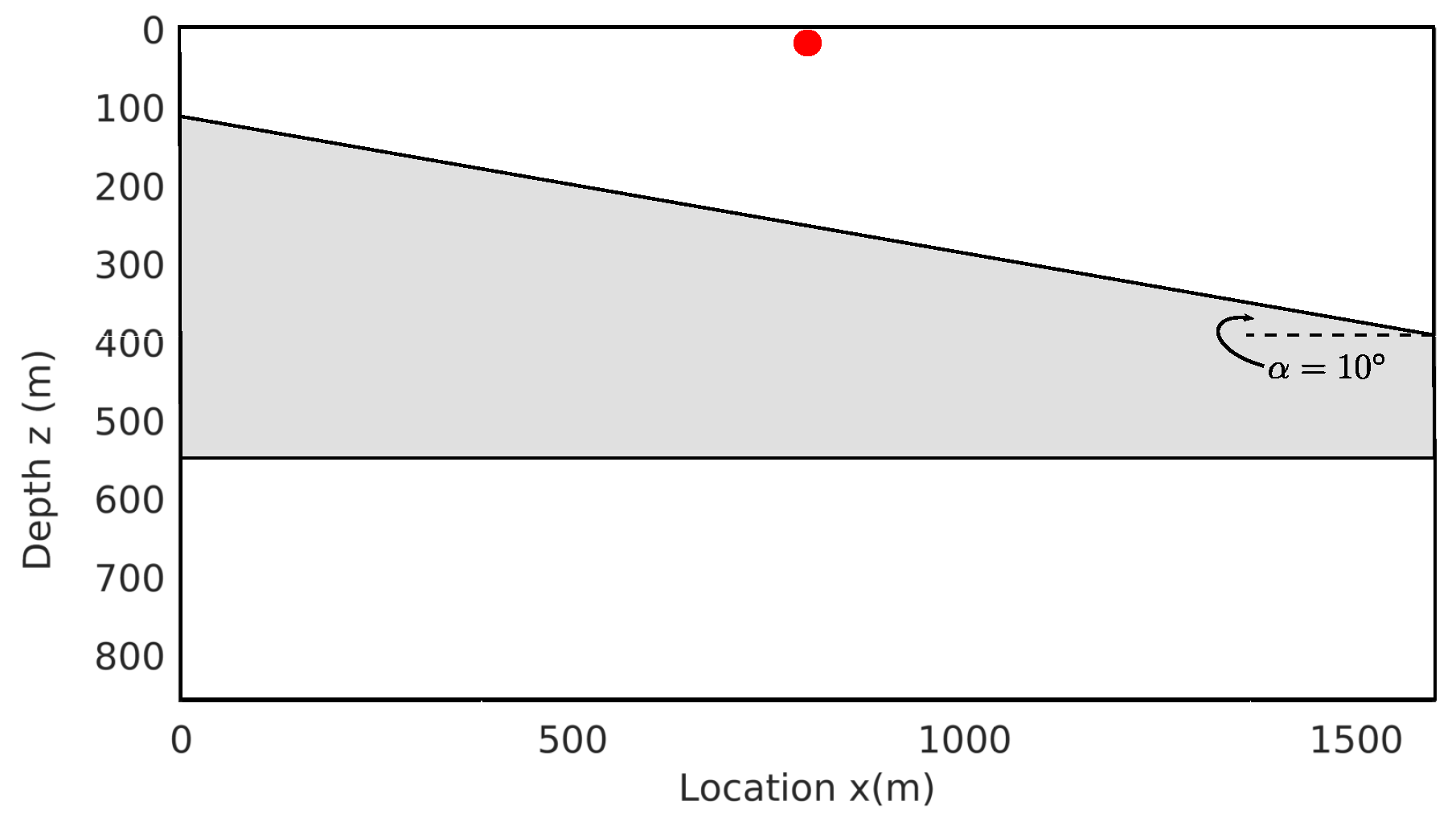
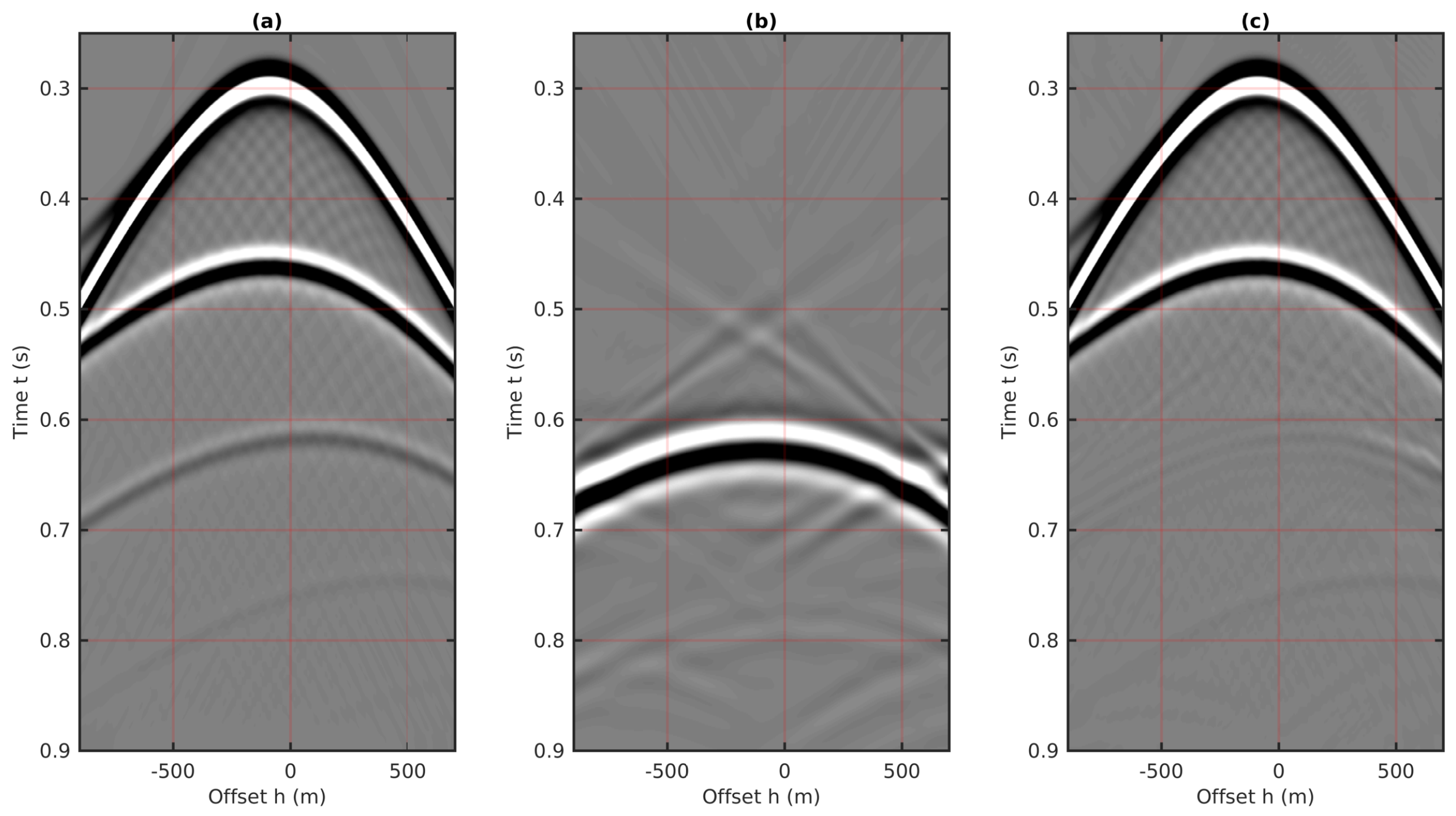
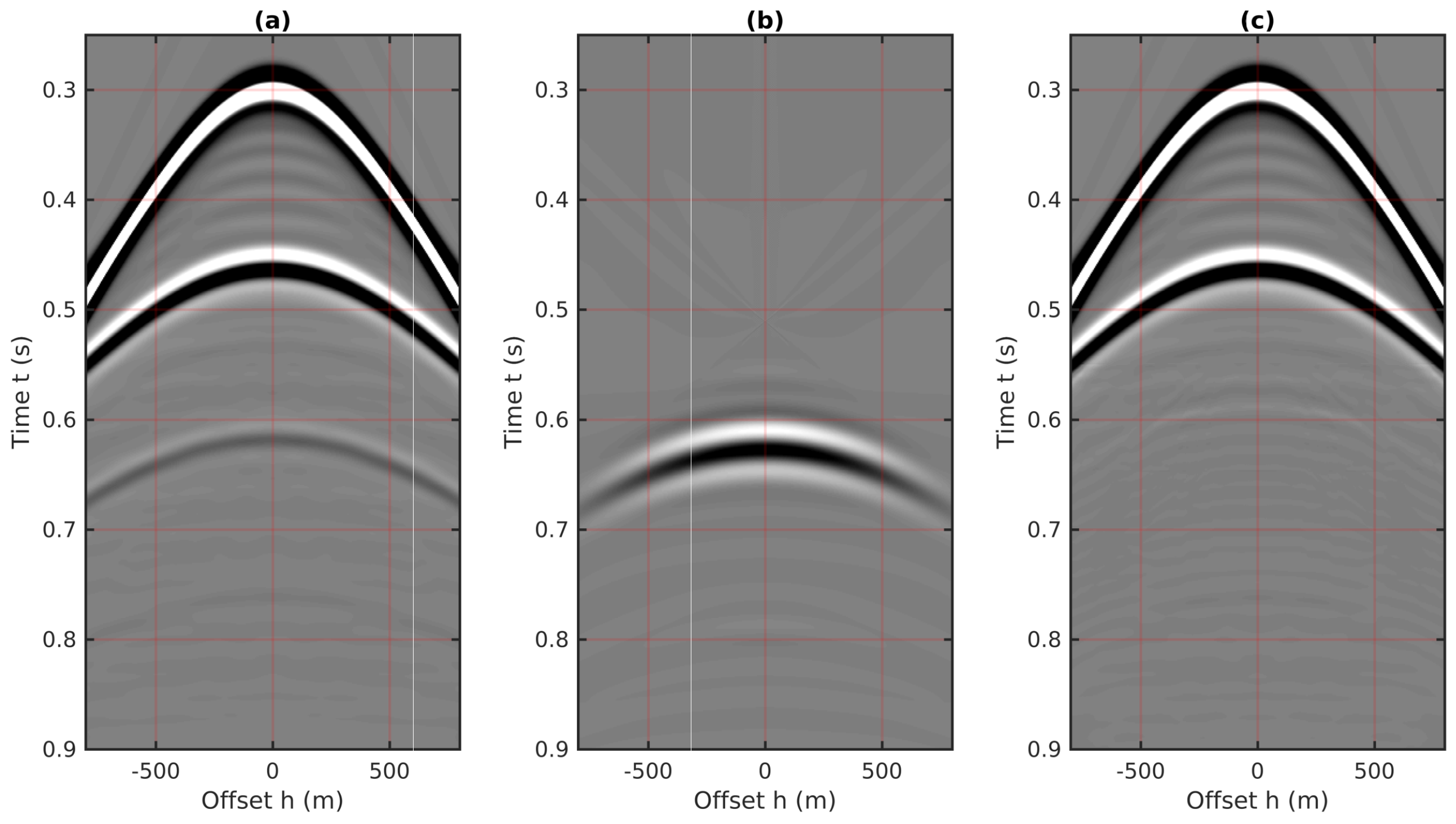
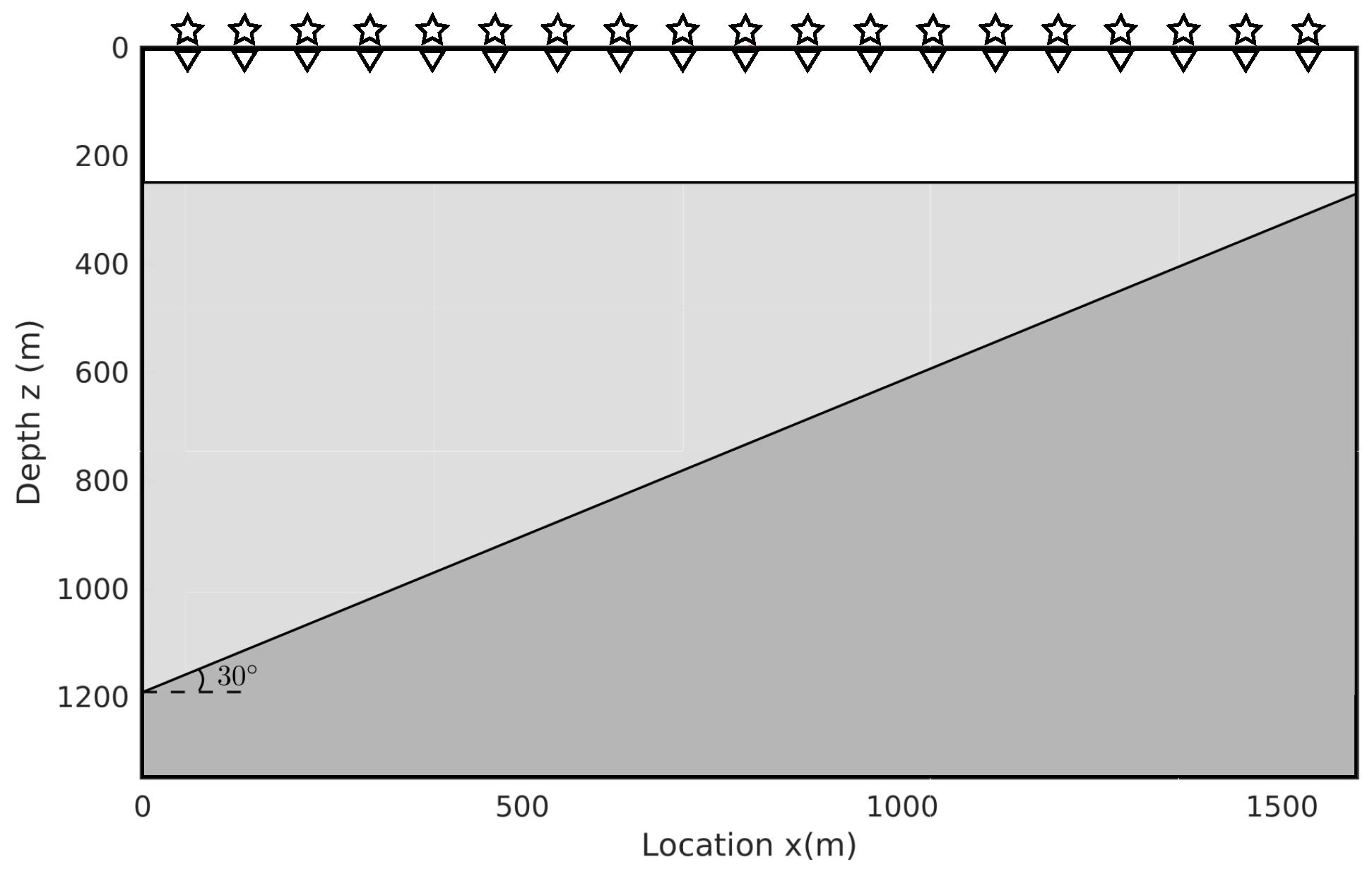
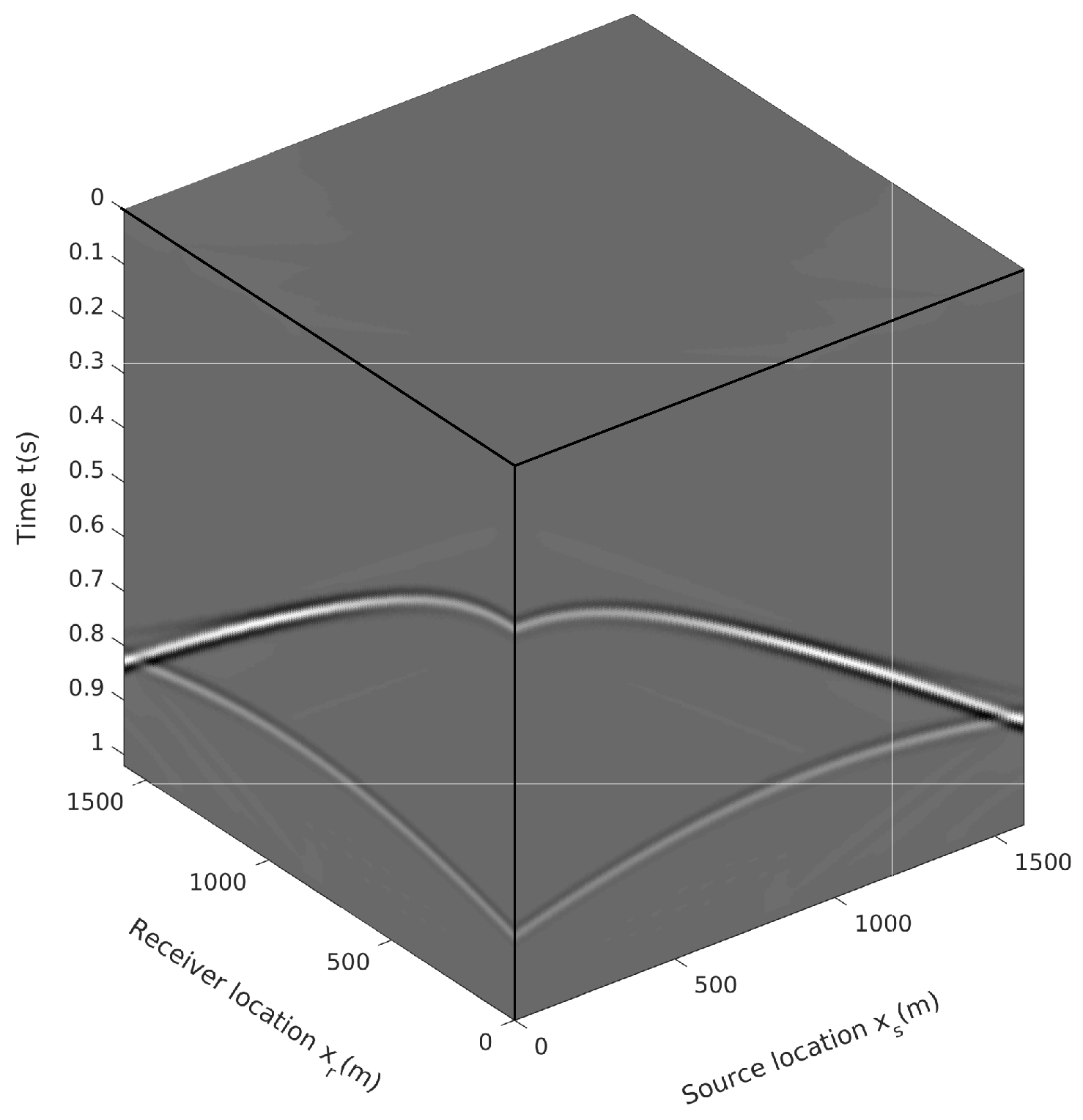
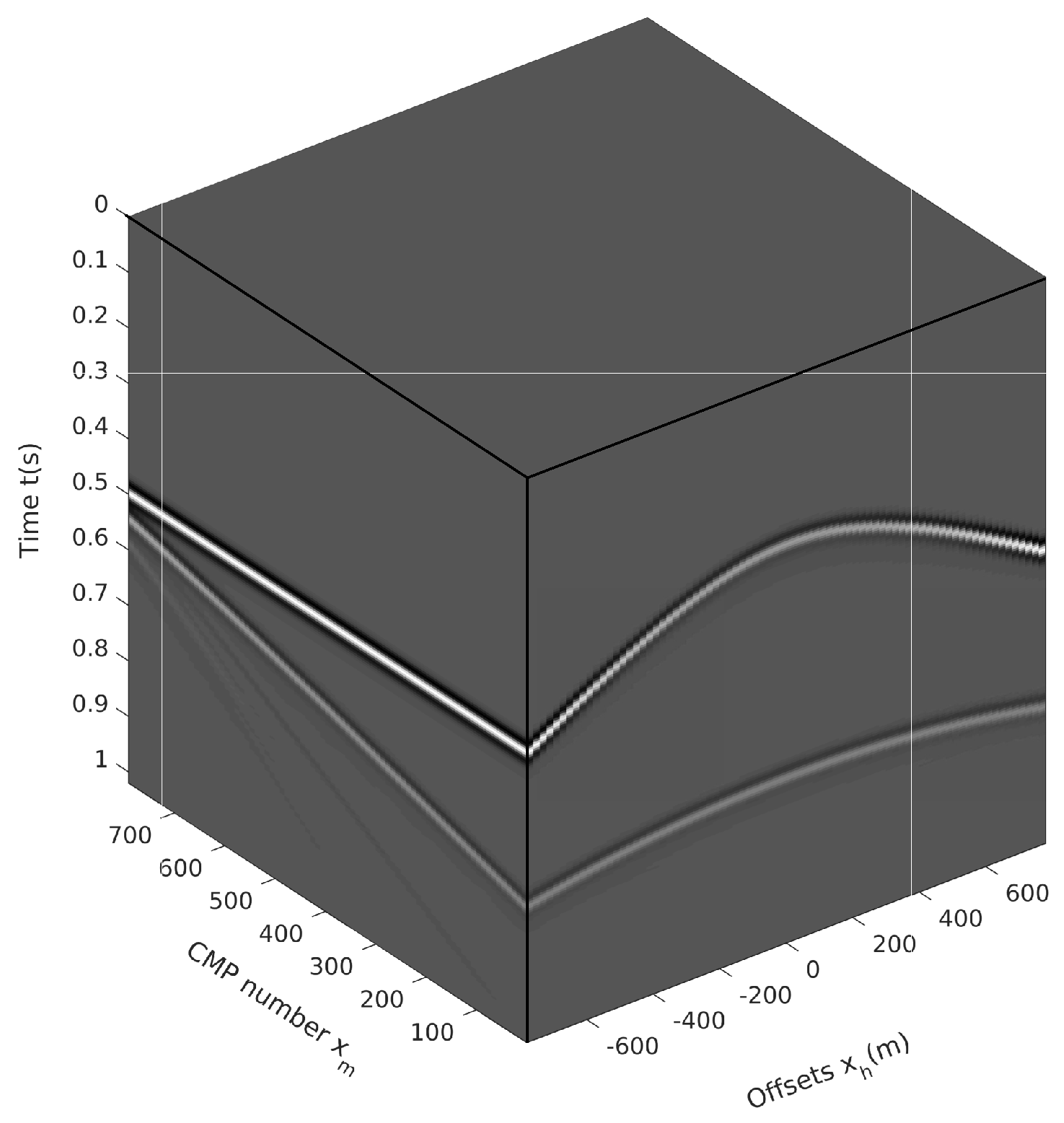
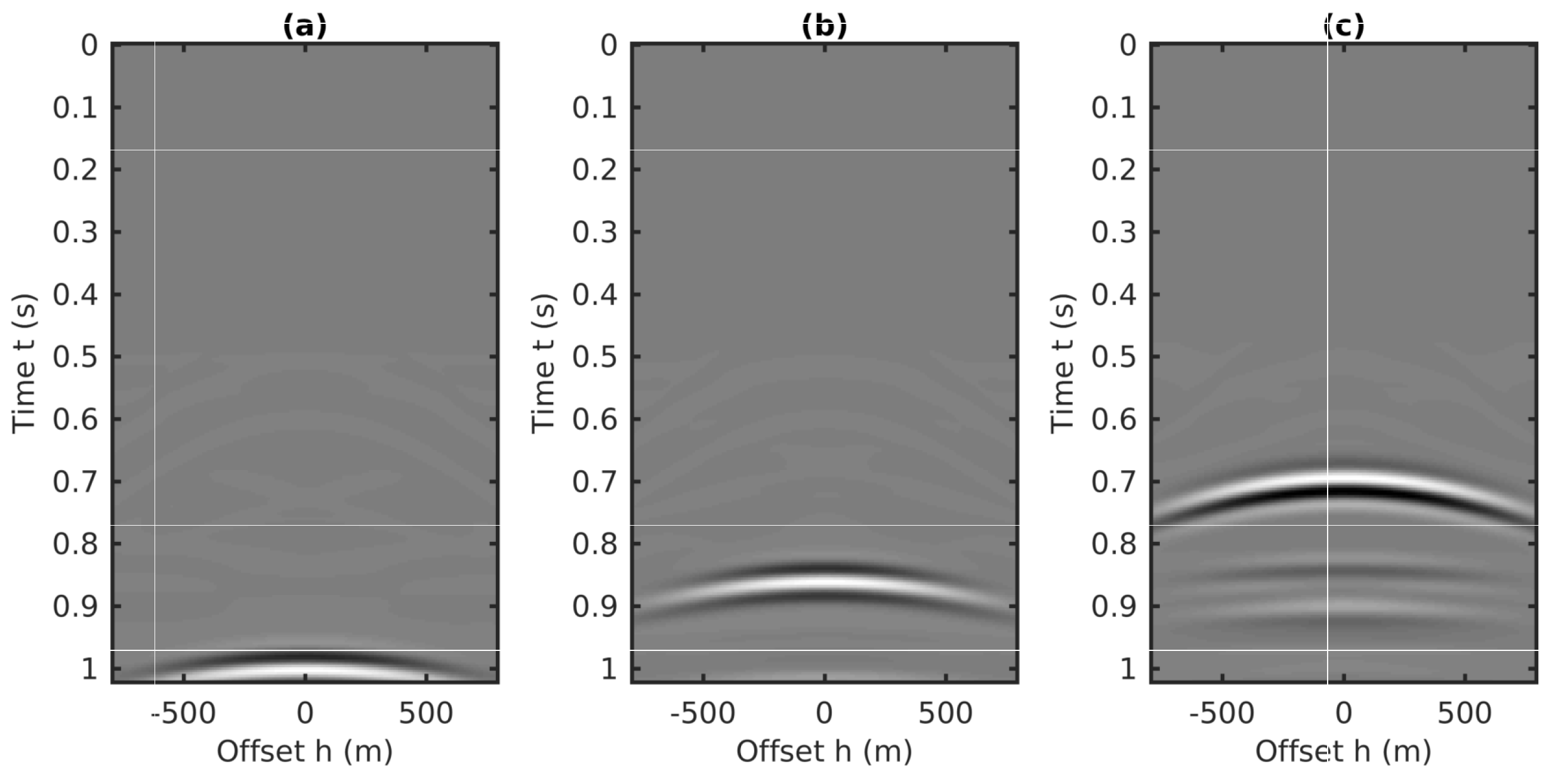
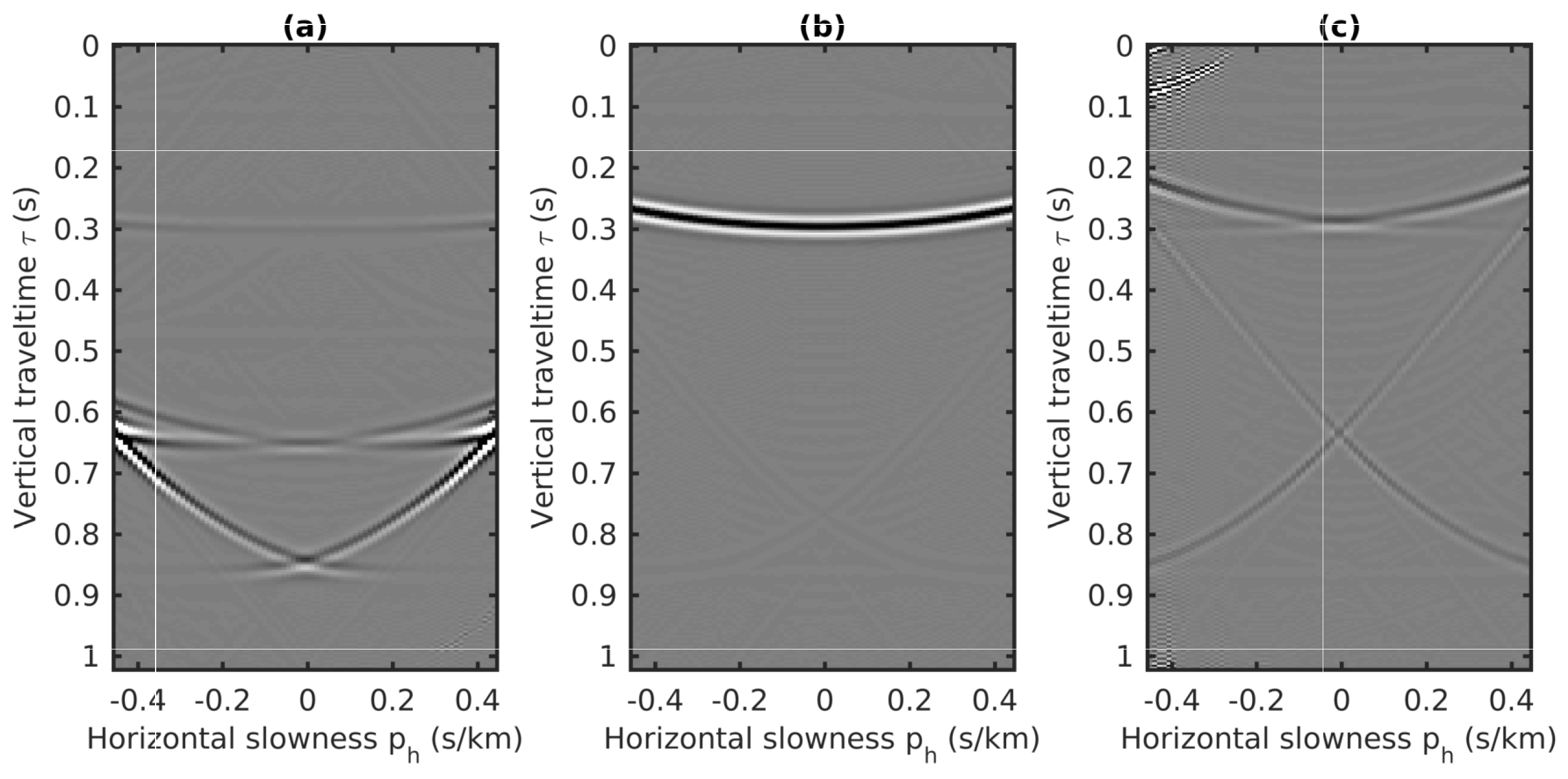
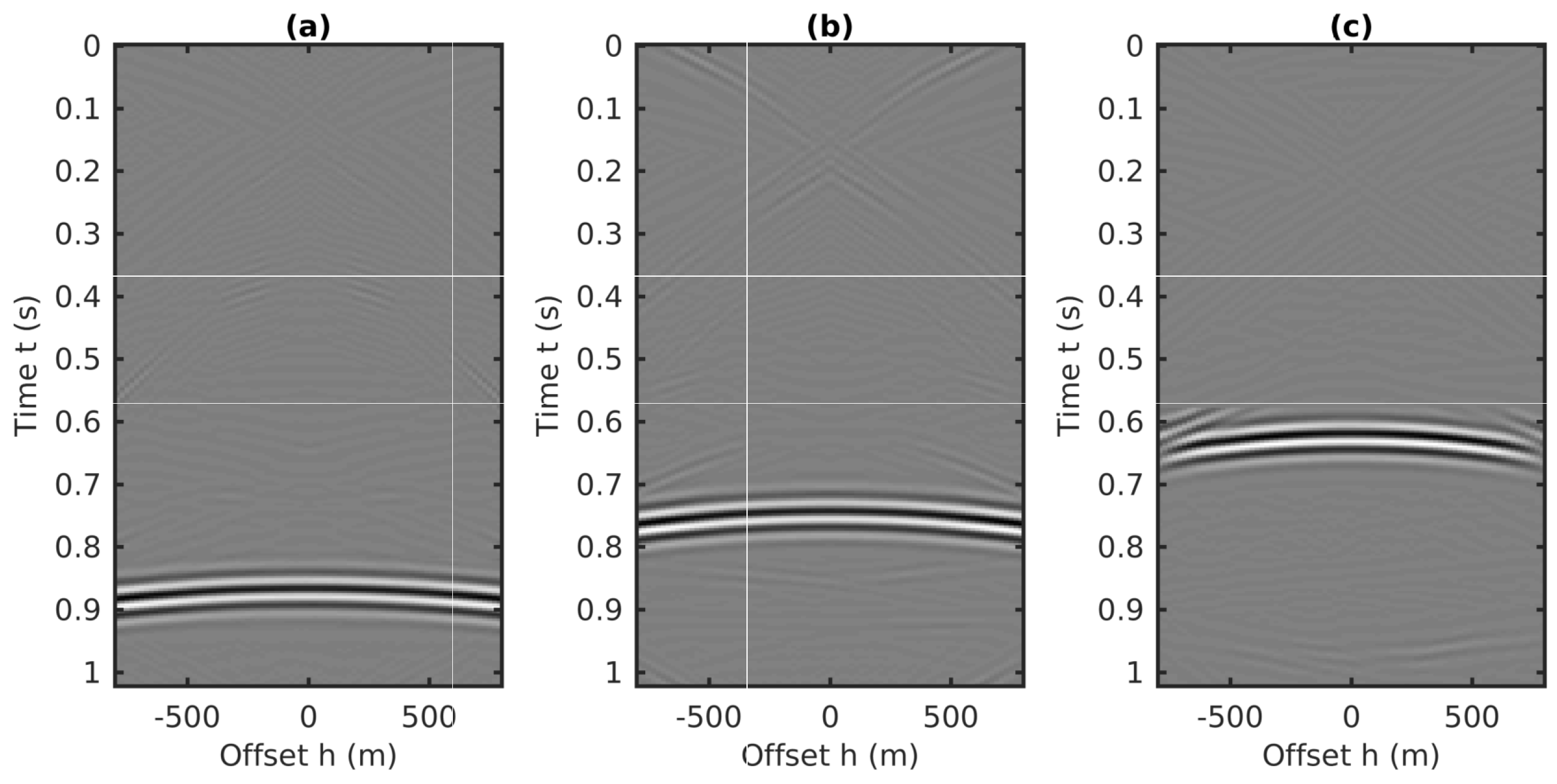
5. Numerical Examples
5.1. Two-Dimensional (2D) Prediction Using the 1.5D Algorithm
5.2. Two-Dimensional (2D) Prediction Using the 2D Algorithm
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Alaei, N.; Roshandel Kahoo, A.; Kamkar Rouhani, A.; Soleimani, M. Seismic resolution enhancement using scale transform in the time-frequency domain. Geophysics 2018, 83, V305–V314. [Google Scholar] [CrossRef]
- Alaei, N.; Soleimani Monfared, M.; Roshandel Kahoo, A.; Bohlen, T. Seismic imaging of complex velocity structures by 2D pseudo-viscoelastic time-domain full-waveform inversion. Appl. Sci. 2022, 12, 7741. [Google Scholar] [CrossRef]
- Foster, D.J.; Mosher, C.C. Suppression of multiple reflections using the Radon transform. Geophysics 1992, 57, 386–395. [Google Scholar] [CrossRef]
- VerWest, B. Suppressing Peg-leg Multiples with Parabolic Radon Demultiple. In Proceedings of the 64th EAGE Conference & Exhibition, Florence, Italy, 27–30 May 2002. [Google Scholar]
- Trad, D. Interpolation and multiple attenuation with migration operators. Geophysics 2003, 68, 2043–2054. [Google Scholar] [CrossRef]
- Hampson, D. Inverse velocity stacking for multiple elimination. In SEG Technical Program Expanded Abstracts 1986; Society of Exploration Geophysicists: Houston, TX, USA, 1986; pp. 422–424. [Google Scholar]
- Lumley, D.E.; Nichols, D.; Rekdal, T. Amplitude-preserved multiple suppression. In Proceedings of the 1995 SEG Annual Meeting. Society of Exploration Geophysicists, Houston, TX, USA, 8–13 October 1995. [Google Scholar]
- Yilmaz, Ö. Seismic Data Analysis; Society of Exploration Geophysicists Tulsa: Houston, TX, USA, 2001; Volume 1. [Google Scholar]
- Zhang, N.; Wang, Y. An Inverse Data Space Method for Interbed Multiple Attenuation in the CMP Domain. In Proceedings of the 73rd EAGE Conference and Exhibition Incorporating SPE EUROPEC 2011, Vienna, Austria, 23–27 May 2011. [Google Scholar]
- Berkhout, A. Seismic processing in the inverse data space. Geophysics 2006, 71, A29–A33. [Google Scholar] [CrossRef]
- Staring, M.; Wapenaar, K. Three-dimensional Marchenko internal multiple attenuation on narrow azimuth streamer data of the Santos basin, Brazil. Geophys. Prospect. 2020, 68, 1864–1877. [Google Scholar] [CrossRef] [PubMed]
- Zhang, L.; Slob, E. A field data example of Marchenko multiple elimination. Geophysics 2020, 85, S65–S70. [Google Scholar] [CrossRef]
- Santos, R.S.; Revelo, D.E.; Pestana, R.C.; Koehne, V.; Barrera, D.F.; Souza, M.S.; Silva, A. An application of the Marchenko internal multiple elimination scheme formulated as a least-squares problem. Geophysics 2021, 86, WC105–WC116. [Google Scholar] [CrossRef]
- Wapenaar, K.; Brackenhoff, J.; Dukalski, M.; Meles, G.; Reinicke, C.; Slob, E.; Staring, M.; Thorbecke, J.; van der Neut, J.; Zhang, L. Marchenko redatuming, imaging, and multiple elimination and their mutual relations. Geophysics 2021, 86, WC117–WC140. [Google Scholar] [CrossRef]
- Thorbecke, J.; Zhang, L.; Wapenaar, K.; Slob, E. Implementation of the Marchenko multiple elimination algorithm. Geophysics 2021, 86, F9–F23. [Google Scholar] [CrossRef]
- Dukalski, M.; de Vos, K. Overburden-borne internal demultiple formula. Geophysics 2022, 87, V227–V246. [Google Scholar] [CrossRef]
- Ma, C.; Guo, M.; Liu, Z.; Sheng, J. Analysis and application of data-driven approaches for internal-multiple elimination. In SEG Technical Program Expanded Abstracts 2020; Society of Exploration Geophysicists: Houston, TX, USA, 2020; pp. 3124–3128. [Google Scholar]
- Araújo, F.V.; Weglein, A.B.; Carvalho, P.M.; Stolt, R.H. Inverse scattering series for multiple attenuation: An example with surface and internal multiples. In Proceedings of the 64th Annual International Meeting SEG Expanded Abstracts, Los Angeles, CA, USA, 23–27 October 1994. [Google Scholar]
- Weglein, A.B.; Gasparotto, F.A.; Carvalho, P.M.; Stolt, R.H. An inverse-scattering series method for attenuating multiples in seismic reflection data. Geophysics 1997, 62, 1975–1989. [Google Scholar] [CrossRef]
- Luo, Y.; Kelamis, P.G.; Fu, Q.; Huo, S.; Sindi, G.; Hsu, S.Y.; Weglein, A.B. Elimination of land internal multiples based on the inverse scattering series. Lead. Edge 2011, 30, 884–889. [Google Scholar] [CrossRef]
- Herrera, W.; Weglein, A.B. Eliminating first-order internal multiples with downward reflection at the shallowest interface: Theory and initial examples. In SEG Technical Program Expanded Abstracts 2013; Society of Exploration Geophysicists: Houston, TX, USA, 2013; pp. 4131–4135. [Google Scholar]
- Zou, Y.; Weglein, A.B. A new method to eliminate first order internal multiples for a normal incidence plane wave on a 1D earth. In Proceedings of the 2013 SEG Annual Meeting, Houston, TX, USA, 22–27 September 2013. [Google Scholar]
- Ramirez, A.; Weglein, A. Progressing the analysis of the phase and amplitude prediction properties of the inverse scattering internal multiple attenuation algorithm. J. Seism. Explor. 2005, 13, 283–301. [Google Scholar]
- Yang, J.; Weglein, A.B. Accommodating the source wavelet and radiation pattern in the internal multiple attenuation algorithm: Theory and initial example that demonstrates impact. In SEG Technical Program Expanded Abstracts 2015; Society of Exploration Geophysicists: Houston, TX, USA, 2015; pp. 4396–4401. [Google Scholar]
- Innanen, K.A. Time-and offset-domain internal multiple prediction with nonstationary parameters. Geophysics 2017, 82, V105–V116. [Google Scholar] [CrossRef]
- Sun, J.; Innanen, K.A. Multidimensional inverse-scattering series internal multiple prediction in the coupled plane-wave domain. Geophysics 2018, 83, V73–V82. [Google Scholar] [CrossRef]
- Sun, J.; Innanen, K.A. A plane-wave formulation and numerical analysis of elastic multicomponent inverse scattering series internal multiple prediction. Geophysics 2019, 84, V255–V269. [Google Scholar] [CrossRef]
- Sun, J. Computational and Practical Developments in Single-and Multi-Component Inverse Scattering Series Internal Multiple Prediction. Ph.D. Thesis, University of Calgary, Calgary, AB, Canada, 2018. [Google Scholar]
- Coates, R.; Weglein, A. Internal multiple attenuation using inverse scattering: Results from prestack 1 & 2D acoustic and elastic synthetics. In SEG Technical Program Expanded Abstracts 1996; Society of Exploration Geophysicists: Houston, TX, USA, 1996. [Google Scholar]
- Ocola, L.C. A nonlinear least-squares method for seismic refraction mapping-Part II: Model studies and performance of Reframap method. Geophysics 1972, 37, 273–287. [Google Scholar] [CrossRef]
- Diebold, J.B.; Stoffa, P.L. The traveltime equation, tau-p mapping, and inversion of common midpoint data. Geophysics 1981, 46, 238–254. [Google Scholar] [CrossRef]






Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sun, J.; Innanen, K.A.; Niu, Z.; Eaid, M.V. Inverse Scattering Series Internal Multiple Attenuation in the Common-Midpoint Domain. Remote Sens. 2023, 15, 3002. https://doi.org/10.3390/rs15123002
Sun J, Innanen KA, Niu Z, Eaid MV. Inverse Scattering Series Internal Multiple Attenuation in the Common-Midpoint Domain. Remote Sensing. 2023; 15(12):3002. https://doi.org/10.3390/rs15123002
Chicago/Turabian StyleSun, Jian, Kristopher A. Innanen, Zhan Niu, and Matthew V. Eaid. 2023. "Inverse Scattering Series Internal Multiple Attenuation in the Common-Midpoint Domain" Remote Sensing 15, no. 12: 3002. https://doi.org/10.3390/rs15123002
APA StyleSun, J., Innanen, K. A., Niu, Z., & Eaid, M. V. (2023). Inverse Scattering Series Internal Multiple Attenuation in the Common-Midpoint Domain. Remote Sensing, 15(12), 3002. https://doi.org/10.3390/rs15123002









