Analyses of DSD Vertical Evolution and Rain Variation Mechanism in Stratiform Cloud Cases Using Micro Rain Radar
Abstract
:1. Introduction
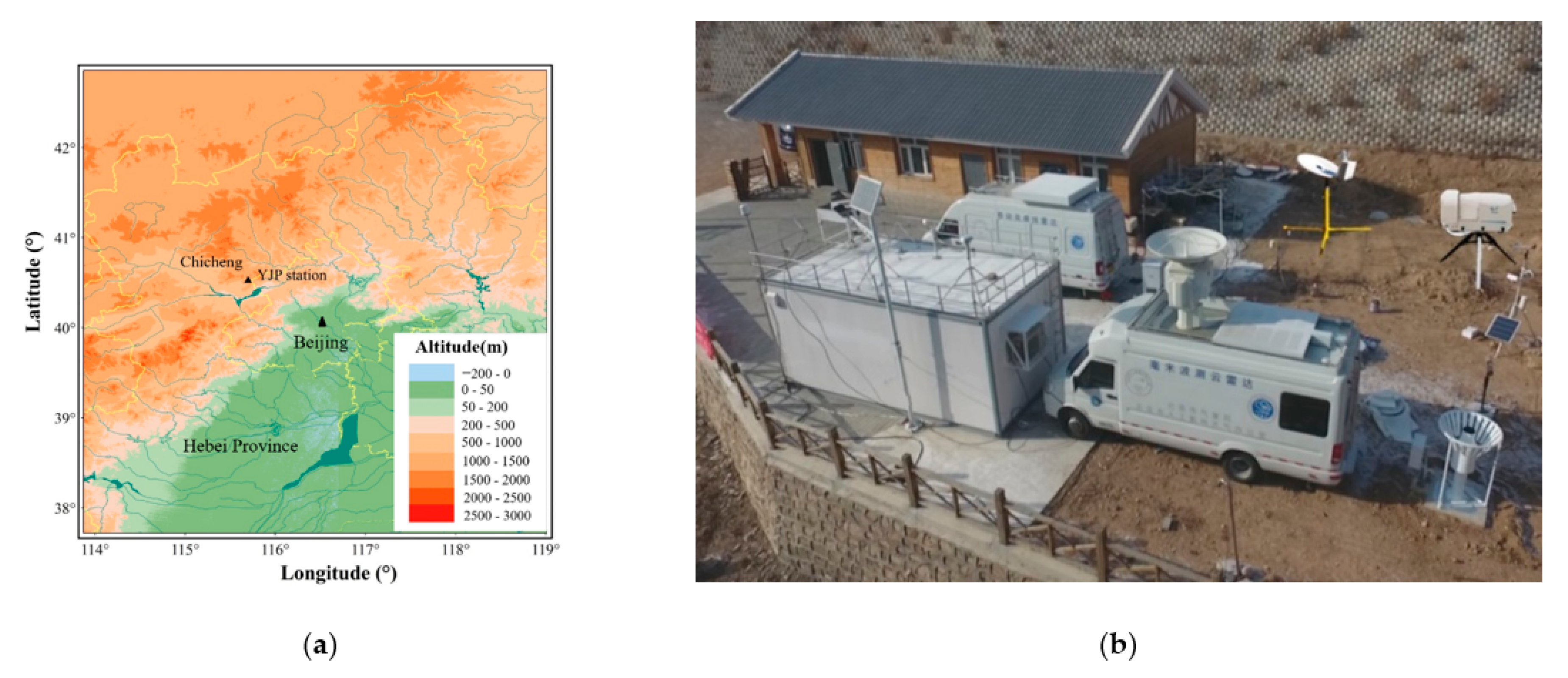
2. Instrumentation and Methods
2.1. Instrumentation
2.2. DSD Retrieval by MRR
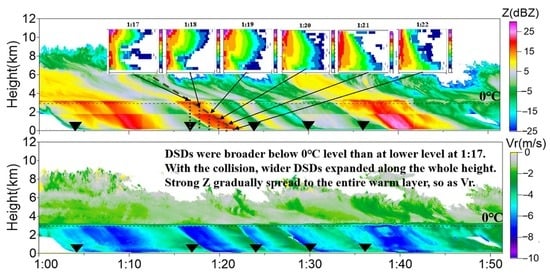
3. Vertical Evolution of DSD in Stratiform Precipitation
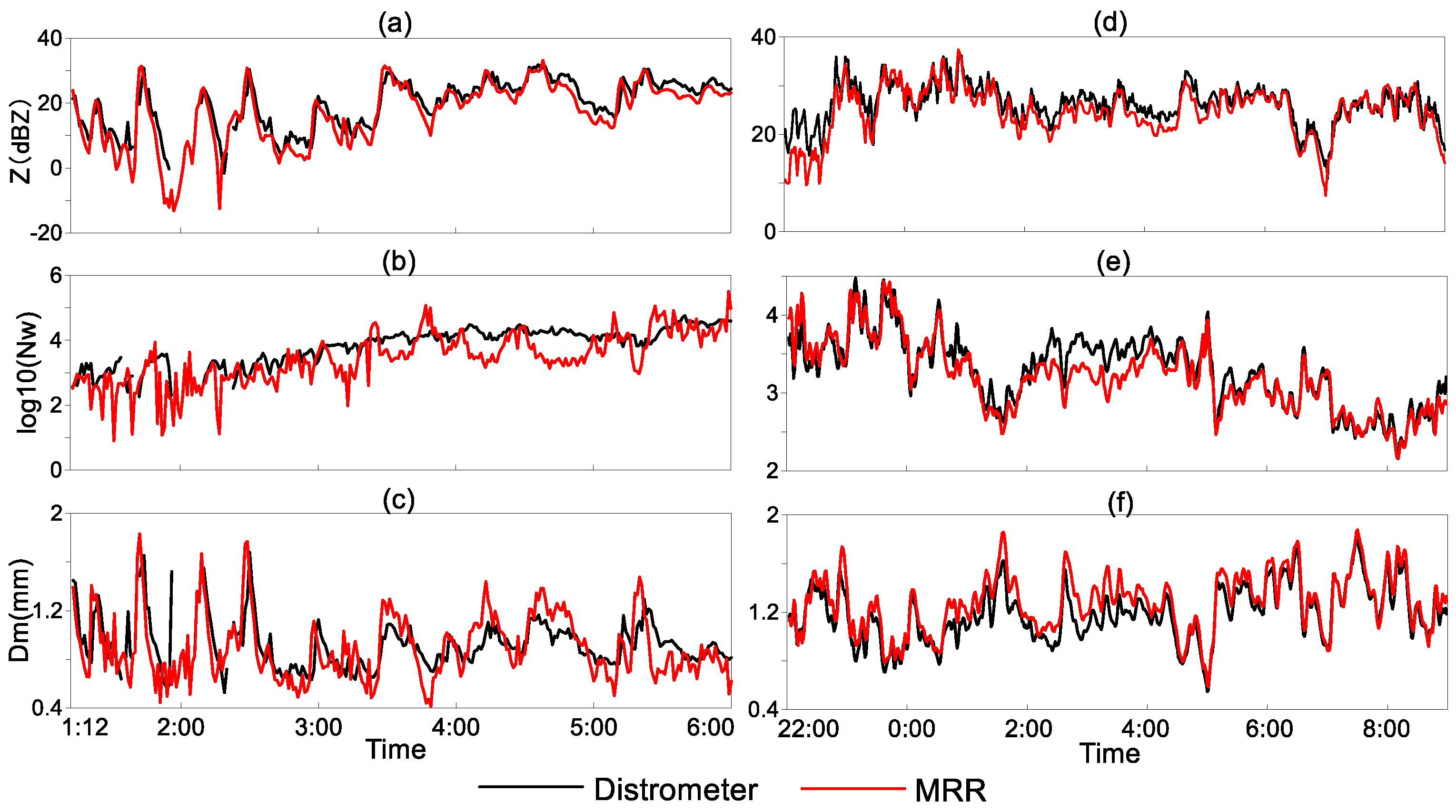
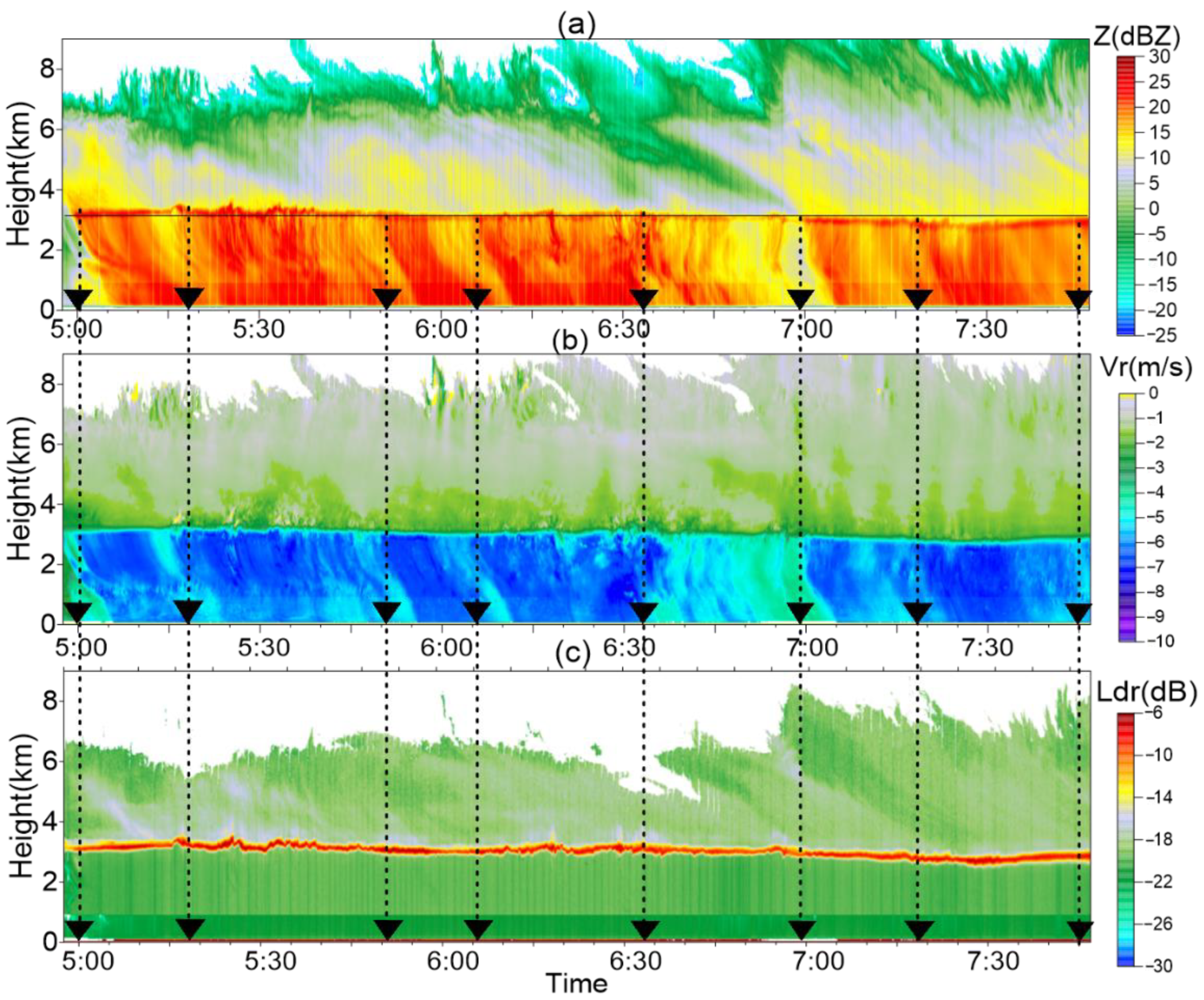
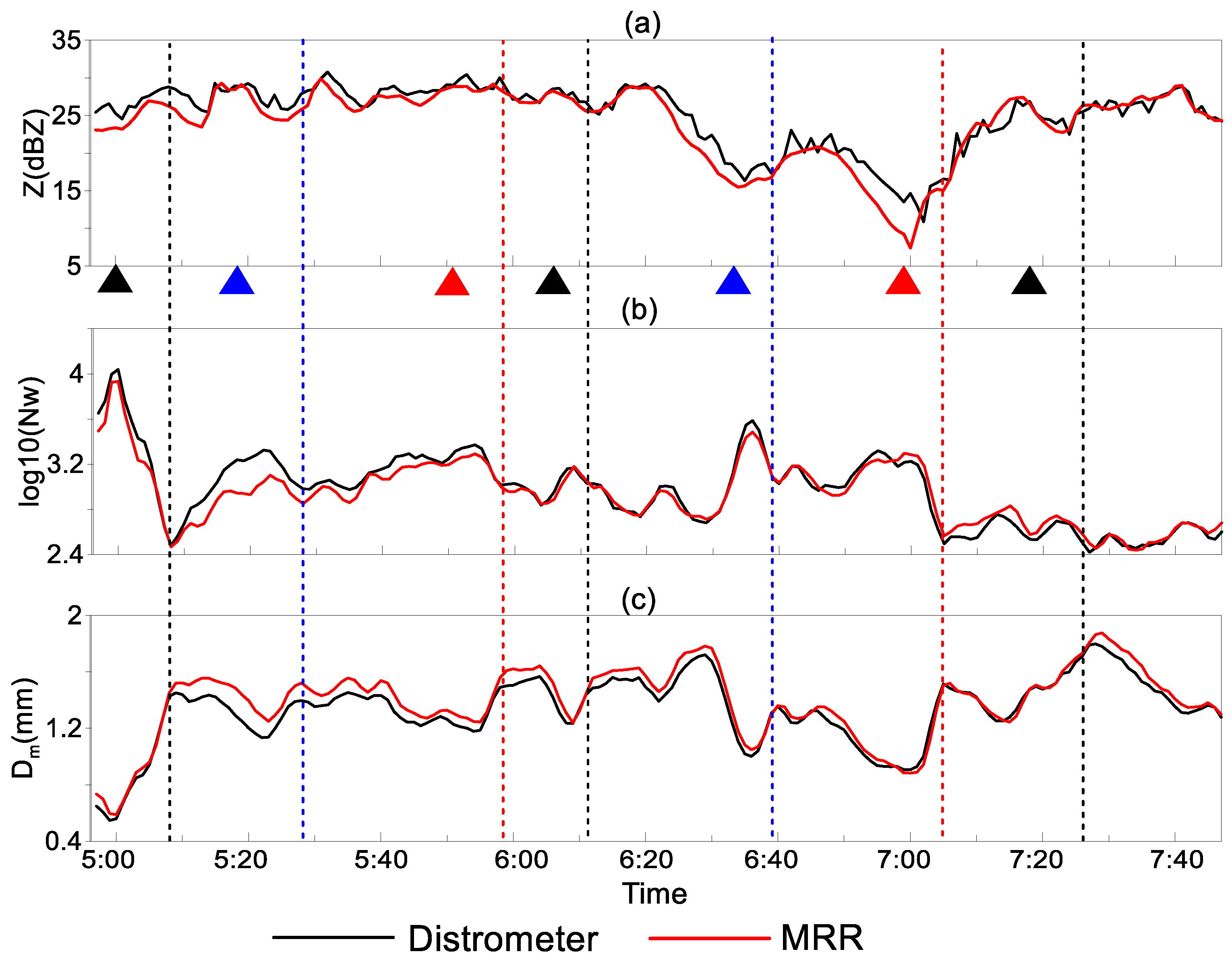
3.1. Reliability of MRR Data
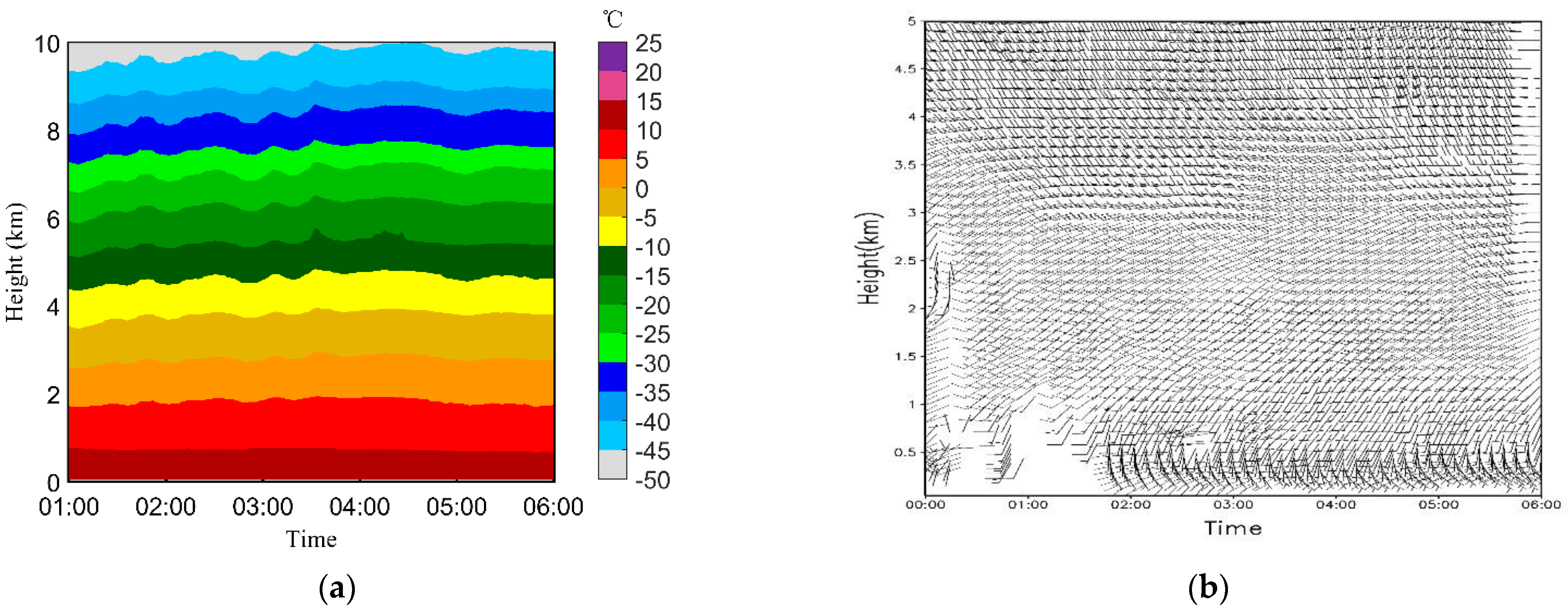
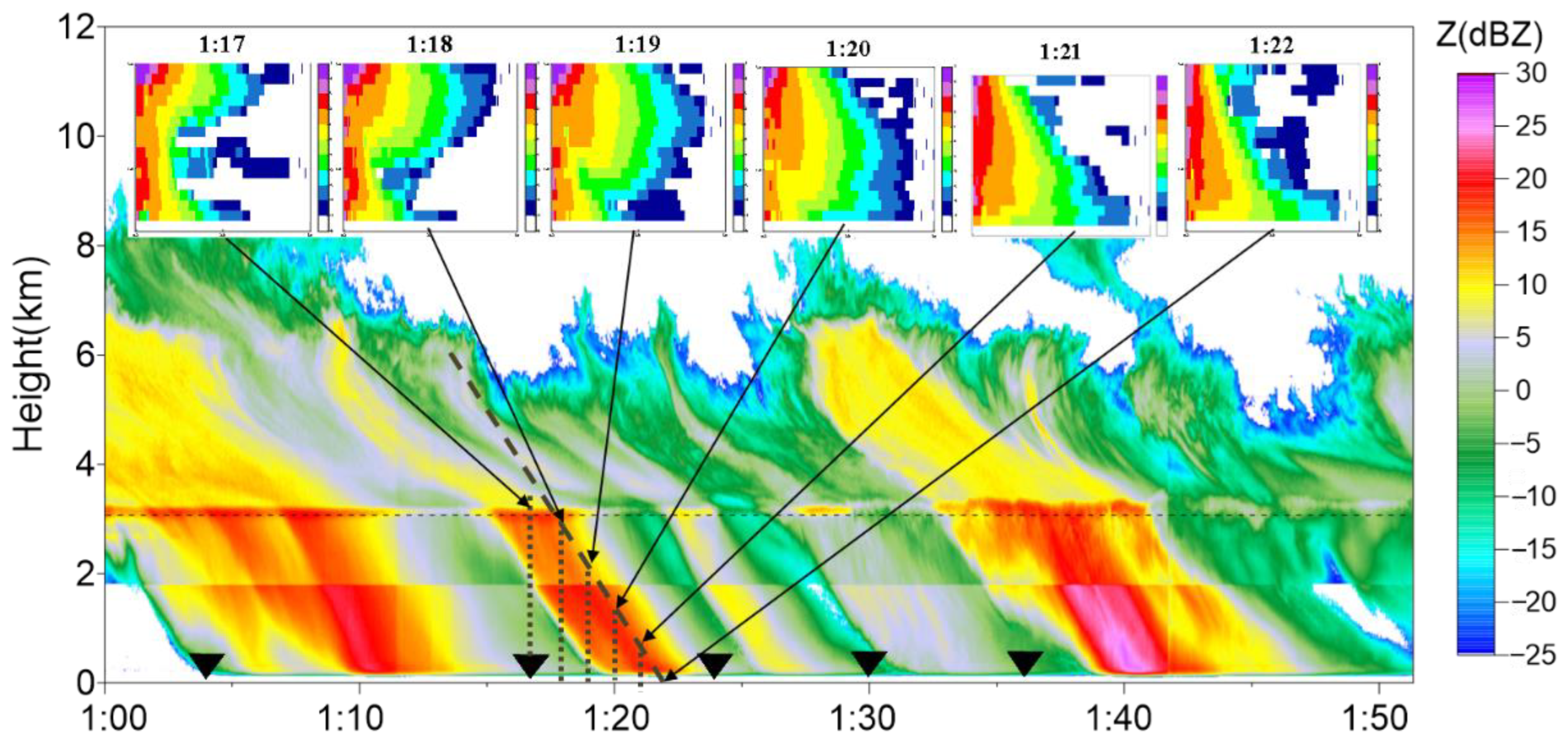
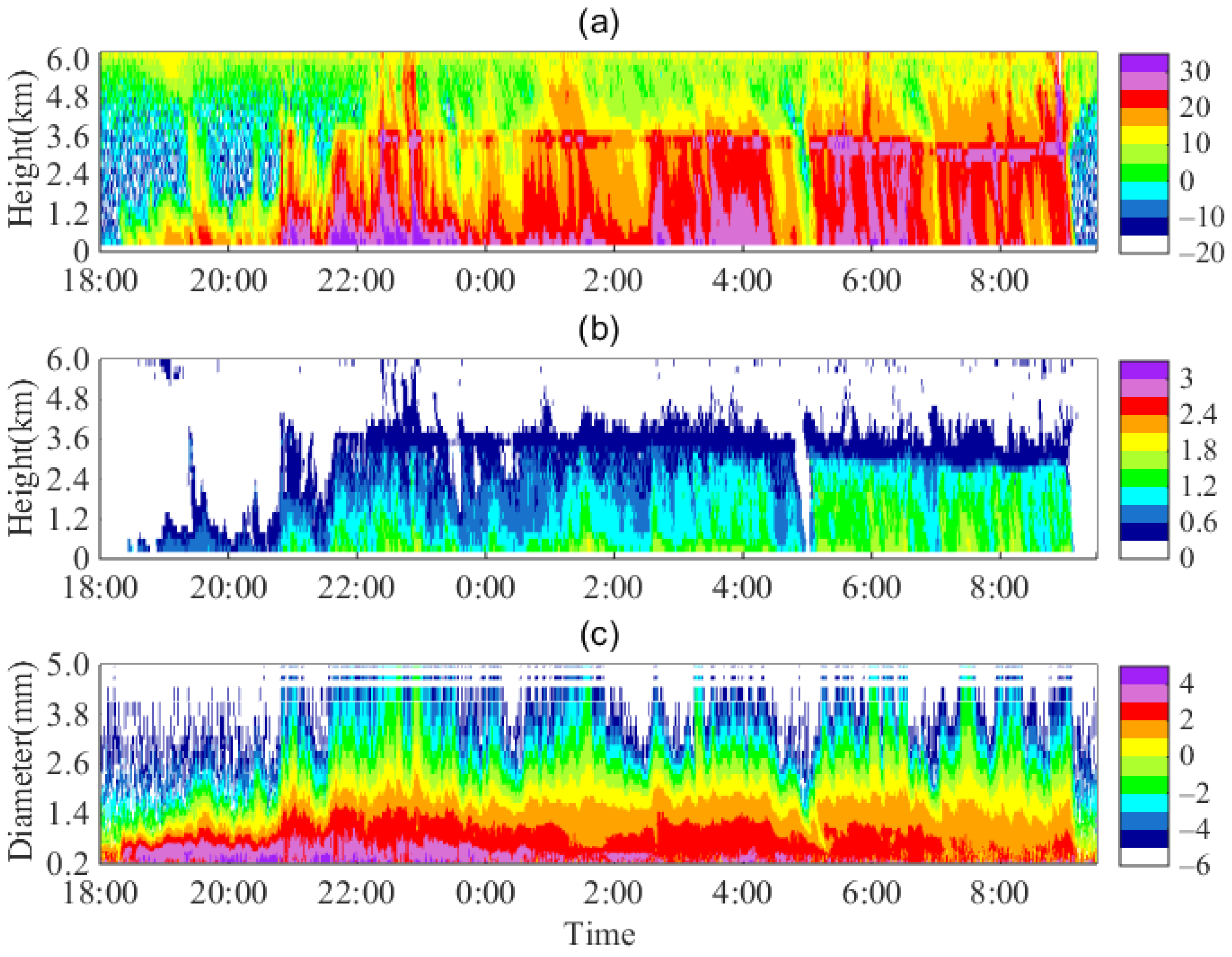
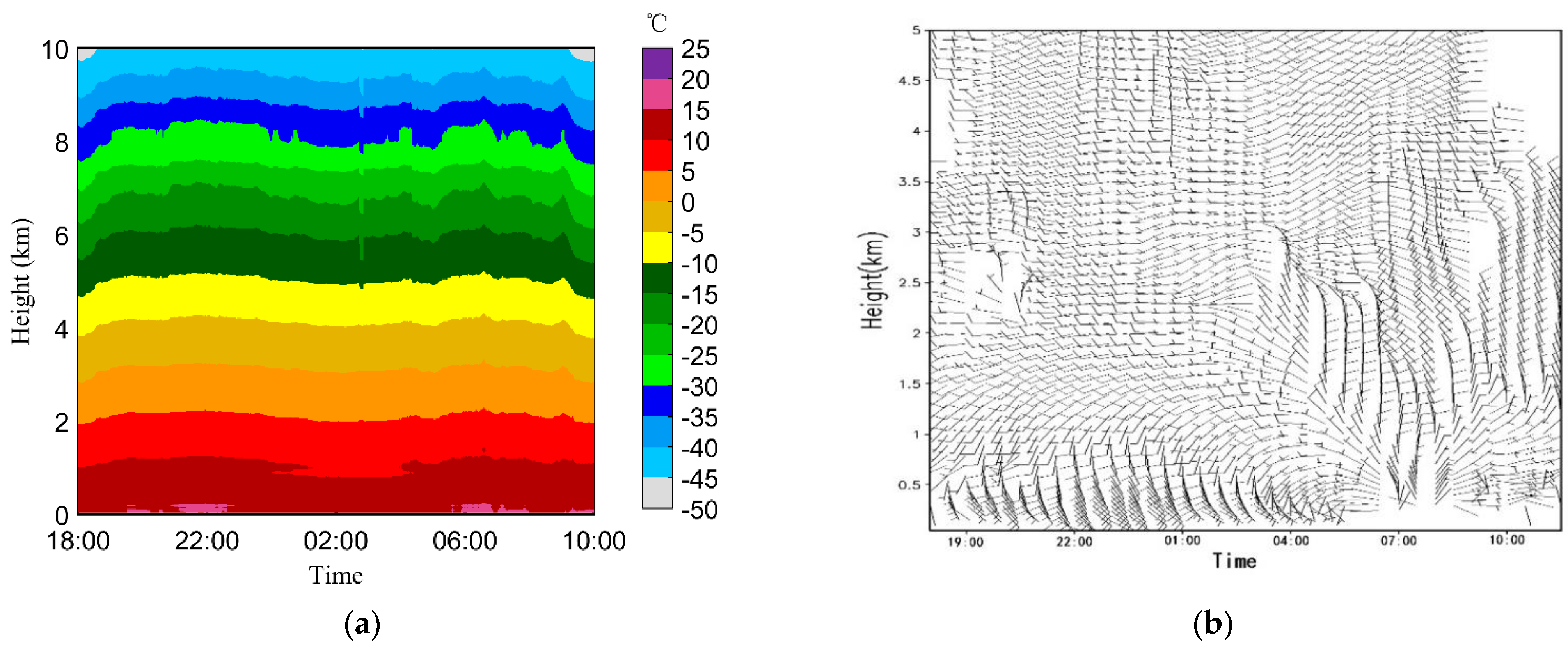
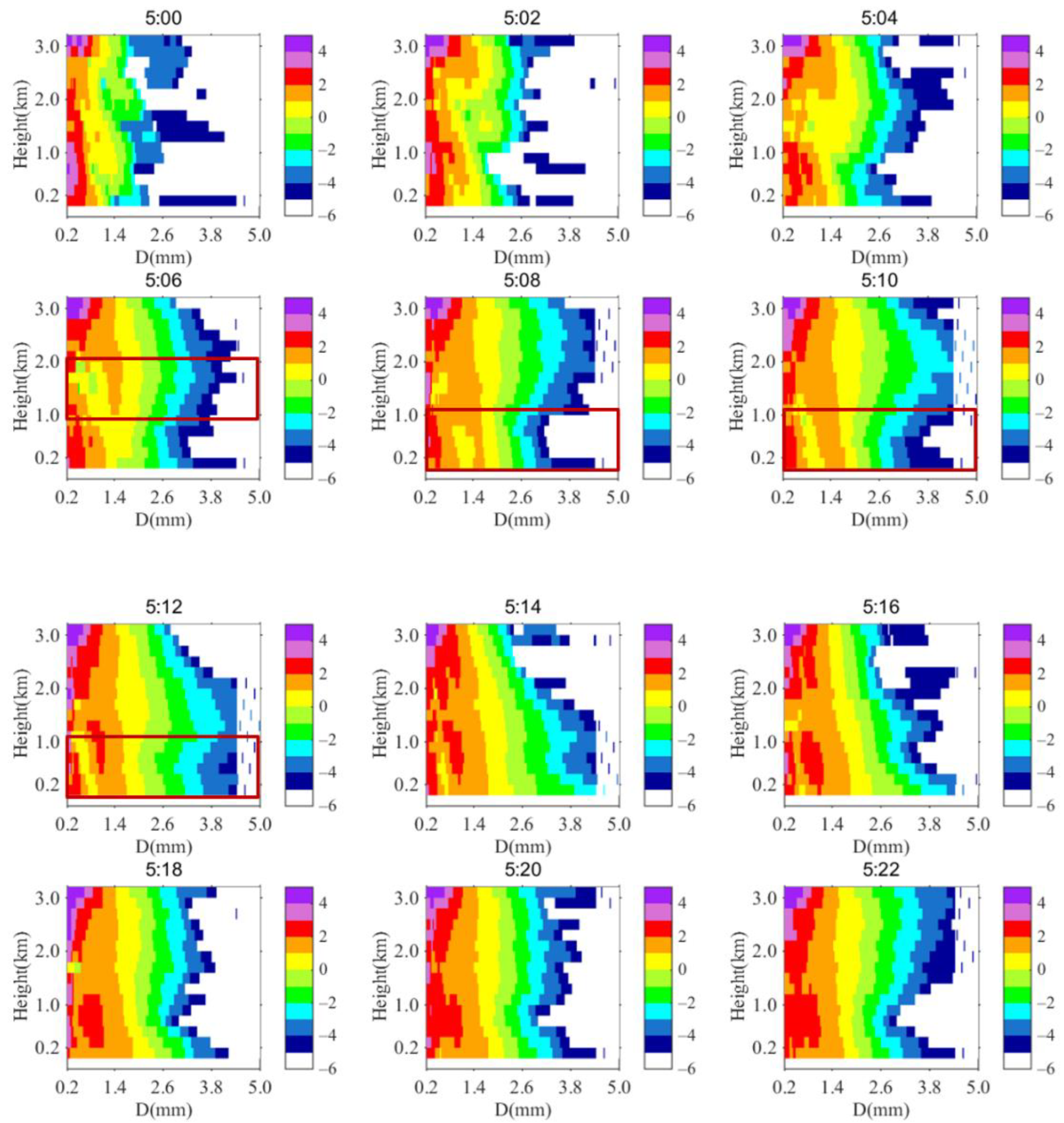
3.2. Stratocumulus Rain on 16 June 2021
3.3. Stratiform Rain on 18–19 August 2021
4. Discussion
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Kollias, P.; Albrecht, B.A. The turbulence structure in a continental stratocumulus cloud from millimeter-wavelength Radar observations. J. Atmos. Sci. 2000, 57, 2417–2434. [Google Scholar] [CrossRef]
- Bringi, V.N.; Chandrasekay, V.; Hubbert, J.; Gorgucci, E.; Randeu, W.L.; Schoenhuber, M. Raindrop size distribution in different climate regimes from distrometer and Dual-Polarized Radar Analysis. J. Atmos. Sci. 2003, 60, 354–365. [Google Scholar] [CrossRef]
- Geoffroy, O.; Siebeama, A.P.; Bumet, F. Characteristics of the raindrop distribution in RICO shallow cumulus. Atmos. Chem. Phys. 2013, 14, 10897–10909. [Google Scholar] [CrossRef] [Green Version]
- Rao, T.N.; Kirankumar, N.V.P.; Radhakrishna, B.; Rao, D.N. On the variability of the shape-slope parameter relations of the shape-slope parameter relations of the gamma raindrop size distribution model. Geophys. Res. Lett. 2006, 33, 351–361. [Google Scholar] [CrossRef]
- Peters, Q.; Fischer, B.; Munster, H.; Clemens, M.; Wagner, A. Profiles of raindrop size distribution as retrieved by Micro rain radars. J. Appl. Meteorol. 2005, 44, 1930–1949. [Google Scholar] [CrossRef]
- Li, H.; Yin, Y.; Shan, Y.P.; Jin, Q. Statistical characteristics of raindrop size distribution for stratiform and convective precipitation at different altitudes in Mt. Huangshan. Chin. J. Atmos. Sci. 2018, 42, 268–280. (In Chinese) [Google Scholar] [CrossRef]
- Shupe, M.D.; Kollias, P.; Poellot, M.; Eloranta, E. On Deriving Vertical Air Motions from Cloud Radar Doppler Spectra. J. Atmos. Ocean. Technol. 2008, 25, 547. [Google Scholar] [CrossRef] [Green Version]
- Liu, L.P.; Xie, L.; Cui, Z.H. Examination and application of Doppler spectral density data in drop size distribution retrieval in weak precipitation by cloud radar. Chin. J. Atmos. Sci. 2014, 38, 223–236. (In Chinese) [Google Scholar] [CrossRef]
- Wen, L.; Liu, S.; Zhao, K.; Li, Y.; Li, L. Precision Evaluation of Micro Rain Radar Observation in Two Precipitation Events. Meter. Mon. 2015, 41, 577–587. (In Chinese) [Google Scholar] [CrossRef]
- Wen, L.; Zhao, K.; Zhang, G.; Xue, M.; Zhou, B.; Liu, S.; Chen, X. Statistical Characteristics of Raindrop Size Distributions Observed in East China during the Asian Summer Monsoon Season using 2D-Video Disdrometer and Micro-rain Radar Data. J. Geophys. Res. Atmos. 2016, 121, 2265–2282. [Google Scholar] [CrossRef] [Green Version]
- Wang, H.; Lei, H.C.; Yang, J.F. Microphysical processes of a stratiform precipitation event over eastern China: Analysis using micro rain radar data. Adv. Atmos. Sci. 2017, 34, 1472–1482. [Google Scholar] [CrossRef]
- Ma, N.K.; Liu, L.P.; Zheng, J.F. Application of Doppler spectral density data in vertical air motions and drop size distribution retrieval in cloud and precipitation by Ka-band Millimeter Radar. Plateau Meteorol. 2019, 38, 325–339. (In Chinese) [Google Scholar] [CrossRef]
- Cifelli, R.; Rutledge, S.A. Vertical motion structure in maritime continent mesoscale convective systems: Results from a 50-MHz profiler. J. Atmos. Sci. 1994, 51, 2631–2652. [Google Scholar] [CrossRef] [Green Version]
- Konwar, M.; Maheskumar, R.S.; Das, S.K.; Morwal, S.B. Nature of light rain during presence and absence of bright band. J. Earth Syst. Sci. 2012, 121, 947–961. [Google Scholar] [CrossRef] [Green Version]
- Konwar, M.; Das, S.K.; Deshpande, S.M.; Chakravarty, K.; Goswami, B.N. Microphysics of clouds and rain over the Western Ghat. J. Geophys. Res. Atmos. 2014, 119, 6140–6159. [Google Scholar] [CrossRef]
- Das, S.; Maitra, A. Vertical profile of rain: Ka band radar observations at tropical locations. J. Hydrol. 2016, 534, 31–41. [Google Scholar] [CrossRef]
- Barber, P.; Yeh, C. Scattering of electromagnetic waves by arbitrarily shaped dielectric bodies. Appl. Opt. 1975, 14, 2864–2872. [Google Scholar] [CrossRef] [PubMed]
- Gossard, E.E. Measurement of cloud droplet size spectra by Doppler radar. J. Atmos. Ocean. Technol. 1994, 11, 712–726. [Google Scholar] [CrossRef] [Green Version]
- Testud, J.; Oury, S.; Black, R.A.; Amayenc, P.; Dou, X. The Concept of “Normalized” Distribution to Describe Raindrop Spectra: A Tool for Cloud Physics and Cloud Remote Sensing. J. Appl. Meteorol. 2001, 40, 1118–1140. [Google Scholar] [CrossRef]
- Reisin, T.G.; Yan, Y.; Levin, Z.; Tzivion, S. Development of giant drops and high-reflectivity cores in Hawaiian clouds: Numerical simulations using a kinematic model with detailed microphysics. Atmos. Res. 1998, 45, 275–297. [Google Scholar] [CrossRef]




| Parameter | MRR | Cloud Radar |
|---|---|---|
| Model | MRR-2 | HMB-KST |
| Transmitting frequency | 24.230 GHz (K-band) | 33.4 GHz (Ka-band) |
| Wavelength | 12.38 mm | 8.5 mm |
| 3 dB beam width | 2° | 0.3° |
| Range resolution | 200 m | 30 m |
| Temporal resolution | 10–3600 s (adjustable) | 3 s |
| Nyquist velocity range | −12.19~12.19 m·s−1 | −18.54~18.54 m·s−1 |
| Height range | 0~6.2 km | 0~15.3 km |
| Primary detection object | Precipitation | Non-precipitation clouds, weak precipitation clouds, weak precipitation |
| Detecting elements | Reflectivity(Z), Doppler spectrum, DSD, R, LWC | Reflectivity (Z), radial velocity (Vr), velocity spectrum width (Sw), liner depolarization ratio (LDR), Doppler spectrum |
| Detection mode | Vertical pointing | Vertical pointing, and other detection modes |
| Parameter | Distrometer |
|---|---|
| Model | OTT Parsivel |
| Sensor type | Laser transmitter |
| Wavelength | 785 nm |
| Sensor components | Red laser shooting |
| Weather type | 22 classes (no rain, fog, rain, snow, drizzle, hail, etc.) |
| Measurement area | 54 cm |
| Measurement range | Particle size: 0~25 mm Velocity: 0~20 m s−1 Rain intensity: 0.001~1200 mm h−1 |
| Height range | 0~15.3 km |
| Measurement range | Non precipitation clouds, weak precipitation clouds, weak precipitation |
| Detection principle | A combination of extinction with forward scattering |
| Working temperature | −40~+60 °C |
| Rain Parameter | Statistics | 16 June 2021 | 18–19 August 2021 |
|---|---|---|---|
| Z | mean deviation | 3.25 | 2.23 |
| correlation coefficient | 0.92 | 0.89 | |
| log10(Nw) | mean deviation | 0.48 | 0.14 |
| correlation coefficient | 0.70 | 0.93 | |
| Dm | mean deviation | 0.16 | 0.09 |
| correlation coefficient | 0.65 | 0.94 |
| BP Number | BS | SS | BPT (min) |
|---|---|---|---|
| 1 | - | 3:17–3:24 (8 min) | |
| 2 | 3:25–3:32 (8 min) | 3:33–3:44 (12 min) | 8 + 8 = 16 |
| 3 | 3:45–3:49 (5 min) | 3:50–3:57 (8 min) | 12 + 5 = 17 |
| 4 | 3:58–4:03 (6 min) | 4:04–4:09 (6 min) | 8 + 6 = 14 |
| 5 | 4:10–4:15 (6 min) | 4:16–4:21 (6 min) | 6 + 6 = 12 |
| 6 | 4:22–4:26 (5 min) | 4:27–4:35 (9 min) | 6 + 5 = 11 |
| 7 | 4:37–4:46 (10 min) | 4:47–4:58 (12 min) | 9 + 10 = 19 |
| 8 | 5:00–5:13 (14 min) | 5:14–5:17 (4 min) | 12 + 14 = 26 |
| 9 | 5:18–5:32 (15 min) | 5:33–5:42 (10 min) | 4 + 15 = 19 |
| 10 | 5:43–5:48 (6 min) | 5:49–5:50 (2 min) | 10 + 6 = 16 |
| 11 | 5:51–5:58 (8 min) | 5:59–6:05 (7 min) | 2 + 8 = 10 |
| 12 | 6:06–6:11 (6 min) | 6:12–6:16 (5 min) | 7 + 6 = 13 |
| 13 | 6:25–6:32 (8 min) | 6:32–6:33 (2 min) | 5 + 8 = 13 |
| 14 | 6:33–6:38 (6 min) | 6:39–6:58 (20 min) | 2 + 6 = 8 |
| 15 | 6:59–7:05 (7 min) | 7:06–7:11 (6 min) | 20 + 7 = 27 |
| 16 | 7:18–7:24 (7 min) | 7:25–7:37 (13 min) | 6 + 7 = 13 |
| 17 | 7:45–7:58 (14 min) | 7:59–8:03 (5 min) | 13 + 14 = 27 |
| 18 | 8:09–8:14 (6 min) | 8:15–8:20 (6 min) | 5 + 6 = 11 |
| 19 | 8:20–8:26 (7 min) | 8:27–8:30 (4 min) | 6 + 7 = 13 |
| 20 | 8:36–8:47 (12 min) | 4 + 12 = 16 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ma, N.; Chen, Y.; Jia, Z.; Liu, L.; Ma, X.; Huang, Y. Analyses of DSD Vertical Evolution and Rain Variation Mechanism in Stratiform Cloud Cases Using Micro Rain Radar. Remote Sens. 2022, 14, 1655. https://doi.org/10.3390/rs14071655
Ma N, Chen Y, Jia Z, Liu L, Ma X, Huang Y. Analyses of DSD Vertical Evolution and Rain Variation Mechanism in Stratiform Cloud Cases Using Micro Rain Radar. Remote Sensing. 2022; 14(7):1655. https://doi.org/10.3390/rs14071655
Chicago/Turabian StyleMa, Ningkun, Yichen Chen, Zuo Jia, Liping Liu, Xincheng Ma, and Yu Huang. 2022. "Analyses of DSD Vertical Evolution and Rain Variation Mechanism in Stratiform Cloud Cases Using Micro Rain Radar" Remote Sensing 14, no. 7: 1655. https://doi.org/10.3390/rs14071655
APA StyleMa, N., Chen, Y., Jia, Z., Liu, L., Ma, X., & Huang, Y. (2022). Analyses of DSD Vertical Evolution and Rain Variation Mechanism in Stratiform Cloud Cases Using Micro Rain Radar. Remote Sensing, 14(7), 1655. https://doi.org/10.3390/rs14071655