A Motion Compensation Method for Shipborne HFSWR by Using Dual Reference RF Signals Generated Onshore
Abstract
:1. Introduction
2. Analysis of the Model and the Principle of Motion Compensation
2.1. System Setting and Receiving the Signal Model
2.2. The Antenna Pattern and the Array Steer Vector of Shipborne Radar-Receiving Antennas
2.3. Principle of Motion Compensation
3. Motion Compensation Method Based on Dual Reference RF Signals
3.1. The Model of Shipborne HFSWR Receiving the Reference RF Signal
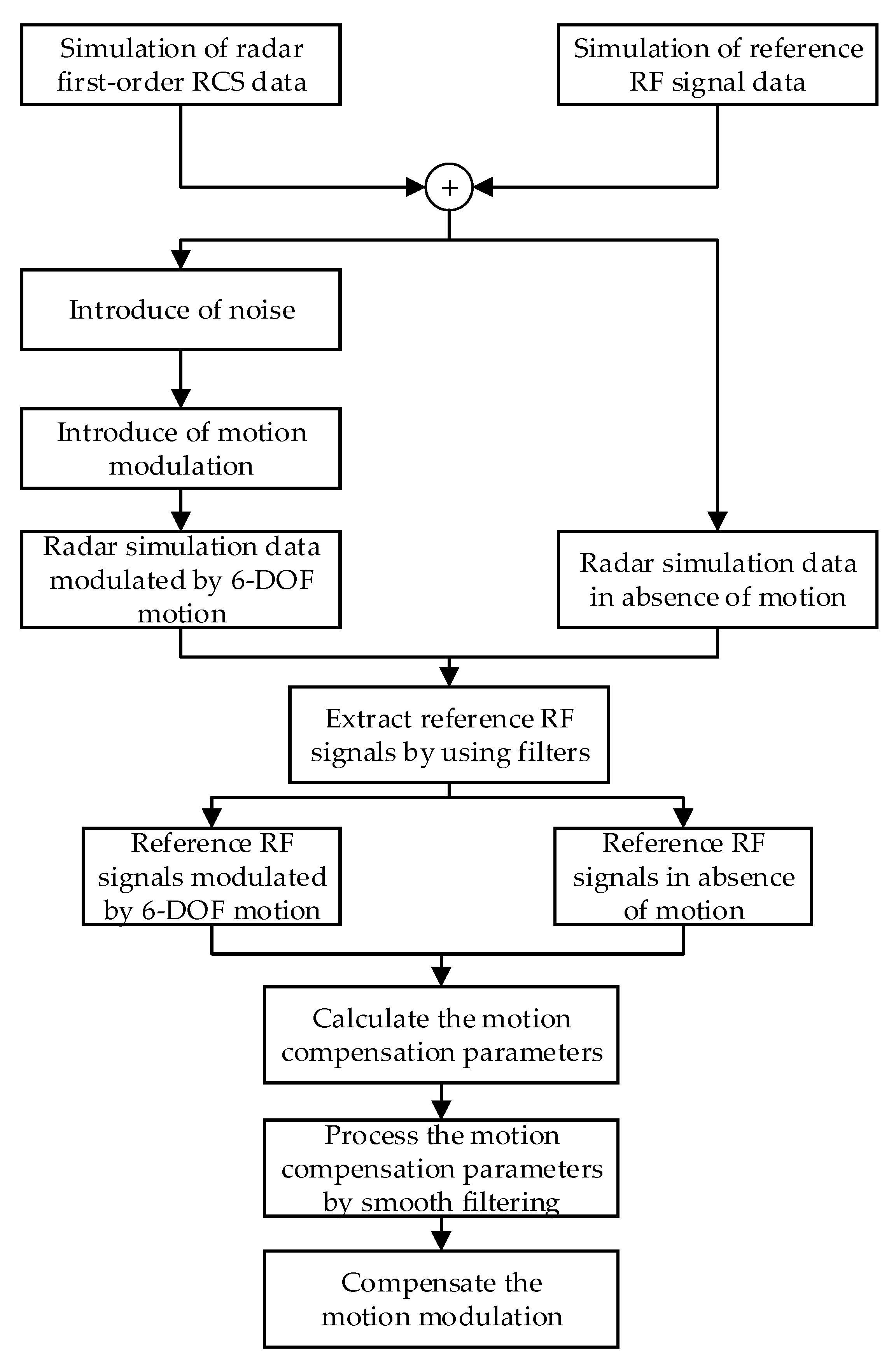
3.2. Calculation of Motion Compensation Parameters
3.3. Several Key Problems of Motion Compensation Based on Dual Reference RF Signals
3.3.1. Extraction of Dual Reference RF Signals
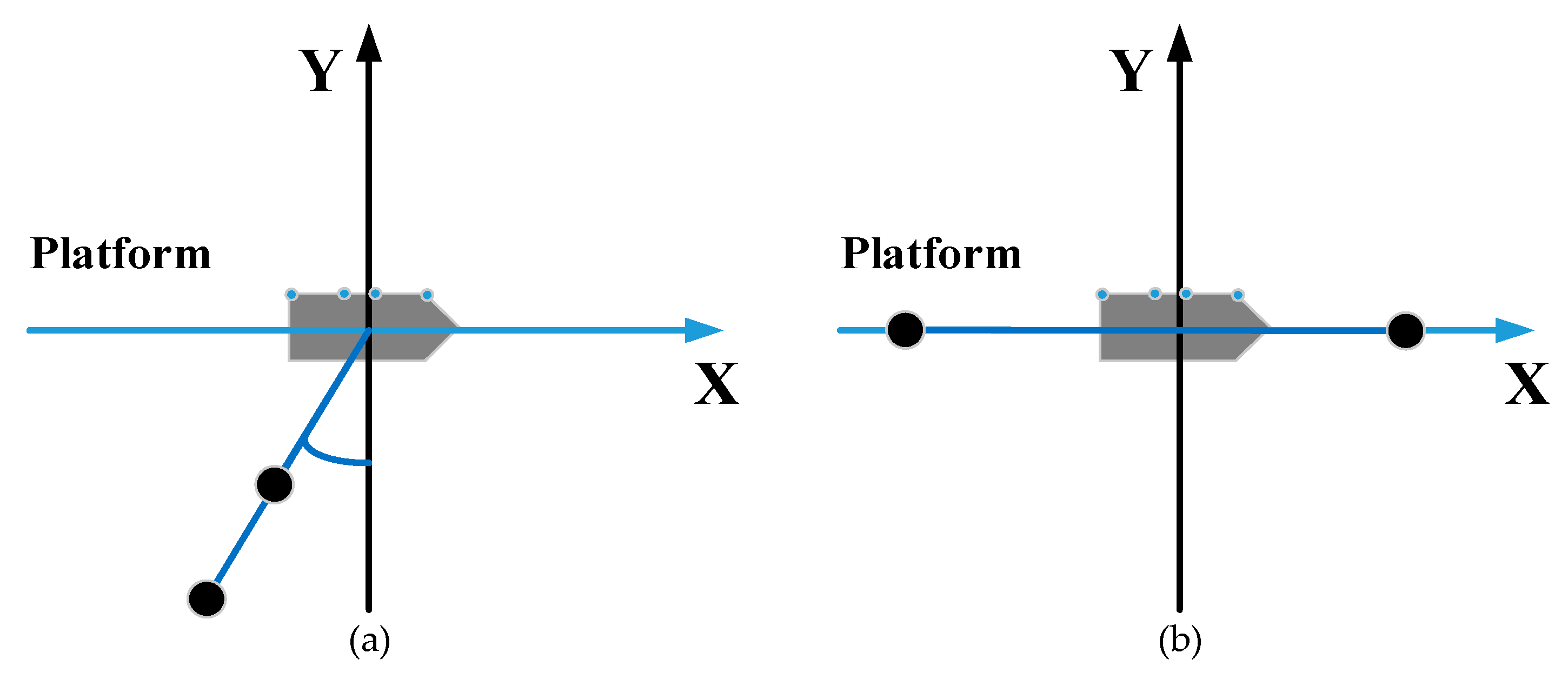
3.3.2. The Influence of Reference RF Signal Locations on Motion Compensation
3.3.3. The Influence of Initial Phase of Reference RF Signals on Motion Compensation
4. Simulation Results
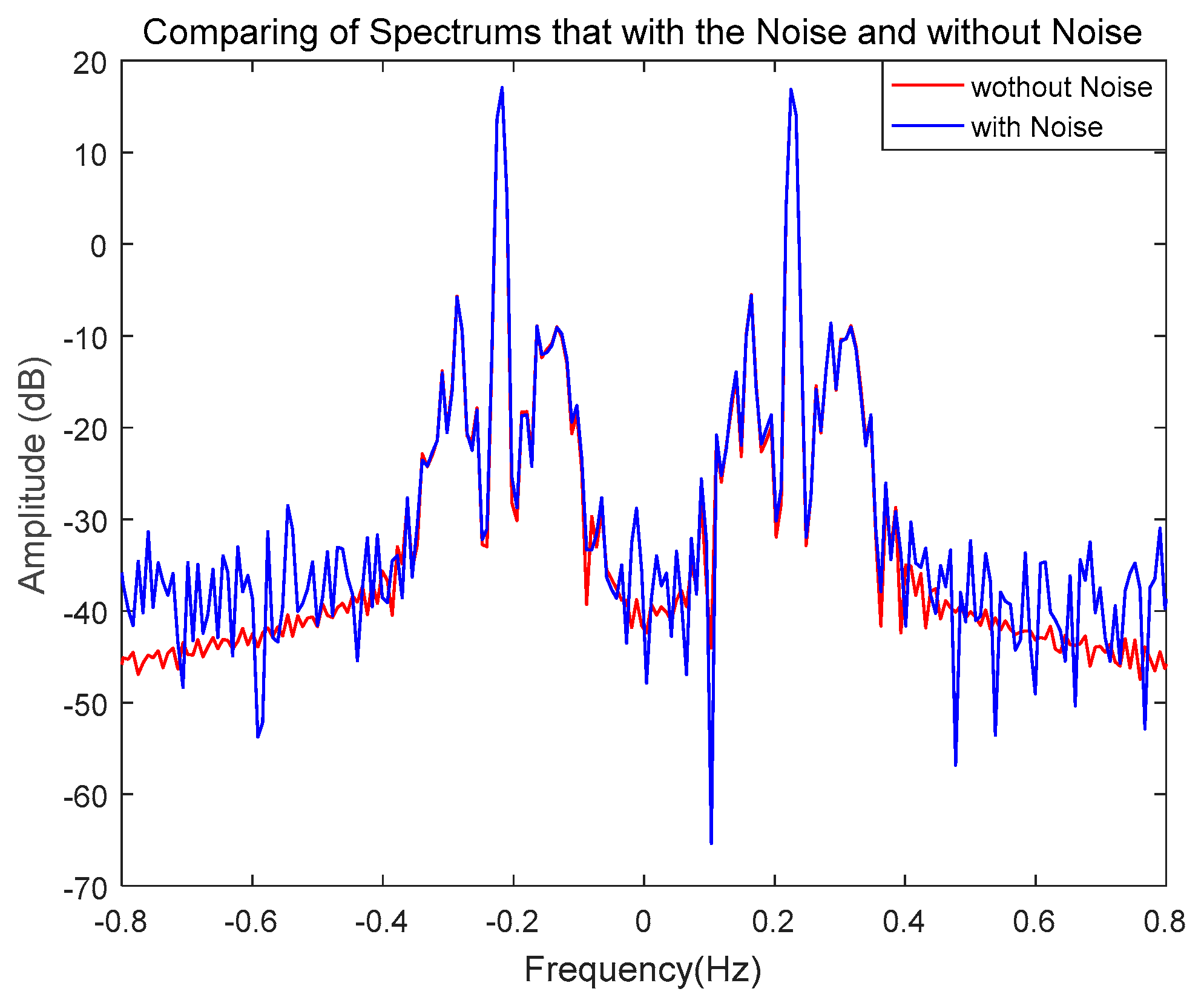
4.1. Extraction of Reference RF Signals in Simulation
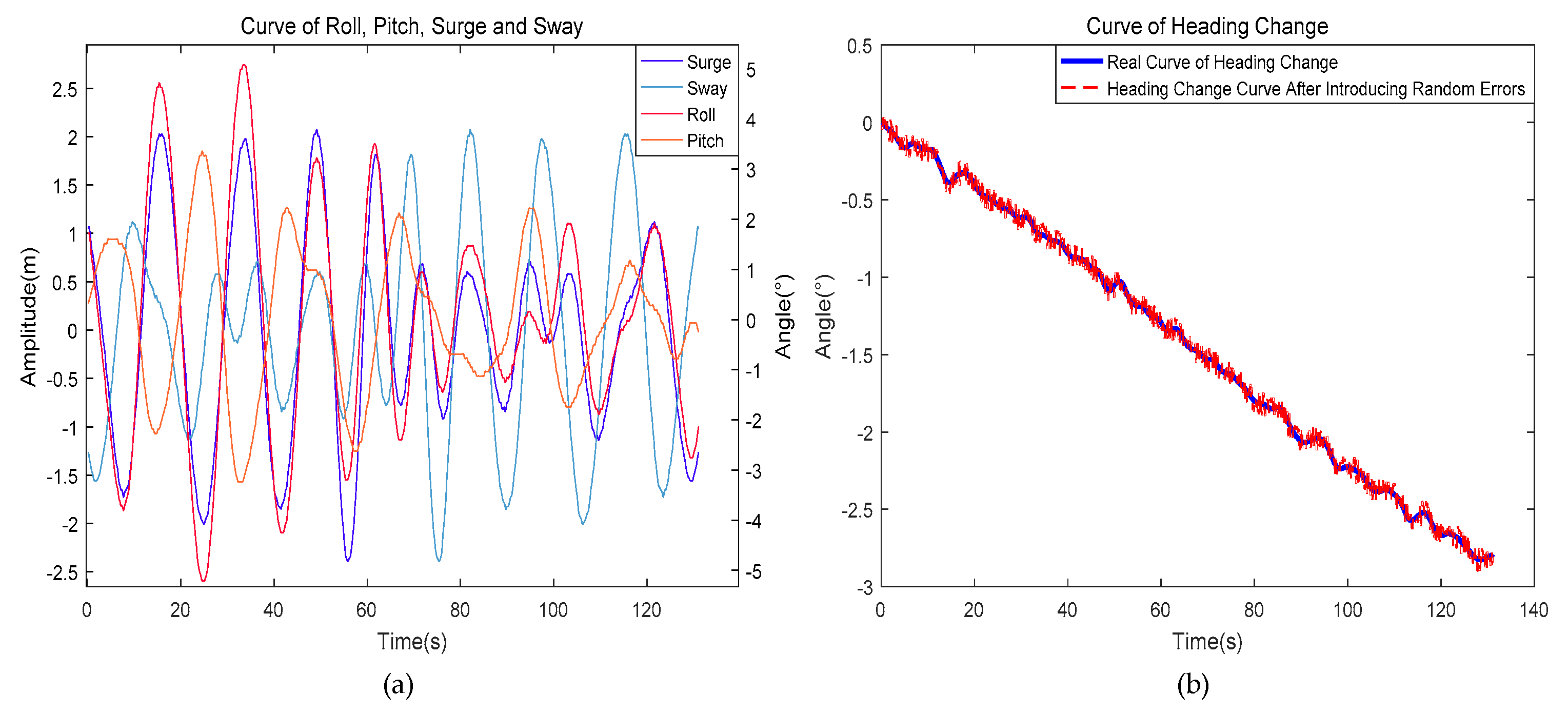
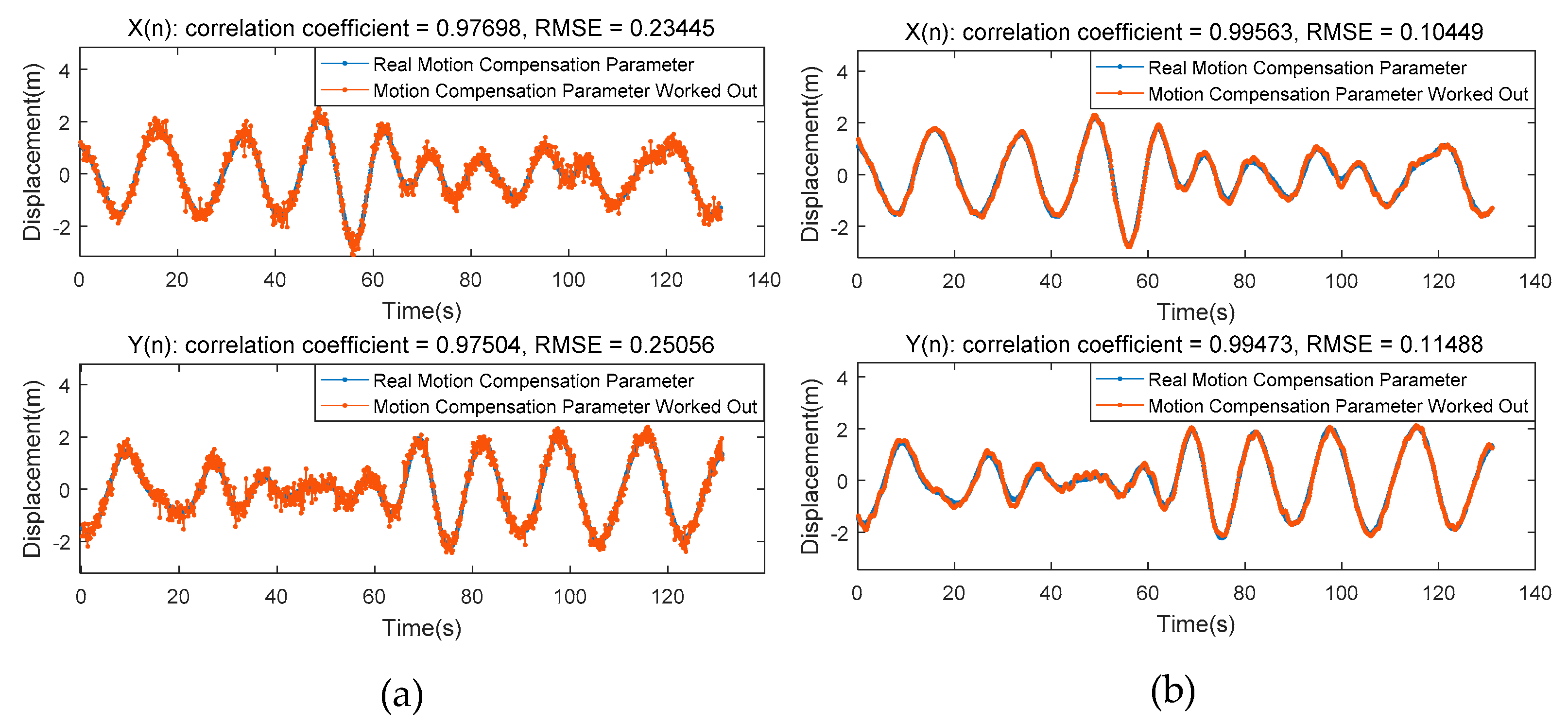
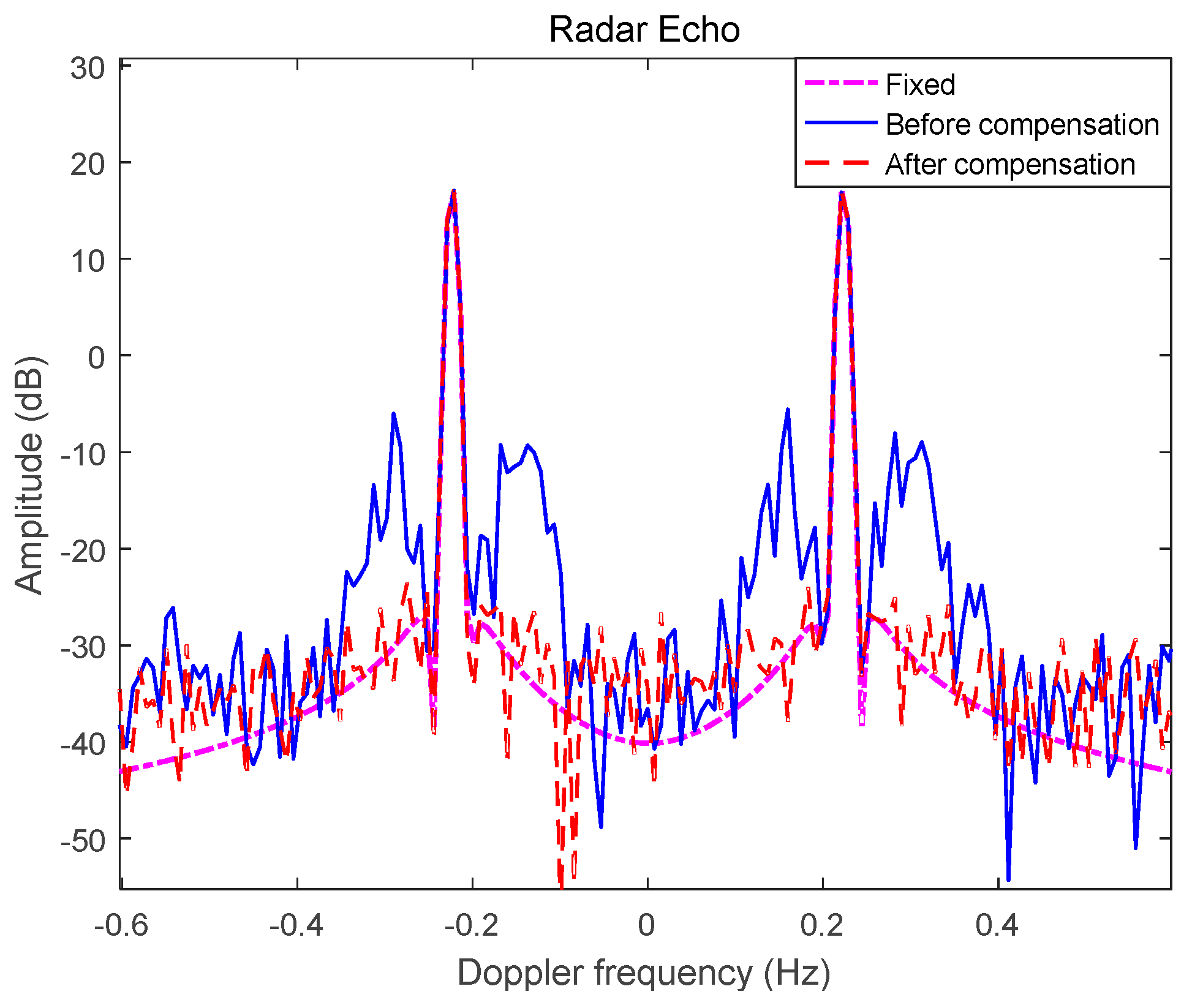
4.2. Calculation Result of Motion Compensation Parameters and Effect of Motion Compensation
4.3. Influence of the Reference RF Signals’ Emission Source Location on the Motion Compensation Effect
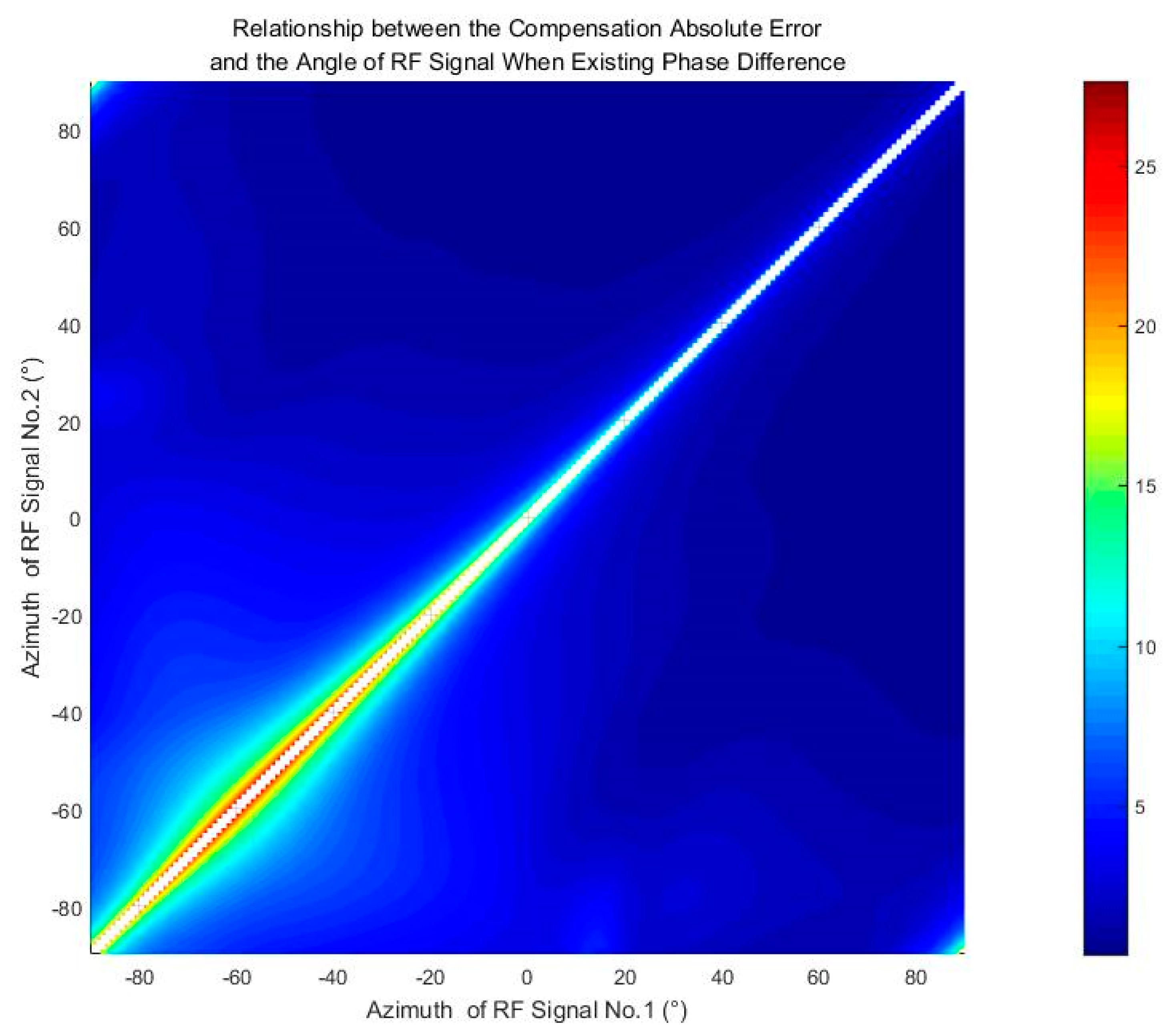
4.3.1. Traversal of the Azimuth of the Dual Reference RF Signals’ Emission Source
4.3.2. Traversal of Azimuth of the Dual Reference RF Signals’ Emission Source with Phase Deviation
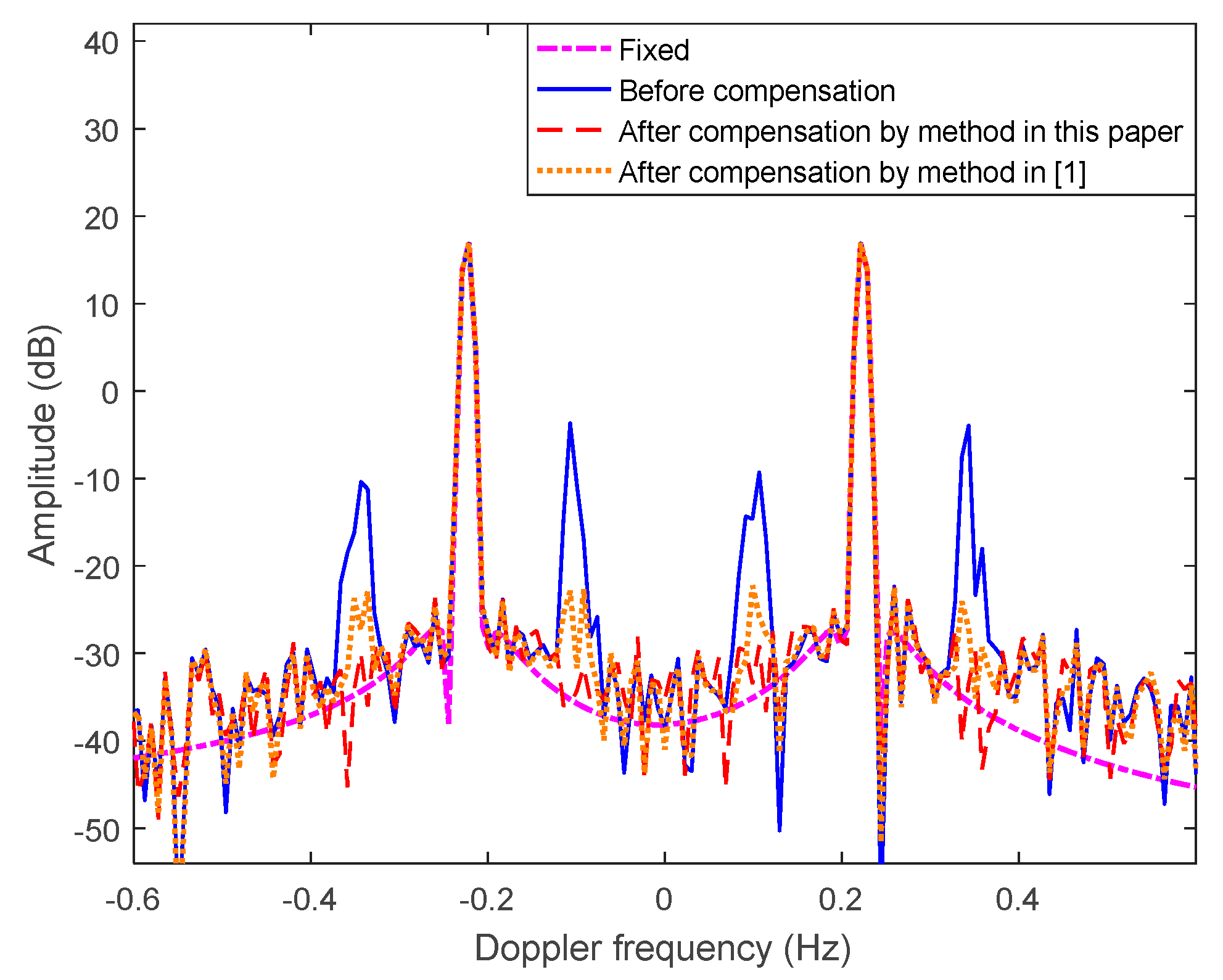
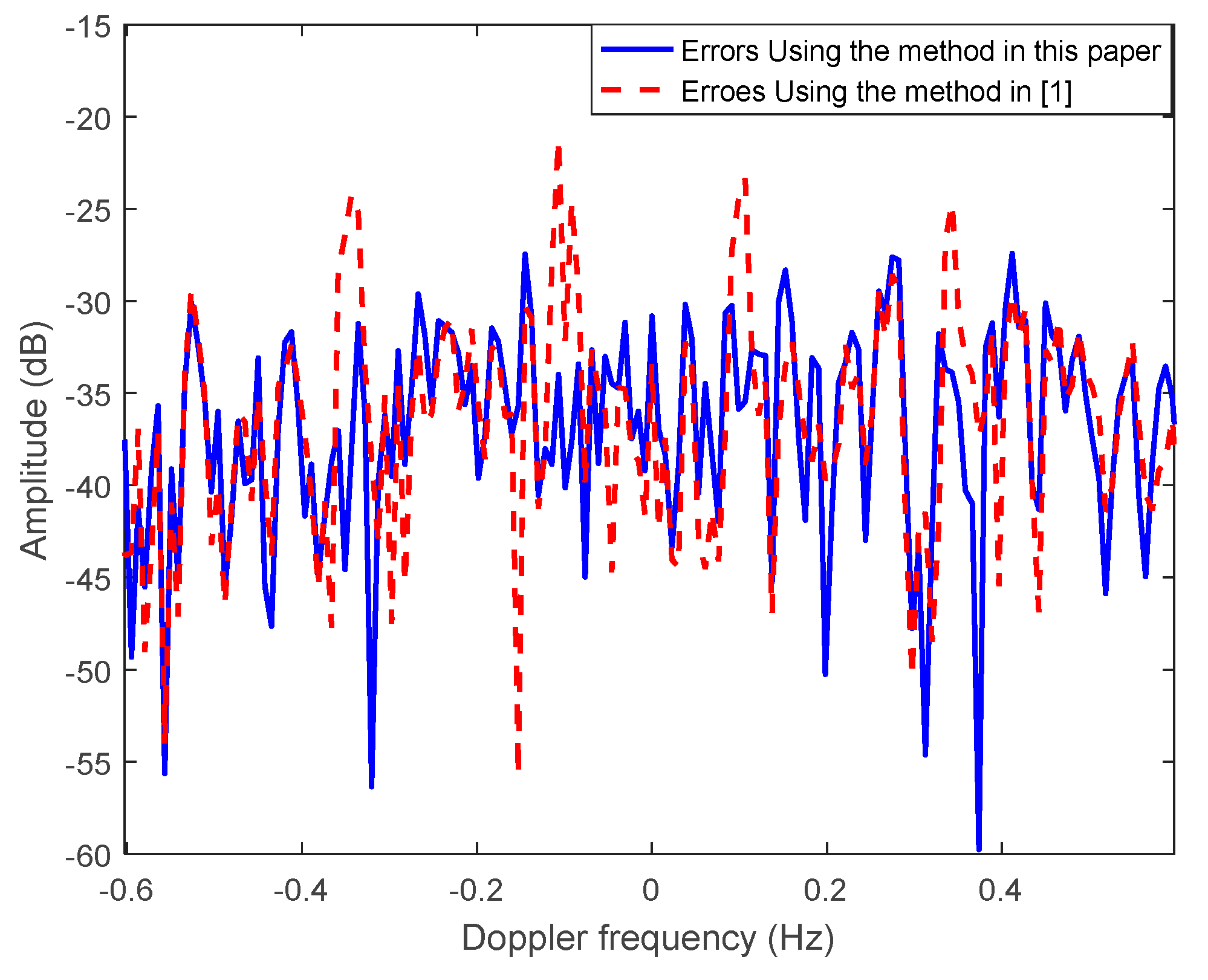
4.4. Method Comparison
5. Discussion
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A
Appendix B
Appendix C
References
- Zhu, D.; Niu, J.; Li, M.; Zhang, L.; Ji, Y.; Wu, Q.M.J. Motion Parameter Identification and Motion Compensation for Shipborne HFSWR by Using the Reference RF Signal Generated at the Shore. Remote Sens. 2020, 12, 2807. [Google Scholar] [CrossRef]
- Xie, J.; Sun, M.; Ji, Z. First-order ocean surface cross-section for shipborne HFSWR. Electron. Lett. 2013, 49, 1025–1026. [Google Scholar] [CrossRef]
- Yao, G.; Xie, J.; Huang, W. First-order ocean surface cross-section for shipborne HFSWR incorporating a horizontal oscillation motion model. IET Radar Sonar Navig. 2018, 12, 973–978. [Google Scholar] [CrossRef]
- Chang, G.; Li, M.; Zhang, L.; Ji, Y.; Xie, J. Measurements of ocean surface currents using shipborne High-Frequency radar. In Proceedings of the IEEE Radar Conference, Cincinnati, OH, USA, 19–23 May 2014. [Google Scholar]
- Chang, G.; Li, M.; Xie, J.; Zhang, L.; Yu, C.; Ji, Y. Ocean Surface Current Measurement Using Shipborne HF Radar: Model and Analysis. IEEE J. Ocean. Eng. 2016, 41, 970–981. [Google Scholar] [CrossRef]
- Wang, J.; Dizaji, R.; Ponsford, A.M. An analysis of phase array radar system on a moving platform. In Proceedings of the IEEE International Radar Conference, Arlington, AG, USA, 9–12 May 2005. [Google Scholar]
- El, K.J.; Guinvarc’H, R.; Gillard, R.; Uguen, B. Sea-echo doppler spectrum perturbation of the received signals from a floating high-frequency surface wave radar. IET Radar Sonar Navig. 2012, 6, 165–171. [Google Scholar]
- Lipa, B.J.; Barrick, D.E.; Isaacson, J.; Lilleboe, P.M. CODAR wave measurements from a North Sea semisubmersible. IEEE J. Oceanic Eng. 1990, 15, 119–125. [Google Scholar] [CrossRef]
- Xie, J.; Yuan, Y.; Liu, Y. Experimental analysis of sea clutter in shipborne HFSWR. IEE Proc. Radar Sonar Navig. 2001, 148, 67–71. [Google Scholar] [CrossRef]
- Gurgel, K.W.; Essen, H.H. On the performance of a shipborne current mapping HF radar. IEEE J. Oceanic Eng. 2000, 25, 183–191. [Google Scholar] [CrossRef]
- Howell, R.; Walsh, J. Measurement of Ocean Wave Spectra Using a Ship-Mounted HF Radar. IEEE J. Oceanic Eng. 1993, 18, 306–310. [Google Scholar] [CrossRef]
- Walsh, J.; Huang, W.; Gill, E. The First-Order High Frequency Radar Ocean Surface Cross Section for an Antenna on a Floating Platform. IEEE Trans. Antenn. Propag. 2010, 58, 2994–3003. [Google Scholar] [CrossRef]
- Walsh, J.; Huang, W.; Gill, E. The Second-Order High Frequency Radar Ocean Surface Cross Section for an Antenna on a Floating Platform. IEEE Trans. Antenn. Propag. 2012, 60, 4804–4813. [Google Scholar] [CrossRef]
- Yao, G.; Xie, J.; Ji, Z.; Sun, M. The first-order ocean surface cross section for shipborne HFSWR with rotation motion. In Proceedings of the 2017 IEEE Radar Conference (RadarConf), Seattle, DC, USA, 8–12 May 2017. [Google Scholar]
- Sun, M.; Xie, J.; Ji, Z.; Yao, G. Ocean surface cross sections for shipborne HFSWR with sway motion. Radio Sci. 2016, 51, 1745–1757. [Google Scholar] [CrossRef] [Green Version]
- Ma, Y.; Gill, E.W.; Huang, W. High Frequency Radar Cross Sections of the Ocean Surface Incorporating Pitch and Roll Motions of a Floating Platform. In Proceedings of the 2018 OCEANS-MTS/IEEE Kobe Techno-Oceans (OTO), Kobe, Japan, 28–31 May 2018. [Google Scholar]
- Ma, Y.; Gill, E.W.; Huang, W. Bistatic High-Frequency Radar Ocean Surface Cross Section Incorporating a Dual-Frequency Platform Motion Model. IEEE J. Oceanic Eng. 2018, 43, 205–210. [Google Scholar] [CrossRef]
- Yao, G.; Xie, J.; Huang, W. Ocean Surface Cross Section for Bistatic HF Radar Incorporating a Six DOF Oscillation Motion Model. Remote Sens. 2019, 11, 2738. [Google Scholar] [CrossRef] [Green Version]
- Gill, E.W.; Ma, Y.; Huang, W. Motion compensation for high-frequency surface wave radar on a floating platform. IET Radar Sonar Navig. 2018, 12, 37–45. [Google Scholar] [CrossRef]
- Bourges, A.; Guinvarc’h, R.; Uguen, B.; Gillard, R. High-frequency surface wave radar based on a sea floating antenna concept. IET Microw. Antennas Propag. 2009, 3, 1237–1244. [Google Scholar] [CrossRef]
- Bourges, A.; Guinvarc’H, R.; Uguen, B.; Gillard, R. Swell Compensation for High Frequency antenna array on buoys. In Proceedings of the 2006 IEEE Antennas and Propagation Society International Symposium, Albuquerque, NM, USA, 9–14 July 2006. [Google Scholar]
- Xu, X. Study on Some Problems of Signal Processing in Buoys-based HF Surface Wave Radar. Ph.D. Thesis, Wuhan University, Wuhan, China, October 2014. [Google Scholar]
- Das, S.N.; Shiraishi, S.; Das, S.K. Mathematical modeling of sway, roll and yaw motions: Order-wise analysis to determine coupled characteristics and numerical simulation for restoring moment’s sensitivity analysis. Acta Mech. 2010, 213, 305–322. [Google Scholar] [CrossRef]
- Tahar, A.; Kim, M.H. Hull/mooring/riser coupled dynamic analysis and sensitivity study of a tanker-based FPSO. Appl. Ocean Res. 2003, 25, 367–382. [Google Scholar] [CrossRef]




| Parameter Type | Parameter Name | Symbol | Value |
|---|---|---|---|
| Parameters of Shipborne Platform | Number of Antennas | 8 | |
| Shipborne Platform Width | 8 m | ||
| Height of Gravity Center and Deck | 7.5 m | ||
| Distance Between Adjacent Receiving Antennas | 14 m | ||
| Height of Receiving Antennas | 2 m | ||
| Parameters of Reference RF Signal No. 1 | Distance Between Reference RF Signal No. 1 and Shipborne Platform | 4,820,126 Hz | |
| Transmitting Frequency of Reference RF Signal No. 1 | 50 km | ||
| Azimuth of Reference RF Signal No. 1 | |||
| Transmitting Power of Reference RF Signal No. 1 | 35 W | ||
| Parameters of Reference RF Signal No. 2 | Distance Between Reference RF Signal No. 2 and Shipborne Platform | 4,789,630 Hz | |
| Transmitting Frequency of Reference RF Signal No. 2 | 50 km | ||
| Azimuth of Reference RF Signal No. 2 | |||
| Transmitting Power of Reference RF Signal No. 2 | 35 W | ||
| Parameters of Radar System | Carrier Frequency of Radar | 4.8 MHz | |
| Initial Frequency of Radar Local Oscillator Signal | 4.77 MHz | ||
| Bandwidth of Radar | 60 kHz | ||
| Sweep Period of Radar | 0.128 s | ||
| Number of Sweep Periods | 1024 | ||
| Coherent Integration Time | 131.072 s | ||
| Change in Range of Each Sea Patch | 2.5 km | ||
| Change in Angle of Each Sea Patch | |||
| Radar Detection in Angle | |||
| Frequency Resolution | Hz | ||
| Signal to Noise Ratio | 40 dB |
| Degree of Freedom (DOF) | Amplitude (a) | Angular Frequency ( ) |
|---|---|---|
| Surge | 1.335 m | 0.7351 rad/s |
| Sway | 1.093 m | 0.8105 rad/s |
| Roll | 0.8859 rad/s | |
| Pitch | 0.7037 rad/s | |
| Yaw | 0.7603 rad/s |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chen, M.; Niu, J.; Li, M.; Zhang, L.; Ji, Y.; Wan, W.; Wu, Q.M.J. A Motion Compensation Method for Shipborne HFSWR by Using Dual Reference RF Signals Generated Onshore. Remote Sens. 2022, 14, 1055. https://doi.org/10.3390/rs14051055
Chen M, Niu J, Li M, Zhang L, Ji Y, Wan W, Wu QMJ. A Motion Compensation Method for Shipborne HFSWR by Using Dual Reference RF Signals Generated Onshore. Remote Sensing. 2022; 14(5):1055. https://doi.org/10.3390/rs14051055
Chicago/Turabian StyleChen, Maorong, Jiong Niu, Ming Li, Ling Zhang, Yonggang Ji, Wenxiang Wan, and Q. M. Jonathan Wu. 2022. "A Motion Compensation Method for Shipborne HFSWR by Using Dual Reference RF Signals Generated Onshore" Remote Sensing 14, no. 5: 1055. https://doi.org/10.3390/rs14051055
APA StyleChen, M., Niu, J., Li, M., Zhang, L., Ji, Y., Wan, W., & Wu, Q. M. J. (2022). A Motion Compensation Method for Shipborne HFSWR by Using Dual Reference RF Signals Generated Onshore. Remote Sensing, 14(5), 1055. https://doi.org/10.3390/rs14051055







