Real-Time BDS-3 Clock Estimation with a Multi-Frequency Uncombined Model including New B1C/B2a Signals
Abstract
:1. Introduction
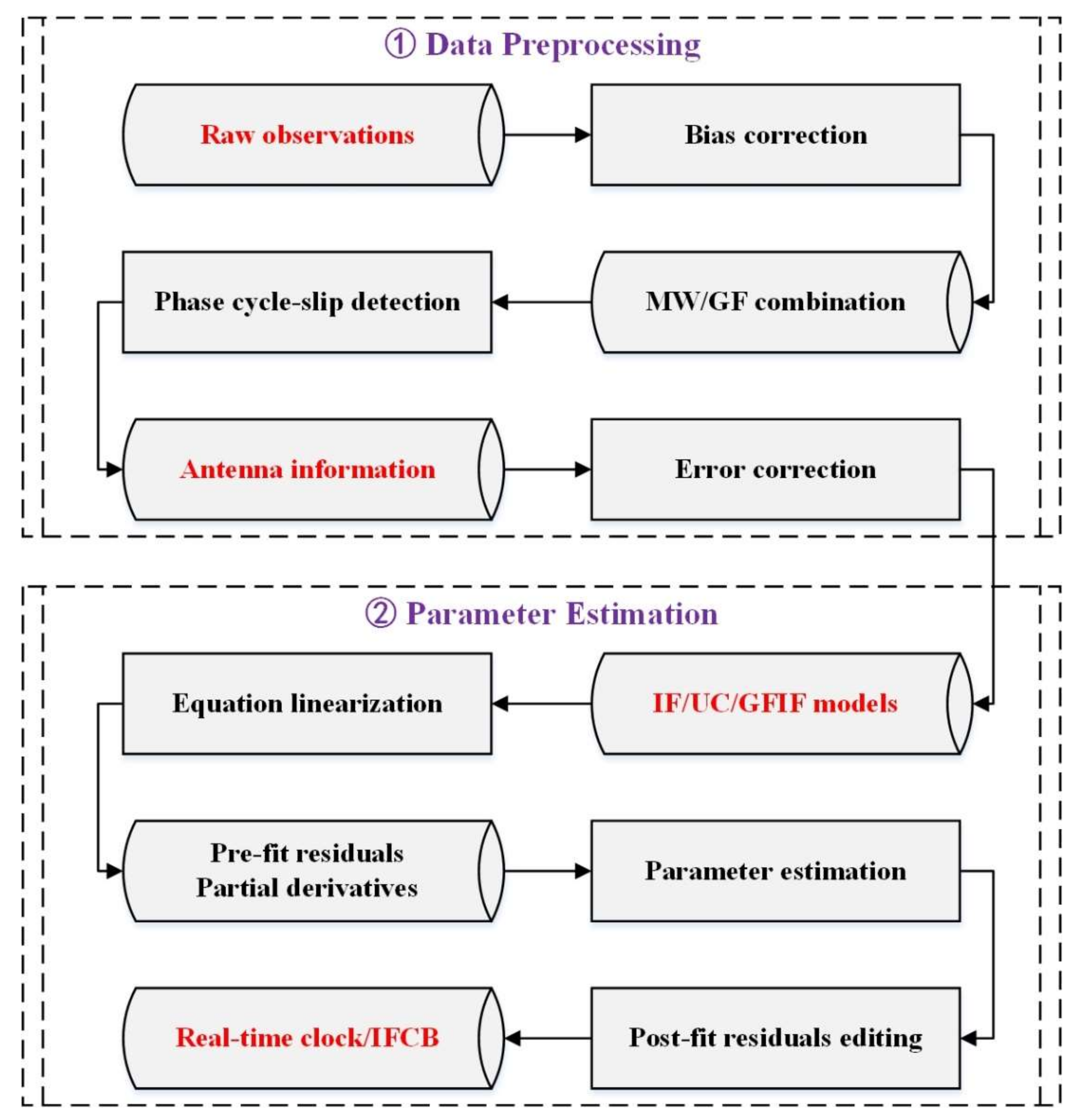
2. Materials and Methods
2.1. Dual-Frequency IF Model
2.2. Multi-Frequency UC Model
2.3. Multi-Frequency GFIF Model
2.4. Clock and IFCB Estimation
3. Results and Discussion
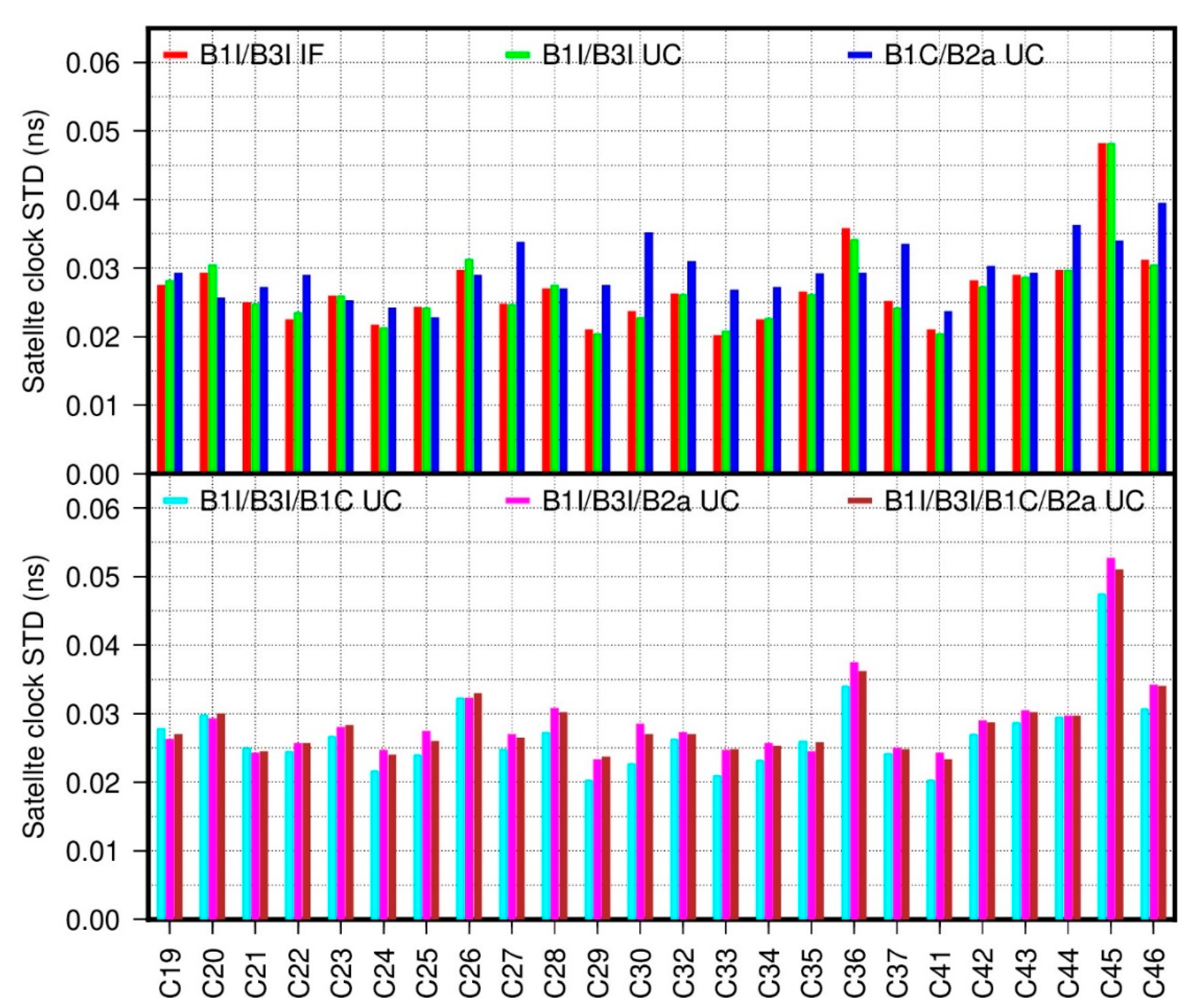
3.1. Satellite Clock Comparison
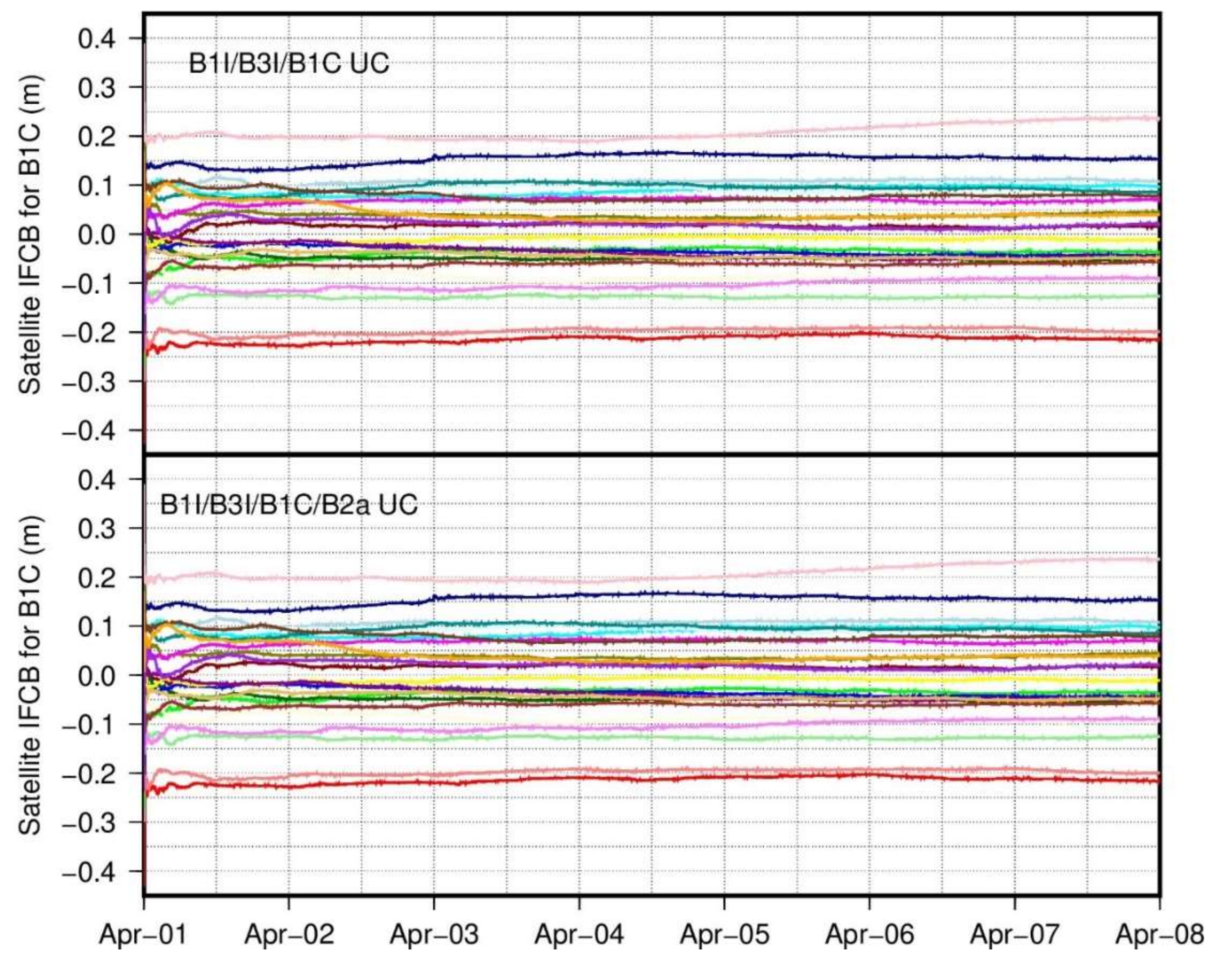
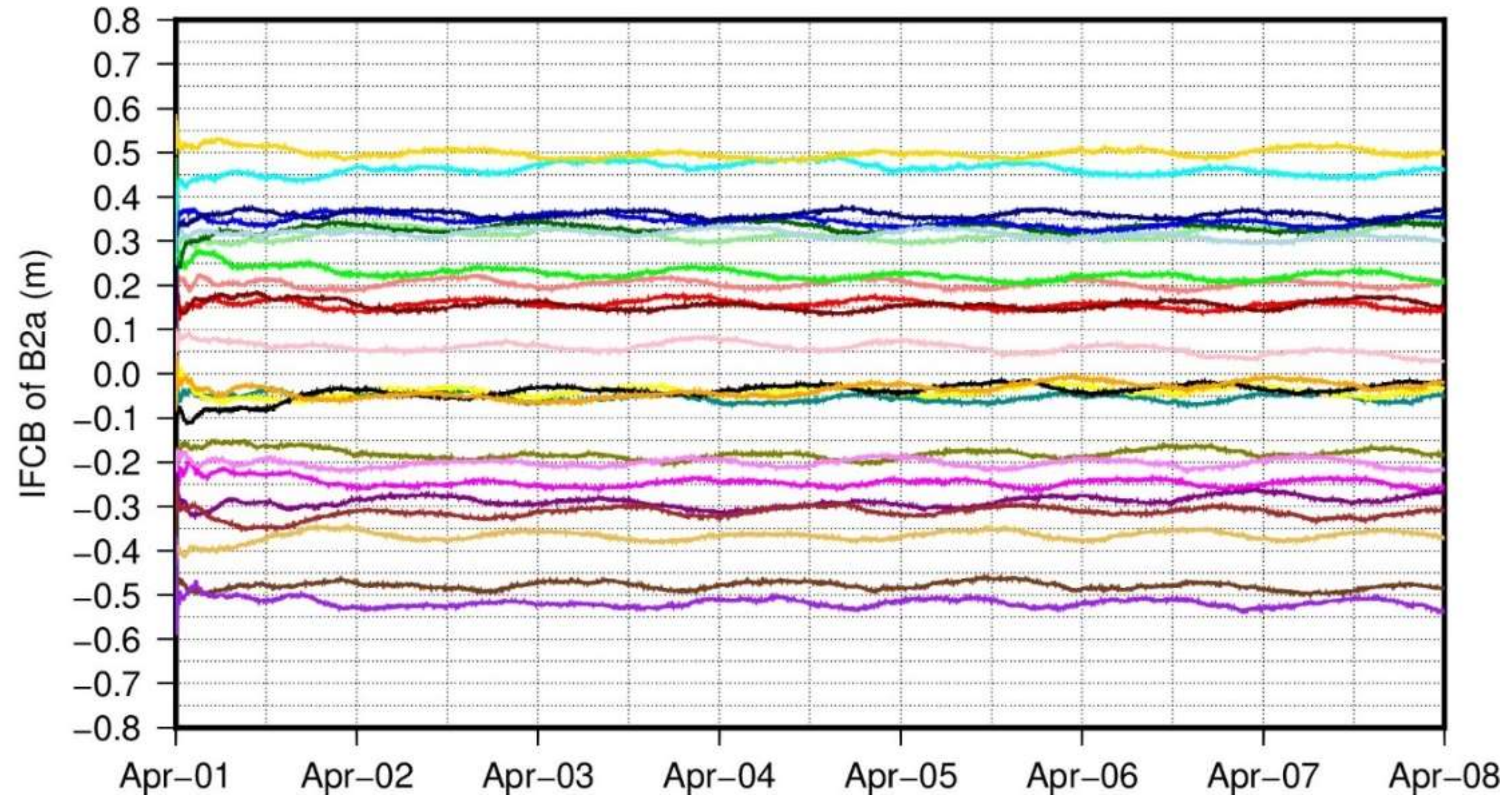
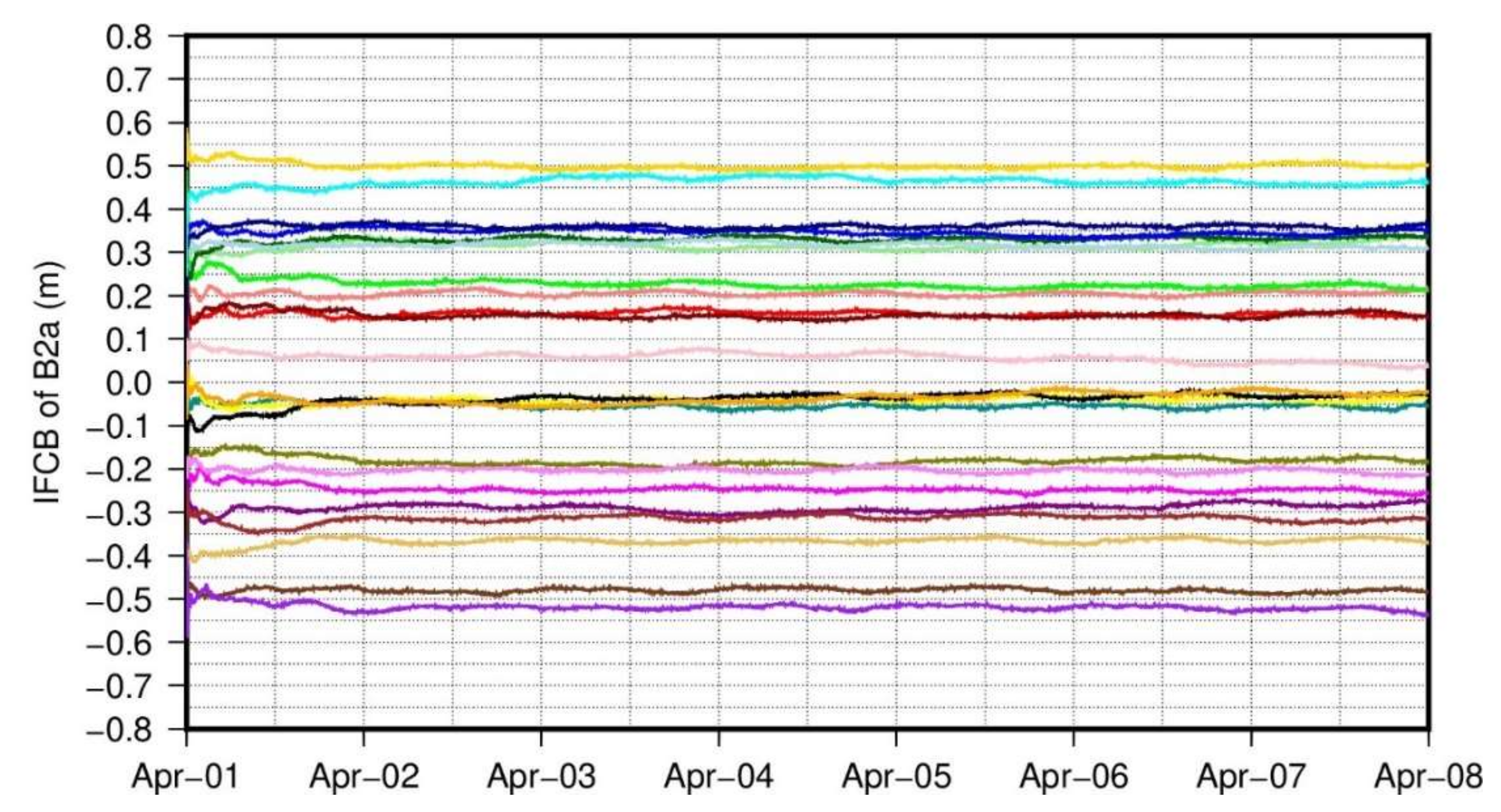
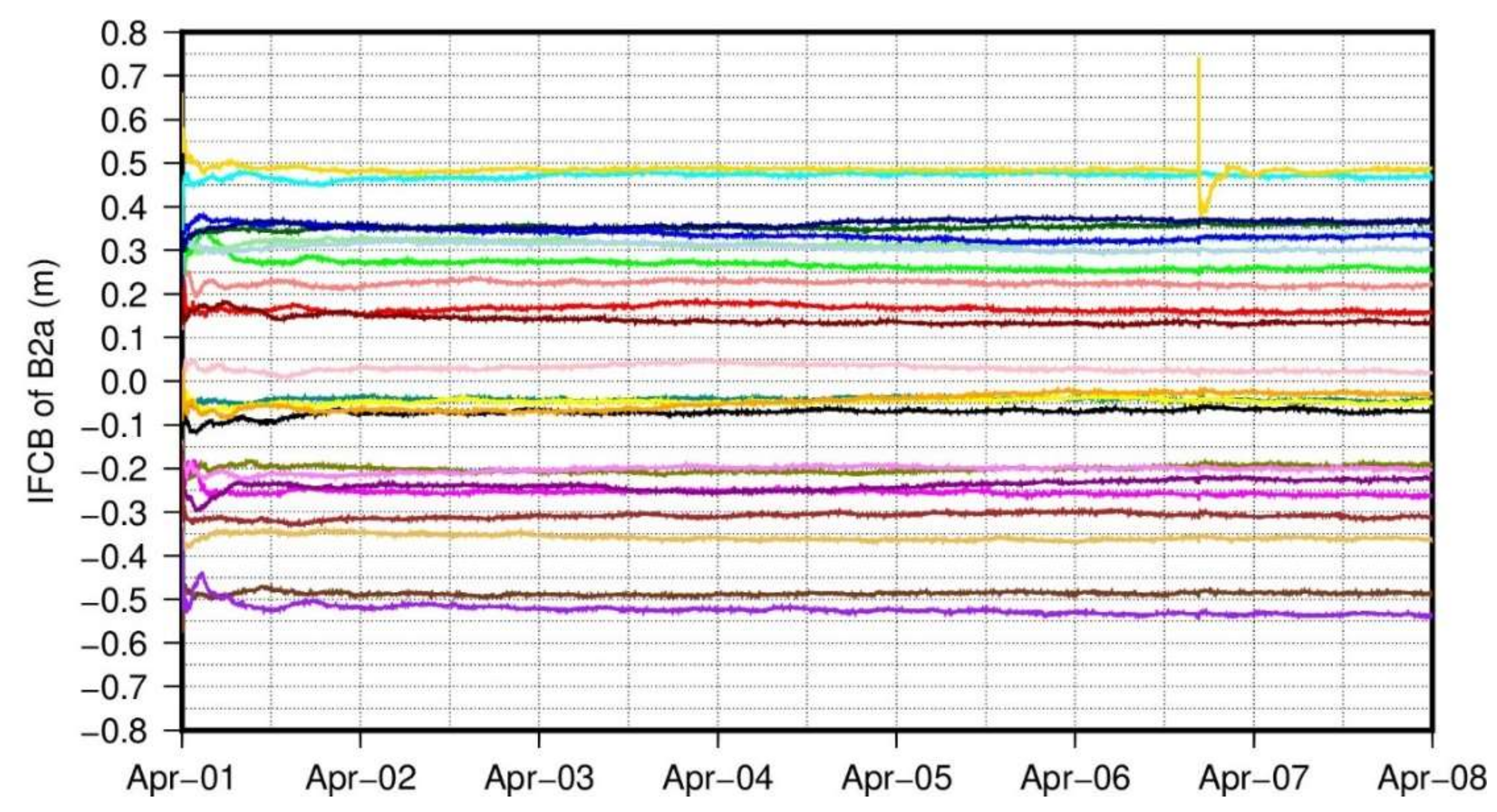
3.2. Satellite IFCB Characteristic
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Johnston, G.; Riddell, A.; Hausler, G. The International GNSS Service. In Springer Handbook of Global Navigation Satellite Systems; Springer Science and Business Media LLC: Berlin/Heidelberg, Germany, 2017; pp. 967–982. [Google Scholar]
- Deng, Z.; Fritsche, M.; Nischan, T.; Bradke, M. Multi-GNSS Ultra Rapid Orbit-, Clock- & EOP-Product Series. GFZ Data Serv. 2016. [Google Scholar] [CrossRef]
- Bock, H.; Dach, R.; Jäggi, A.; Beutler, G. High-rate GPS clock corrections from CODE: Support of 1 Hz applications. J. Geod. 2009, 83, 1083–1094. [Google Scholar] [CrossRef] [Green Version]
- Hauschild, A.; Montenbruck, O. Kalman-filter-based GPS clock estimation for near real-time positioning. GPS Solut. 2008, 13, 173–182. [Google Scholar] [CrossRef]
- Gong, X.; Gu, S.; Lou, Y.; Zheng, F.; Ge, M.; Liu, J. An efficient solution of real-time data processing for multi-GNSS network. J. Geod. 2017, 92, 797–809. [Google Scholar] [CrossRef]
- Li, X.; Xiong, Y.; Yuan, Y.; Wu, J.; Li, X.; Zhang, K.; Huang, J. Real-time estimation of multi-GNSS integer recovery clock with undifferenced ambiguity resolution. J. Geod. 2019, 93, 2515–2528. [Google Scholar] [CrossRef]
- Fu, W.; Huang, G.; Zhang, Q.; Gu, S.; Ge, M.; Schuh, H. Multi-GNSS real-time clock estimation using sequential least square adjustment with online quality control. J. Geod. 2018, 93, 963–976. [Google Scholar] [CrossRef]
- Zhao, Q.; Sun, B.; Dai, Z.; Hu, Z.; Shi, C.; Liu, J. Real-time detection and repair of cycle slips in triple-frequency GNSS measurements. GPS Solut. 2014, 19, 381–391. [Google Scholar] [CrossRef]
- Li, T.; Melachroinos, S. An enhanced cycle slip repair algorithm for real-time multi-GNSS, multi-frequency data processing. GPS Solut. 2019, 23, 1. [Google Scholar] [CrossRef]
- Geng, J.; Bock, Y. Triple-frequency GPS precise point positioning with rapid ambiguity resolution. J. Geod. 2013, 87, 449–460. [Google Scholar] [CrossRef]
- Li, X.; Li, X.; Liu, G.; Yuan, Y.; Freeshah, M.; Zhang, K.; Zhou, F. BDS multi-frequency PPP ambiguity resolution with new B2a/B2b/B2a + b signals and legacy B1I/B3I signals. J. Geod. 2020, 94, 107. [Google Scholar] [CrossRef]
- Schönemann, E.; Becker, M.; Springer, T. A new Approach for GNSS Analysis in a Multi-GNSS and Multi-Signal Environment. J. Géod. Sci. 2011, 1, 204–214. [Google Scholar] [CrossRef] [Green Version]
- Strasser, S.; Mayer-Gürr, T.; Zehentner, N. Processing of GNSS constellations and ground station networks using the raw observation approach. J. Geod. 2018, 93, 1045–1057. [Google Scholar] [CrossRef] [Green Version]
- Lu, M.; Li, W.; Yao, Z.; Cui, X. Overview of BDS III new signals. Navigation 2019, 66, 19–35. [Google Scholar] [CrossRef] [Green Version]
- Jin, S.; Su, K. PPP models and performances from single- to quad-frequency BDS observations. Satell. Navig. 2020, 1, 16. [Google Scholar] [CrossRef]
- Li, R.; Wang, N.; Li, Z.; Zhang, Y.; Wang, Z.; Ma, H. Precise orbit determination of BDS-3 satellites using B1C and B2a dual-frequency measurements. GPS Solut. 2021, 25, 95. [Google Scholar] [CrossRef]
- Fu, W.; Wang, L.; Chen, R.; Han, Y.; Zhou, H.; Li, T. Combined BDS-2/BDS-3 real-time satellite clock estimation with the overlapping B1I/B3I signals. Adv. Space Res. 2021, 68, 4470–4483. [Google Scholar] [CrossRef]
- Montenbruck, O.; Hauschild, A.; Steigenberger, P.; Langley, R.B. Three’s the challenge: A close look at GPS SVN62 triple-frequency signal combinations finds carrier-phase variations on the new L5. GPS World 2010, 21, 8–19. [Google Scholar]
- Li, X.; Hu, X.; Guo, R.; Tang, C.; Zhou, S.; Liu, S.; Chen, J. Orbit and Positioning Accuracy for New Generation Beidou Satellites during the Earth Eclipsing Period. J. Navig. 2018, 71, 1069–1087. [Google Scholar] [CrossRef]
- Saastamoinen, J. Atmospheric correction for the troposphere and stratosphere in radio ranging satellites. Bull. Géodésique 1972, 15, 247–251. [Google Scholar] [CrossRef]
- Davis, J.L.; Herring, T.A.; Shapiro, I.I.; Rogers, A.E.E.; Elgered, G. Geodesy by radio interferometry: Effects of atmospheric modeling errors on estimates of baseline length. Radio Sci. 1985, 20, 1593–1607. [Google Scholar] [CrossRef]
- Böhm, J.; Moeller, G.; Schindelegger, M.; Pain, G.; Weber, R. Development of an improved empirical model for slant delays in the troposphere (GPT2w). GPS Solut. 2015, 19, 433–441. [Google Scholar] [CrossRef] [Green Version]
- Böhm, J.; Werl, B.; Schuh, H. Troposphere mapping functions for GPS and very long baseline interferometry from European Centre for Medium-Range Weather Forecasts operational analysis data. J. Geophys. Res. Solid Earth 2006, 111, B02406. [Google Scholar] [CrossRef]
- Petit, G.; Luzum, B. (Eds.) IERS Conventions; Verlag des Bundesamts für Kartographie und Geodäsie: Frankfurt, Germany, 2010. [Google Scholar]
- Lyard, F.; Lefevre, F.; Letellier, T.; Francis, O. Modelling the global ocean tides: Modern insights from FES2004. Ocean Dyn. 2006, 56, 394–415. [Google Scholar] [CrossRef]
- Wu, J.T.; Wu, S.C.; Hajj, G.A.; Bertiger, W.I.; Lichten, S.M. Effects of antenna orientation on GPS carrier phase. Manuscr. Geod. 1993, 18, 91–98. [Google Scholar]





| Items | Models |
|---|---|
| Basic observables | Raw code and phase; cut-off elevation: 10.0 deg; sampling rate: 30.0 s |
| Weighting strategy | Code: 0.3 m; phase: 0.3 cm; the sigma increases with decreasing elevation angle using the function 1/sin(elevation) |
| Antenna phase center | IF/UC model for clock estimation: corrected with PCO and PCV values from igs14.atx file, GPS values adopted for BDS-3 at the receiver end; GFIF model for IFCB estimation: corrected with PCO and PCV values from either igs14.atx or igsR3.atx file |
| Attitude model | Modeled [19] |
| Tropospheric delay | Hydrostatic part according to the formula of [20] as given by [21] with surface pressure computed with GPT2w model [22]; Wet part estimated as random walk process with VMF1 (Vienna Mapping Function 1) [23]; Horizontal gradient model not considered |
| Ionospheric delay | 1st order eliminated for IF/GFIF model and estimated for UC model, higher-order not considered |
| Tidal displacements | Solid Earth tides, ocean loading and pole tides according to IERS Conventions 2010 [24] with FES2004 ocean tide model [25] |
| Phase wind-up effect | Corrected [26] |
| Receiver clock | Estimated as white noise process |
| Satellite clock | Estimated as white noise process |
| Phase ambiguities | Estimated as constant each observation pass |
| Satellite orbit | Fixed to the post-processed solution from IGS ACs |
| Earth orientation parameters | Fixed to IERS (International Earth Rotation Service) Bulletin A |
| Estimator | Extended Kalman filter |
| Parameters | Initial State | Initial Variance | Process Noise |
|---|---|---|---|
| Satellite clock | Post-processed solution | 1 × 104 m2 | - |
| Receiver clock | SPP | 1 × 104 m2 | - |
| Station zenith wet delay | 0.0 m | 0.25 m2 | 3 × 10−8 × 30 m2 |
| Ambiguity | Aligned to code | 1 × 106 m2 | 0.0 m2 |
| Satellite IFCB | 0.0 m | 1 × 104 m2 | - |
| Receiver IFCB | 0.0 m | 1 × 104 m2 | - |
| Day\Model | A | B | C | D | E | F |
|---|---|---|---|---|---|---|
| April-01 | 0.087 | 0.087 | 0.082 | 0.084 | 0.085 | 0.085 |
| April-02 | 0.027 | 0.027 | 0.033 | 0.027 | 0.028 | 0.028 |
| April-03 | 0.025 | 0.025 | 0.028 | 0.025 | 0.027 | 0.026 |
| April-04 | 0.030 | 0.030 | 0.033 | 0.030 | 0.030 | 0.031 |
| April-05 | 0.025 | 0.025 | 0.027 | 0.025 | 0.028 | 0.027 |
| April-06 | 0.026 | 0.026 | 0.028 | 0.026 | 0.029 | 0.028 |
| April-07 | 0.029 | 0.029 | 0.028 | 0.029 | 0.031 | 0.032 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kuang, K.; Wang, J.; Han, H. Real-Time BDS-3 Clock Estimation with a Multi-Frequency Uncombined Model including New B1C/B2a Signals. Remote Sens. 2022, 14, 966. https://doi.org/10.3390/rs14040966
Kuang K, Wang J, Han H. Real-Time BDS-3 Clock Estimation with a Multi-Frequency Uncombined Model including New B1C/B2a Signals. Remote Sensing. 2022; 14(4):966. https://doi.org/10.3390/rs14040966
Chicago/Turabian StyleKuang, Kaifa, Jian Wang, and Houzeng Han. 2022. "Real-Time BDS-3 Clock Estimation with a Multi-Frequency Uncombined Model including New B1C/B2a Signals" Remote Sensing 14, no. 4: 966. https://doi.org/10.3390/rs14040966
APA StyleKuang, K., Wang, J., & Han, H. (2022). Real-Time BDS-3 Clock Estimation with a Multi-Frequency Uncombined Model including New B1C/B2a Signals. Remote Sensing, 14(4), 966. https://doi.org/10.3390/rs14040966







