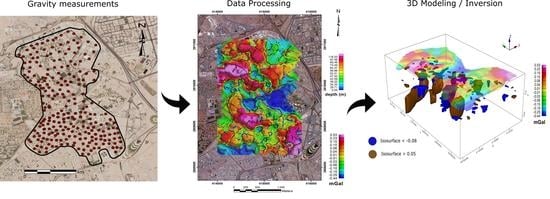
Gravity Survey on the Oil-Bearing Dammam Dome (Eastern Saudi Arabia) and Its Implications
Abstract
:1. Introduction
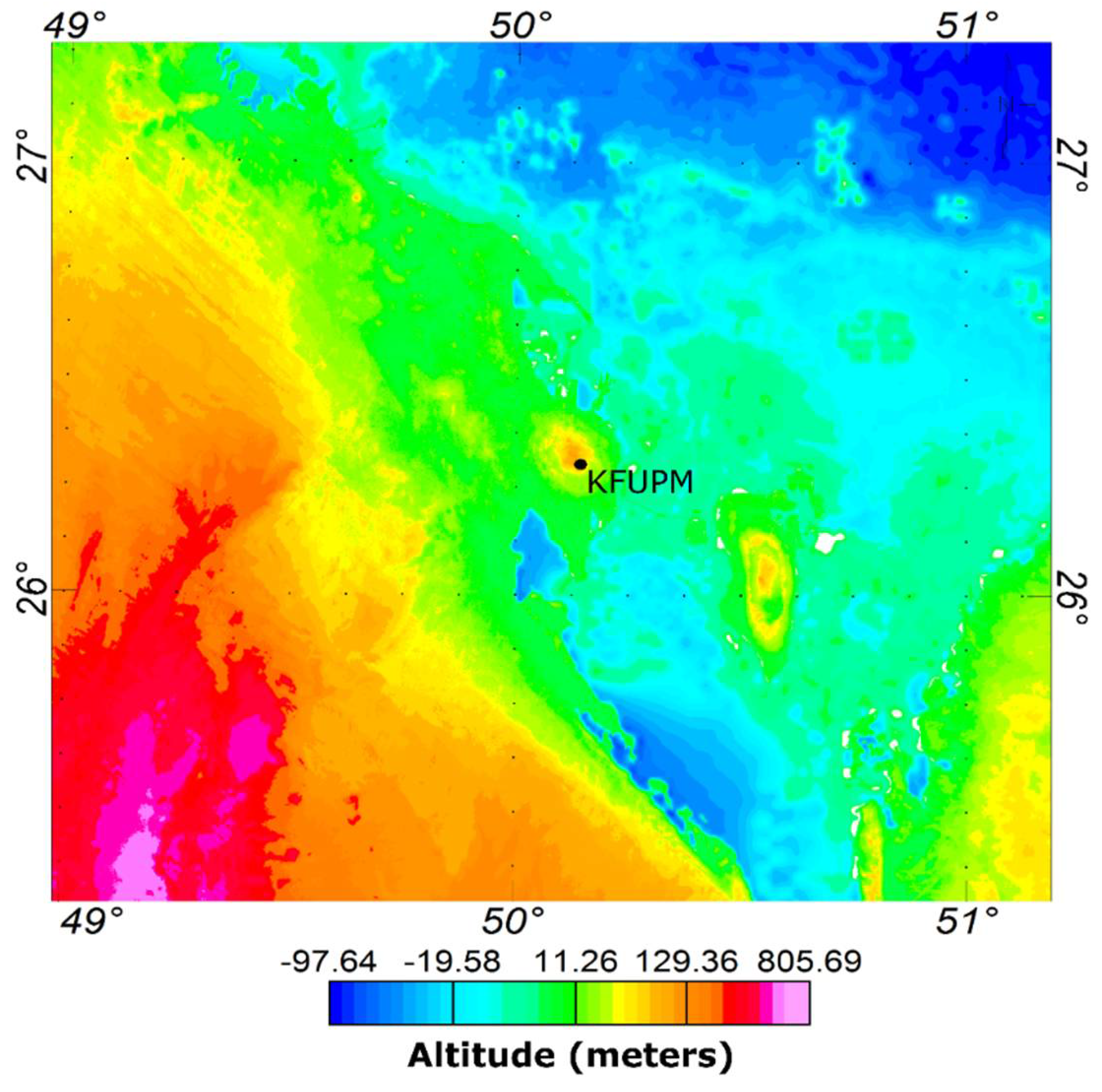
2. Geological Setting of the Dammam Dome
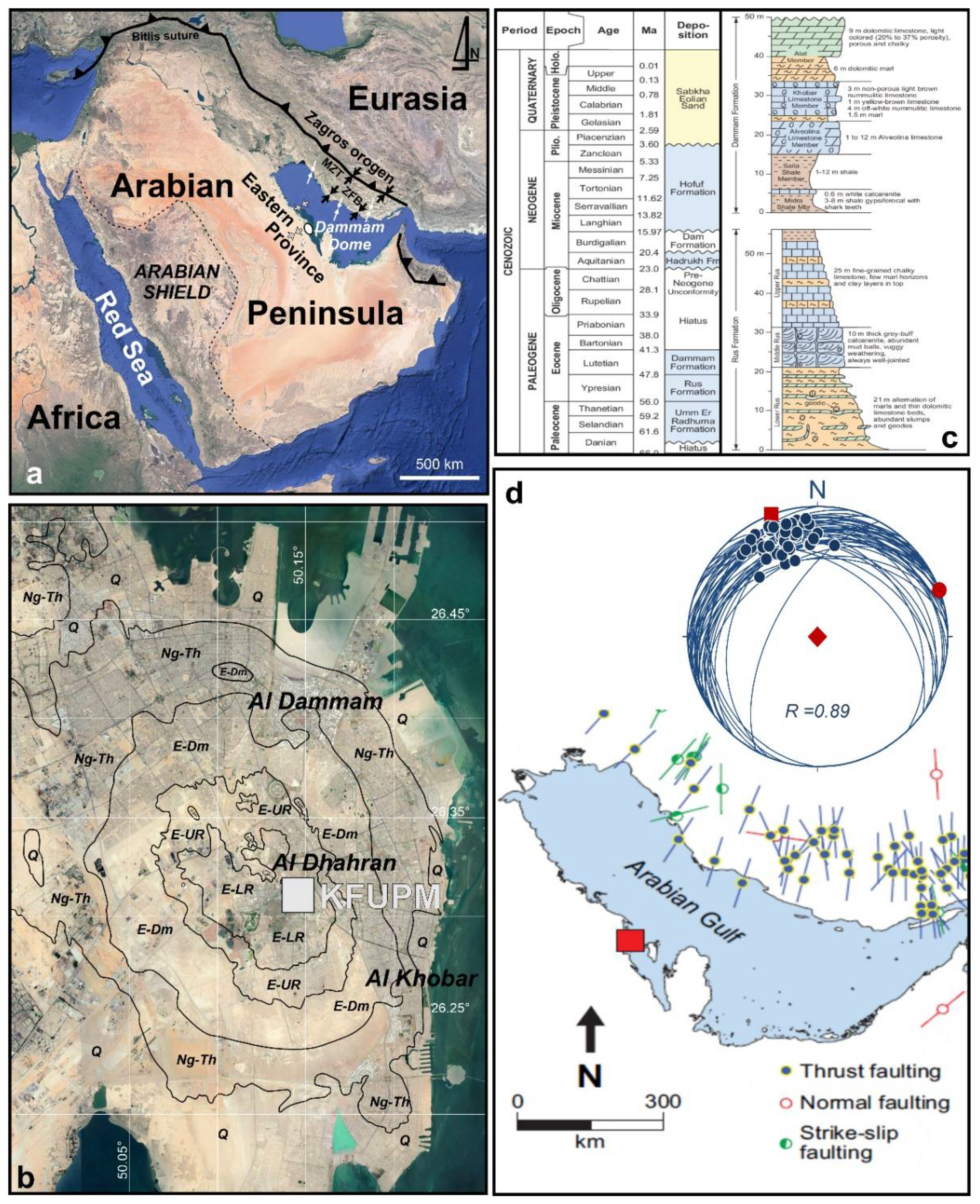
3. Material and Methods
3.1. Gravity Method
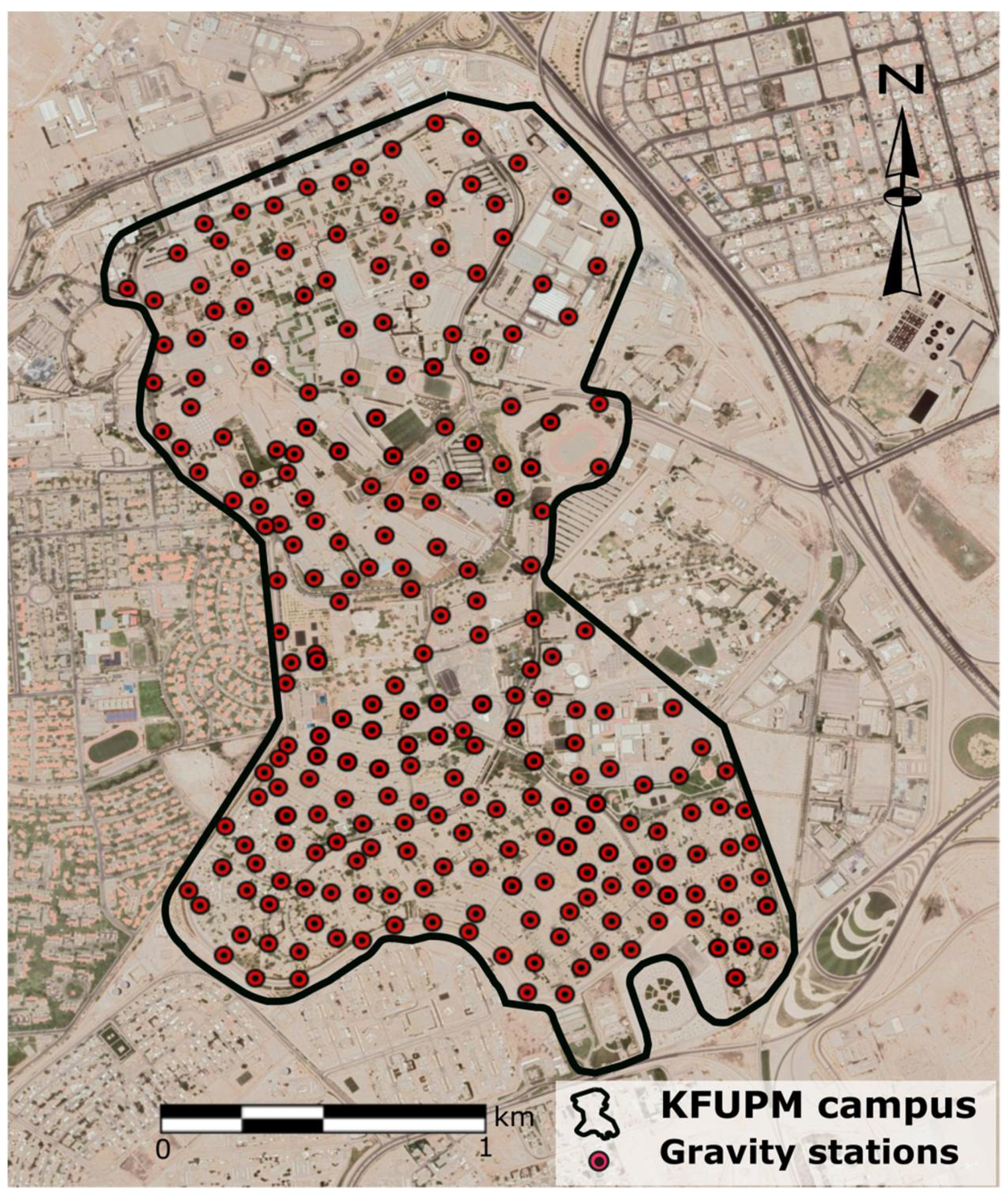
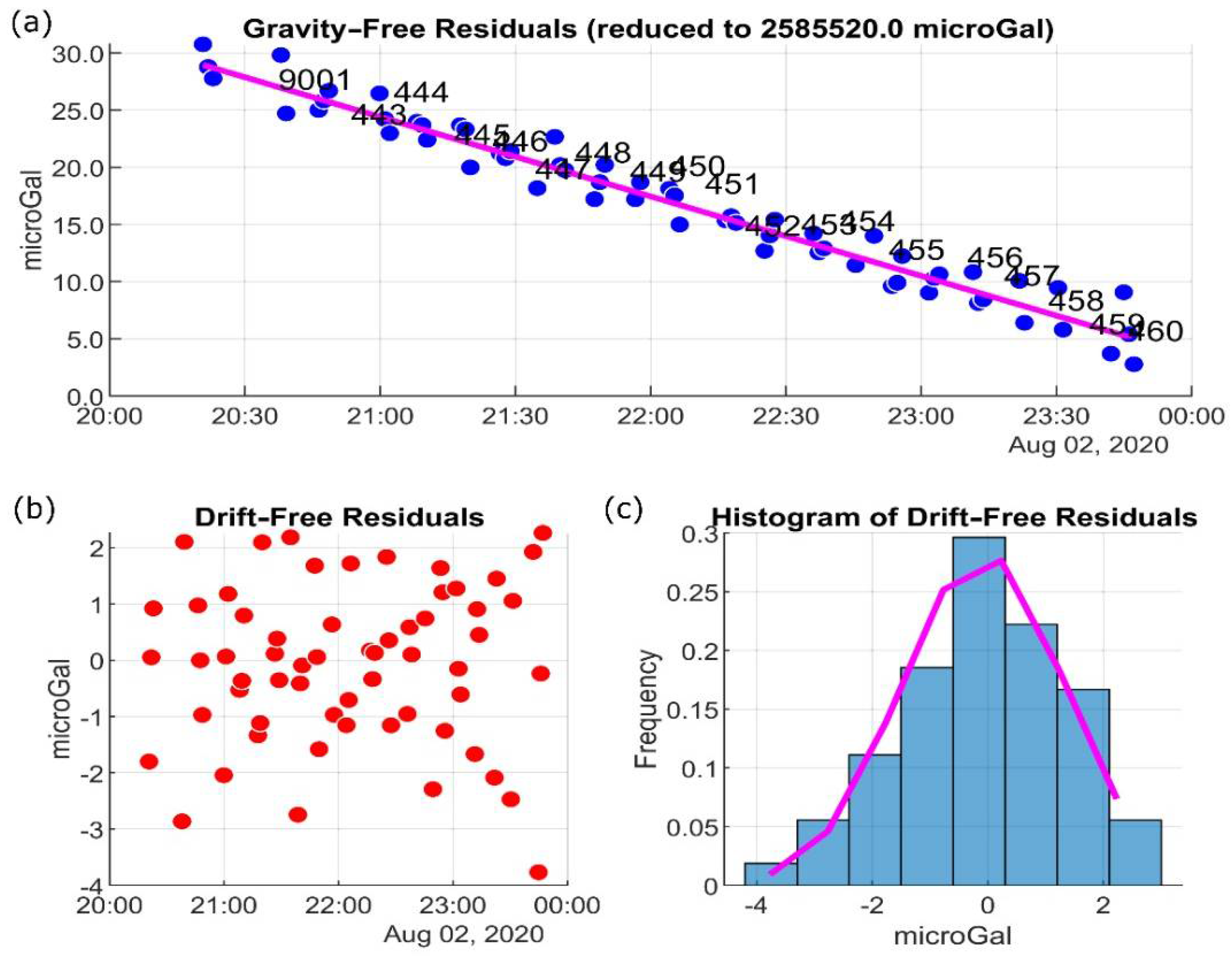
3.2. Gravity Survey—Data Acquisition and Quality Control
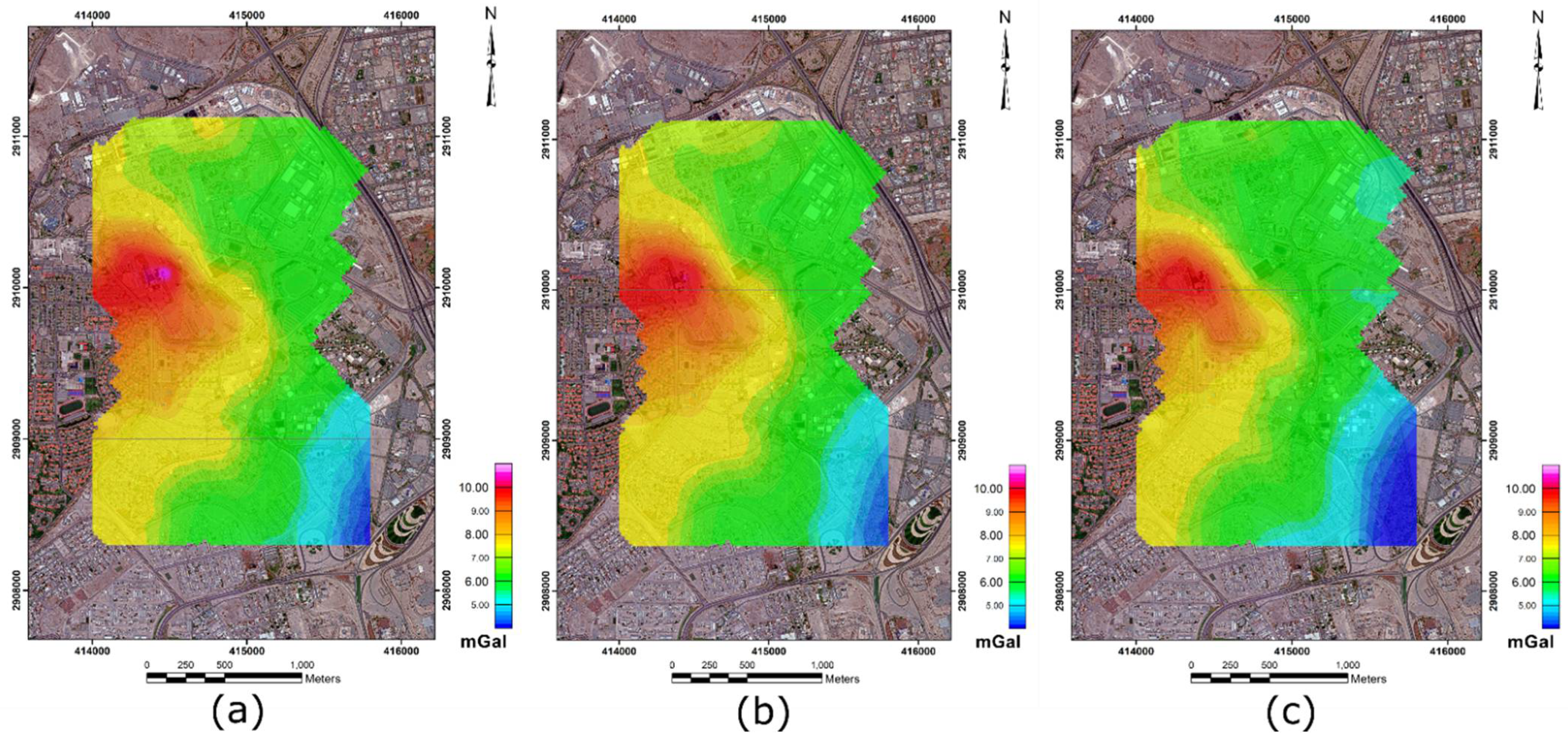
4. Results
4.1. Model and Data Processing Results
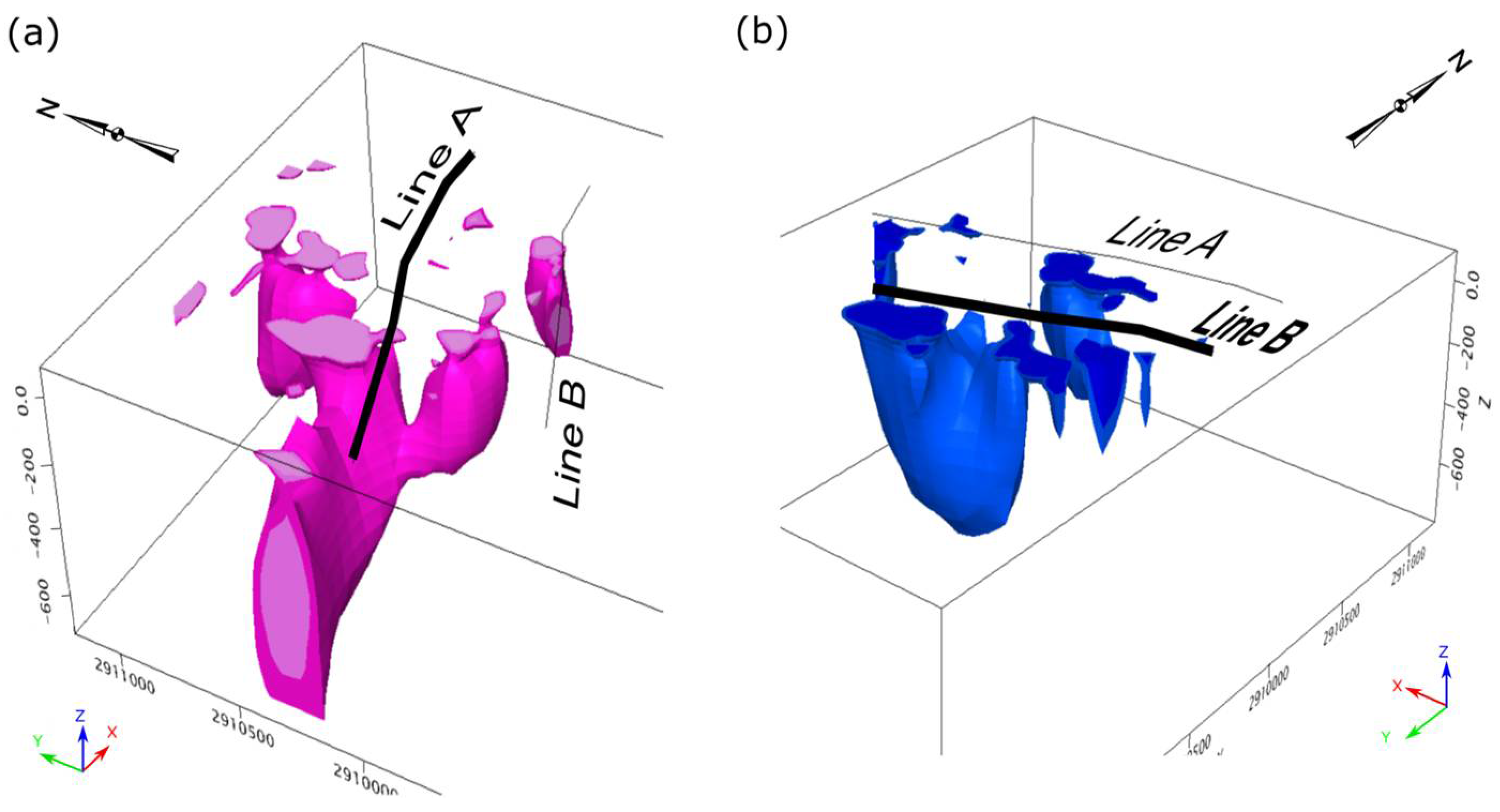
4.2. 3D Modeling
5. Interpretation-Discussion
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Onajide, E. Seismic Data Analysis Techniques in Hydrocarbon Exploration; Elsevier: Boston, MA, USA, 2014; ISBN 9780124200234. [Google Scholar]
- Magoon, L.B.; Dow, W.G. The Petroleum System—From Source to Trap; American Association of Petroleum Geologists: Tulsa, OK, USA, 1994; ISBN 9781629810928. [Google Scholar]
- Al-Fahmi, M.M. Fractures of the Dammam Dome Carbonate Outcrops: Their Characterization, Evolution and Implications to Deep Reservoirs. Master’s Thesis, University of Massachusetts Amherst, Amherst, MA, USA, 2012. [Google Scholar]
- Al-Fahmi, M.M.; Cooke, M.L.; Cole, J.C. Modeling of the Dammam outcrop fractures: Case study for fracture development in salt-cored structures. GeoArabia 2014, 19, 49–80. [Google Scholar] [CrossRef]
- Pollastro, R.M. Total Petroleum Systems of the Paleozoic and Jurassic, Greater Ghawar Uplift and Adjoining Provinces of Central Saudi Arabia and Northern Arabian-Persian Gulf; U.S. Geological Survey Bulletin; U.S. Geological Survey: Reston, VA, USA, 2003; Volume 52. [CrossRef]
- Tranos, M.D.; Osman, M.S. Rus detachment in Dammam Dome, Eastern Saudi Arabia: A new soft-sediment structure as a “sensitive stress tensor” for the Zagros collision. Geol. Mag. 2021, 1–13. [Google Scholar] [CrossRef]
- Hanif, M. Near-surface investigations of the Dammam Dome on the KFUPM campus using the gravity method. Master’s Thesis, King Fahd University of Petroleum & Minerals, Dhahran, Saudi Arabia, 2018. [Google Scholar]
- Tleel, J.W. Surface geology of Dammam Dome, Eastern province, Saudi Arabia. Am. Assoc. Pet. Geol. Bull. 1973, 57, 558–576. [Google Scholar] [CrossRef]
- Steineke, M.; Harriss, T.; Parsons, K.; Berg, E. Geographic Map of the Western Persian Gulf Quadrangle, Kingdom of Saudi Arabia; U.S. Geological Survey: Reston, VA USA, 1958.
- Powers, R.; Ramirez, L.; Redmond, C.; Elberg, E. Geology of the Arabian Peninsula—Sedimentary geology of Saudi Arabia. Geol. Surv. Prof. Pap. 1966, 560, 147. [Google Scholar]
- Çaġatay, M.N. Palygorskite in the eocene rocks of the Dammam Dome, Saudi Arabia. Clays Clay Miner. 1990, 38, 299–307. [Google Scholar] [CrossRef]
- Weijermars, R. Surface geology, lithostratigraphy and tertiary growth of the Dammam Dome, Saudi Arabia: A new field guide. GeoArabia 1999, 4, 199–226. [Google Scholar] [CrossRef]
- Hariri, M.M. Fractures system within Dammam Dome and its relationship to the doming process, Eastern Saudi Arabia. Arab. J. Geosci. 2014, 7, 4943–4956. [Google Scholar] [CrossRef]
- Al-Shuhail, A.A.; Hariri, M.M.; Makkawi, M.H. Using ground-penetrating radar to delineate fractures in the Rus Formation, Dammam Dome, Eastern Saudi Arabia. Int. Geol. Rev. 2004, 46, 91–96. [Google Scholar] [CrossRef]
- Hariri, M.M.; Abdullatif, O. Use of the GIS to delineate lineaments from landsat images, Dammam Dome, Eastern Saudi Arabia. In Proceedings of the XXII International Cartographic Conference (ICC2005), A Coruna, Spain, 11–16 July 2005; p. 7. [Google Scholar]
- Berger, Z. Satellite Hydrocarbon Exploration; Springer: Berlin/Heidelberg, Germany, 1994; ISBN 9783642785870. [Google Scholar]
- Frassy, F.; Maianti, P.; Marchesi, A.; Nodari, F.R.; Dalla Via, G.; De Paulis, R.; Biffi, P.G.; Gianinetto, M. Satellite remote sensing for hydrocarbon exploration in new venture areas. Int. Geosci. Remote Sens. Symp. 2015, 2015, 2884–2887. [Google Scholar] [CrossRef]
- Strack, K.M. Future directions of electromagnetic methodsfor hydrocarbon applications. Surv. Geophys. 2014, 35, 157–177. [Google Scholar] [CrossRef]
- Lyatsky, H. Gravity and magnetic geophysical methods in oil exploration. EP Mag. 2016, 52–54. [Google Scholar]
- Constable, S. Perspectives on Marine Electromagnetic Methods. Perspect. Earth Space Sci. 2020, 1, e2019CN000123. [Google Scholar] [CrossRef]
- Alsadi, H.N. Seismic Hydrocarbon Exploration: 2D and 3D Techniques; Springer: Berlin/Heidelberg, Germany, 2017; ISBN 9783319404356. [Google Scholar]
- Edgell, H.S. Basement tectonics of Saudi Arabia as related to oil field structures. In Basement Tectonics 9; Rickard, M.J., Ed.; Kluwer Academic Publishers: Dordrecht, The Netherlands, 1992; pp. 169–193. [Google Scholar]
- Stewart, S.A. Hormuz salt distribution and influence on structural style in NE Saudi Arabia. Pet. Geosci. 2017, 24, 143–158. [Google Scholar] [CrossRef]
- Stöcklin, J. Structural history and tectonics of Iran: A review. Am. Assoc. Pet. Geol. Bull. 1968, 52, 1229–1258. [Google Scholar] [CrossRef]
- Talbot, C.J. Extrusions of Hormuz salt in Iran. Geol. Soc. Spec. Publ. 1998, 143, 315–334. [Google Scholar] [CrossRef]
- Al-Husseini, M.I. Origin of the Arabian plate structures: Amar Collision and Najd Rift. GeoArabia 2000, 5, 527–542. [Google Scholar] [CrossRef]
- Konert, G.; Afifi, A.M.; Al-Hajri, S.A.; Droste, H.J. Paleozoic stratigraphy and hydrocarbon habitat of the Arabian plate. GeoArabia 2001, 6, 407–442. [Google Scholar] [CrossRef]
- Aftabi, P.; Roustaie, M.; Ian Alsop, G.; Talbot, C.J. InSAR mapping and modelling of an active iranian salt extrusion. J. Geol. Soc. 2010, 167, 155–170. [Google Scholar] [CrossRef]
- Khodabakhshnezhad, A.; Arian, M. Salt tectonics in the Southern Iran. Int. J. Geosci. 2016, 07, 367–377. [Google Scholar] [CrossRef] [Green Version]
- Hassanpour, J.; Yassaghi, A.; Muñoz, J.A.; Jahani, S. Salt tectonics in a double salt-source layer setting (Eastern Persian Gulf, Iran): Insights from interpretation of seismic profiles and sequential cross-section restoration. Basin Res. 2020, 33, 159–185. [Google Scholar] [CrossRef]
- Milton, D.I. Geology of the Arabian Peninsula, Kuwait; USGS Numbered Series; United States Government Printing Office: Washington, DC, USA, 1967; Volume 14. [CrossRef]
- Tanoli, S.K.; Al-Bloushi, A.J. Depositional history of the eocene Dammam formation in Kuwait. In Proceedings of the Society of Petroleum Engineers—SPE Kuwait Oil and Gas Show and Conference 2017, Kuwait City, Kuwait, 15–18 October 2017; pp. 1–14. [Google Scholar] [CrossRef]
- Al-Saad, H.; Ibrahim, M.I. Stratigraphy, micropaleontology, and paleoecology of the Miocene Dam Formation, Qatar. GeoArabia 2002, 7, 9–28. [Google Scholar] [CrossRef]
- Dewey, J.F.; Pitman, W.C.; Ryan, W.B.F.; Bonnin, J. Plate tectonics and the evolution of the alpine system. Bull. Geol. Soc. Am. 1973, 84, 3137–3180. [Google Scholar] [CrossRef]
- Dercourt, J.; Zonenshain, L.P.; Ricou, L.E.; Kazmin, V.G.; Le Pichon, X.; Knipper, A.L.; Grandjacquet, C.; Sbortshikov, I.M.; Geyssant, J.; Lepvrier, C.; et al. Geological evolution of the tethys belt from the atlantic to the pamirs since the LIAS. Tectonophysics 1986, 123, 241–315. [Google Scholar] [CrossRef]
- Stampfli, G.M.; Borel, G.D. A plate tectonic model for the paleozoic and mesozoic constrained by dynamic plate boundaries and restored synthetic oceanic isochrons. Earth Planet. Sci. Lett. 2002, 196, 17–33. [Google Scholar] [CrossRef]
- Lacombe, O.; Mouthereau, F.; Kargar, S.; Meyer, B. Late cenozoic and modern stress fields in the western Fars (Iran): Implications for the tectonic and kinematic evolution of central Zagros. Tectonics 2006, 25. [Google Scholar] [CrossRef] [Green Version]
- Ameen, M.S.; Hailwood, E.A. A new technology for the characterization of microfractured reservoirs (test case: Unayzah reservoir, Wudayhi field, Saudi Arabia). Am. Assoc. Pet. Geol. Bull. 2008, 92, 31–52. [Google Scholar] [CrossRef]
- Ameen, M.S. Fracture and in-situ stress patterns and impact on performance in the Khuff structural prospects, eastern offshore Saudi Arabia. Mar. Pet. Geol. 2014, 50, 166–184. [Google Scholar] [CrossRef]
- Heidbach, O.; Rajabi, M.; Cui, X.; Fuchs, K.; Müller, B.; Reinecker, J.; Reiter, K.; Tingay, M.; Wenzel, F.; Xie, F.; et al. The World Stress Map database release 2016: Crustal stress pattern across scales. Tectonophysics 2018, 744, 484–498. [Google Scholar] [CrossRef]
- Alfaraj, M.N.; Nebrija, E.L.; Ferguson, M.D. The challenge of interpreting 3-D seismic in Shaybah Field, Saudi Arabia. GeoArabia 1998, 3, 209–226. [Google Scholar] [CrossRef]
- Hinze, W.J.; Von Frese, R.R.B.; Saad, A.H. Gravity and Magnetic Exploration: Principles, Practices, and Applications; Cambridge University Press: Cambridge, UK, 2010; ISBN 9780511843129. [Google Scholar]
- Fairhead, J.D. Advances in Gravity and Magnetic Processing and Interpretation; EAGE Publications: Houten, The Netherlands, 2016; ISBN 9462821771. [Google Scholar]
- Blakely, R. Potential Theory in Gravity & Magnetic Applications; Cambridge University Press: Cambridge, UK, 1995. [Google Scholar] [CrossRef]
- Coates, R. The Defining Series: Geophysics. Oilfield Review. 2016. Available online: https://www.slb.com/-/media/files/oilfield-review/defining-geophysics.ashx (accessed on 10 December 2021).
- Hábel, B.; Janák, J.; Papčo, J.; Val’ko, M. Impact of environmental phenomena on continuous relative gravity measurements performed in urban area. Stud. Geophys. Geod. 2020, 64, 330–348. [Google Scholar] [CrossRef]
- Van Camp, M.; Vauterin, P. Tsoft: Graphical and interactive software for the analysis of time series and Earth tides. Comput. Geosci. 2005, 31, 631–640. [Google Scholar] [CrossRef]
- Dilalos, S.; Alexopoulos, J.D.; Tsatsaris, A. Calculation of building correction for urban gravity surveys. A case study of Athens metropolis (Greece). J. Appl. Geophys. 2018, 159, 540–552. [Google Scholar] [CrossRef]
- Moritz, H. Geodetic reference system 1980. Bull. Géodésique 1980, 54, 395–405. [Google Scholar] [CrossRef]
- Tozer, B.; Sandwell, D.T.; Smith, W.H.F.; Olson, C.; Beale, J.R.; Wessel, P. Global bathymetry and topography at 15 arc sec: SRTM15+. Earth Space Sci. 2019, 6, 1847–1864. [Google Scholar] [CrossRef]
- Cella, F. GTeC-A versatile MATLAB® tool for a detailed computation of the terrain correction and Bouguer gravity anomalies. Comput. Geosci. 2015, 84, 72–85. [Google Scholar] [CrossRef]
- Zahorec, P.; Marušiak, I.; Mikuška, J.; Pašteka, R.; Papčo, J. Numerical calculation of terrain correction within the Bouguer anomaly evaluation (program Toposk). In Understanding the Bouguer Anomaly: A Gravimetry Puzzle; Pašteka, R., Mikuška, J., Meurers, B., Eds.; Elsevier: Amsterdam, The Netherlands, 2017; pp. 79–92. ISBN 9780128129142. [Google Scholar]
- Holzrichter, N.; Szwillus, W.; Götze, H.J. An adaptive topography correction method of gravity field and gradient measurements by polyhedral bodies. Geophys. J. Int. 2019, 218, 1057–1070. [Google Scholar] [CrossRef]
- Telford, W.M.; Geldart, L.P.; Sheriff, R.E. Applied Geophysics, 2nd ed.; Cambridge University Press: Cambridge, UK, 1990; ISBN 0521326931. [Google Scholar]
- Cordell, L.; Grauch, V.J.S. Mapping basement magnetitation zones from aeromagnetic data in the San Juan Basin, New Mexico. In The Utility of Regional Gravity and Magnetic Anomaly Maps; Hinze, W.J., Ed.; Society of Exploration Geophysicists: Houston, TX, USA, 1985; pp. 246–247. [Google Scholar]
- Phillips, J.D.; Hansen, R.O.; Blakely, R.J. The use of curvature in potential-field interpretation. Explor. Geophys. 2007, 38, 111–119. [Google Scholar] [CrossRef]
- Cordell, L.; McCafferty, A.E. A terracing operator for physical property mapping with potential field data. Geophysics 1989, 54, 621–634. [Google Scholar] [CrossRef]
- Miller, H.G.; Singh, V. Potential field tilt: A new concept for location of potential field resources. J. Appl. Geophys. 1994, 32, 213–217. [Google Scholar] [CrossRef]
- Verduzco, B.; Fairhead, J.D.; Green, C.M.; MacKenzie, C. New insights into magnetic derivatives for structural mapping. Lead. Edge 2004, 23, 116–119. [Google Scholar] [CrossRef]
- Salem, A.; Williams, S.; Fairhead, D.; Smith, R.; Ravat, D. Interpretation of magnetic data using tilt-angle derivatives. Geophysics 2008, 73, L1–L10. [Google Scholar] [CrossRef]
- Elllis, R.G.; de Wet, B.; Macleod, I.N. Inversion of magnetic data from remanent and induced sources. ASEG Ext. Abstr. 2012, 2012, 1–4. [Google Scholar] [CrossRef] [Green Version]
- Tranos, M.D. Slip preference analysis of faulting driven by strike-slip Andersonian stress regimes: An alternative explanation of the Rhodope metamorphic core complex (northern Greece). J. Geol. Soc. 2016, 174, 129–141. [Google Scholar] [CrossRef]


| Tested Algorithms (Linear Regression) | Slope | Intercept | R (Correlation Coefficient) | RMS Error |
|---|---|---|---|---|
| Gravity and Terrain Correction vs. TriTop | 1.0138 | 0.20264 | 0.9920512 | 0.0258723 |
| Gravity and Terrain Correction vs. Toposk | 0.97503 | 0.16008 | 0.9987044 | 0.0038686 |
| Toposk vs. TriTop | 1.0398 | 0.03643 | 0.9933058 | 0.0218021 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chavanidis, K.; Stampolidis, A.; Kirmizakis, P.; Tranos, M.; Fedi, M.; Pasteka, R.; Al-Ramadan, K.; Kaka, S.; Tsokas, G.N.; Soupios, P. Gravity Survey on the Oil-Bearing Dammam Dome (Eastern Saudi Arabia) and Its Implications. Remote Sens. 2022, 14, 735. https://doi.org/10.3390/rs14030735
Chavanidis K, Stampolidis A, Kirmizakis P, Tranos M, Fedi M, Pasteka R, Al-Ramadan K, Kaka S, Tsokas GN, Soupios P. Gravity Survey on the Oil-Bearing Dammam Dome (Eastern Saudi Arabia) and Its Implications. Remote Sensing. 2022; 14(3):735. https://doi.org/10.3390/rs14030735
Chicago/Turabian StyleChavanidis, Konstantinos, Alexandros Stampolidis, Panagiotis Kirmizakis, Markos Tranos, Maurizio Fedi, Roman Pasteka, Khalid Al-Ramadan, SanLinn Kaka, Grigorios N. Tsokas, and Pantelis Soupios. 2022. "Gravity Survey on the Oil-Bearing Dammam Dome (Eastern Saudi Arabia) and Its Implications" Remote Sensing 14, no. 3: 735. https://doi.org/10.3390/rs14030735
APA StyleChavanidis, K., Stampolidis, A., Kirmizakis, P., Tranos, M., Fedi, M., Pasteka, R., Al-Ramadan, K., Kaka, S., Tsokas, G. N., & Soupios, P. (2022). Gravity Survey on the Oil-Bearing Dammam Dome (Eastern Saudi Arabia) and Its Implications. Remote Sensing, 14(3), 735. https://doi.org/10.3390/rs14030735