A Contrast Minimization Approach to Remove Sun Glint in Landsat 8 Imagery
Abstract
:1. Introduction
2. Materials and Methods
2.1. Data Sources and Processing
2.1.1. The Operational Land Imager
2.1.2. OLI Level-1 to Level-2 Conversion
2.1.3. Areas of Interest
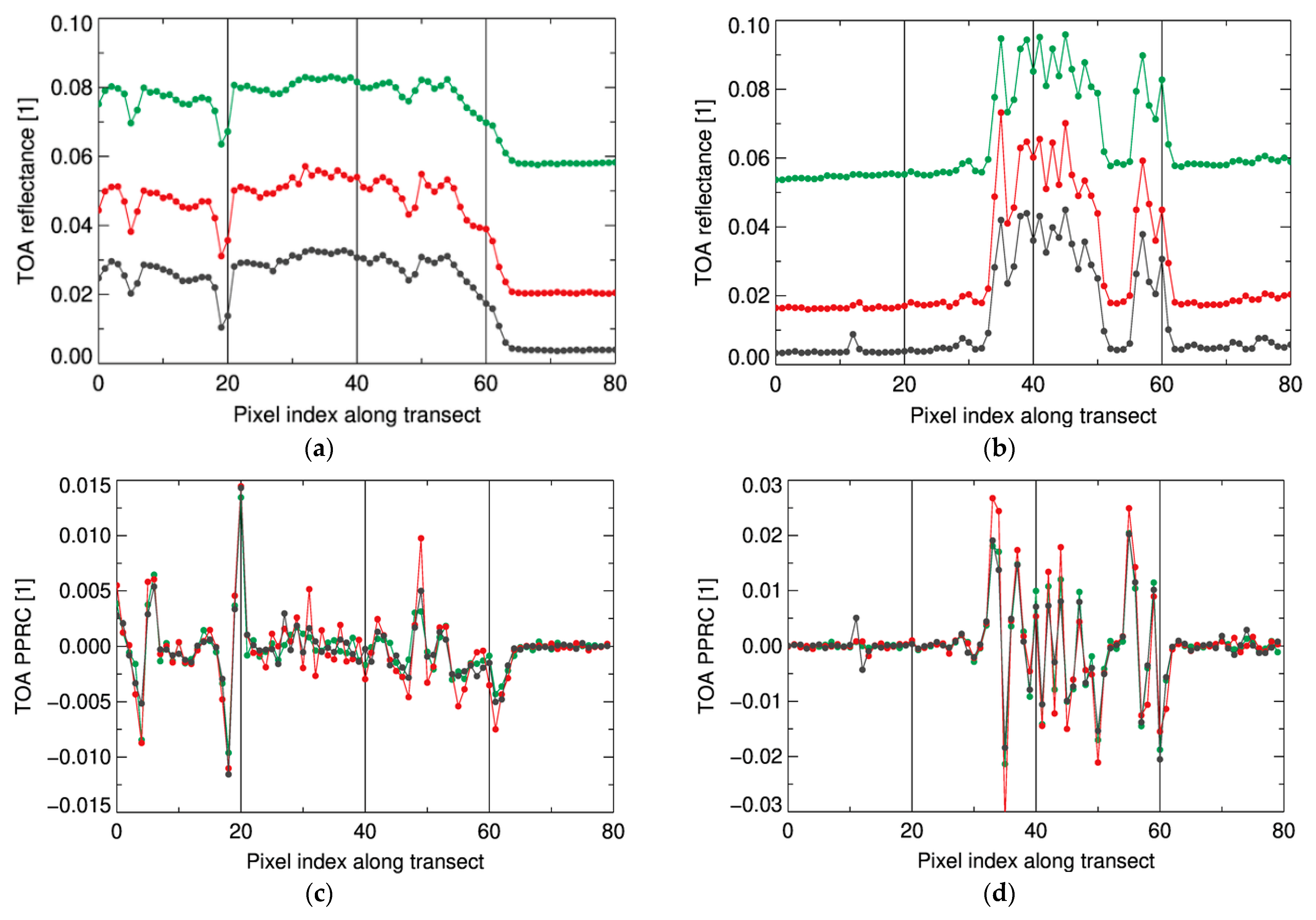
2.2. Morphological Aspects of Sun Glint
2.3. A Sun Glint Mask Derived from the Local Reflectance Contrast in the SWIR
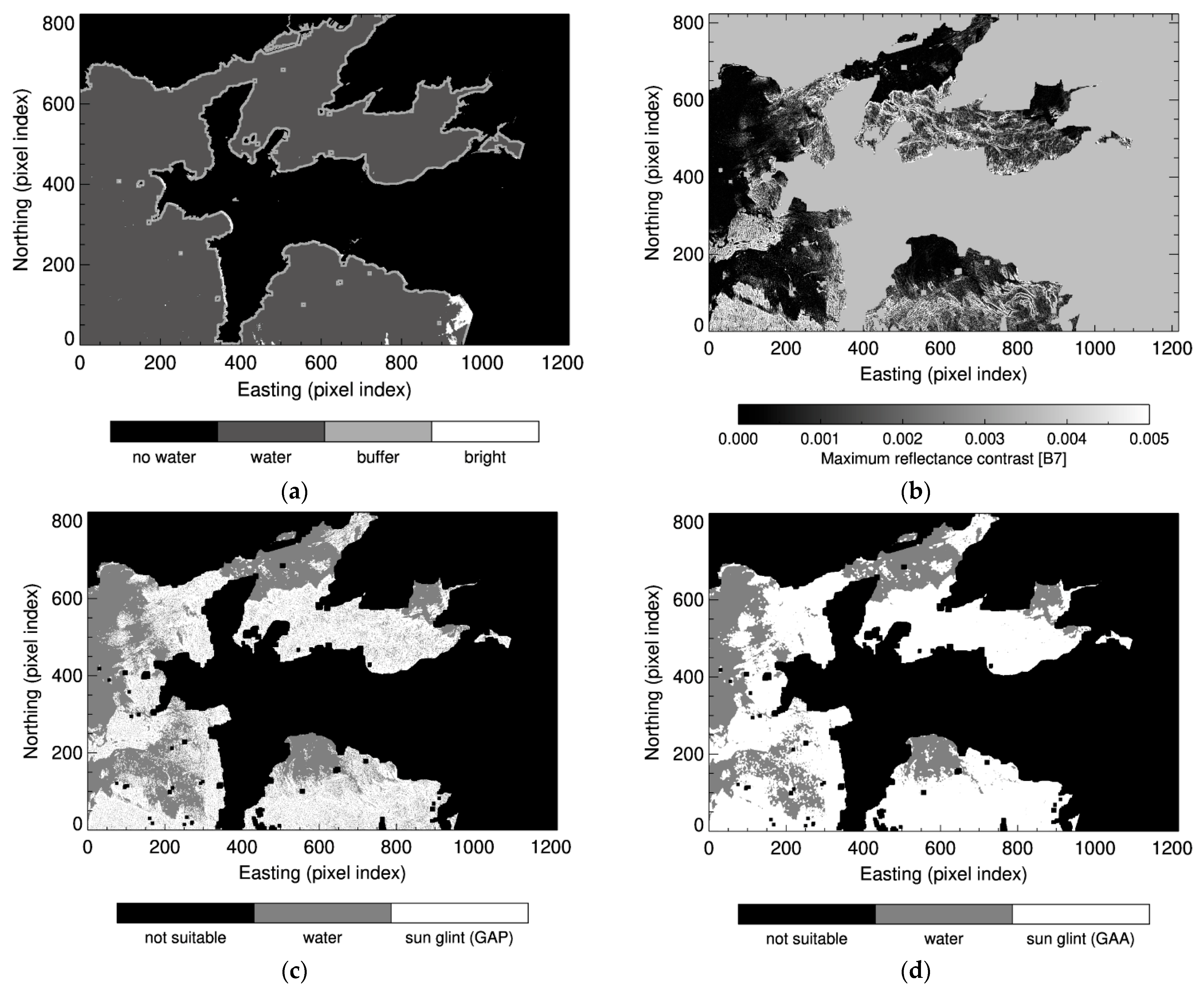
2.3.1. Identifying Sun Glint Affected Pixels and Areas
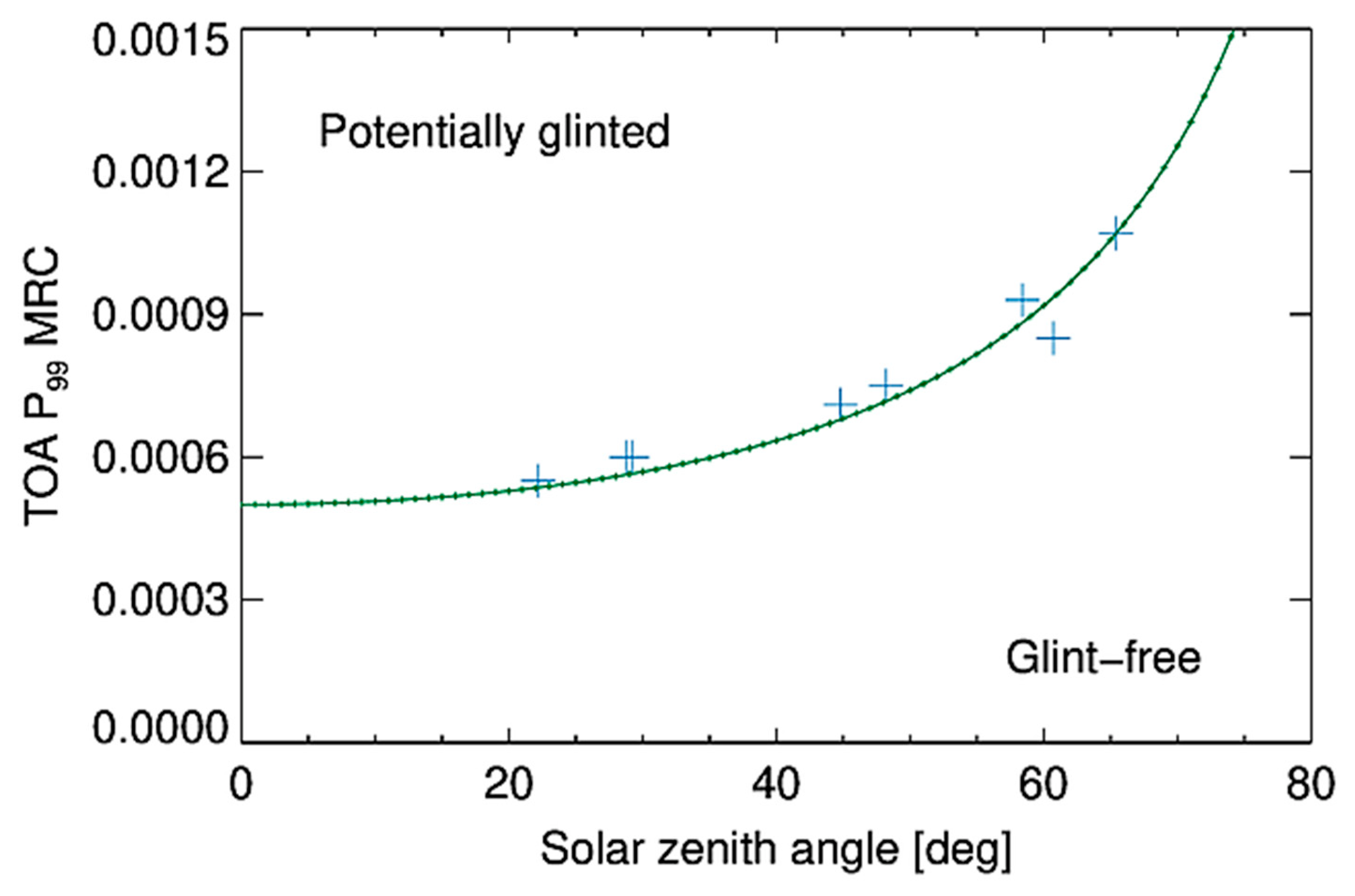
2.3.2. Determining the Model Parameters for the Sun Glint Mask
- Eight low cloud cover OLI scenes were preselected, encompassing an otherwise wide range of environmental conditions.
- Within each scene, one cloud-free area of negligible glint occurrence was determined from visual inspection.
- For each such cloud and glint-free area, the 99th percentile of MRC in channel B7 was determined.
- –
- A potentially glinted pixel at position (i, j) is considered glint affected if at least four further pixels within a window centered at (i, j) are also potentially glinted, hence, .
- –
- A pixel (whether glint affected or not) at position (i, j) is considered part of a GAA if at least one glint affected pixel is located within a window centered at (i, j), hence, .
2.4. Contrast-Based Estimation of the Sun Glint at TOA
2.5. Sun Glint Based Estimation of the Spectral Atmospheric Transmittance
3. Results
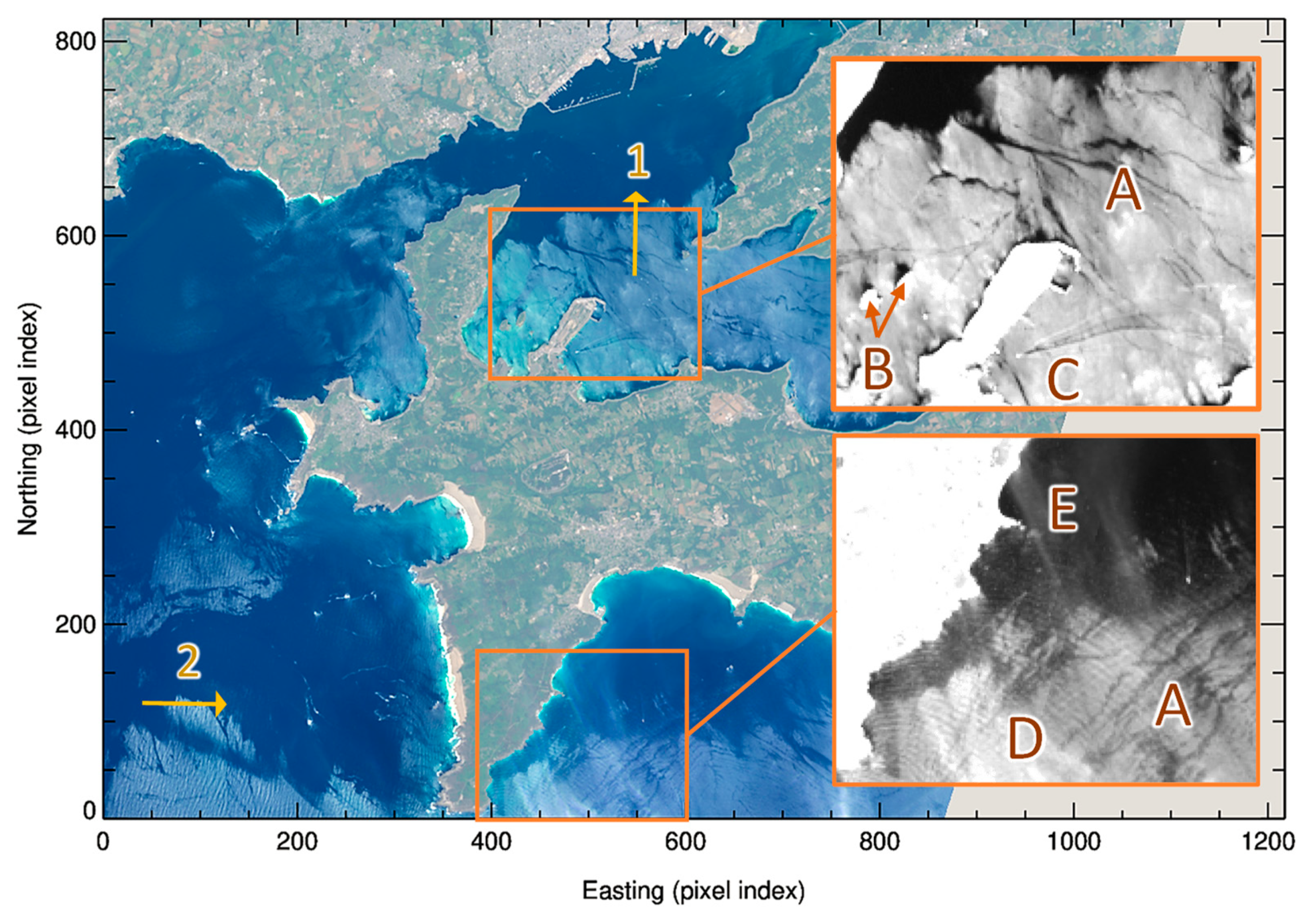
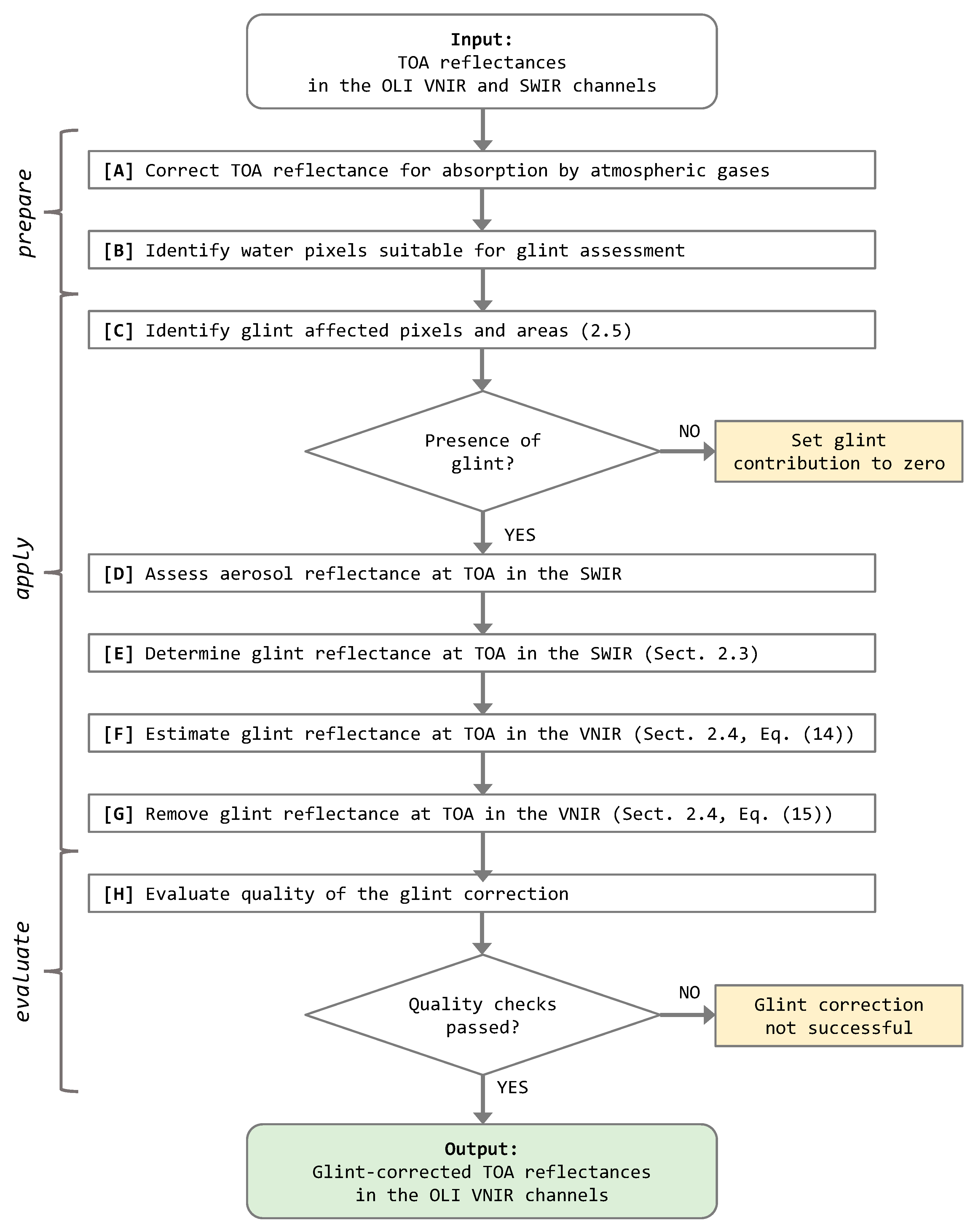
3.1. Overview of the GRCM Implementation
3.2. Preparing GRCM
3.2.1. [A] Correcting the TOA Reflectance for Absorption by Atmospheric Gases
3.2.2. [B] Identifying Water Pixels Suitable for Glint Assessment
3.3. Applying GRCM
3.3.1. [C] Identifying Glint Affected Pixels and Areas
3.3.2. [D] Assessing the Aerosol Reflectance at TOA in the SWIR
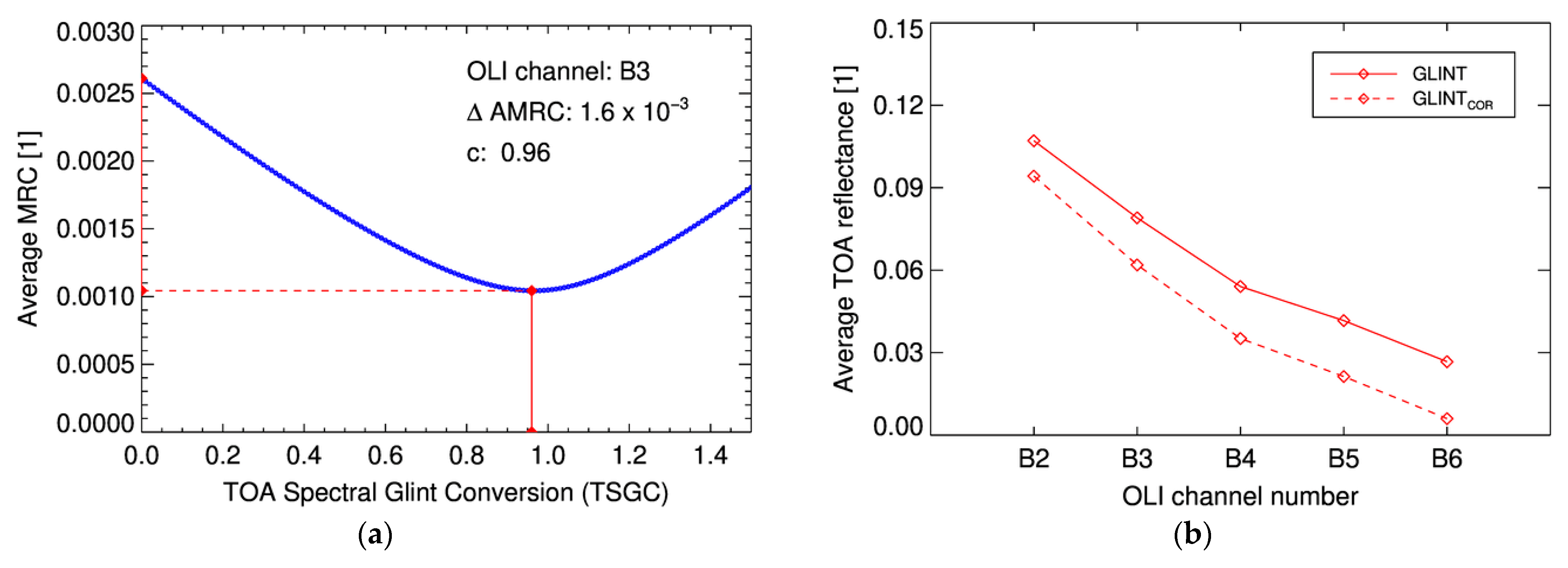
3.3.3. [E] Determining the Glint Reflectance at TOA in the SWIR
3.3.4. [F] Estimating the Glint Reflectance at TOA in the VNIR
3.3.5. [G] Removing the Glint Reflectance at TOA in the VNIR
3.4. Evaluating GRCM
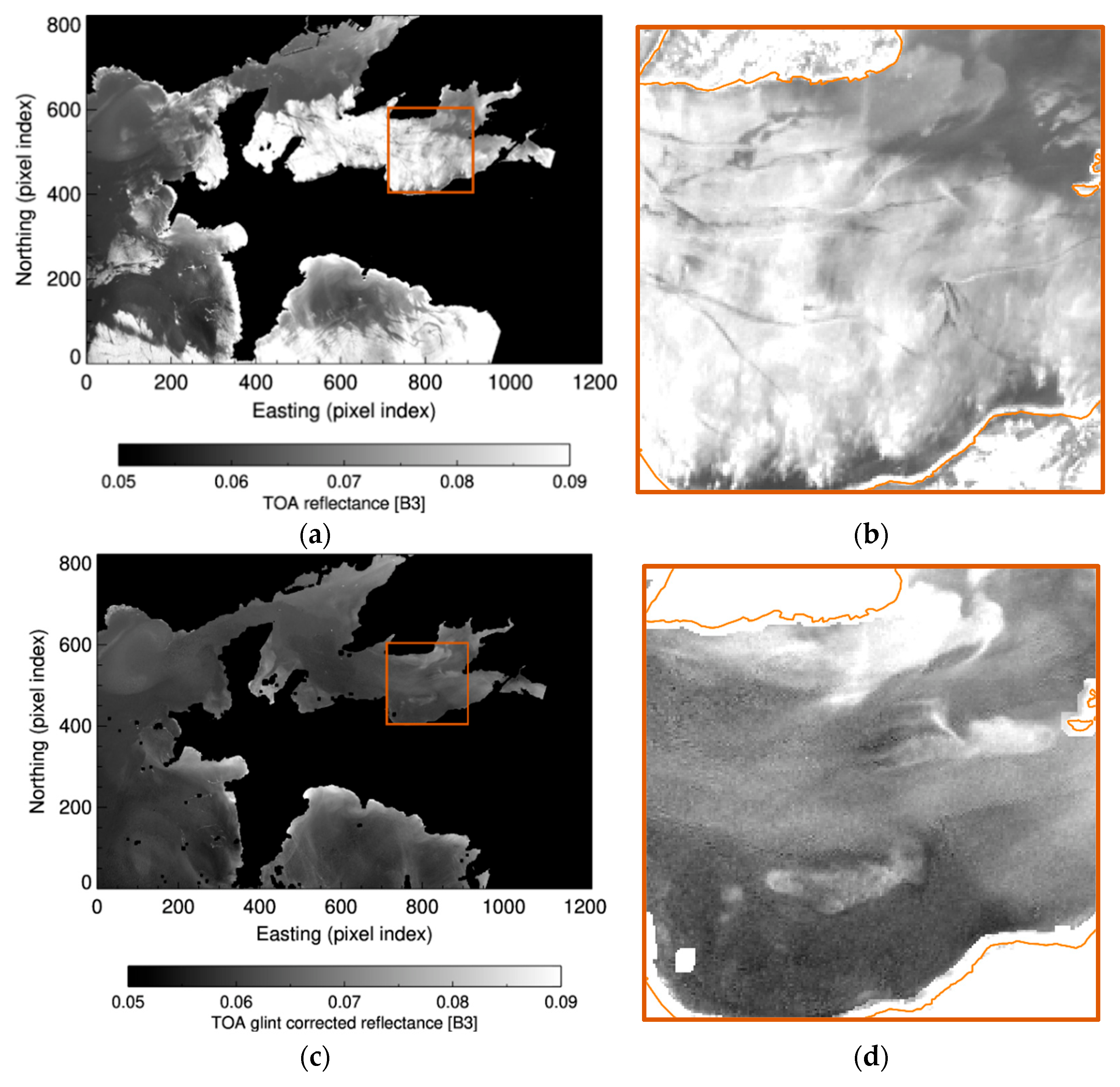
3.4.1. [H-1] Qualitative Evaluation
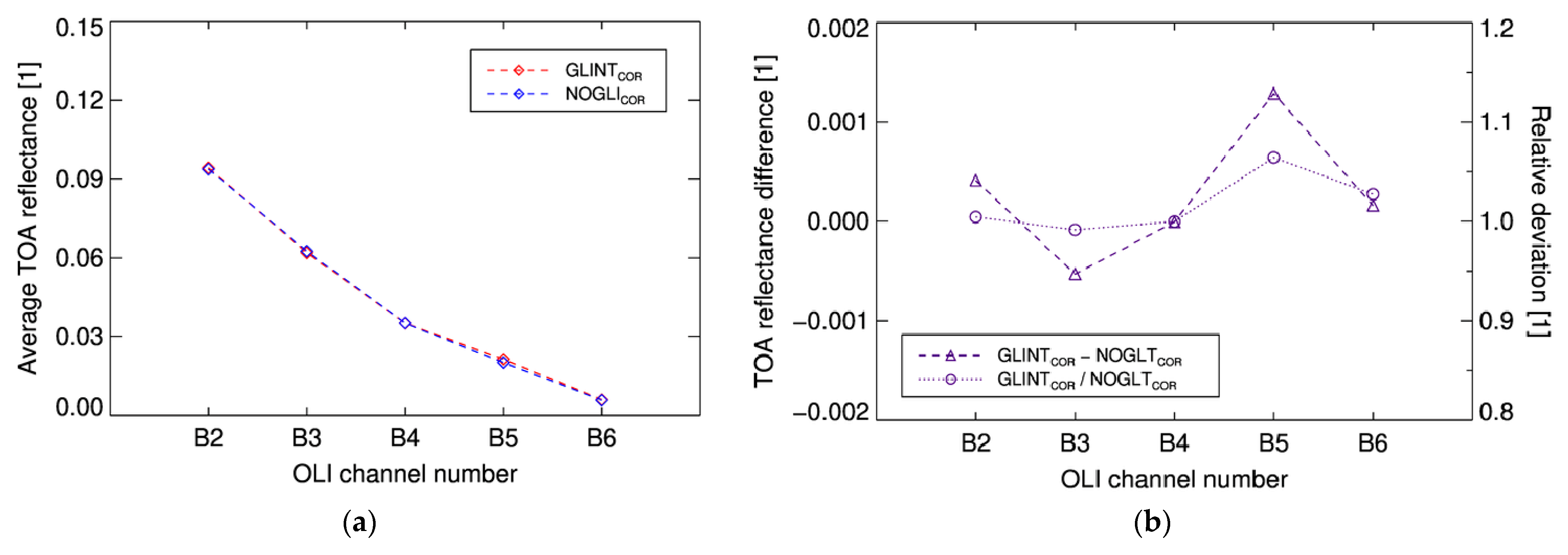
3.4.2. [H-2] Quantitative Evaluation
4. Discussion
4.1. Requirements on the Observing Imager
- The imager must be able to resolve morphological fine structures typical of sun glint, requiring a spatial resolution of ≤ca. 50 m.
- The imager must dispose of at least one channel in the SWIR, preferably at wavelengths to avoid sub-surface contributions.
- All applied spectral channels need to provide approximately identical representations of the observed water surface in terms of observation time, spatial resolution, and image registration.
4.2. Practical Application
4.3. Additional Aspects
4.4. Can Sun Glint Contribute to Atmospheric Correction?
5. Conclusions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| Acronym/Subscript | Explanation |
| ACIX | Atmospheric Correction Intercomparison Exercise |
| AER | Aerosol |
| AERONET | Aerosol Robotic Network |
| AMRC | Average maximum reflectance contrast |
| AOD | Aerosol optical depth |
| AR | Aerosol-Rayleigh (coupling term) |
| AOI | Area of interest |
| BRDF | Bidirectional reflectance distribution function |
| BGT | Bright (pixel) |
| BRS | Brest AOI (France) |
| BUF | Buffer |
| CLD | Cloud |
| COR | (Glint) Corrected |
| ERA5 | ECMWF Reanalysis 5th Generation |
| GAA | Glint affected area |
| GAP | Glint affected pixel |
| GRCM | Contrast Removal through Contrast Minimization |
| HFA | Haifa Bay AOI (Israel) |
| L1TP | Level 1 Terrain Precision |
| LCE | Lake Constance East AOI (Germany, Austria, Switzerland) |
| LPY | Lake Puma Yumco AOI (China) |
| MERIS | Medium Resolution Imaging Spectrometer |
| MODIS | Moderate Resolution Imaging Spectroradiometer |
| MRC | Maximum reflectance contrast |
| MSI | Multi-Spectral Imager |
| MSK | Mask (image) |
| MSL | Mean sea level |
| NDWI | Normalized difference water index |
| NIR | Near infrared, wavelength range ca. 0.7–1.5 µm |
| OLCI | Ocean, Land and Cloud Imager |
| OLI | Operational Land Imager |
| PGP | Potentially glinted pixel |
| POLYMER | Polynomial based algorithm applied to MERIS |
| PPRC | Pixel-to-pixel reflectance contrast |
| RAY | Rayleigh |
| SHD | (Cloud) Shadow |
| SMAC | Simplified Method for Atmospheric Correction |
| SUG | Sun glint |
| SWIR | Shortwave infrared, wavelength range ca. 1.5–2.5 µm |
| THR | Threshold |
| TOA | Top-of-atmosphere |
| TSGC | TOA Spectral Glint Conversion |
| VIS | Visible, wavelength range ca. 0.4–0.7 µm |
| VNIR | Visible and near infrared, wavelength range ca. 0.4–1.5 µm |
| WAT | Water |
| WCP | White caps |
Appendix A
Appendix A.1. Sample Scene HFA-5: Haifa Bay, 11 June 2022
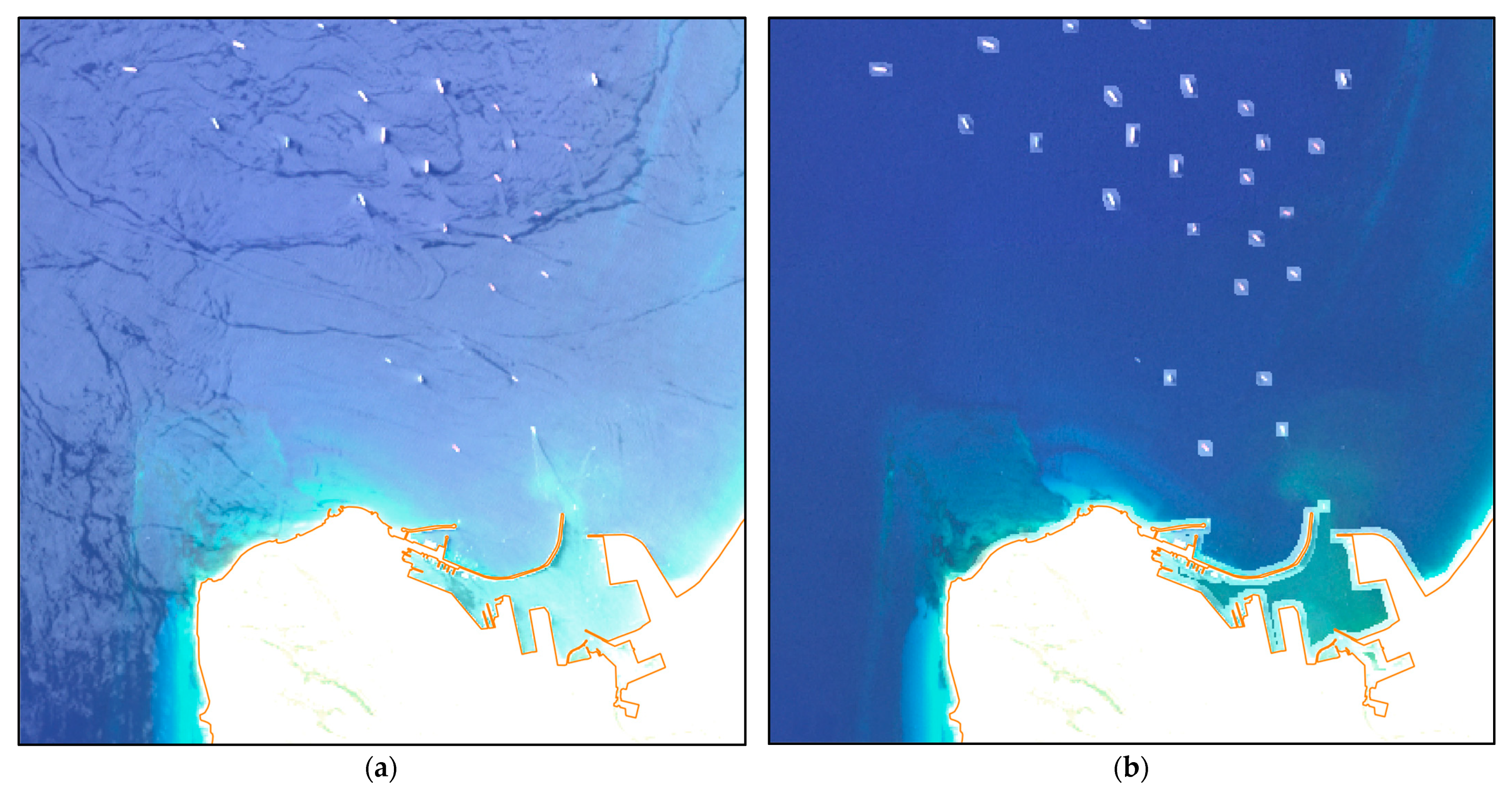

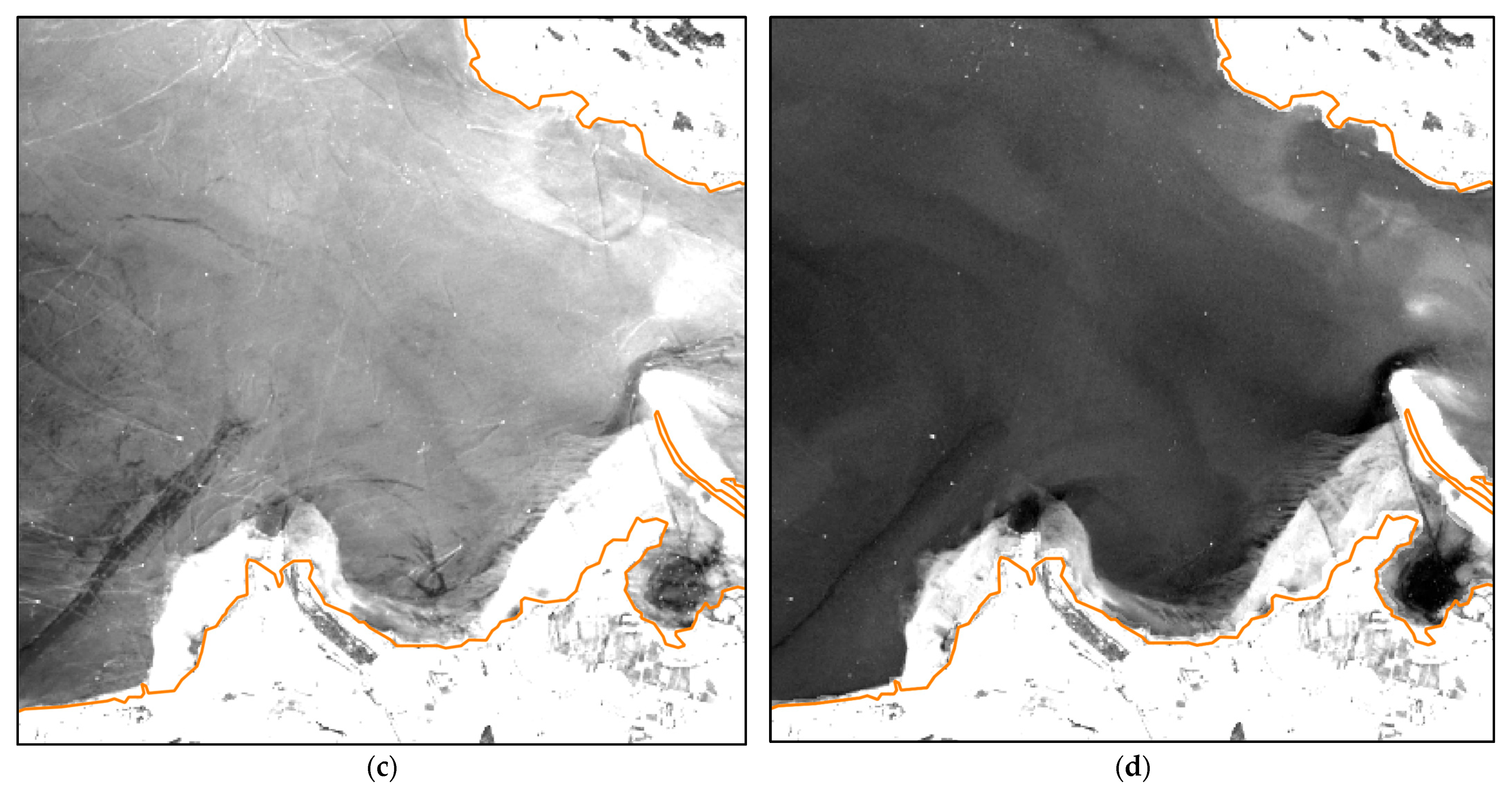
Appendix A.2. Sample Scene LCE-2: Lake Constance East, 1 June 2020

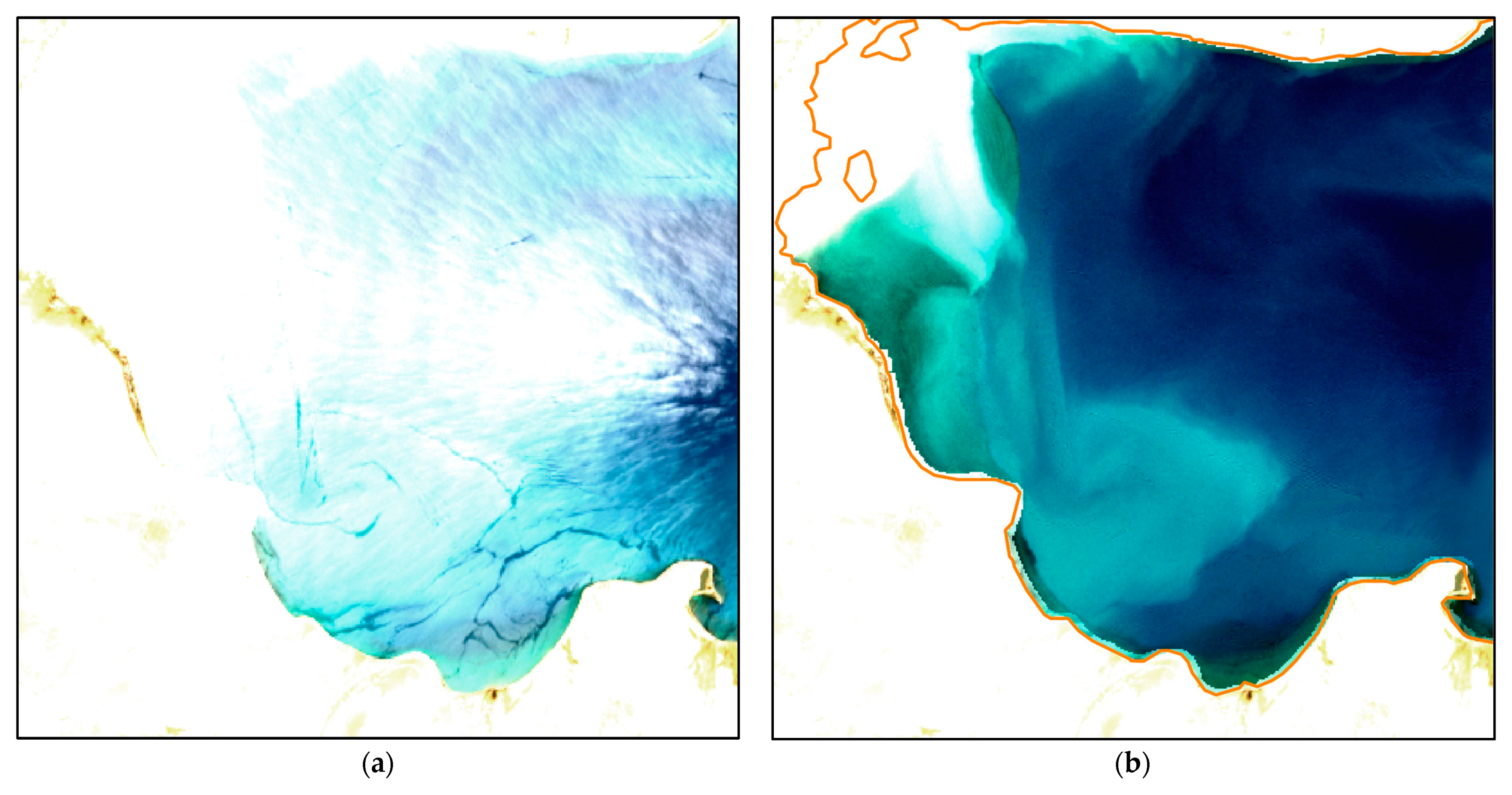
Appendix A.3. Sample Scene LPY-1: Lake Puma Yumco, 6 July 2018

References
- Sentinel-3 OLCI User Guide. Available online: https://sentinels.copernicus.eu/web/sentinel/user-guides/sentinel-3-olci (accessed on 20 June 2022).
- Cox, C.; Munk, W. Measurement of the Roughness of the Sea Surface from Photographs of the Sun’s Glitter. J. Opt. Soc. Am. 1954, 44, 838–850. [Google Scholar] [CrossRef]
- Duntley, S.Q. Measurements of the distribution of water wave slopes. J. Opt. Soc. Amer. 1954, 44, 574–575. [Google Scholar] [CrossRef]
- Hwang, P.A.; Shemdin, O.H. The dependence of sea surface slope on atmospheric stability and swell conditions. J. Geophys. Res. 1988, 93, 13903–13912. [Google Scholar] [CrossRef]
- Fukushima, H.; Suzuki, K.; Li, L.; Suzuki, N.; Murakami, H. Improvement of the ADEOS-II/GLI sun-glint algorithm using concomitant microwave scatterometer-derived wind data. Adv. Space Res. 2009, 43, 941–947. [Google Scholar] [CrossRef]
- Rascle, N.; Nouguier, F.; Chapron, B.; Mouche, A.; Ponte, A. Surface Roughness Changes by Finescale Current Gradients: Properties at Multiple Azimuth View Angles. J. Phys. Oceanogr. 2016, 46, 3681–3694. [Google Scholar] [CrossRef]
- Jackson, C. Internal Wave Detection Using the Moderate Resolution Imaging Spectroradiometer (MODIS). J. Geophys. Res. 2007, 112, C11012:1–C11012:13. [Google Scholar] [CrossRef]
- Askari, F. Multi-sensor remote sensing of eddy-induced upwelling in the southern coastal region of Sicily. Int. J. Remote Sens. 2001, 22, 2899–2910. [Google Scholar] [CrossRef]
- Alpers, W.; Hühnerfuss, H. The damping of ocean waves by surface films: A new look at an old problem. J. Geophys. Res. 1989, 94, 6251–6265. [Google Scholar] [CrossRef]
- Hennings, I.; Matthews, J.; Metzner, M. Sun glitter radiance and radar cross-section modulations of the seabed. J. Geophys. Res. 1994, 99, 16303–16326. [Google Scholar] [CrossRef]
- Fresnel Equations. Available online: https://en.wikipedia.org/wiki/Fresnel_equations (accessed on 20 June 2022).
- Quan, X.; Fry, E. Empirical equation for the index of refraction of seawater. Appl. Opt. 1995, 34, 3477–3480. [Google Scholar] [CrossRef]
- Harmel, T.; Chami, M.; Tormos, T.; Reynaud, N.; Danis, P.-A. Sunglint correction of the Multi-Spectral Instrument (MSI)-SENTINEL-2 imagery over inland and sea waters from SWIR bands. Remote Sens. Environ. 2018, 204, 308–321. [Google Scholar] [CrossRef]
- Liu, Y.; Yan, X.-H.; Liu, W.T.; Hwang, P.A. The Probability Density Function of Ocean Surface Slopes and Its Effects on Radar Backscatter. J. Phys. Oceanogr. 1997, 27, 782–797. [Google Scholar] [CrossRef]
- Kay, S.; Hedley, J.D.; Lavender, S. Sun Glint Correction of High and Low Spatial Resolution Images of Aquatic Scenes: A Review of Methods for Visible and Near-Infrared Wavelengths. Remote Sens. 2009, 1, 697–730. [Google Scholar] [CrossRef]
- Emberton, S.; Chittka, L.; Cavallaro, A.; Wang, M. Sensor Capability and Atmospheric Correction in Ocean Colour Remote Sensing. Remote Sens. 2016, 8, 1. [Google Scholar] [CrossRef]
- Wang, M.; Bailey, S. Correction of sun glint contamination on the SeaWiFS ocean and atmosphere products. Appl. Opt. 2001, 40, 4790–4798. [Google Scholar] [CrossRef]
- Hochberg, E.J.; Andrefouet, S.; Tyler, M.R. Sea surface correction of high spatial resolution Ikonos images to improve bottom mapping in near-shore environments. IEEE Trans. Geosci. Remote Sens. 2003, 41, 1724–1729. [Google Scholar] [CrossRef]
- Hedley, J.D.; Harborne, A.R.; Mumby, P.J. Technical note: Simple and robust removal of sun glint for mapping shallow—Water benthos. Int. J. Remote Sens. 2005, 26, 2107–2112. [Google Scholar] [CrossRef]
- Hu, C. An empirical approach to derive MODIS ocean color patterns under severe sun glint. Geophys. Res. Lett. 2011, 38, L01603. [Google Scholar] [CrossRef]
- Steinmetz, F.; Deschamps, P.; Ramon, D. Atmospheric correction in presence of sun glint: Application to MERIS. Opt. Express 2011, 19, 9783–9800. [Google Scholar] [CrossRef]
- Shi, W.; Wang, M. An assessment of the black ocean pixel assumption for MODIS SWIR bands. Remote Sens. Environ. 2009, 113, 1587–1597. [Google Scholar] [CrossRef]
- Zorrilla, N.A.; Vantrepotte, V.; Ngoc, D.D.; Huybrechts, N.; Gardel, A. Automated SWIR based empirical sun glint correction of Landsat 8-OLI data over coastal turbid water. Opt. Express 2019, 27, A294–A318. [Google Scholar] [CrossRef]
- Landsat 8 Data Users Handbook, Version 5.0, November 2019, Document Number LSDS-1574. Available online: https://www.usgs.gov/landsat-missions/landsat-8-data-users-handbook (accessed on 15 June 2022).
- Pahlevan, N.; Smith, B.; Alikas, K.; Anstee, J.; Barbosa, C.; Binding, C.; Bresciani, M.; Cremella, B.; Giardino, C.; Gurlin, D.; et al. Simultaneous retrieval of selected optical water quality indicators from Landsat-8, Sentinel-2, and Sentinel-3. Remote Sens. Environ. 2022, 270, 112860. [Google Scholar] [CrossRef]
- Franz, B.A.; Bailey, S.W.; Kuring, N.; Werdell, P.J. Ocean color measurements with the Operational Land Imager on Landsat-8: Implementation and evaluation in SeaDAS. J. Appl. Remote Sens. 2015, 9, 096070. [Google Scholar] [CrossRef]
- Landsat Collection 2. Available online: https://www.usgs.gov/landsat-missions/landsat-collection-2 (accessed on 17 June 2022).
- Sinyuk, A.; Holben, B.N.; Eck, T.F.; Giles, D.M.; Slutsker, I.; Korkin, S.; Schafer, J.S.; Smirnov, A.; Sorokin, M.; Lyapustin, A. The AERONET Version 3 aerosol retrieval algorithm, associated uncertainties and comparisons to Version 2. Atmos. Meas. Tech. 2020, 13, 3375–3411. [Google Scholar] [CrossRef]
- Tomasi, C.; Vitale, V.; Petkov, B.; Lupi, A.; Cacciari, A. Improved algorithm for calculations of Rayleigh-scattering optical depth in standard atmospheres. Appl. Opt. 2005, 44, 3320–3341. [Google Scholar] [CrossRef] [PubMed]
- Dierssen, H.M. Hyperspectral Measurements, Parameterizations, and Atmospheric Correction of Whitecaps and Foam from Visible to Shortwave Infrared for Ocean Color Remote Sensing. Front. Earth Sci. 2019, 7, 14. [Google Scholar] [CrossRef]
- Philpot, W. Estimating Atmospheric Transmission and Surface Reflectance from a Glint-Contaminated Spectral Image. IEEE Trans. Geosci. Remote Sens. 2007, 45, 448–457. [Google Scholar] [CrossRef]
- Rahman, H.; Dedieu, G. SMAC: A simplified method for the atmospheric correction of satellite measurements in the solar spectrum. Int. J. Remote Sens. 1994, 15, 123–143. [Google Scholar] [CrossRef]
- SMAC Python Code for Atmospheric Correction. Available online: https://github.com/olivierhagolle/SMAC/tree/master/COEFS (accessed on 15 June 2022).
- Hersbach, H.; Bell, B.; Berrisford, P.; Biavati, G.; Horányi, A.; Muñoz Sabater, J.; Nicolas, J.; Peubey, C.; Radu, R.; Rozum, I.; et al. ERA5 Hourly Data on Single Levels from 1979 to Present. Copernicus Climate Change Service (C3S) Climate Data Store (CDS). 2018. Available online: https://cds.climate.copernicus.eu/cdsapp#!/dataset/reanalysis-era5-single-levels?tab=overview (accessed on 15 June 2022).
- Xu, H. Modification of normalised difference water index (NDWI) to enhance open water features in remotely sensed imagery. Int. J. Remote Sens. 2006, 27, 3025–3033. [Google Scholar] [CrossRef]
- Sang, B.; Schubert, J.; Kaiser, S.; Mogulsky, V.; Neumann, C.; Förster, K.P.; Hofer, S.; Stuffler, T.; Kaufmann, H.; Müller, A.; et al. The EnMAP hyperspectral imaging spectrometer: Instrument concept, calibration, and technologies. In Proceedings of the SPIE Imaging Spectrometry XIII, San Diego, CA, USA, 27 August 2008; Volume 7086, p. 708605. [Google Scholar] [CrossRef]
- MultiSpectral Instrument (MSI) Overview, Table 2: The Temporal Offset (in Seconds) between Selected Bands. Available online: https://sentinels.copernicus.eu/web/sentinel/technical-guides/sentinel-2-msi/msi-instrument (accessed on 23 June 2022).
- Pahlevan, N.; Mangin, A.; Balasubramanian, S.V.; Smith, B.; Alikas, K.; Arai, K.; Barbosa, C.; Bélanger, S.; Binding, C.; Bresciani, M.; et al. ACIX-Aqua: A global assessment of atmospheric correction methods for Landsat-8 and Sentinel-2 over lakes, rivers, and coastal waters. Remote Sens. Environ. 2021, 258, 112366. [Google Scholar] [CrossRef]
- AERONET Aerosol Optical Depth Data Display Interface, Site: Brest_MF. Available online: https://aeronet.gsfc.nasa.gov/cgi-bin/data_display_aod_v3?site=Brest_MF (accessed on 29 June 2022).
| Spectral Band | Spectral Range | Spatial Resolution | Signal-to-Noise Ratio |
|---|---|---|---|
| B1, Coastal/Aerosol | 0.435–0.451 µm | 30 m | 238 |
| B2, Blue | 0.452–0.512 µm | 30 m | 364 |
| B3, Green | 0.533–0.590 µm | 30 m | 302 |
| B4, Red | 0.636–0.673 µm | 30 m | 227 |
| B5, NIR | 0.851–0.879 µm | 30 m | 204 |
| B6, SWIR-1 | 1.566–1.651 µm | 30 m | 265 |
| B7, SWIR-2 | 2.107–2.294 µm | 30 m | 334 |
| B8, Pan | 0.503–0.676 µm | 15 m | 149 |
| B9, Cirrus | 1.363–1.384 µm | 30 m | 165 |
| AOI Designation | Geographical Extension | Elevation above MSL | Description | Remarks |
|---|---|---|---|---|
| Brest [BRS] | 48.170–48.386°N 4.700–4.214°W | 0 m | Estuary and coastal waters of varying degrees of turbidity, frequent occurrence of swell from the open Atlantic. | AERONET [28] station “Brest_MF” within AOI. |
| Haifa Bay [HFA] | 32.750–32.966°N 34.714–35.100°E | 0 m | Inner Haifa Bay strongly impacted by anthropogenic activities (harbor), oligotrophic conditions offshore. | AERONET [28] station “Technion_Hai-fa_IL” within AOI. |
| Lake Con-stance East [LCE] | 47.450–47.666°N 9.270–9.750°E | 395 m | Large (536 km2) and mostly oligotrophic lake in central Europe, intensively used for recreational purposes. | |
| Lake Puma Yumco [LPY] | 28.434–28.650°N 90.215–90.574°E | 5013 m | Large (280 km2) oligotrophic lake on the Qinghai-Tibet Plateau, significantly reduced Rayleigh optical depth. |
| Sample Scene ID | Area of Interest | Date | Landsat Product ID | WRS2 Path/Row |
|---|---|---|---|---|
| BRS-1 | Brest | 13 May 2019 | LC08_L1TP_203026_20190513_20200828_02_T1 | 203/026 |
| BRS-2 | Brest | 4 April 2020 | LC08_L1TP_204026_20200404_20200822_02_T1 | 204/026 |
| BRS-3 | Brest | 23 June 2020 | LC08_L1TP_204026_20200623_20200823_02_T1 | 204/026 |
| BRS-4 | Brest | 10 August 2020 | LC08_L1TP_204026_20200810_20200918_02_T1 | 204/026 |
| HFA-1 | Haifa Bay | 9 January 2022 | LC08_L1TP_175037_20220109_20220114_02_T1 | 175/037 |
| HFA-2 | Haifa Bay | 15 April 2022 | LC08_L1TP_175037_20220415_20220420_02_T1 | 175/037 |
| HFA-3 | Haifa Bay | 10 May 2022 | LC08_L1TP_174037_20220510_20220518_02_T1 | 174/037 |
| HFA-4 | Haifa Bay | 26 May 2022 | LC08_L1TP_174037_20220526_20220602_02_T1 | 174/037 |
| HFA-5 | Haifa Bay | 11 June 2022 | LC08_L1TP_174037_20220611_20220617_02_T1 | 174/037 |
| LCE-1 | Lake Constance East | 22 July 2021 | LC08_L1TP_194027_20210722_20210729_02_T1 | 194/027 |
| LCE-2 | Lake Constance East | 1 June 2020 | LC08_L1TP_194027_20200601_20200824_02_T1 | 194/027 |
| LCE-3 | Lake Constance East | 19 July 2020 | LC08_L1TP_194027_20200719_20200911_02_T1 | 194/027 |
| LCE-4 | Lake Constance East | 20 August 2020 | LC08_L1TP_194027_20200820_20200905_02_T1 | 194/027 |
| LPY-1 | Lake Puma Yumco | 6 July 2018 | LC08_L1TP_138040_20180706_20200831_02_T1 | 138/040 |
| LPY-2 | Lake Puma Yumco | 8 September 2018 | LC08_L1TP_138040_20180908_20200831_02_T1 | 138/040 |
| AOI-ID | p_srf [hPa] | GAA [%] | (B2) | (B3) | (B4) | (B5) | (B6) | ||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| BRS-1 | 1035 | 33.3 | 72 | 0.0044 | 0.00029 | 0.0003 | 0.71 | 0.92 | 0.98 | 1.00 | 1.04 |
| BRS-2 | 1017 | 45.6 | 100 | 0.0051 | 0.00109 | 0.0001 | 0.84 | 1.06 | 1.15 | 1.21 | 1.15 |
| BRS-3 | 1022 | 29.2 | 72 | 0.0031 | 0.00157 | 0.0005 | 0.72 | 0.96 | 1.06 | 1.14 | 1.16 |
| BRS-4 | 1014 | 36.9 | 78 | 0.0044 | 0.00039 | 0.0009 | 0.55 | 0.79 | 0.91 | 1.04 | 1.11 |
| HFA-1 | 1012 | 59.5 | 6 | 0.0030 | 0.00021 | 0.0002 | 0.55 | 0.76 | 0.83 | 0.91 | 0.90 |
| HFA-2 | 1015 | 30.7 | 100 | 0.0244 | 0.00091 | 0.0004 | 0.39 | 0.99 | 0.90 | 0.80 | 1.14 |
| HFA-3 | 1015 | 24.2 | 81 | 0.0054 | 0.00020 | 0.0004 | 0.61 | 0.87 | 0.89 | 0.89 | 0.95 |
| HFA-4 | 1016 | 22.0 | 99 | 0.0124 | 0.00037 | 0.0003 | 0.57 | 0.91 | 0.98 | 1.00 | 1.05 |
| HFA-5 | 1011 | 21.4 | 76 | 0.0080 | 0.00073 | 0.0006 | 0.85 | 1.02 | 1.11 | 1.17 | 1.12 |
| LCE-1 | 973 | 31.5 | 15 | 0.0031 | 0.00020 | 0.0008 | 0.46 | 0.65 | 0.78 | 0.96 | 1.09 |
| LCE-2 | 972 | 28.9 | 54 | 0.0008 | 0.00035 | 0.0008 | 0.75 | 0.97 | 1.04 | 1.09 | 1.10 |
| LCE-3 | 971 | 31.0 | 47 | 0.0006 | 0.00049 | 0.0029 | 0.74 | 0.99 | 1.08 | 1.15 | 1.16 |
| LCE-4 | 966 | 38.6 | 5 | 0.0007 | 0.00024 | 0.0018 | 0.68 | 0.93 | 1.05 | 1.11 | 1.12 |
| LPY-1 | 538 | 22.2 | 72 | 0.0054 | 0.00147 | 0.0027 | 1.09 | 1.19 | 1.25 | 1.25 | 1.13 |
| LPY-2 | 541 | 31.1 | 18 | 0.0003 | 0.00071 | 0.0055 | 1.09 | 1.17 | 1.22 | 1.22 | 1.10 |
| Average of 9 BRS and HFA open ocean sample scenes | 0.64 | 0.92 | 0.98 | 1.02 | 1.07 | ||||||
| Corresponding standard deviation | 0.15 | 0.10 | 0.11 | 0.14 | 0.09 | ||||||
| Average of 227 OLI scenes offshore French Guiana. Source: [23, Table 2] | 0.83 | 0.90 | 0.99 | 1.08 | 1.09 | ||||||
| Corresponding standard deviation | 0.15 | 0.14 | 0.07 | 0.13 | 0.11 | ||||||
| B2 | B3 | ||||
|---|---|---|---|---|---|
| 0.72 | 0.96 | 1.06 | 1.14 | 1.16 | |
| 1.27 | 1.25 | 1.23 | 1.21 | 1.13 | |
| 0.70 | 0.83 | 0.90 | 0.97 | 1.00 | |
| 0.43 | 0.21 | 0.12 | 0.04 | −0.01 | |
| ~0.22 | ~0.18 | ~0.15 | ~0.10 | ~0.07 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Fell, F. A Contrast Minimization Approach to Remove Sun Glint in Landsat 8 Imagery. Remote Sens. 2022, 14, 4643. https://doi.org/10.3390/rs14184643
Fell F. A Contrast Minimization Approach to Remove Sun Glint in Landsat 8 Imagery. Remote Sensing. 2022; 14(18):4643. https://doi.org/10.3390/rs14184643
Chicago/Turabian StyleFell, Frank. 2022. "A Contrast Minimization Approach to Remove Sun Glint in Landsat 8 Imagery" Remote Sensing 14, no. 18: 4643. https://doi.org/10.3390/rs14184643
APA StyleFell, F. (2022). A Contrast Minimization Approach to Remove Sun Glint in Landsat 8 Imagery. Remote Sensing, 14(18), 4643. https://doi.org/10.3390/rs14184643






