Orbit Predictions for Space Object Tracked by Ground-Based Optical and SLR Stations
Abstract
:1. Introduction
2. Sites Description
2.1. Optical Satellite Tracking Station
2.2. The Satellite Laser Ranging Stations
3. Precise Orbit Determination
4. Results and Discussions
4.1. Orbital Element of Space Object Descriptions
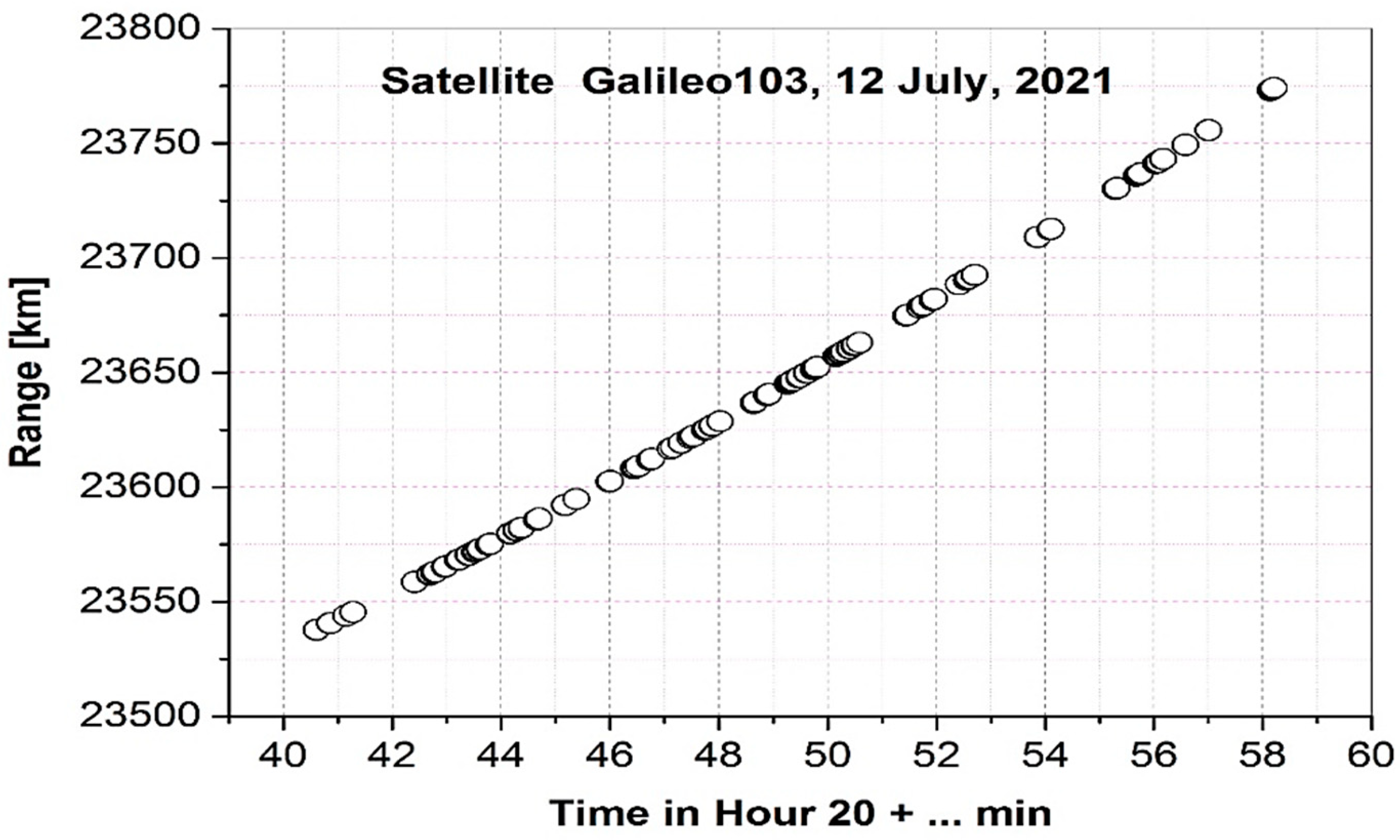
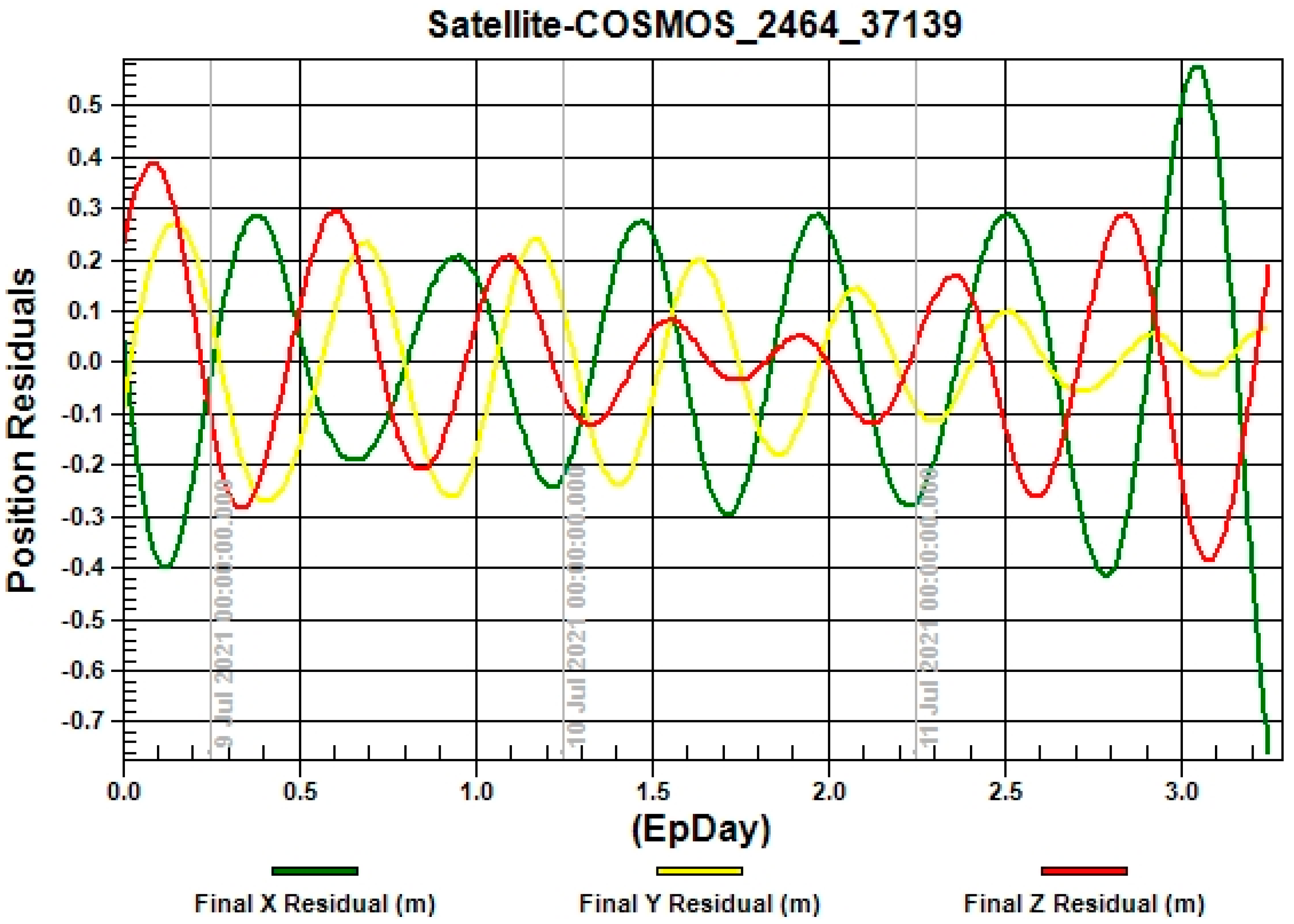
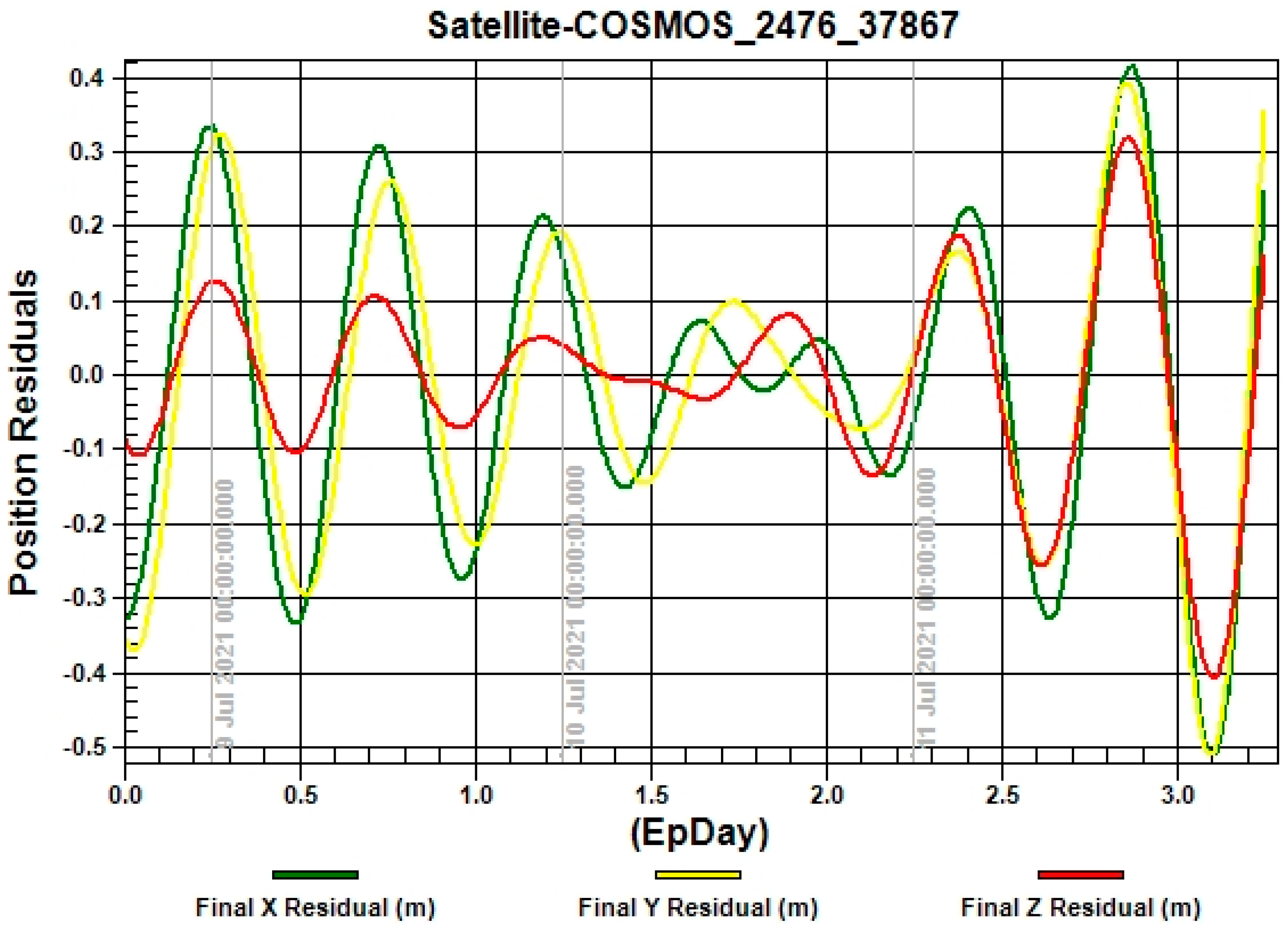
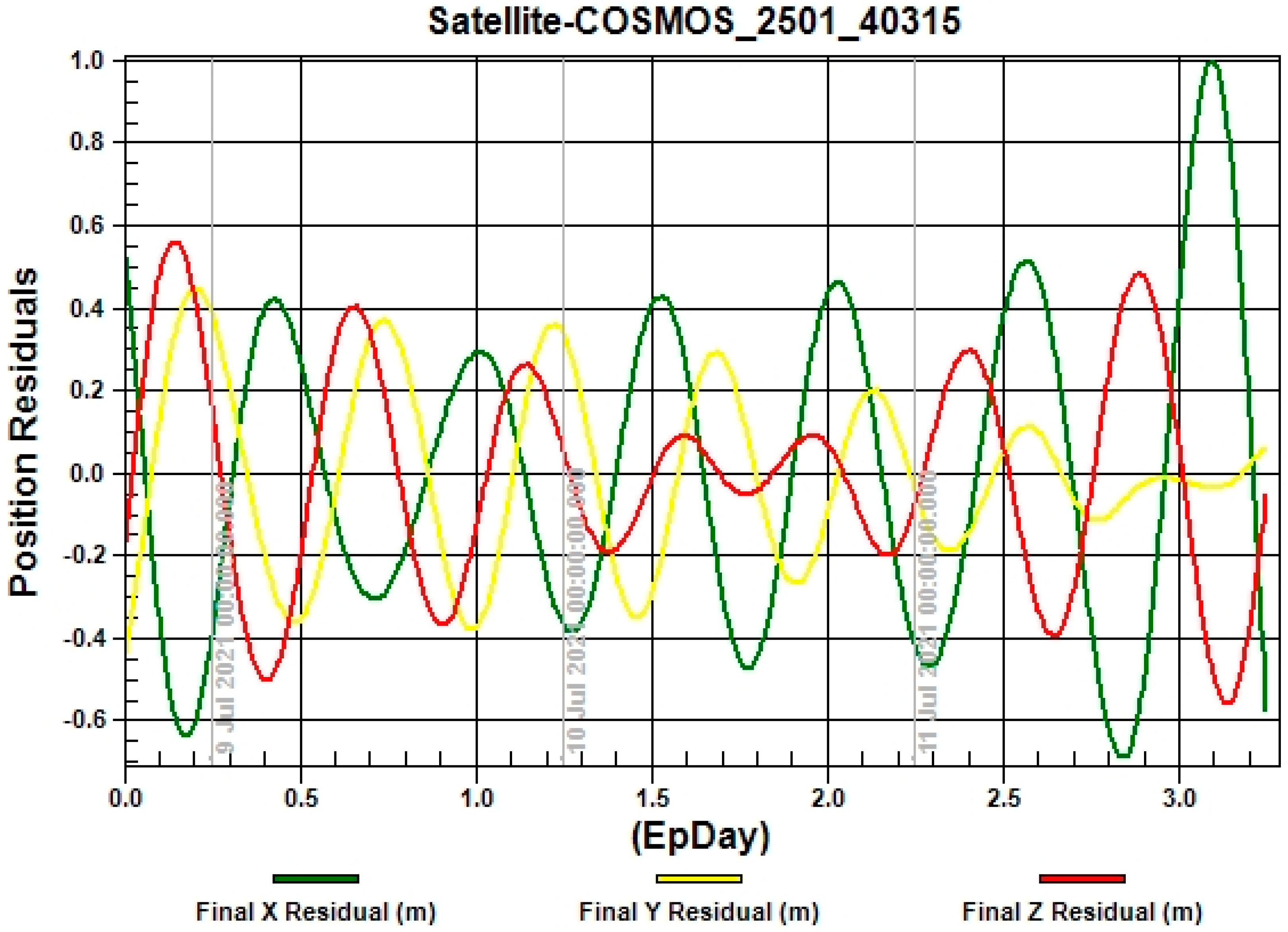
4.2. The Numerical Simulations for Optical and SLR Observation
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Appendix A. Cartesian Coordinates to Classical Orbital Elements (Elliptical Case)
References
- Ibrahim, M.; Hanna, Y.; Samwel, S.; Hegazy, M. Satellite Laser Ranging in Egypt. NRIAG J. Astron. Geophys. 2015, 4, 123–129. [Google Scholar] [CrossRef]
- Ibrahim, M. 17 years of ranging from the Helwan SLR station. Astrophys. Space Sci. 2011, 335, 379–387. [Google Scholar] [CrossRef]
- Sośnica, K.; Prange, L.; Kazmierski, K.; Bury, G.; Drożdżewski, M.; Zajdel, R.; Hadas, T. Validation of Galileo orbits using SLR with a focus on satellites launched into incorrect orbital planes. J. Geod. 2017, 92, 131–148. [Google Scholar] [CrossRef]
- Kim, S.; Lim, H.-C.; Bennett, J.C.; Lachut, M.; Jo, J.H.; Choi, J.; Choi, M.; Park, E.; Yu, S.-Y.; Sung, K.-P. Analysis of Space Debris Orbit Prediction Using Angle and Laser Ranging Data from Two Tracking Sites under Limited Observation Environment. Sensors 2020, 20, 1950. [Google Scholar] [CrossRef] [PubMed]
- Vallado, D.; Agapov, V. Orbit Determination Results for Optical Measurements. In Proceedings of the AIAA/AAS Astrodynamics Specialist Conference, Toronto, ON, Canada, 2–5 August 2010. [Google Scholar] [CrossRef]
- Cordelli, E.; Vananti, A.; Schildknecht, T. Fusion of laser range and angle measurements data for LEO and GEO space debris orbit determination. In Proceedings of the 7th European Conference on Space Debris, Darmstadt, Germany, 18–21 April 2017; Available online: https://conference.sdo.esoc.esa.int/proceedings/sdc7/paper/445 (accessed on 31 July 2022).
- Li, B.; Sang, J.; Zhang, Z. A Real-Time Orbit Determination Method for Smooth Transition from Optical Tracking to Laser Ranging of Debris. Sensors 2016, 16, 962. [Google Scholar] [CrossRef] [PubMed]
- Lejba, P.; Schillak, S. Determination of station positions and velocities from laser ranging observations to Ajisai, Starlette and Stella satellites. Adv. Space Res. 2011, 47, 654–662. [Google Scholar] [CrossRef]
- Schillak, S.; Lejba, P.; Michalek, P. Analysis of the Quality of SLR Station Coordinates Determined from Laser Ranging to the LARES Satellite. Sensors 2021, 21, 737. [Google Scholar] [CrossRef] [PubMed]
- Jagoda, M.; Rutkowska, M. Estimation of the Love numbers: k2, k3 using SLR data of the LAGEOS1, LAGEOS2, STELLA and STARLETTE satellites. Acta Geod. Geophys. 2016, 51, 493–504. [Google Scholar] [CrossRef]
- Rutkowska, M.; Jagoda, M. Estimation of the Elastic Earth Parameters using the SLR LAGEOS 1 and LAGEOS 2 Data. Acta Geophys. 2010, 58, 705–716. [Google Scholar] [CrossRef]
- Sośnica, K.; Thaller, D.; Jäggi, A.; Dach, R.; Beutler, G. Sensitivity of Lageos Orbits to Global Gravity Field Models. Artif. Satell. 2012, 47, 47–65. [Google Scholar] [CrossRef]
- Sośnica, K. LAGEOS Sensitivity to Ocean Tides. Acta Geophys. 2015, 63, 1181–1203. [Google Scholar] [CrossRef]
- Abdel-Aziz, Y.; Abdelaziz, A.; Tealib, S.; Attia, G.; Molotov, I.; Schmalz, S. First Optical Satellite Tracking Station (OSTS) at NRIAG-Egypt. New Astron. 2020, 77, 101361. [Google Scholar] [CrossRef]
- Gasdia, F.; Barjatya, A.; Bilardi, S. Multi-Site Simultaneous Time-Resolved Photometry with a Low Cost Electro-Optics System. Sensors 2017, 17, 1239. [Google Scholar] [CrossRef] [PubMed]
- Abdelaziz, A.M.; Tealib, S.K.; Molotov, I. Analytical study of Egyptian TIBA-1 satellite orbit from Optical Satellite Tracking Station (OSTS), NRIAG-Egypt. Astrophys. Space Sci. 2021, 366, 81. [Google Scholar] [CrossRef]
- Molotov, I.E.; Krugly, Y.N.; Elenin, L.V.; Schildknecht, T.; Rumyantsev, V.V.; Inasaridze, R.Y.; Aivazyan, V.R.; Kapanadze, G.V.; Canals, L.R.; Graziani, F.; et al. Search and study of the space debris and asteroids within ISON project. An. Acad. Bras. Ciênc. 2021, 93 (Suppl. S1), e20200145. [Google Scholar] [CrossRef] [PubMed]
- Jagoda, M.; Rutkowska, M.; Kraszewska, K. The evaluation of time variability of tidal parameters h and l using SLR technique. Acta Geodyn. Geomater. 2016, 14, 153–158. [Google Scholar] [CrossRef]
- Schillak, S. Analysis of the process of the determination of station coordinates by the Satellite Laser Ranging based on results of the Borowiec SLR station in 1993.5–2000.5. Artif. Satell. 2004, 39, 265–287. [Google Scholar]
- Schillak, S.; Lejba, P.; Michałek, P.; Suchodolski, T.; Smagło, A.; Zapaśnik, S. Analysis of the Results of the Borowiec SLR Station (7811) for the Period 1993–2019 as an Example of the Quality Assessment of Satellite Laser Ranging Stations. Sensors 2022, 22, 616. [Google Scholar] [CrossRef] [PubMed]
- Schaeperkoetter, A.V. A Comprehensive Comparison between Angles-Only Initial Orbit Determination Techniques. Doctoral Dissertation, Texas A & M University, College Station, TX, USA, 2012. Available online: https://oaktrust.library.tamu.edu/handle/1969.1/ETD-TAMU-2011-12-10242 (accessed on 31 July 2022).
- Feng, F.; Zhang, Y.; Li, H.; Fang, Y.; Huang, Q.; Tao, X. A Novel Space-Based Orbit Determination Method Based on Distribution Regression and Its Sparse Solution. IEEE Access 2019, 7, 133203–133217. [Google Scholar] [CrossRef]
- Tao, X.; Li, Z.; Gong, Q.; Zhang, Y.; Jiang, P. Uncertainty Analysis of the Short-Arc Initial Orbit Determination. IEEE Access 2020, 8, 38045–38059. [Google Scholar] [CrossRef]




| Site Name | OSTS—NRIAG, Egypt |
|---|---|
| Latitude (deg) | 29.933°N |
| Longitude (deg) | 31.8823°E |
| Elevation (m) | 470 |
| Telescope Series | Celestron 11″ Schmidt Astrograph |
| Telescope Aperture | 280 mm |
| Telescope Focal Length | 620 (mm) |
| Mount Type | CGE Pro Equatorial |
| CCD device | FLI MicroLine ML11002Monochrome |
| Field of View (deg) | 2.3 × 3.4 |
| Pad ID | 1874 | 1889 | 7237 | 7249 | 7825 | 7841 | 7941 |
|---|---|---|---|---|---|---|---|
| Latitude (deg) | 56°N | 43°N | 43.79°N | 39.61°N | 35.31°S | 52.38°N | 40.65°N |
| Longitude (deg) | 37°E | 41°E | 125.44°E | 115.9°E | 149.01°E | 13.06°E | 16.71°E |
| Elevation (m) | 229 | 1155 | 274.9 | 82.300 | 805 | 123.5 | 536.9 |
| Aperture (m) | 0.25 | 0.25 | 0.60 | 0.60 | 1 | 0.44 | 1.50 |
| Field of View (deg) | 0.32 | 0.32 | 0.05 | 0.30 | 0.07 | - | 0.016 |
| Magnitude (mag) | 15 | 15 | 13 | 13 | 10 | 12 | 15 |
| Transmit Efficiency | 0.5 | 0.6 | - | 0.70 | 0.95 | 0.8 | 0.75 |
| Receive Efficiency | 0.5 | 0.5 | - | 0.70 | 0.95 | 0.4 | 0.87 |
| Laser System Information | |||||||
| Laser Type | ND: YAG | ||||||
| Final Beam Diam (m) | 0.03 | 0.03 | 0.06 | 0.01 | 1 | 0.15 | 0.010 |
| Receiver System | |||||||
| Wavelength (nm) | 532 | 532 | 532 | 532 | 532 | 532 | 532 |
| Field of View (″) | 20–120 | - | 20–420 | - | 12 | 10–60 | 60 |
| NORAD ID | 8 July 2021 | 9 July 2021 | 10 July 2021 | 11 July 2021 | ||||
|---|---|---|---|---|---|---|---|---|
| Optical | SLR | Optical | SLR | Optical | SLR | Optical | SLR | |
| 37139 | - | - | OSTS | 1874 | - | - | - | - |
| 32393 | OSTS | - | - | - | -- | 7237 | - | 1889 |
| 40315 | - |
7249 7941 | - |
7249 1889 | - | 7825 | OSTS | |
| 38857 | - | 7249 | OSTS | - | - | - | 7841 | |
| 37867 | OSTS | - | - | - | - | - | - | - |
| NORAD ID | Object | Eccentricity | Perigee (km) | Apogee (km) | Semi-Major Axis (km) | Inclination Degree |
|---|---|---|---|---|---|---|
| 37139 | COSMOS 2464 (GLONASS 122) | 0.0026558 | 19,062 | 19,198 | 25,508 | 64.044 |
| 32393 | COSMOS 2434 (GLONASS107) | 0.0001098 | 19,127 | 19,133 | 25,508 | 64.445 |
| 40315 | COSMOS 2501 (GLONASS134) | 0.0018922 | 19,082 | 19,178 | 25,508 | 63.755 |
| 38857 | GALILEO-FM3 (Galileo103) | 0.0003213 | 23,213 | 23,232 | 29,600 | 55.059 |
| 37867 | COSMOS 2476 (GLONASS 128) | 0.0020470 | 19,078 | 19,182 | 25,508 | 64.580 |
| Satellite Name | Time of Observer | Type of Data | Classical Orbital Elements | |||||
|---|---|---|---|---|---|---|---|---|
| a (km) | e | i (Degree) | ||||||
| COSMOS 2476 (37867) | 8 July 2021 | TLE | 25,508.24 | 0.69 | 0.0021 | 0.0002 | 64.575 | 0 |
| Obs | 25,507.55 | 0.0023 | 64.575 | |||||
| COSMOS 2434 (32393) | 8 July 2021 | TLE | 25,510.57 | 0.98 | 0.0002 | 0.0001 | 64.455 | 0.002 |
| Obs | 25,511.55 | 0.0001 | 64.457 | |||||
| COSMOS 2464 | 9 July 2021 | TLE | 25,508.31 | 1.47 | 0.0026 | 0 | 64.054 | 0.001 |
| Obs | 25,506.84 | 0.0026 | 64.053 | |||||
| GALILEO-FM3 (38857) | 9 July 2021 | TLE | 29,600.35 | 2.28 | 0.0004 | 0 | 55.054 | 0.003 |
| Obs | 29,598.07 | 0.0004 | 55.051 | |||||
| COSMOS 2501 (40315) | 11 July 2021 | TLE | 25,508.24 | 1.28 | 0.0018 | 0 | 63.764 | 0 |
| Obs | 25,509.52 | 0.0018 | 63.764 | |||||
| Stations ID | Time of Observer | Type of Data | Classical Orbital Elements | |||||
|---|---|---|---|---|---|---|---|---|
| a (km) | e | i (Degree) | ||||||
| COSMOS 2501 (40315) | 8 July 2021 | TLE | 25,508.25 | 0.03 | 0.0018 | 0 | 63.764 | 0 |
| SLR7249 | 25,508.22 | 0.0018 | 63.764 | |||||
| SLR7941 | 25,508.24 | 0.01 | 0.0018 | 0 | 63.764 | 0 | ||
| 9 July 2021 | SLR7249 | 25,508.21 | 0.04 | 0.0018 | 0 | 63.764 | 0 | |
| SLR1889 | 25,508.24 | 0.01 | 0.0018 | 0 | 63.764 | 0 | ||
| 10 July 2021 | SLR7825 | 25,508.21 | 0.04 | 0.0018 | 0 | 63.764 | 0 | |
| COSMOS 2464 (37139) | 9 July 2021 | TLE | 25,508.31 | 0.05 | 0.0026 | 0 | 64.054 | 0 |
| SLR | 25,508.26 | 0.0026 | 64.054 | |||||
| COSMOS 2434 (32393) | 10 July 2021 | TLE | 25,508.13 | 0.02 | 0.0002 | 0 | 64.455 | 0 |
| SLR.7237 | 25,508.11 | 0.0002 | 64.455 | |||||
| 11 July 2021 | SLR1889 | 25,508.13 | 0 | 0.0002 | 0 | 64.454 | 0 | |
| GALILEO-FM3 (38857) | 8 July 2021 | TLE | 29,600.35 | 0.48 | 0.0004 | 0 | 55.054 | 0.03 |
| SLR7249 | 29,599.87 | 0.0004 | 55.051 | |||||
| 11 July 2021 | SLR7841 | 29,600.36 | 0.01 | 0.0004 | 0 | 55.054 | 0 | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Abdelaziz, A.M.; Ibrahim, M.; Liang, Z.; Dong, X.; Tealib, S.K. Orbit Predictions for Space Object Tracked by Ground-Based Optical and SLR Stations. Remote Sens. 2022, 14, 4493. https://doi.org/10.3390/rs14184493
Abdelaziz AM, Ibrahim M, Liang Z, Dong X, Tealib SK. Orbit Predictions for Space Object Tracked by Ground-Based Optical and SLR Stations. Remote Sensing. 2022; 14(18):4493. https://doi.org/10.3390/rs14184493
Chicago/Turabian StyleAbdelaziz, A. M., Makram Ibrahim, Zhipeng Liang, Xue Dong, and S. K. Tealib. 2022. "Orbit Predictions for Space Object Tracked by Ground-Based Optical and SLR Stations" Remote Sensing 14, no. 18: 4493. https://doi.org/10.3390/rs14184493
APA StyleAbdelaziz, A. M., Ibrahim, M., Liang, Z., Dong, X., & Tealib, S. K. (2022). Orbit Predictions for Space Object Tracked by Ground-Based Optical and SLR Stations. Remote Sensing, 14(18), 4493. https://doi.org/10.3390/rs14184493









