Bistatic Radar Scattering from Non-Gaussian Height Distributed Rough Surfaces
Abstract
:1. Introduction
2. The Statistical Description of Rough Surfaces
2.1. Height Distribution
2.2. Power Spectral Density
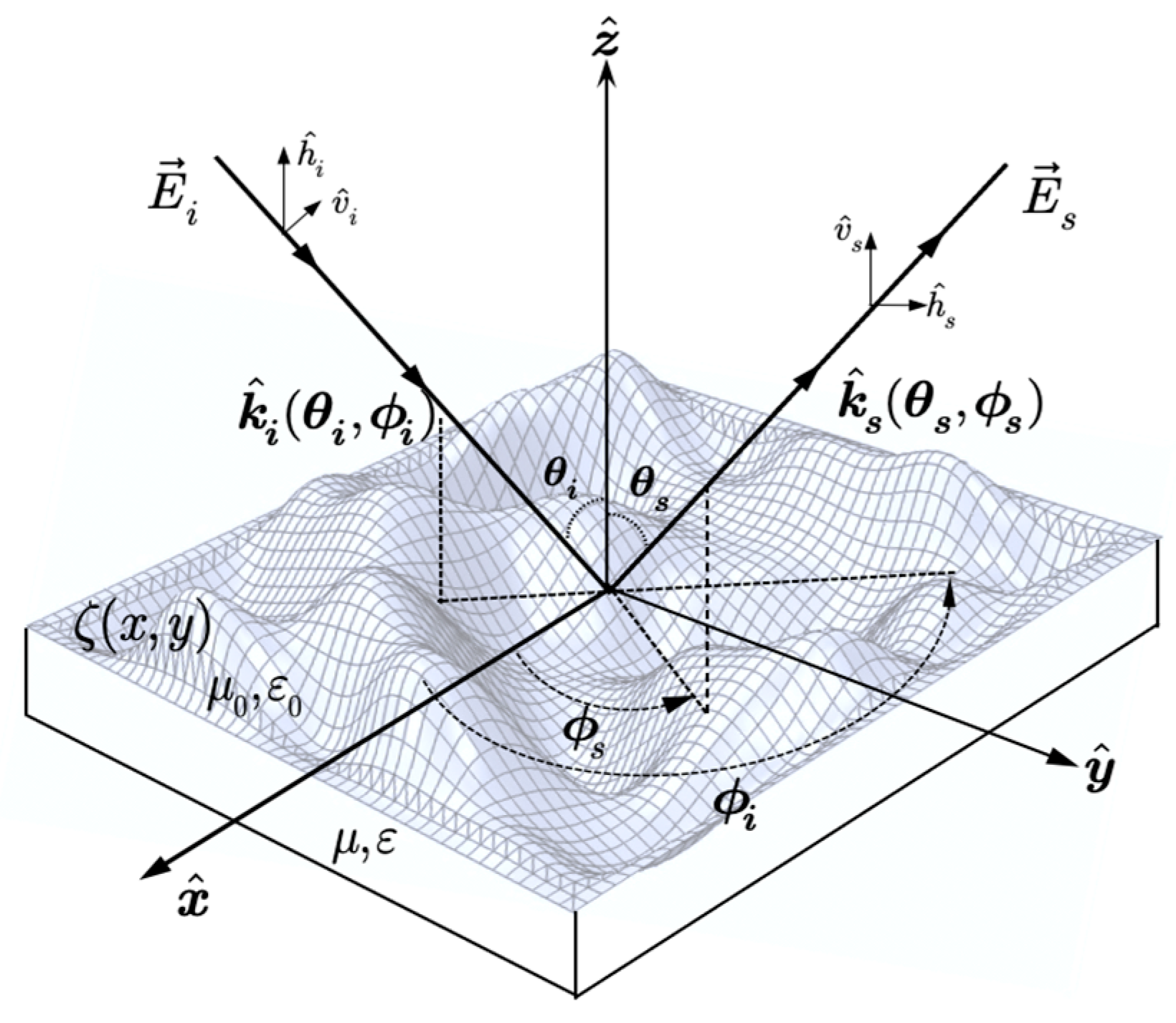
3. Formulation of the Scattering Problem
3.1. Scattered Field
3.2. Scattered Power
3.3. Scattering Coefficients
- (1)
- For a Gaussian height distribution surface:
- (2)
- For an exponential height distribution surface:
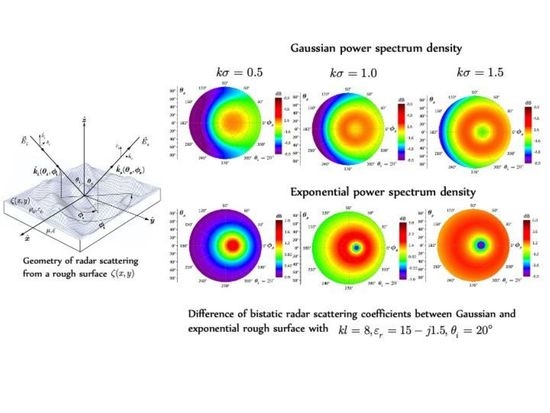
4. Results and Discussion
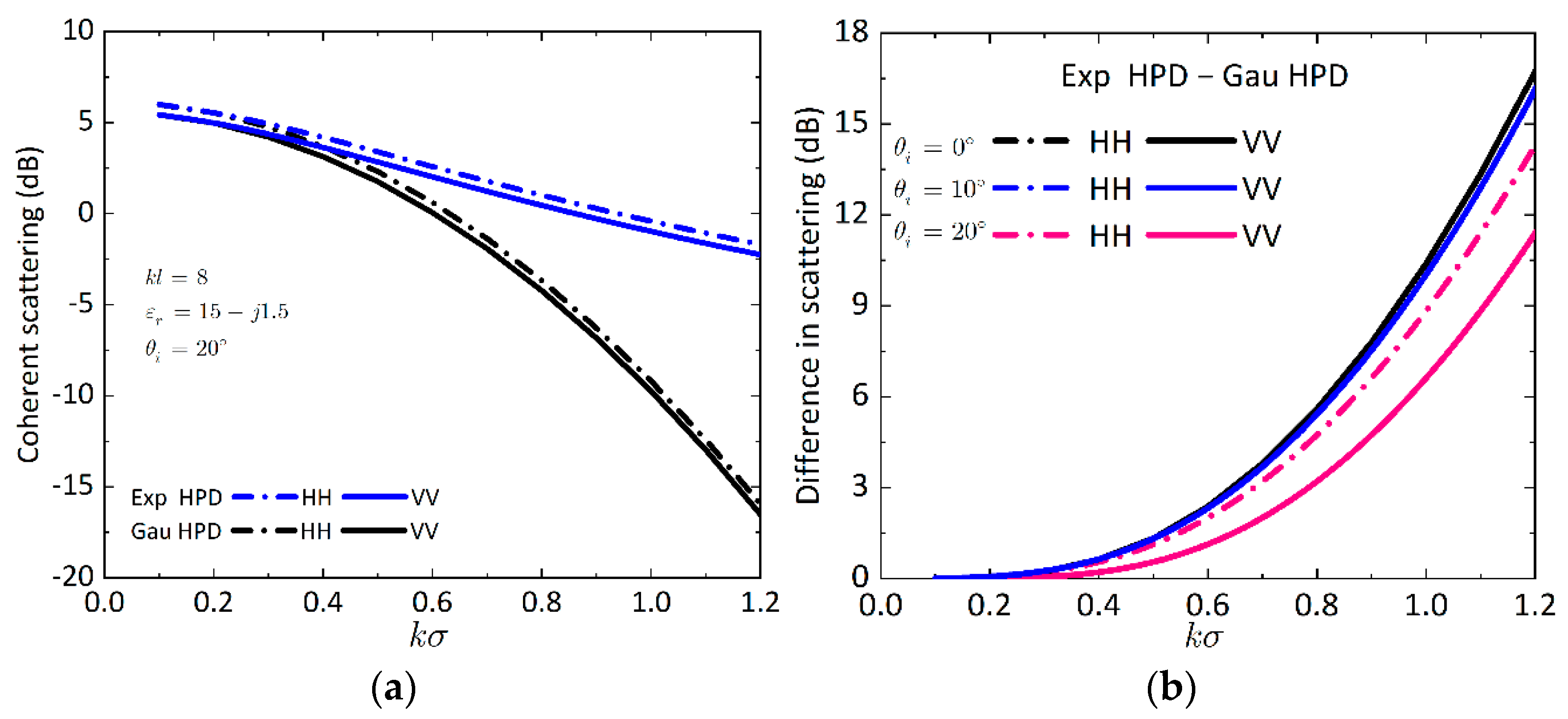
4.1. Surface Roughness Dependence
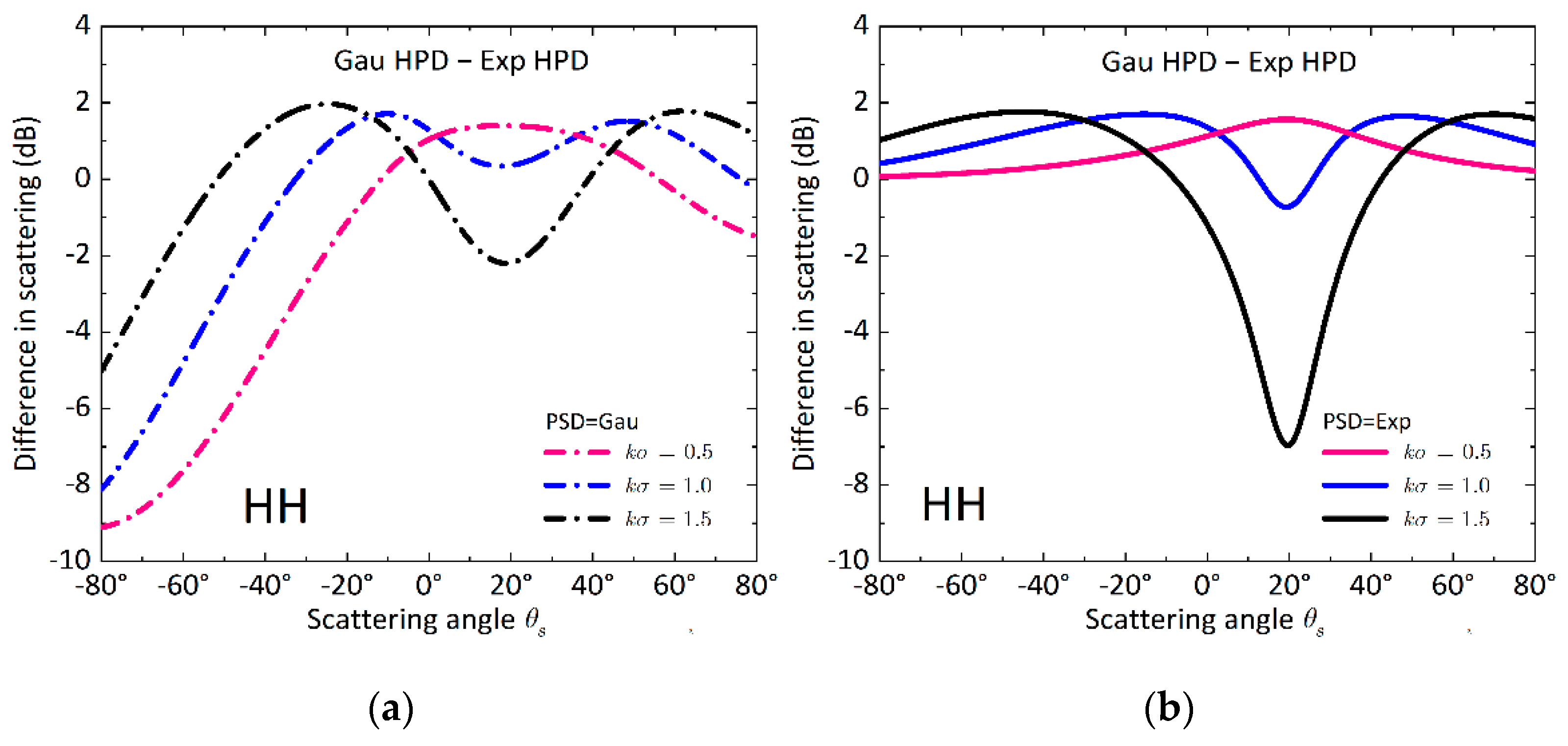
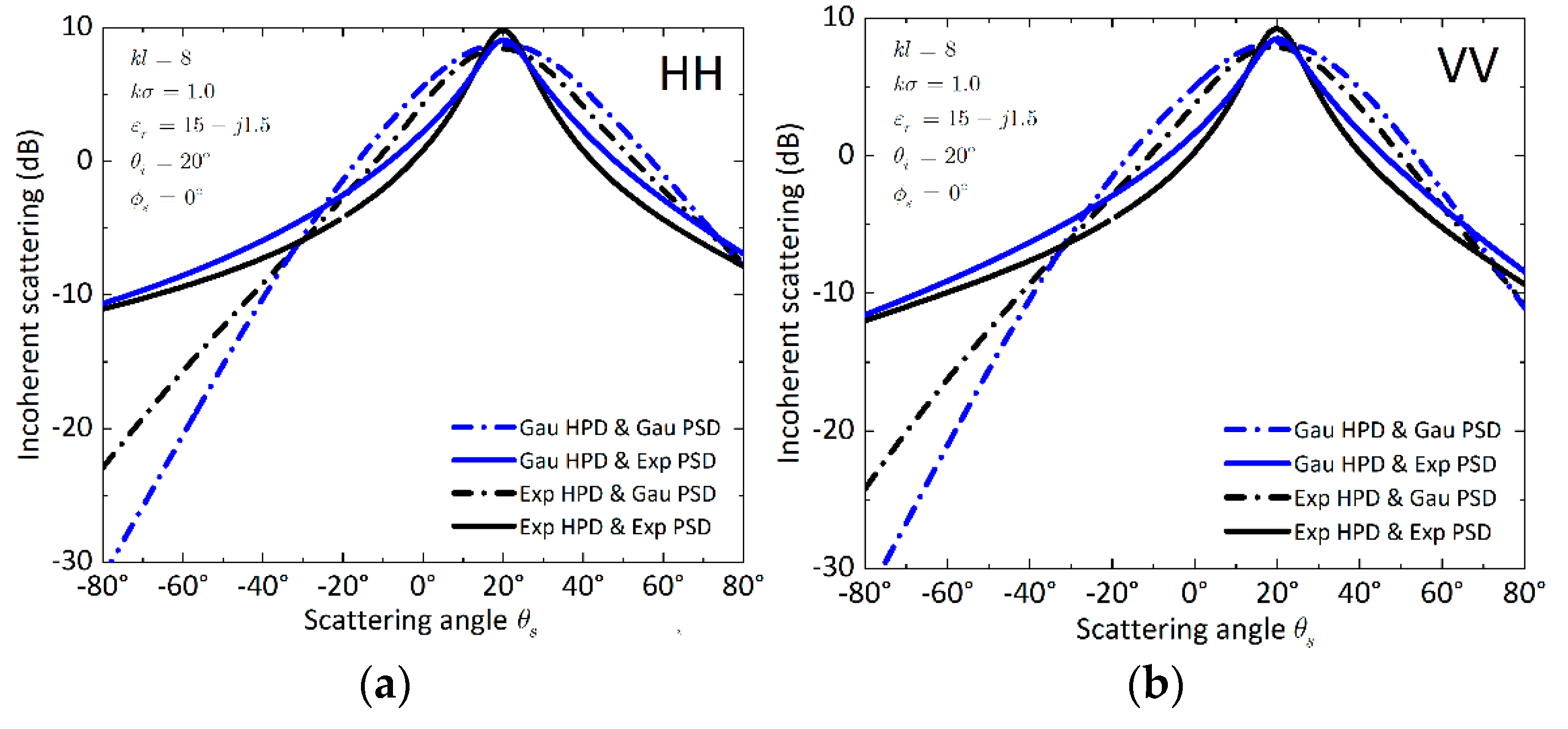
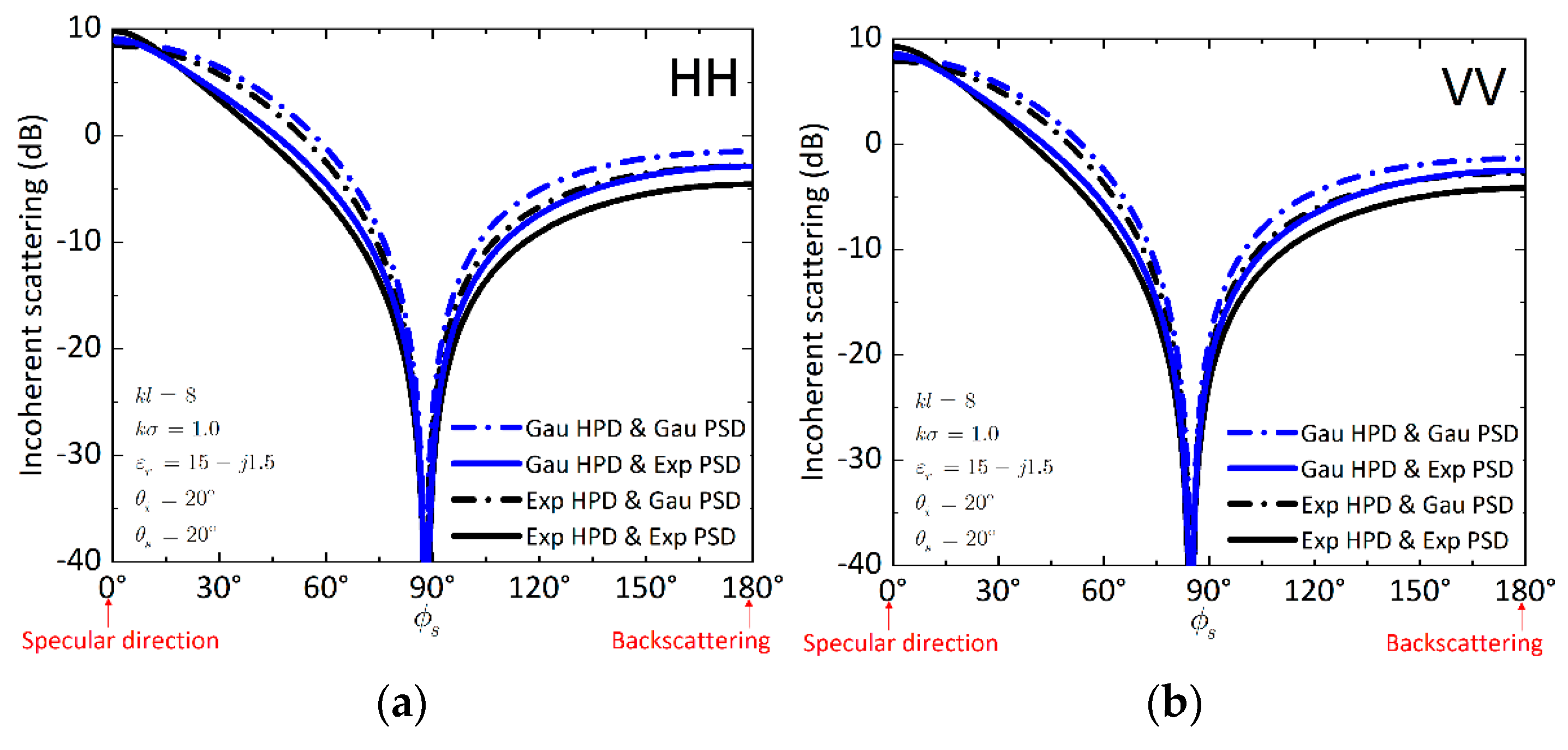
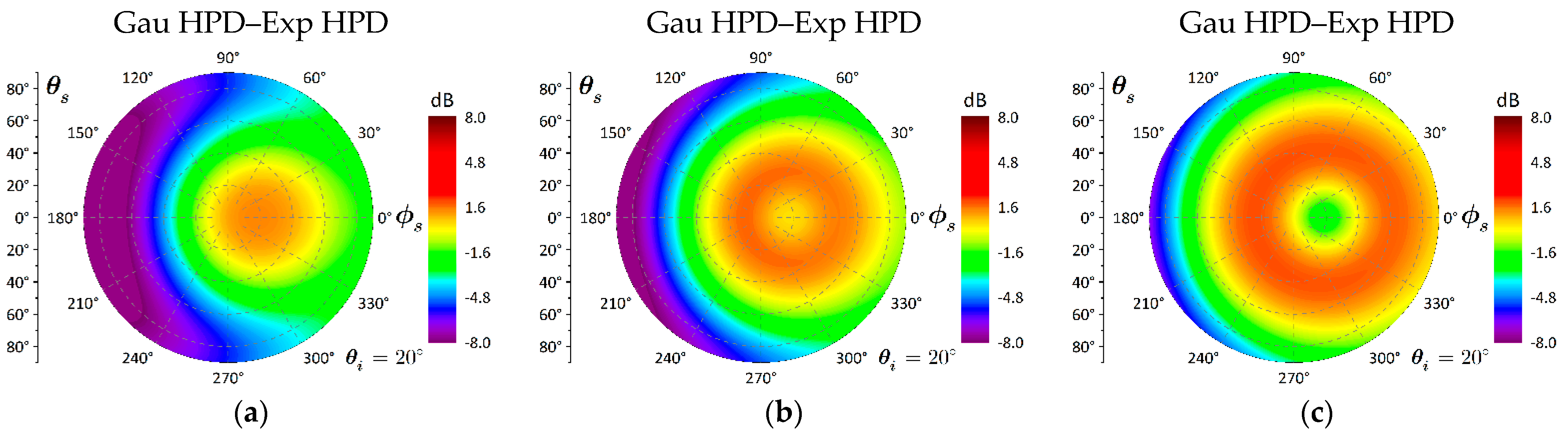
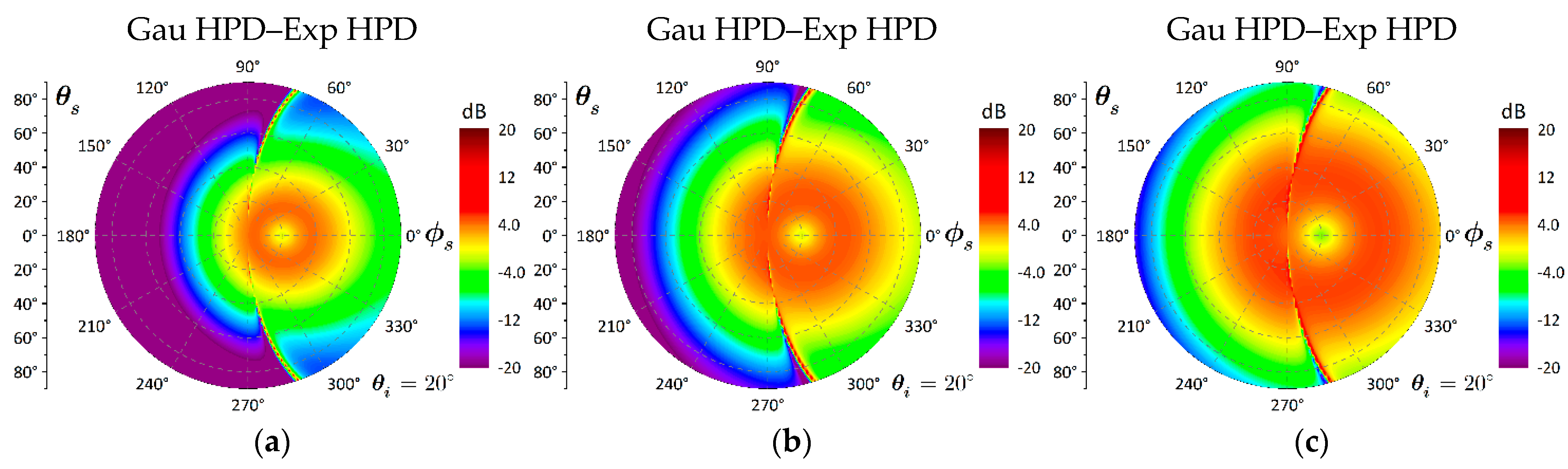
4.2. Scattering Angular Dependence
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Newland, D.E.; Saunders, H. An Introduction to Random Vibration and Spectral Analysis, 2nd ed.; Longman: White Palins, NY, USA, 1984. [Google Scholar]
- Franceschetti, G.; Migliaccio, M.; Riccio, D. An electromagnetic fractal-based model for the study of fading. Radio Sci. 1996, 31, 1749–1759. [Google Scholar] [CrossRef]
- Ward, K.D.; Tough, R.A.; Watts, S. Sea Clutter: Scattering, the K-Distribution and Radar Performance; IET: London, UK, 2006. [Google Scholar]
- Goodman, J.W. Speckle Phenomena in Optics: Theory and Applications; Robert & Company: Englewood, CO, USA, 2007. [Google Scholar]
- Chiang, C.-Y.; Chen, K.S.; Yang, Y.; Zhang, Y.; Wu, L. Radar imaging statistics of non-Gaussian rough surface: A physics-based simulation study. Remote Sens. 2022, 14, 311. [Google Scholar] [CrossRef]
- Huang, N.E.; Long, S.R.; Tung, C.-C.; Yuan, Y.; Bliven, L.F. A non-Gaussian statistical model for surface elevation of nonlinear random wave fields. J. Geophys. Res. 1983, 88, 7597. [Google Scholar] [CrossRef]
- Brown, G.S. A theory for near-normal incidence microwave scattering from first-year sea ice. Radio Sci. 1982, 17, 233–243. [Google Scholar] [CrossRef]
- Stout, K.J. Surface roughness∼measurement, interpretation and significance of data. Mater. Des. 1981, 2, 260–265. [Google Scholar] [CrossRef]
- Saniman, M.N.F.; Wahid, K.A.A.; Foudzi, F.M.; Ladin, H.H.; Ihara, I. Quantitative roughness characterization of non-Gaussian random rough surfaces by ultrasonic method using pitch-catch and pulse-echo configurations. Int. J. Mech. Mechatron. Eng. 2016, 20, 80–87. [Google Scholar]
- Srivastava, D.K.; Agarwal, A.K.; Kumar, J. Effect of liner surface properties on wear and friction in a non-firing engine simulator. Mater. Des. 2007, 28, 1632–1640. [Google Scholar] [CrossRef]
- Eriten, M.; Polycarpou, A.A.; Bergman, L.A. Effects of surface roughness and lubrication on the early stages of fretting of mechanical lap joints. Wear 2011, 271, 2928–2939. [Google Scholar] [CrossRef]
- Jeng, Y. Impact of plateaued surface on tribological performance. Tribol. Trans. 1996, 39, 354–361. [Google Scholar] [CrossRef]
- Wen, H. Design factors affecting the initial roughness of asphalt pavements. Int. J. Pavement Res. Technol. 2011, 4, 268–273. [Google Scholar]
- Taylor, J.B.; Carrano, A.L.; Kandlikar, S.G. Characterization of the effect of surface roughness and texture on fluid flow—Past, present, and future. Int. J. Therm. Sci. 2006, 45, 962–968. [Google Scholar] [CrossRef]
- Eom, H.J.; Fung, A.K. A comparison between backscattering coefficients using Gaussian and non-Gaussian surface statistics. IEEE Trans. Antennas Propag. 1983, AP-31, 635–638. [Google Scholar] [CrossRef]
- Church, E.; Takacs, P. Light scattering from non-Gaussian surfaces. Proc. SPIE 1995, 2541. [Google Scholar] [CrossRef]
- Beckmann, P. Scattering by non-Gaussian surfaces. IEEE Trans. Antenna Propag. 1973, AP-21, 169–175. [Google Scholar] [CrossRef]
- Wu, S.C.; Chen, M.F.; Fung, A.K. Scattering from non-Gaussian randomly rough surfaces-cylindrical case. IEEE Trans. Geosci. Remote Sens. 1988, 26, 790–798. [Google Scholar] [CrossRef]
- Saniman, M.N.F.; Ihara, I. Feasibility study on characterization of non-Gaussian rough surface by ultrasonic reflection method with the Kirchhoff theory. Adv. Technol. Exp. Mech. 2016, 3, 16–00162. [Google Scholar] [CrossRef]
- Guérin, C.A. Scattering on rough surfaces with alpha-stable non-Gaussian height distributions. Waves Random Media 2002, 12, 293–306. [Google Scholar] [CrossRef]
- Chen, K.S. Radar Scattering and Imaging of Rough Surface: Modeling and Applications with MATLAB®; CRC Press: Boca Raton, FL, USA, 2020. [Google Scholar]
- Wu, T.D.; Chen, K.S.; Shi, J.; Lee, H.W.; Fung, A.K. A study of an AIEM model for bistatic scattering from randomly rough surfaces. IEEE Trans. Geosci. Remote Sens. 2008, 46, 2584–2598. [Google Scholar]
- Yang, Y.; Chen, K.S.; Tsang, L.; Yu, L. Depolarized backscattering of rough surface by AIEM model. IEEE J. Sel. Topics Appl. Earth Observ. Remote Sens. 2017, 10, 4740–4752. [Google Scholar] [CrossRef]
- Kim, M.J.; Mendez, E.R.; O’Donnell, K.A. Scattering from Gamma-distributed Surfaces. J. Mod. Opt. 1987, 34, 1107–1119. [Google Scholar] [CrossRef]






Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yang, Y.; Chen, K.-S.; Wang, S. Bistatic Radar Scattering from Non-Gaussian Height Distributed Rough Surfaces. Remote Sens. 2022, 14, 4457. https://doi.org/10.3390/rs14184457
Yang Y, Chen K-S, Wang S. Bistatic Radar Scattering from Non-Gaussian Height Distributed Rough Surfaces. Remote Sensing. 2022; 14(18):4457. https://doi.org/10.3390/rs14184457
Chicago/Turabian StyleYang, Ying, Kun-Shan Chen, and Suyun Wang. 2022. "Bistatic Radar Scattering from Non-Gaussian Height Distributed Rough Surfaces" Remote Sensing 14, no. 18: 4457. https://doi.org/10.3390/rs14184457
APA StyleYang, Y., Chen, K.-S., & Wang, S. (2022). Bistatic Radar Scattering from Non-Gaussian Height Distributed Rough Surfaces. Remote Sensing, 14(18), 4457. https://doi.org/10.3390/rs14184457