Verification and Validation of the COSMIC-2 Excess Phase and Bending Angle Algorithms for Data Quality Assurance at STAR
Abstract
:1. Introduction
- (i)
- POD calculation for the LEO satellite
- (ii)
- Conversion of the COSMIC-2 carrier phase to excess phases
- (iii)
- Inversion of excess phase to bending angle
2. STAR Algorithms to Convert Carrier Phase to Excess Phase and Bending Angle Profiles
2.1. Flow Chart for Converting COSMIC-2 L0 Observations to L2 Data Products
2.2. Preparation of COSMIC-2 L0 and L1a Data
2.3. LEO Orbit and Receiver Clock Bias Determination
2.4. COSMIC-2 Excess Phase Calculation
2.4.1. Carrier Phase Model for Deriving the STAR COSMIC-2 Excess Phase
2.4.2. COSMIC-2 Receiver and GNSS Transmitter Clock Bias Removal
2.4.3. Geometric Range Corrections
2.4.4. Extra Excess Phase Error Corrections
2.5. Using ROPP to Convert COSMIC-2 Excess Phases to Bending Angle Profiles and Refractivity Profiles
2.6. Using ERA5 Data for Validation
3. Verification of the STAR-Derived Excess Phase and Bending Angle Profiles
3.1. Excess Phase Comparisons
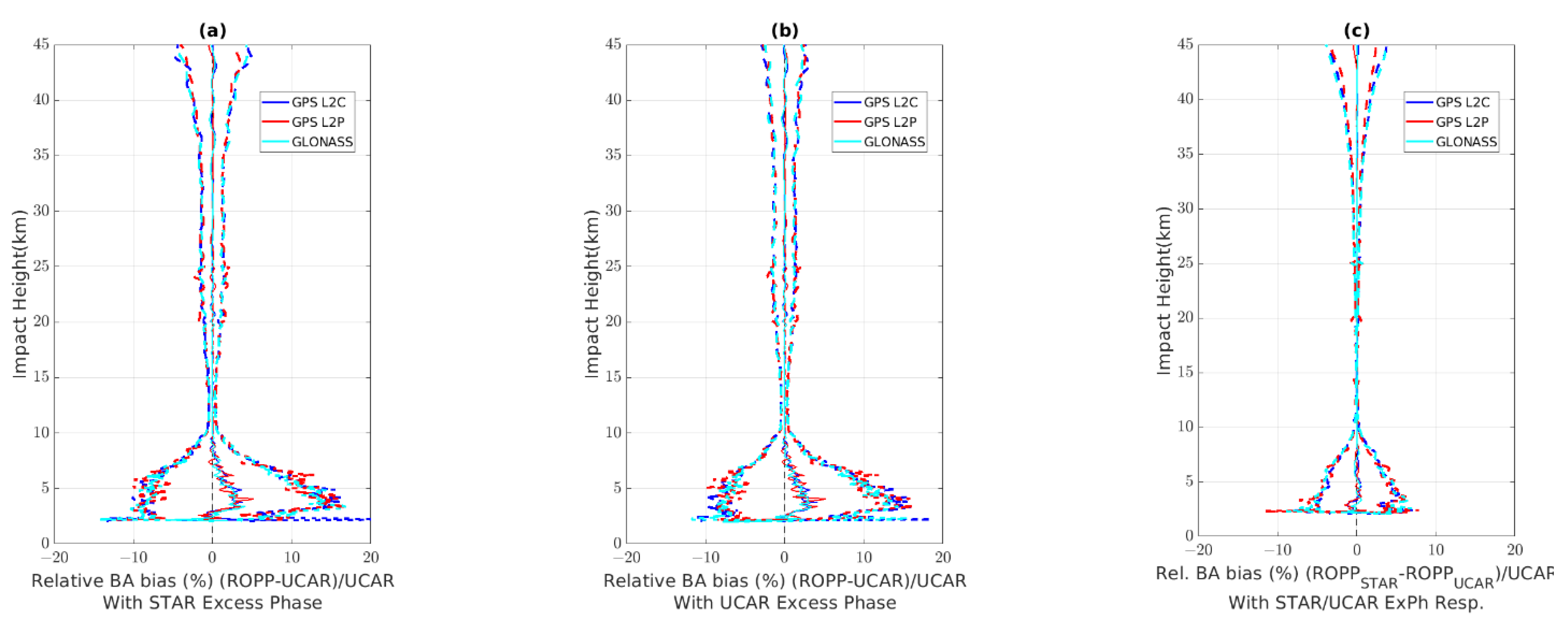
3.2. STAR Bending Angle Comparison with UCAR and ERA5
3.3. Bending Angle Uncertainties Caused by Inversion Algorithm Implementation
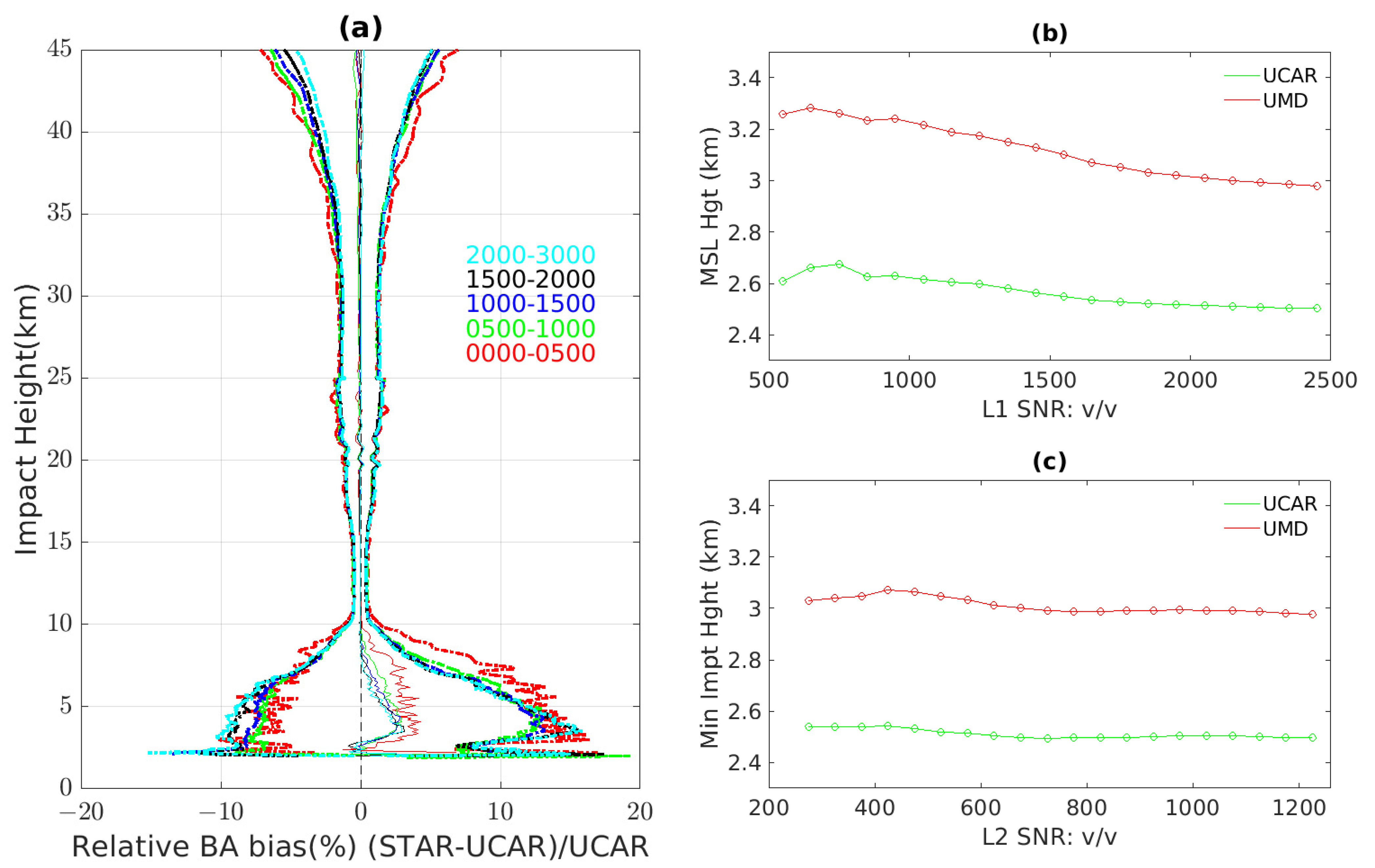
3.4. Comparisons to UCAR Bending Angle for Different SNR Groups
3.5. Refractivity Comparison with UCAR and ERA5
4. Discussions
4.1. POD Uncertainties on the Excess Phase and Bending Angle Inversion
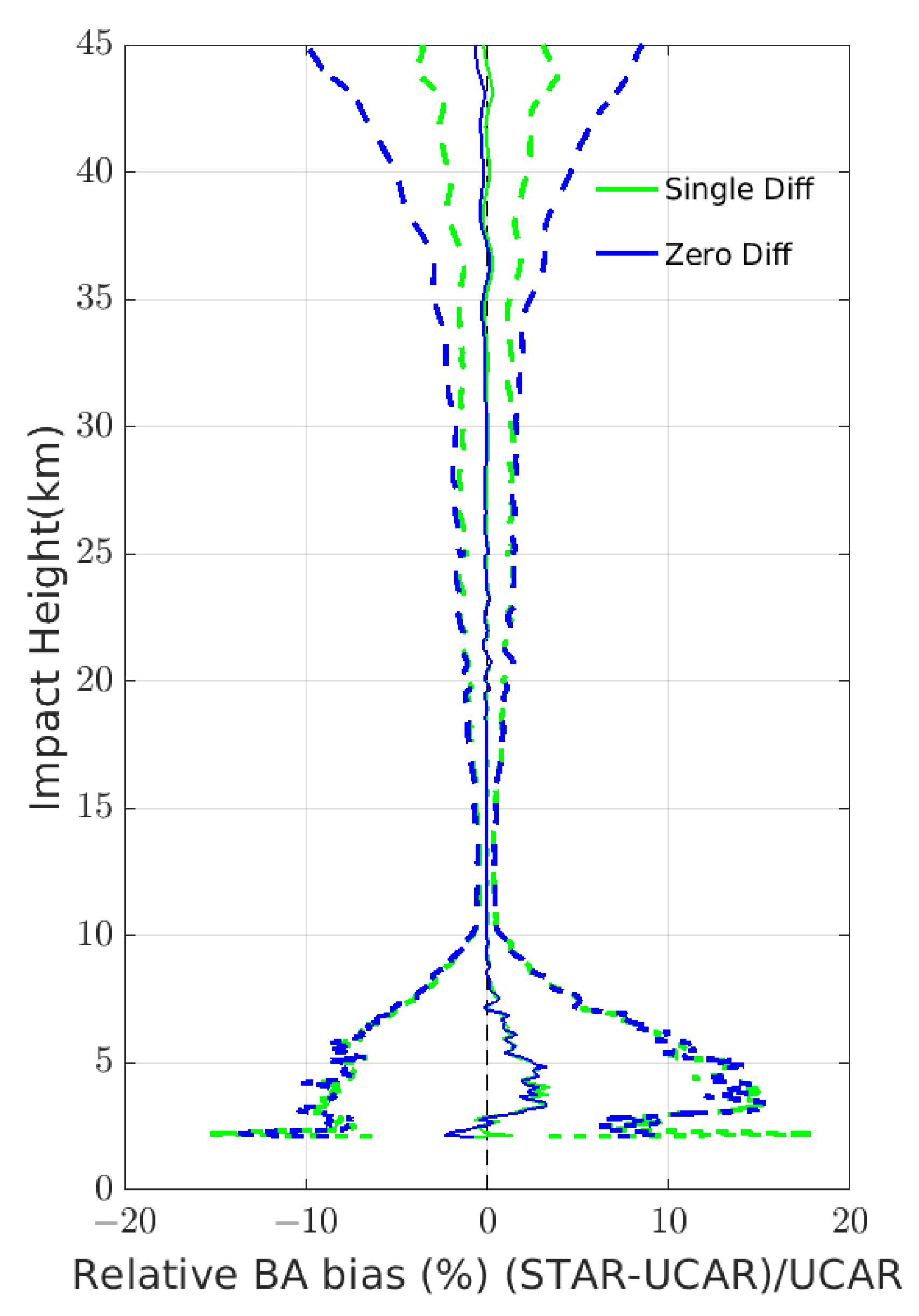
4.2. Zero Differencing versus Single Differencing
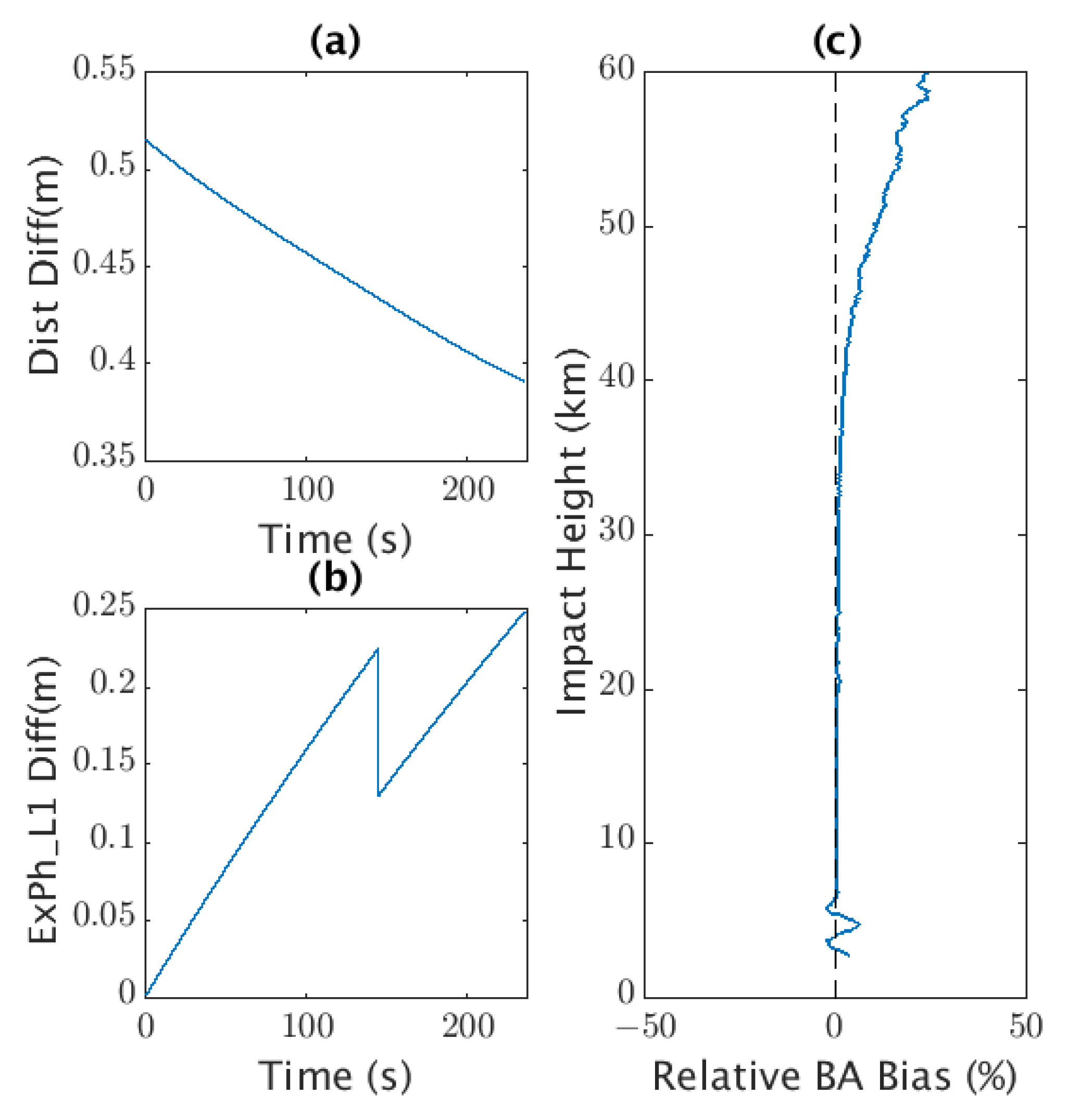
4.3. Setting and Rising Differences
5. Conclusions
- (1)
- While we use UCAR CDAAC’s COSMIC-2 Orbit solution, the receiver clock solution from the Bernese GNSS software is used as input for the excess phase calculation. The Bernese is also used as a tool for GNSS satellite orbital interpolation. However, the receiver clock bias removal in the excess phase is carried out with a single differencing method by differencing with the low rate (1 HZ) POD observations with proper reference satellite observations based on the SNR and zenith angle. We also tested the zero-differencing receiver bias clock removal method. We attributed the STD difference at high altitude from single differencing to large COSMIC-2 receiver clock bias, compared with the COSMIC-1 mission.
- (2)
- The comparison results show good agreement between STAR and UCAR excess phase above 10 km, and gradually, the mean difference and standard deviation increase below that level. There are setting and rising bias differences in the excess phase, reflecting differences in the bending angle conversion.
- (3)
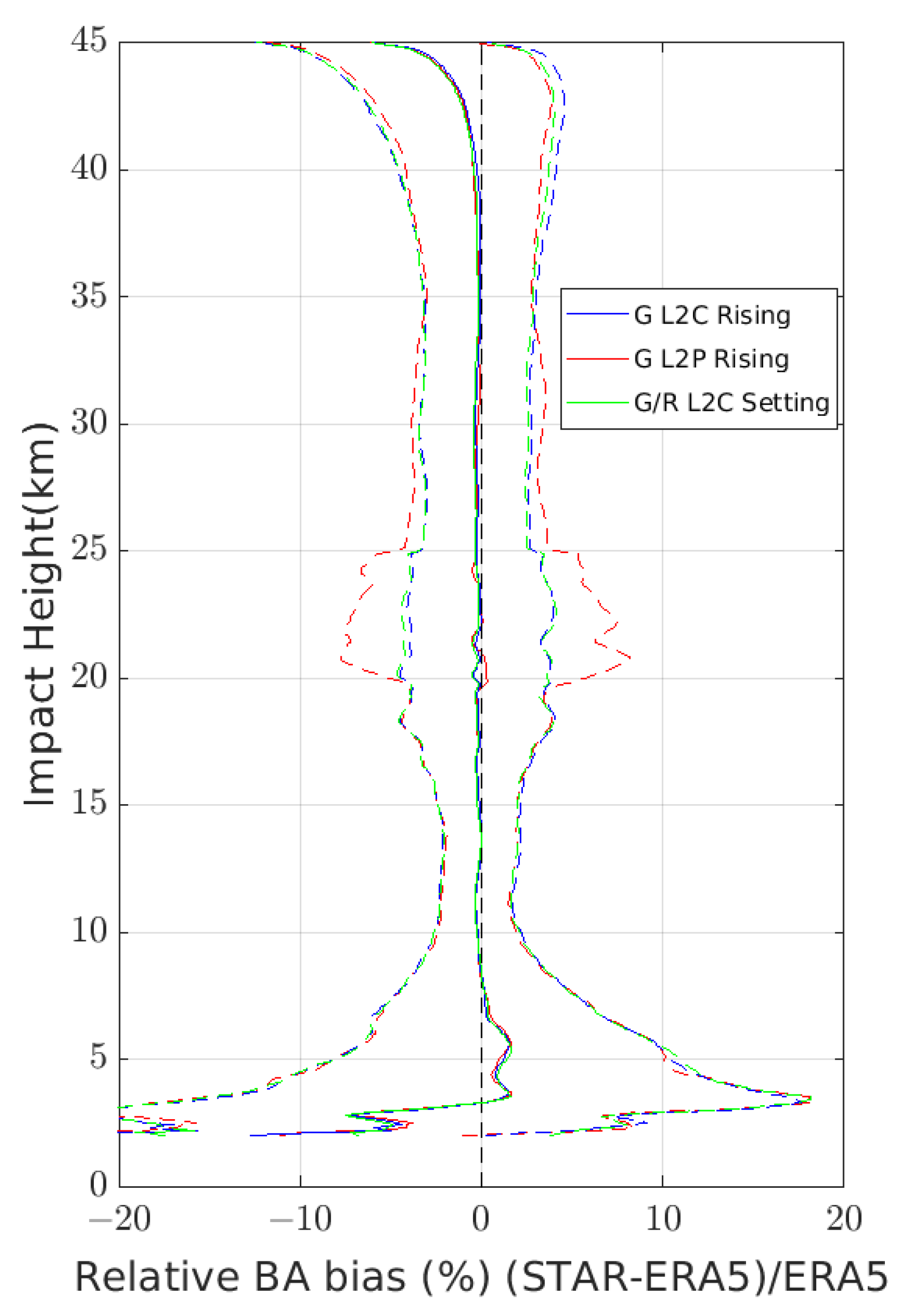
- The ROPP package has been modified to accommodate the newly calculated COSMIC-2 excess phase from GPS and GLONASS. The results show excellent agreement in the relative bending angle compared with ERA5 simulated bending angle profiles and UCAR CDAAC bending angle profiles. Comparison with ERA5 shows that at the impact height of 10–35 km, the mean relative difference is about −0. 34 ± 2. 69% for GPS non-L2P signals, −0. 28 ± 3. 81% for GPS L2P, and −0.09 ± 3.20% for GLONASS. The difference below 8km gradually increases toward a maximum at 5km and decreases toward the surface. Above 35 km, there is an increase with altitude in relative bending angle standard deviation. The relative refractivity bears a similar feature with good agreement between 8 km and 35 km.
- (4)
- Compared with UCAR, from impact height of 10 km to 35 km, the mean relative difference is less than 0.06%. The standard deviation is usually less than 1.3%, and the difference for GPS L2P is also clearly relatively larger at heights 20–25 km. The GLONASS has a similar performance to the GPS L2C.
- (5)
- The L1 SNR value at 80 km is a good indicator of the strength of the GNSS signals transmitted. Comparison among the retrieval quality (comparison with UCAR) and the SNR values shows that the SNR values are correlated with the standard deviations and mean difference comparison with UCAR, implying the SNR impact on the RO bending angle inversion quality. We also found that L2 SNR at the same height (80 km) weakly correlates with the penetration depth only below 600 v/v.
- (6)
- While ROPP has been widely used for previous missions reprocessing by ROM SAF and some new missions, such as China’s GNOS FY−3 Fengyun mission, some improvements or modifications may be needed for the new mission. The bending angle algorithm difference between ROPP and UCAR can explain most lower troposphere differences. In contrast, the upper altitude difference is attributed to the excess phase algorithm regarding the clock bias removal, the ionospheric correction, and the positional errors, which are small but can be amplified due to the small bending angle comparison as the relative bending angle difference.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
References
- Ho, S.-P.; Zhou, X.; Shao, X.; Zhang, B.; Adhikari, L.; Kireev, S.; He, Y.; Yoe, J.G.; Xia-Serafino, W.; Lynch, E. Initial Assessment of the COSMIC-2/FORMOSAT-7 Neutral Atmosphere Data Quality in NESDIS/STAR Using In Situ and Satellite Data. Remote Sens. 2020, 12, 4099. [Google Scholar] [CrossRef]
- Schreiner, W.S.; Weiss, J.P.; Anthes, R.A.; Braun, J.; Chu, V.; Fong, J.; Hunt, D.; Kuo, Y.H.; Meehan, T.; Serafino, W.; et al. COSMIC-2 Radio Occultation Constellation: First Results. Geophys. Res. Lett. 2020, 47, e2019GL086841. [Google Scholar] [CrossRef]
- Ho, S.-P.; Yue, X.; Zeng, Z.; Ao, C.O.; Huang, C.-Y.; Kursinski, E.R.; Kuo, Y.-H. Applications of COSMIC Radio Occultation Data from the Troposphere to Ionosphere and Potential Impacts of COSMIC-2 Data. Bull. Am. Meteorol. Soc. 2014, 95, ES18–ES22. [Google Scholar] [CrossRef]
- Ho, S.-P.; Peng, L.; Anthes, R.A.; Kuo, Y.-H.; Lin, H.-C. Marine Boundary Layer Heights and Their Longitudinal, Diurnal, and Interseasonal Variability in the Southeastern Pacific Using COSMIC, CALIOP, and Radiosonde Data. J. Clim. 2015, 28, 2856–2872. [Google Scholar] [CrossRef] [Green Version]
- Ho, S.-P.; Peng, L.; Vömel, H. Characterization of the long-term radiosonde temperature biases in the upper troposphere and lower stratosphere using COSMIC and Metop-A/GRAS data from 2006 to 2014. Atmos. Chem. Phys. 2017, 17, 4493–4511. [Google Scholar] [CrossRef] [Green Version]
- Ho, S.-P.; Peng, L.; Mears, C.; Anthes, R.A. Comparison of global observations and trends of total precipitable water derived from microwave radiometers and COSMIC radio occultation from 2006 to 2013. Atmos. Chem. Phys. 2018, 18, 259–274. [Google Scholar] [CrossRef] [Green Version]
- Ho, S.-P.; Anthes, R.A.; Ao, C.O.; Healy, S.; Horanyi, A.; Hunt, D.; Mannucci, A.J.; Pedatella, N.; Randel, W.J.; Simmons, A.; et al. The COSMIC/FORMOSAT-3 Radio Occultation Mission after 12 Years: Accomplishments, Remaining Challenges, and Potential Impacts of COSMIC-2. Bull. Am. Meteorol. Soc. 2020, 101, E1107–E1136. [Google Scholar] [CrossRef] [Green Version]
- Biondi, R.; Randel, W.J.; Ho, S.-P.; Neubert, T.; Syndergaard, S. Thermal structure of intense convective clouds derived from GPS radio occultations. Atmos. Chem. Phys. 2012, 12, 5309–5318. [Google Scholar] [CrossRef] [Green Version]
- Biondi, R.; Ho, S.-P.; Randel, W.; Syndergaard, S.; Neubert, T. Tropical cyclone cloud-top height and vertical temperature structure detection using GPS radio occultation measurements. J. Geophys. Res. Atmos. 2013, 118, 5247–5259. [Google Scholar] [CrossRef]
- Huang, C.Y.; Teng, W.H.; Ho, S.P.; Kuo, Y.H. Global variation of COSMIC precipitable water over land: Comparisons with ground-based GPS measurements and NCEP reanalyses. Geophys. Res. Lett. 2013, 40, 5327–5331. [Google Scholar] [CrossRef]
- Teng, W.-H.; Huang, C.-Y.; Ho, S.-P.; Kuo, Y.-H.; Zhou, X.-J. Characteristics of global precipitable water in ENSO events revealed by COSMIC measurements. J. Geophys. Res. Atmos. 2013, 118, 8411–8425. [Google Scholar] [CrossRef]
- Scherllin-Pirscher, B.; Kirchengast, G.; Steiner, A.K.; Kuo, Y.-H.; Foelsche, U. Quantifying uncertainty in climatological fields from GPS radio occultation: An empirical-analytical error model. Atmos. Meas. Tech. 2011, 4, 2019–2034. [Google Scholar] [CrossRef] [Green Version]
- Zeng, Z.; Ho, S.-P.; Sokolovskiy, S.; Kuo, Y.-H. Structural evolution of the Madden-Julian Oscillation from COSMIC radio occultation data. J. Geophys. Res. Atmos. 2012, 117, D22108. [Google Scholar] [CrossRef]
- Rieckh, T.; Anthes, R.; Randel, W.; Ho, S.-P.; Foelsche, U. Tropospheric dry layers in the tropical western Pacific: Comparisons of GPS radio occultation with multiple data sets. Atmos. Meas. Tech. 2017, 10, 1093–1110. [Google Scholar] [CrossRef] [Green Version]
- Schröder, M.; Lockhoff, M.; Shi, L.; August, T.; Bennartz, R.; Brogniez, H.; Calbet, X.; Fell, F.; Forsythe, J.; Gambacorta, A.; et al. The GEWEX Water Vapor Assessment: Overview and Introduction to Results and Recommendations. Remote Sens. 2019, 11, 251. [Google Scholar] [CrossRef] [Green Version]
- Xue, Y.; Li, J.; Menzel, W.P.; Borbas, E.; Ho, S.P.; Li, Z.; Li, J. Characteristics of Satellite Sampling Errors in Total Precipitable Water from SSMIS, HIRS, and COSMIC Observations. J. Geophys. Res. Atmos. 2019, 124, 6966–6981. [Google Scholar] [CrossRef] [Green Version]
- Mears, C.; Ho, S.-P.; Wang, J.; Peng, L. Total Column Water Vapor, [In “States of the Climate in 2017”]. Bull. Amer. Meteor. Sci. 2018, 99, S26–S27. [Google Scholar] [CrossRef]
- Mears, C.; Ho, S.-P.; Bock, O.; Zhou, X.; Nicolas, J. Total Column Water Vapor, [In “States of the Climate in 2018”]. Bull. Amer. Meteor. Sci. 2019, 100, S27–S28. [Google Scholar] [CrossRef] [Green Version]
- Anthes, R.A.; Bernhardt, P.A.; Chen, Y.; Cucurull, L.; Dymond, K.F.; Ector, D.; Healy, S.B.; Ho, S.-P.; Hunt, D.C.; Kuo, Y.-H.; et al. The COSMIC/FORMOSAT-3 Mission: Early Results. Bull. Am. Meteorol. Soc. 2008, 89, 313–334. [Google Scholar] [CrossRef]
- Syndergaard, S. On the ionosphere calibration in GPS radio occultation measurements. Radio Sci. 2000, 35, 865–883. [Google Scholar] [CrossRef]
- Cardinali, C.; Healy, S. Impact of GPS radio occultation measurements in the ECMWF system using adjoint-based diagnostics. Q. J. R. Meteorol. Soc. 2014, 140, 2315–2320. [Google Scholar] [CrossRef]
- Ruston, B.; Healy, S. Forecast Impact of FORMOSAT -7/ COSMIC -2 GNSS Radio Occultation Measurements. Atmos. Sci. Lett. 2021, 22, e1019. [Google Scholar] [CrossRef]
- Kursinski, E.R.; Hajj, G.A.; Bertiger, W.I.; Leroy, S.S.; Meehan, T.K.; Romans, L.J.; Schofield, J.T.; McCleese, D.J.; Melbourne, W.G.; Thornton, C.L.; et al. Initial Results of Radio Occultation Observations of Earth’s Atmosphere Using the Global Positioning System. Science 1996, 271, 1107–1110. [Google Scholar] [CrossRef]
- Kursinski, E.R.; Hajj, G.A.; Schofield, J.T.; Linfield, R.P.; Hardy, K.R. Observing Earth’s atmosphere with radio occultation measurements using the Global Positioning System. J. Geophys. Res. Atmos. 1997, 102, 23429–23465. [Google Scholar] [CrossRef]
- Schreiner, W.; Rocken, C.; Sokolovskiy, S.; Hunt, D. Quality assessment of COSMIC/FORMOSAT-3 GPS radio occultation data derived from single- and double-difference atmospheric excess phase processing. GPS Solut. 2009, 14, 13–22. [Google Scholar] [CrossRef]
- Hwang, C.; Tseng, T.-P.; Lin, T.; Švehla, D.; Schreiner, B. Precise orbit determination for the FORMOSAT-3/COSMIC satellite mission using GPS. J. Geod. 2008, 83, 477–489. [Google Scholar] [CrossRef]
- Weiss, J.-P.; Hunt, D.; Schreiner, W.; VanHove, T.; Arnold, D.; Jaeggi, A. COSMIC-2 Precise Orbit Determination Results. In Proceedings of the EGU General Assembly 2020, Online, 4–8 May 2020. [Google Scholar]
- Xia, P.; Ye, S.; Jiang, K.; Chen, D. Estimation and evaluation of COSMIC radio occultation excess phase using undifferenced measurements. Atmos. Meas. Tech. 2017, 10, 1813–1821. [Google Scholar] [CrossRef] [Green Version]
- Wang, Y.; Yang, R.; Morton, Y.T. Kalman Filter-based Robust Closed-loop Carrier Tracking of Airborne GNSS Radio-Occultation Signals. IEEE Trans. Aerosp. Electron. Syst. 2020, 56, 3384–3393. [Google Scholar] [CrossRef]
- Steiner, A.K.; Ladstädter, F.; Ao, C.O.; Gleisner, H.; Ho, S.-P.; Hunt, D.; Schmidt, T.; Foelsche, U.; Kirchengast, G.; Kuo, Y.-H.; et al. Consistency and structural uncertainty of multi-mission GPS radio occultation records. Atmos. Meas. Tech. 2020, 13, 2547–2575. [Google Scholar] [CrossRef]
- Xu, X.; Zou, X. Comparison of MetOp-A/-B GRAS radio occultation data processed by CDAAC and ROM. GPS Solut. 2020, 24, 34. [Google Scholar] [CrossRef]
- Gorbunov, M.E.; Shmakov, A.V.; Leroy, S.S.; Lauritsen, K.B. COSMIC Radio Occultation Processing: Cross-Center Comparison and Validation. J. Atmos. Ocean. Technol. 2011, 28, 737–751. [Google Scholar] [CrossRef]
- Adhikari, L.; Ho, S.-P.; Zhou, X. Inverting COSMIC-2 Phase Data to Bending Angle and Refractivity Profiles Using the Full Spectrum Inversion Method. Remote Sens. 2021, 13, 1793. [Google Scholar] [CrossRef]
- UCAR COSMIC Program, COSMIC-2 Data Products (Level0, Level 1a and Level1b). Available online: https://www.cosmic.ucar.edu/what-we-do/cosmic-2/data (accessed on 16 June 2022).
- Ho, S.-P.; Sho, X.; Chen, Y.; Zhang, B.; Adhikari, L.; Zhou, X. NESDIS STAR GNSS RO Processing, Validation, and Monitoring System: Initial Validation of the STAR COSMIC-2 Data Products. TAO COSMIC-2 Spec. Issue 2022. [Google Scholar]
- Steiner, A.K.; Hunt, D.; Ho, S.P.; Kirchengast, G.; Mannucci, A.J.; Scherllin-Pirscher, B.; Gleisner, H.; von Engeln, A.; Schmidt, T.; Ao, C.; et al. Quantification of structural uncertainty in climate data records from GPS radio occultation. Atmos. Chem. Phys. 2013, 13, 1469–1484. [Google Scholar] [CrossRef] [Green Version]
- Culverwell, I.D.; Lewis, H.W.; Offiler, D.; Marquardt, C.; Burrows, C.P. The Radio Occultation Processing Package, ROPP. Atmos. Meas. Tech. 2015, 8, 1887–1899. [Google Scholar] [CrossRef] [Green Version]
- Liao, M.; Healy, S.; Zhang, P. Processing and quality control of FY-3C GNOS data used in numerical weather prediction applications. Atmos. Meas. Tech. 2019, 12, 2679–2692. [Google Scholar] [CrossRef] [Green Version]
- UCAR/CDAAC RO Operational Data File Name Convention. Available online: https://cdaac-www.cosmic.ucar.edu/cdaac/doc/formats.html (accessed on 16 June 2022).
- Dach, R.; Lutz, S.; Walser, P.; Fridez, P. (Eds.) Bernese GNSS Software Version 5.2. User Manual; Publikation Digital AG: Biel, Switzerland, 2015. [Google Scholar]
- Dach, R.; Schaer, S.; Arnold, D.; Orliac, E.; Prange, L.; Susnik, A.; Villiger, A.; Jäggi, A. CODE Final Product Series for the IGS. 2016. Available online: http://ftp.aiub.unibe.ch/CODE/ (accessed on 4 July 2022).
- Hajj, G.A.; Kursinski, E.R.; Romans, L.J.; Bertiger, W.I.; Leroy, S.S. A technical description of atmospheric sounding by GPS occultation. J. Atmos. Sol. -Terr. Phys. 2002, 64, 451–469. [Google Scholar] [CrossRef]
- Wickert, J.; Beyerle, G.; Hajj, G.A.; Schwieger, V.; Reigber, C. GPS radio occultation with CHAMP: Atmospheric profiling utilizing the space-based single difference technique. Geophys. Res. Lett. 2002, 29, 28-21–28-24. [Google Scholar] [CrossRef] [Green Version]
- Bernese GNSS Satellite Information, Problem and Phase Center Correction Files. Available online: ftp://ftp.aiub.unibe.ch/BSWUSER52/GEN (accessed on 16 June 2022).
- Wu, J.; Wu, S.; Hajj, G.; Bertiger, W.; Lichten, S. Effects of antenna orientation on GPS carrier phase measurements. Manuscr. Geod. 1993, 18, 91–98. [Google Scholar]
- Pedatella, N.M.; Zakharenkova, I.; Braun, J.J.; Cherniak, I.; Hunt, D.; Schreiner, W.S.; Straus, P.R.; Valant-Weiss, B.L.; Vanhove, T.; Weiss, J.; et al. Processing and Validation of FORMOSAT-7/COSMIC-2 GPS Total Electron Content Observations. Radio Sci. 2021, 56, e2021RS007267. [Google Scholar] [CrossRef]
- Gorbunov, M.E.; Lauritsen, K.B. Analysis of wave fields by Fourier integral operators and their application for radio occultations. Radio Sci. 2004, 39, RS4010. [Google Scholar] [CrossRef]
- Sokolovskiy, S.; Rocken, C.; Schreiner, W.; Hunt, D.; Johnson, J. Postprocessing of L1 GPS radio occultation signals recorded in open-loop mode. Radio Sci. 2009, 44, RS2002. [Google Scholar] [CrossRef]
- FORMOSAT-7/COSMIC-2 Neutral Atmosphere Provisional Data Release 1. Available online: https://data.cosmic.ucar.edu/gnss-ro/cosmic2/provisional/F7C2_NA_Provisional_Data_Release_1_Memo.pdf (accessed on 16 June 2022).
- Gorbunov, M. The influence of the signal-to-noise ratio upon radio occultation inversion quality. Atmos. Meas. Tech. Discuss. 2020, 2020, 1–11. [Google Scholar] [CrossRef] [Green Version]
- Ho, S.-P.; Hunt, D.; Steiner, A.K.; Mannucci, A.J.; Kirchengast, G.; Gleisner, H.; Heise, S.; von Engeln, A.; Marquardt, C.; Sokolovskiy, S.; et al. Reproducibility of GPS radio occultation data for climate monitoring: Profile-to-profile inter-comparison of CHAMP climate records 2002 to 2008 from six data centers. J. Geophys. Res. Atmos. 2012, 117, D18111. [Google Scholar] [CrossRef]
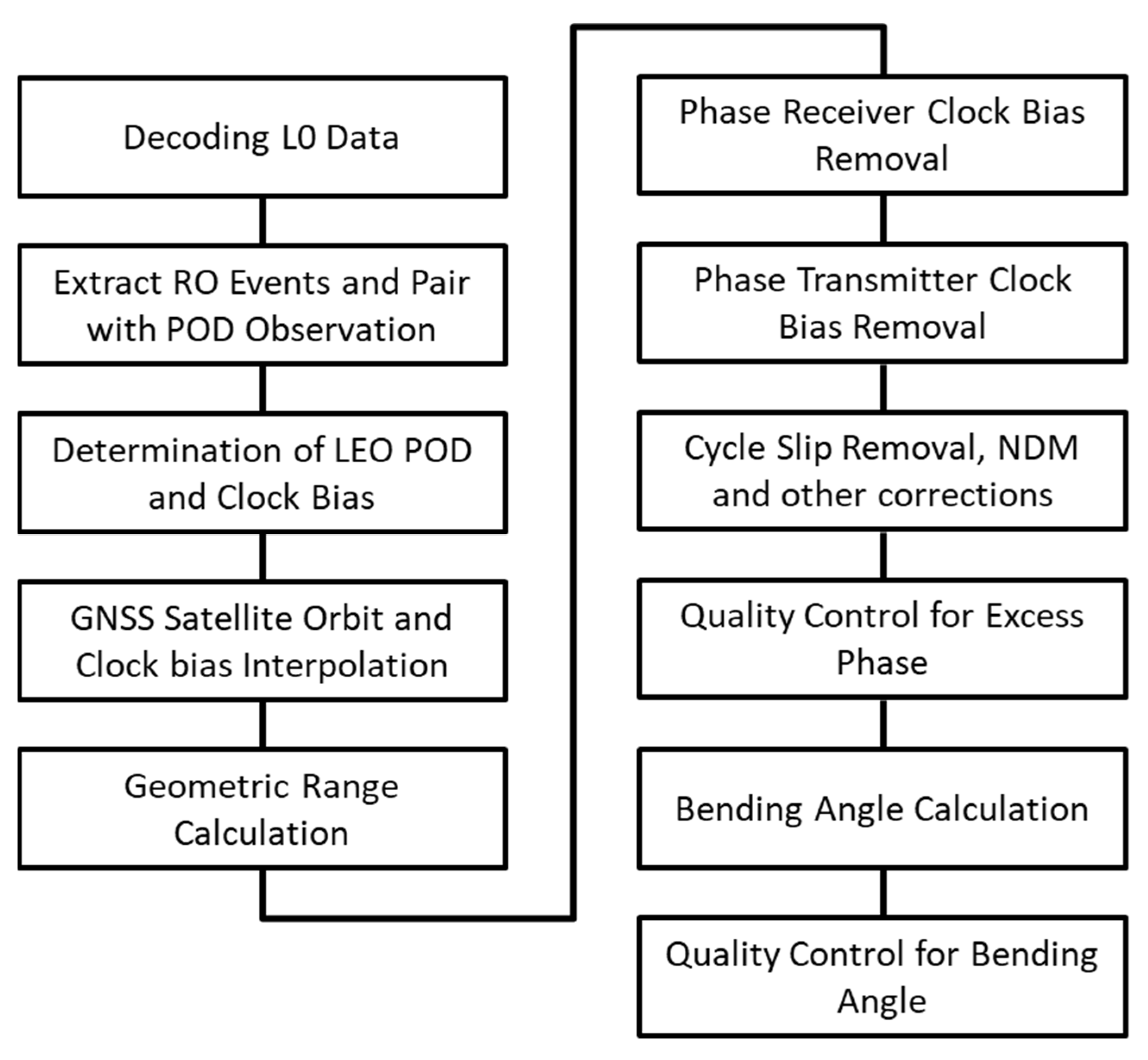
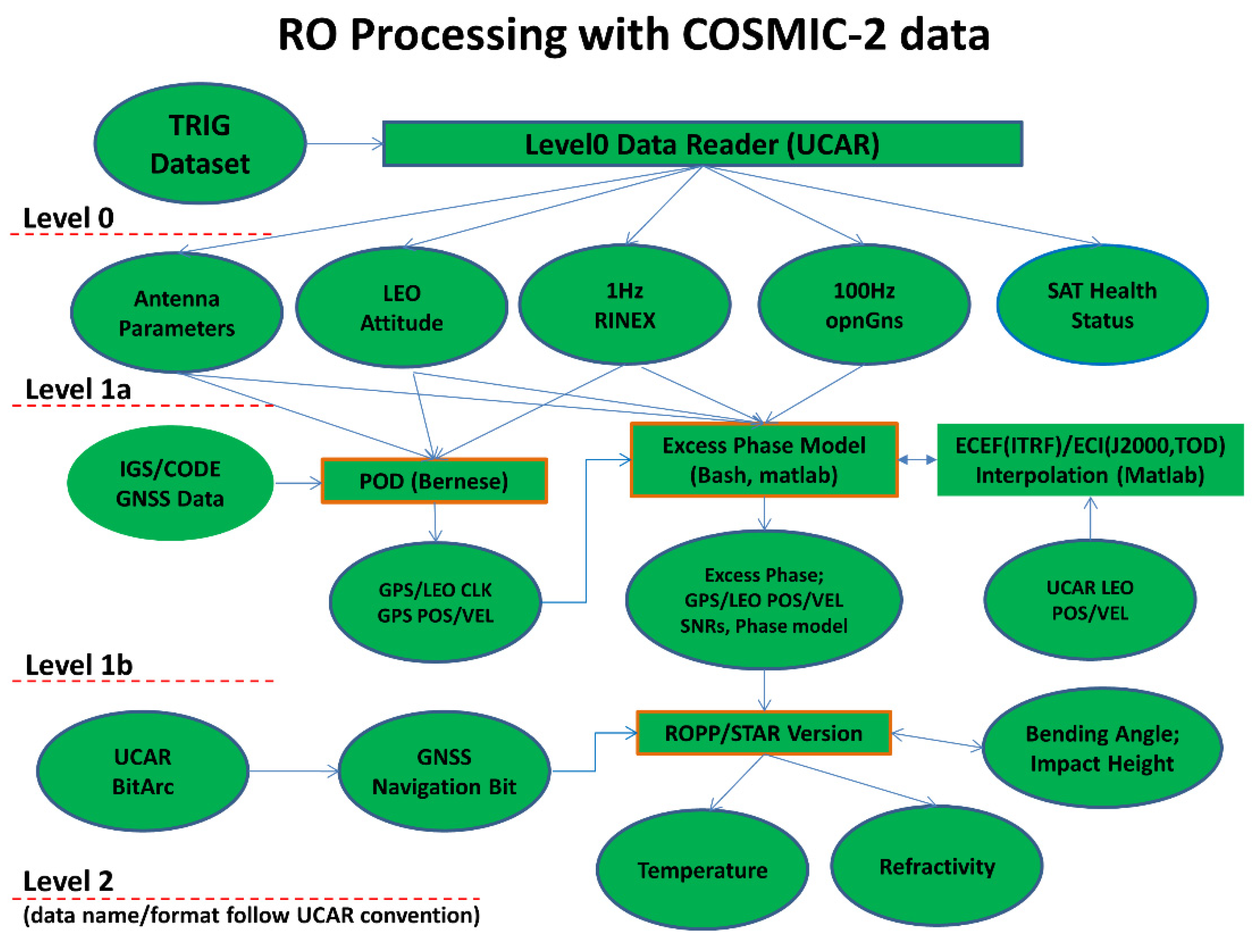

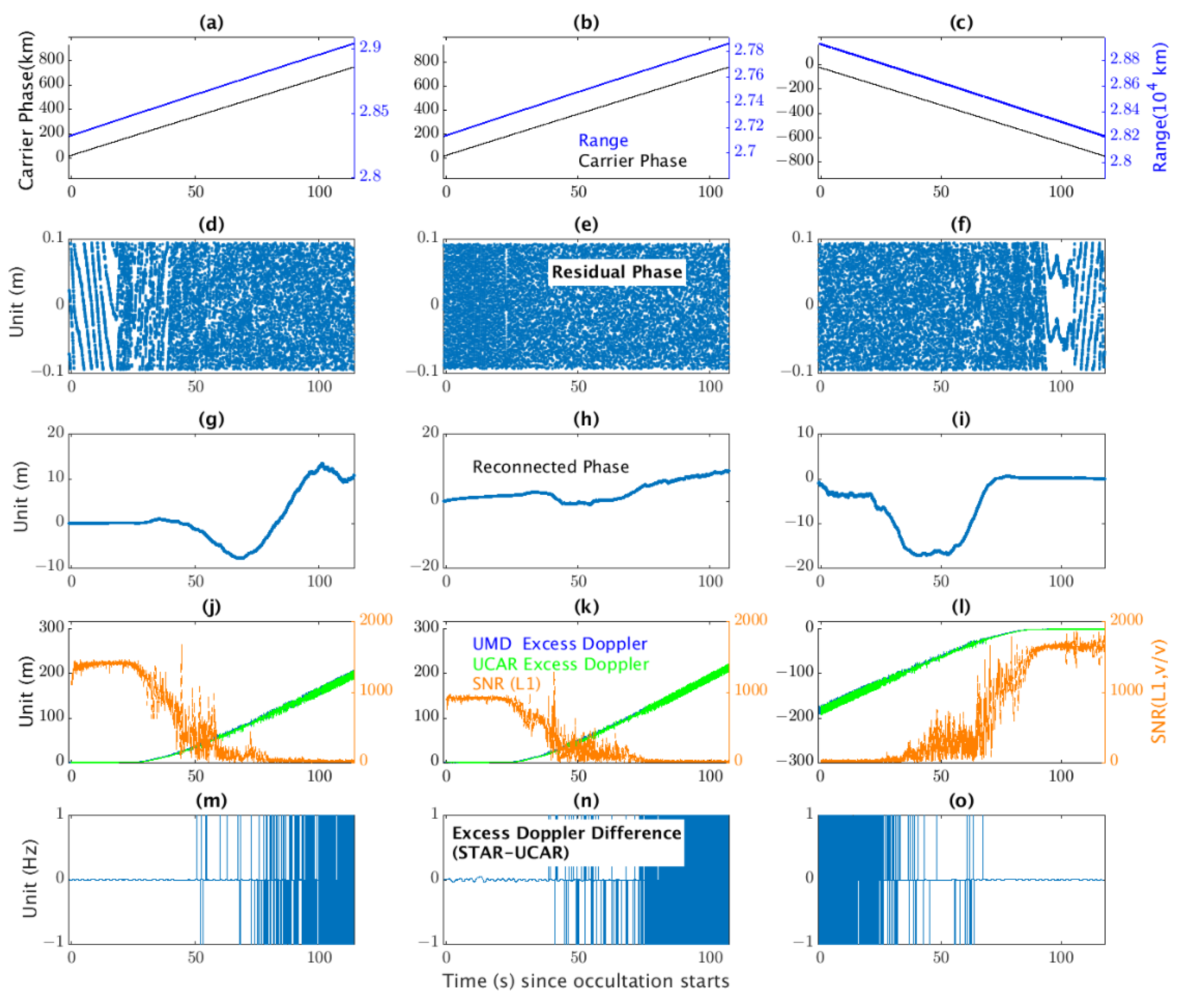

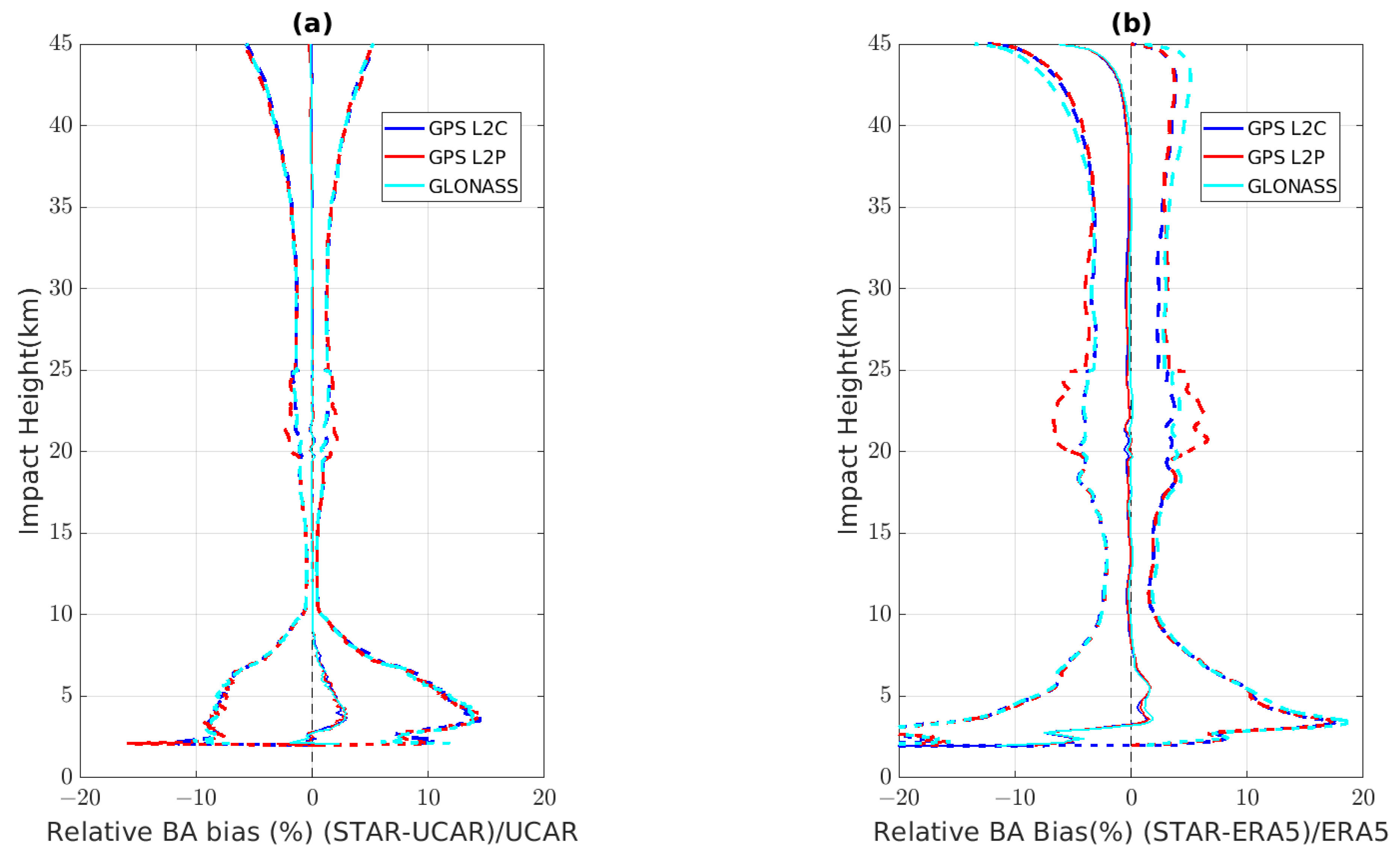

| Processing Step | Input Data | Main Output Data | Processing Methods/Libraries |
|---|---|---|---|
| Decoding L0 Data Set | COSMIC-2 L0 Datasets | COSMIC-2 L1a Dataset (opnGns and podCrx) | UCAR’s L0 to L1a Perl script |
| Extract RO events and pair with POD observations for reference GNSS satellite | OpnGns datasets podCrx datasets | Separate RO events and associated reference POD observation | opnGnss decoder (Perl) podCrx/RINEX reader, Matlab/IDL/bash scripts |
| The LEO Precise Orbit and Receiver Clock error estimation | COSMIC-2 Attitude Files; COSMIC-2 podCrx POD observations; CODE Final Orbital Products | COSMIC-2 POD (30 s, not used in this paper) and Receiver Clock bias at 2 s intervals (leoClk) | Bernese POD software Bash scripts for data preparation |
| GNSS/LEO Satellite Antenna Position (phase center), Geometric range calculation | CODE Final Orbit Products COSMIC-2 OpnGns and podCRX COSMIC-2 LeoOrb (UCAR), leoClk | GNSS/COSMIC-2 Position (ECI), Corrected receiver/transmitter time tag, the direct distance between GNSS/LEO. | GNSS Satellite Attitude Solution Model, GNSS transmitter position instrument ECEF/ECI conversion, Orbital polynomial interpolation, Signal propagation time calculation (Matlab) |
| COSMIC-2 receiver clock bias removal for excess phase calculation | COSMIC-2 carrier phase and receiver phase model(opnGns), 1 Hz POD observation (RINEX); Corrected receiver time tag, geometric range | Receiver clock bias and geometric range removed excess phase | Single differencing for ionospheric-free, position-free receiver clock bias calculation (Matlab); low pass filtering; |
| GNSS transmitter clock bias removal for excess phase calculation | Receiver Clock bias removed from the excess phase; Corrected transmitter time tag; CODE GNSS clock products | Receiver clock and GNSS clock bias and geometric range removed excess phase | Zero differencing; 4th Order polynomial interpolation (Matlab) |
| Excess Phase Cycle Slip correction and Navigation Bit Demodulation | Cleaned excess phase; GNSS navigation bit time series; Climatology (ROPP) | Cleaned excess phase | Multi-screen phase model with ROPP; Closed-loop cycle slip correction (Matlab); Open loop NDM and cycle slip correction with phase model (Matlab). |
| Other corrections | Receiver ambient multi-path; Phase wind up | Excess phase; corrected excess phase | Evaluated, not included. |
| Quality Control (Excess Phase) | Excess phase/position/time tag/SNR | NetCDF file with excess phase, transmitter/receiver position in ECI, receiver time tag in GPS seconds | Slight quality control, no output when excess phase not complete, e.g., broken time series. |
| Bending Angle Calculation | NetCDF with excess phase, positions, SNRs, and time tag | Bending angle/impact height, refractivity, the dry temperature at mean sea level, channels L1/L2, and optimal bending angle. | Interface implementation to ROPP |
| Quality Control (Bending Angle) | Bending angle/refractivity profiles | Quality flag (good/bad) insertion | Forward Operator using ROPP and ECMWF/ERA5, 15 sigma criteria in bending angle comparison. |
| Relative Percent (%) | GPS L2C | GPS L2P | GLONASS | |||
|---|---|---|---|---|---|---|
| UCAR | ERA5 | UCAR | ERA5 | UCAR | ERA5 | |
| BA | −0.04 ± 1.15 | −0.34 ± 2.96 | −0.04 ± 1.32 | −0.28 ± 3.81 | −0.06 ± 1.15 | −0.09 ± 3.20 |
| Refractivity | −0.02 ± 0.83 | −0.04 ± 1.06 | −0.04 ± 0.97 | 0.09 ± 176 | −0.05 ± 0.91 | 0.03 ± 1.08 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, B.; Ho, S.-p.; Cao, C.; Shao, X.; Dong, J.; Chen, Y. Verification and Validation of the COSMIC-2 Excess Phase and Bending Angle Algorithms for Data Quality Assurance at STAR. Remote Sens. 2022, 14, 3288. https://doi.org/10.3390/rs14143288
Zhang B, Ho S-p, Cao C, Shao X, Dong J, Chen Y. Verification and Validation of the COSMIC-2 Excess Phase and Bending Angle Algorithms for Data Quality Assurance at STAR. Remote Sensing. 2022; 14(14):3288. https://doi.org/10.3390/rs14143288
Chicago/Turabian StyleZhang, Bin, Shu-peng Ho, Changyong Cao, Xi Shao, Jun Dong, and Yong Chen. 2022. "Verification and Validation of the COSMIC-2 Excess Phase and Bending Angle Algorithms for Data Quality Assurance at STAR" Remote Sensing 14, no. 14: 3288. https://doi.org/10.3390/rs14143288
APA StyleZhang, B., Ho, S.-p., Cao, C., Shao, X., Dong, J., & Chen, Y. (2022). Verification and Validation of the COSMIC-2 Excess Phase and Bending Angle Algorithms for Data Quality Assurance at STAR. Remote Sensing, 14(14), 3288. https://doi.org/10.3390/rs14143288









