Progress in Compact HF Radar Measurement of Bimodal Ocean Wave Parameters

Abstract

We describe an extension of methods used for the derivation of wave information from compact high frequency radar systems and give examples of observations which are compared to measurements from wave buoys close to the radar coverage area. Previous methods were based on the application of the Pierson–Moskowitz wave energy model in the analysis of radar spectra. We describe the extension of the methods to apply a bimodal ocean wave spectral model which includes two different spectra covering different wave frequencies, e.g., from swell and wind-waves. Results are presented from data sets obtained from radar stations located in the USA and Portugal.

1. Introduction

High frequency radar devices have been used for the remote measurement of sea surface parameters since Crombie [1] first identified the distinctive features of sea echo Doppler spectra. Barrick [2] modeled the first-order scatter of radar waves from ocean waves of half the radar wavelength to obtain data on surface current velocities. and provided an analysis of the second order spectral echoes that surround the first-order peaks [3], showing that they can be interpreted to provide detailed information on the ocean-wave spectrum.

In this paper, we describe advances in the process of analysis to produce ocean wave parameters from broad beam systems [4]; data is available from many SeaSonde radars, which have been available commercially for 3 decades. The SeaSonde has three coaxially located small antennas, a pair of crossed loops and a monopole. The interpretation of the signalyield these antennas yields both surface current velocities and directional ocean wave parameters. Wave parameters are derived from the portion of the weaker second-order radar Doppler echo surrounding the first-order Bragg peaks, which is obtained from semi-circular range cells surrounding the radar system. The analysis of radar data for wave information is therefore restricted to closer ranges than for currents. At present, wave information is derived from radar echo from single range cells, assuming that the ocean wave spectrum is homogeneous over the area covered. When wave fields are not homogeneous around the semi-circular range cells, the analysis methods produce an average of the output parameters.

Methods have been developed to produce swell and wind-wave results from phased array radars; these methods are described in [5,6,7,8,9,10], along with a comparison to model results and buoy measurements. We also note that bimodal ocean wave monitoring from spaceborne remote sensing devices provides the powerful monitoring of swells propagating across the ocean [11,12].

Lipa and Nyden [13] used a Pierson–Moskowitz wave energy model to interpret the radar echo Doppler spectrum. An analysis of the second-order spectrum produces estimates of waveheight, centroid period and direction. This method has been in use worldwide for over a decade. Examination of the second-order Doppler spectral region reveals that the frequency spectrum can display two or more distinct sections, produced for example by swell and wind waves. “Swell” refers to waves that come from distant storms. With the passage of time, the longest period waves outrun the shorter ones, as the wave phase velocity increases with its period. In the radar coverage area, the swell mixes with wind-generated waves for shorter periods, resulting in the development of a bimodal sea state. The second-order radar echo then contains two frequency regions: region (i) from a swell that came from a distant area and region (ii), from locally generated wind waves. A previous analysis for wave parameters has been extended [14] to deal with this bimodal situation. This paper describes recent progress in handling bimodal wave scenarios with SeaSondes and presents results from SeaSondes located on U.S. and Portuguese coasts.

Wind-waves produce a broad second-order echo in the radar Doppler spectrum, appearing as sidebands surrounding the first-order peaks. The dominant second-order echo arises from interaction of the radar beam with pairs of ocean wave trains. An analysis of the Doppler frequency distribution of these pairs [15,16] shows that the second-order spectrum close to the first-order Bragg spectrum is produced by waves with longer periods and that further away is produced by shorter ocean waves, typically locally generated wind-waves. Swell waves usually approach the shore, which results in the dominant echoes occurring at positive Doppler frequencies [13]. Figure 1 shows examples of the radar spectra from Bodega Bay, California and Sagres, Portugal, which demonstrate separated regions in the Doppler second-order structure, occurring when swell waves are present.

2. Materials and Methods

The theory of HF radar spectra and the methods used to derive ocean wave parameters from the radar spectra has been outlined in [14] and are briefly summarized here.

The ocean wave spectrum is defined in terms of the wavenumber k and azimuth angle $\phi .$ We use as a model for the wind-wave spectrum, $S_{ww}\left(k,\phi \right)$, the product of a nondirectional spectrum based on the Pierson-Moskowitz model and a cardioid directional distribution around the dominant direction ${\phi }_{ww}$:

$$S_{ww}\left(k,\phi \right)=\frac{A_{ww }{e}^{-0.74{\left(k_c/k\right)}^2}}{k^4} co{s}^4\left(\frac{\phi -{\phi }_{ww}}{2}\right)$$

(1)

with parameters k_c, ${\phi }_{ww}$ and a multiplicative constant $A_{ww }$. The waveheight, centroid period and direction can be defined in terms of the model parameters.

When the radar spectrum exhibits narrow swell peaks, these are interpreted using a simple model for the ocean wave swell spectrum, $S_{sw}\left(f,\phi \right)$, at wave frequency $f$ and azimuth angle $\phi$ given by:

$$\begin{array}{c}{S}_{sw}\left(f,\phi \right)=A_{sw }\left(f\right)\delta \left(\phi -{\phi }_{sw}\right), \lfloor f-f_{sw}\rfloor <\mathsf{\Delta }\\ =0 \lfloor f-f_{sw}\rfloor > \mathsf{\Delta } \end{array}$$

(2)

where swell is defined to have a single direction ${\phi }_{sw}$ and $\mathsf{\Delta }$ defines the width of the swell peak centered at wave frequency $f_{sw}$. The function $A_{sw }$ is an isosceles triangle that peaks at the swell period and decays to zero at the boundary.

Barrick’s equations [2,3] describe the narrow-beam first and second-order radar cross sections at a given frequency and azimuthal direction in terms of the ocean wave spectrum. For a broad beam system, these cross-sections need to be convolved with the antenna patterns. The basic data set measured by the three broad-beam antennas is converted into complex voltage cross spectra that can then be used along with the measured antenna patterns to derive ocean wave results. The broad-beam radar echo spectra from circular range cells over the coverage area are analyzed to produce ocean wave parameters.

The first- and second-order frequency regions are separated, and the first-order spectrum is analyzed to give the ocean-wave spectrum at the Bragg wavenumber. Proceeding with bimodal analysis, the second-order echo spectrum is separated into two frequency regions: region (i) close to the first-order Bragg region, which is produced by long-period waves (e.g., swell) and region (ii), further displaced from the Bragg region produced by shorter-period waves (e.g., wind-waves). The frequency boundary between these regions is found by first identifying the spectral maxima of the two regions in the monopole data and then finding the Doppler frequency F_B of the spectral minimum between them.

The frequency spectra in the two regions are then fitted with the ocean wave spectral models given by (1), (2) to find the optimum wave parameters. Doppler spectra in region (ii) (produced by shorter-period waves) are fit to the Pierson–Moskowitz model. Doppler spectra in region (i) (produced by longer-period waves) are fitted in turn to the triangular swell model defined by (2) and then the Pierson–Moskowitz model defined by (1). The wave model that produces the optimum fit is identified using the least squares technique in which the optimum parameters are found by minimizing the sum of the squares of the residuals between the observed value and the fitted value provided by the model.

The ocean wave parameters derived from each Doppler spectra using this process are waveheight, period and direction for both swell and wind-waves, as well as the total waveheight. Gaps in estimates of swell wave parameters result when the least-squares analysis indicates only wind-waves are present, i.e., when the optimum fit is provided by the Pierson–Moskowitz model for all the second-order radar spectrum.

In the next section, results are given from the application of these techniques to measured radar spectra from sites on the US East and West coasts and in Portugal.

3. Results

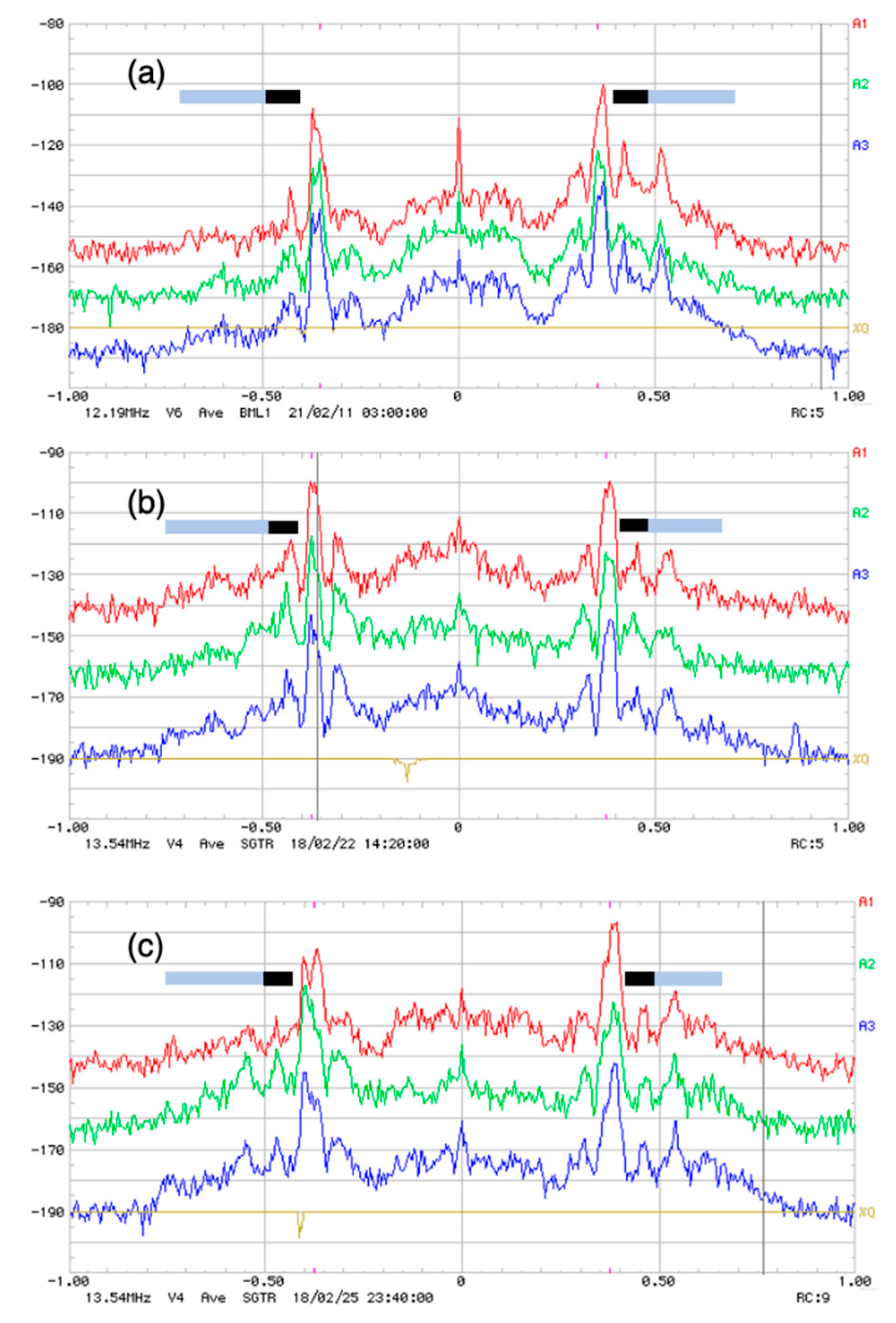
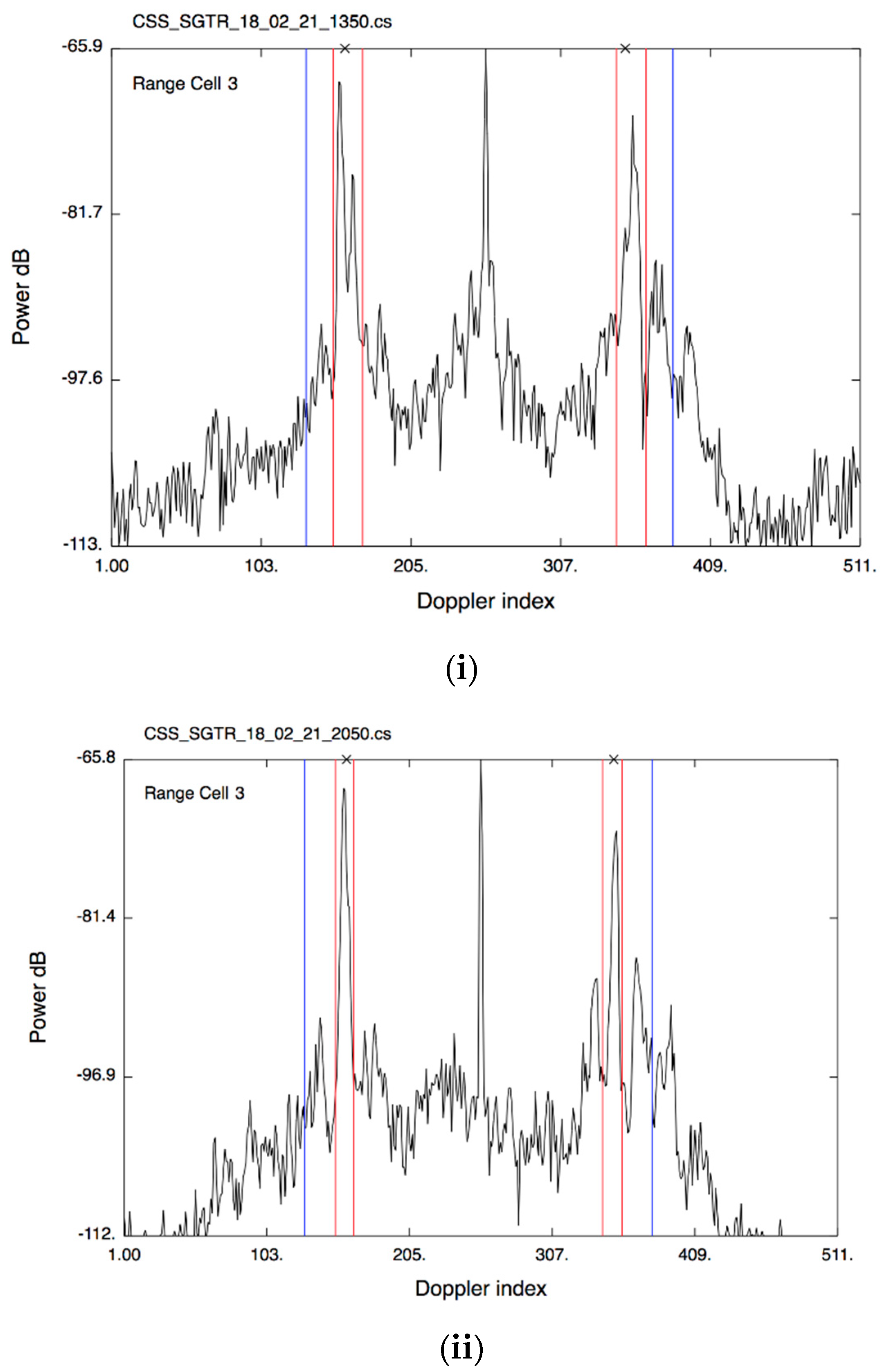
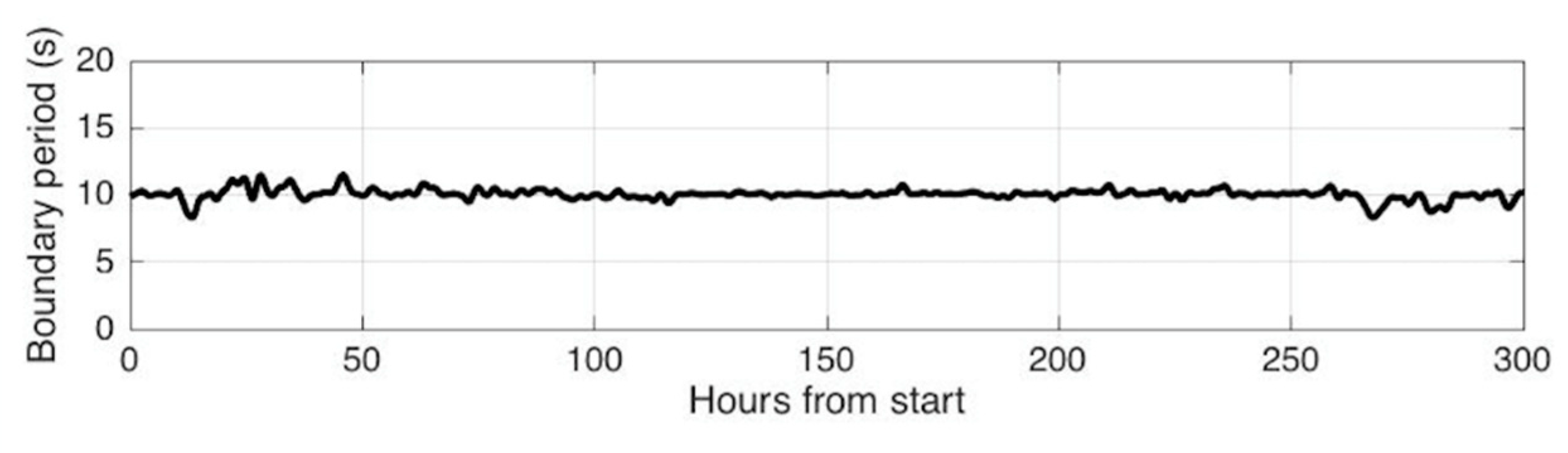
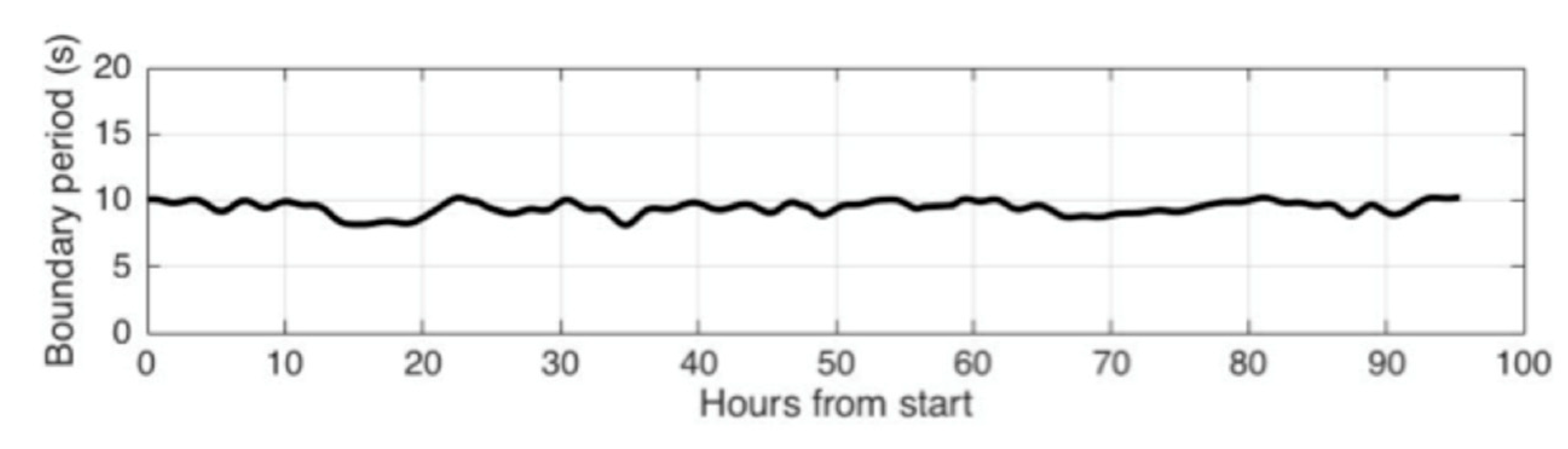
3.1. Derived Frequency Boundaries

Figure 2 shows examples of calculated frequency boundaries between Regions (i) and (ii) in the radar echo spectra at Sagres, Portugal, which is located at 36°59.669′N, 008°56.954′W.

3.2. Bimodal Wave Results

We now present results obtained from extended data samples measured at three radar sites located at Bodega Bay, CA, USA (6–18 January 2021), Seaside Park, NJ, USA (29–31 January 2018) and Espichel, Portugal (12–15 March 2015). Results obtained for ocean wave parameters calculated from the radar measurements are plotted vs. time, with comparison to measurements from neighboring buoys, including buoy spectral data from Bodega Bay and Espichel. The frequency boundaries between spectral regions (i) and (ii) for the wave buoys were assumed to be the same as those obtained from the radar spectra.

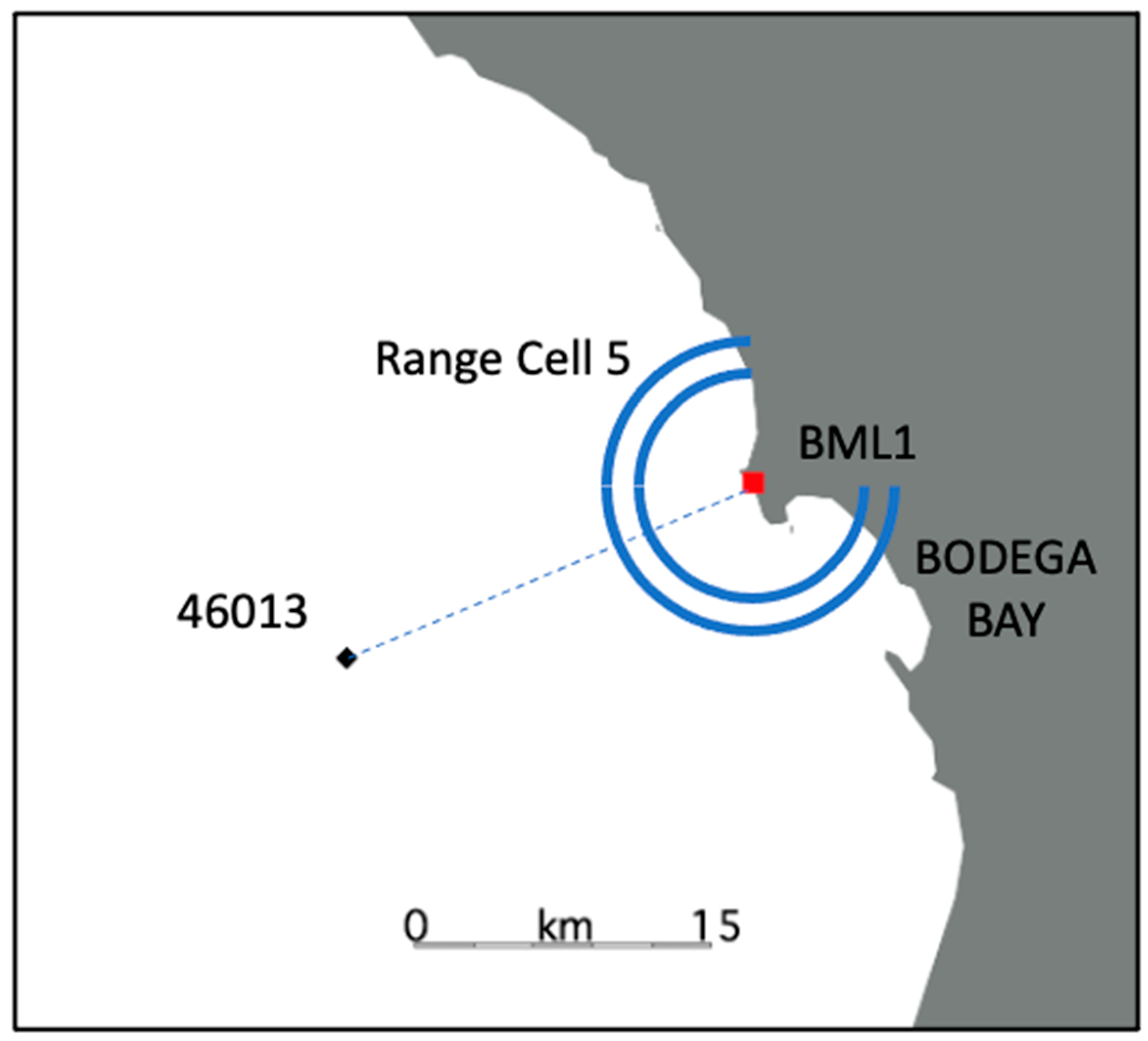
3.2.1. Results from Bodega Bay, California

The Bodega Bay SeaSonde, California (transmit frequency 12.16 MHz) is located at 38°19.039′N, 123°04.348′W. The locations of the radar and the NOAA wave buoy 46,013 are shown in Figure 3.

The offshore water depth ranges from 1 to 150 m over a 30 km range from the radar.

Figure 4 shows the time dependence of the boundary periods (1/F_B) between Regions (i) and (ii) calculated from the radar monopole spectra.

Figure 5 shows the measured spectral wave parameters obtained every 10-min from the Bodega Bay radar, hourly and from the NDBC 46,013 spectral wave data.

3.2.2. Results from Seaside Park, New Jersey

The Seaside Park radar (transmit frequency 13.4 MHz) is located at 39°55.950′N, 074°04.353′W. The locations of the radar and the NOAA wave buoy 44,091 are shown in Figure 6. The offshore water depth ranges from 1 to 30 m over a 30 km range from the radar.

Figure 7 shows the time dependence of the boundary periods (1/F_B) between Regions (i) and (ii), calculated from the radar monopole spectra.

Figure 8 shows the measured spectral wave parameters obtained every 10-min from the Seaside Park radar from the NOAA data buoy NDBC 46,091.

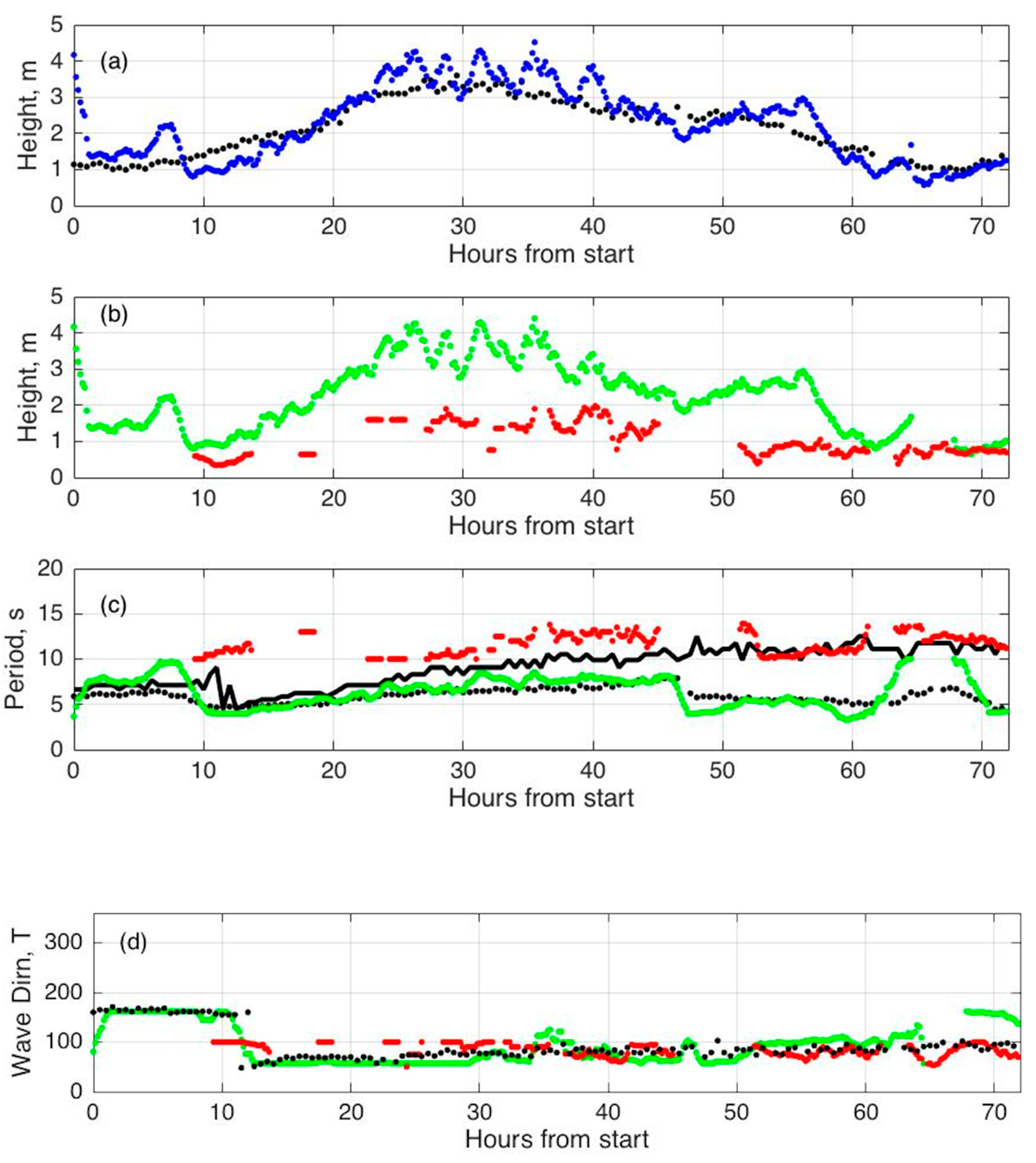
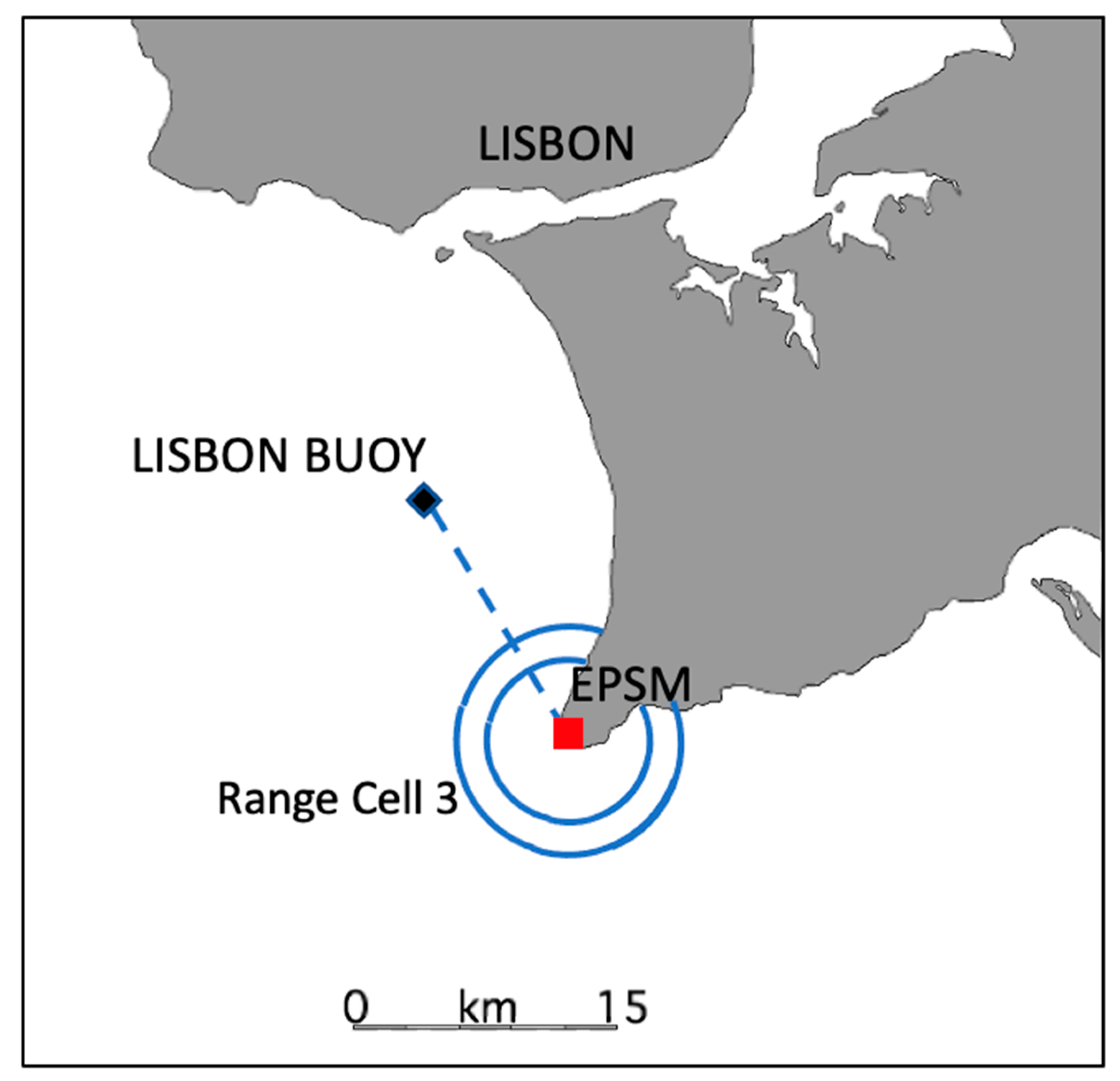
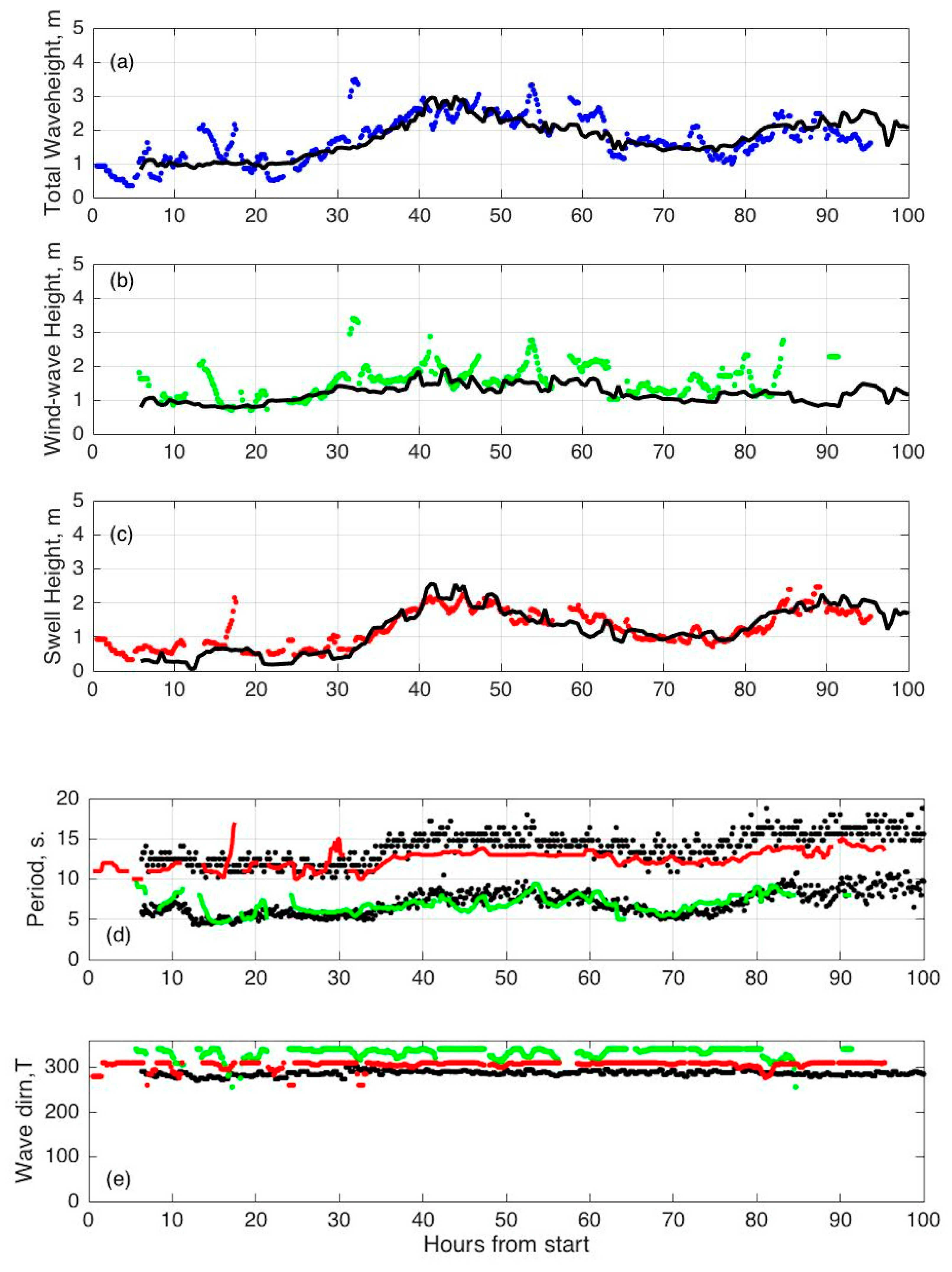
3.2.3. Wave Results from Espichel, Portugal

The Espichel SeaSonde (transmit frequency 12.92 MHz) is located at 38°24.928′N, 9°13.001′W. The locations of the radar and the Lisbon buoy are shown in Figure 9. The offshore water depth ranges from 1 to 120 m over a 20 km range from the radar.

Figure 10 shows the time dependence of the boundary periods (1/F_B) between Regions (i) and (ii) calculated from the radar monopole spectra.

Figure 11 shows the measured spectral wave parameters obtained from the radar at Espichel, Portugal and from Lisbon buoy wave data.

4. Discussion

Results obtained from the analysis of radar echo spectra represent spatial averages over circular range cells, while buoys measure waves at a single point, which may be some distance from the radar. These differences limit the extent to which detailed agreement between the two sets of measurements can be expected. Improved comparisons would require the deployment of multiple sensors within a given radar range cell. Similar results are obtained typically from several range cells for which the second-order echo exceeds the noise floor.

We have described the extension and testing of analysis methods to handle more complex bimodal ocean wave scenarios involving both swell and wind-waves. Examples were given showing the effect of bimodal waves on the radar echo spectrum, which is used to provide the boundary periods between the ocean wave components. Further analysis involves the least-squares fitting of two ocean wave spectral models to measure radar echo spectra. When the analysis indicates bimodal sea spectrum, the algorithm provides heights, directions and periods for both swell and wind-waves. Results are shown for three radar sites in Portugal and USA, which are in good agreement with neighboring buoy observations, including buoy spectral data when available. Optimal ocean wave parameters can be provided automatically for each measured radar echo spectrum. This ocean wave information can now be obtained from a shore-based radar system; no offshore instrumentation is required, greatly reducing maintenance issues.

Future improvements involve the use of more realistic swell spectral models and more robust minimum detection algorithms. Progress could also be made in the calculation of wave parameters in optimized area bands rather than the circular range cells used at present. Further downstream, it may be useful to incorporate wind forecasts in the analysis to reduce uncertainties. It would also be interesting to study the period of the boundary between the ocean wave components obtained from wave buoys.