Ultra-Low Sidelobe Waveforms Design for LPI Radar Based on Joint Complementary Phase-Coding and Optimized Discrete Frequency-Coding
Abstract
:1. Introduction
- (1)
- Using the characteristics of complementary inter-code cancellation and zero autocorrelation sidelobe, the phase-coding waveform is designed to increase the peak sidelobe ratio of the transmitted waveform. GA optimizes the DFC to form a new GADFC codeword, which increases the orthogonality between the codewords and reduces the autocorrelation sidelobe level.
- (2)
- A CPC-GADFC joint coding waveform based on LFM is designed. The joint coding waveform can make up for the drawbacks of a single modulation waveform, and enhance the radar waveforms’ LPI and anti-interference abilities to ensure the radar range and speed measuring resolution.
- (3)
- Through the combination of CPC and GADFC, the echo pulse compression of the radar transmit waveform has the characteristics of ultra-low sidelobes, and the main lobe width is narrower so that the designed waveform has more advantages in target detection.
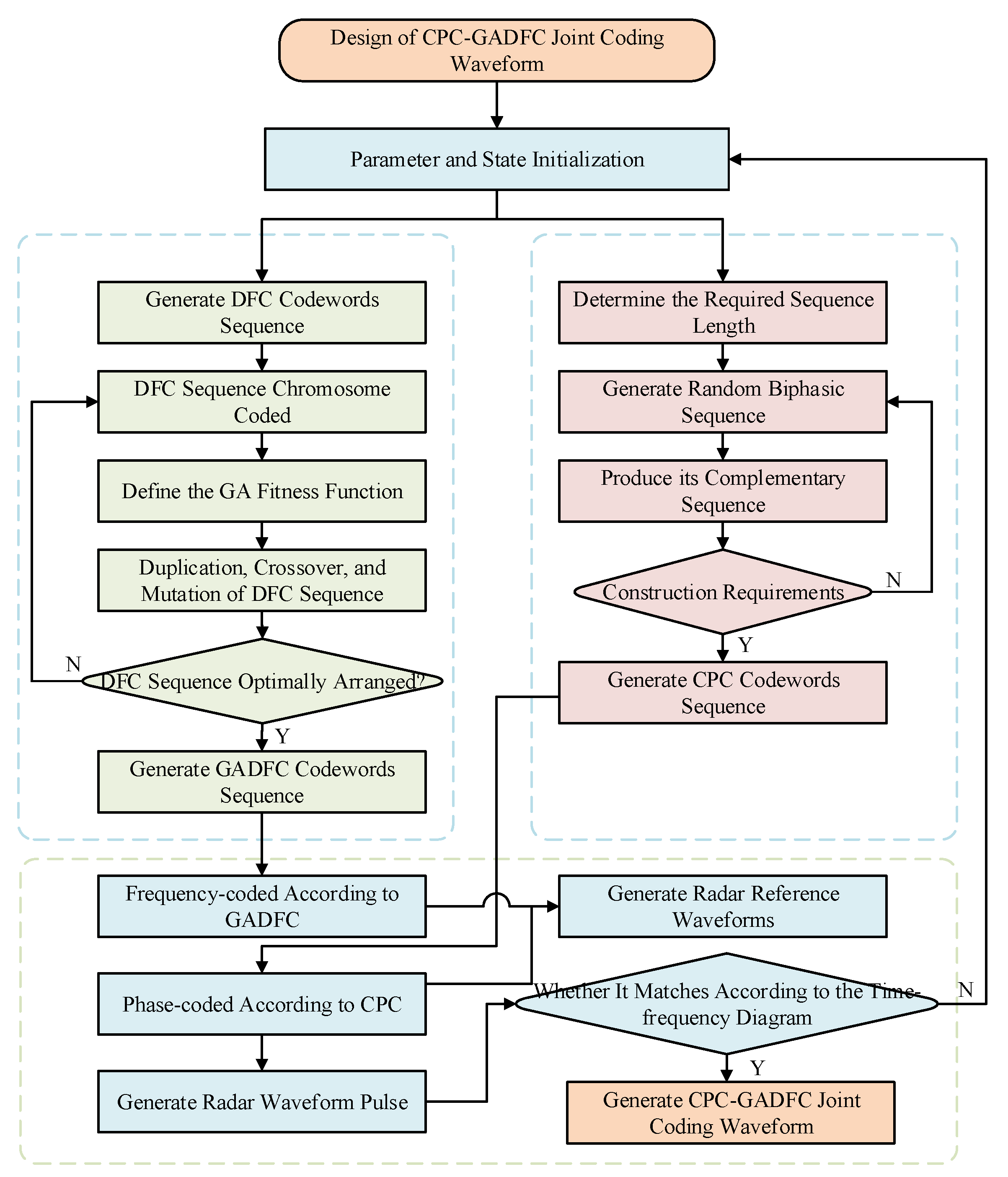
2. Algorithm Model
2.1. Complementary Code Model
2.2. Genetic Algorithm
- (1)
- Chromosome coding. The optimal arrangement between DFC codewords is converted into a search space that the GA can process with Gray encoding. Since the codewords of DFC are decimal, it is necessary to first convert them into binary representation. The variation range of the DFC codewords is , the encoding length is l and the encoding precision of the binary encoding is . The conversion relationship between the DFC binary codewords and its decimal is given by:where a is an argument between and b is a m-bit binary code represented as .Using Gray coding can enhance the local search ability of the GA. The Gray code corresponding to binary code B is . The conversion equation between binary code and Gray code is expressed as:where ⊕ denotes the exclusive-or operation, that is, when the two numbers are the same, it leads to 0, and when they are not, it leads to 1.
- (2)
- Define the fitness function and generate the initialization population. Before using the GA, the fitness function should be used to determine the final goal. In this study, the maximum Euclidean distance of DFC should be found. That is, the objective function is the optimal codeword combination mode to form a new GADFC codeword and achieve a lower sidelobe autocorrelation level. The objective function is given by:where represents the maximum Euclidean distance between two adjacent codewords after gray coding, passes through the fitness function, is the Gray codeword and is the target optimal codewords arrangement.In order to ensure the diversity of chromosomes in the population, while ensuring the regular operation of the genetic algorithm, the dispersion of chromosome fitness values is improved, and the high performance of the GA algorithm is ensured. It is necessary to transform the objective function into a function of the fitness function by an exponential transformation:where is the optimization objective function, is the fitness function and is the optimization coefficient which is usually a constant.
- (3)
- Select replication, crossover and mutation operations on the obtained population to generate the next generation population. This selection is the key to GA. It is based on the evaluation of individual fitness, while the purpose is to avoid gene deletion and improve the global convergence. The crossover consists in selecting a more significant value of the random number than the crossover probability for the next step. The mutation first selects some individuals from the population with a certain high probability, and performs the inverse operation on each chosen individual. In this paper, the probability of mutation is 0.001. The global search ability of the GA is mainly provided by selection, crossover and mutation. The mutation ensures that the algorithm can search every point from the problem space to the solution space, making the algorithm have global optimality and enhancing the GA’s robustness.
- (4)
- Determine whether the algorithm satisfies the stopping criterion. If it is not satisfied, then repeat step (3).
- (5)
- The algorithm ends, and the optimal GADFC codewords are obtained.
3. Cpc-Gadfc Joint Coding Waveform Design, Performance and Processing Method Analysis
3.1. Joint Coding Waveform Expression
3.2. Performance Analysis and Processing Method Design
3.2.1. Ambiguity Function Analysis
3.2.2. Lpi Characteristic Analysis
3.2.3. Analysis of the Algorithm Complexity
3.2.4. Signal Processing Flow
4. Result and Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| LPI | Low Probability of Intercept |
| UWB | Ultra-Wide Band |
| LFM | Linear Frequency Modulation |
| FSK | Frequency Shift Keying |
| PSK | Phase Shift Keying |
| CPC | Complementary Phase-Coding |
| DFC | Discrete Frequency-Coding |
| GA | Genetic Algorithm |
| GADFC | Discrete Frequency-Coding Optimized by Genetic Algorithm |
References
- Giubbolini, L. A multistatic microwave radar sensor for short range anticollision warning. IEEE Trans. Veh. Technol. 2000, 49, 2270–2275. [Google Scholar] [CrossRef]
- Ahmed, A.; Zhang, Y.D.; Hassanien, A. Joint Radar-Communications Exploiting Optimized OFDM Waveforms. Remote. Sens. 2021, 13, 4376. [Google Scholar] [CrossRef]
- Shi, C.; Wang, F.; Zhou, J.; Chen, J. Adaptive radar jamming waveform design based on low probability of intercept. In Proceedings of the 2015 IEEE China Summit and International Conference on Signal and Information Processing (ChinaSIP), Chengdu, China, 12–15 July 2015; pp. 1017–1021. [Google Scholar]
- Wang, W.Q. Moving-Target Tracking by Cognitive RF Stealth Radar Using Frequency Diverse Array Antenna. IEEE T. Geosci. Remote 2016, 54, 3764–3773. [Google Scholar] [CrossRef]
- Hejazikookamari, F.; Norouzi, Y.; Kashani, E.S.; Nayebi, M.M. A Novel Method to Detect and Localize LPI Radars. IEEE Trans. Signal Process. 2019, 55, 2327–2336. [Google Scholar] [CrossRef]
- Kamble, J.; Pasha, I.A.; Madhavilatha, M. Design of HRR detection system for measurement of RF signal power. In Proceedings of the 2016 10th International Conference on Intelligent Systems and Control (ISCO), Coimbatore, India, 7–8 January 2016; pp. 1–5. [Google Scholar]
- Guey, J.C.; Bell, M.R. Diversity waveform sets for delay-Doppler imaging. IEEE Trans. Inf. Theory 1998, 44, 1504–1522. [Google Scholar] [CrossRef]
- Abd, M.H.; Al-Suhail, G.A.; Tahir, F.R.; Ali Ali, A.M.; Abbood, H.A.; Dashtipour, K.; Jamal, S.S.; Ahmad, J. Synchronization of Monostatic Radar Using a Time-Delayed Chaos-Based FM Waveform. Remote. Sens. 2022, 14, 1984. [Google Scholar] [CrossRef]
- Hongbo, S.; Yilong, L.; Guosui, L. Ultra-wideband technology and random signal radar: An ideal combination. IEEE Aero. El. Sys. Mag. 2003, 18, 3–7. [Google Scholar] [CrossRef]
- De Witte, E.; Griffiths, H.D. Improved Waveforms for Satellite-Borne Precipitation Radar; IET Digital Library: London, UK, 2011. [Google Scholar]
- Dietl, G.; Wang, J.; Ding, P.; Zoltowski, M.D.; Love, D.J.; Utschick, W. Hybrid transmit waveform design based on beam-forming and orthogonal space-time block coding. In Proceedings of the (ICASSP ’05) IEEE International Conference on Acoustics, Speech, and Signal Processing, Philadelphia, PA, USA, 18–23 March 2005; pp. 893–896. [Google Scholar]
- Kassab, R.; Lesturgie, M.; Fiorina, J. Quasi-continuous waveform design for dynamic range reduction. Electron. Lett. 2008, 44, 646–647. [Google Scholar] [CrossRef] [Green Version]
- Geroleo, F.G.; Brandt-Pearce, M.; Brown, C.L. Detection and estimation of multi-pulse LFMCW radar signals. In Proceedings of the 2010 IEEE Radar Conference, Arlington, VA, USA, 10–14 May 2010; pp. 1009–1013. [Google Scholar]
- Zhang, Y.X.; Hong, R.J.; Pan, P.P.; Deng, Z.M.; Liu, Q.F. Frequency-Domain Range Sidelobe Correction in Stretch Processing for Wideband LFM Radars. IEEE Trans. Aerosp. Electron. Syst. 2017, 53, 111–121. [Google Scholar] [CrossRef]
- Bao, H.; Ziemann, A.; He, Z.S. Design and Measurements of MSK-LFM RadCom System. In Proceedings of the 2020 17th European Radar Conference (EuRAD), London, UK, 16–18 February 2021; pp. 9–12. [Google Scholar]
- Raghavendra, C.G.; Prajwal, B.R.; Sagar, G.D.; Keerthana, R.; Meghna, S.; Prasad, N.N.S.S.R.K. Reduction of Envelope Fluctuations in OFDM Radar Signals Using Coding Technique. In Proceedings of the 2018 International Conference on Communication and Signal Processing (ICCSP), Chennai, India, 3–5 April 2018; pp. 802–806. [Google Scholar]
- Li, Z.; Perera, S.; Zhang, Y.; Zhang, G.; Doviak, R. Phased-Array Radar System Simulator (PASIM): Development and Simulation Result Assessment. Remote. Sens. 2019, 11, 422. [Google Scholar] [CrossRef] [Green Version]
- Suh, J.; Lee, J.; Gil, G.T.; Hong, S. Time-and-Frequency Hybrid Multiplexing for Flexible Ambiguity Controls of DFT-coded MIMO OFDM Radar. IEEE Access 2021, 9, 137793–137808. [Google Scholar] [CrossRef]
- Park, B.; Ahn, J.M. Intra-pulse modulation recognition using pulse description words and complex waveforms. In Proceedings of the 2017 International Conference on Information and Communication Technology Convergence (ICTC), Jeju Island, Korea, 18–20 October 2017; pp. 555–560. [Google Scholar]
- Hou, J.; Tao, R.; Shan, T.; Qi, L. A novel LPI radar signal based on hyperbolic frequency hopping combined with Barker phase code. In Proceedings of the 7th International Conference on Signal Processing, Beijing, China, 31 August–4 September 2004; pp. 2070–2073. [Google Scholar]
- Saifullah, Y.; Yang, G.; Feng, X.U. A Four-leaf Clover-shaped Coding Metasurface For Ultra-wideband Diffusion-like Scattering. J. Radars 2021, 10, 382–390. [Google Scholar]
- Song, X. An Improved Algorithm for Pulse Compression of Taylor Four-phase Coding Signal and Its Simulation. Ship Electron. Eng. 2006, 06, 160–163. [Google Scholar]
- Chunhong, Y.; Zengli, L. The Superiority Analysis of Linear Frequency Modulation and Barker Code Composite Radar Signal. In Proceedings of the 2013 Ninth International Conference on Computational Intelligence and Security, Emeishan, China, 14–15 December 2013; pp. 182–184. [Google Scholar]
- Zhang, T.; Shuai, C.; Zhou, Y. Deep Learning for Robust Automatic Modulation Recognition Method for IoT Applications. IEEE Access 2020, 1, 117689–117697. [Google Scholar] [CrossRef]
- Agrawal, D.; Maheshwari, S. Design and implementation of current mode circuit for digital modulation. Integration 2021, 78, 118–123. [Google Scholar] [CrossRef]
- Kundu, N.K.; Mallik, R.K.; Mckay, M.R. Signal Design for Frequency-Phase Keying. IEEE Trans. Wirel. Commun. 2020, 1, 4067–4079. [Google Scholar] [CrossRef]
- Jahangir, M.; Baker, C.J.; Oswald, G.A. Doppler characteristics of micro-drones with L-Band multibeam staring radar. In Proceedings of the 2017 IEEE Radar Conference (RadarConf), Seattle, WA, USA, 8–12 May 2017; pp. 1052–1057. [Google Scholar]
- Golay, M. Complementary series. Information Theory. IRE Trans. 1961, 7, 82–87. [Google Scholar]
- Mott, M.H.; Roome, A.T.; Erickson, C.W. Signal Processing System Employing Reference-Signal Controlled-Integrator for Integrating Resultant of Two Summing-Circuits Having Complementary Inputs. U.S. Patent US3316492 A, 25 April 1967. [Google Scholar]
- Jing, X.U.; Ming-Hao, H.E.; Chen, C.X.; Zhou, L. Algorithm Complexity Analysis of Radar Emitter Characteristic Parameter Extraction. J. China Acad. Electron. Inf. Technol. 2013, 8, 43–47. [Google Scholar]
- Kang, B.; Rangaswamy, M. Radar Waveform Design Under Communication Sum Capacity Constraint. IEEE Trans. Signal Process. 2021, 69, 2795–2806. [Google Scholar] [CrossRef]
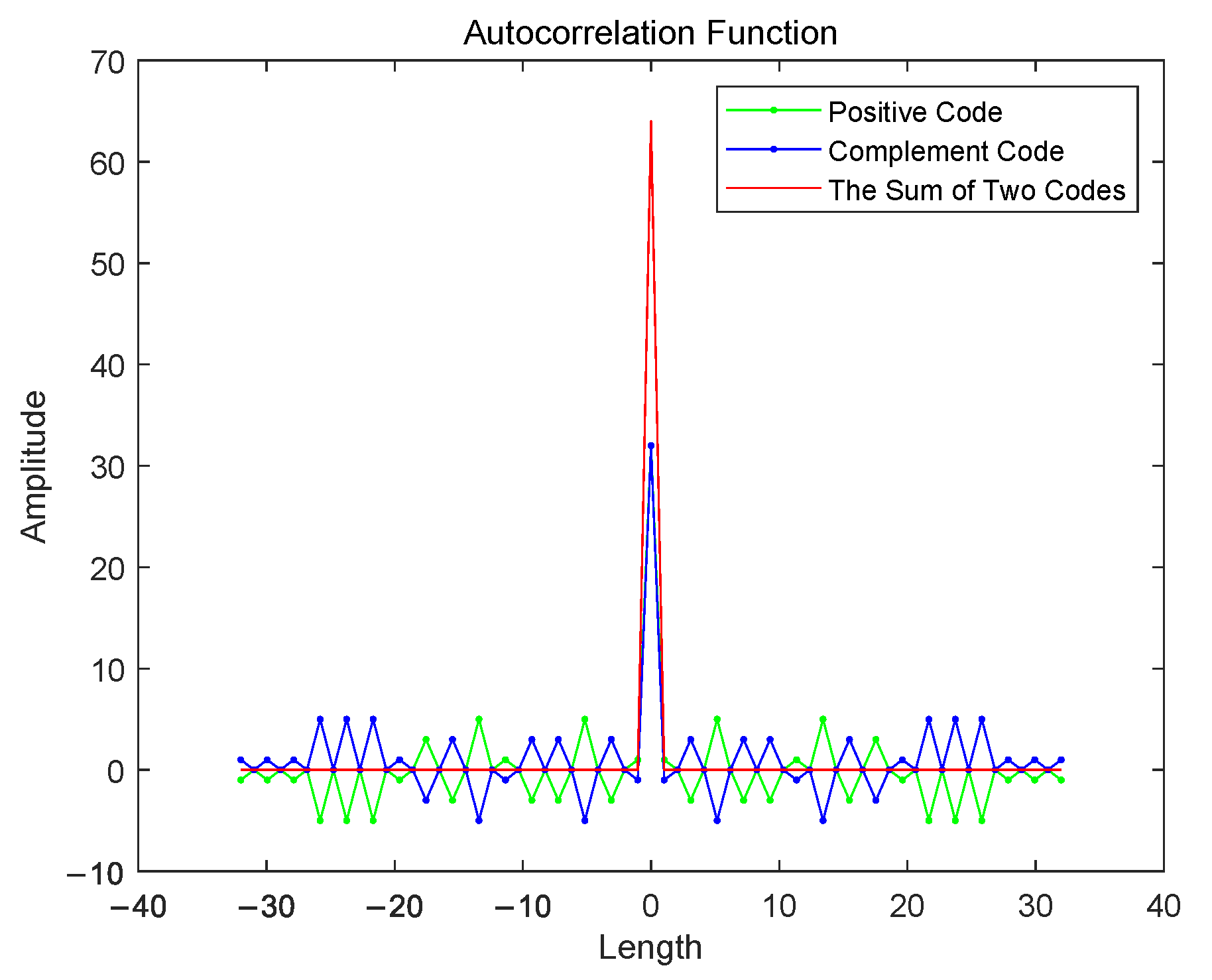


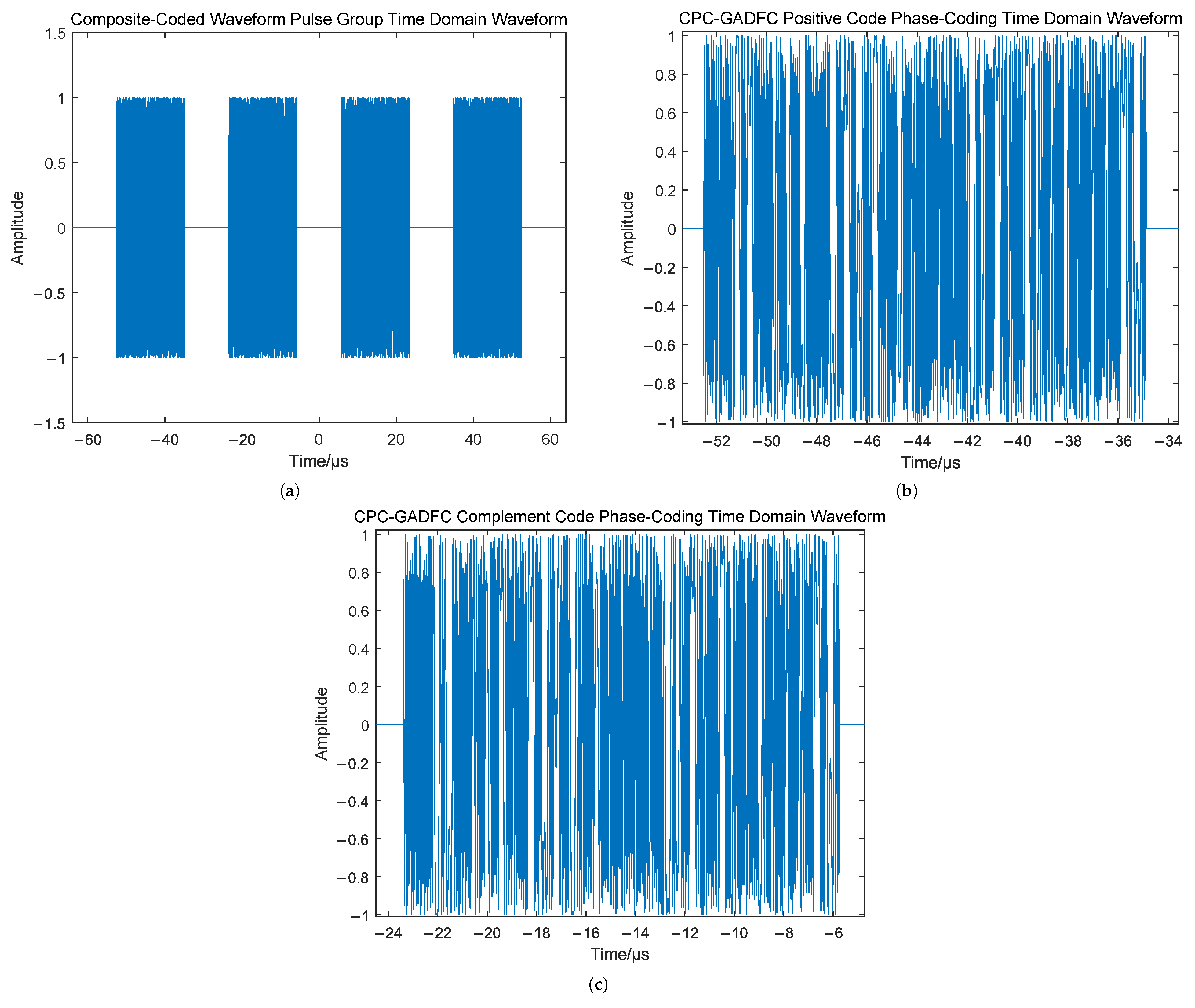
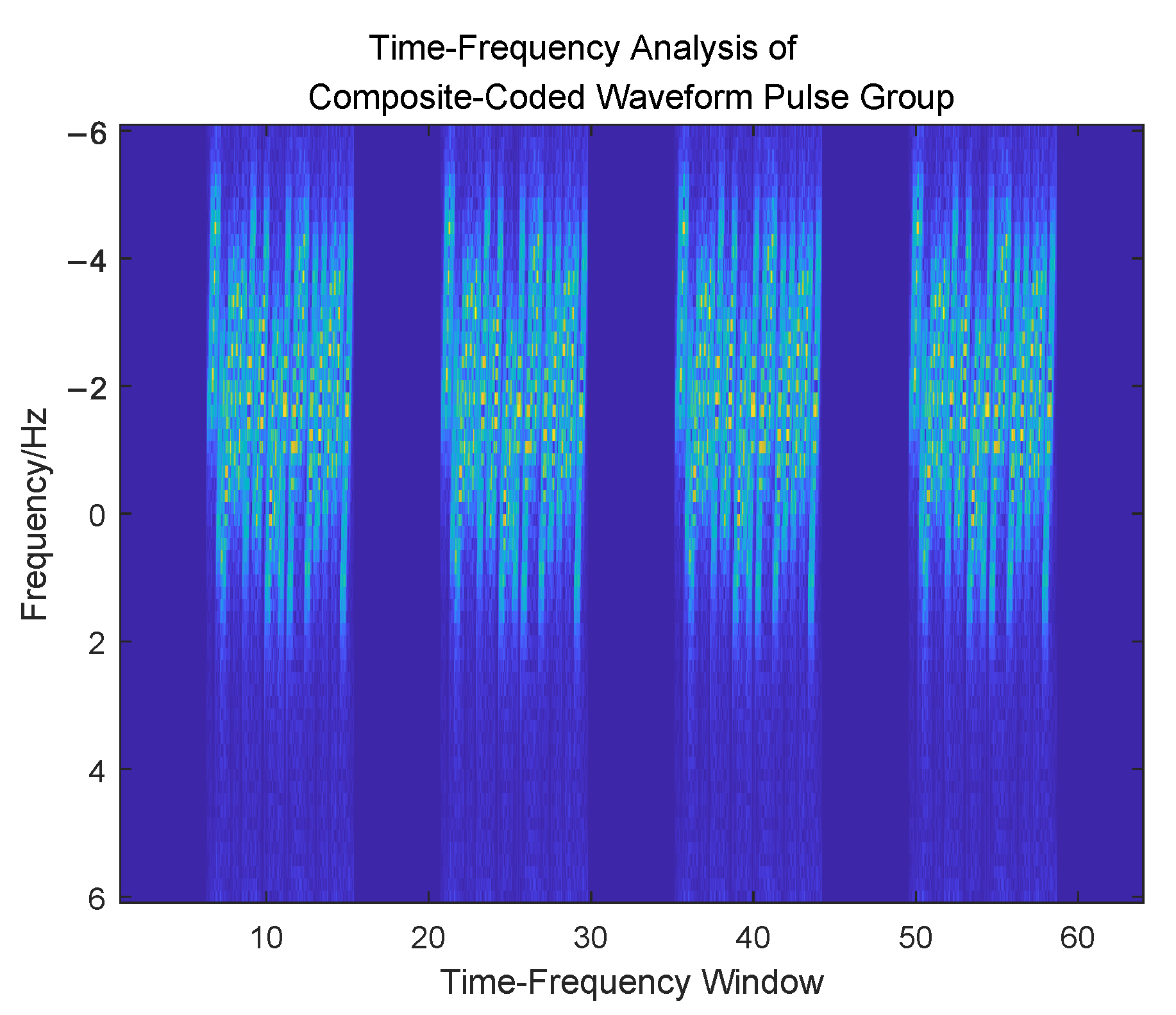
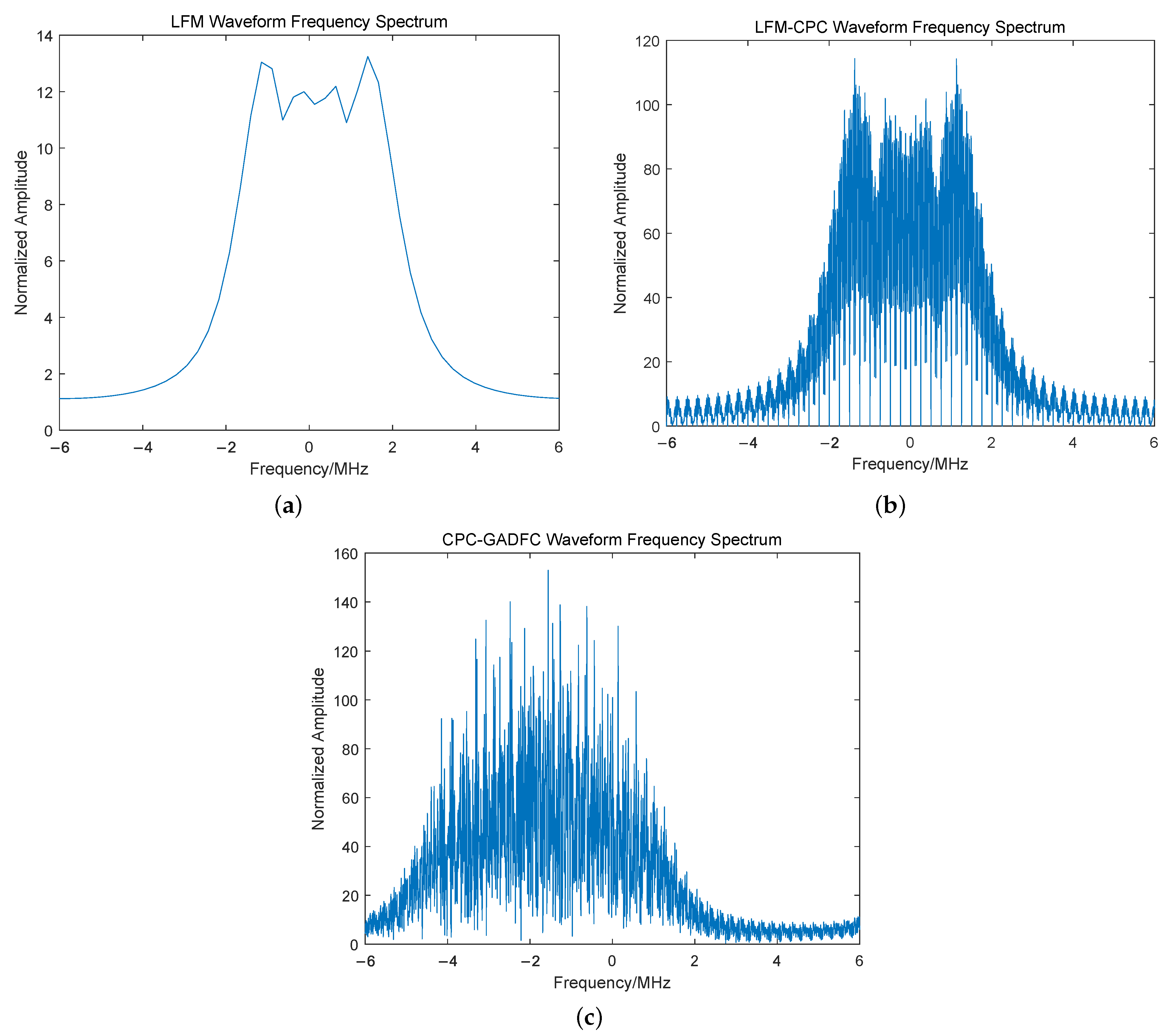
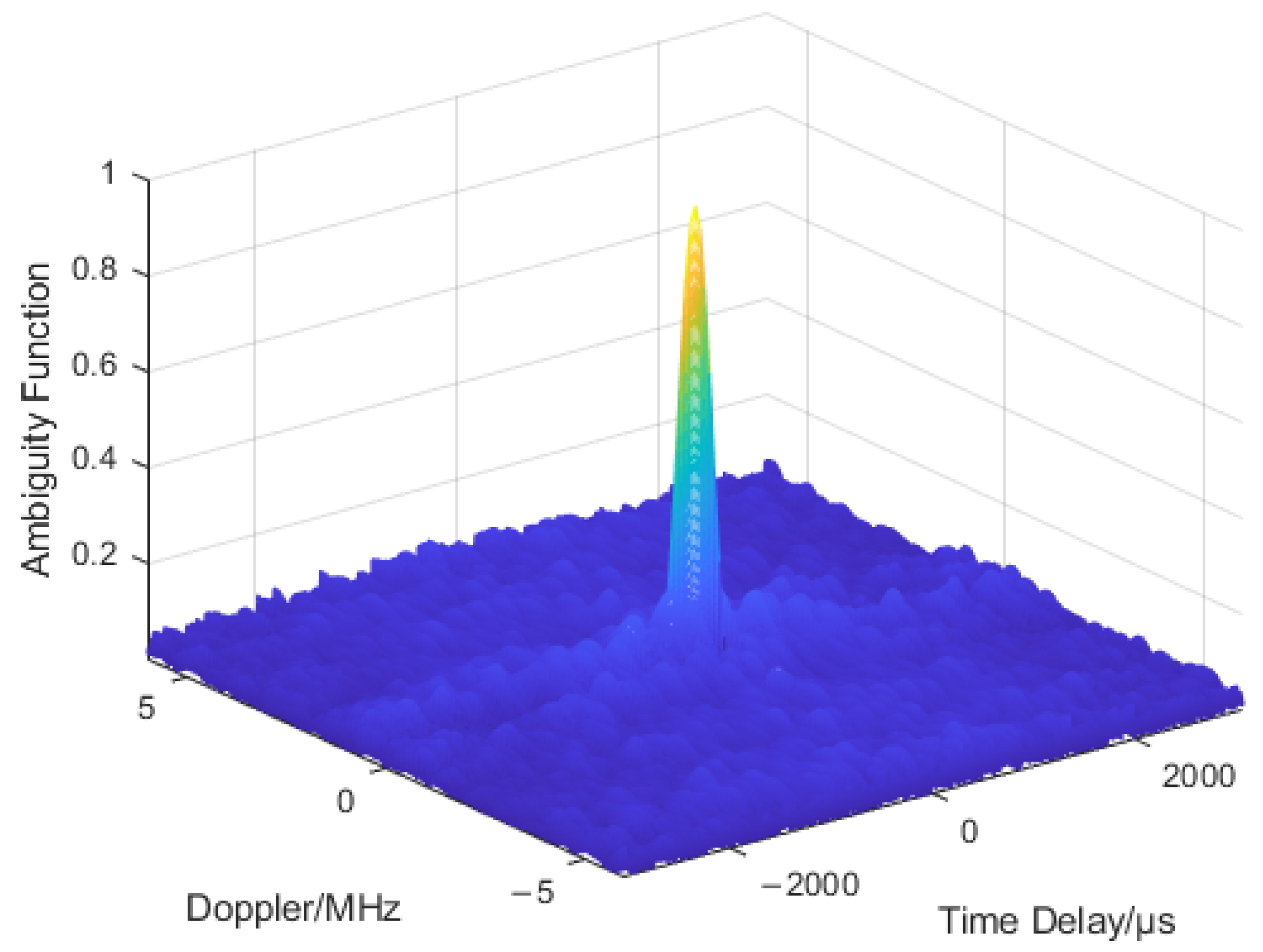
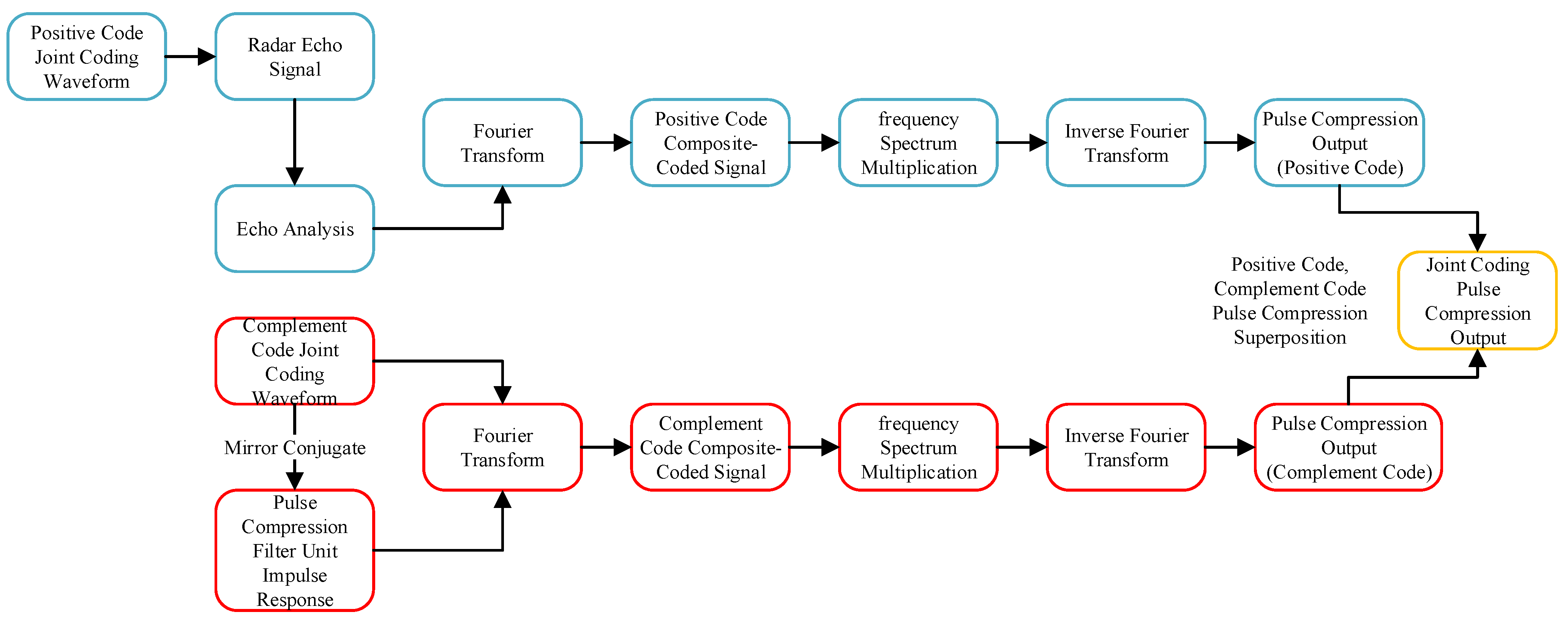

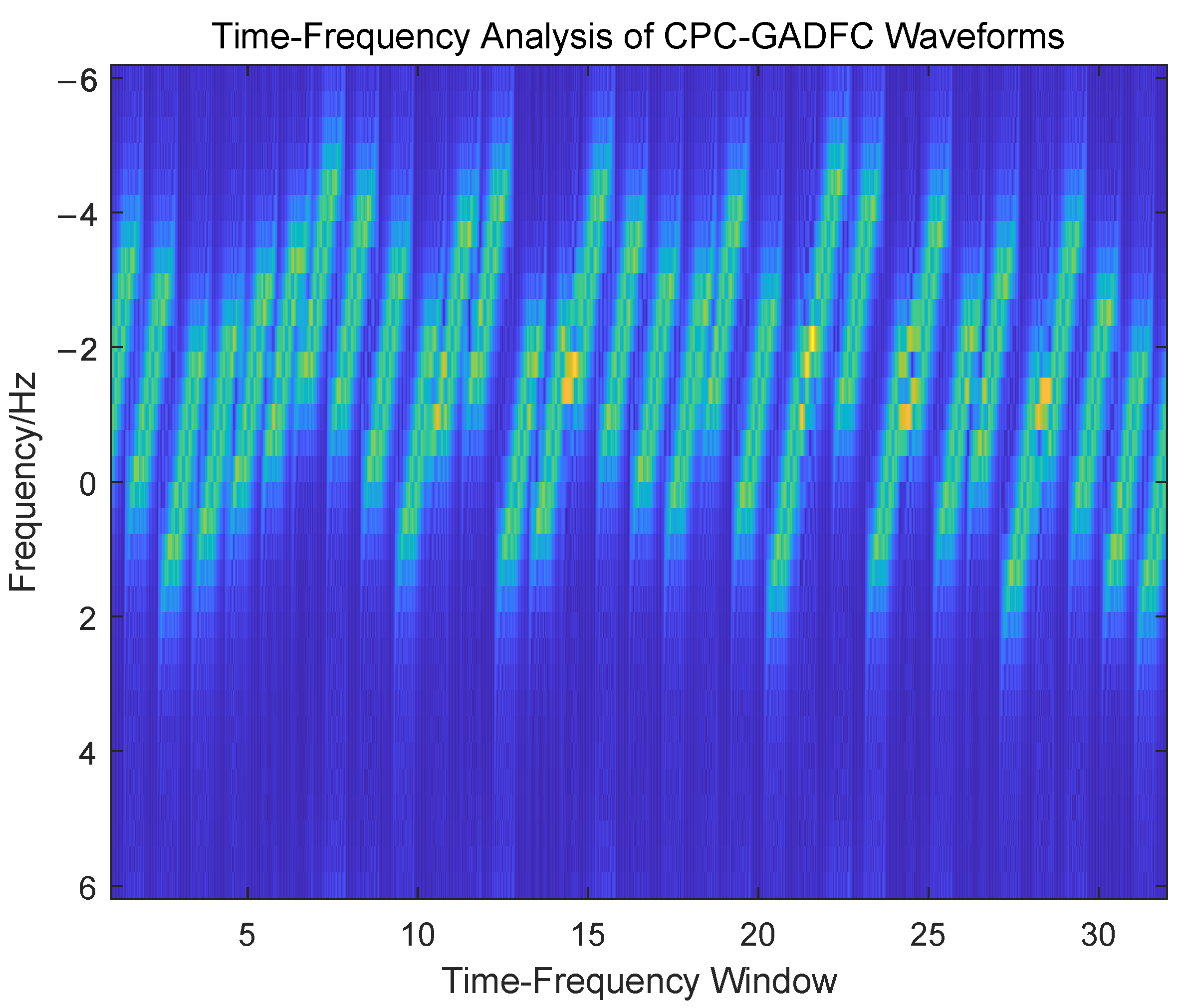
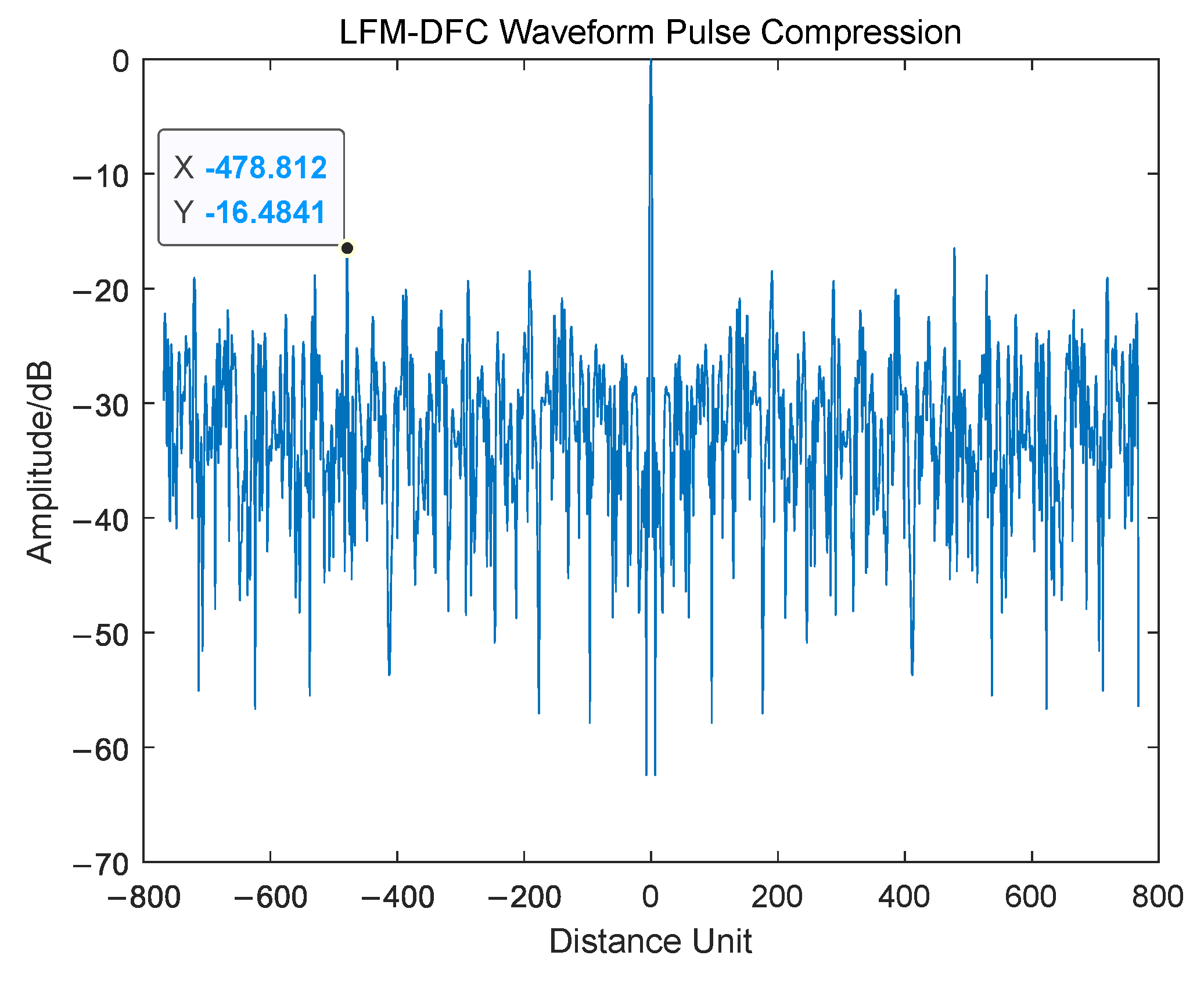
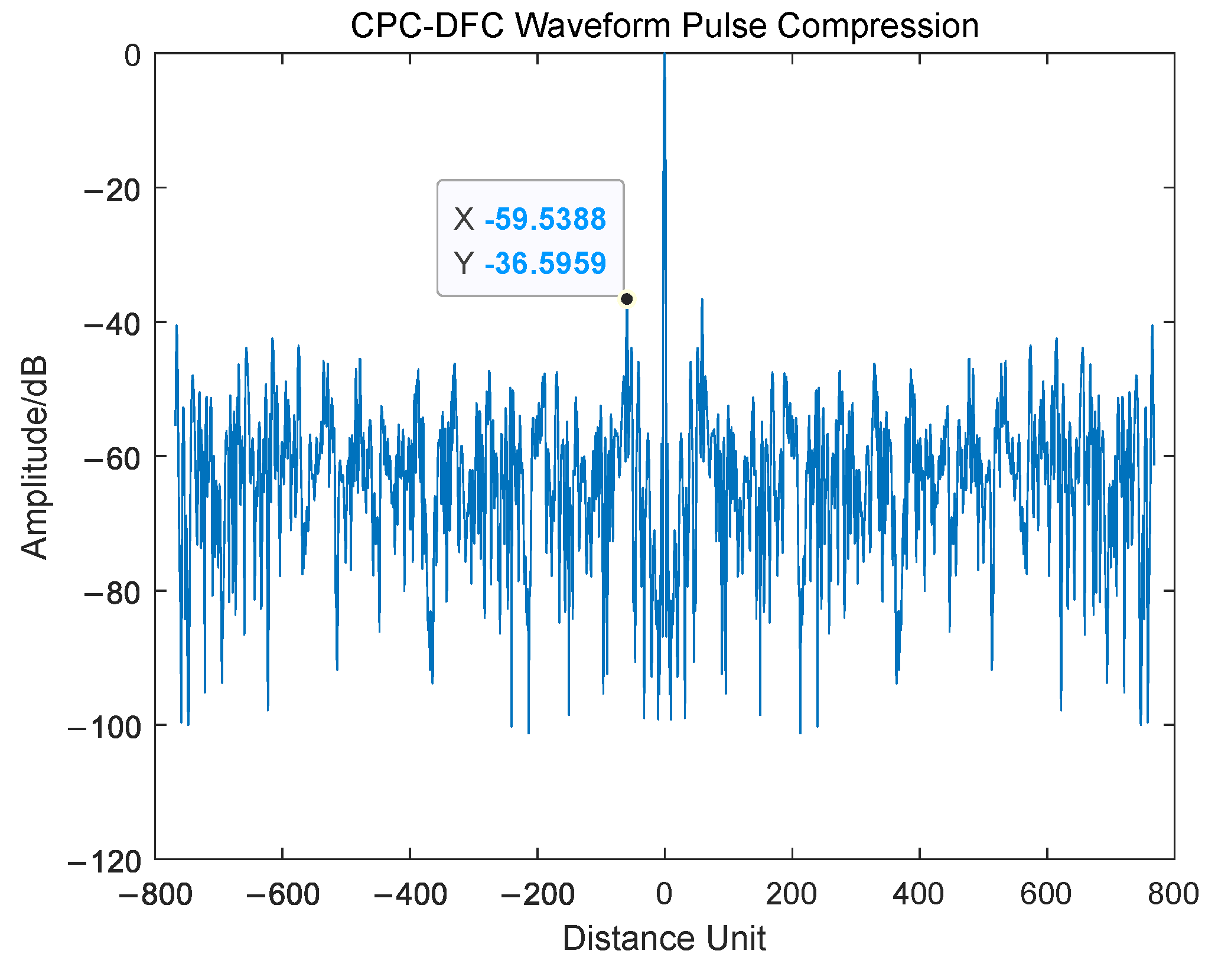
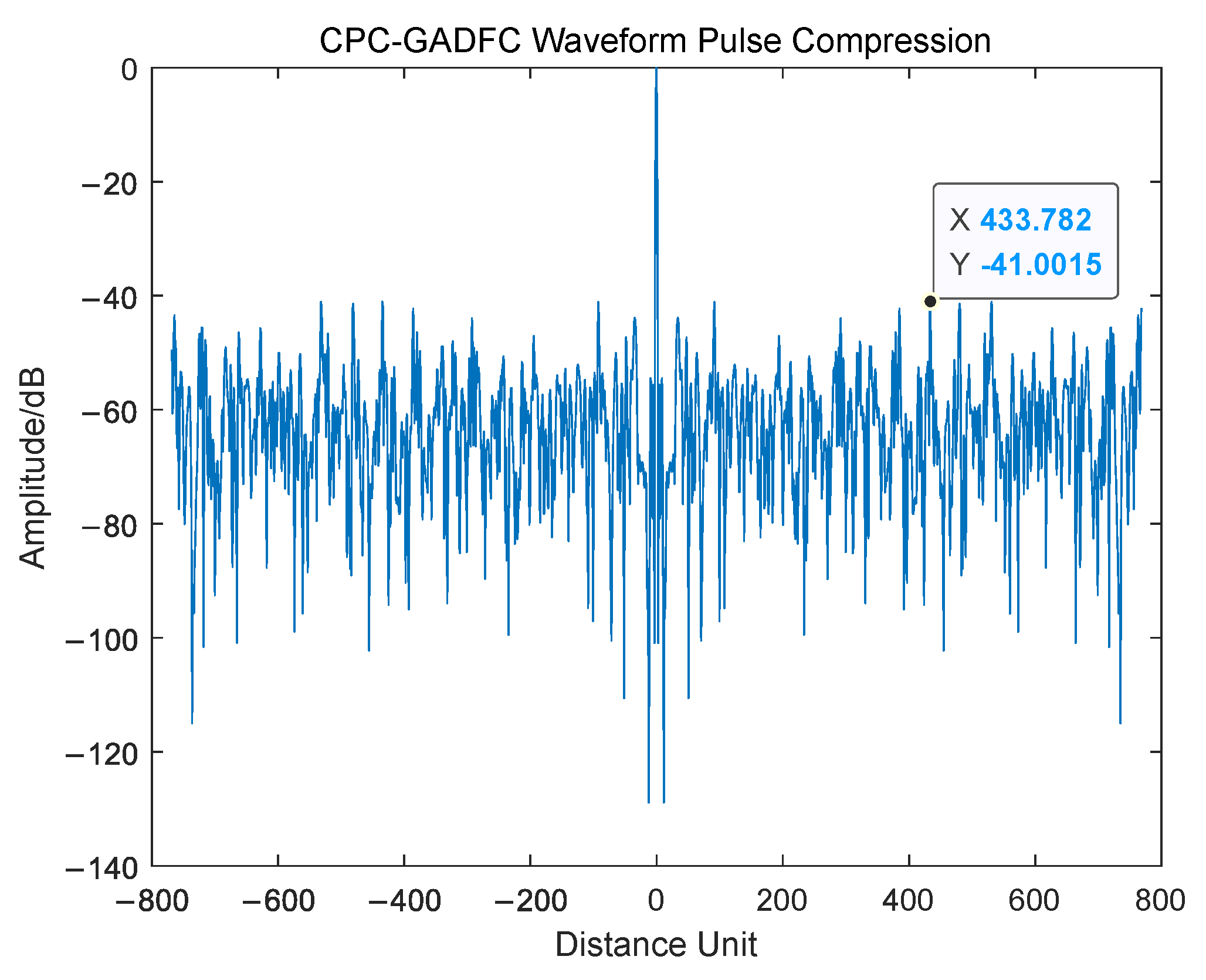
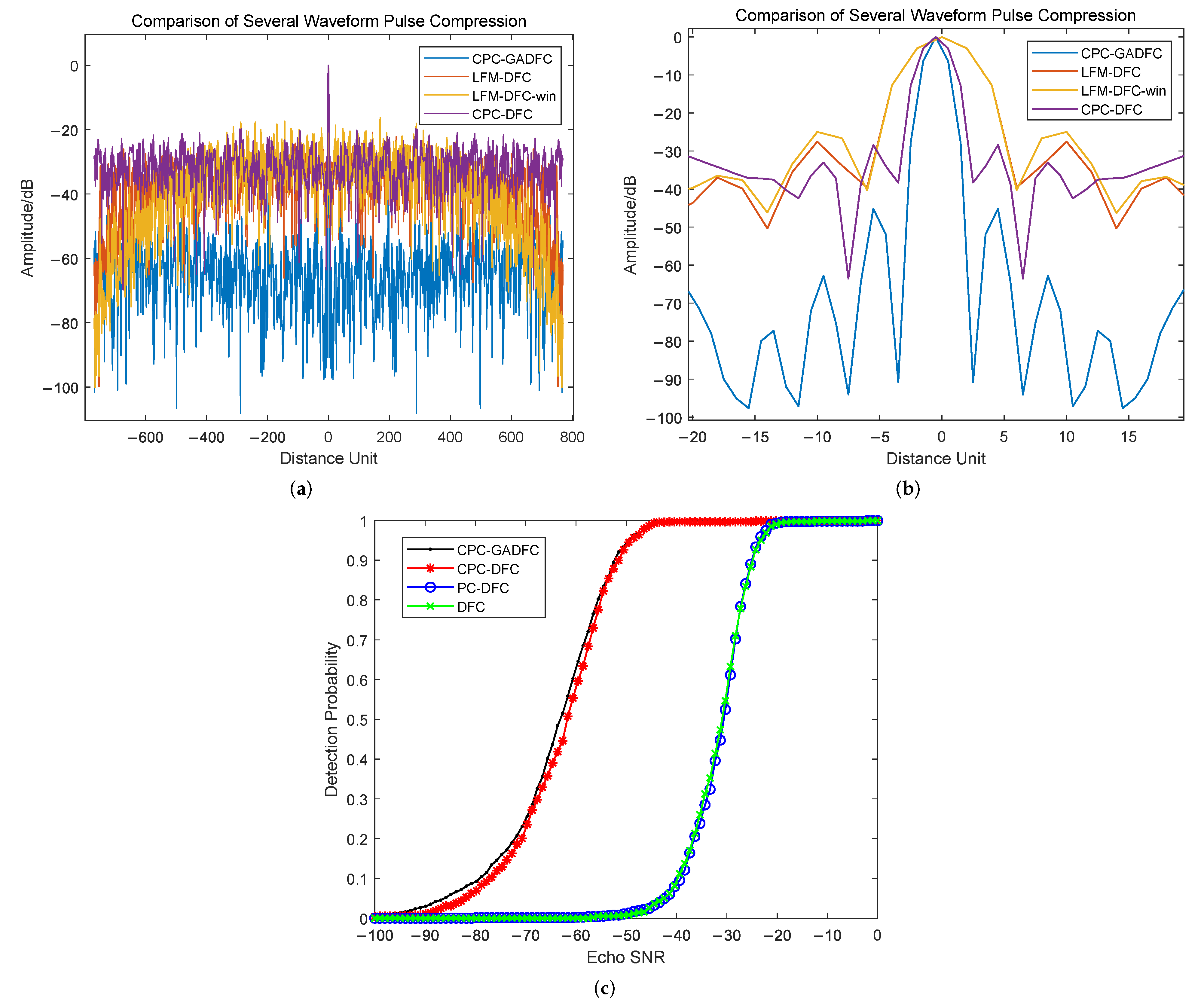
| Radar Waveform | Correl. Coeff. |
|---|---|
| DFC Waveform | 0.558 |
| GADFC Waveform | 0.164 |
| Coding Mode | Codewords |
|---|---|
| Positive Code | 1, 1, 1, 1, 1, −1, 1, −1, −1, −1, 1, 1, 1, −1, −1, 1, 1, 1, −1, −1, 1, −1, −1, 1, −1, −1, −1, −1, 1, −1, 1, −1 |
| Complement Code | −1, −1, −1, −1, −1, 1, −1, 1, 1, 1, −1, −1, −1, 1, 1, −1, 1, 1, −1, −1, 1, −1, −1, 1, −1, −1, −1, −1, 1, −1, 1, −1 |
| DFC | 12, 13, 25, 15, 6, 10, 19, 9, 18, 16, 5, 22, 7, 30, 32, 1, 11, 24, 27, 17, 14, 31, 2, 4, 3, 20, 29, 23, 8, 21, 28, 26 |
| GADFC | 31.5455, 31.5152, 10.9091, 20.5455, 28, 9.2424, 31.9091, 5.9394, 20.4848, 10.6364, 18.7273, 17.7273, 9, 17.6667, 17.3939, 6, 12.3939, 11.2121, 5, 4.8788, 4.5152, 3.1212, 9.7576, 7.0606, 18.0303, 5.2424, 7.6364, 4.9394, 12.0606, 28.8181, 16.9394, 31.3636 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Song, Y.; Wang, Y.; Xie, J.; Yang, Y.; Tian, B.; Xu, S. Ultra-Low Sidelobe Waveforms Design for LPI Radar Based on Joint Complementary Phase-Coding and Optimized Discrete Frequency-Coding. Remote Sens. 2022, 14, 2592. https://doi.org/10.3390/rs14112592
Song Y, Wang Y, Xie J, Yang Y, Tian B, Xu S. Ultra-Low Sidelobe Waveforms Design for LPI Radar Based on Joint Complementary Phase-Coding and Optimized Discrete Frequency-Coding. Remote Sensing. 2022; 14(11):2592. https://doi.org/10.3390/rs14112592
Chicago/Turabian StyleSong, Yuxiao, Yu Wang, Jingyang Xie, Yiming Yang, Biao Tian, and Shiyou Xu. 2022. "Ultra-Low Sidelobe Waveforms Design for LPI Radar Based on Joint Complementary Phase-Coding and Optimized Discrete Frequency-Coding" Remote Sensing 14, no. 11: 2592. https://doi.org/10.3390/rs14112592
APA StyleSong, Y., Wang, Y., Xie, J., Yang, Y., Tian, B., & Xu, S. (2022). Ultra-Low Sidelobe Waveforms Design for LPI Radar Based on Joint Complementary Phase-Coding and Optimized Discrete Frequency-Coding. Remote Sensing, 14(11), 2592. https://doi.org/10.3390/rs14112592








