Distinct Variability between Semidiurnal and Diurnal Internal Tides at the East China Sea Shelf
Abstract
:1. Introduction
2. Materials and Methods
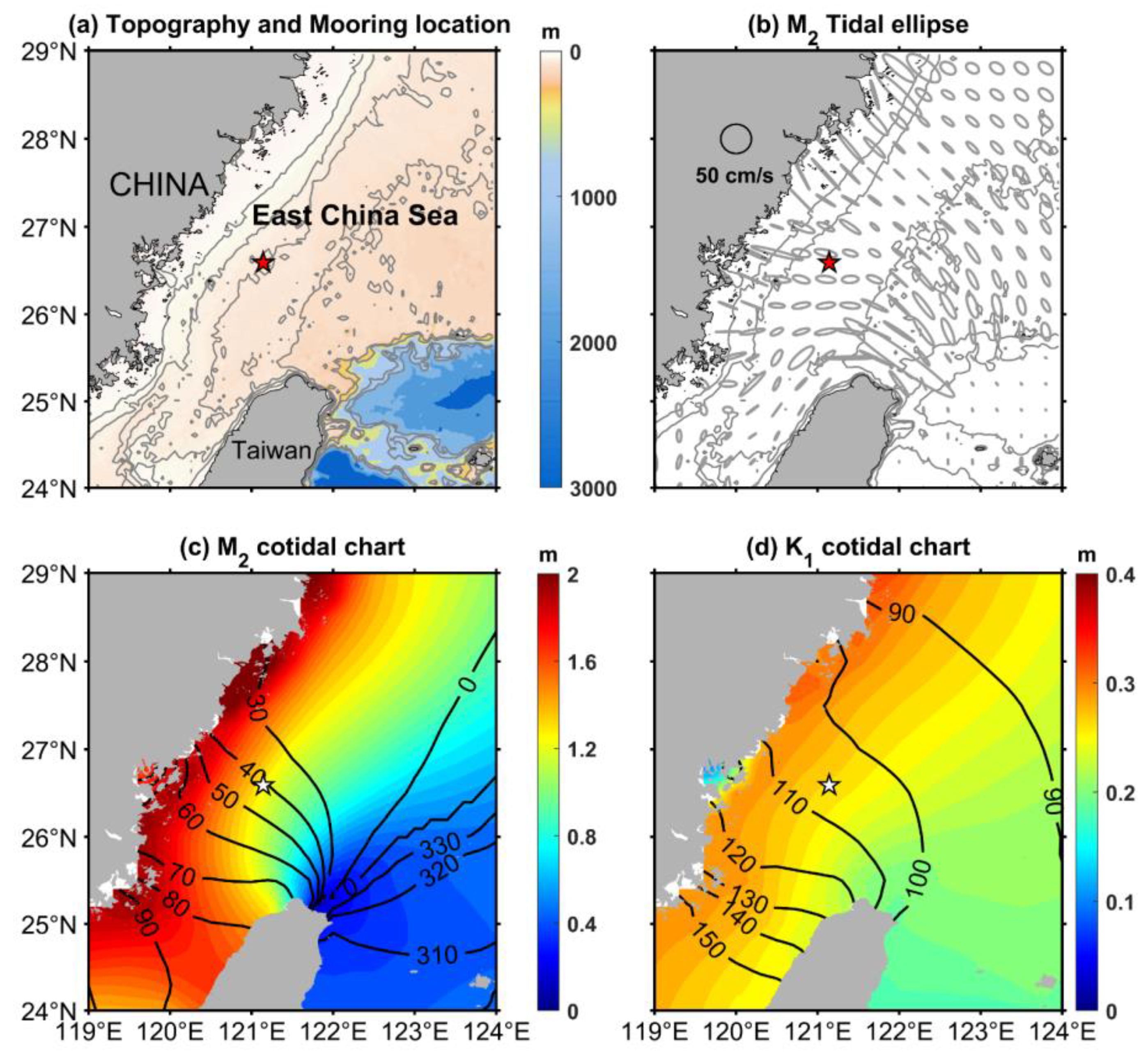
2.1. Mooring Observations
2.2. Satellite Altimetric Data
2.3. Body Force Calculation
2.4. Critical Latitude and Effective Latitude
2.5. Barotropic Tides
3. Results
3.1. Spectral Characteristics and Tidal Ellipses
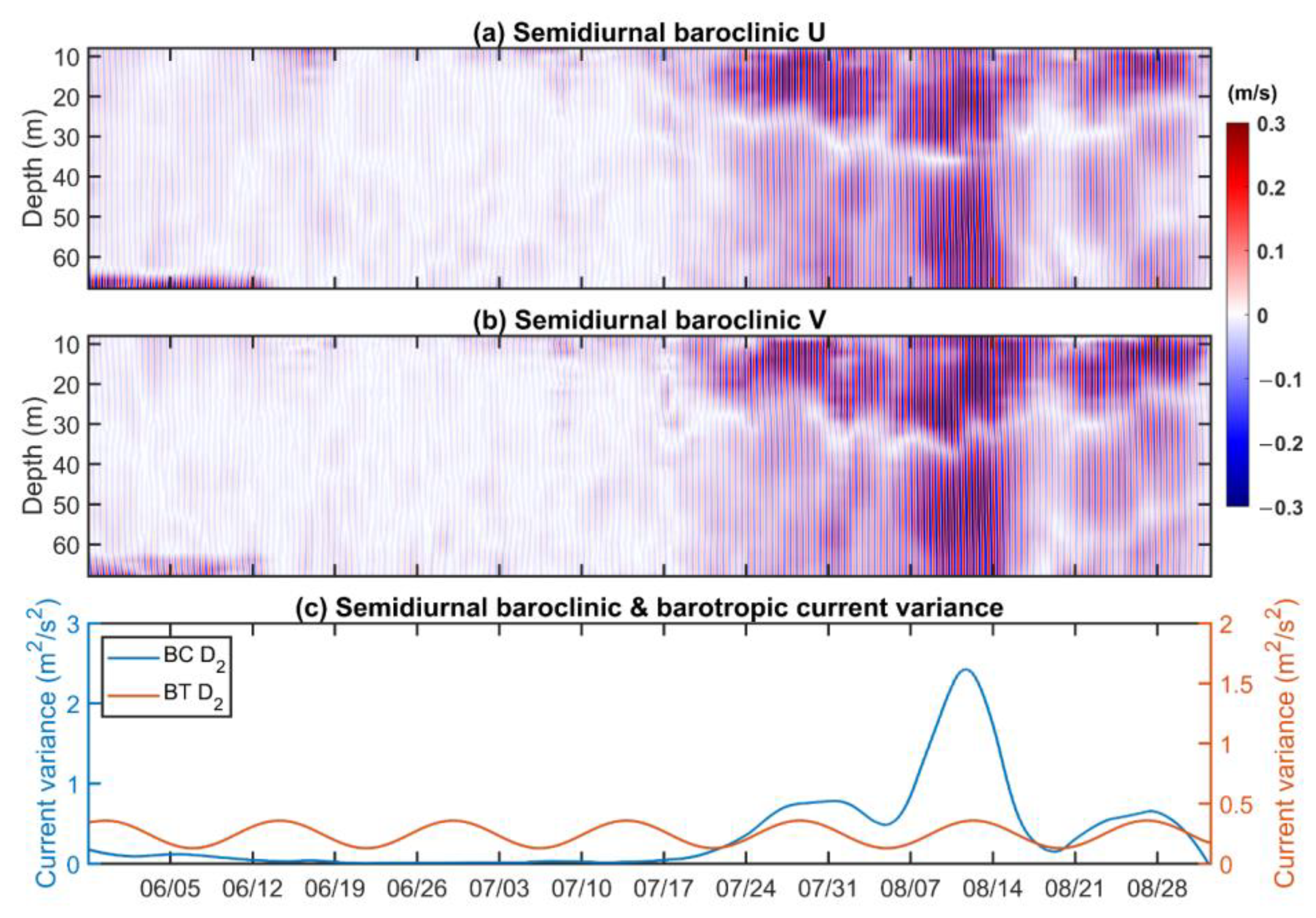
3.2. Distinct Variability of Semidiurnal and Diurnal Internal Tides
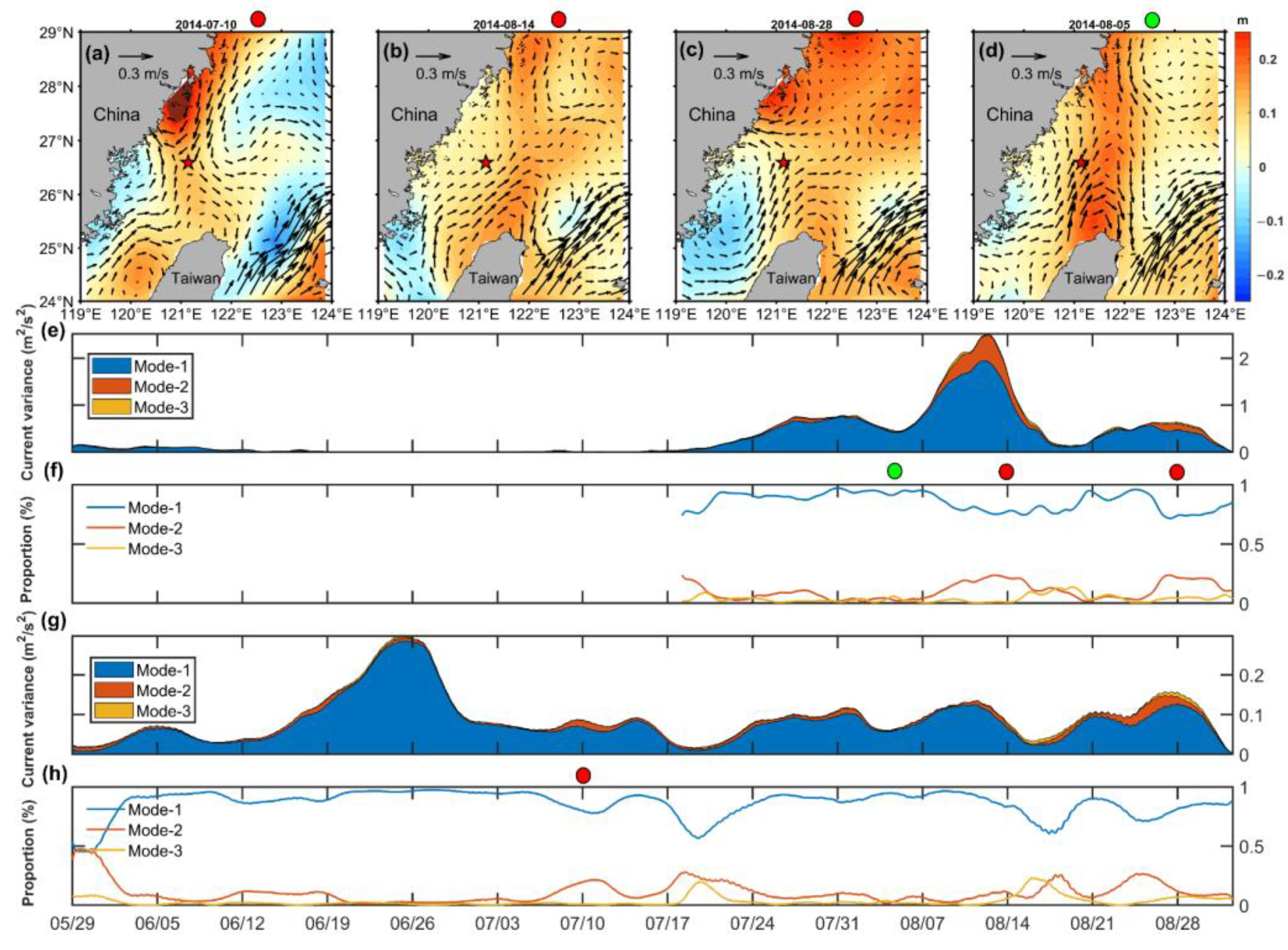
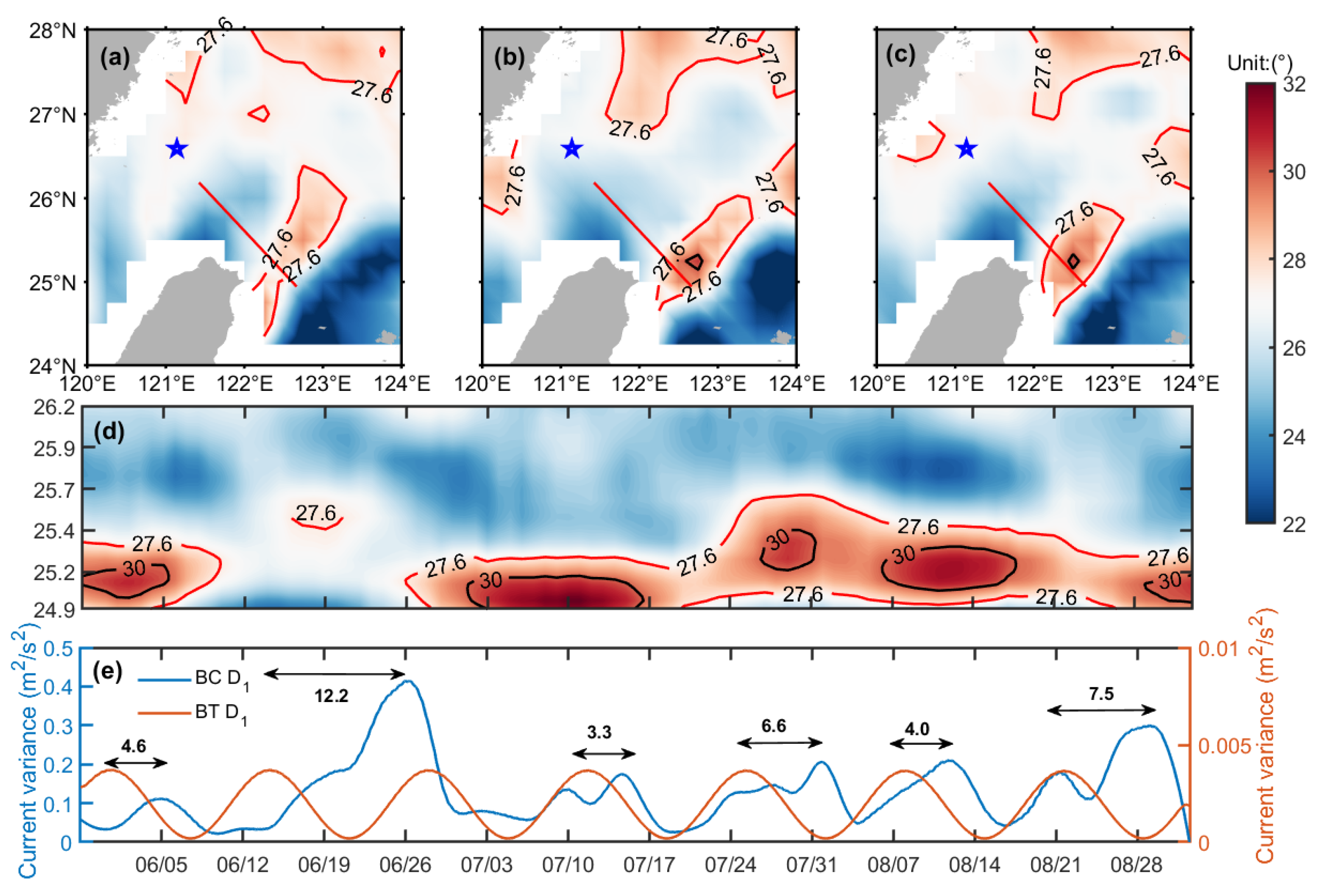
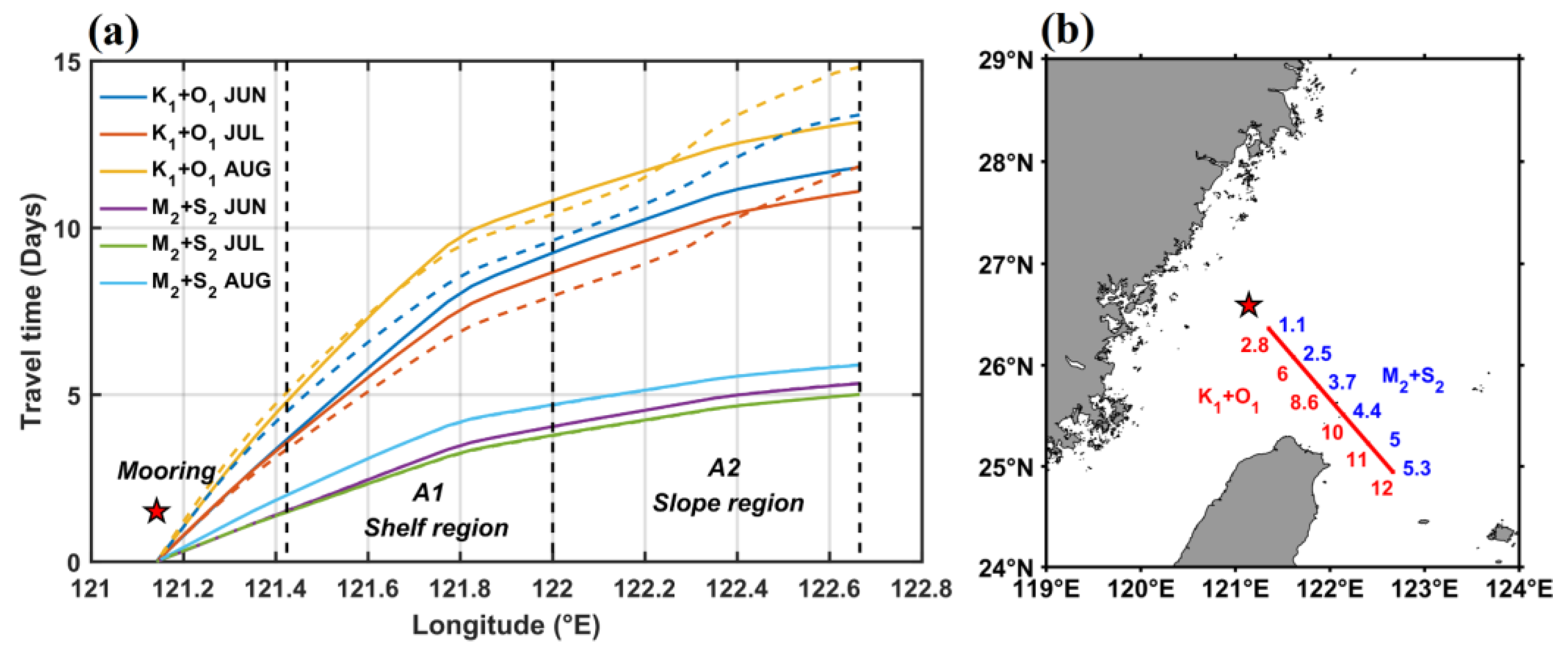
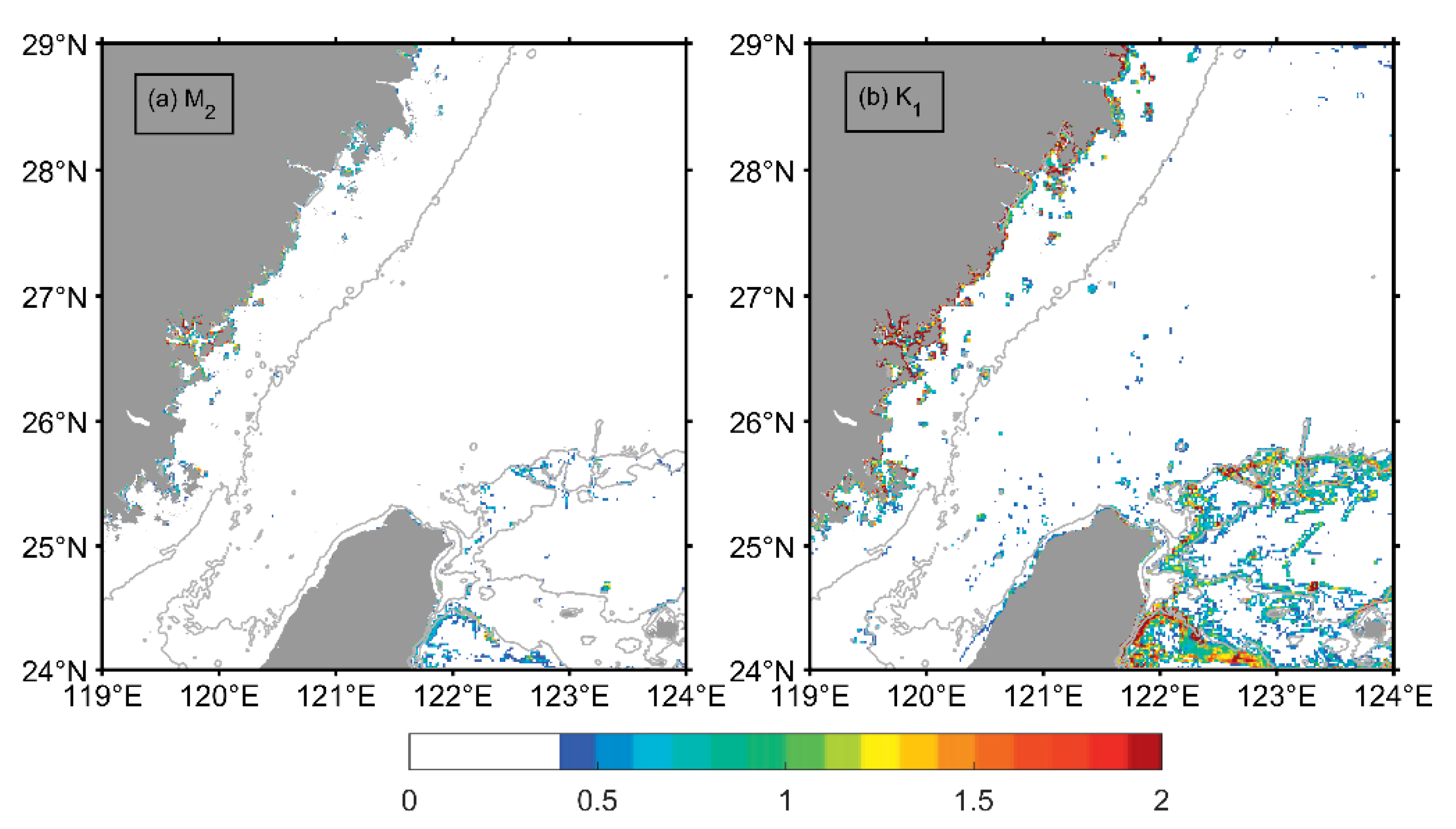
3.3. Internal Tide Generation Sites
3.4. Modal Contribution Modulated by Background Current
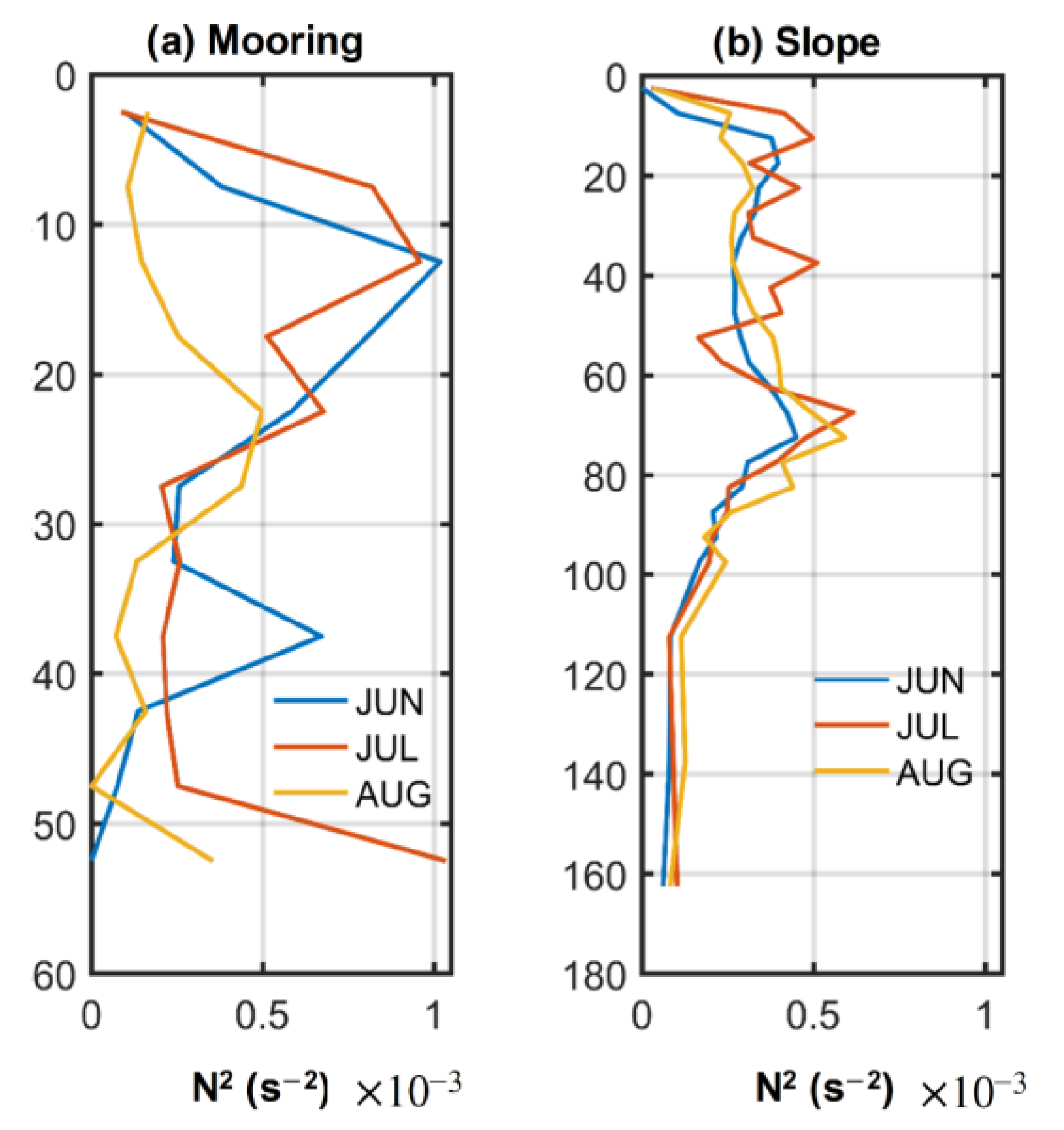
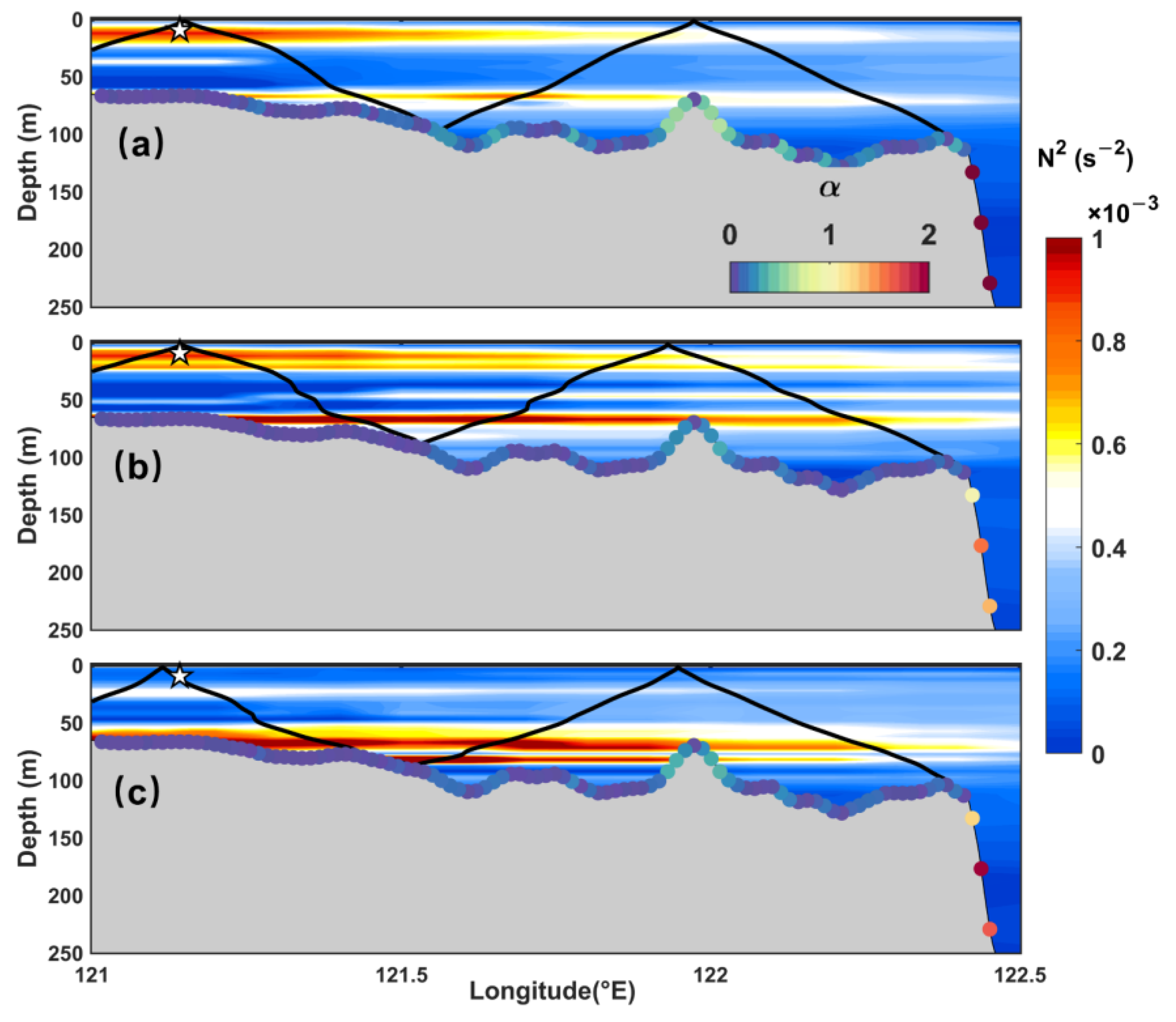
3.5. Diurnal Tidal Critical Latitude Effect
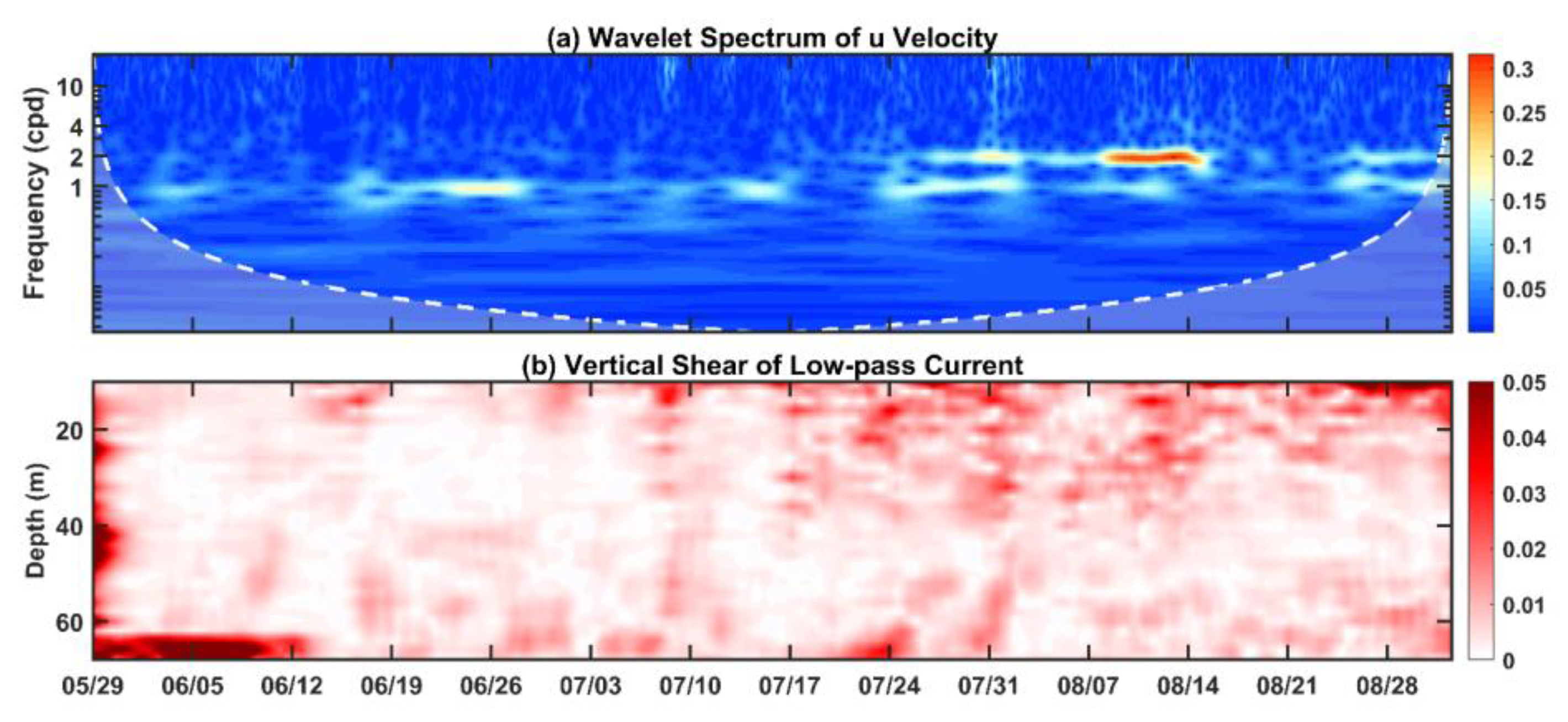
3.6. High-Frequency Internal Wave and Energy Cascades
4. Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Baines, P.G. On Internal Tide Generation Models. Deep Sea Res. Part A Oceanogr. Res. Pap. 1982, 29, 307–338. [Google Scholar] [CrossRef]
- Kelly, S.M.; Jones, N.L.; Nash, J.D.; Waterhouse, A.F. The Geography of Semidiurnal Mode-1 Internal-Tide Energy Loss. Geophys. Res. Lett. 2013, 40, 4689–4693. [Google Scholar] [CrossRef]
- Cacchione, D.A.; Pratson, L.F.; Ogston, A.S. The Shaping of Continental Slopes by Internal Tides. Science 2002, 296, 724–727. [Google Scholar] [CrossRef] [Green Version]
- Müller, M. On the Space- and Time-Dependence of Barotropic-to-Baroclinic Tidal Energy Conversion. Ocean Model. 2013, 72, 242–252. [Google Scholar] [CrossRef]
- Simmons, H.L.; Hallberg, R.W.; Arbic, B.K. Internal Wave Generation in a Global Baroclinic Tide Model. Deep. Res. Part II Top. Stud. Oceanogr. 2004, 51, 3043–3068. [Google Scholar] [CrossRef]
- Zhao, Z.; Alford, M.H.; Girton, J.B.; Rainville, L.; Simmons, H.L. Global Observations of Open-Ocean Mode-1 M2 Internal Tides. J. Phys. Oceanogr. 2016, 46, 1657–1684. [Google Scholar] [CrossRef]
- Carrere, L.; Arbic, B.K.; Dushaw, B.; Egbert, G.; Erofeeva, S.; Lyard, F.; Ray, R.D.; Ubelmann, C.; Zaron, E.; Zhao, Z.; et al. Accuracy Assessment of Global Internal-Tide Models Using Satellite Altimetry. Ocean Sci. 2021, 17, 147–180. [Google Scholar] [CrossRef]
- Zaron, E.D. Baroclinic Tidal Sea Level from Exact-Repeat Mission Altimetry. J. Phys. Oceanogr. 2019, 49, 193–210. [Google Scholar] [CrossRef]
- Zhao, Z. Seasonal Mode-1 M2 Internal Tides from Satellite Altimetry. J. Phys. Oceanogr. 2021, 51, 3015–3035. [Google Scholar] [CrossRef]
- Niwa, Y.; Hibiya, T. Three-Dimensional Numerical Simulation of M2 Internal Tides in the East China Sea. J. Geophys. Res. Ocean. 2004, 109, 1–14. [Google Scholar] [CrossRef]
- Wang, Y.; Xu, Z.; Yin, B.; Hou, Y.; Chang, H. Long-Range Radiation and Interference Pattern of Multisource M2 Internal Tides in the Philippine Sea. J. Geophys. Res. Ocean. 2018, 123, 5091–5112. [Google Scholar] [CrossRef] [Green Version]
- Tian, J.; Zhou, L.; Zhang, X.; Liang, X.; Zheng, Q.; Zhao, W. Estimates of M2 Internal Tide Energy Fluxes along the Margin of Northwestern Pacific Using TOPEX/POSEIDON Altimeter Data. Geophys. Res. Lett. 2003, 30, 4–7. [Google Scholar] [CrossRef]
- Chang, H.; Xu, Z.; Yin, B.; Hou, Y.; Liu, Y.; Li, D.; Wang, Y.; Cao, S.; Liu, A.K. Generation and Propagation of M2 Internal Tides Modulated by the Kuroshio Northeast of Taiwan. J. Geophys. Res. Ocean. 2019, 124, 2728–2749. [Google Scholar] [CrossRef]
- Lien, R.C.; Sanford, T.B.; Jan, S.; Chang, M.H.; Ma, B.B. Internal Tides on the East China Sea Continental Slope. J. Mar. Res. 2013, 71, 151–186. [Google Scholar] [CrossRef]
- Yang, W.; Wei, H.; Zhao, L. Parametric Subharmonic Instability of the Semidiurnal Internal Tides at the East China Sea Shelf Slope. J. Phys. Oceanogr. 2020, 50, 907–920. [Google Scholar] [CrossRef]
- Hsu, M.K.; Liu, A.K.; Liu, C. A Study of Internal Waves in the China Seas and Yellow Sea Using SAR. Cont. Shelf Res. 2000, 20, 389–410. [Google Scholar] [CrossRef]
- Zhao, Z.; Liu, B.; Li, X. Internal Solitary Waves in the China Seas Observed Using Satellite Remote-Sensing Techniques: A Review and Perspectives. Int. J. Remote Sens. 2014, 35, 3926–3946. [Google Scholar] [CrossRef]
- Li, X.; Zhao, Z.; Han, Z.; Xu, L. Internal Solitary Waves in the East China Sea. Acta Oceanol. Sin. 2008, 27, 51–59. [Google Scholar]
- Zhao, Z.; Alford, M.H.; Lien, R.C.; Gregg, M.C.; Carter, G.S. Internal Tides and Mixing in a Submarine Canyon with Time-Varying Stratification. J. Phys. Oceanogr. 2012, 42, 2121–2142. [Google Scholar] [CrossRef]
- Xu, Z.; Yin, B.; Hou, Y.; Liu, A.K. Seasonal Variability and North-South Asymmetry of Internal Tides in the Deep Basin West of the Luzon Strait. J. Mar. Syst. 2014, 134, 101–112. [Google Scholar] [CrossRef]
- Cao, A.; Guo, Z.; Lv, X.; Song, J.; Zhang, J. Coherent and Incoherent Features, Seasonal Behaviors and Spatial Variations of Internal Tides in the Northern South China Sea. J. Mar. Syst. 2017, 172, 75–83. [Google Scholar] [CrossRef]
- Liu, J.; He, Y.; Wang, D.; Liu, T.; Cai, S. Observed Enhanced Internal Tides in Winter near the Luzon Strait. J. Geophys. Res. Ocean. 2015, 120, 6637–6652. [Google Scholar] [CrossRef]
- Zhai, R.W.; Chen, G.Y.; Liang, C.R.; Shang, X.D.; Xie, J.S. The Influence of ENSO on the Structure of Internal Tides in the Xisha Area. J. Geophys. Res. Ocean. 2020, 125, e2019JC015405. [Google Scholar] [CrossRef]
- Devlin, A.T.; Jay, D.A.; Talke, S.A.; Zaron, E. Can Tidal Perturbations Associated with Sea Level Variations in the Western Pacific Ocean Be Used to Understand Future Effects of Tidal Evolution? Ocean Dyn. 2014, 64, 1093–1120. [Google Scholar] [CrossRef] [Green Version]
- Li, Q.; Wang, B.; Chen, X.; Chen, X.; Park, J.H. Variability of Nonlinear Internal Waves in the South China Sea Affected by the Kuroshio and Mesoscale Eddies. J. Geophys. Res. Ocean. 2016, 121, 2098–2118. [Google Scholar] [CrossRef] [Green Version]
- Song, P.; Chen, X. Investigation of the Internal Tides in the Northwest Pacific Ocean Considering the Background Circulation and Stratification. J. Phys. Oceanogr. 2020, 50, 3165–3188. [Google Scholar] [CrossRef]
- Huang, X.; Wang, Z.; Zhang, Z.; Yang, Y.; Zhou, C.; Yang, Q.; Zhao, W.; Tian, J. Role of Mesoscale Eddies in Modulating the Semidiurnal Internal Tide: Observation Results in the Northern South China Sea. J. Phys. Oceanogr. 2018, 48, 1749–1770. [Google Scholar] [CrossRef]
- Wang, Y.; Xu, Z.; Hibiya, T.; Yin, B.; Wang, F. Radiation Path of Diurnal Internal Tides in the Northwestern Pacific Controlled by Refraction and Interference. J. Geophys. Res. Ocean. 2021, 126, e2020JC016972. [Google Scholar] [CrossRef]
- Dunphy, M.; Lamb, K.G. Focusing and Vertical Mode Scattering of the First Mode Internal Tide by Mesoscale Eddy Interaction. J. Geophys. Res. Ocean. 2014, 119, 523–536. [Google Scholar] [CrossRef]
- Klymak, J.M.; Alford, M.H.; Pinkel, R.; Lien, R.C.; Yang, Y.J.; Tang, T.Y. The Breaking and Scattering of the Internal Tide on a Continental Slope. J. Phys. Oceanogr. 2011, 41, 926–945. [Google Scholar] [CrossRef]
- Robertson, R.; Dong, J.; Hartlipp, P. Diurnal Critical Latitude and the Latitude Dependence of Internal Tides, Internal Waves, and Mixing Based on Barcoo Seamount. J. Geophys. Res. Ocean. 2017, 122, 7838–7866. [Google Scholar] [CrossRef] [Green Version]
- Dong, J.; Robertson, R.; Dong, C.; Hartlipp, P.S.; Zhou, T.; Shao, Z.; Lin, W.; Zhou, M.; Chen, J. Impacts of Mesoscale Currents on the Diurnal Critical Latitude Dependence of Internal Tides: A Numerical Experiment Based on Barcoo Seamount. J. Geophys. Res. Ocean. 2019, 124, 2452–2471. [Google Scholar] [CrossRef]
- Rainville, L.; Pinkel, R. Propagation of Low-Mode Internal Waves through the Ocean. J. Phys. Oceanogr. 2006, 36, 1220–1236. [Google Scholar] [CrossRef]
- Nash, J.D.; Kelly, S.M.; Shroyer, E.L.; Moum, J.N.; Duda, T.F. The Unpredictable Nature of Internal Tides on Continental Shelves. J. Phys. Oceanogr. 2012, 42, 1981–2000. [Google Scholar] [CrossRef] [Green Version]
- Savage, A.C.; Waterhouse, A.F.; Kelly, S.M. Internal Tide Nonstationarity and Wave-Mesoscale Interactions in the Tasman Sea. J. Phys. Oceanogr. 2020, 50, 2931–2951. [Google Scholar] [CrossRef]
- Liu, Z.; Hou, Y. Kuroshio Front in the East China Sea from Satellite SST and Remote Sensing Data. IEEE Geosci. Remote Sens. Lett. 2012, 9, 517–520. [Google Scholar] [CrossRef]
- Yang, D.; Huang, R.X.; Yin, B.S.; Feng, X.R.; Chen, H.Y.; Qi, J.F.; Xu, L.J.; Shi, Y.L.; Cui, X.; Gao, G.D.; et al. Topographic Beta Spiral and Onshore Intrusion of the Kuroshio Current. Geophys. Res. Lett. 2018, 45, 287–296. [Google Scholar] [CrossRef] [Green Version]
- Lian, E.; Yang, S.; Wu, H.; Yang, C.; Li, C.; Liu, J.T. Kuroshio Subsurface Water Feeds the Wintertime Taiwan Warm Current on the Inner East China Sea Shelf. J. Geophys. Res. Ocean. 2016, 121, 4790–4803. [Google Scholar] [CrossRef]
- Shi, Y.; Yang, D.; He, Y. Numerical Study on Interaction between Eddies and the Kuroshio Current East of Taiwan, China. J. Oceanol. Limnol. 2021, 39, 388–402. [Google Scholar] [CrossRef]
- Yin, Y.; Lin, X.; He, R.; Hou, Y. Impact of Mesoscale Eddies on Kuroshio Intrusion Variability Northeast of Taiwan. J. Geophys. Res. Ocean. 2017, 122, 3021–3040. [Google Scholar] [CrossRef]
- Niwa, Y.; Hibiya, T. Numerical Study of the Spatial Distribution of the M2 Internal Tide in the Pacific Ocean. J. Geophys. Res. Ocean. 2001, 106, 22441–22449. [Google Scholar] [CrossRef] [Green Version]
- Van Haren, H. Shear at the Critical Diurnal Latitude. Geophys. Res. Lett. 2007, 34, 2–5. [Google Scholar] [CrossRef] [Green Version]
- Codiga, D.L. Unified Tidal Analysis and Prediction Using the UTide Matlab Functions; Technical Report 2011-01; Graduate School of Oceanography, University of Rhode Island: Narragansett, RI, USA, 2011; p. 59. [Google Scholar] [CrossRef]
- Xu, Z.; Yin, B.; Hou, Y. Multimodal Structure of the Internal Tides on the Continental Shelf of the Northwestern South China Sea. Estuar. Coast. Shelf Sci. 2011, 95, 178–185. [Google Scholar] [CrossRef]
- Xu, Z.; Yin, B.; Hou, Y.; Xu, Y. Variability of Internal Tides and Near-Inertial Waves on the Continental Slope of the Northwestern South China Sea. J. Geophys. Res. Ocean. 2013, 118, 197–211. [Google Scholar] [CrossRef]
- Xie, J.; Pan, J.; Jay, D.A. Multimodal Internal Waves Generated over a Subcritical Ridge: Impact of the Upper-Ocean Stratification. J. Phys. Oceanogr. 2015, 45, 904–926. [Google Scholar] [CrossRef] [Green Version]
- Zhao, Z. Southward Internal Tides in the Northeastern South China Sea. J. Geophys. Res. Ocean. 2020, 125, e2020JC016554. [Google Scholar] [CrossRef]
- Bai, X.; Liu, Z.; Li, X.; Hu, J. Generation Sites of Internal Solitary Waves in the Southern Taiwan Strait Revealed by MODIS True-Colour Image Observations. Int. J. Remote Sens. 2014, 35, 4086–4098. [Google Scholar] [CrossRef]
- Egbert, G.D.; Erofeeva, S.Y. Efficient Inverse Modeling of Barotropic Ocean Tides. J. Atmos. Ocean. Technol. 2002, 19, 183–204. [Google Scholar] [CrossRef] [Green Version]
- Smith, W.H.F.; Sandwell, D.T. Global Sea Floor Topography from Satellite Altimetry and Ship Depth Soundings. Science 1997, 277, 1956–1962. [Google Scholar] [CrossRef] [Green Version]
- Kunze, E.; Toole, J.M. Tidally Driven Vorticity, Diurnal Shear, and Turbulence atop Fieberling Seamount. J. Phys. Oceanogr. 1997, 27, 2663–2693. [Google Scholar] [CrossRef]
- Robertson, R. Modeling Internal Tides over Fieberling Guyot: Resolution, Parameterization, Performance. Ocean Dyn. 2006, 56, 430–444. [Google Scholar] [CrossRef]
- Robertson, R. Tidally Induced Increases in Melting of Amundsen Sea Ice Shelves. J. Geophys. Res. Ocean. 2013, 118, 3138–3145. [Google Scholar] [CrossRef]
- Kunze, E. Near-Inertial Wave Propagation in Geostrophic Shear. J. Phys. Oceanogr. 1985, 15, 544–565. [Google Scholar] [CrossRef]
- Gerkema, T.; Zimmerman, J.T.F.; Maas, L.R.M.; Van Haren, H. Geophysical and Astrophysical Fluid Dynamics beyond the Traditional Approximation. Rev. Geophys. 2008, 46, 1–33. [Google Scholar] [CrossRef] [Green Version]
- Yin, Y.; Liu, Z.; Zhang, Y.; Chu, Q.; Liu, X.; Hou, Y.; Zhao, X. Internal Tides and Their Intraseasonal Variability on the Continental Slope Northeast of Taiwan Island Derived from Mooring Observations and Satellite Data. Remote Sens. 2021, 14, 59. [Google Scholar] [CrossRef]
- Kelly, S.M.; Nash, J.D. Internal-Tide Generation and Destruction by Shoaling Internal Tides. Geophys. Res. Lett. 2010, 37, L23611. [Google Scholar] [CrossRef] [Green Version]
- Jan, S.; Chen, C.C.; Tsai, Y.L.; Yang, Y.J.; Wang, J.; Chern, C.S.; Gawarkiewicz, G.; Lien, R.C.; Centurioni, L.; Kuo, J.Y. Mean Structure and Variability of the Cold Dome Northeast of Taiwan. Oceanography 2011, 24, 100–109. [Google Scholar] [CrossRef] [Green Version]
- Gill, A.E.; Adrian, E. Atmosphere-Ocean Dynamics; Academic Press: Cambridge, MA, USA, 1982; Volume 30. [Google Scholar]
- Wunsch, C. Baroclinic Motions and Energetics as Measured by Altimeters. J. Atmos. Ocean. Technol. 2013, 30, 140–150. [Google Scholar] [CrossRef]
- Zhao, Z. Propagation of the Semidiurnal Internal Tide: Phase Velocity Versus Group Velocity. Geophys. Res. Lett. 2017, 44, 11942–11950. [Google Scholar] [CrossRef] [Green Version]
- Xu, Z.; Wang, Y.; Liu, Z.; McWilliams, J.C.; Gan, J. Insight Into the Dynamics of the Radiating Internal Tide Associated With the Kuroshio Current. J. Geophys. Res. Ocean. 2021, 126, 1–14. [Google Scholar] [CrossRef]
- Ansong, J.K.; Arbic, B.K.; Buijsman, M.C.; Richman, J.G.; Shriver, J.F.; Wallcraft, A.J. Indirect Evidence for Substantial Damping of Low-Mode Internal Tides in the Open Ocean. J. Geophys. Res. Ocean. 2015, 120, 6057–6071. [Google Scholar] [CrossRef] [Green Version]




| Constituent | Major, cm/s | Minor, cm/s | Inclination, Deg | Phase 1, Deg |
|---|---|---|---|---|
| M2 | 55.0 | 18.9 | 176.5 | 336 |
| S2 | 16.8 | 7.5 | 169.5 | 6.3 |
| K1 | 5.05 | 2.28 | 170 | 34 |
| O1 | 4.8 | 0.72 | 11 | 17 |
| June | July | August | |||
|---|---|---|---|---|---|
| Value (m2/s2) | Value (m2/s2) | Increasing Rate 1 (%) | Value (m2/s2) | Increasing Rate 2 (%) | |
| M2 all | 4462 | 5281 | 18.35 | 5486 | 22.95 |
| M2 in A1 | 893 | 1117 | 25.22 | 1230 | 51.12 |
| M2 in A2 | 3569 | 4164 | 16.67 | 4256 | 19.25 |
| K1 all | 1104 | 1297 | 17.50 | 1348 | 22.09 |
| K1 in A1 | 213 | 266 | 24.88 | 293 | 37.56 |
| K1 in A2 | 891 | 1031 | 15.71 | 1055 | 18.41 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, W.; Robertson, R.; Wang, Y.; Zhao, C.; Hao, Z.; Yin, B.; Xu, Z. Distinct Variability between Semidiurnal and Diurnal Internal Tides at the East China Sea Shelf. Remote Sens. 2022, 14, 2570. https://doi.org/10.3390/rs14112570
Wang W, Robertson R, Wang Y, Zhao C, Hao Z, Yin B, Xu Z. Distinct Variability between Semidiurnal and Diurnal Internal Tides at the East China Sea Shelf. Remote Sensing. 2022; 14(11):2570. https://doi.org/10.3390/rs14112570
Chicago/Turabian StyleWang, Weidong, Robin Robertson, Yang Wang, Chen Zhao, Zhanjiu Hao, Baoshu Yin, and Zhenhua Xu. 2022. "Distinct Variability between Semidiurnal and Diurnal Internal Tides at the East China Sea Shelf" Remote Sensing 14, no. 11: 2570. https://doi.org/10.3390/rs14112570
APA StyleWang, W., Robertson, R., Wang, Y., Zhao, C., Hao, Z., Yin, B., & Xu, Z. (2022). Distinct Variability between Semidiurnal and Diurnal Internal Tides at the East China Sea Shelf. Remote Sensing, 14(11), 2570. https://doi.org/10.3390/rs14112570








