Modelling of the Electron Density and Total Electron Content in the Quiet and Solar X-ray Flare Perturbed Ionospheric D-Region Based on Remote Sensing by VLF/LF Signals
Abstract
:1. Introduction
2. Observational Setups, Studied Area, and Considered Events
- The DHO signal amplitude and phase are recorded by the AWESOME receiver in Belgrade during the period when the D-region is perturbed by a solar X-ray flare. Significant changes in amplitude and phase resulting from the influence of other phenomena or technical problems in signal emission/reception are not recorded during the period significant for the presented analysis;
- The impact of an X-ray flare is in the midday period. This condition makes it possible to avoid the effects of diurnal changes which are more pronounced during the morning and afternoon when an approximation of the horizontally uniform ionosphere cannot be taken during the whole period of perturbation;
- The X-ray flare class is from C1 to M5. The lower limit is set because weaker flares do not cause clear changes in the signal characteristics, while the upper limit is introduced because modelling of the electron density by the procedure given in [21] is more appropriate for not too intensive flares.
3. Methodology
3.1. Determination of Wait’s Parameters
- Determination of the time evolutions of Wait’s parameters.Modelling of these dependencies is based on observational data and the LWPC numerical model [15]. For the considered VLF/LF signal and receiver location, the input parameters in this numerical program are Wait’s parameters, and its outputs are the modelled amplitude () and phase (). The presented procedure for the determination of and at time t is based on the comparison of the observed changes in the recorded signal amplitude () and phase () with respect to quiet conditions with the corresponding modelled changes:andIn these expressions, and are considered known, while and are determined based on the best agreement of the left and right sides of Equations (1) and (2) at time t. This procedure is well known and used in many papers [19,22], but it differs according to the mentioned criteria and the taken values and . In some studies, these criteria are not clearly defined, while and are usually taken as constants. The consequence of the last approximation is the neglect of daily and seasonal variations, variations during a solar cycle, as well as variations due to various sudden influences.In this paper, the criterion for the best agreement of the recorded and modelled changes in the signal characteristics (for pre-estimated values and ) is the minimum value of the sum of the corresponding differences normalised to the corresponding maximum recorded values.whereHere, and are all possible values of Wait’s parameters.In this paper, special attention is paid to the choice of parameters and . We propose a new methodology for their determination that represents an upgrade of the QIonDR model. An explanation of the significance of this analysis and a description of the proposed methodology are given in the following text.
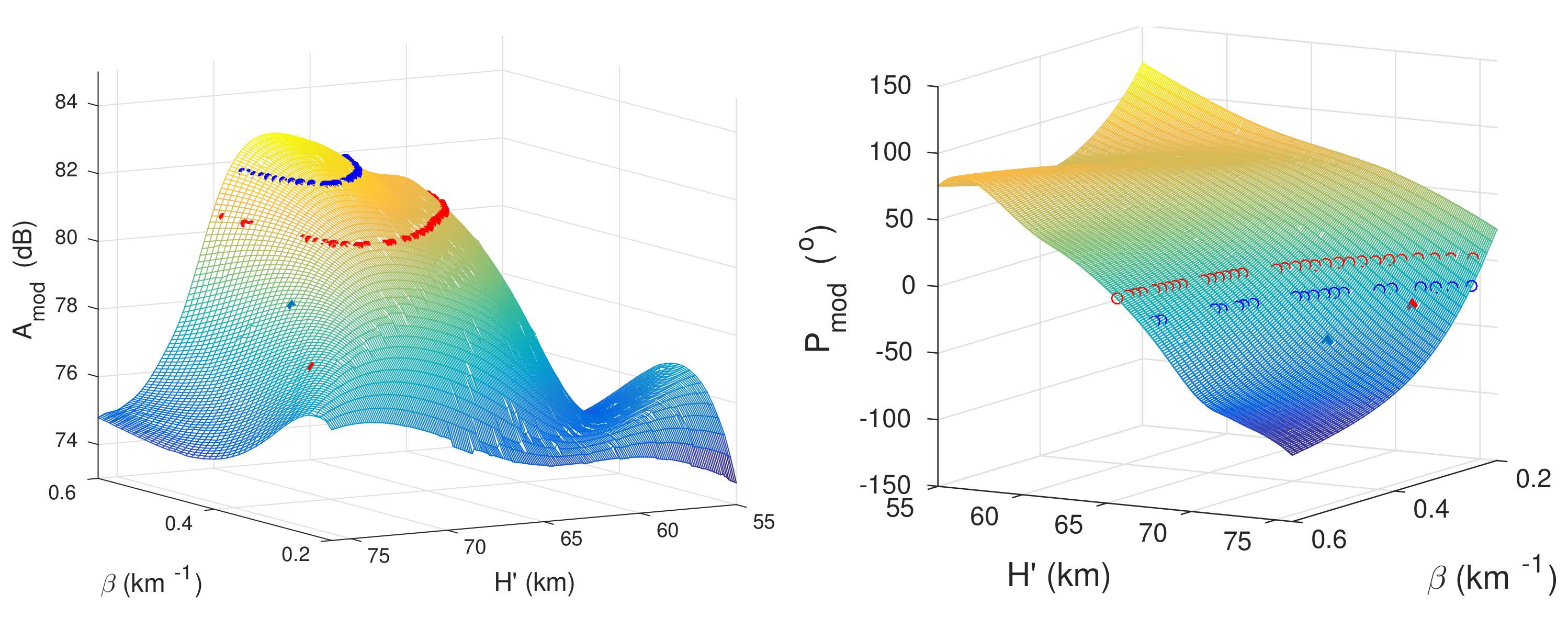
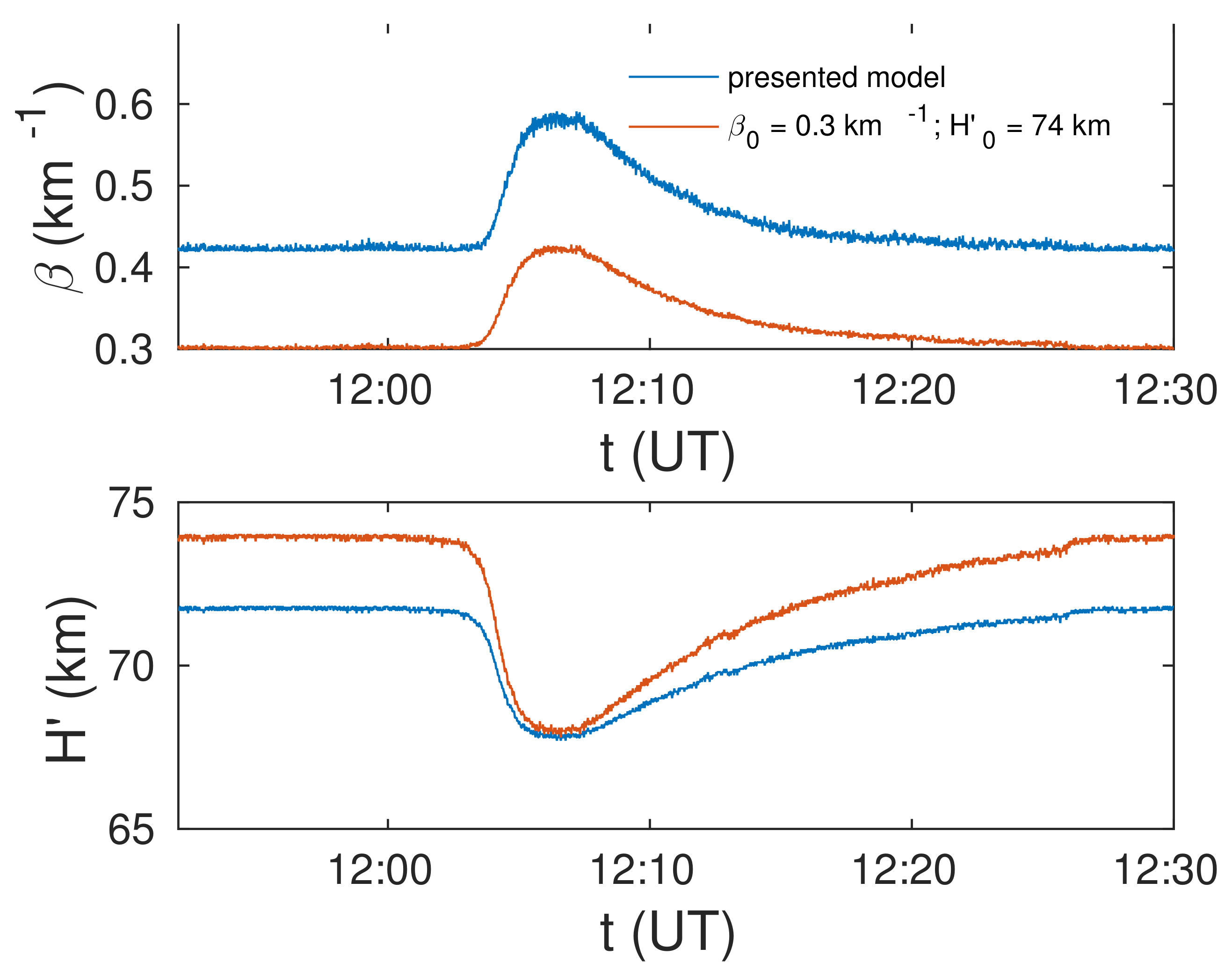
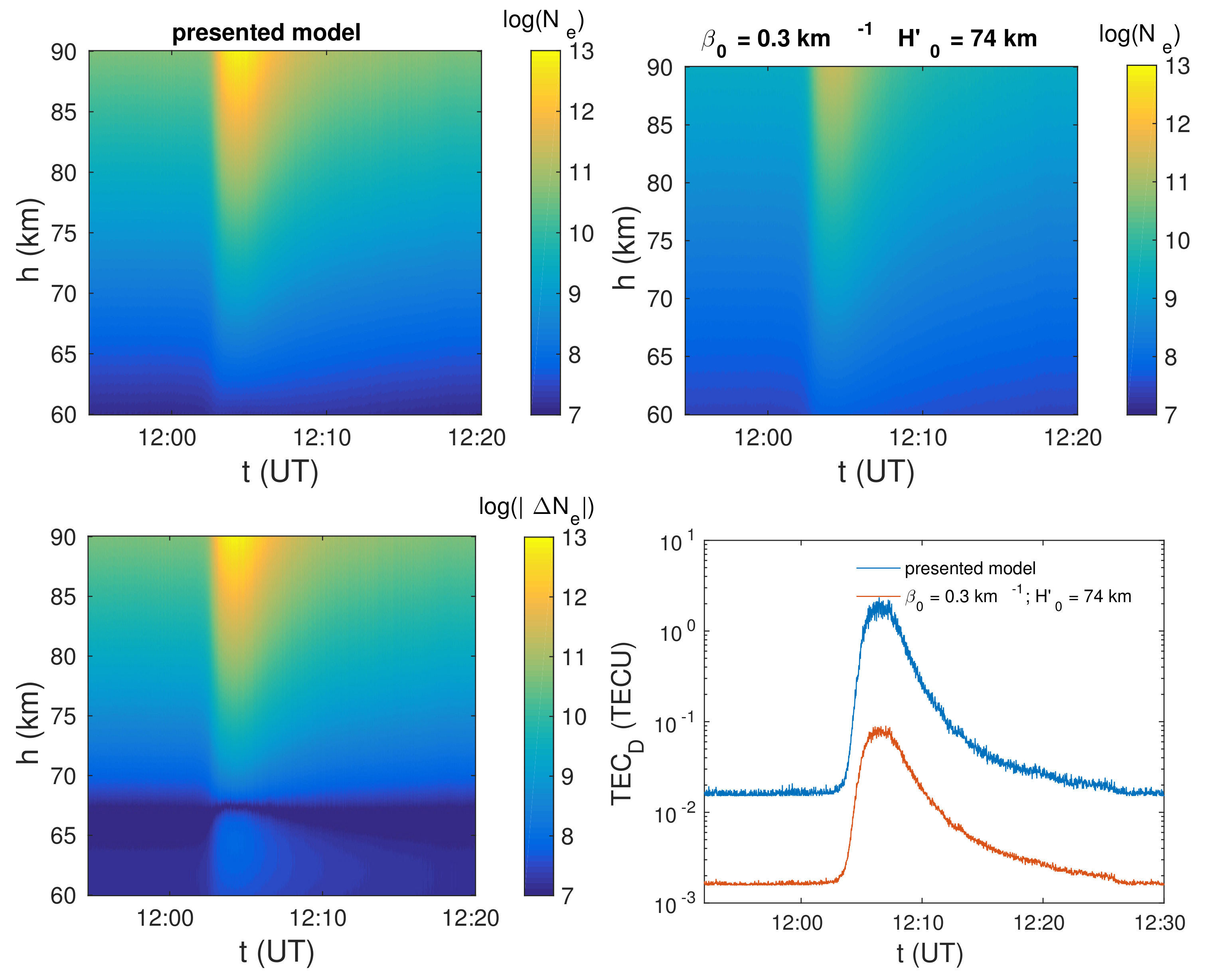
- Description of the influence of Wait’s parameters describing quiet ionosphere before the considered solar X-ray flare on modelling of their values under the disturbed conditions.As it can be seen from Equation (4), the pair () affects the value of G and, consequently, the value of the pair () obtained by applying the criterion given by Equation (3). To better explain this impact, we analyse the results of modelling by the LWPC numerical program for two pairs () and for the given registered amplitude and phase changes of 3 dB and 30°, respectively. The surfaces presented in Figure 2 show and (left and right panel, respectively) corresponding to all combinations of the considered values of Wait’s parameters. The isolated points (blue and red diamonds) correspond to the two combinations of and : (0.4 km, 72 km) and (0.3 km, 74 km), respectively. The points that form the blue and red “lines” indicate the pairs of Wait’s parameters for which the modelled amplitude (left panel) and phase (right panel) are approximative 3 dB and 30° larger than the corresponding values obtained for the two considered initial states, respectively.Although there are a number of pairs () for which one of the modelled changes is approximately equal to the corresponding given change, there are only a few combinations of Wait’s parameters (for one observed initial state) that give approximate agreement of both signal characteristics changes. It can be seen in Figure 3, where the obtained modelled pairs are presented in the 2D Wait’s parameter space. The estimated intersection points on the left and right panels ((0.48 km, 68.2 km) and (0.38 km, 68.4 km), respectively) represent Wait’s parameters obtained for their initial combinations (0.4 km, 72 km) and (0.3 km, 74 km), respectively. Based on the estimated pairs of Wait’s parameters, the electron density values at 65 km, 75 km, and 85 km are m, m, and m, in the first case, and m, m, and m, in the second case, while the corresponding values of TEC are 0.2 TECU and 0.03 TECU, respectively (the procedures for modelling these parameters are given in Section 3.2 and Section 3.3). A comparison of these values indicates a significant influence of the choice of and on modelling of the perturbed D-region. In order to better understand its significance, it is necessary to emphasise that the given changes in the signal characteristics are not too large and that the selected pairs of initial Wait’s parameters represent their real values that are not quiet close to the corresponding intervals limits. In other words, the obtained differences may be more pronounced in some other, also realistic, conditions.
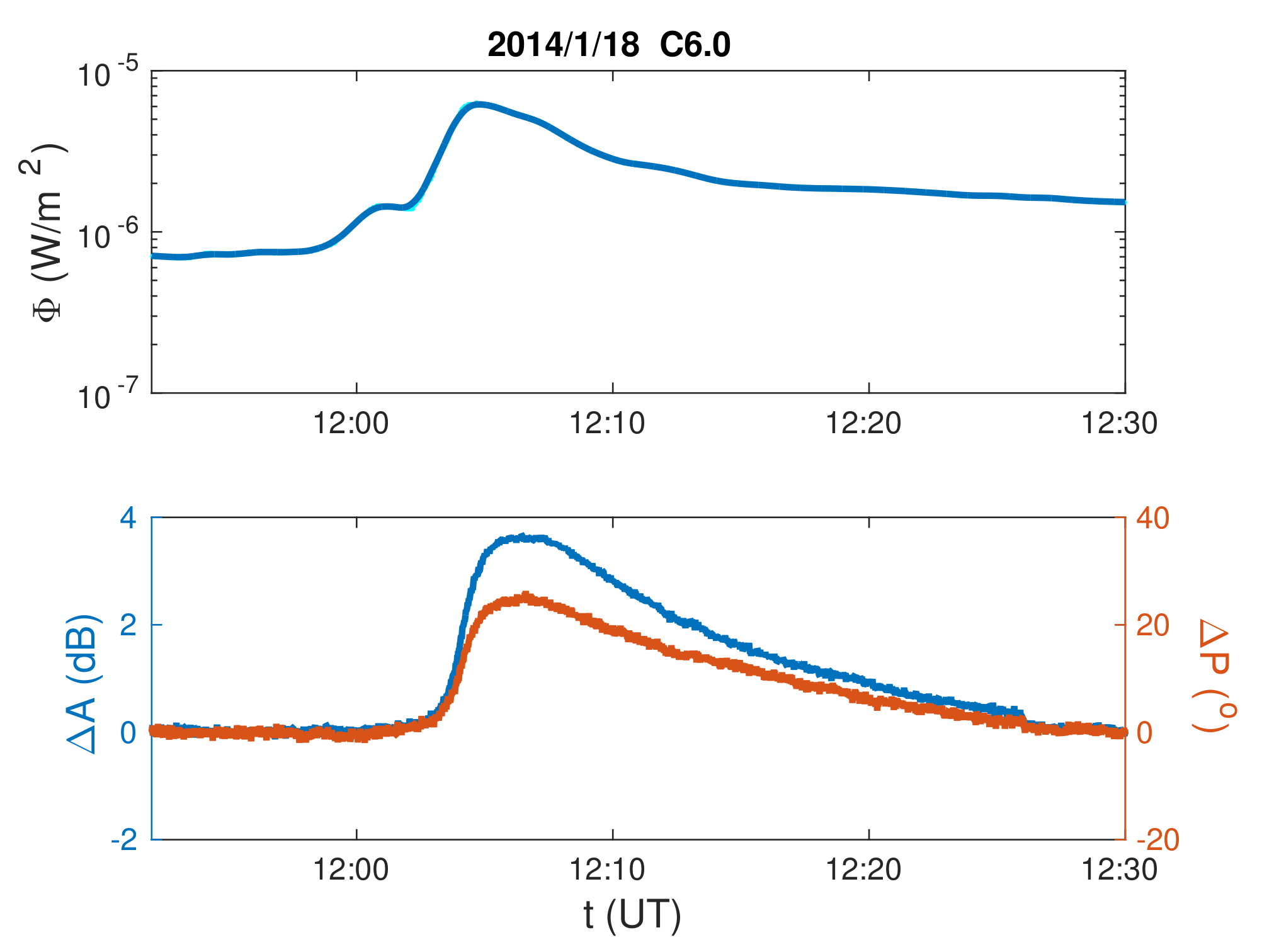
- Criteria for estimation of Wait’s parameters in the quiet state before the considered solar X-ray flare.Intensification of a D-region disturbance causes an increase/decrease in and , respectively, while the tendencies of these time evolutions are opposite during the return to the steady state of the ionosphere [2,13,17]. However, the choice of and significantly influences the shapes of their time evolutions, which allows us to introduce criteria for choosing the combination that gives the best dependences and . To better explain the differences in the time evolutions of Wait’s parameters, we present four different shapes for an X-ray flare that occurred on 18 January 2014 (see Figure 4). In the presented graphs, the points represent the corresponding values obtained at time t using the criterion given by Equation (3), while the lines show their smoothed values.As it can be seen in the upper panels, there are values of pairs () for which the time evolutions of decrease (upper left panel) or reach the maximum possible value in a longer time period (right panel) which is not in accordance with the expected form. These discrepancies allow us to exclude all combinations () for which the corresponding forms are similar to those shown on these two panels. In addition, many combinations () give dependences that fall very quickly to the initial values with respect to the time evolutions of , A, and P, which also excludes the corresponding pairs (). One such example is given in the bottom left panel. An example of the shape of function that can describe the time evolution of this Wait’s parameter is given in the bottom right panel.To estimate the initial conditions and model the time evolutions of Wait’s parameters we use the following criteria:
- 1.
- 2.
- The shape of the smoothed time evolution of is characterised by an increase to the maximum value (occurs after the maximum X-ray flux in the period, when reaches minimum value) followed by a decrease. Deviations from this shape are possible if the corresponding characteristics are observed in the signal amplitude/phase but, in that case, we consider only events in which these variations do not affect the analysis, i.e., when these variations occur after extreme values of Wait’s parameters.
- 3.
- The relaxation of to its value in the quiet state after the considered disturbance should be as similar as possible to the signal amplitude relaxation. For this reason, we introduce the condition that the time when reaches values corresponding to quiet conditions should be after the time when falls to some given value. This value is not unique due to differences in the characteristics of various impacts on the observed area during the analysed time period. Based on the presented analysis, the estimated value of this parameter is between 0.5 dB and 1 dB.
- 4.
- Generally, there are several combinations of and that meet criteria 1 and 2 and have a similar time when reaches values corresponding to quiet conditions. Therefore, we introduce an additional criterion that allows us to determine the combination (,) that deviates the least from Wait’s parameters, and , predicted by the QIonDR model. This deviation () is calculated by the following expression:where dependencies of midday Wait’s parameters on the daily smoothed sunspot number () and the seasonal parameter () estimated by the QIonDR model are given by following equations [13]:andHere, km and km are the estimated maximal absolute deviations of and from and , respectively. These values are estimated based on the maximum absolute deviations of Wait’s parameters from their fitted values for data shown in [10] (0.03 km and 1.5663 km), [19] (0.07 km and 1.8 km), and [13] (0.08 km and 3.6 km). We note that the fitted functions for the first two sets of data are given in [24].Here, it is important to emphasise that the values of and are estimated and that they can influence the choice of pairs () if the analysed deviations are similar for multiple combinations of initial Wait’s parameters. In this case, it is necessary to check which values of Wait’s parameters at the time of the X-ray flux maximum fit best with those obtained in other cases. In our study, this correction procedure is applied in only three cases (10% of the total number of the analysed cases). The correction for these class-C4.1, -C6.1, and -C8.0 solar X-ray flares is made due to the excessive value of (0.59 km , 0.55 km , and 0.57 km , respectively) at the X-ray flux maximum obtained before correction.
3.2. Determination of the Electron Density
3.3. Determination of the D-Region Total Electron Content
4. Results and Discussions
4.1. Time Evolutions of the Considered Ionospheric Parameters during a Single Flare
- The time dependences and are determined for all combinations of Wait’s parameters in quiet conditions in the ranges 0.2 km to 0.55 km with a step of 0.01 km for and 65 km to 76 km with a step of 0.1 km for .
- Wait’s parameters and are determined by applying criteria 1–4 given in Section 3 to the obtained dependences and .
4.2. Statistical Analysis
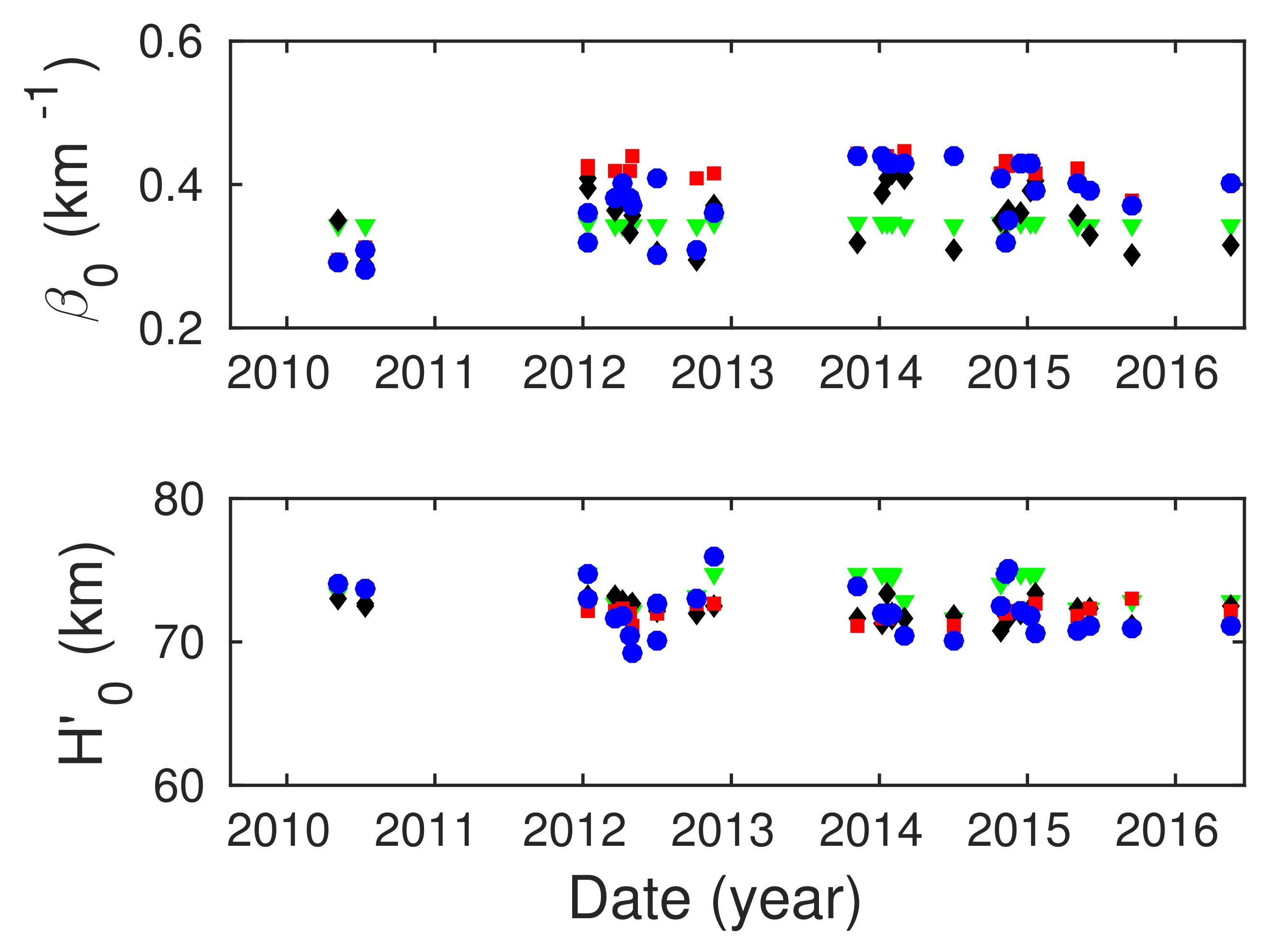
Wait’s Parameters
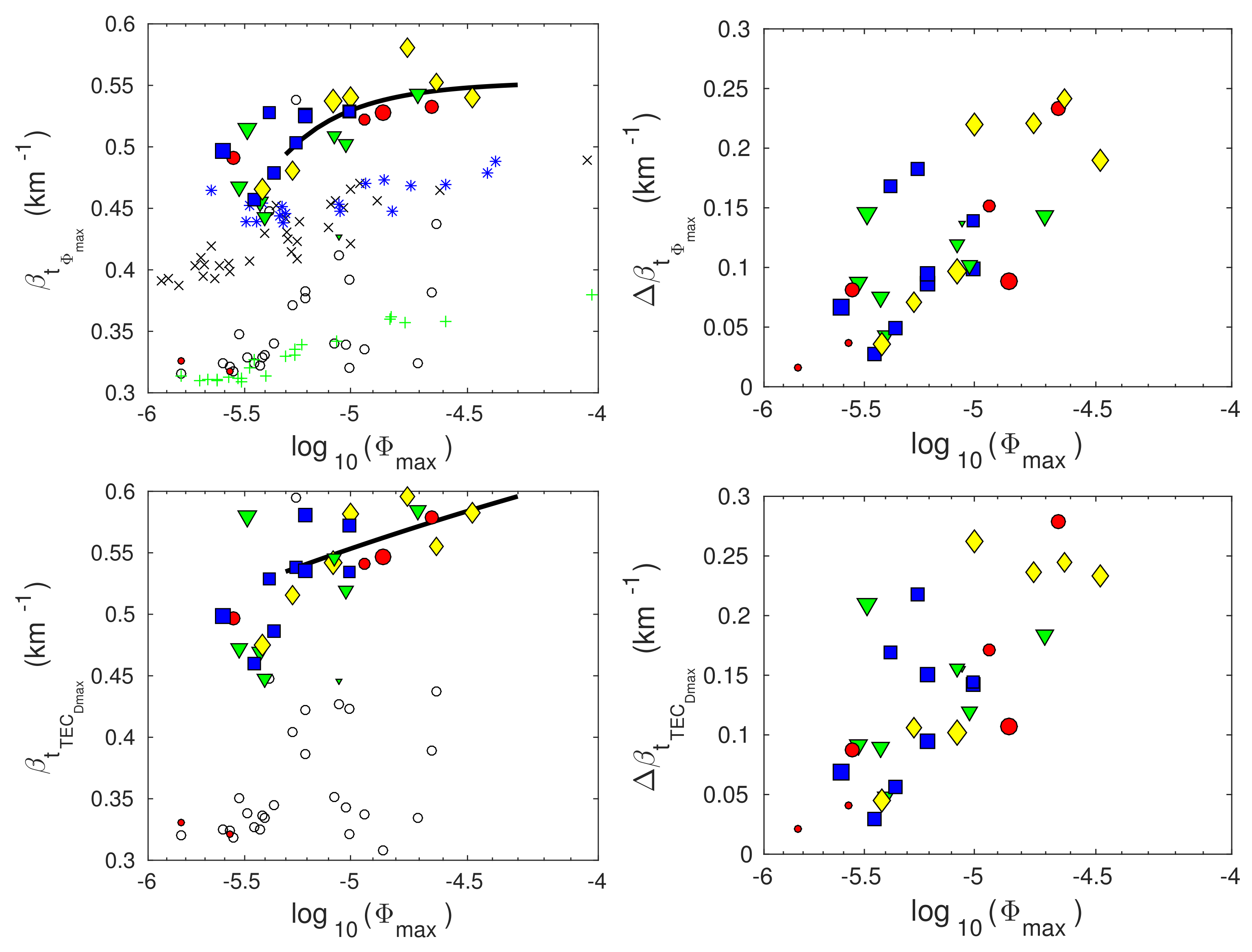
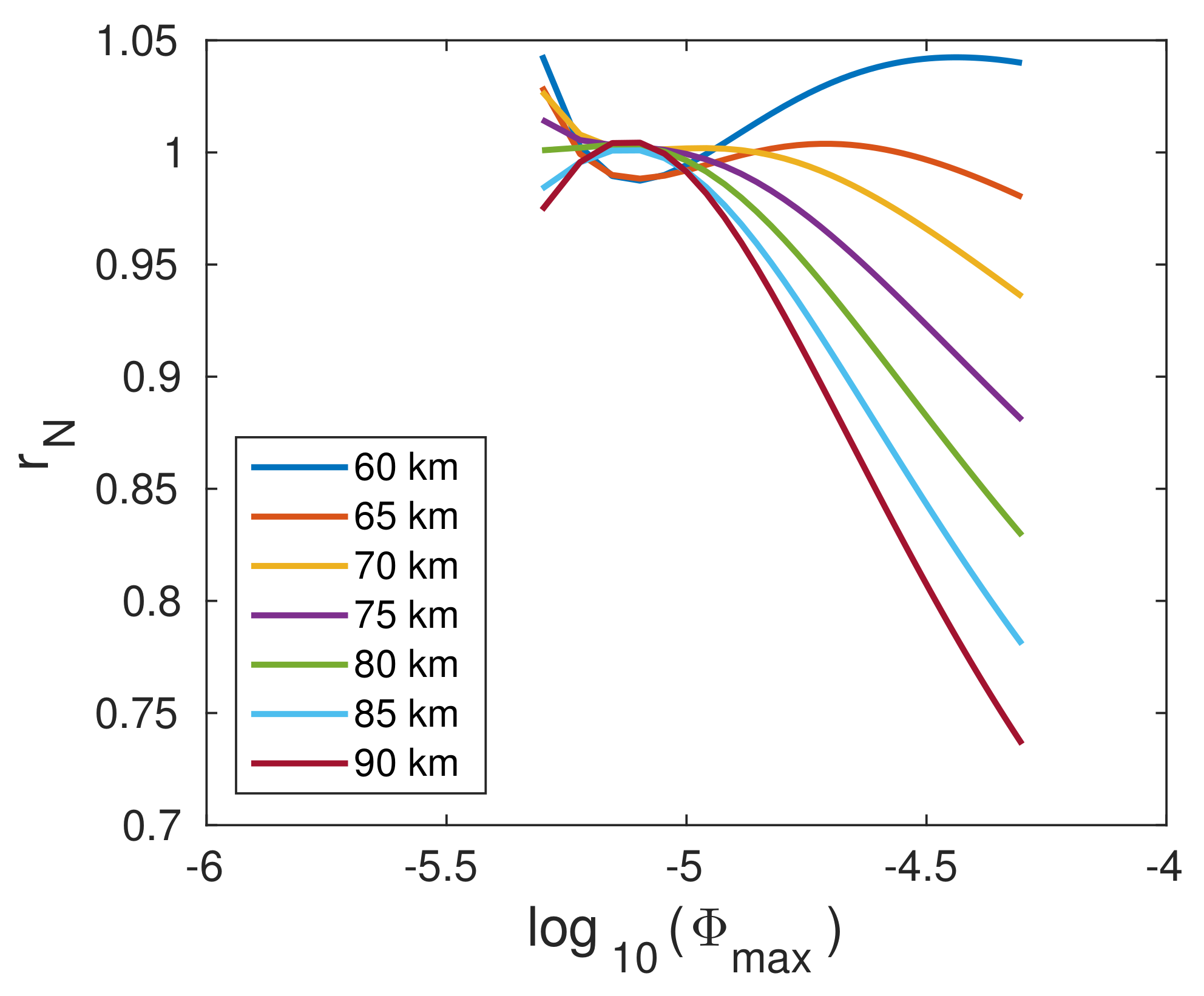
- The dispersion of points on the graph is greater at the time , which can be explained by the additional influence of differences in the radiation characteristics after the maximum of its flux. This difference is not significant for .
- The dispersion of the obtained values decreases with , which indicates a decrease in the influence of the initial state of the ionosphere on the considered parameters during disturbance with the flare class. This can be explained by the fact that the solar X-radiation dominates the solar hydrogen Ly and cosmic radiation (these two radiations are the most important sources of ionisation in the unperturbed D-region) in electron gain processes at the time of the X-ray flux maximum [36]. This dominance increases with and, consequently, the considered differences in Wait’s parameters decrease with .
- The effect of variations in the radiation intensity during a solar cycle on at times and is significant in the period around the solar cycle minimum. In the cases of the two X-ray flares of classes C1.4 and C2.6, which occurred in this period, has significantly less values than those estimated for the other analysed flares of the similar classes. This difference is reduced for the stronger flare of class-C8.8. The values of for all three events which occurred during this period are similar to the corresponding values for the other analysed events.
- The influence of seasonal changes on the observed parameters is not visible for less intense flares. In the case of more intense flares (starting with class-C5), there is indication that and are slightly higher during the winter (blue squares) and autumn (yellow diamonds) periods than during the second part of year (green triangles and red circles). However, these differences are not significant which is why we analyse all these flares together (see the next item).
- The dispersion of the obtained values is significant for the considered weak X-ray flares of low intensity. Therefore, and due to the mentioned differences in and for events which occurred in the period around the solar cycle minimum, we fit the obtained values for the X-ray flares whose maximum flux is greater than Wm and which occurred on days for which 50. The fitted functions have the form:where f is the considered parameter. The corresponding values of a, b, and c are given in Supplementary Materials (Table S2).
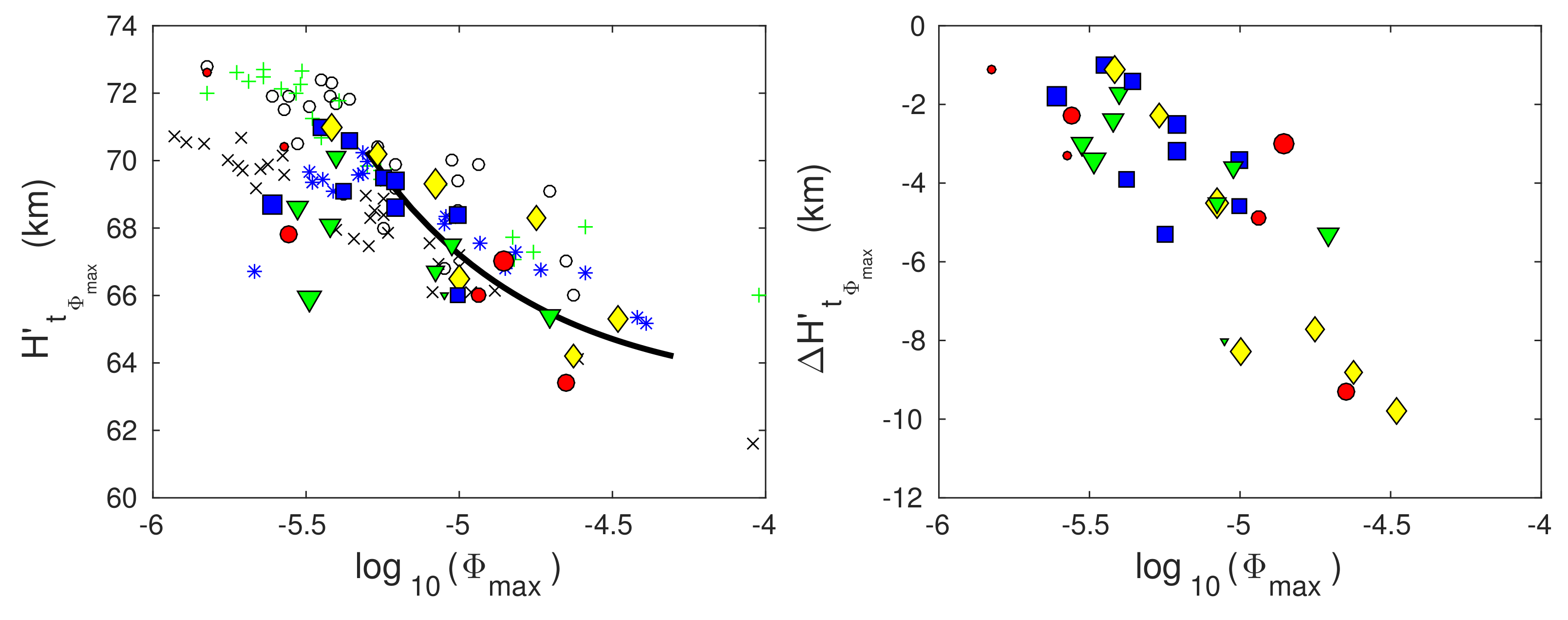
- In the first case, we compare studies presented in [18,35] that analyse solar X-ray flares that occurred in 2011 (medium solar cycle conditions) and the D-region area monitored by the NWC signal emitted in Australia and recorded in India. The pairs of Wait’s parameters () used in [18,35] are (0.43 km, 71 km) and (0.3 km, 74 km), respectively. A comparison of these values (presented in the upper left panels in Figure 8 and Figure 9) shows that is higher and is less in the first case.
- In the second case, we compare Wait’s parameters in the time obtained in [23] with those shown in [18,35]. The study presented in [23] analyses perturbations caused by X-ray flares which occurred during the solar cycle minimum and medium (1994–1998). It is based on data related to the NPM and NLK signals from USA recorded in New Zealand. The values shown in [23] are between the values given in [18,35]. They are very similar to those given in [35] which corresponds to similar values of (this value is 0.39 km) and the same value of .Finally, the agreement of the results obtained in this study with those given in the previous three can be explained in the same way as in the previous analysis: agreement is best with the results of analyses in which the pair () has similar values to those estimated for a single observed event analysed in this research. The values of shown in [35] are greater than the corresponding values shown in [18,23]. Consequently, they are in the best agreement with the results obtained in this study for events that occurred since 2012, i.e., for events for which the values of are higher and the values of are less than the corresponding values during the minimum of the solar cycle. The agreement of the results of this study is better with those given in [18] in the cases of weak flares that occurred in 2010, i.e., in the period around the solar cycle minimum. In these cases, the initial values of Wait’s parameters are similar in both studies.
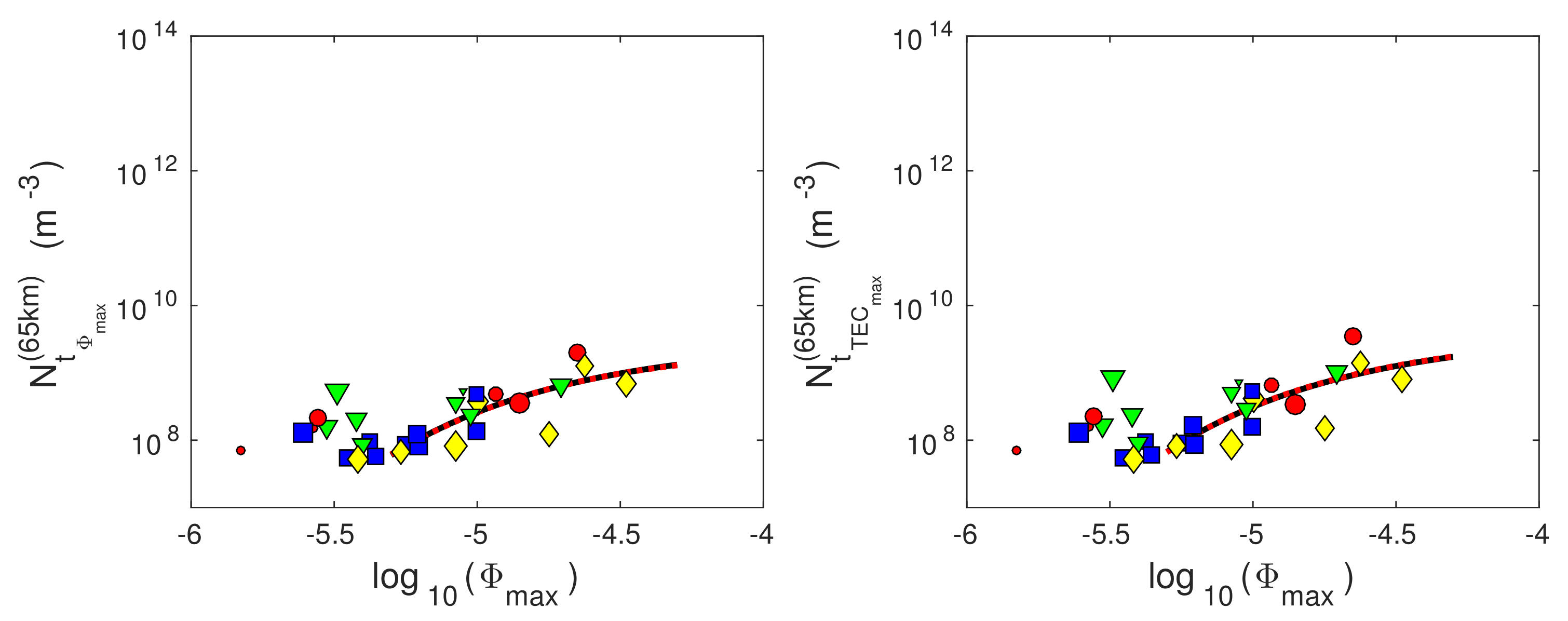
4.3. Determination of the Electron Density
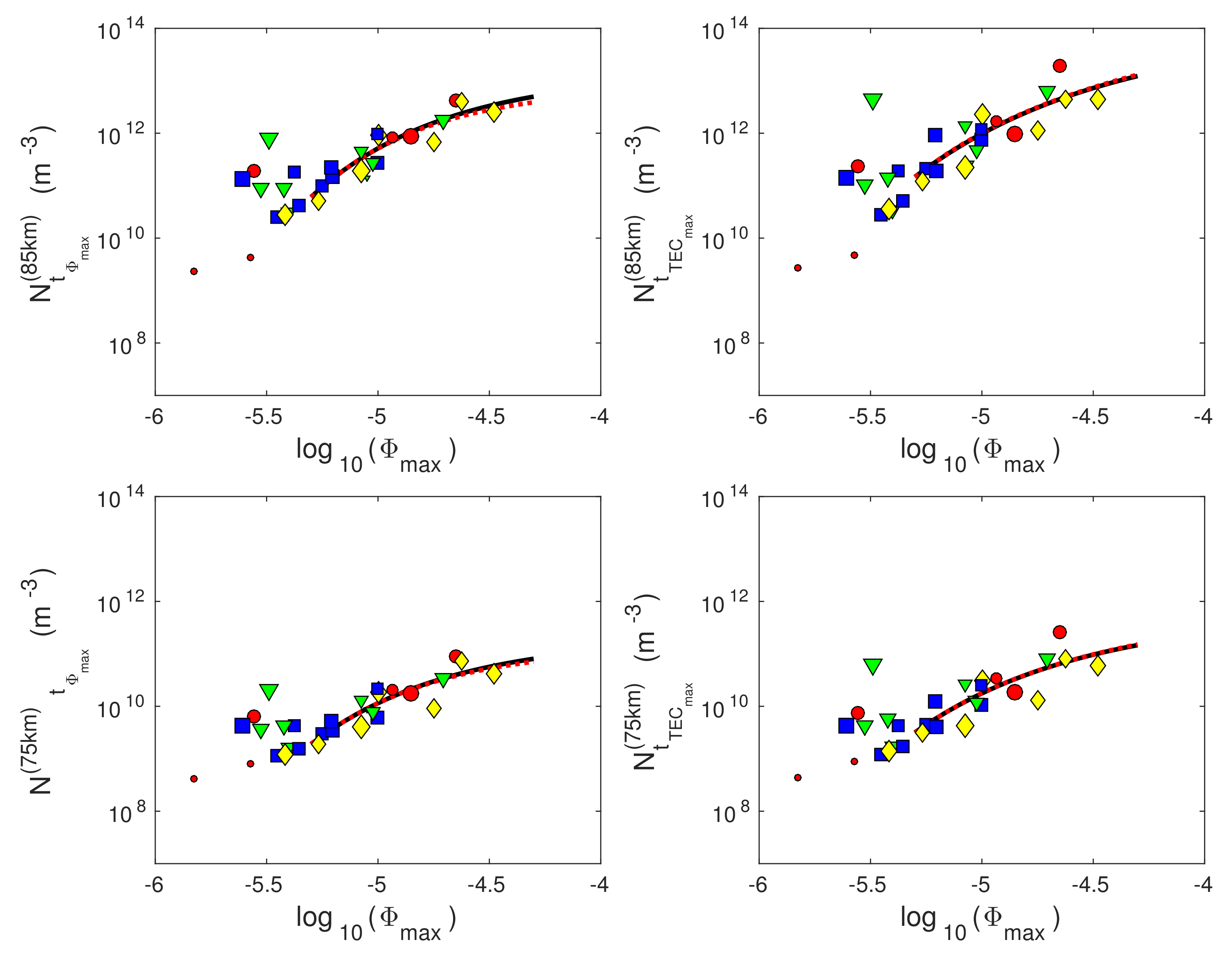
- As in the case of Wait’s parameter , the dispersion of the obtained values of is greater at the time .
- The influence of the ionospheric initial state is more manifested in the cases of weak flares, which is reflected in more pronounced dispersion of the obtained values at all heights for the corresponding part of the observed flux domain.
- Deviations of points representing weak flares that occurred during periods near the solar cycle minimum are visible at 85 km for both the considered times.
- Fitting of the modelled values refers to the considered flares for which Wm and 50. The obtained fitted functions, shown by black lines in Figure 10, have the form given by Equation (10), where the corresponding parameters a, b, and c are given in Supplementary Materials (Table S2).
- The changes in within the considered flux domain are greater at the time . This is expected because the perturbation intensity is the most pronounced at that time.
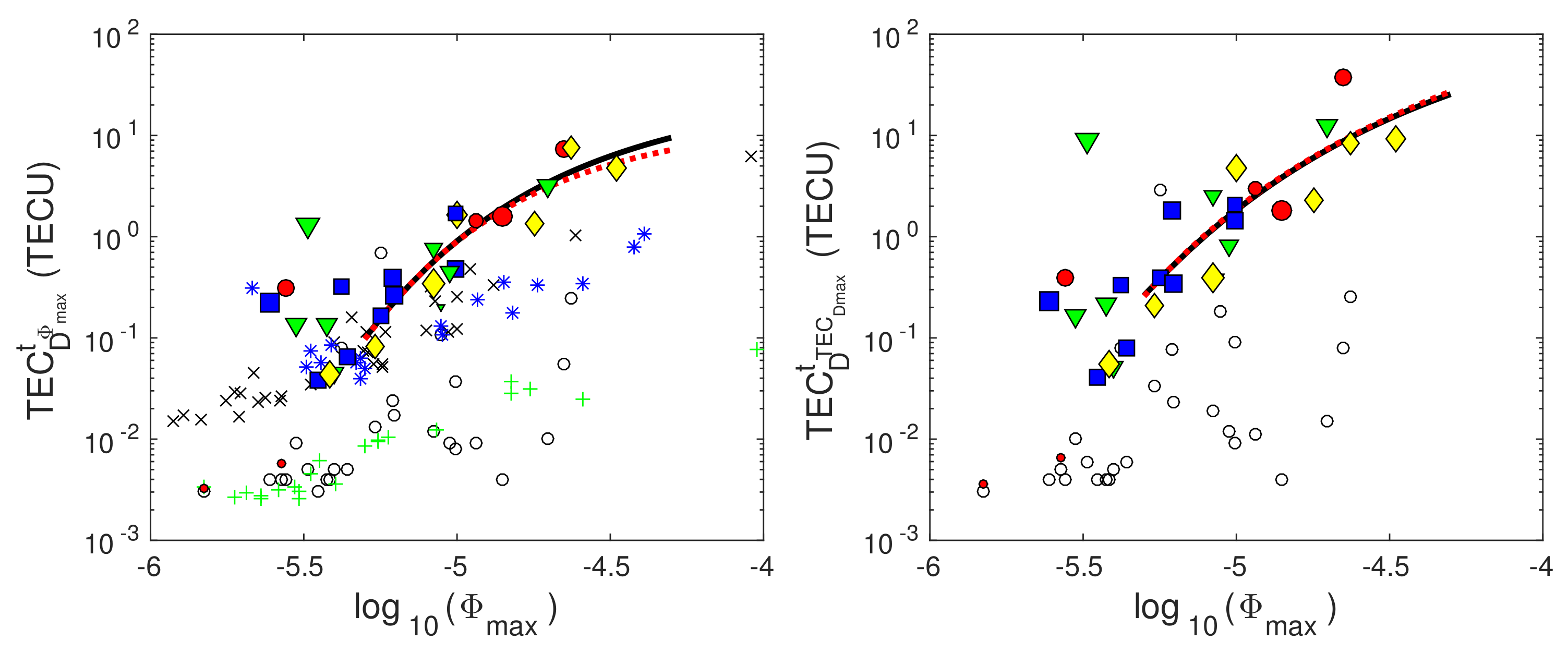
4.4. Determination of the Total Electron Content in the D-Region
- The values of TEC are higher at the time . This is consistent with the change in the intensity of the D-region perturbation which is the largest at this time.
- Due to the additional influence of the differences in the X-ray flux time evolutions after the analysed flare intensity maxima, the dispersion of the shown points is more pronounced at the time .
- The influence of seasonal variations is not pronounced.
- The effect of the X-ray flux variation during a solar cycle is visible only for the considered weak flares that occurred in the period around the solar cycle minimum.
- The dispersion of points representing weak flares is expressed at both times due to the significant influence of the initial conditions on the characteristics of the corresponding perturbations.
- The influence of initial conditions on a perturbation decreases with the X-ray flux. This reduces the dispersion of the obtained points and, consequently, allows the fitting of points that represent flares for which Wm and 50 (black lines on the chart). In both cases, the fitted function has the form given by Equation (10), where the corresponding parameters a, b, and c are given in Supplementary Materials (Table S2).
- As in the case of the parameter , the values of TEC obtained by the presented procedure are greater than those obtained for the initial values of Wait’s parameters 0.3 km and 74 km in both observed times (except in one case). The tendency of the dependence TEC is more pronounced in the first than in the second case.
- Compared to the studies presented in [18,23,35], the values obtained in this paper are in good agreement with:
- -
- -
- The values obtained from Wait’s parameters presented in [18] for two weak X-ray flares that occurred in the period around the solar cycle minimum (2010).
The values obtained in this study are greater in the cases of the other considered weak X-ray flares and in the cases of more intense ones.
5. Conclusions
- The choice of Wait’s parameters describing the quiet ionosphere affect the time evolutions of the considered parameters during the entire period of a perturbation induced by an X-ray flare.
- The influence of the quiet ionosphere state in the period preceding perturbation on the electron density and total electron content in the perturbed D-region is significant for weak X-ray flares.
- The influence of the initial conditions on the considered ionospheric parameters at the times of the radiation maximum and the most intense D-region perturbation decreases with the X-ray flux.
- Significant differences caused by the variations in the radiation intensity during the 24th solar cycle are obtained for:
- -
- The “sharpness”—the obtained values are significantly lower for events that occurred in the period around the solar cycle minimum;
- -
- The electron density in the D-region upper part—the obtained values are significantly lower for weak solar X-ray flares that occurred in the period around the solar cycle minimum;
- -
- The total electron content in the D-region—the obtained values are significantly lower for weak solar X-ray flares that occurred in the period around the solar cycle minimum.
The stated differences are obtained at the times of the X-ray flux maximum and the most intense D-region disturbance. The variations in the radiation intensity during the 24th solar cycle do not affect the signal reflection height. - The influence of the seasonal variations on the analysed parameters is not significant at the times of the X-ray flux maximum and the most intense D-region disturbance.
- Due to the pronounced influence of the quiet ionosphere state before perturbation on the analysed parameters in the cases of weak flares, the dispersions of the points describing these events on the corresponding graphs are large. For this reason, we fit only the obtained values describing the X-ray flares of class-C5 or stronger. Due to the differences induced by the variations in the radiation intensity during a solar cycle, the presented fits are relevant for the events which occurred during the days when the smoothed daily sunspot number is greater than 50.
- The obtained results indicate the need to correct the linear dependences of the observed parameters on the logarithm of the X-ray flux maximum obtained in the cases when the initial conditions are considered the same for all analysed cases. The dependences obtained by the method presented in this study indicate a tendency towards saturation of the observed values with the logarithm of the X-ray flux maximum.
- The dispersion of points representing the dependences of the observed parameters on the logarithm of the X-ray flux is higher at the time of maximum D-region perturbation than at the time of maximum X-ray flux. This can be explained by the additional influence of the difference in the time evolutions of the X-ray flux after its maximum on the ionosphere.
- The fitted functions of Wait’s parameters can be used to determine the fitted dependences of the electron density and total electron content in the D-region.
Supplementary Materials
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Raulin, J.P.; Trottet, G.; Kretzschmar, M.; Macotela, E.L.; Pacini, A.; Bertoni, F.C.P.; Dammasch, I.E. Response of the low ionosphere to X-ray and Lyman-α solar flare emissions. J. Geophys. Res. Space Phys. 2013, 118, 570–575. [Google Scholar] [CrossRef] [Green Version]
- Schmitter, E.D. Modeling solar flare induced lower ionosphere changes using VLF/LF transmitter amplitude and phase observations at a midlatitude site. Ann. Geophys. 2013, 31, 765–773. [Google Scholar] [CrossRef] [Green Version]
- Ammar, A.; Ghalila, H. Ranking of Sudden Ionospheric Disturbances by Means of the Duration of Vlf Perturbed Signal in Agreement with Satellite X-ray Flux Classification. Acta Geophys. 2016, 64, 2794–2809. [Google Scholar] [CrossRef]
- Kumar, S.; Kumar, A.; Menk, F.; Maurya, A.K.; Singh, R.; Veenadhari, B. Response of the low-latitude D region ionosphere to extreme space weather event of 14–16 December 2006. J. Geophys. Res. Space Phys. 2015, 120, 788–799. [Google Scholar] [CrossRef] [Green Version]
- Kumar, S.; NaitAmor, S.; Chanrion, O.; Neubert, T. Perturbations to the lower ionosphere by tropical cyclone Evan in the South Pacific Region. J. Geophys. Res. Space Phys. 2017, 122, 8720–8732. [Google Scholar] [CrossRef]
- Zhao, S.; Shen, X.; Liao, L.; Zhima, Z.; Zhou, C.; Wang, Z.; Cui, J.; Lu, H. Investigation of Precursors in VLF Subionospheric Signals Related to Strong Earthquakes (M > 7) in Western China and Possible Explanations. Remote Sens. 2020, 12, 3563. [Google Scholar] [CrossRef]
- Nina, A.; Nico, G.; Odalović, O.; Čadež, V.; Todorović Drakul, M.; Radovanović, M.; Popović, L.Č. GNSS and SAR Signal Delay in Perturbed Ionospheric D-Region During Solar X-ray Flares. IEEE Geosci. Remote Sens. Lett. 2020, 17, 1198–1202. [Google Scholar] [CrossRef]
- Nina, A.; Radović, J.; Nico, G.; Popović, L.Č.; Radovanović, M.; Biagi, P.F.; Vinković, D. The Influence of Solar X-ray Flares on SAR Meteorology: The Determination of the Wet Component of the Tropospheric Phase Delay and Precipitable Water Vapor. Remote Sens. 2021, 13, 2609. [Google Scholar] [CrossRef]
- Bilitza, D. IRI the International Standard for the Ionosphere. Adv. Radio Sci. 2018, 16, 1–11. [Google Scholar] [CrossRef] [Green Version]
- Thomson, N.R.; Rodger, C.J.; Clilverd, M.A. Large solar flares and their ionospheric D region enhancements. J. Geophys. Res. Space Phys. 2005, 110, A06306. [Google Scholar] [CrossRef] [Green Version]
- Han, F.; Cummer, S.A.; Li, J.; Lu, G. Daytime ionospheric D region sharpness derived from VLF radio atmospherics. J. Geophys. Res. Space Phys. 2011, 116, 5314. [Google Scholar] [CrossRef] [Green Version]
- McRae, W.M.; Thomson, N.R. VLF phase and amplitude: Daytime ionospheric parameters. J. Atmos. Sol.-Terr. Phys. 2000, 62, 609–618. [Google Scholar] [CrossRef]
- Nina, A.; Nico, G.; Mitrović, S.T.; Čadež, V.M.; Milošević, I.R.; Radovanović, M.; Popović, L.Č. Quiet Ionospheric D-Region (QIonDR) Model Based on VLF/LF Observations. Remote Sens. 2021, 13, 483. [Google Scholar] [CrossRef]
- Singh, A.K.; Singh, A.; Singh, R.; Singh, R. Solar flare induced D-region ionospheric perturbations evaluated from VLF measurements. Astrophys. Space Sci. 2014, 350, 1–9. [Google Scholar] [CrossRef]
- Ferguson, J.A. Computer Programs for Assessment of Long-Wavelength Radio Communications, Version 2.0; Space and Naval Warfare Systems Center: San Diego, CA, USA, 1998. [Google Scholar]
- Cohen, M.B.; Inan, U.S.; Paschal, E.W. Sensitive Broadband ELF/VLF Radio Reception with the AWESOME Instrument. IEEE Trans. Geosci. Remote Sens. 2010, 48, 3–17. [Google Scholar] [CrossRef]
- Nina, A.; Čadež, V.M.; Bajčetić, J.; Mitrović, S.T.; Popović, L.Č. Analysis of the Relationship Between the Solar X-ray Radiation Intensity and the D-Region Electron Density Using Satellite and Ground-Based Radio Data. Sol. Phys. 2018, 293, 64. [Google Scholar] [CrossRef]
- Basak, T.; Chakrabarti, S.K. Effective recombination coefficient and solar zenith angle effects on low-latitude D-region ionosphere evaluated from VLF signal amplitude and its time delay during X-ray solar flares. Astrophys. Space Sci. 2013, 348, 315–326. [Google Scholar] [CrossRef] [Green Version]
- Grubor, D.P.; Šulić, D.M.; Žigman, V. Classification of X-ray solar flares regarding their effects on the lower ionosphere electron density profile. Ann. Geophys. 2008, 26, 1731–1740. [Google Scholar] [CrossRef] [Green Version]
- Chakraborty, S.; Basak, T. Numerical analysis of electron density and response time delay during solar flares in mid-latitudinal lower ionosphere. Astrophys. Space Sci. 2020, 365, 1–9. [Google Scholar] [CrossRef]
- Thomson, N.R. Experimental daytime VLF ionospheric parameters. J. Atmos. Terr. Phys. 1993, 55, 173–184. [Google Scholar] [CrossRef]
- Žigman, V.; Grubor, D.; Šulić, D. D-region electron density evaluated from VLF amplitude time delay during X-ray solar flares. J. Atmos. Sol.-Terr. Phys. 2007, 69, 775–792. [Google Scholar] [CrossRef]
- McRae, W.M.; Thomson, N.R. Solar flare induced ionospheric D-region enhancements from VLF phase and amplitude observations. J. Atmos. Sol.-Terr. Phys. 2004, 66, 77–87. [Google Scholar] [CrossRef]
- Todorović Drakul, M.; Čadež, V.M.; Bajčetić, J.; Popović, L.Č.; Blagojević, D.; Nina, A. Behaviour of electron content in the ionospheric D-region during solar X-ray flares. Serb. Astron. J. 2016, 193, 11–18. [Google Scholar] [CrossRef]
- Hayes, L.A.; Gallagher, P.T.; McCauley, J.; Dennis, B.R.; Ireland, J.; Inglis, A. Pulsations in the Earth’s Lower Ionosphere Synchronized With Solar Flare Emission. J. Geophys. Res. Space Phys. 2017, 122, 9841–9847. [Google Scholar] [CrossRef] [Green Version]
- Nina, A.; Čadež, V. Electron production by solar Ly-α line radiation in the ionospheric D-region. Adv. Space Res. 2014, 54, 1276–1284. [Google Scholar] [CrossRef] [Green Version]
- Kumar, A.; Kumar, S. Solar flare effects on D-region ionosphere using VLF measurements during low- and high-solar activity phases of solar cycle 24. Earth Planets Space 2018, 70, 29. [Google Scholar] [CrossRef]
- Klobuchar, J.A. Design and characteristics of the GPS ionospheric time delay algorithm for single frequency users. In PLANS ’86—Position Location and Navigation Symposium; Institute of Electrical and Electronics Engineers: New York, NY, USA, 1986. [Google Scholar]
- Zhao, J.; Zhou, C. On the optimal height of ionospheric shell for single-site TEC estimation. GPS Solut. 2018, 22, 48. [Google Scholar] [CrossRef]
- Daniell, R.J.; Brown, L. PRISM: A Parameterized Real-Time Ionospheric Specification Model, Version 1.5; Computational Physics Inc: Newton, MA, USA, 1995. [Google Scholar]
- Nava, B.; Coïsson, P.; Radicella, S. A new version of the NeQuick ionosphere electron density model. J. Atmos. Sol.-Terr. Phys. 2008, 70, 1856–1862. [Google Scholar] [CrossRef]
- Scherliess, L.; Schunk, R.W.; Sojka, J.J.; Thompson, D.C.; Zhu, L. Utah State University Global Assimilation of Ionospheric Measurements Gauss-Markov Kalman filter model of the ionosphere: Model description and validation. J. Geophys. Res. Space Phys. 2006, 111, A11315. [Google Scholar] [CrossRef] [Green Version]
- Mechtly, E.; Bilitza, D. Models of D-Region Electron Concentration, Report IPW-WB1; Institut fur Physik Weltraumforsch: Freiburg, Germany, 1974. [Google Scholar]
- Friedrich, M.; Torkar, K.M. FIRI: A semiempirical model of the lower ionosphere. J. Geophys. Res. 2001, 106, 21409–21418. [Google Scholar] [CrossRef]
- Pandey, U.; Singh, B.; Singh, O.; Saraswat, V. Solar flare induced ionospheric D-region perturbation as observed at a low latitude station Agra, India. Astrophys. Space. Sci. 2015, 357, 35. [Google Scholar] [CrossRef]
- Nina, A.; Čadež, V.; Bajčetić, J. Contribution of Solar Hydrogen Lyα Line Emission in Total Ionization Rate in Ionospheric D-region During the Maximum of Solar X-flare. Serb. Astron. J. 2015, 191, 51–57. [Google Scholar] [CrossRef]




| No | Date | Time (UT) | Flare Class | ||
|---|---|---|---|---|---|
| 1 | 5 May 2010 | 11:37 | C8.8 | 10.714 | 0.345 |
| 2 | 13 July 2010 | 10:43 | C2.6 | 18.381 | 0.534 |
| 3 | 14 July 2010 | 12:11 | C1.4 | 18.524 | 0.537 |
| 4 | 14 January 2012 | 12:00 | C4.1 | 96.952 | 0.038 |
| 5 | 16 January 2012 | 10:31 | C5.5 | 101.190 | 0.044 |
| 6 | 21 March 2012 | 12:38 | C2.9 | 86.333 | 0.222 |
| 7 | 9 April 2012 | 12:12 | C3.9 | 71.000 | 0.274 |
| 8 | 25 April 2012 | 12:07 | C3.7 | 83.238 | 0.318 |
| 9 | 2 May 2012 | 11:32 | C3.2 | 107.952 | 0.337 |
| 10 | 29 June 2012 | 09:13 | M2.2 | 72.952 | 0.496 |
| 11 | 30 June 2012 | 10:48 | C2.7 | 72.857 | 0.499 |
| 12 | 8 October 2012 | 11:05 | M2.3 | 78.524 | 0.773 |
| 13 | 20 November 2012 | 12:36 | M1.7 | 88.571 | 0.890 |
| 14 | 5 November 2013 | 11:51 | C8.0 | 130.905 | 0.849 |
| 15 | 8 January 2014 | 11:56 | C6.1 | 124.571 | 0.022 |
| 16 | 18 January 2014 | 11:57 | C6.0 | 122.000 | 0.049 |
| 17 | 1 February 2014 | 10:43 | C3.5 | 106.048 | 0.088 |
| 18 | 3 February 2014/2/3 | 10:58 | C4.4 | 105.810 | 0.093 |
| 19 | 2 March 2014 | 11:55 | C2.4 | 149.571 | 0.170 |
| 20 | 1 July 2014 | 11:05 | M1.4 | 102.714 | 0.501 |
| 21 | 29 October 2014 | 11:02 | C5.3 | 86.048 | 0.827 |
| 22 | 7 November 2014 | 10:13 | M1.0 | 107.905 | 0.855 |
| 23 | 15 November 2014 | 11:40 | M3.2 | 100.143 | 0.877 |
| 24 | 13 December 2014 | 10:49 | C3.8 | 108.810 | 0.953 |
| 25 | 6 January 2015 | 11:40 | C9.7 | 112.571 | 0.016 |
| 26 | 21 January 2015 | 11:32 | C9.9 | 87.619 | 0.058 |
| 27 | 6 May 2015 | 11:45 | M1.9 | 84.857 | 0.348 |
| 28 | 4 June 2015 | 09:36 | C8.1 | 60.238 | 0.427 |
| 29 | 17 September 2015 | 09:34 | M1.1 | 53.952 | 0.715 |
| 30 | 14 May 2016 | 11:28 | C7.4 | 68.619 | 0.370 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Nina, A. Modelling of the Electron Density and Total Electron Content in the Quiet and Solar X-ray Flare Perturbed Ionospheric D-Region Based on Remote Sensing by VLF/LF Signals. Remote Sens. 2022, 14, 54. https://doi.org/10.3390/rs14010054
Nina A. Modelling of the Electron Density and Total Electron Content in the Quiet and Solar X-ray Flare Perturbed Ionospheric D-Region Based on Remote Sensing by VLF/LF Signals. Remote Sensing. 2022; 14(1):54. https://doi.org/10.3390/rs14010054
Chicago/Turabian StyleNina, Aleksandra. 2022. "Modelling of the Electron Density and Total Electron Content in the Quiet and Solar X-ray Flare Perturbed Ionospheric D-Region Based on Remote Sensing by VLF/LF Signals" Remote Sensing 14, no. 1: 54. https://doi.org/10.3390/rs14010054
APA StyleNina, A. (2022). Modelling of the Electron Density and Total Electron Content in the Quiet and Solar X-ray Flare Perturbed Ionospheric D-Region Based on Remote Sensing by VLF/LF Signals. Remote Sensing, 14(1), 54. https://doi.org/10.3390/rs14010054







