3D Wavelet Finite-Element Modeling of Frequency-Domain Airborne EM Data Based on B-Spline Wavelet on the Interval Using Potentials
Abstract
:1. Introduction
2. Methodology
2.1. Governing Equations
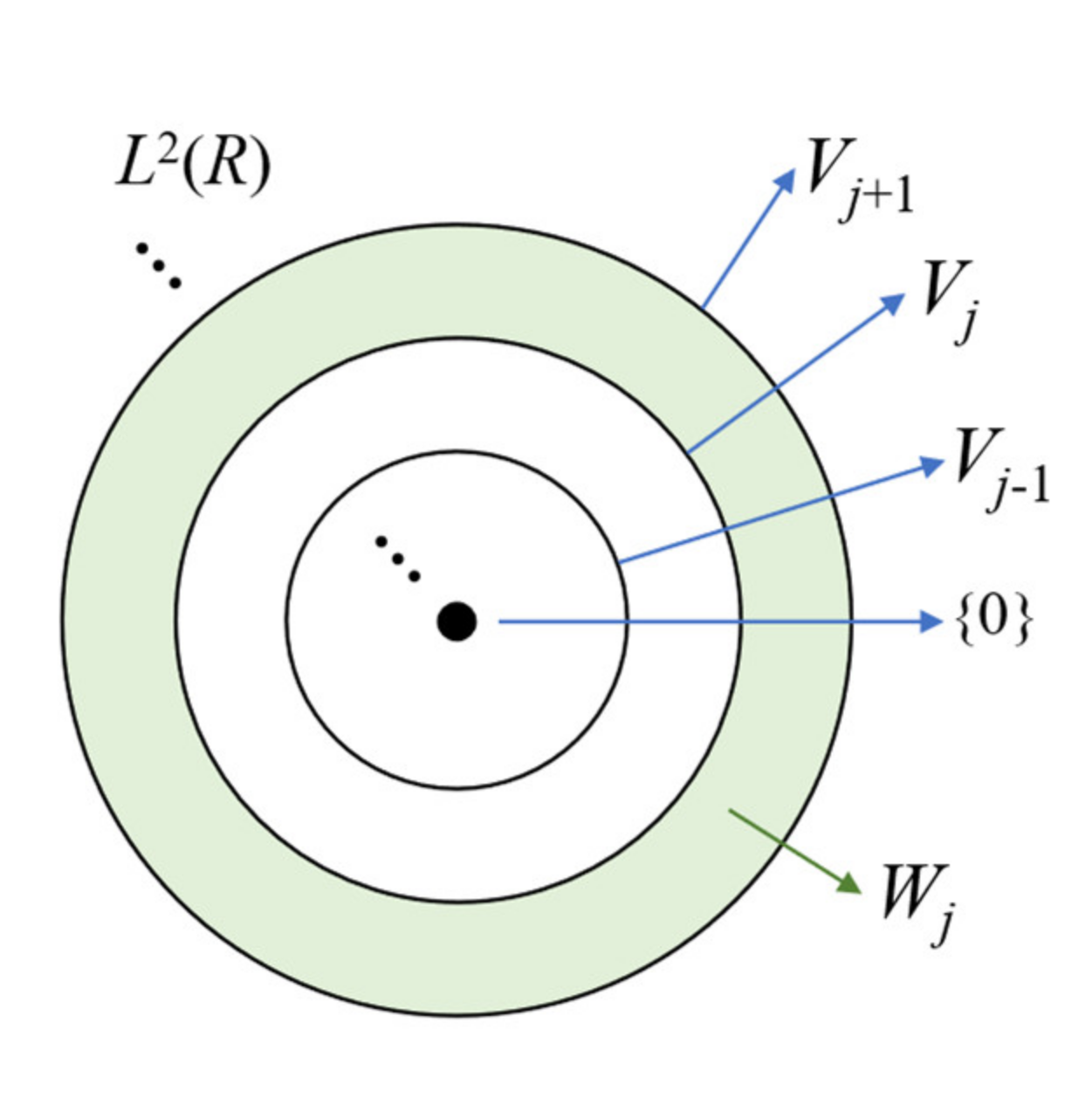
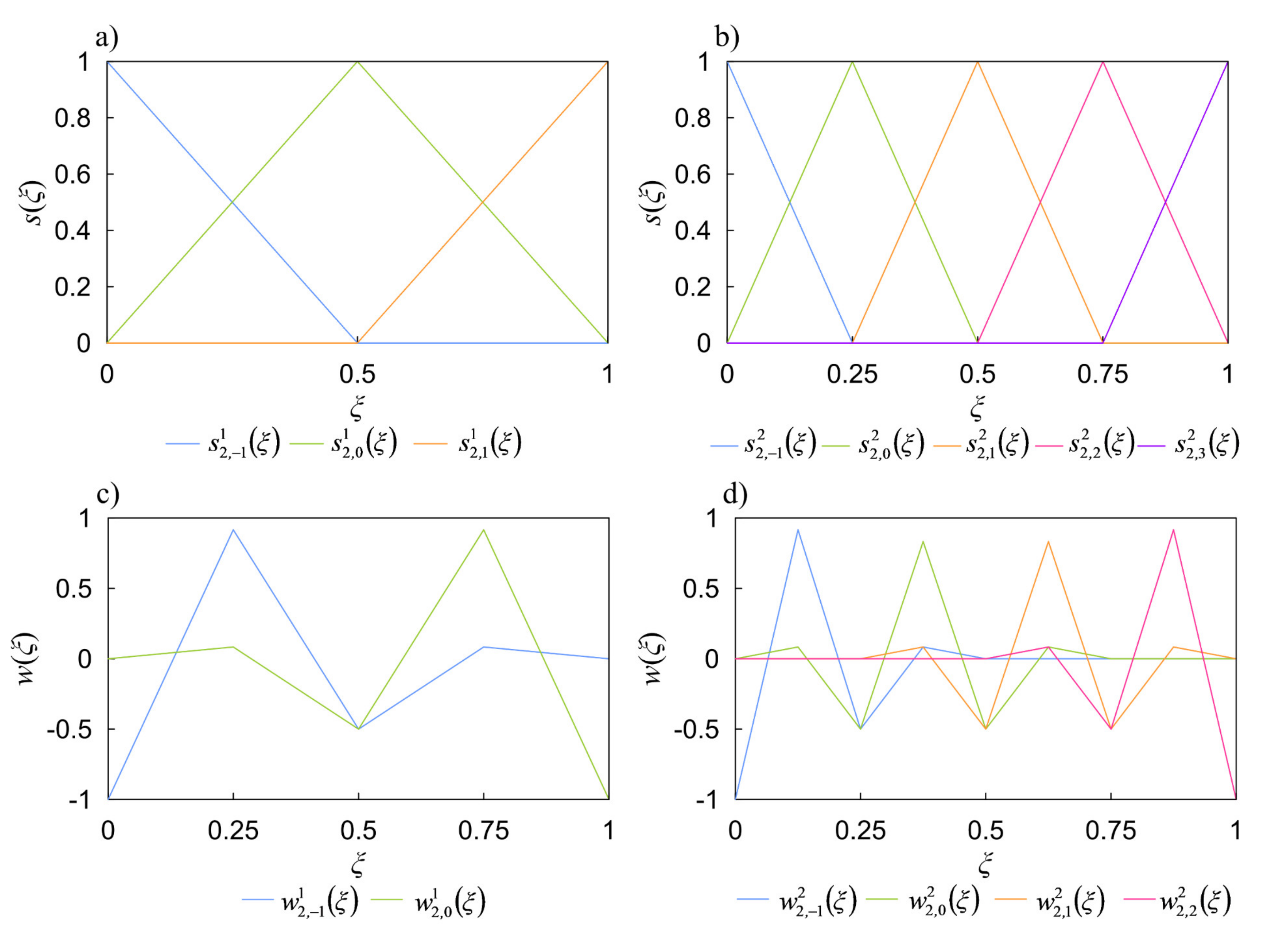
2.2. B-Spline Wavelet on the Interval
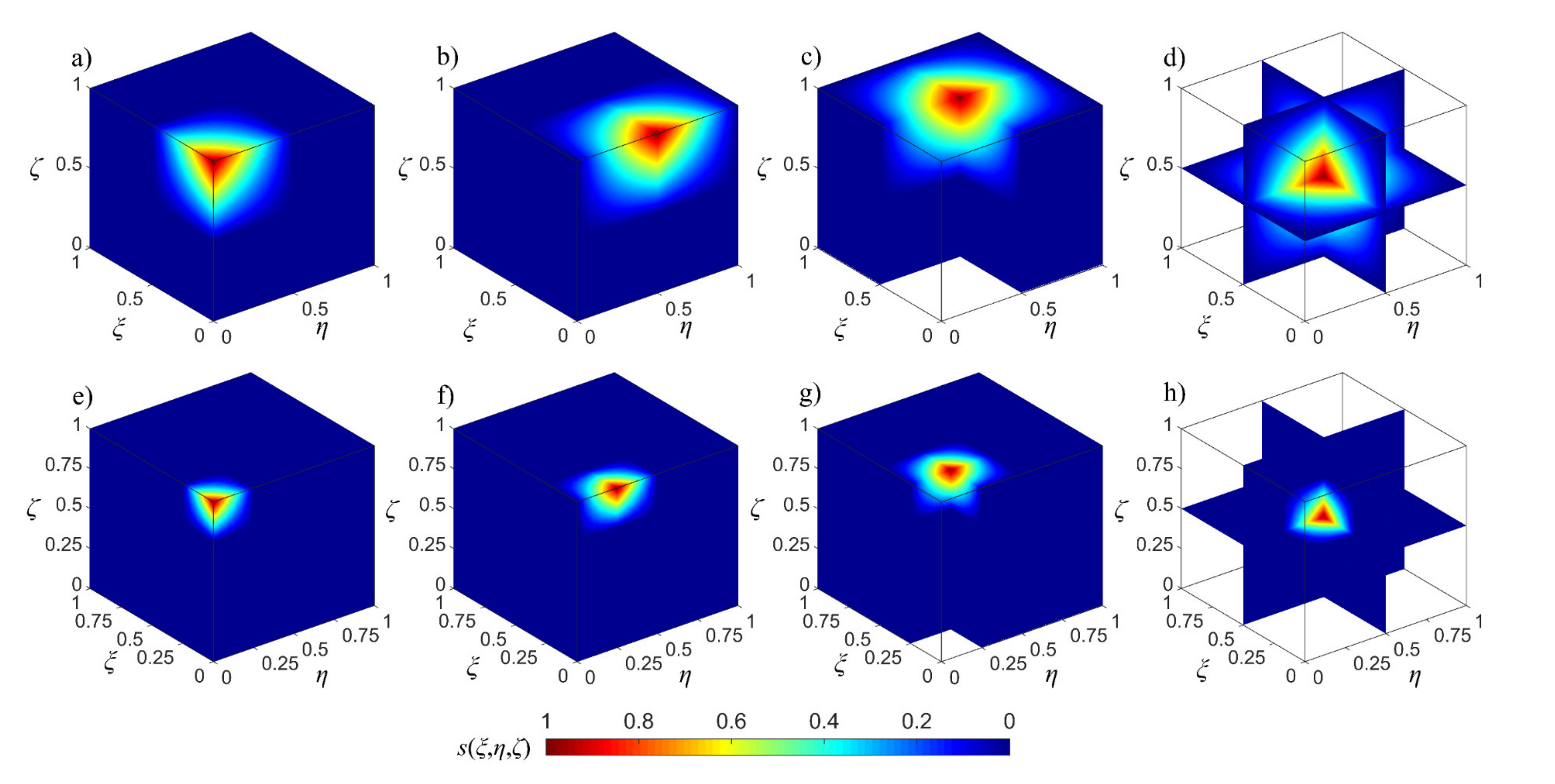
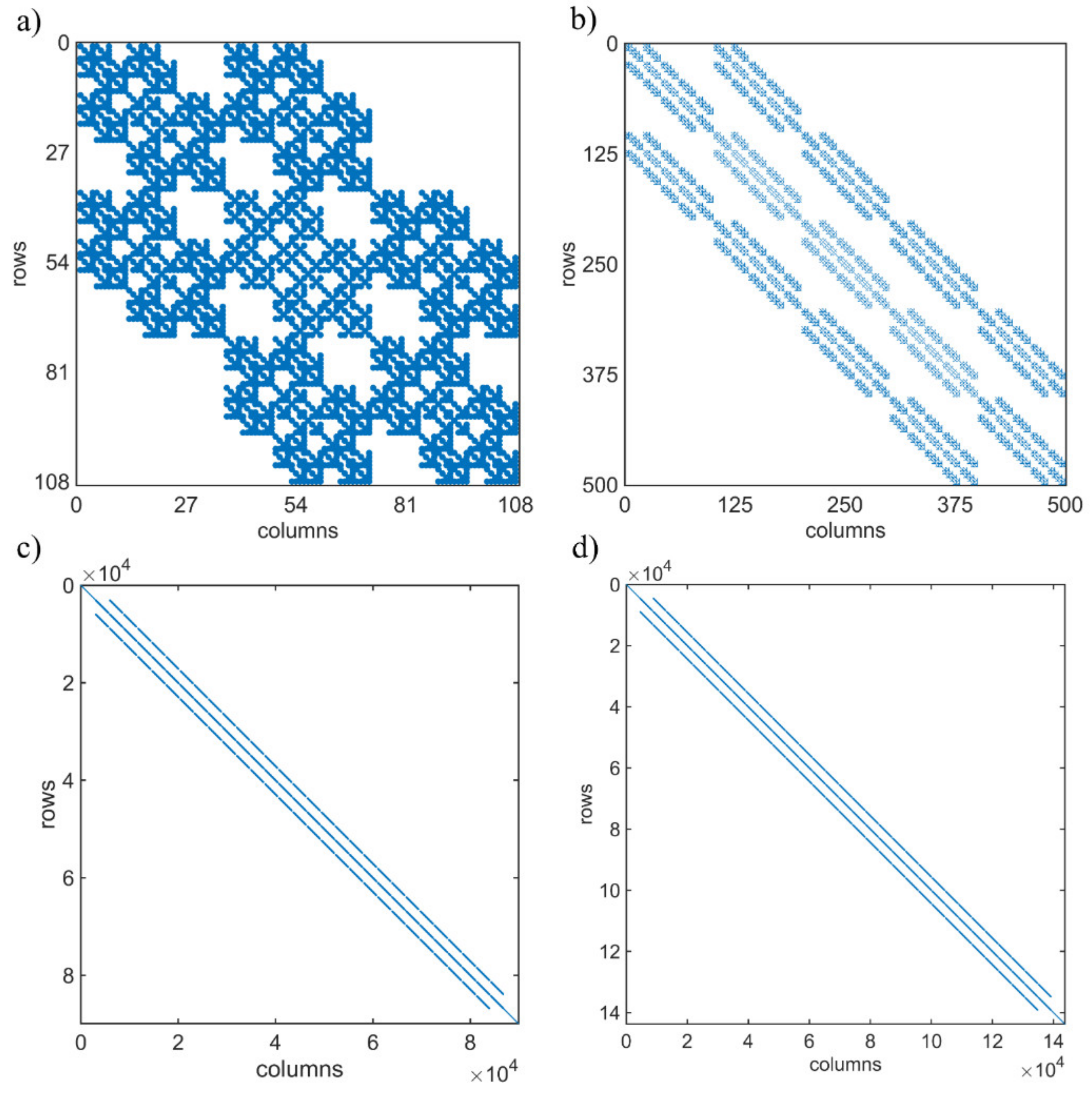
2.3. BSWI Based Wavelet Finite-Element Method
2.4. WFEM Analysis
2.5. Moving Least-Squares Interpolation
3. Numerical Experiments
3.1. Accuracy Verification
3.2. 3D Synthetic Models
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
Appendix A. The Connection Coefficients
References
- Pfaffhuber, A.A.; Monstad, S.; Rudd, J. Airborne electromagnetic hydrocarbon mapping in Mozambique. Explor. Geophys. 2009, 40, 237–245. [Google Scholar] [CrossRef]
- Tan, K.; Munday, T.; Halas, L.; Cahill, K. Utilizing airborne electromagnetic data to map groundwater salinity and salt store at Chowilla, SA. ASEG Ext. Abstr. 2009, 2009, 1–6. [Google Scholar] [CrossRef]
- Yang, D.K.; Oldenburg, D.W. 3D conductivity models of Lalor Lake VMS deposit from ground loop and airborne EM data sets. In Proceedings of the 23rd International Geophysical Conference and Exhibition, Melbourne, Australia, 11–14 August 2013. [Google Scholar]
- Wolfgram, P.; Golden, H. Airborne EM applied to sulphide nickel-examples and analysis. Explor. Geophys. 2001, 32, 136–140. [Google Scholar] [CrossRef]
- Hodges, G.; Aniston, C. Airborne Geophysics applied to Engineering and Environmental Problems. In Proceedings of the International Conference on Engineering Geophysics, Al Ain, United Arab Emirates, 15–18 November 2015. [Google Scholar]
- Siemon, B.; Seht, M.; Steuer, A.; Deus, N.; Wiederhold, H. Airborne Electromagnetic, Magnetic, and Radiometric Surveys at the German North Sea Coast Applied to Groundwater and Soil Investigations. Remote Sens. 2020, 12, 1629. [Google Scholar] [CrossRef]
- Best, M.E.; Levson, V.M.; Ferbey, T.; McConnell, D. Airborne electromagnetic mapping for buried quaternary sands and gravels in northeast British Columbia, Canada. J. Environ. Eng. Geophys. 2006, 11, 17–26. [Google Scholar] [CrossRef]
- Avdeev, D.B.; Kuvshinov, A.V.; Pankratov, O.V.; Newman, G.A. Three-dimensional frequency-domain modeling of airborne electromagnetic responses. Explor. Geophys. 1998, 29, 111–119. [Google Scholar] [CrossRef]
- Newman, G.A.; Alumbaugh, D.L. Frequency-domain modelling of airborne electromagnetic responses using staggered finite differences1. Geophys. Prospect. 1995, 43, 1021–1042. [Google Scholar] [CrossRef]
- Liu, Y.; Yin, C. 3D anisotropic modeling for airborne EM systems using finite-difference method. J. Appl. Geophys. 2014, 109, 186–194. [Google Scholar] [CrossRef]
- Yin, C.; Zhang, B.; Liu, Y.; Cai, J. A goal-oriented adaptive finite-element method for 3D scattered airborne electromagnetic method modeling. Geophysics 2016, 81, 337–346. [Google Scholar] [CrossRef]
- Huang, W.; Yin, C.; Ben, F.; Liu, Y.; Chen, H.; Cai, J. 3D forward modeling for frequency AEM by vector finite element. Earth Sci. 2016, 41, 331–342. (In Chinese) [Google Scholar]
- Yee, K.S. Numerical solution of initial boundary value problems involving maxwell’s equations in isotropic media. IEEE Trans. Antennas Propag. 1966, 14, 302–307. [Google Scholar]
- Yin, C.; Huang, X.; Liu, Y.; Cai, J. 3-D modeling for airborne EM using spectral-element method. J. Environ. Eng. Geophys. 2017, 22, 13–23. [Google Scholar] [CrossRef]
- Octavio, C.R.; Josep, D.L.P.; Emilio, G.C.; José, M.C. Parallel 3D marine controlled-source electromagnetic modelling using high-order tetrahedral Nédélec elements. Geophys. J. Int. 2019, 219, 39–65. [Google Scholar]
- Schwarzbach, C.; Börner, R.; Spitzer, K. Three-dimensional adaptive higher order finite element simulation for geo-electromagnetics-a marine CSEM example. Geophys. J. Int. 2011, 187, 63–74. [Google Scholar] [CrossRef] [Green Version]
- Runge, C. Über empirische Funktionen und die Interpolation zwischen äquidistanten Ordinaten. Z. Math. Phys. 1901, 46, 224–243. [Google Scholar]
- Morlet, J.; Arens, G.; Fourgeau, E.; Giard, D. Wave propagation and sampling theory-Part II: Sampling theory and complex waves. Geophysics 1982, 47, 222–236. [Google Scholar] [CrossRef]
- Meyer, Y. Principe d’incertitude, bases hilbertiennes et algèbres d’opérateurs. Semin. Bourbaki Paris 1986, 4, 209–223. [Google Scholar]
- Grossmann, A.; Morlet, J. Decomposition of Hardy Functions into Square Integrable Wavelets of Constant Shape. SIAM J. Math. Anal. 1984, 15, 723–736. [Google Scholar] [CrossRef]
- Mallat, S.G. Multiresolution approximations and wavelet orthonormal bases of L2(R). Trans. Am. Math. Soc. 1989, 315, 69–87. [Google Scholar]
- Daubechies, I. Orthonormal bases of compactly supported wavelets. Commun. Pure Appl. Math. 1989, 3115, 69–81. [Google Scholar]
- Sweldens, W. The Lifting Scheme: A Custom-Design Construction of Biorthogonal Wavelets. Appl. Comput. Harmon. Anal. 1996, 3, 186–200. [Google Scholar] [CrossRef] [Green Version]
- Donoho, D.L.; Johnstone, I.M. Ideal spatial adaptation by wavelet shrinkage. Biometrika 1994, 81, 425–455. [Google Scholar] [CrossRef]
- Mallat, S.G. A Wavelet Tour of Signal Processing; Academic Press: Cambridge, MA, USA, 1999. [Google Scholar]
- Plattner, A.; Maurer, H.R.; Vorloeper, J.; Blome, J. 3-D electrical resistivity tomography using adaptive wavelet parameter grids. Geophys. J. Int. 2012, 189, 317–330. [Google Scholar] [CrossRef] [Green Version]
- Liu, Y.; Farquharson, C.G.; Yin, C.; Vikas, C.B. Wavelet-based 3D inversion for frequency-domain airborne EM data. Geophys. J. Int. 2017, 213, 1–15. [Google Scholar] [CrossRef]
- Christensen, N. Interpretation attributes derived from airborne electromagnetic inversion models using the continuous wavelet transform. Near Surf. Geophys. 2018, 16, 665–678. [Google Scholar] [CrossRef]
- Hustdet, B.; Operto, S.; Virieux, J. A multi-level direct-iterative solver for seismic wave propagation modelling: Space and wavelet approaches. Geophys. J. Int. 2003, 155, 953–980. [Google Scholar] [CrossRef] [Green Version]
- Chakraborty, A.; Okaya, D. Frequency-time decomposition of seismic data using wavelet-based methods. Geophysics 1995, 60, 1906–1916. [Google Scholar] [CrossRef] [Green Version]
- Amaratunga, K.; Williams, J.R. Wavelet based Green’s function approach to 2D PDEs. Eng. Comput. 1993, 10, 349–367. [Google Scholar] [CrossRef] [Green Version]
- Cohen, A.; Kaber, S.M.; Postel, M. Adaptive multiresolution for finite volume solutions of gas dynamics. Comput. Fluids 2003, 32, 31–38. [Google Scholar] [CrossRef]
- Sarkar, T.K.; Adve, R.S.; Garcia-Castillo, L.E.; Salazar-Palma, M. Utilization of wavelet concepts in finite elements for an efficient solution of Maxwell’s equations. Radio Sci. 1994, 29, 965–977. [Google Scholar] [CrossRef]
- Castro, L.M.; Barbosa, A.R. Implementation of an hybrid-mixed stress model based on the use of wavelets. Comput. Struct. 2006, 84, 718–731. [Google Scholar] [CrossRef]
- Zhang, X.; Liu, K.; Liu, J. A wavelet finite element method for the 2D wave equation in fluid-saturated porous media. Chin. J. Geophys. 2005, 48, 1156–1166. [Google Scholar] [CrossRef]
- Feng, D.; Yang, B.; Wang, X.; Du, H. Daubechies wavelet finite element method for solving the GPR wave equations. Chin. J. Geophys. 2016, 59, 342–354. [Google Scholar]
- Hussain, N.; Karsiti, M.N.; Joeti, V.; Yahya, N. Use of wavelets in marine controlled source electromagnetic method for geophysical modeling. Int. J. Appl. Electromagn. Mech. 2016, 51, 431–443. [Google Scholar] [CrossRef]
- Hussain, N.; Karsiti, M.N.; Yahya, N.; Joeti, V.; Yahya, N. 2D wavelets galerkin method for the computation of EM field on seafloor excited by a point source. Int. J. Appl. Electromagn. Mech. 2017, 53, 631–644. [Google Scholar] [CrossRef]
- Chen, H.; Li, T. 3D marine controlled-source electromagnetic modelling in an anisotropic medium using a Wavelet–Galerkin method with a secondary potential formulation. Geophys. J. Int. 2019, 219, 373–393. [Google Scholar] [CrossRef]
- He, Y.; Chen, X.; Xiang, J.; He, Z. Adaptive multiresolution finite element method based on second generation wavelets. Finite Elem. Anal. Des. 2007, 43, 566–579. [Google Scholar] [CrossRef]
- Castro, L. Polynomial wavelets in hybrid-mixed stress finite element models. Int. J. Numer. Methods Biomed. Eng. 2010, 26, 1293–1312. [Google Scholar] [CrossRef]
- Chui, C.K.; Quak, E. Wavelets on a bounded interval. In Numerical Methods in Approximation Theory; Birkhäuser: Basel, Switzerland, 1992; Volume 9, pp. 53–75. [Google Scholar]
- Schoenberg, J. Contribution to the problem of approximation of equidistant data by analytic functions. Quart. Appl. Math. 1946, 4, 112–141. [Google Scholar] [CrossRef] [Green Version]
- Goswami, J.C.; Chan, A.K.; Chui, C.K. On solving first-kind integral equations using wavelets on a bounded interval. IEEE Trans. Antennas Propag. 1995, 43, 614–622. [Google Scholar] [CrossRef]
- Xiang, J.; Chen, X.; He, Y.; He, Z. The construction of plane elastomechanics and Mindlin plate elements of B-spline wavelet on the interval. Finite Elem. Anal. Des. 2006, 42, 1269–1280. [Google Scholar] [CrossRef]
- Shen, W.; Li, D.; Ou, J. Dispersion Analysis of Multiscale Wavelet Finite Element for 2D Elastic Wave Propagation. J. Eng. Mech. 2020, 146, 04020022. [Google Scholar] [CrossRef]
- Feng, D.; Wang, X.; Zhang, B.; Ren, Z. An investigation into the electromagnetic response of porous media with GPR using stochastic processes and FEM of B-spline wavelet on the interval. J. Appl. Geophys. 2019, 169, 174–182. [Google Scholar] [CrossRef]
- Barton, M.L.; Cendes, A.J. New vector finite elements for three-dimensional magnetic field computation. J. Appl. Phys. 1987, 61, 3919–3921. [Google Scholar] [CrossRef]
- Biro, O.; Preis, K. On the use of the magnetic vector potential in the finite element analysis of the three-dimensional eddy currents. IEEE Trans. Magn. 1989, 25, 3145–3159. [Google Scholar] [CrossRef]
- Nédéléc, J.C. Mixed finite element in R3. Numer. Math. 1980, 35, 315–341. [Google Scholar] [CrossRef]
- Ward, S.H.; Hohmann, G.W. Electromagnetic theory for geophysical applications. In Electromagnetic Method in applied Geophysics; Society of Exploration Geophysicists: Tulsa, OK, USA, 1988; pp. 131–311. [Google Scholar]
- Badea, E.A.; Everett, M.E.; Newman, G.A.; Biro, O. Finite-element analysis of controlled-source electromagnetic induction using Coulomb-gauged potentials. Geophysics 2001, 66, 786–799. [Google Scholar] [CrossRef]
- Everett, M.E.; Schultz, A. Geomagnetic induction in a heterogeneous sphere: Azimuthally symmetric test computations and the response of an undulating 660-km discontinuity. J. Geophys. Res. Solid Earth 1996, 101, 2765–2783. [Google Scholar] [CrossRef]
- Mitsuhata, Y.; Uchida, T. 3D magnetotelluric modeling using the T-Ω method. Geophysics 2004, 69, 108–119. [Google Scholar] [CrossRef]
- Puzyrev, V.; Koldan, J.; Puente, J.; Houzeaux, G.; Vázquez, M.; Cela, J.M. A parallel finite-element method for three-dimensional controlled-source electromagnetic forward modelling. Geophys. J. Int. 2013, 193, 678–693. [Google Scholar] [CrossRef] [Green Version]
- Weiss, C.J. Project APhiD: A Lorenz-gauged A-ϕ decomposition for parallelized computation of ultra-broadband electromagnetic induction in a fully heterogeneous earth. Comput. Geosci. 2013, 58, 40–52. [Google Scholar] [CrossRef]
- Yang, S.; Ni, G. Wavelet-Galerkin method for computations of electromagnetic fields-computation of connection coefficients. IEEE Trans. Magn. 2000, 36, 644–648. [Google Scholar] [CrossRef] [Green Version]
- Lin, E.; Zhou, X. Connection coefficients on an interval and wavelet solutions of Burgers equation. J. Comput. Appl. Math. 2001, 135, 63–78. [Google Scholar] [CrossRef] [Green Version]
- Amestoy, P.R.; Duff, I.S.; L’Excellent, J.Y.; Koster, J. A fully asynchronous multifrontal solver using distributed dynamic scheduling. SIAM J. Matrix Anal. Appl. 2001, 23, 15–41. [Google Scholar] [CrossRef] [Green Version]
- Amestoy, P.R.; Duff, I.S.; L’Excellent, J.Y.; Robert, Y.; Rouet, F.H.; Ucar, B. On computing inverse entries of a sparse matrix in an out-of-core environment. SIAM J. Sci. Comput. 2012, 34, 1975–1999. [Google Scholar] [CrossRef] [Green Version]
- Tabbara, M.; Blacker, T.; Belytschko, T. Finite element derivative recovery by moving least square interpolants. Comput. Methods Appl. Mech. Eng. 1994, 117, 211–223. [Google Scholar] [CrossRef]
- Yin, C.; Fraser, D.C. The effect of the electrical anisotropy on the response of helicopter-borne frequency-domain electromagnetic systems. Geophys. Prospect. 2004, 52, 399–416. [Google Scholar] [CrossRef]
- Yin, C.; Liu, L.; Liu, Y.; Zhang, B.; Qiu, C.; Huang, X. 3D frequency-domain airborne EM forward modelling using spectral element method with gauss–lobatto–chebyshev polynomials. Explor. Geophys. 2019, 50, 461–471. [Google Scholar] [CrossRef]



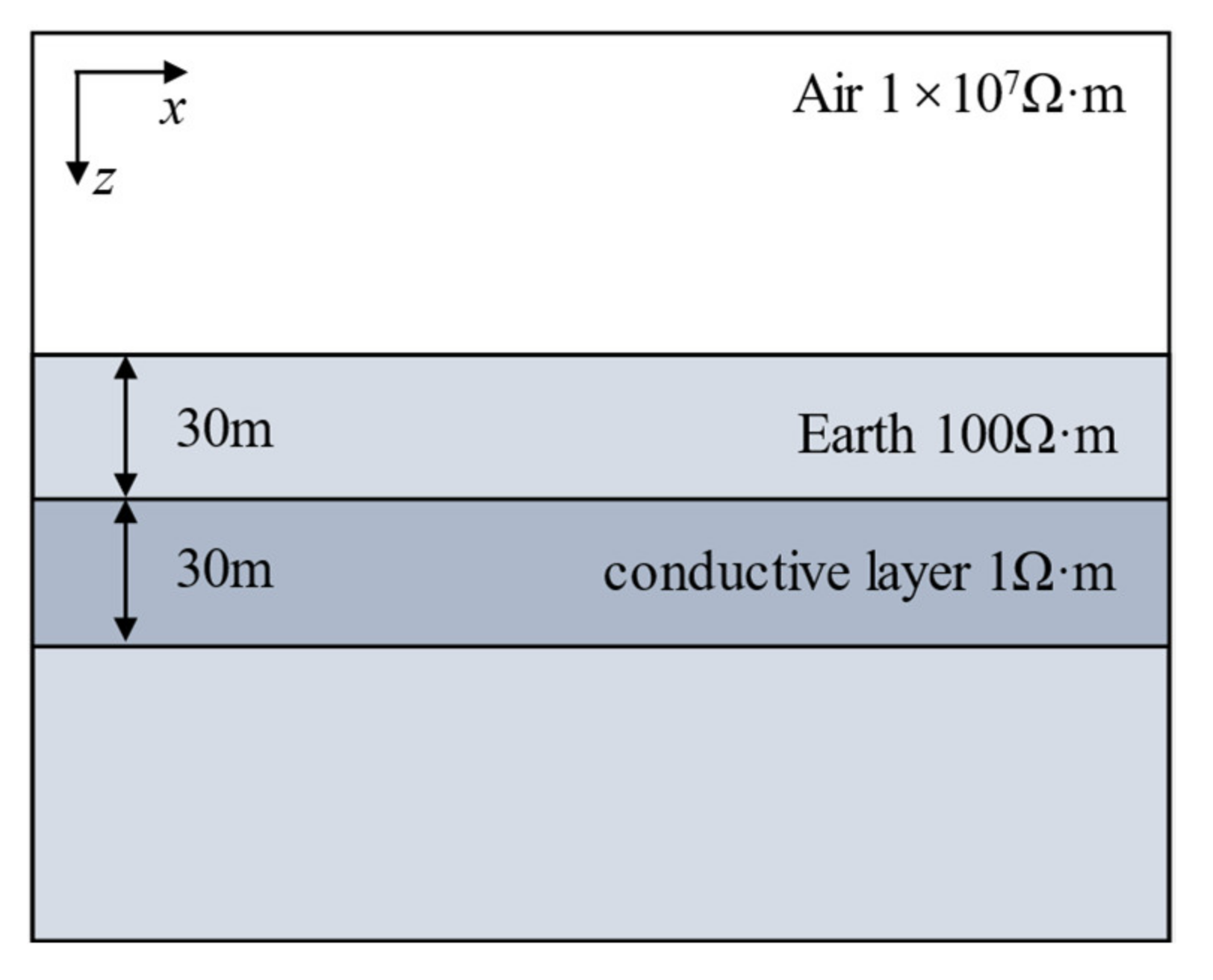

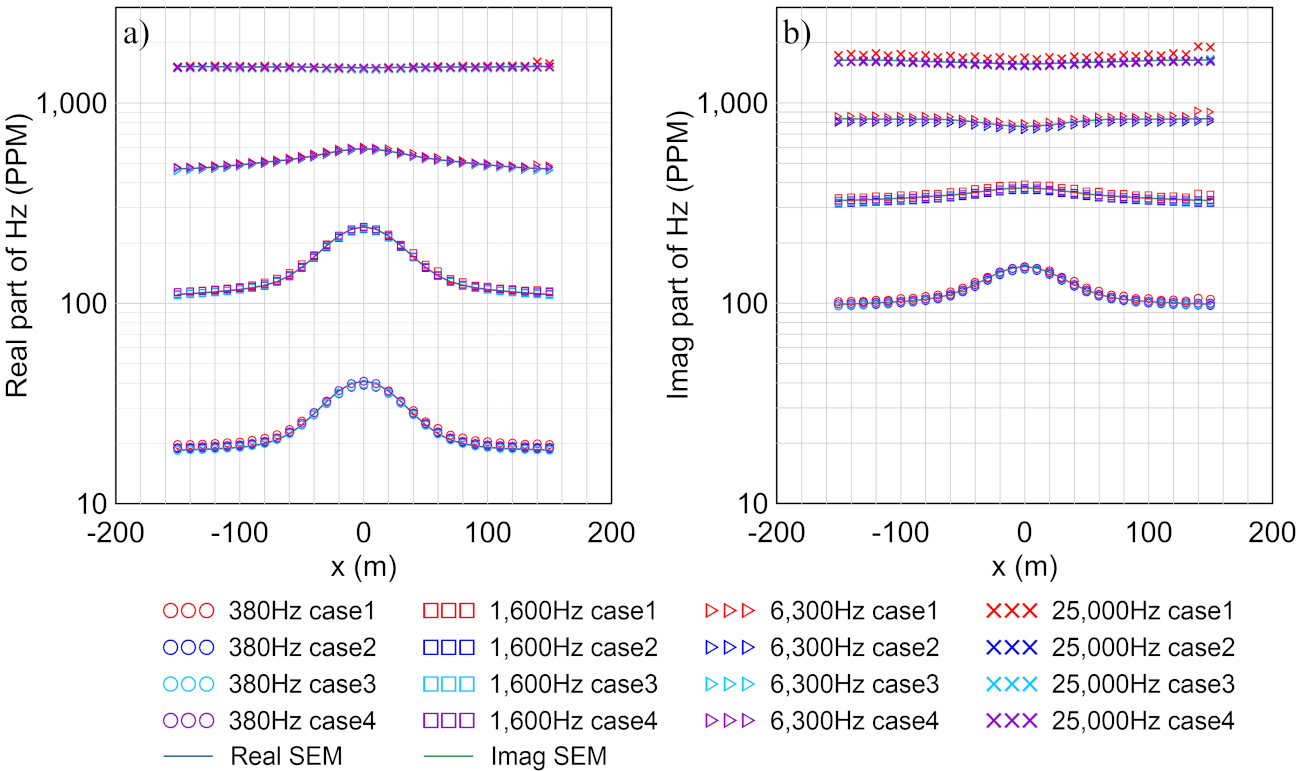
| Type of Elements | Case | Number of Meshes | DOFs | Time Consumption (at 100 Hz) |
|---|---|---|---|---|
| BSWI21 | 1 | 10 × 10 × 10 | 37,044 | 4.390 s |
| - | 2 | 14 × 14 × 14 | 97,556 | 15.161 s |
| - | 3 | 25 × 25 × 25 | 530,604 | 340.165 s |
| BSWI22 | 1 | 10 × 10 × 10 | 275,684 | 100.281 s |
| - | 2 | 14 × 14 × 14 | 740,772 | 673.973 s |
| Method | Case | Number of Meshes | DOFs | Maximum Mesh Size | Minimum Mesh Size | Time Consumption (at 100 Hz) |
|---|---|---|---|---|---|---|
| WFEM (BSWI21) | 1 | 21 × 21 × 24 | 362,404 | 80 m × 80 m × 80 m | 10 m × 10 m × 10 m | 162.929 s |
| Edge-based FEM | 1 | 48 × 48 × 52 | 374,164 | 40 m × 40 m × 40 m | 5 m × 5 m × 5 m | 83.226 s |
| - | 2 | 54 × 54 × 58 | 525,950 | 20 m × 20 m × 20 m | 2.5 m × 2.5 m × 2.5 m | 158.505 s |
| Nodal-based FEM | 1 | 42 × 42 × 48 | 362,404 | 40 m × 40 m × 40 m | 5 m × 5 m × 5 m | 176.186 s |
| - | 2 | 42 × 42 × 52 | 391,988 | 40 m × 40 m × 40 m | 2.5 m × 2.5 m × 2.5 m | 184.530 s |
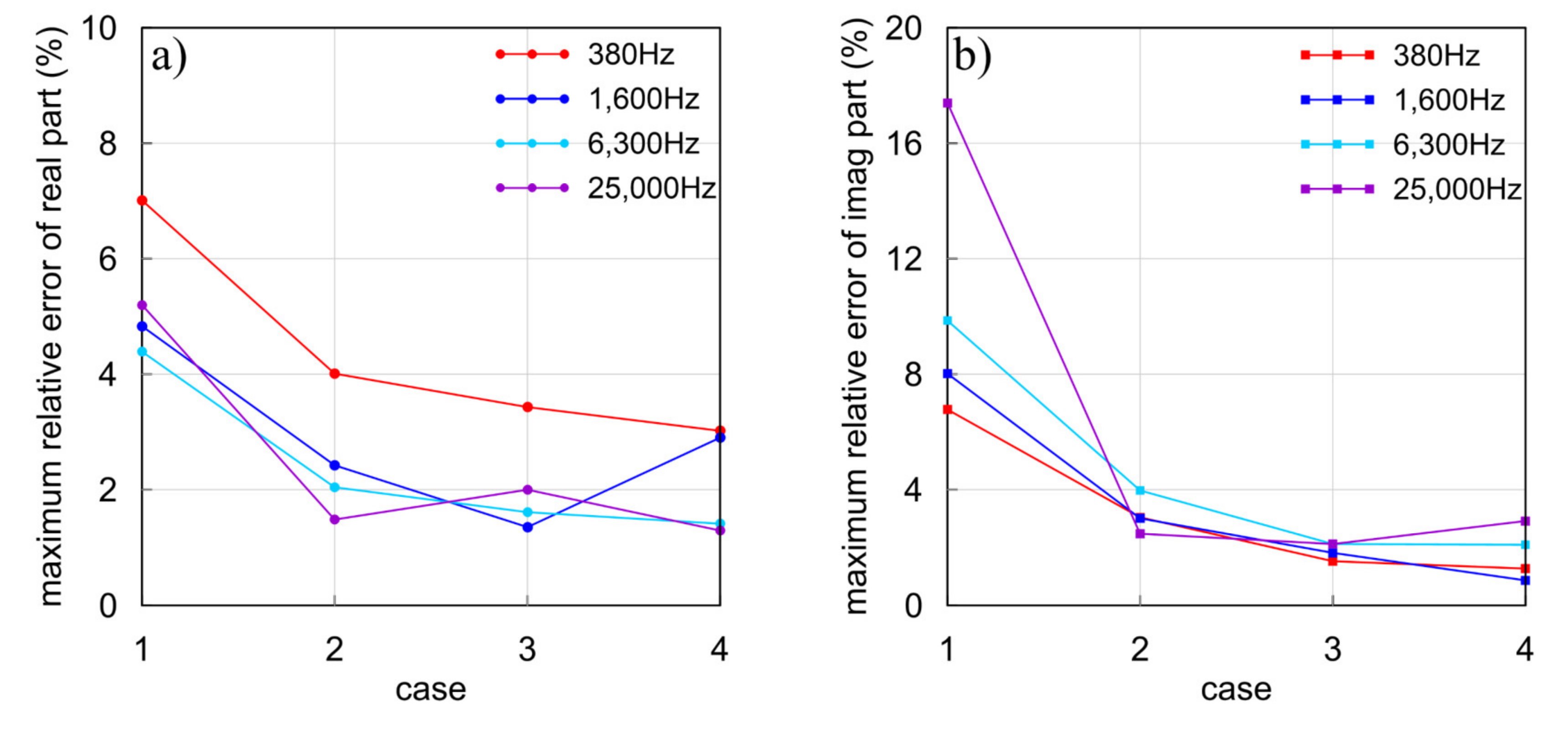
| Method | Case | Type of Elements | DOFs | Maximum Mesh Size | Minimum Mesh Size | Time Consumption (at 380 Hz) | Maximum Relative Error |
|---|---|---|---|---|---|---|---|
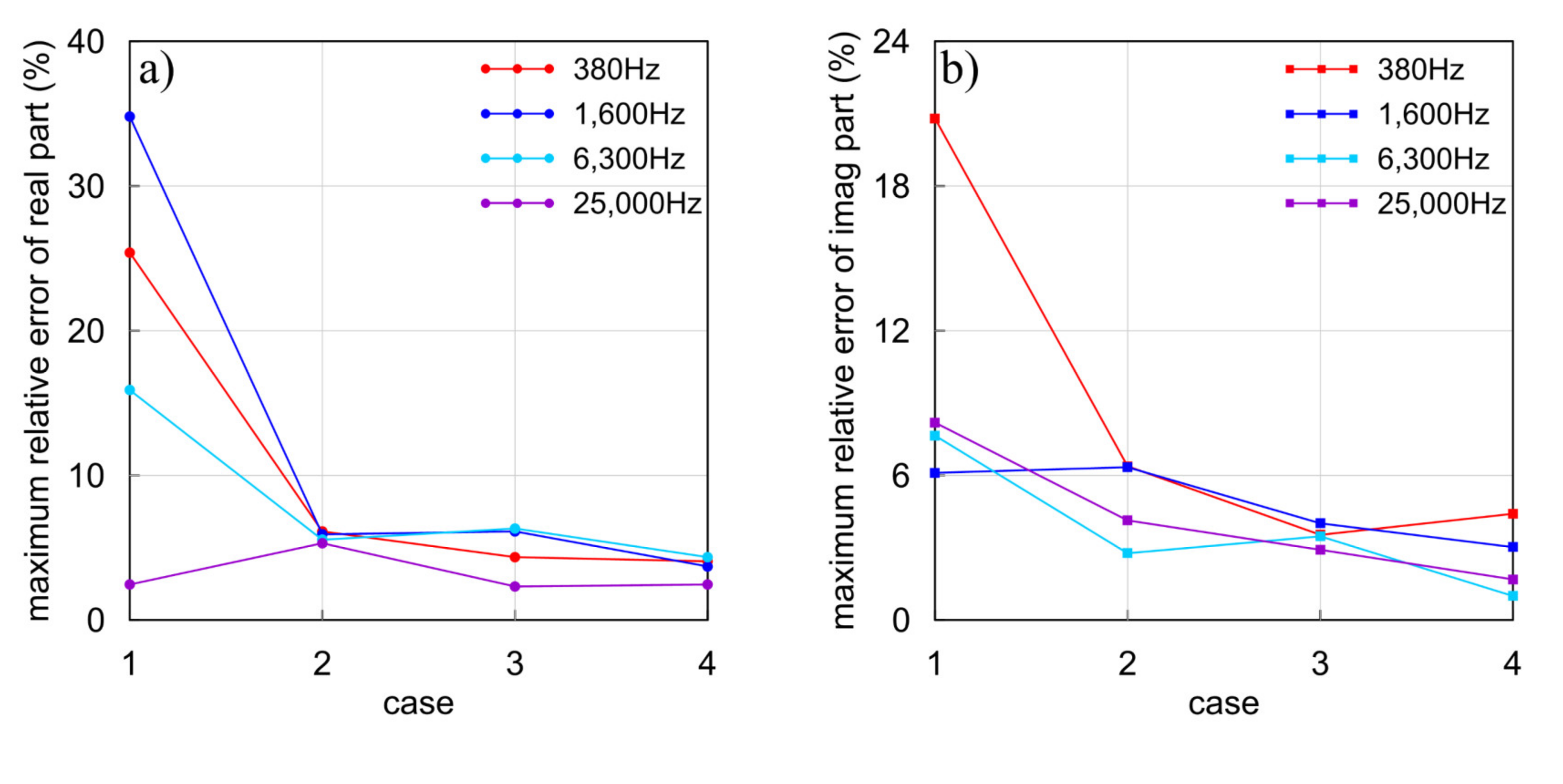
| WFEM | 1 | BSWI21 | 89,900 | 40 m × 40 m × 40 m | 40 m × 40 m × 20 m | 27.618 s | 17.4% |
| - | 2 | BSWI21 | 414,540 | 20 m × 20 m × 20 m | 20 m × 20 m × 20 m | 310.479 s | 4.01% |
| - | 3 | BSWI22 | 792,756 | 40 m × 40 m × 40 m | 40 m × 40 m × 20 m | 811.019 s | 3.43% |
| - | 4 | BSWI22 | 968,436 | 20 m × 40 m × 40 m | 20 m × 20 m × 20 m | 1281.788 s | 3.02% |
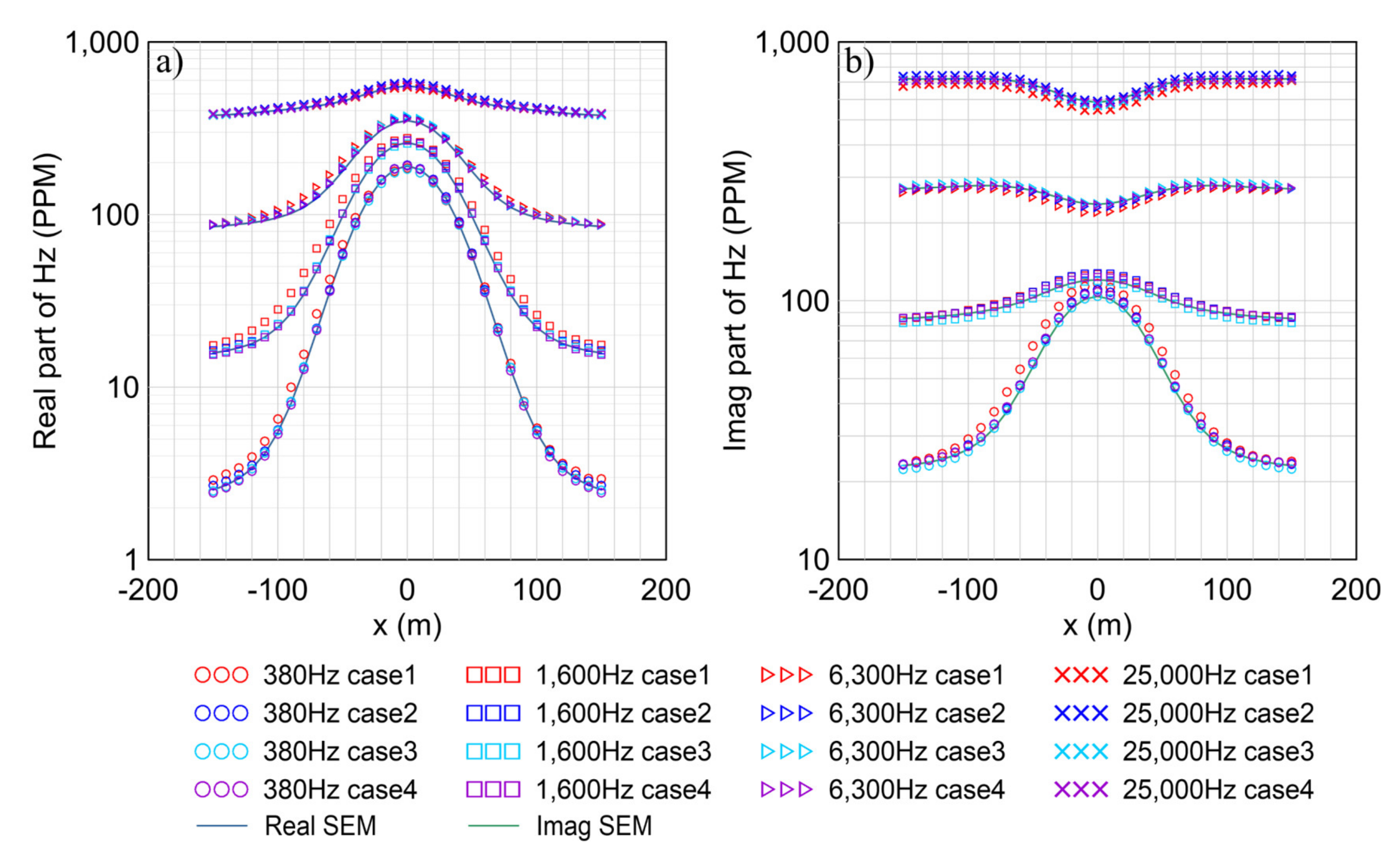
| Method | Case | Type of Elements | DOFs | Maximum Mesh Size | Minimum Mesh Size | Time Consumption (at 380 Hz) | Maximum Relative Error |
|---|---|---|---|---|---|---|---|
| WFEM | 1 | BSWI21 | 89,900 | 40 m × 40 m × 40 m | 40 m × 40 m × 20 m | 25.360 s | 34.8% |
| - | 2 | BSWI21 | 414,540 | 20 m × 20 m × 20 m | 20 m × 20 m × 20 m | 320.319 s | 6.38% |
| - | 3 | BSWI22 | 792,756 | 40 m × 40 m × 40 m | 40 m × 40 m × 20 m | 806.477 s | 6.34% |
| - | 4 | BSWI22 | 968,436 | 20 m × 40 m × 40 m | 20 m × 20 m × 20 m | 1322.695 s | 4.41% |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gao, L.; Yin, C.; Wang, N.; Zhu, J.; Liu, Y.; Ren, X.; Zhang, B.; Xiong, B. 3D Wavelet Finite-Element Modeling of Frequency-Domain Airborne EM Data Based on B-Spline Wavelet on the Interval Using Potentials. Remote Sens. 2021, 13, 3463. https://doi.org/10.3390/rs13173463
Gao L, Yin C, Wang N, Zhu J, Liu Y, Ren X, Zhang B, Xiong B. 3D Wavelet Finite-Element Modeling of Frequency-Domain Airborne EM Data Based on B-Spline Wavelet on the Interval Using Potentials. Remote Sensing. 2021; 13(17):3463. https://doi.org/10.3390/rs13173463
Chicago/Turabian StyleGao, Lingqi, Changchun Yin, Ning Wang, Jiao Zhu, Yunhe Liu, Xiuyan Ren, Bo Zhang, and Bin Xiong. 2021. "3D Wavelet Finite-Element Modeling of Frequency-Domain Airborne EM Data Based on B-Spline Wavelet on the Interval Using Potentials" Remote Sensing 13, no. 17: 3463. https://doi.org/10.3390/rs13173463
APA StyleGao, L., Yin, C., Wang, N., Zhu, J., Liu, Y., Ren, X., Zhang, B., & Xiong, B. (2021). 3D Wavelet Finite-Element Modeling of Frequency-Domain Airborne EM Data Based on B-Spline Wavelet on the Interval Using Potentials. Remote Sensing, 13(17), 3463. https://doi.org/10.3390/rs13173463







