Comparison of the Segmentation Results of Two Carrier Tracking Loop Types and Analysis of Theoretical Influencing Factors
Abstract
1. Introduction
- (1)
- An accurate quantitative loop segmentation method is proposed, which provides a theoretical basis for the realization of robust fusion directly at the signal level in a new-generation tracking loop design. The segmentation results show the performance differences between the FLL and PLL;
- (2)
- The influence of external hardware factors on the loop is removed, and the loop design factors are modeled directly. The analysis results show that the integration time and bandwidth affect the segmentation results of both FLL and PLL, while the gain and filter coefficients result in a difference between the PLL and FLL.
2. Materials and Methods
2.1. Derivation of Segmentation Points
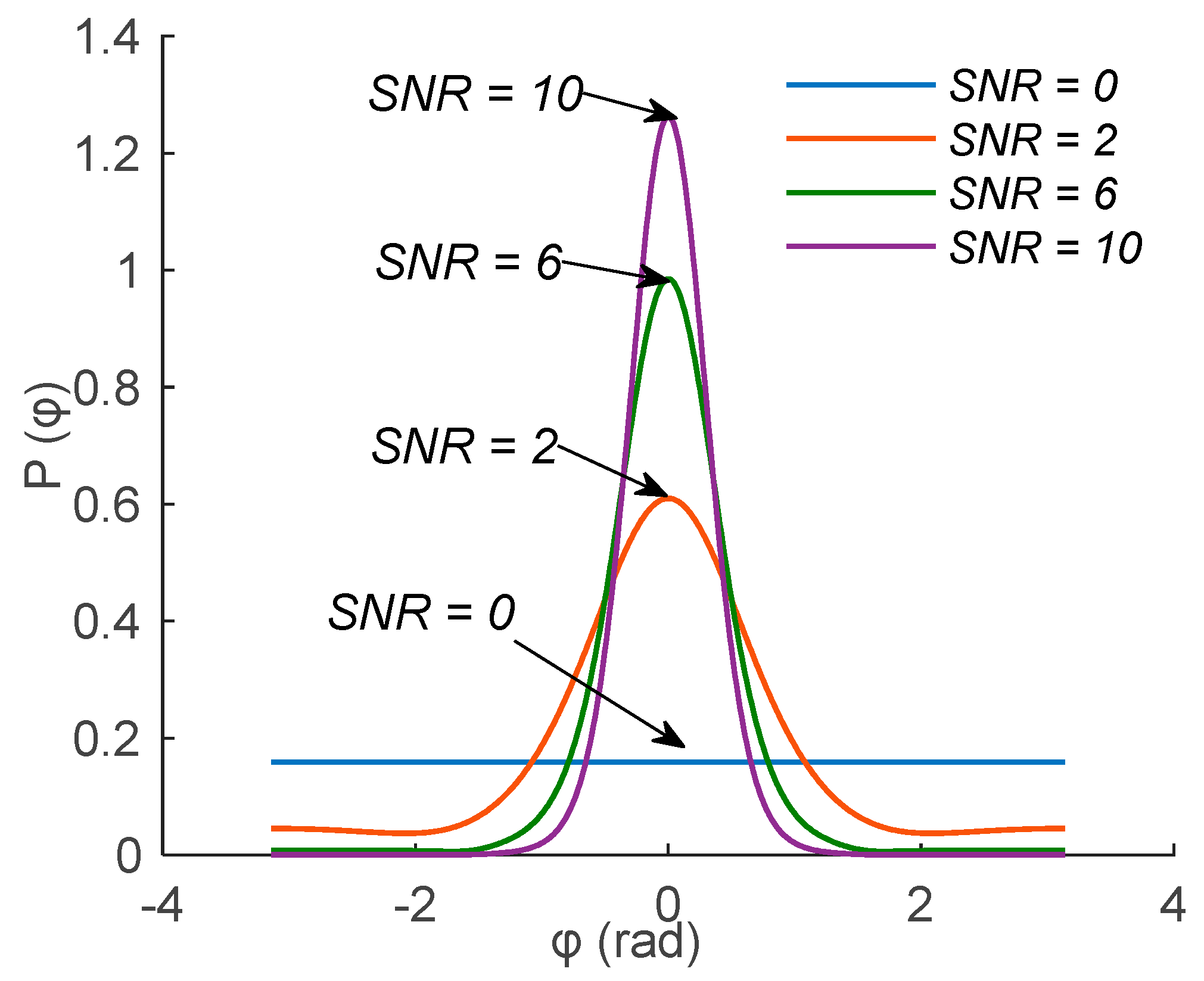
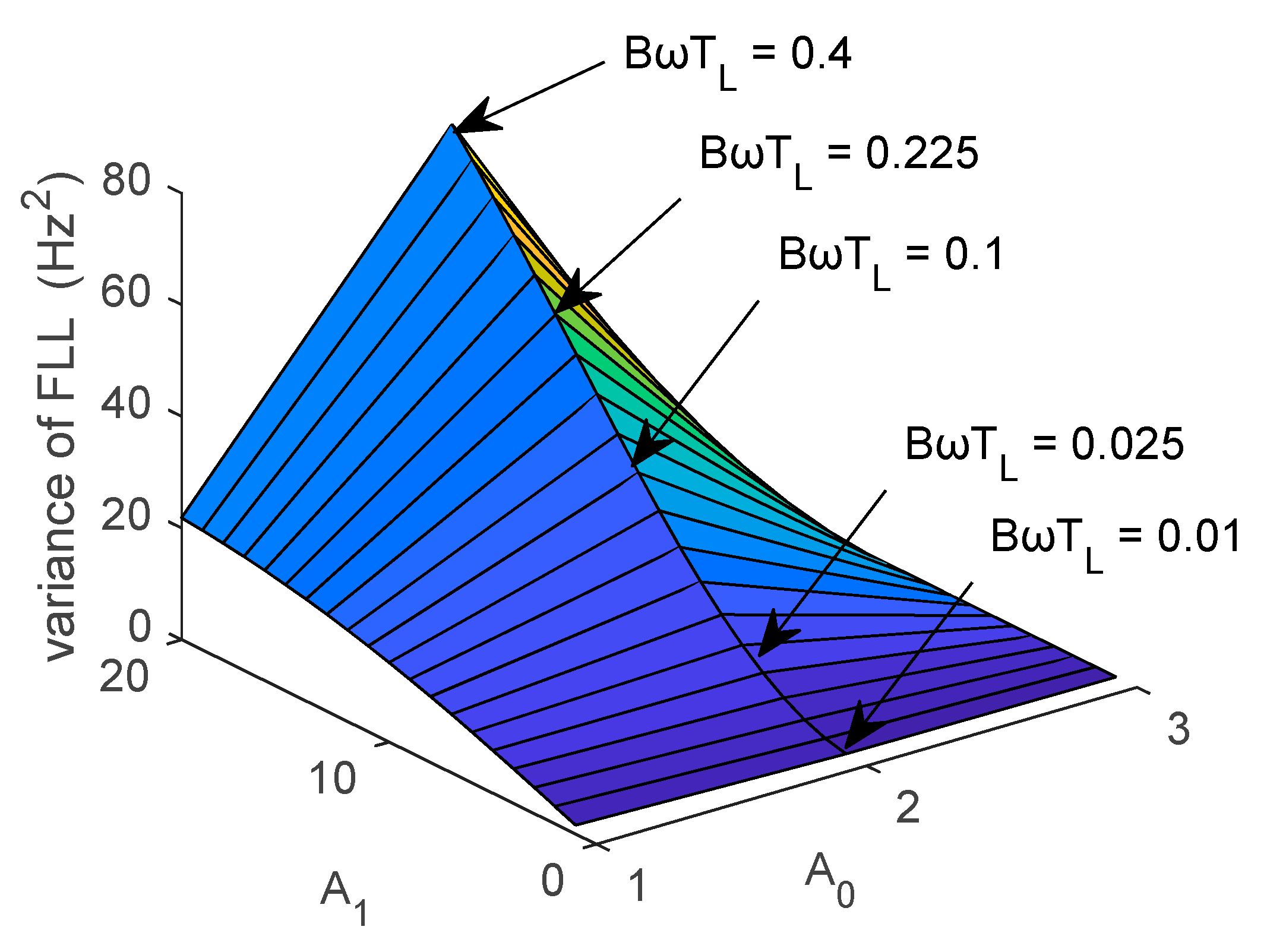
2.2. FLL Analysis Based on Related Work
2.3. PLL Analysis
2.3.1. Phase Discriminator Variance
2.3.2. Filter Coefficient Determination
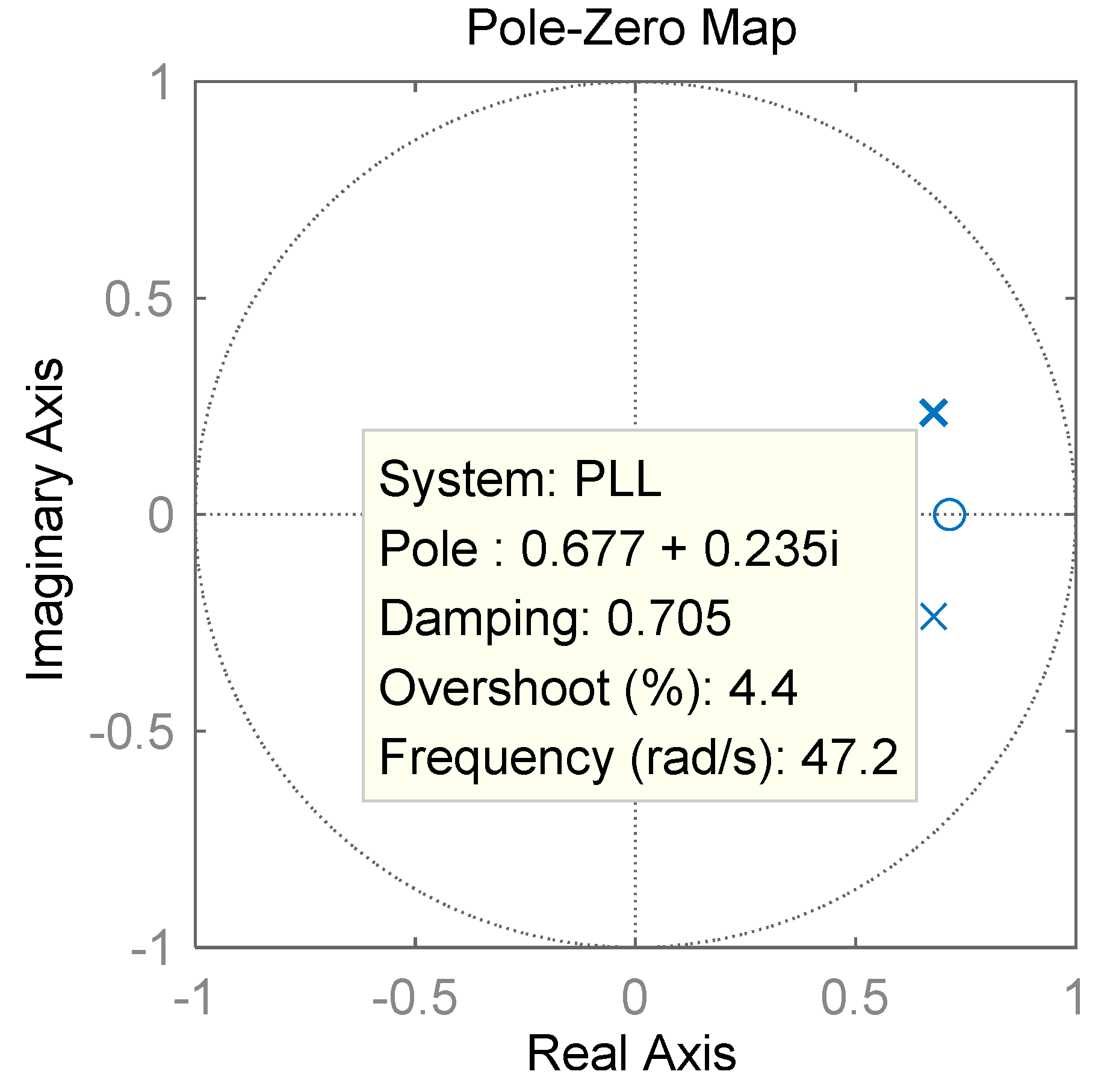
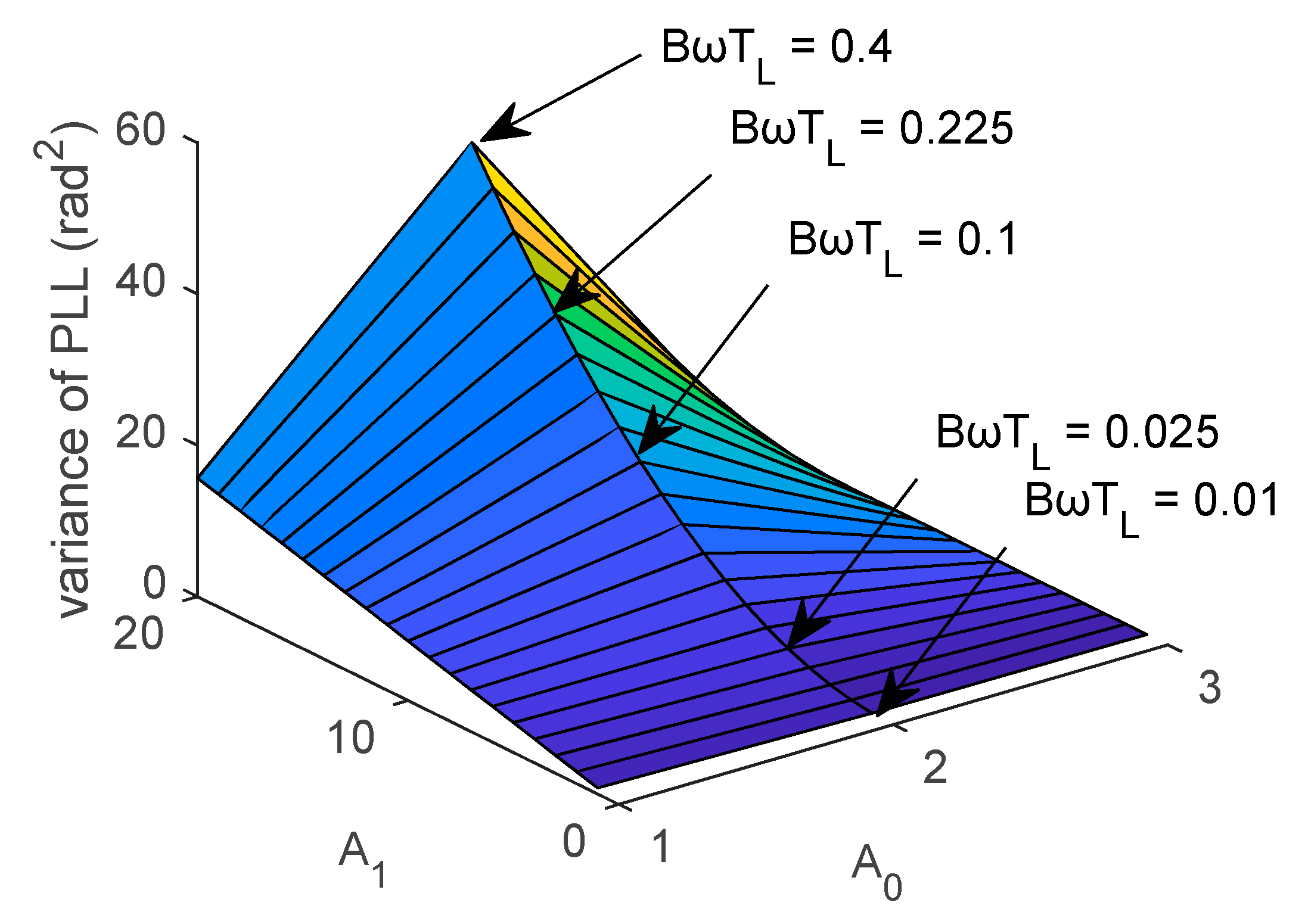
2.3.3. PLL Segmentation Results
3. Segmentation Point-Affecting Factors
3.1. Simulation Verification of Segmentation Points
3.1.1. FLL Simulation Verification
3.1.2. PLL Simulation Verification
3.1.3. Difference between FLL and PLL Segmentation Points
3.2. Common Factors Affecting Segmentation Results
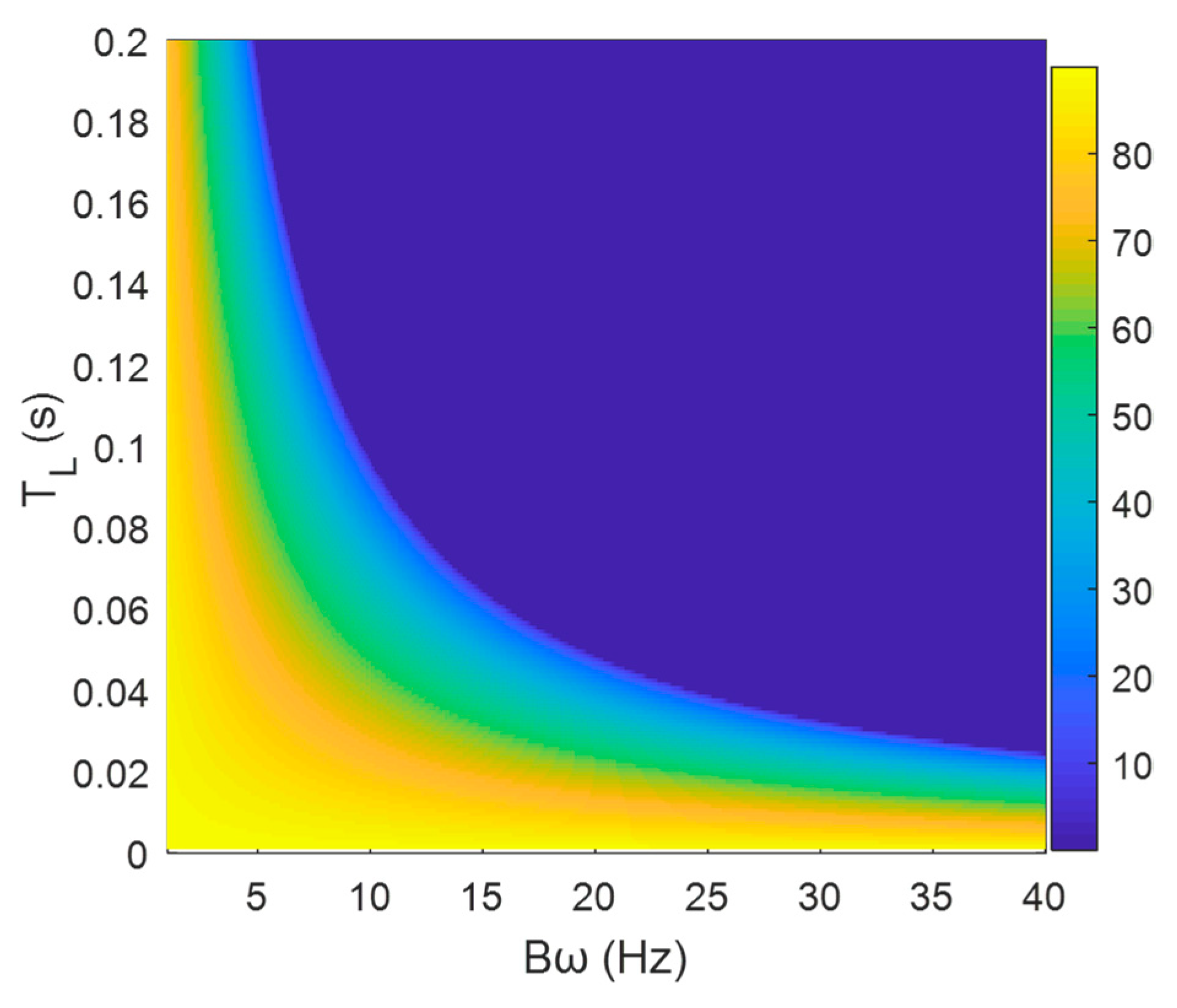
3.2.1. Effect of Integration Time
3.2.2. Effect of Bandwidth
3.3. Factors Causing Differences in Segmentation Results
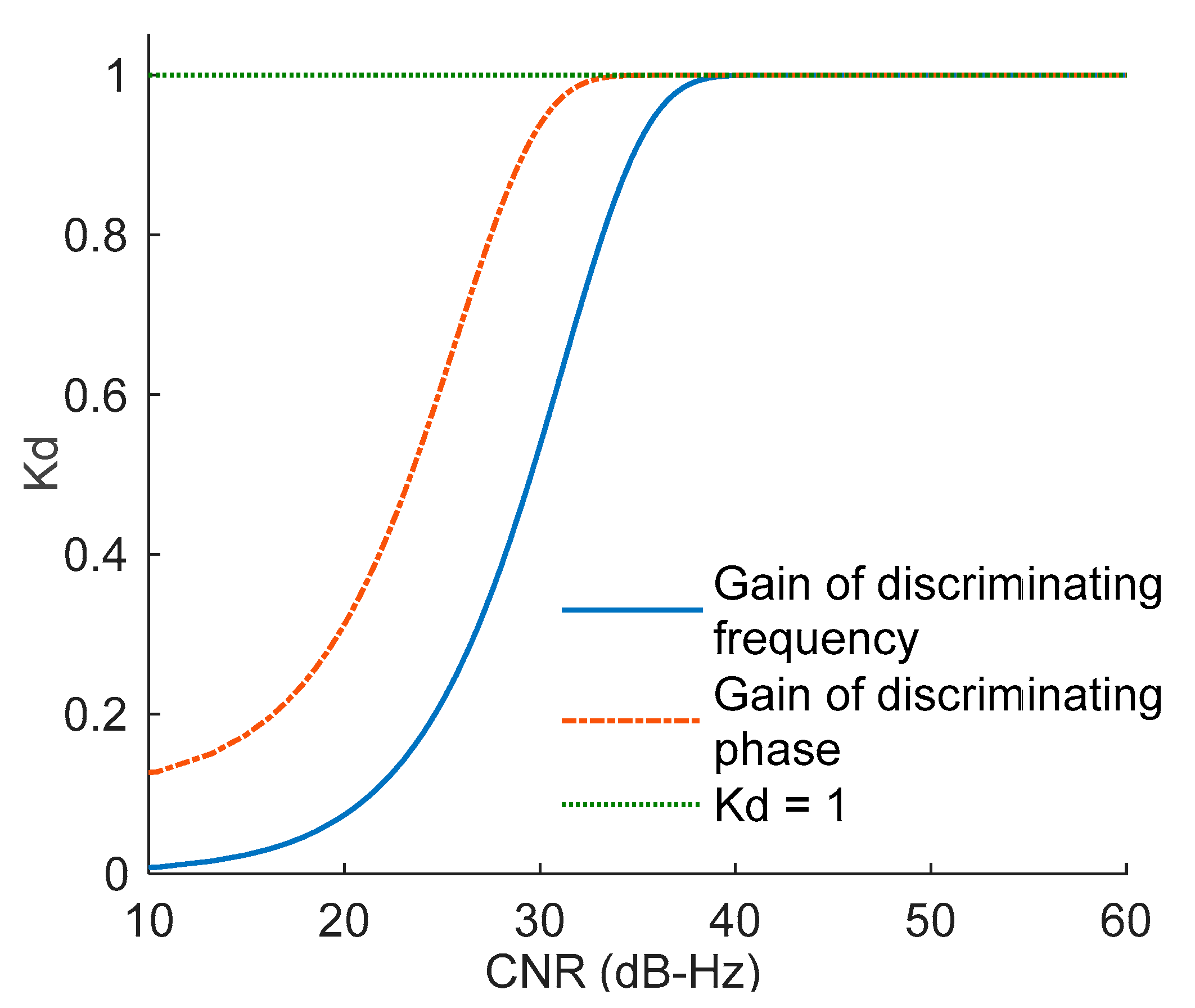
3.3.1. Effect of Discriminator Gain
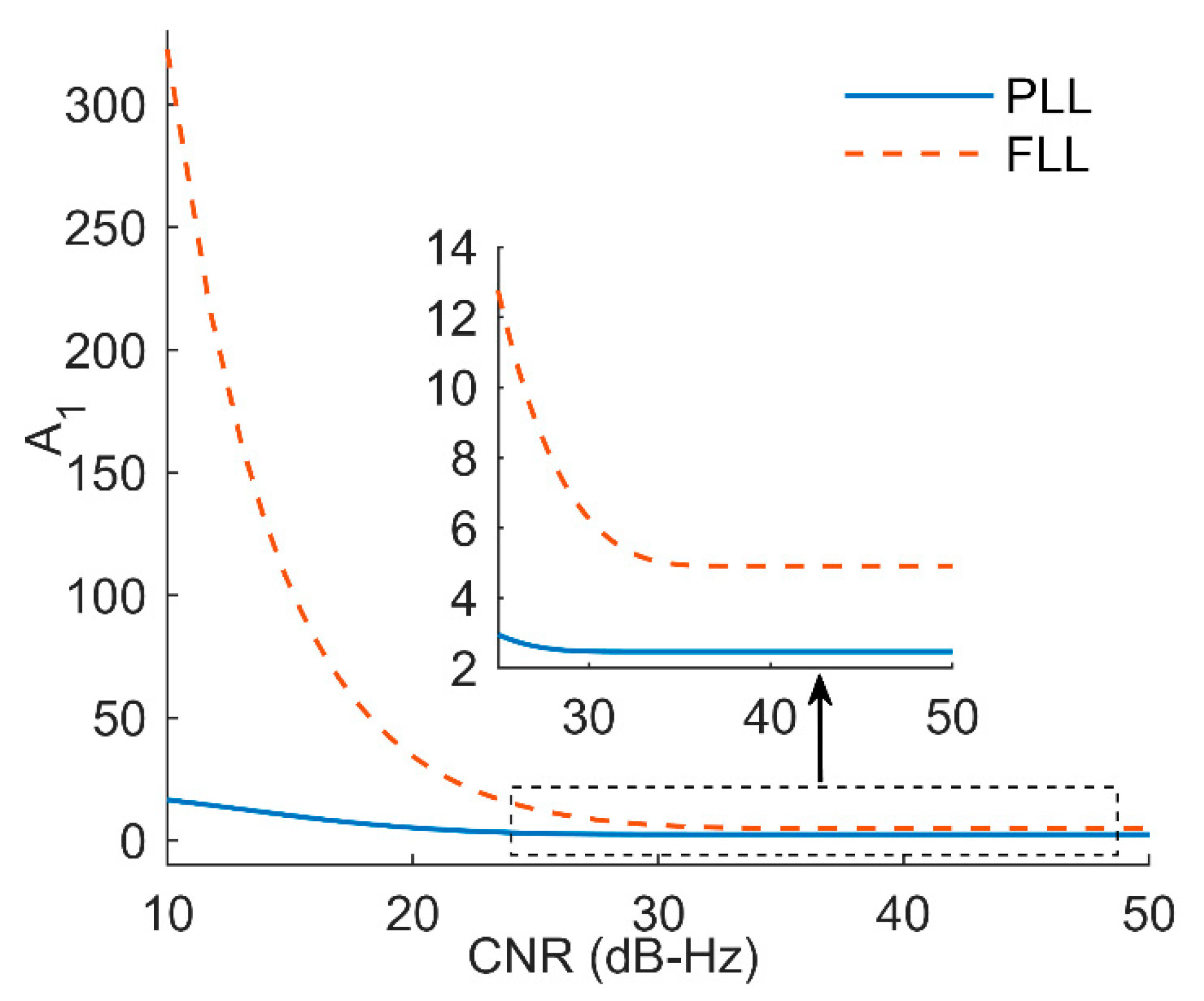
3.3.2. Influence of Filter Coefficients
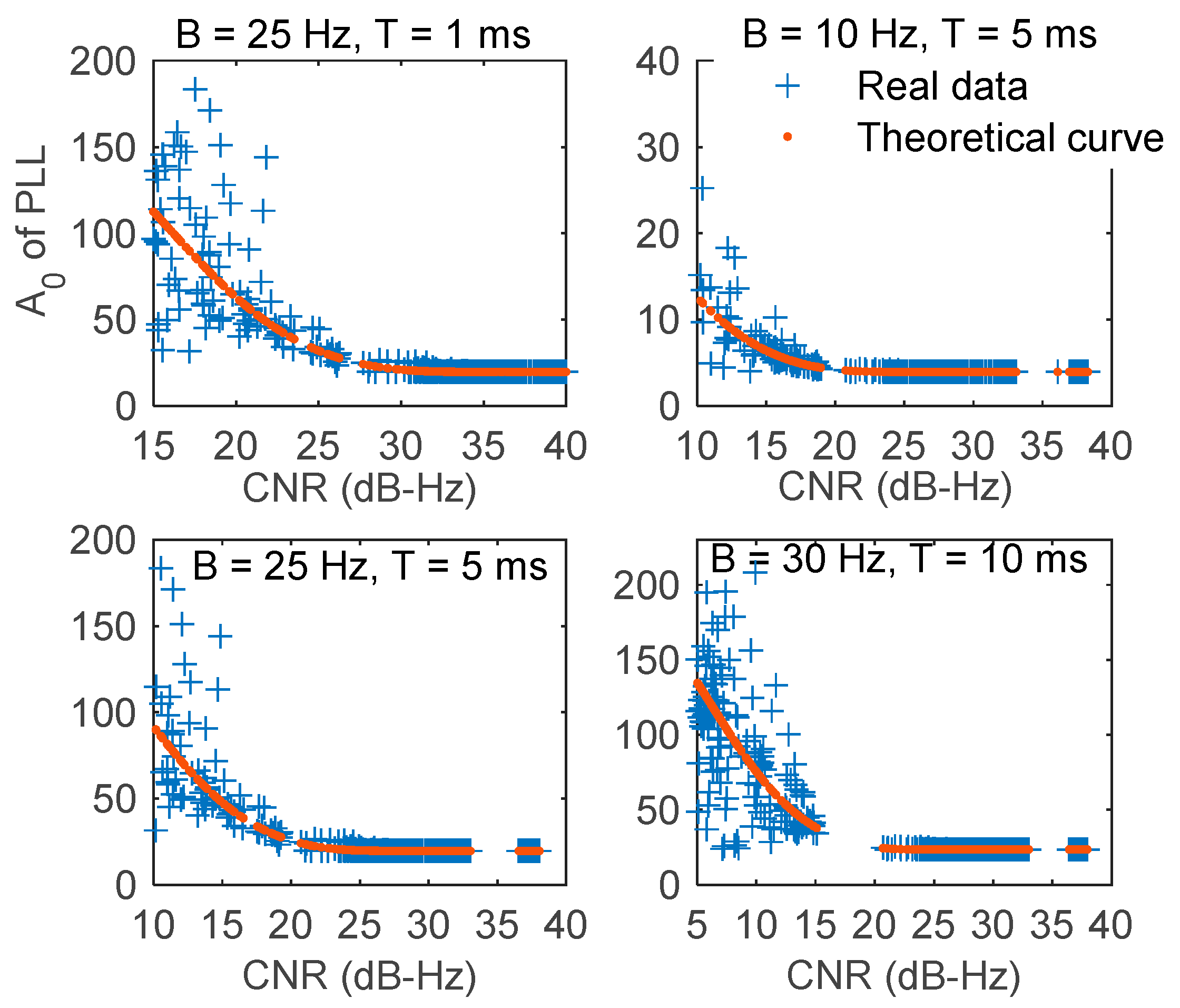
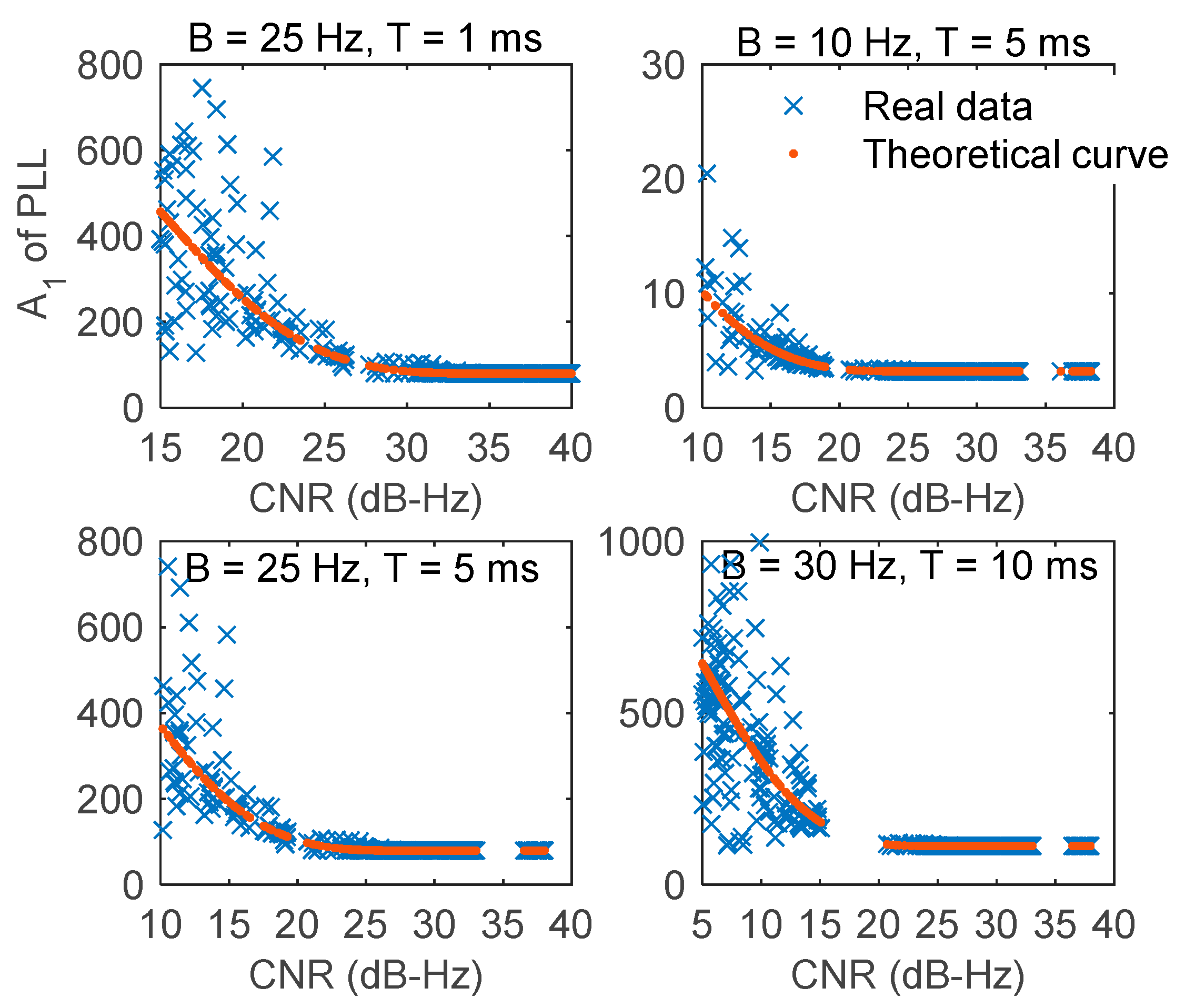
4. Discussion and Validation with Real Data
4.1. Discussion on Filter Coefficients
4.2. Validation with Real Data
5. Conclusions
- (1)
- The concept of the forward loop segmentation is introduced, and the segmentation results of the PLL and FLL are analyzed based on the characteristics of variance under different SNR. The difference in the segmentation results indicates the performance difference between the PLL and FLL, which demonstrates that the FLL can track weaker signals than the PLL. The segmentation results of both the theoretical derivation and simulation show that FLL can track about 2.5 dB-Hz more weaker signals than the PLL under the integration time of 2 ms and the filter bandwidth of 25 Hz;
- (2)
- The main reasons for the performance difference between the two loops are the discriminator gain and filter coefficients. In the discriminator stage, the FLL has only about a 0.2 dB-Hz advantage over the PLL, but this advantage increases to 2.5 dB-Hz when the discrimination gain is combined with the subsequent filtering. Therefore, the difference in performance between the two loops is caused by the combination of discrimination gain and filtering;
- (3)
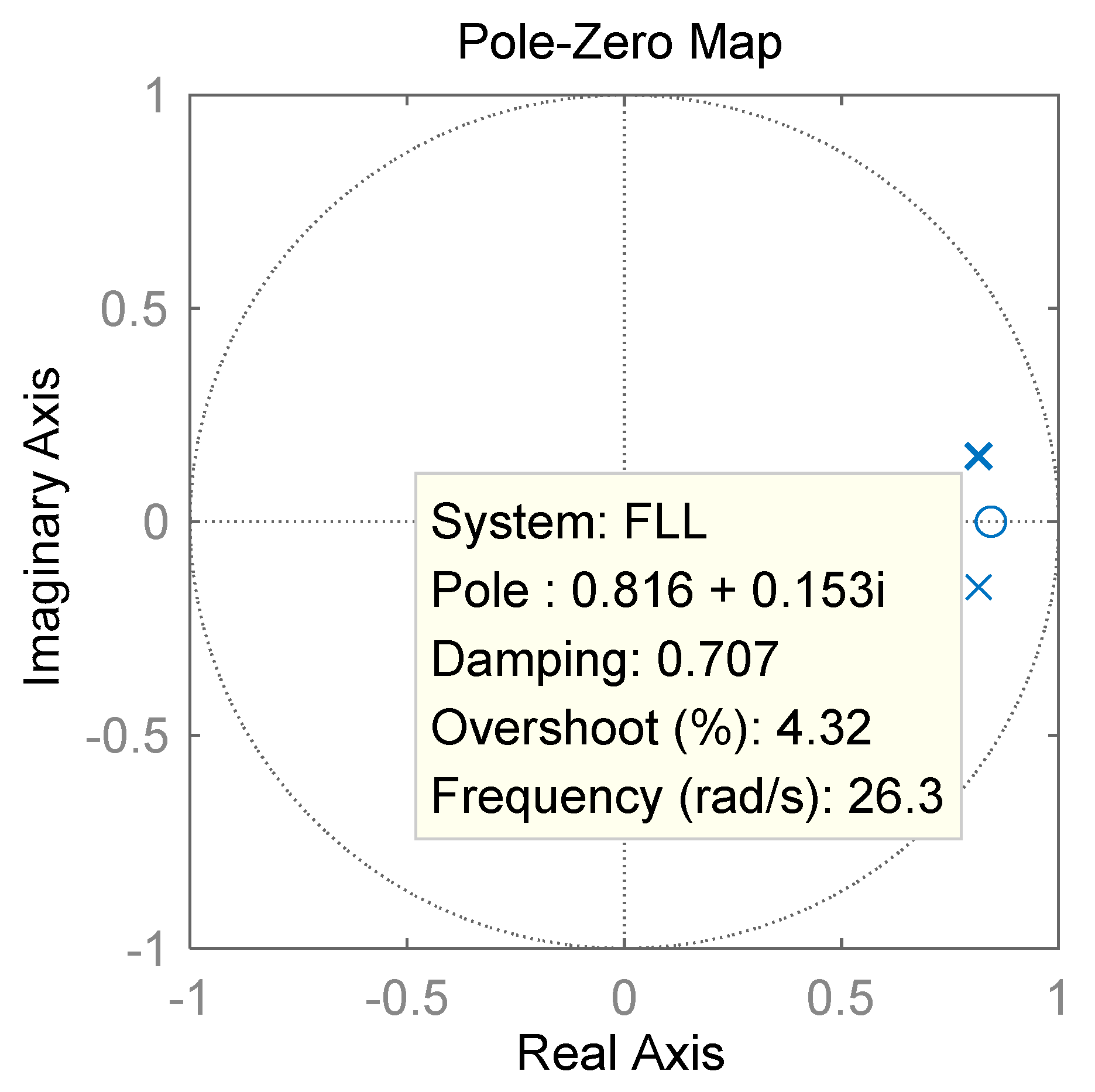
- The proportion coefficient (A0) of the FLL is larger than that of the PLL, so the FLL has better robustness and dynamic performance than the PLL. The integration coefficient (A1) of the FLL is also larger than that of the PLL, so the FLL has a larger tracking error than the PLL. The difference is also reflected in the phase margin of the PLL and FLL. Moreover, reducing the normalized bandwidth can reduce the tracking variance of both the PLL and the FLL, but a dynamic balance between the integration time and bandwidth is necessary to achieve the best possible performance. The proposed method directly evaluates the tracking performance from the design factors of a tracking loop.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Curran, J.T.; Lachapelle, G.; Murphy, C.C. Improving the Design of Frequency Lock Loops for GNSS Receivers. IEEE Trans. Aerosp. Electron. Syst. 2012, 48, 850–868. [Google Scholar] [CrossRef]
- Han, M.F.; Ning, J.Z.; Zhao, D.; Dou, J. A Digital FLL and Its Steady-State Performance Analysis in Z Domain. In Proceedings of the IET International Radar Conference, Institution of Engineering and Technology, Hangzhou, China, 14–16 October 2015. [Google Scholar]
- Mo, J.; Deng, Z.; Jia, B.; Jiang, H.; Bian, X. A Novel FLL-Assisted PLL With Fuzzy Control for TC-OFDM Carrier Signal Tracking. IEEE Access 2018, 6, 52447–52459. [Google Scholar] [CrossRef]
- Chen, S.; Gao, Y. Improvement of Carrier Phase Tracking in High Dynamics Conditions Using an Adaptive Joint Vector Tracking Architecture. GPS Solut. 2018, 23, 15. [Google Scholar] [CrossRef]
- Guo, K.; Aquino, M.; Veettil, S.V. Effects of GNSS Receiver Tuning on the PLL Tracking Jitter Estimation in the Presence of Ionospheric Scintillation. Space Weather 2020, 18. [Google Scholar] [CrossRef]
- Jiang, R.; Wang, K.; Liu, S.; Li, Y. Performance Analysis of a Kalman Filter Carrier Phase Tracking Loop. GPS Solut. 2016, 21, 551–559. [Google Scholar] [CrossRef]
- Yang, R.; Xu, D.; Morton, Y.T. Generalized Multifrequency GPS Carrier Tracking Architecture: Design and Performance Analysis. IEEE Trans. Aerosp. Electron. Syst. 2019, 56, 2548–2563. [Google Scholar] [CrossRef]
- Dou, J.; Xu, B.; Dou, L. Performance Assessment of GNSS Scalar and Vector Frequency Tracking Loops. Optik 2020, 202, 163552. [Google Scholar] [CrossRef]
- Cheng, Y.; Chang, Q. A Carrier Tracking Loop Using Adaptive Strong Tracking Kalman Filter in GNSS Receivers. IEEE Commun. Lett. 2020, 24, 2903–2907. [Google Scholar] [CrossRef]
- Zhang, Z.; Li, B.; Shen, Y.; Gao, Y.; Wang, M. Site-Specific Unmodeled Error Mitigation for GNSS Positioning in Urban Environments Using a Real-Time Adaptive Weighting Model. Remote Sens. 2018, 10, 1157. [Google Scholar] [CrossRef]
- Fu, W.; Huang, G.; Zhang, Y.; Zhang, Q.; Cui, B.; Ge, M.; Schuh, H. Multi-GNSS Combined Precise Point Positioning Using Additional Observations with Opposite Weight for Real-Time Quality Control. Remote Sens. 2019, 11, 311. [Google Scholar] [CrossRef]
- Lyu, Z.; Gao, Y. An SVM Based Weight Scheme for Improving Kinematic GNSS Positioning Accuracy with Low-Cost GNSS Receiver in Urban Environments. Sensors 2020, 20, 7265. [Google Scholar] [CrossRef] [PubMed]
- Cortés, I.; Van Der Merwe, J.R.; Nurmi, J.; Rügamer, A.; Felber, W. Evaluation of Adaptive Loop-Bandwidth Tracking Techniques in GNSS Receivers. Sensors 2021, 21, 502. [Google Scholar] [CrossRef] [PubMed]
- Li, Y.; Wang, M.; Shivaramaiah, N.C. Design and Analysis of a Generalized DLL/FLL Discriminator for GPS Receivers. GPS Solut. 2018, 22, 64. [Google Scholar] [CrossRef]
- Ma, L.; Shi, L.; Wang, Z. Performance Analysis of a Second Order FLL Assisted Third Order PLL for Tracking Doppler Rates. WSEAS IEEE Trans. Commun. 2014, 13, 26–43. [Google Scholar]
- Hagmann, W.; Habermann, J. On the Phase Error Distribution of an Open Loop Phase Estimator. In Proceedings of the IEEE International Conference on Communications (ICC’88), Philadelphia, PA, USA, 12–15 June 1988. [Google Scholar] [CrossRef]
- Xie, G. Principle of GPS and Receiver Design; Publishing House of Electronics Industry: Beijing, China, 2009; p. 300. [Google Scholar]
- Alam, N.; Jin, T.; Khan, F. Theoretical Performance Analysis and Comparison of VDFLL and Traditional FLL Tracking Loops. In Proceedings of the IEEE International Conference on Communications (ICC’88), Gothenburg, Sweden, 14–17 May 2018; pp. 46–53. [Google Scholar] [CrossRef]
- Han, M.; Wang, Q.; Wen, Y.; He, M.; He, X. The Application of Robust Least Squares Method in Frequency Lock Loop Fusion for Global Navigation Satellite System Receivers. Sensors 2020, 20, 1224. [Google Scholar] [CrossRef] [PubMed]
- Shen, N.; Zhang, X. The Design for High Dynamic GPS Receiver in a Combinated Method of FLL and PLL. Emerging Technologies for Information Systems, Computing, and Management; Springer: New York, NY, USA, 2013; Volume 236, pp. 3–11. [Google Scholar]
- He, W.D. Research on Key Technology of Tracking Loop Design for GNSS Receiver; National Time Service Center, Chinese Academy of Science: Xi’an, China, 2014. [Google Scholar]
- Tang, X.M.; Huang, Y.B.; Wang, F.X. Performance and Design of Carrier Tracking Loop Based on Atan Detector in GNSS Receiver. J. Electron. 2010, 32, 1747–1751. [Google Scholar]
- Jiang, Y. High Performance Tracking and Acquisition Loop Algorithms for a GNSS Receiver; Dalian Maritime University: Dalian, China, 2010. [Google Scholar]
- Niu, X.; Li, B.; Ziedan, N.I.; Guo, W.; Liu, J. Analytical and Simulation-Based Comparison between Traditional and Kalman Filter-Based Phase-Locked Loops. GPS Solut. 2017, 21, 123–135. [Google Scholar] [CrossRef]
- Jin, T.; Ren, J. Stability Analysis of GPS Carrier Tracking Loops by Phase Margin Approach. GPS Solut. 2013, 17, 423–431. [Google Scholar] [CrossRef]
- Wang, Y.; Chen, X.; Zhang, Y.; Chen, J.; Gong, C. Frequency Characteristics Analysis and Stability Research of Phase Locked Loop for Three-phase Grid-connected Inverters. Proc. CSEE 2017, 37, 3843–3853. [Google Scholar]
- Tang, X.; Falco, G.; Falletti, E.; Presti, L.L. Theoretical analysis and tuning criteria of the Kalman filter-based tracking loop. GPS Solut. 2014, 19, 489–503. [Google Scholar] [CrossRef]
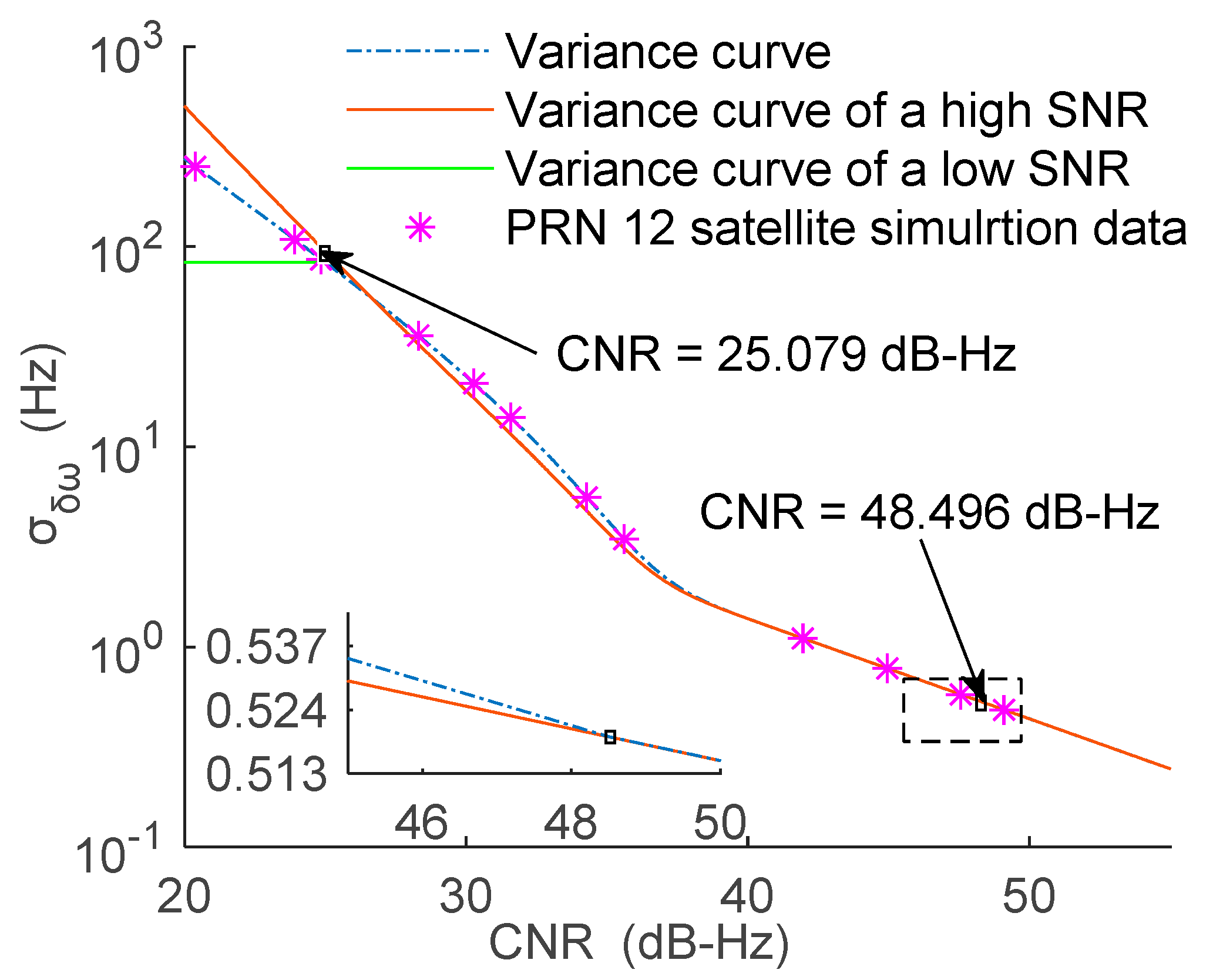
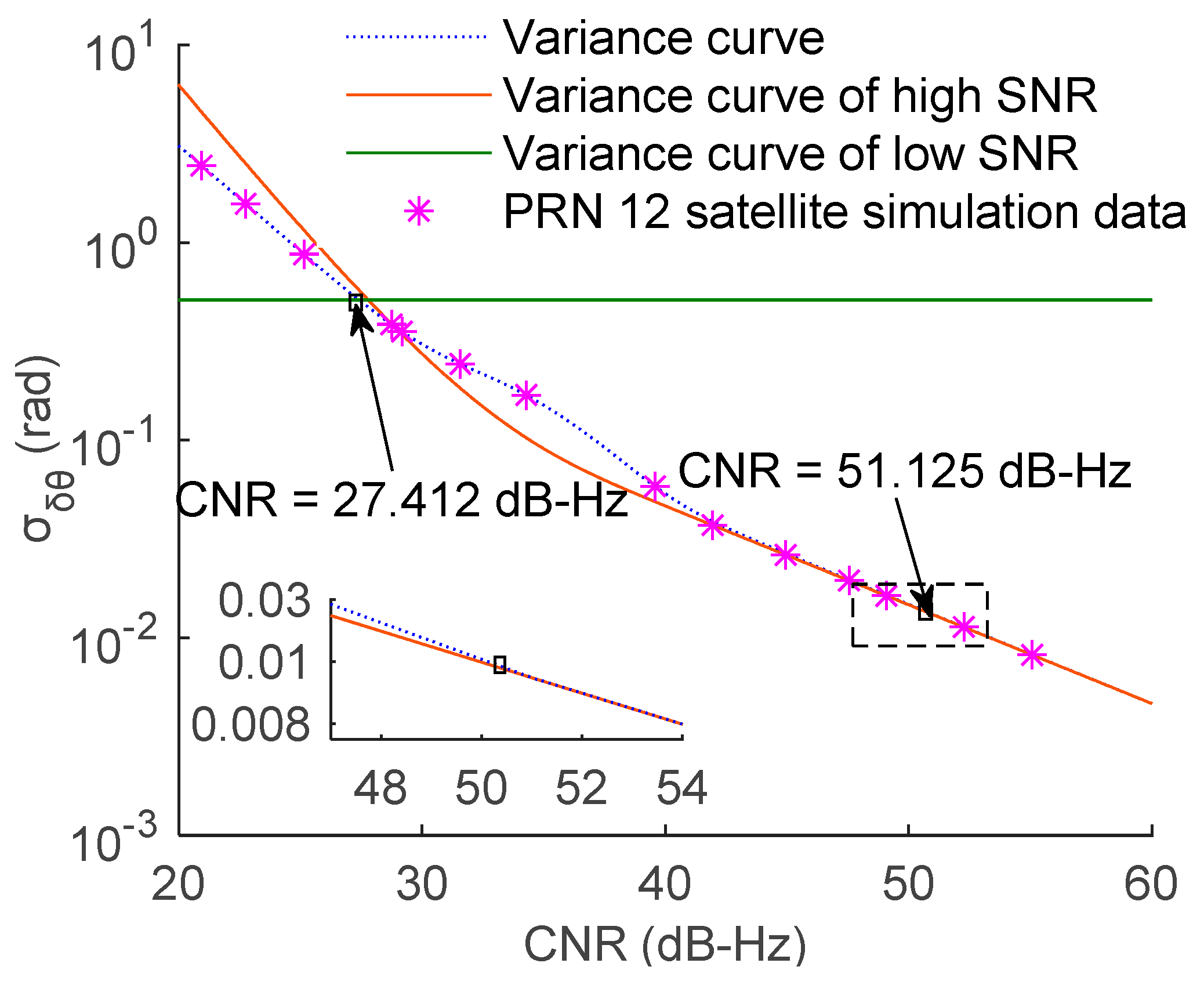
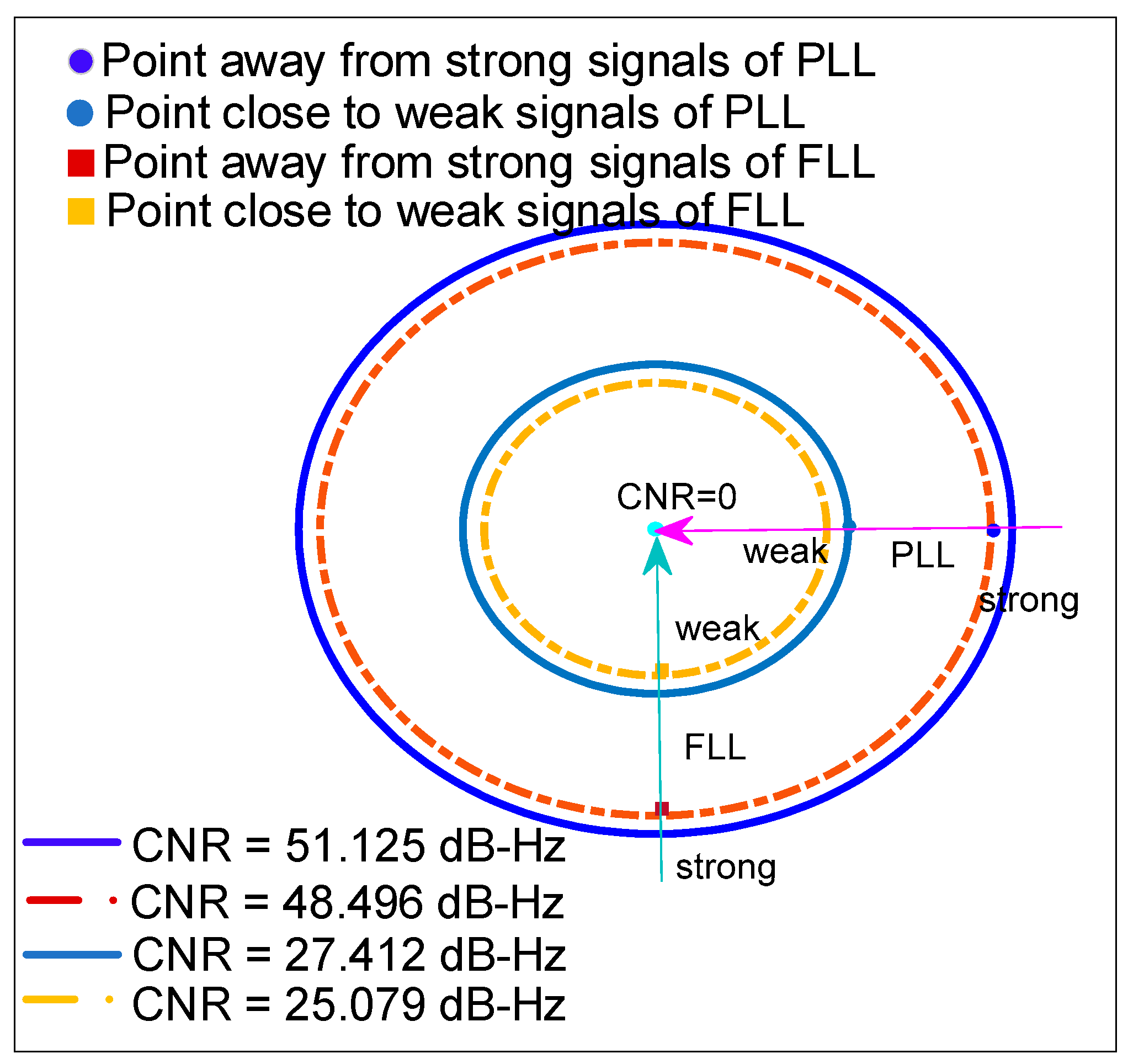







| Strong Signal (dB-Hz) | Weak Signal (dB-Hz) | |
|---|---|---|
| FLL | CNR ≥ 48.496 | CNR ≤ 25.079 |
| PLL | CNR ≥ 51.125 | CNR ≤ 27.412 |
| FLL | PLL | |||
|---|---|---|---|---|
| Weak Signal (dB-Hz) | Strong Signal (dB-Hz) | Weak Signal (dB-Hz) | Strong Signal (dB-Hz) | |
| T = 2 ms | CNR ≤ 25.079 | CNR ≥ 48.496 | CNR ≤ 27.412 | CNR ≥ 51.125 |
| T = 4 ms | CNR ≤ 23.429 | CNR ≥ 43.022 | CNR ≤ 26.026 | CNR ≥ 46.887 |
| T = 10 ms | CNR ≤ 21.358 | CNR ≥ 39.024 | CNR ≤ 24.716 | CNR ≥ 42.853 |
| FLL | PLL | |||
|---|---|---|---|---|
| Weak Signal (dB-Hz) | Strong Signal (dB-Hz) | Weak Signal (dB-Hz) | Strong Signal (dB-Hz) | |
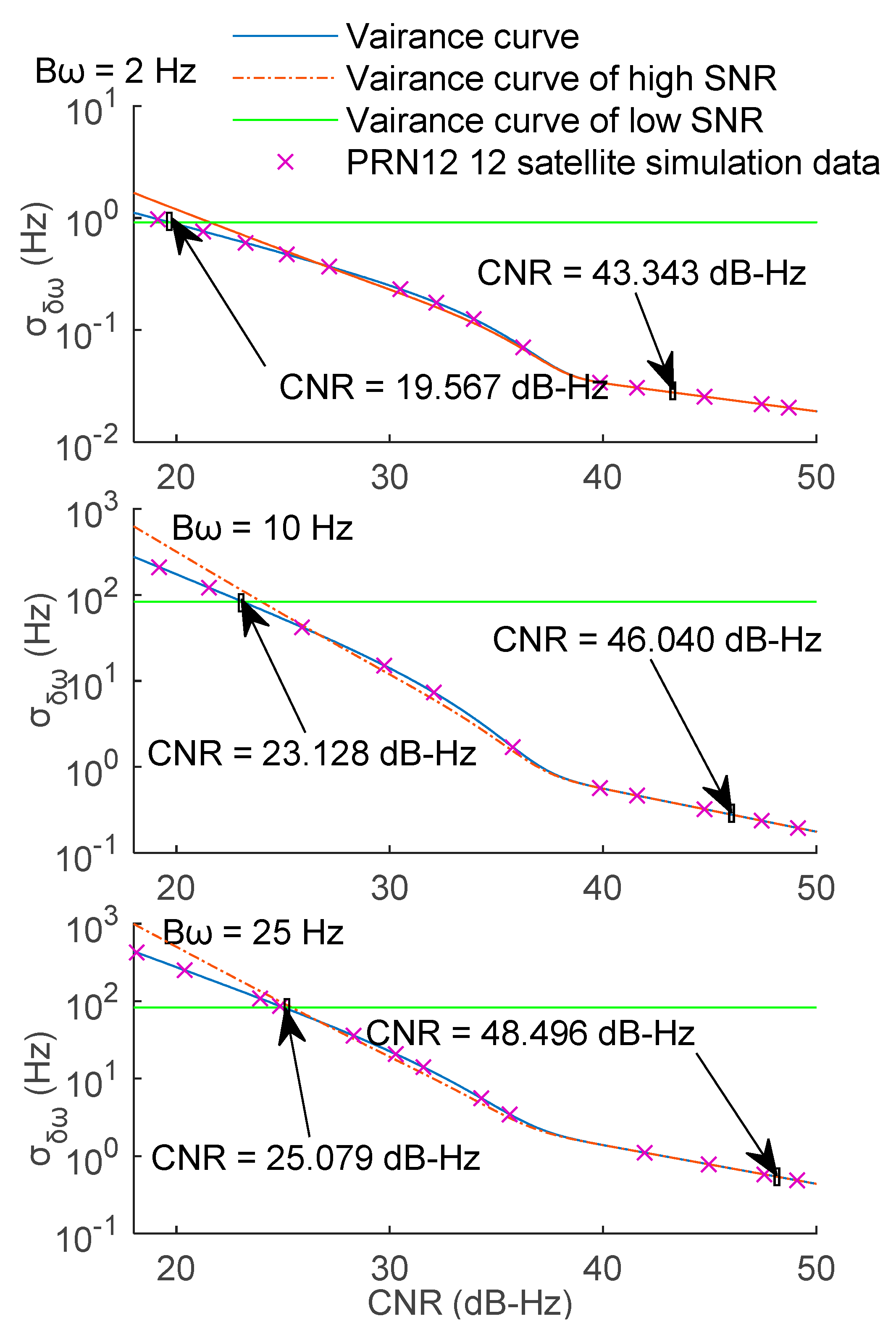
| Bω = 2 Hz | CNR ≤ 19.567 | CNR ≥ 43.343 | CNR ≤ 22.330 | CNR ≥ 48.722 |
| Bω = 10 Hz | CNR ≤ 23.128 | CNR ≥ 46.040 | CNR ≤ 25.453 | CNR ≥ 49.027 |
| Bω = 25 Hz | CNR ≤ 25.079 | CNR ≥ 48.496 | CNR ≤ 27.412 | CNR ≥ 51.125 |
| Bω = 25 Hz, T = 1 ms | Bω = 10 Hz, T = 5 ms | Bω = 25 Hz, T = 5 ms | Bω = 30 Hz, T = 10 ms | Bω = 50 Hz, T = 15 ms | |
|---|---|---|---|---|---|
| 4.24 | 1.78 | 4.25 | 4.48 | 6.23 | |
| 8.56 | 1.60 | 8.54 | 10.00 | 17.17 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, Q.; Han, M.; Wen, Y.; He, M.; He, X. Comparison of the Segmentation Results of Two Carrier Tracking Loop Types and Analysis of Theoretical Influencing Factors. Remote Sens. 2021, 13, 2035. https://doi.org/10.3390/rs13112035
Wang Q, Han M, Wen Y, He M, He X. Comparison of the Segmentation Results of Two Carrier Tracking Loop Types and Analysis of Theoretical Influencing Factors. Remote Sensing. 2021; 13(11):2035. https://doi.org/10.3390/rs13112035
Chicago/Turabian StyleWang, Qian, Mengyue Han, Yuanlan Wen, Min He, and Xiufeng He. 2021. "Comparison of the Segmentation Results of Two Carrier Tracking Loop Types and Analysis of Theoretical Influencing Factors" Remote Sensing 13, no. 11: 2035. https://doi.org/10.3390/rs13112035
APA StyleWang, Q., Han, M., Wen, Y., He, M., & He, X. (2021). Comparison of the Segmentation Results of Two Carrier Tracking Loop Types and Analysis of Theoretical Influencing Factors. Remote Sensing, 13(11), 2035. https://doi.org/10.3390/rs13112035






